Extending the A Priori Procedure (APP) to Analysis of Variance Models under Normality
Abstract
:1. Extending the A Priori Procedure to One-Way Analysis of Variance under Normality
2. Previous APP Advances
3. Expansion to Multiple Contrasts
3.1. Estimation of Parameters in the One-Way ANOVA Model
3.2. A Priori Procedures Applied for One-Way ANOVA Models
- (i)
- Since F test is used in two-way or multi-way ANOVA models for testing the main effects and their interactions, we can use the similar method as in Equations (10) and (11) by adjusting degrees of freedoms k and . Therefore, the required sample size n can be obtained from our online calculator given in next section.
- (ii)
- For our ANOVA model in Equation (8), we focus only on the estimation of parameters with the minimum required sample size. To study the robustness of estimators or the shrinkage estimation of in high-dimensional generalized linear models, we suggest Roozbeh et al. (2023) [9], Yüzbaşı et al. (2020) [10], and Yüzbaşı et al. (2021) [11] for interested readers.
- (iii)
4. Implications
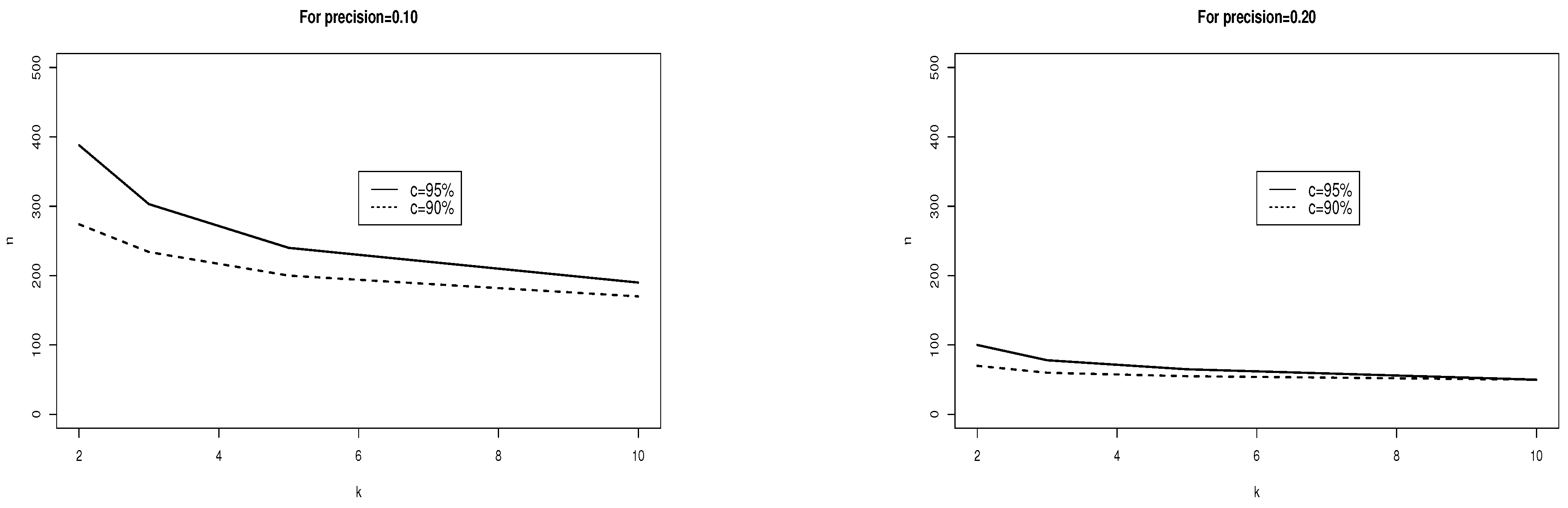
4.1. Having Means for All Groups Fall within Specifications
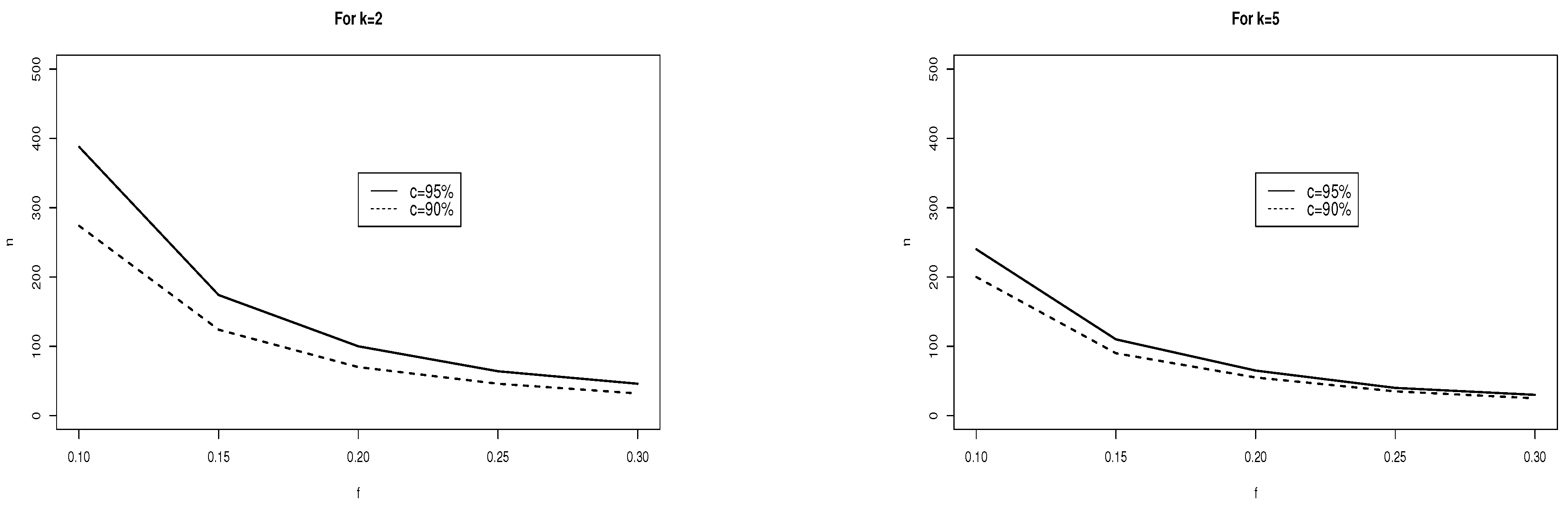
4.2. Contrasts between Means
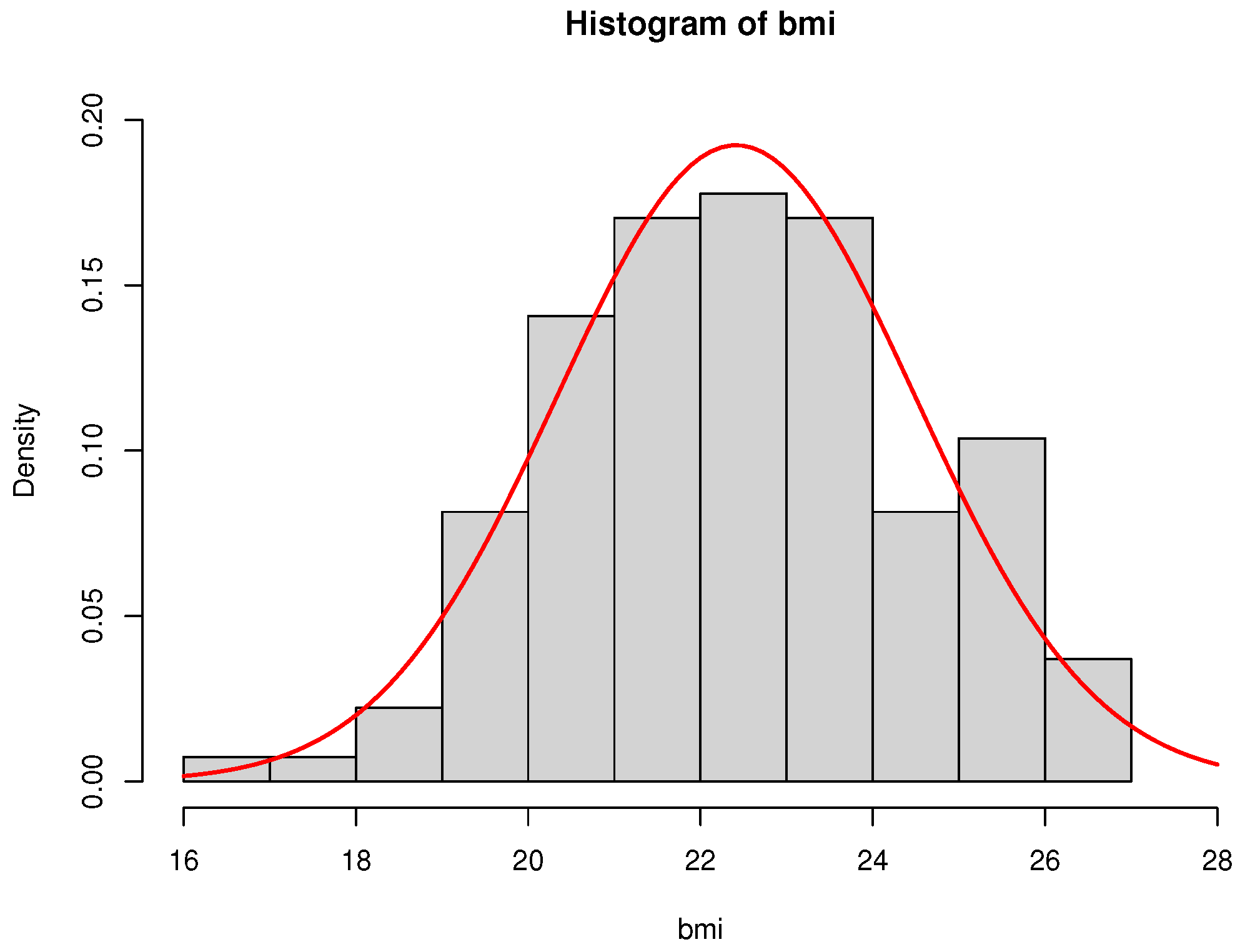
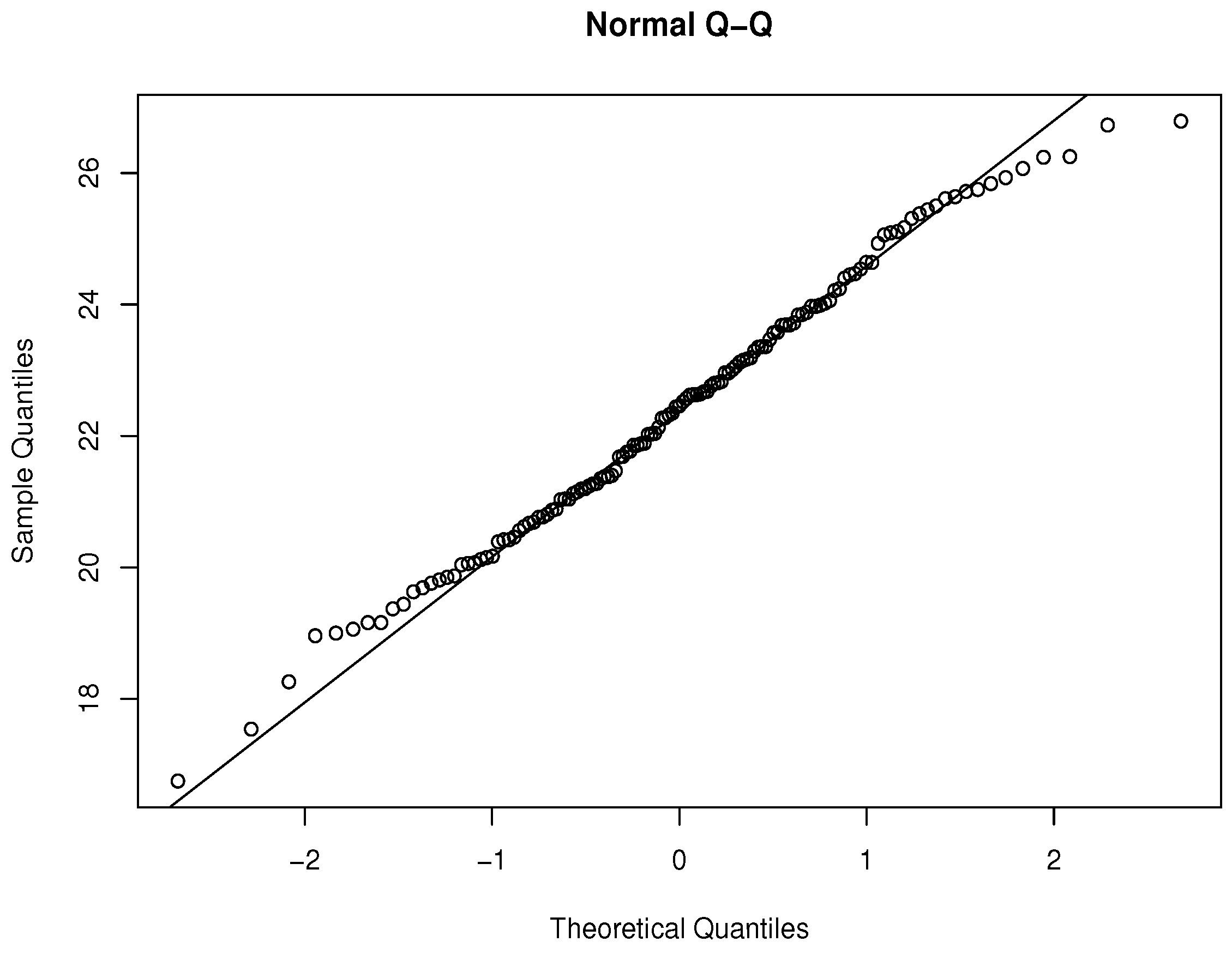
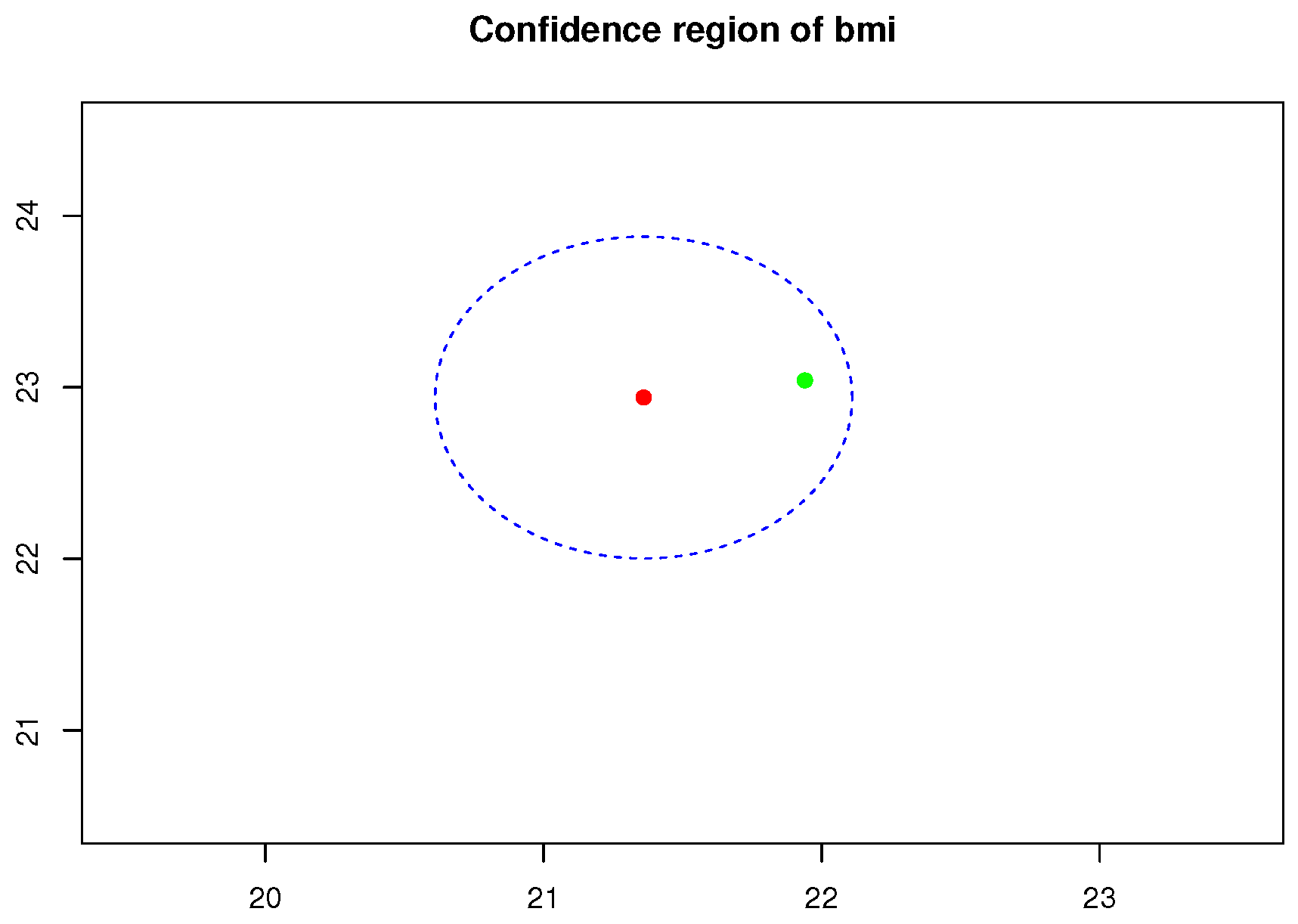
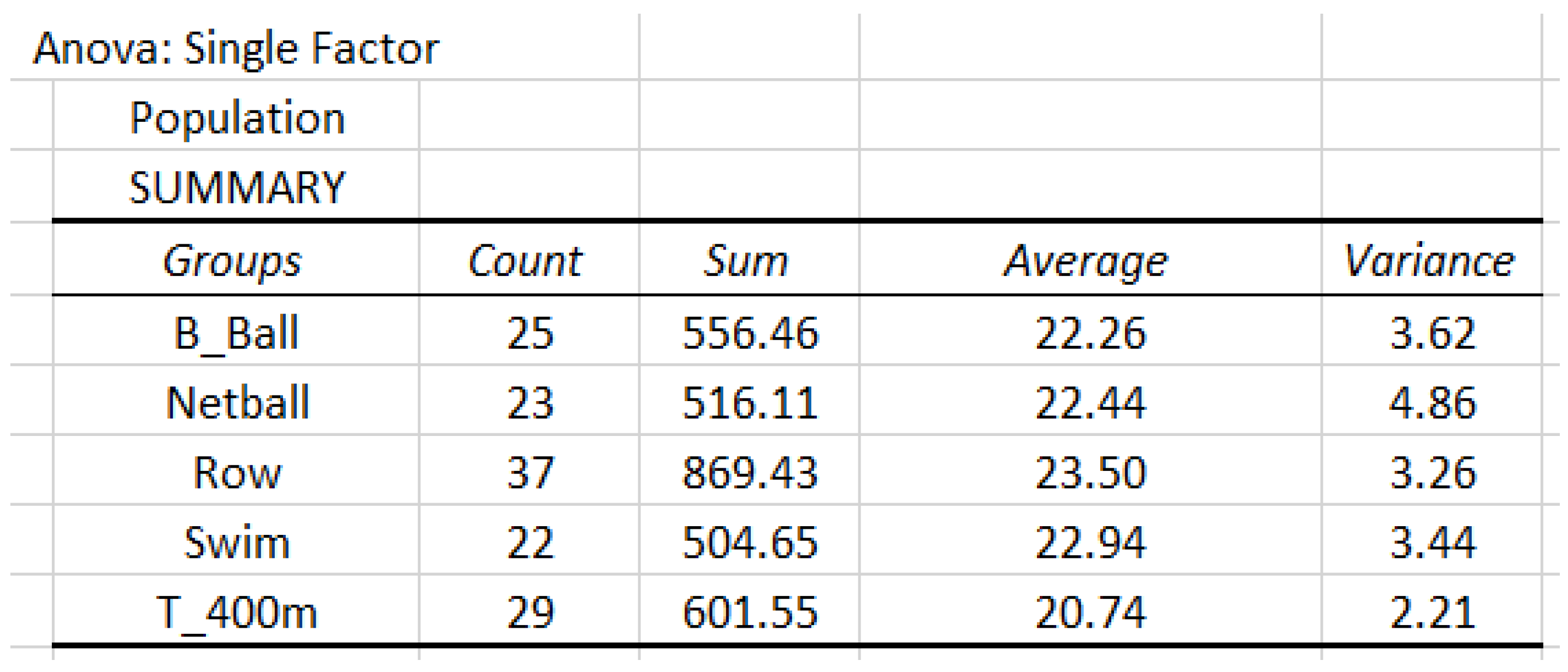
5. Simulation and Real-Data Example
6. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- (i)
- , the p-dimensional normal distribution with mean , and covariance , where is a generalized inverse of .
- (ii)
- is invariant to the choice of so that we can select the easiest
- (i)
- For estimation of k means , we choose , an matrix with rank . It is easy to check that so that the required m can be obtained from Equation (A6).
- (ii)
- For the estimation of ’s with constraint , we choose the
References
- Trafimow, D. Using the coefficient of confidence to make the philosophical switch from a posteriori to a priori inferential statistics. Educ. Psychol. Meas. 2017, 77, 831–854. [Google Scholar] [CrossRef] [PubMed]
- Trafimow, D.; MacDonald, J.A. Performing inferential statistics prior to data collection. Educ. Psychol. Meas. 2017, 77, 204–219. [Google Scholar] [CrossRef] [PubMed]
- Trafimow, D.; Wang, C.; Wang, T. Making the a priori procedure (APP) work for differences between means. Educ. Psychol. Meas. 2020, 80, 186–198. [Google Scholar] [CrossRef] [PubMed]
- Rencher, A.C.; Schaalje, G.B. Linear Models in Statistics; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Bogartz, R.S. An Introduction to the Analysis of Variance; Praeger Publishers/Greenwood Publishing Group: New York, NY, USA, 1994. [Google Scholar]
- Lindman, H.R. Analysis of Variance in Experimental Design; Springer Science & Business Media: New York, NY, USA, 2012. [Google Scholar]
- Scheffe, H. The Analysis of Variance; John Wiley & Sons: New York, NY, USA, 1999. [Google Scholar]
- McCulloch, C.E.; Searle, R. Generalized, Linear, and Mixed Models; John Wiley & Sons: New York, NY, USA, 2001. [Google Scholar]
- Roozbeh, M.; Maanavi, M.; Mohamed, N.A. A robust counterpart approach for the ridge estimator to tackle outlier effect in restricted multicollinear regression models. J. Stat. Comput. Simul. 2023, 1–18. [Google Scholar] [CrossRef]
- Yüzbaşı, B.; Arashi, M.; Ejaz, A.S. Shrinkage Estimation Strategies in Generalised Ridge Regression Models: Low/High-Dimension Regime. Int. Stat. Rev. 2020, 88, 229–251. [Google Scholar] [CrossRef]
- Yüzbaşı, B.; Arashi, M.; Akdeniz, F. Penalized regression via the restricted bridge estimator. Soft Comput. 2021, 25, 8401–8416. [Google Scholar] [CrossRef]
- Azzalini, A. The Skew-Normal and Related Families; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Ye, R.; Du, W.; Lu, Y. Bootstrap inference for skew-normal unbalanced heteroscedastic one-way classification random effects model. J. Stat. Comput. Simul. 2023, 93, 2672–2702. [Google Scholar] [CrossRef]
- Ye, R.; Du, W.; Lu, Y. Bootstrap inference for unbalanced one-way classification model with skew-normal random effects. Commun. Stat.-Simul. Comput. 2023, 1–22. [Google Scholar] [CrossRef]
- Zhu, Y.; Phillip, L.; Srijana, D.; Robert, S.; Tom, W.; Zhang, J. Evaluation of commercial Upland (Gossypium hirsutum) and Pima (G. barbadense) cotton cultivars, advanced breeding lines and glandless cotton for resistance to Alternaria leaf spot (Alternaria alternata) under field conditions. Euphytica 2018, 214, 147. [Google Scholar] [CrossRef]
- Zhu, Y.; Abdelraheem, A.; Wheeler, T.A.; Dever, J.K.; Wedegaertner, T.; Hake, K.D.; Zhang, J. Interactions between cotton genotypes and Fusarium wilt race 4 isolates from Texas and resistance evaluation in cotton. Crop Sci. 2021, 61, 1809–1825. [Google Scholar] [CrossRef]
- Zhu, Y.; Heather, E.; Terry, A.B.; Jane, D.; Derek, W.; Kater, H.; Tom, W.; Zhang, J. Effect of growth stage, cultivar, and root wounding on disease development in cotton caused by Fusarium wilt race 4 (Fusarium oxysporum f. sp. vasinfectum). Crop Sci. 2022, 63, 101–114. [Google Scholar] [CrossRef]

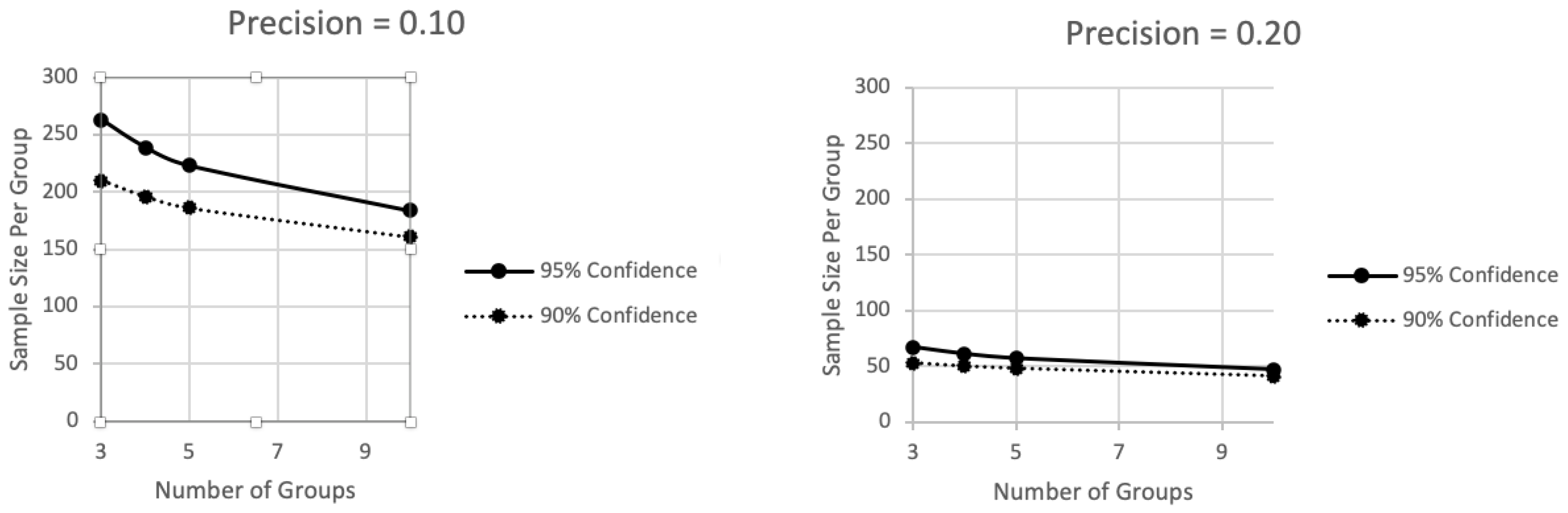




| c | f | ||||
|---|---|---|---|---|---|
| 0.90 | 0.1 | 210(446) | 196(498) | 186(534) | 161(656) |
| 0.2 | 53(112) | 50(125) | 48(134) | 41(164) | |
| 0.3 | 25(50) | 23(56) | 22(60) | 19(73) | |
| 0.4 | 14(28) | 13(32) | 13(34) | 11(41) | |
| 0.5 | 10(18) | 9(20) | 9(22) | 7(27) | |
| 0.95 | 0.1 | 263(572) | 239(621) | 223(661) | 184(784) |
| 0.2 | 67(143) | 61(156) | 57(166) | 47(196) | |
| 0.3 | 31(64) | 28(69) | 26(74) | 21(88) | |
| 0.4 | 18(36) | 16(39) | 15(42) | 12(49) | |
| 0.5 | 12(23) | 11(25) | 10(27) | 8(32) |
| c | f | ||||
|---|---|---|---|---|---|
| 0.90 | 0.1 | 274 | 234 | 200 | 170 |
| 0.15 | 124 | 105 | 90 | 80 | |
| 0.2 | 70 | 60 | 55 | 50 | |
| 0.25 | 46 | 39 | 35 | 30 | |
| 0.3 | 32 | 30 | 25 | 20 | |
| 0.95 | 0.1 | 388 | 303 | 240 | 190 |
| 0.15 | 174 | 138 | 110 | 90 | |
| 0.2 | 100 | 78 | 65 | 50 | |
| 0.25 | 64 | 51 | 40 | 40 | |
| 0.3 | 46 | 36 | 30 | 30 |
| f | |||||
| n | 388 | 174 | 100 | 64 | 46 |
| cr | 0.9476 | 0.9531 | 0.9452 | 0.9481 | 0.9521 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, L.; Wang, T.; Trafimow, D.; Choy, S.T.B. Extending the A Priori Procedure (APP) to Analysis of Variance Models under Normality. Axioms 2024, 13, 22. https://doi.org/10.3390/axioms13010022
Hu L, Wang T, Trafimow D, Choy STB. Extending the A Priori Procedure (APP) to Analysis of Variance Models under Normality. Axioms. 2024; 13(1):22. https://doi.org/10.3390/axioms13010022
Chicago/Turabian StyleHu, Liqun, Tonghui Wang, David Trafimow, and S. T. Boris Choy. 2024. "Extending the A Priori Procedure (APP) to Analysis of Variance Models under Normality" Axioms 13, no. 1: 22. https://doi.org/10.3390/axioms13010022
APA StyleHu, L., Wang, T., Trafimow, D., & Choy, S. T. B. (2024). Extending the A Priori Procedure (APP) to Analysis of Variance Models under Normality. Axioms, 13(1), 22. https://doi.org/10.3390/axioms13010022








