The Zakharov–Shabat Spectral Problem for Complexification and Perturbation of the Korteweg–de Vries Equation
Abstract
:1. Introduction
2. Application of the Zakharov and Shabat Method
- At least one of the numbers is not equal to zero;
- None of is equal to .
2.1. Representation of the Complexification of the Korteweg–de Vries Equation in the Form of the Zero-Curvature Equation
2.2. Representation of the Perturbed Korteweg–de Vries Equation in the Form of the Zero-Curvature Equation
- Only one equation contains a derivative with respect to the variable t; this is equality (29), so it will determine the sought relations between the functions and . The other equations should identically turn to zero.
- The coefficient q1 enters Equations (29) and (30) and determines the smallest power s at which the system (29)–(32) is consistent, i.e., s ≥ 0. To make the number of equations the smallest, consider the limiting case where s = 0.
- Comparing relations (26)–(29), we can note that the matrices defined in the upper equations then do not enter into the subsequent relations themselves but their derivatives with respect to the variable x, so it is easy to see that the degree of the highest derivative included in Equation (28) will determine the value of m. Next, consider the case where m = 2.
3. Construction of Conservation Laws
3.1. Conservation Laws for cKdV
- (1).
- The solution of (60) can be represented in the form (63), where
- (2).
- The functions are defined recurrently by the formulas
- All contain a linear part in the form of higher derivatives of order n–1 from and ; in the recurrence formula it is created by terms .
- In , there are no linear terms with derivatives of order lower than n–1.
- All other terms (except for those highlighted in item (1) of the recurrence Formula (65)) give non-linear terms.
- If we give the functions and a weight coefficient of 1, and the derivatives of the k-th order with respect to the variable x a weight coefficient of , where m is the weight coefficient of f (when functions and their derivatives are multiplied their weights are added), then all the terms of the polynomial have the same weight: .
3.2. Conservation Laws for pKdV System
- (1).
- The solution of (76) can be represented as (64).
- (2).
- The functions are determined recurrently by the formulas
- (81)—conservation of mass of the wave process (fully coincides with the first law for the classical soliton in the unperturbed KdV equation);
- (81)—shows the conservation of momentum of interacting functions;
- (82)—conservation of energies (similar terms for the KdV equation are ) with the interaction element of and or their functional connection .
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Faddeev, L.D.; Takhtajan, L.A. Hamiltonian Methods in the Theory of Solitons; Springer: Berlin/Heidelberg, Germany, 1987. [Google Scholar]
- Zakharov, V.E.; Shabat, A.B. A scheme for integrating the nonlinear equations of mathematical physics by the method of the inverse scattering problem. I. Funct. Anal. Its Appl. 1974, 8, 226–235. [Google Scholar] [CrossRef]
- Newell, A.C. Solitons in Mathematics and Physics; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1985. [Google Scholar]
- Flaschka, H.; Newell, A.C.; Ratiu, T. Kac–Moody Lie algebras and soliton equations. II. Lax equations associated with . Physica D 1983, 9, 303–323. [Google Scholar]
- Holod, P. Integrable Hamiltonian Systems on the Orbits of Affine Lie Groups and Periodical Problem for the Modified Korteweg-deVries Equation; Preprint ITP-82-144; Institute for Theoretical Physics: Kyiv, Ukraine, 1982. (In Russian) [Google Scholar]
- Bobenko, A.I.; Reyman, A.G.; Semenov-Tian-Shansky, M.A. The Kowalewski top 99 years later: A Lax pair, generalizations and explicit solutions. Commun. Math. Phys. 1989, 122, 321–354. [Google Scholar] [CrossRef]
- Reyman, A.G.; Semenov-Tian-Shansky, M.A. A new integrable case of the motion of the 4-dimensional rigid body. Commun. Math. Phys. 1986, 105, 461–472. [Google Scholar] [CrossRef]
- Clarkson, P.A.; Joshi, N.; Mazzocco, M. The Lax pair for the mKdV hierarchy. Séminaire et Congrès 2006, 14, 53–64. [Google Scholar]
- Skrypnyk, T.V. Quasigraded Lie algebras and modified Toda field equations. Symmetry Integr. Geom. Methods Appl. 2006, 2, 043. [Google Scholar] [CrossRef] [Green Version]
- Skrypnyk, T.V. Deformations of loop algebras and integrable systems: Hierarchies of integrable equations. J. Math. Phys. 2004, 45, 4578–4595. [Google Scholar] [CrossRef]
- Hu, B.; Ma, W.-X.; Xia, T.; Zhang, L. Nonlinear integrable couplings of a generalized super Ablowitz-Kaup-Newell-Segur hierarchy and its super bi-Hamiltonian structures. Math. Methods Appl. Sci. 2018, 41, 1565–1577. [Google Scholar] [CrossRef] [Green Version]
- Redkina, T.V.; Zakinyan, A.R.; Zakinyan, R.G.; Surneva, O.B. Hierarchies of the Korteweg– de Vries equation related to complex expansion and perturbation. Axioms 2023, 12, 371. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Shabat, A.B. Integration of nonlinear equations of mathematical physics by the method of inverse scattering. II. Funct. Anal. Appl. 1979, 13, 166–174. [Google Scholar] [CrossRef]
- Zakharov, V.E. Benney equations and quasiclassical approximation in the method of the inverse problem. Funct. Anal. Appl. 1980, 14, 89–98. [Google Scholar] [CrossRef]
- Takhtadzhyan, L.A.; Faddeev, L.D. Simple connection between the geometric and the Hamiltonian representations of integrable nonlinear equations. J. Math. Sci. 1985, 28, 800–806. [Google Scholar] [CrossRef]
- Wahlquist, H.D.; Estabrook, F.B. Prolongation structures of nonlinear evolution equations. J. Math. Phys. 1975, 16, 1–7. [Google Scholar] [CrossRef]
- Dodd, R.K.; Eilbeck, J.C.; Gibbon, J.D.; Morris, H.C. Solitons and Nonlinear Wave Equations; Academic Press: Cambridge, MA, USA, 1982. [Google Scholar]
- Calogero, F.; Degasperis, A. Spectral Transform and Solitons I; North Holland: Amsterdam, The Netherlands, 1982. [Google Scholar]
- Novikov, S.P.; Manakov, S.V.; Pitaevskii, L.P.; Zakharov, V.E. Theory of Soliton, the Inverse Scattering Method; Plenum: New York, NY, USA, 1984. [Google Scholar]
- Ekomasov, E.G.; Gumerov, A.M. Collective influence of impurities on the dynamics of kinks of modified sine-Gordon equation. Comput. Res. Model. 2013, 5, 403–412. [Google Scholar] [CrossRef] [Green Version]
- Zabusky, N.J.; Kruskal, M.D. Interaction of “solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 1965, 15, 240–243. [Google Scholar] [CrossRef] [Green Version]
- Saifullah, S.; Fatima, N.; Abdelmohsen, S.A.M.; Alanazi, M.M.; Ahmad, S.; Baleanu, D. Analysis of a conformable generalized geophysical KdV equation with Coriolis effect. Alexandria Eng. J. 2023, 73, 651–663. [Google Scholar] [CrossRef]
- Lü, X.; Chen, S.-J. N-soliton solutions and associated integrability for a novel (2+1)-dimensional generalized KdV equation. Chaos Solitons Fractals 2023, 169, 113291. [Google Scholar] [CrossRef]
- Saifullah, S.; Ahmad, S.; Alyami, M.A.; Inc, M. Analysis of interaction of lump solutions with kink-soliton solutions of the generalized perturbed KdV equation using Hirota-bilinear approach. Phys. Lett. A 2022, 454, 128503. [Google Scholar] [CrossRef]
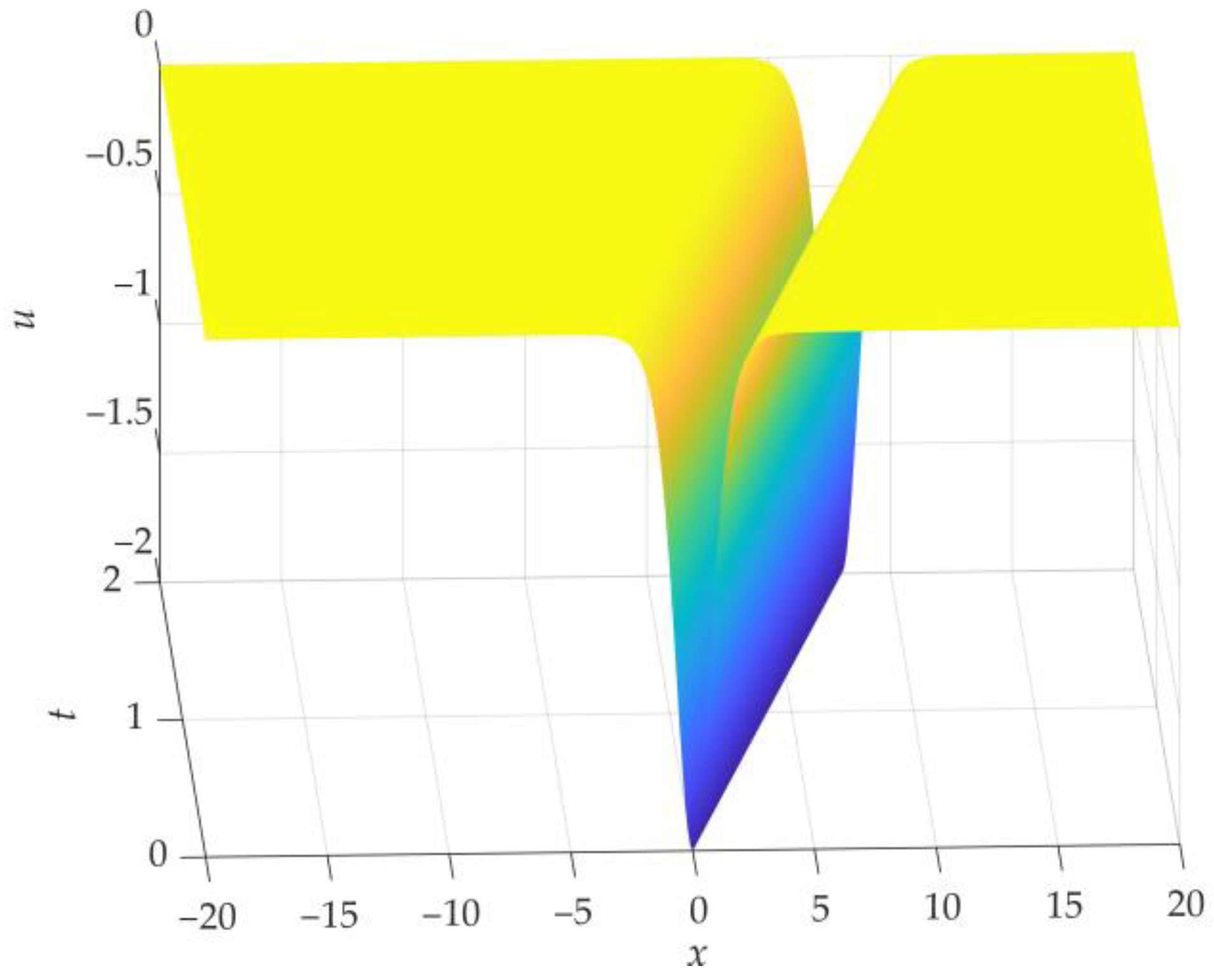
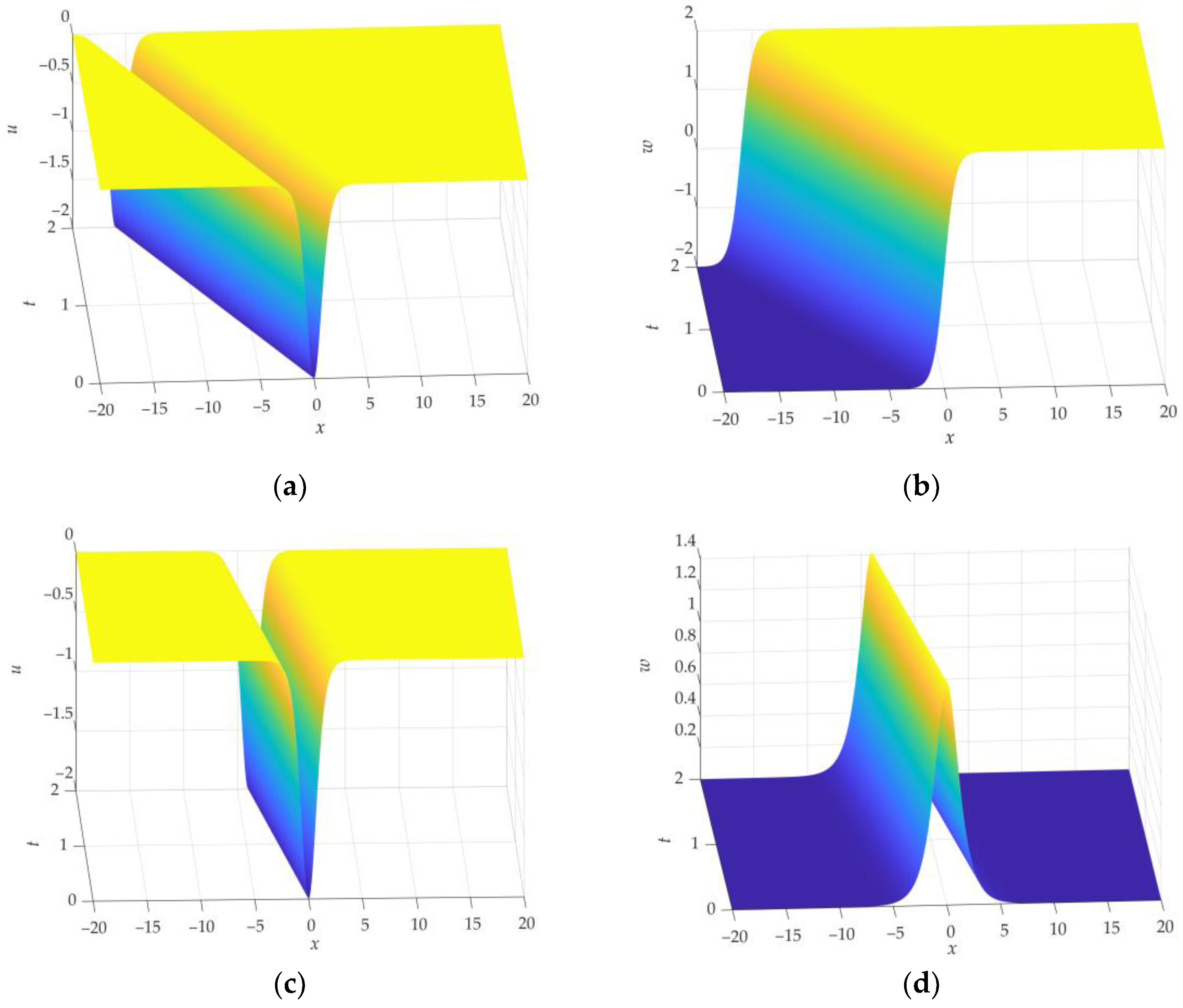
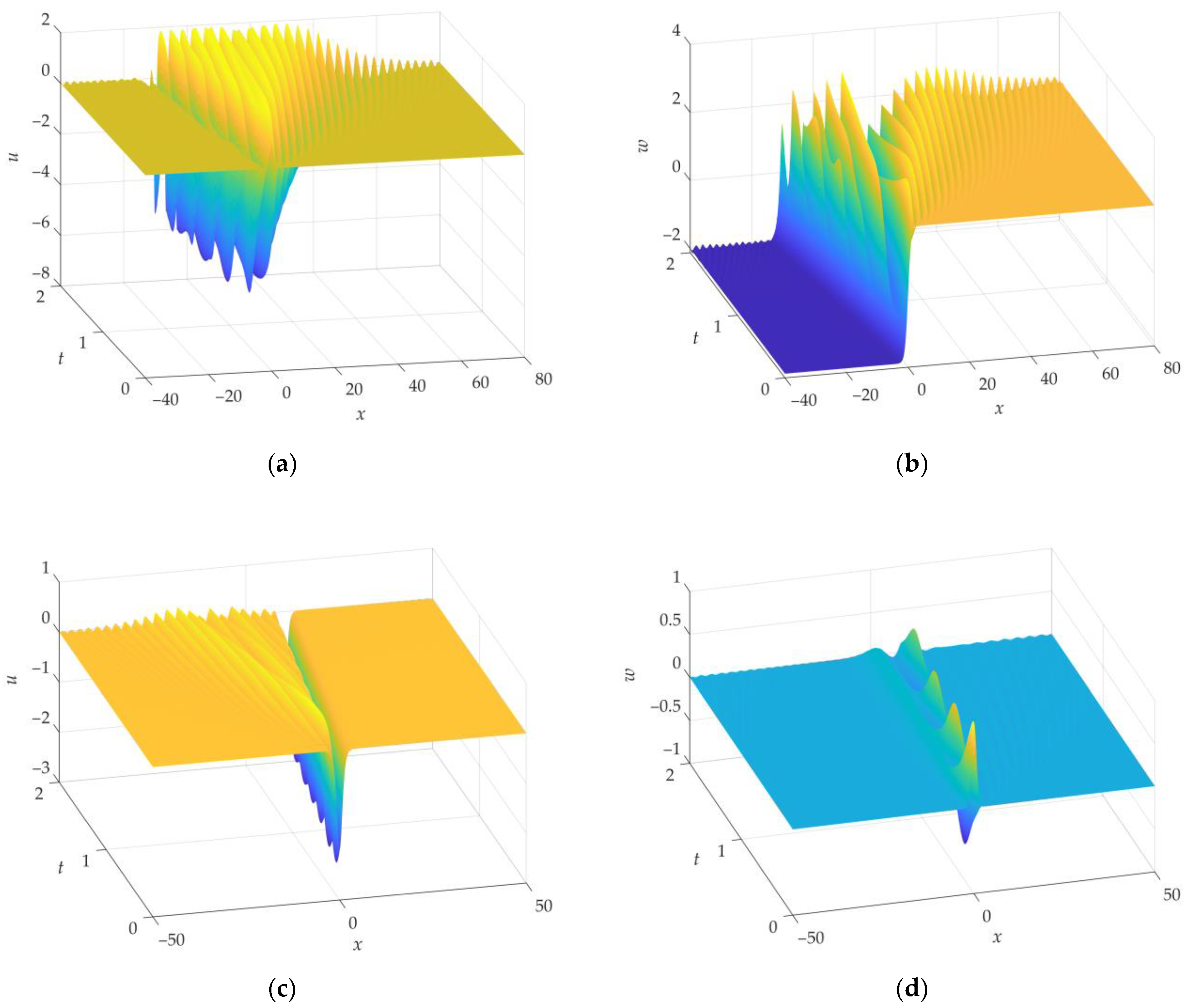
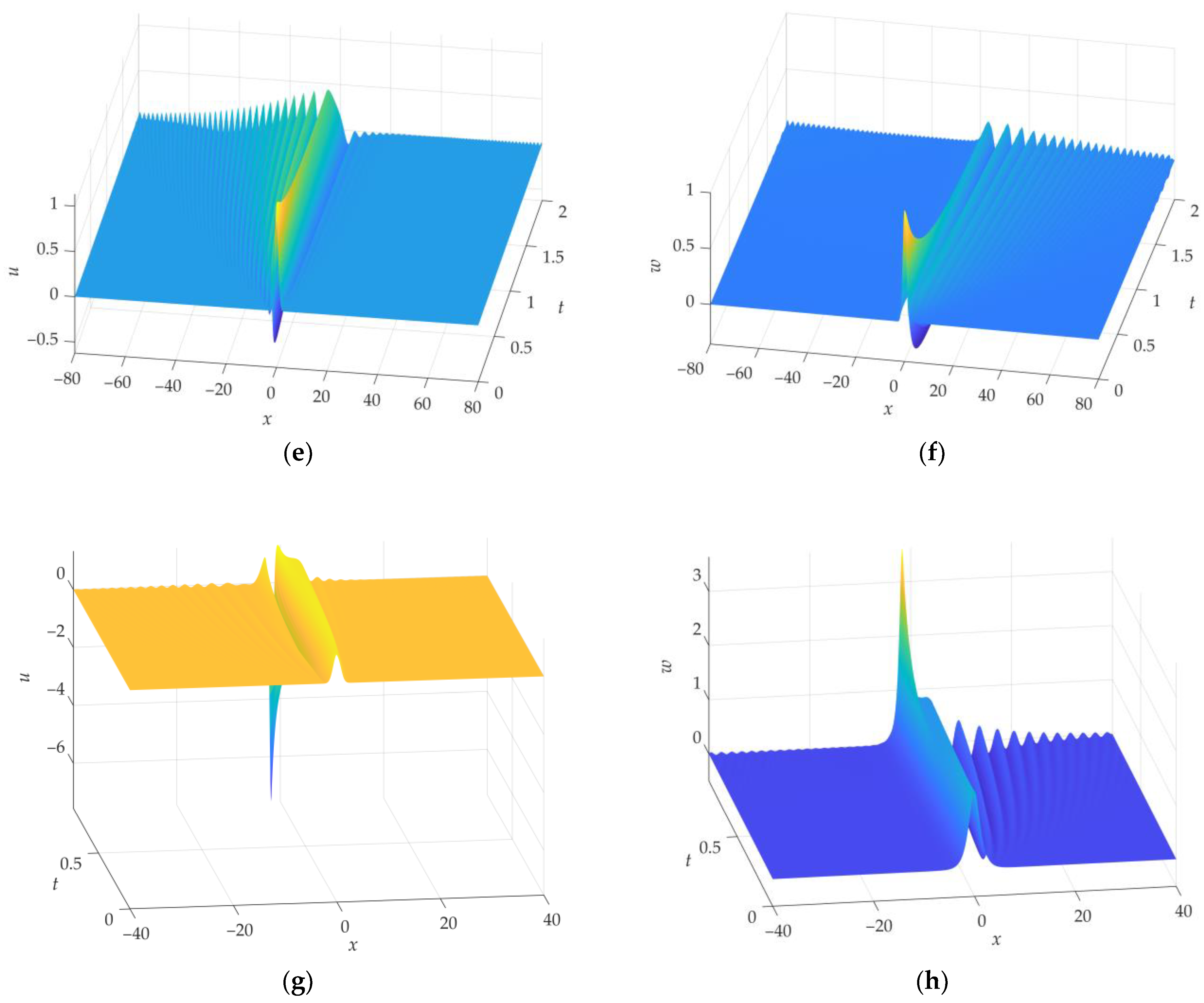
| Y | M | N | K | L | T | U | S | H | E | F | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| X | |||||||||||
| M | 0 | 0 | K | −L | T | −U | 2S | 0 | 0 | −2F | |
| N | 0 | 0 | K | −L | −T | U | 0 | 2H | −2E | 0 | |
| K | −K | −K | 0 | −M − N | 2S | 2H | 0 | 0 | T | U | |
| L | L | L | M + N | 0 | 2E | 2F | −T | −U | 0 | 0 | |
| T | −T | T | −2S | −2E | 0 | M − N | 0 | −K | 0 | −L | |
| U | U | −U | −2H | −2F | N − M | 0 | −K | 0 | −L | 0 | |
| S | −2S | 0 | 0 | T | 0 | K | 0 | 0 | 0 | M | |
| H | 0 | −2H | 0 | U | K | 0 | 0 | 0 | N | 0 | |
| E | 0 | 2E | T | 0 | 0 | L | 0 | −N | 0 | 0 | |
| F | 2F | 0 | U | 0 | L | 0 | −M | 0 | 0 | 0 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Redkina, T.V.; Zakinyan, A.R.; Zakinyan, R.G. The Zakharov–Shabat Spectral Problem for Complexification and Perturbation of the Korteweg–de Vries Equation. Axioms 2023, 12, 703. https://doi.org/10.3390/axioms12070703
Redkina TV, Zakinyan AR, Zakinyan RG. The Zakharov–Shabat Spectral Problem for Complexification and Perturbation of the Korteweg–de Vries Equation. Axioms. 2023; 12(7):703. https://doi.org/10.3390/axioms12070703
Chicago/Turabian StyleRedkina, Tatyana V., Arthur R. Zakinyan, and Robert G. Zakinyan. 2023. "The Zakharov–Shabat Spectral Problem for Complexification and Perturbation of the Korteweg–de Vries Equation" Axioms 12, no. 7: 703. https://doi.org/10.3390/axioms12070703
APA StyleRedkina, T. V., Zakinyan, A. R., & Zakinyan, R. G. (2023). The Zakharov–Shabat Spectral Problem for Complexification and Perturbation of the Korteweg–de Vries Equation. Axioms, 12(7), 703. https://doi.org/10.3390/axioms12070703





