On the Wave Structures to the (3+1)-Dimensional Boiti–Leon–Manna–Pempinelli Equation in Incompressible Fluid
Abstract
1. Introduction
2. The Two Methods
2.1. The EFM
2.2. The HFF
3. Applications
3.1. Application of the EFM
3.2. Application of the HFF
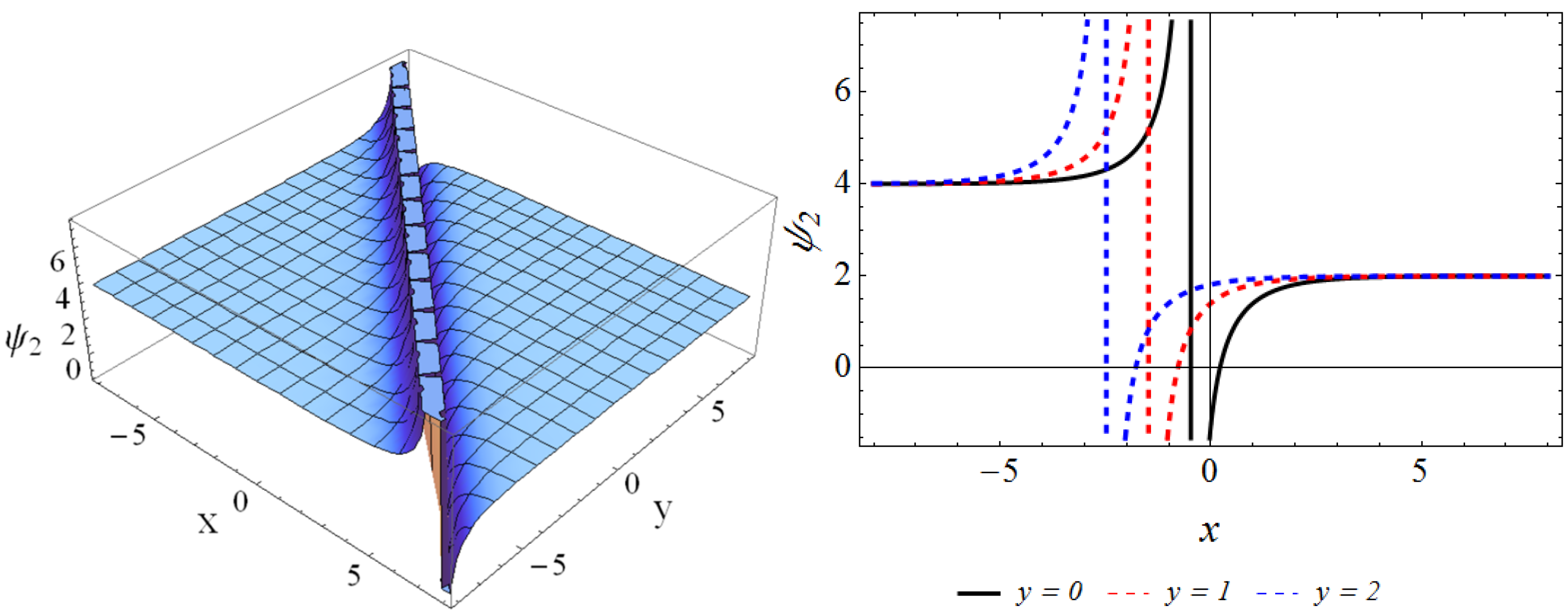
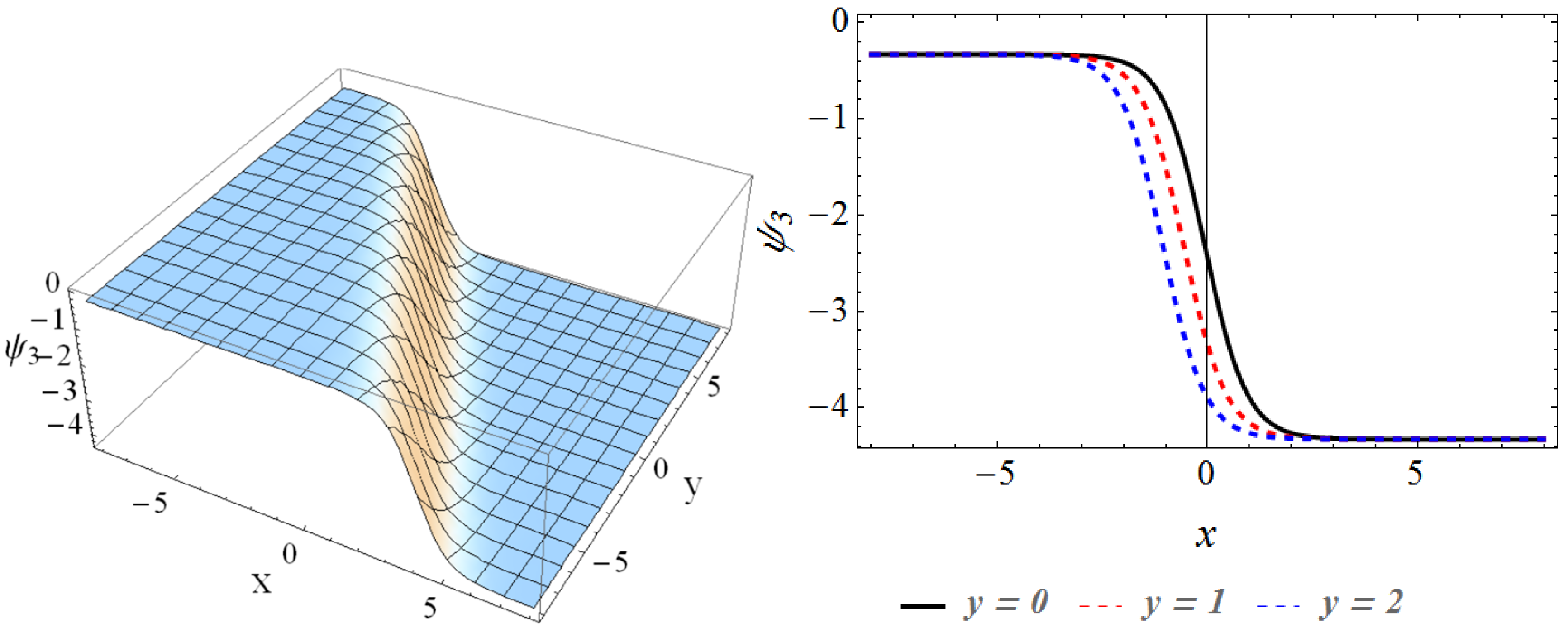
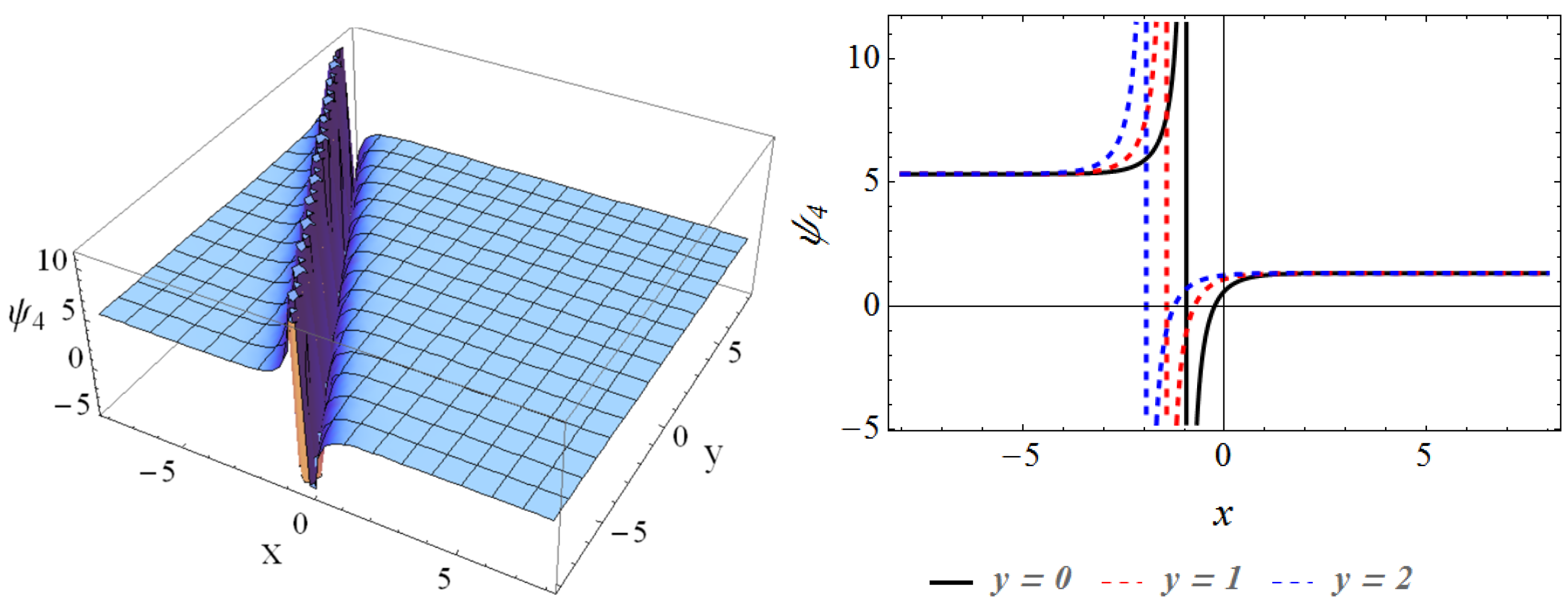
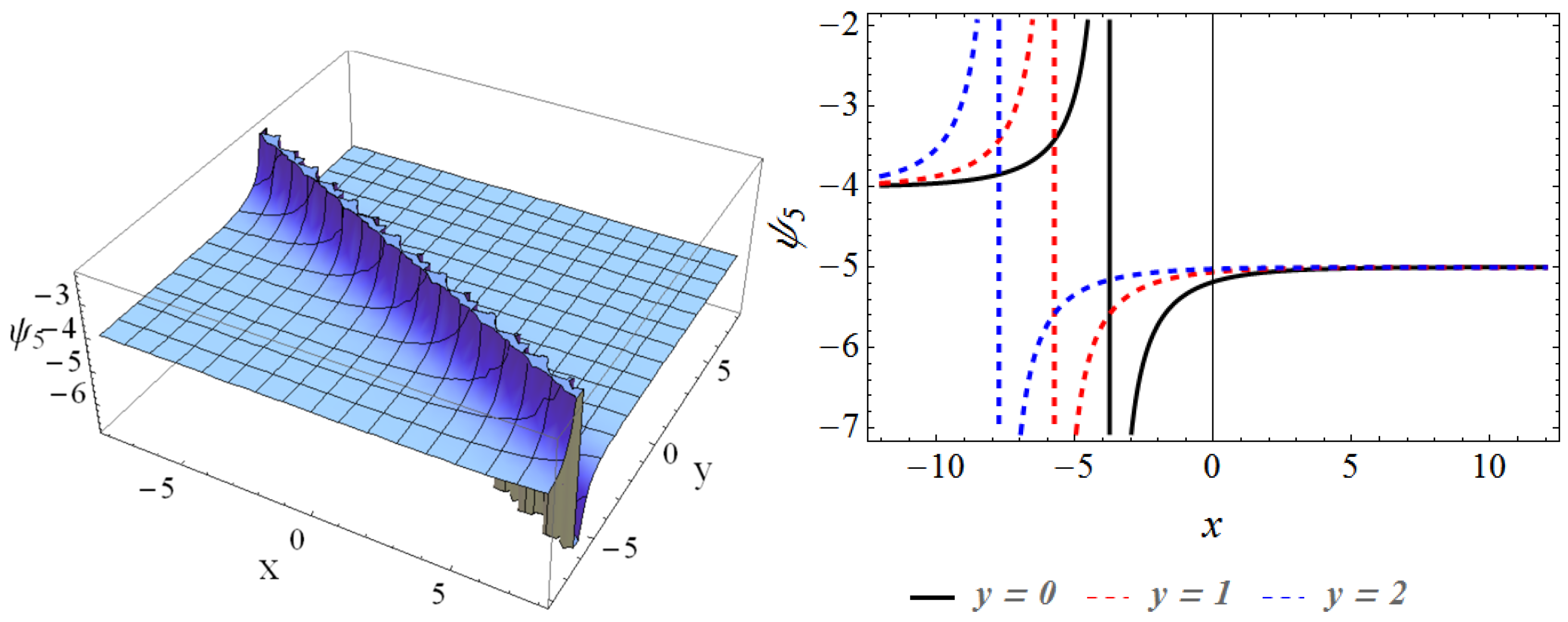
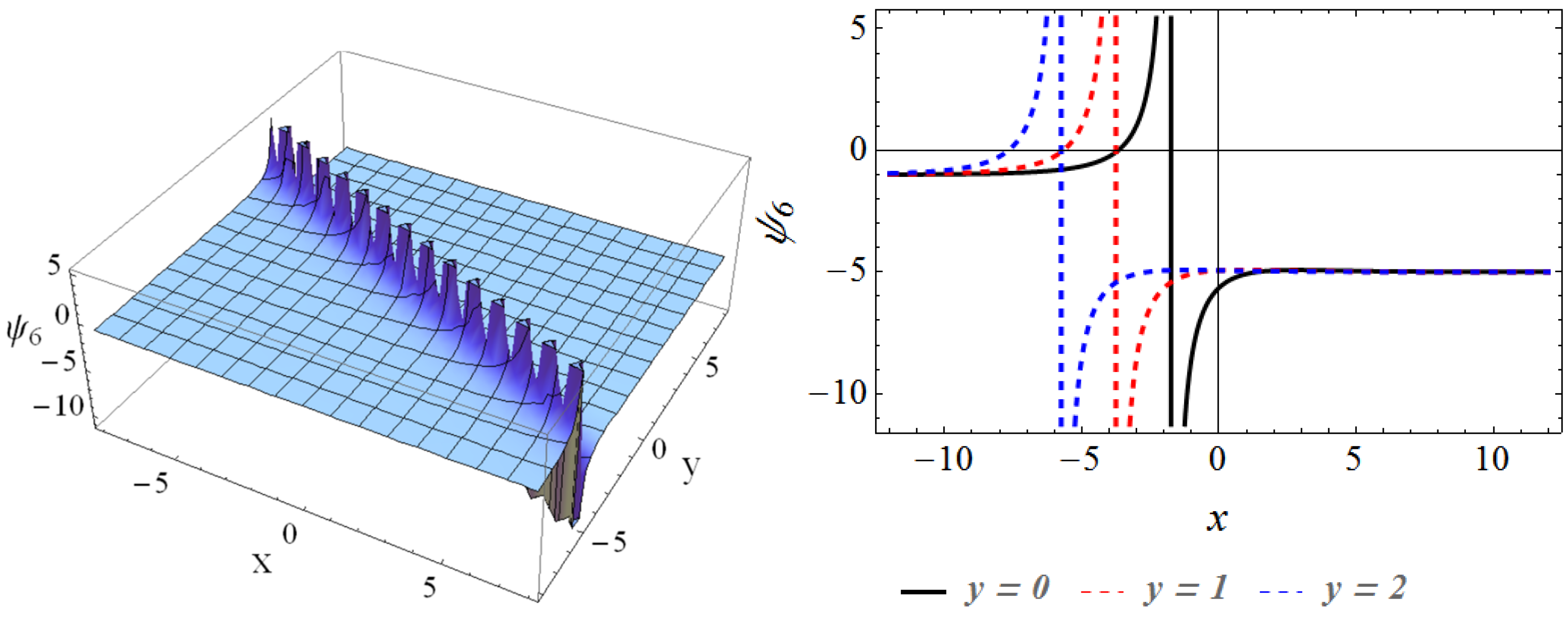
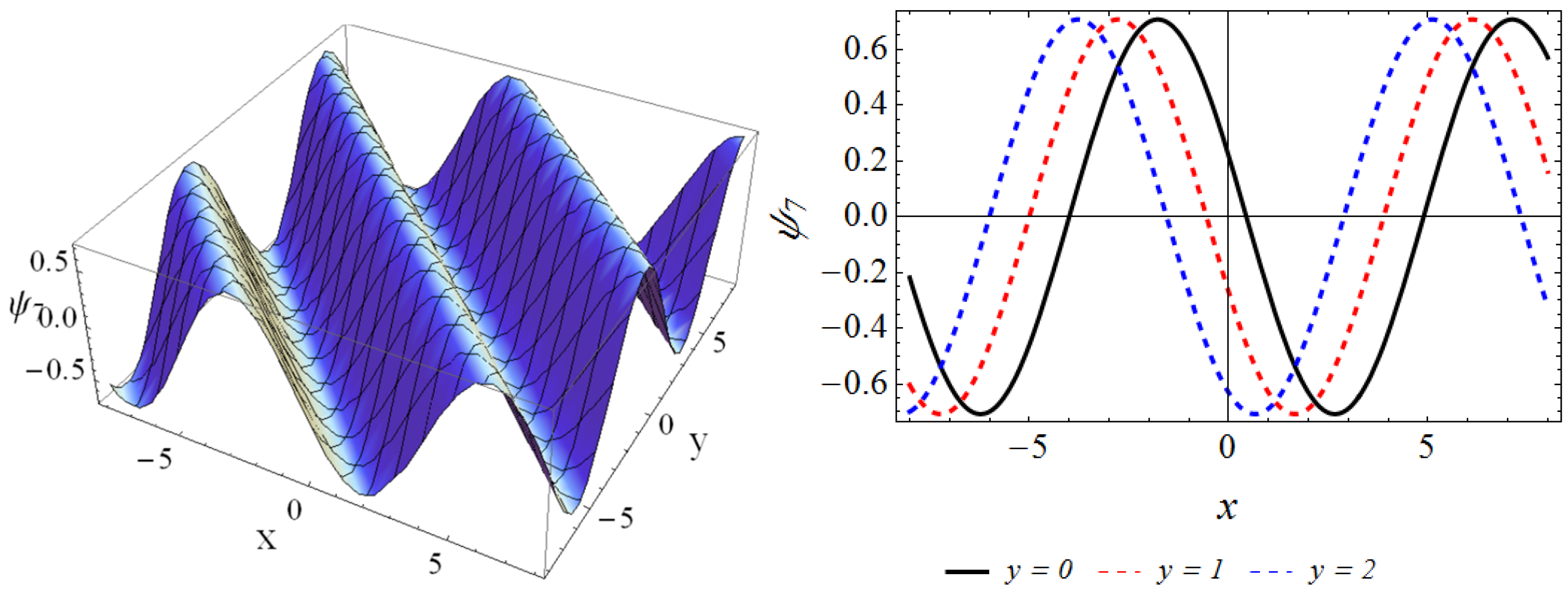
4. Physical Explanation
5. Conclusions and Future Recommendation
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Notation and Abbreviations
| PDEs | partial differential equations |
| EFM | Exp-function method |
| HFF | He’s frequency formulation |
| BLMP | Boiti–Leon–Manna–Pempinelli |
References
- Akram, G.; Sarfraz, M. Multiple optical soliton solutions for CGL equation with Kerr law nonlinearity via extended modified auxiliary equation mapping method. Optik 2021, 242, 167258. [Google Scholar] [CrossRef]
- Cheemaa, N.; Seadawy, A.R.; Chen, S. More general families of exact solitary wave solutions of the nonlinear Schrödinger equation with their applications in nonlinear optics. Eur. Phys. J. Plus 2018, 133, 547. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.H. Diverse optical solitons to the nonlinear Schrödinger equation via two novel techniques. Eur. Phys. J. Plus 2023, 138, 74. [Google Scholar] [CrossRef]
- Wang, K.J.; Si, J.; Wang, G.D.; Shi, F. A new fractal modified Benjamin-Bona-Mahony equation: Its generalized variational principle and abundant exact solutions. Fractals 2023. [Google Scholar] [CrossRef]
- Arshad, M.; Seadawy, A.; Lu, D.; Wang, J. Travelling wave solutions of generalized coupled Zakharov–Kuznetsov and dispersive long wave equations. Results Phys. 2016, 6, 1136–1145. [Google Scholar] [CrossRef]
- Soliman, A.A. The modified extended direct algebraic method for solving nonlinear partial differential equations. Int. J. Nonlinear Sci. 2008, 6, 136–144. [Google Scholar]
- Asjad, M.I.; Munawar, N.; Muhammad, T.; Hamoud, A.A.; Emadifar, H.; Hamasalh, F.K.; Azizi, H.; Khademi, M. Traveling wave solutions to the Boussinesq equation via Sardar sub-equation technique. AIMS Math. 2022, 7, 11134–11149. [Google Scholar]
- Onder, I.; Secer, A.; Ozisik, M.; Bayram, M. On the optical soliton solutions of Kundu-Mukherjee-Naskar equation via two different analytical methods. Optik 2022, 257, 168761. [Google Scholar] [CrossRef]
- Wang, K.J. Diverse wave structures to the modified Benjamin-Bona-Mahony equation in the optical illusions field. Mod. Phys. Lett. B 2023, 37, 2350012. [Google Scholar] [CrossRef]
- Wang, K.-J.; Shi, F.; Wang, G.-D. Abundant soliton structures to the (2+1)-dimensional Heisenberg ferromagnetic spin chain dynamical model. Adv. Math. Phys. 2023, 2023, 4348758. [Google Scholar] [CrossRef]
- Yildirim, Y. Optical solitons of Biswas-Arshed equation by trial equation technique. Optik 2019, 182, 876–883. [Google Scholar] [CrossRef]
- Ekici, M.; Sonmezoglu, A.; Biswas, A.; Belic, M.R. Optical solitons in (2+1)-Dimensions with Kundu-Mukherjee-Naskar equation by extended trial function scheme. Chin. J. Phys. 2019, 57, 72–77. [Google Scholar] [CrossRef]
- Özkan, Y.S.; Yaşar, E.; Seadawy, A.R. On the multi-waves, interaction and Peregrine-like rational solutions of perturbed Radhakrishnan–Kundu–Lakshmanan equation. Phys. Scr. 2020, 95, 085205. [Google Scholar] [CrossRef]
- Duan, X.; Lu, J. The exact solutions for the (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Results Phys. 2021, 21, 103820. [Google Scholar] [CrossRef]
- Yel, G.; Aktürk, T. A new approach to (3+ 1) dimensional Boiti–Leon–Manna–Pempinelli equation. Appl. Math. Nonlinear Sci. 2020, 5, 309–316. [Google Scholar] [CrossRef]
- Kaplan, M. Two different systematic techniques to find analytical solutions of the (2+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Chin. J. Phys. 2018, 56, 2523–2530. [Google Scholar] [CrossRef]
- Ali, M.R.; Ma, W.X. New exact solutions of nonlinear (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Adv. Math. Phys. 2019, 2019, 9801638. [Google Scholar] [CrossRef]
- Tang, Y.; Zai, W. New periodic-wave solutions for (2+1)- and (3+1)-dimensional Boiti–Leon–Manna–Pempinelli equations. Nonlinear Dyn. 2015, 81, 249–255. [Google Scholar] [CrossRef]
- Liu, J.G. Double-periodic soliton solutions for the (3+1)-dimensional Boiti–Leon–Manna–Pempinelli equation in incompressible fluid. Comput. Math. Appl. 2018, 75, 3604–3613. [Google Scholar] [CrossRef]
- Ali, K.K.; Mehanna, M.S. On some new soliton solutions of (3+1)-dimensional Boiti–Leon–Manna–Pempinelli equation using two different methods. Arab. J. Basic Appl. Sci. 2021, 28, 234–243. [Google Scholar] [CrossRef]
- Najafi, M.N.S.A.M. New exact solutions to the integrable (2+1) and (3+1)-dimensional Boiti-Leon-Manna-Pempinelli equations. Chin. Phys. C 2013, 37, 010201. [Google Scholar]
- Alenezi, A.M. Lump solutions of nonlinear (3+1)-dimensional for nonlinear partial differential equations. Partial. Differ. Equ. Appl. Math. 2020, 2, 100008. [Google Scholar] [CrossRef]
- He, J.H.; Wu, X.H. Exp-function method for nonlinear wave equations. Chaos Solitons Fractals 2006, 30, 700–708. [Google Scholar] [CrossRef]
- Zulfiqar, A.; Ahmad, J. Soliton solutions of fractional modified unstable Schrödinger equation using Exp-function method. Results Phys. 2020, 19, 103476. [Google Scholar] [CrossRef]
- Wu, X.H.B.; He, J.H. Exp-function method and its application to nonlinear equations. Chaos Solitons Fractals 2008, 38, 903–910. [Google Scholar]
- Ellahi, R.; Mohyud-Din, S.T.; Khan, U. Exact traveling wave solutions of fractional order Boussinesq-like equations by applying Exp-function method. Results Phys. 2018, 8, 114–120. [Google Scholar]
- Wu, X.H.B.; He, J.H. Solitary solutions, periodic solutions and compacton-like solutions using the Exp-function method. Comput. Math. Appl. 2007, 54, 966–986. [Google Scholar] [CrossRef]
- He, J.H. The simplest approach to nonlinear oscillators. Results Phys. 2019, 15, 102546. [Google Scholar] [CrossRef]
- He, J.H. The simpler, the better: Analytical methods for nonlinear oscillators and fractional oscillators. J. Low Freq. Noise Vib. Act. Control. 2019, 38, 1252–1260. [Google Scholar] [CrossRef]
- Alyousef, H.A.; Salas, A.H.; Alharthi, M.R.; El-Tantawy, S.A. Galerkin method, ansatz method, and He’s frequency formulation for modeling the forced damped parametric driven pendulum oscillators. J. Low Freq. Noise Vib. Act. Control. 2022, 41, 1426–1445. [Google Scholar] [CrossRef]
- He, J.H. Variational principle and periodic solution of the Kundu–Mukherjee–Naskar equation. Results Phys. 2020, 17, 103031. [Google Scholar] [CrossRef]
- Wang, K.; Si, J. Dynamic properties of the attachment oscillator arising in the nanophysics. Open Phys. 2023, 21, 20220214. [Google Scholar] [CrossRef]
- İlhan, E.; Kıymaz, İ.O. A generalization of truncated M-fractional derivative and applications to fractional differential equations. Appl. Math. Nonlinear Sci. 2020, 5, 171–188. [Google Scholar] [CrossRef]
- Wang, K.L. Exact travelling wave solution for the fractal Riemann wave model arising in ocean science. Fractals 2022, 30, 2250143. [Google Scholar] [CrossRef]
- Singh, J. Analysis of fractional blood alcohol model with composite fractional derivative. Chaos Solitons Fractals 2020, 140, 110127. [Google Scholar] [CrossRef]
- Wang, K.-J.; Shi, F.; Si, J.; Liu, J.-H.; Wang, G.-D. Non-differentiable exact solutions of the local fractional Zakharov-Kuznetsov equation on the Cantor sets. Fractals 2023, 31, 2350028. [Google Scholar] [CrossRef]
- He, J.H.; Ji, F.Y. Two-scale mathematics and fractional calculus for thermodynamics. Therm. Sci. 2019, 23, 2131–2133. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.H.; Si, J.; Shi, F.; Wang, G.-D. N-soliton, breather, lump solutions and diverse travelling wave solutions of the fractional (2+1)-dimensional Boussinesq equation. Fractals 2023, 31, 2350023. [Google Scholar] [CrossRef]
- He, C.H.; Liu, C.; He, J.H.; Gepreel, K.A. Low frequency property of a fractal vibration model for a concrete beam. Fractals 2021, 29, 2150117. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-N.; Wang, K.-J. On the Wave Structures to the (3+1)-Dimensional Boiti–Leon–Manna–Pempinelli Equation in Incompressible Fluid. Axioms 2023, 12, 519. https://doi.org/10.3390/axioms12060519
Chen Y-N, Wang K-J. On the Wave Structures to the (3+1)-Dimensional Boiti–Leon–Manna–Pempinelli Equation in Incompressible Fluid. Axioms. 2023; 12(6):519. https://doi.org/10.3390/axioms12060519
Chicago/Turabian StyleChen, Yan-Nan, and Kang-Jia Wang. 2023. "On the Wave Structures to the (3+1)-Dimensional Boiti–Leon–Manna–Pempinelli Equation in Incompressible Fluid" Axioms 12, no. 6: 519. https://doi.org/10.3390/axioms12060519
APA StyleChen, Y.-N., & Wang, K.-J. (2023). On the Wave Structures to the (3+1)-Dimensional Boiti–Leon–Manna–Pempinelli Equation in Incompressible Fluid. Axioms, 12(6), 519. https://doi.org/10.3390/axioms12060519






