Robust Stability of Switched-Interval Positive Linear Systems with All Modes Unstable Using the Φ-Dependent Dwell Time Technique
Abstract
1. Introduction
2. Problem Formulation and Preliminaries
3. Main Results
3.1. Continuous-Time Case
3.2. Discrete-Time Case
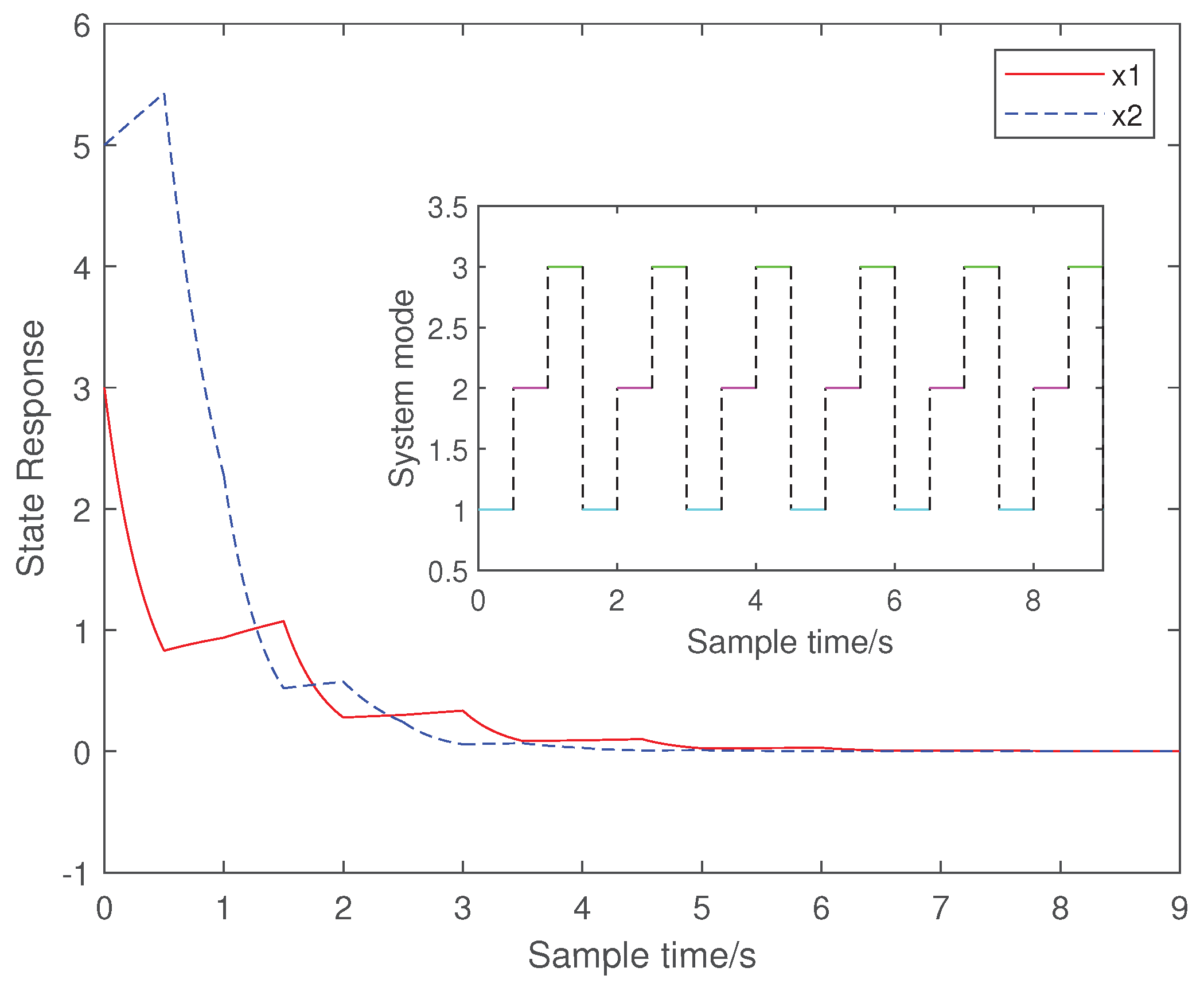
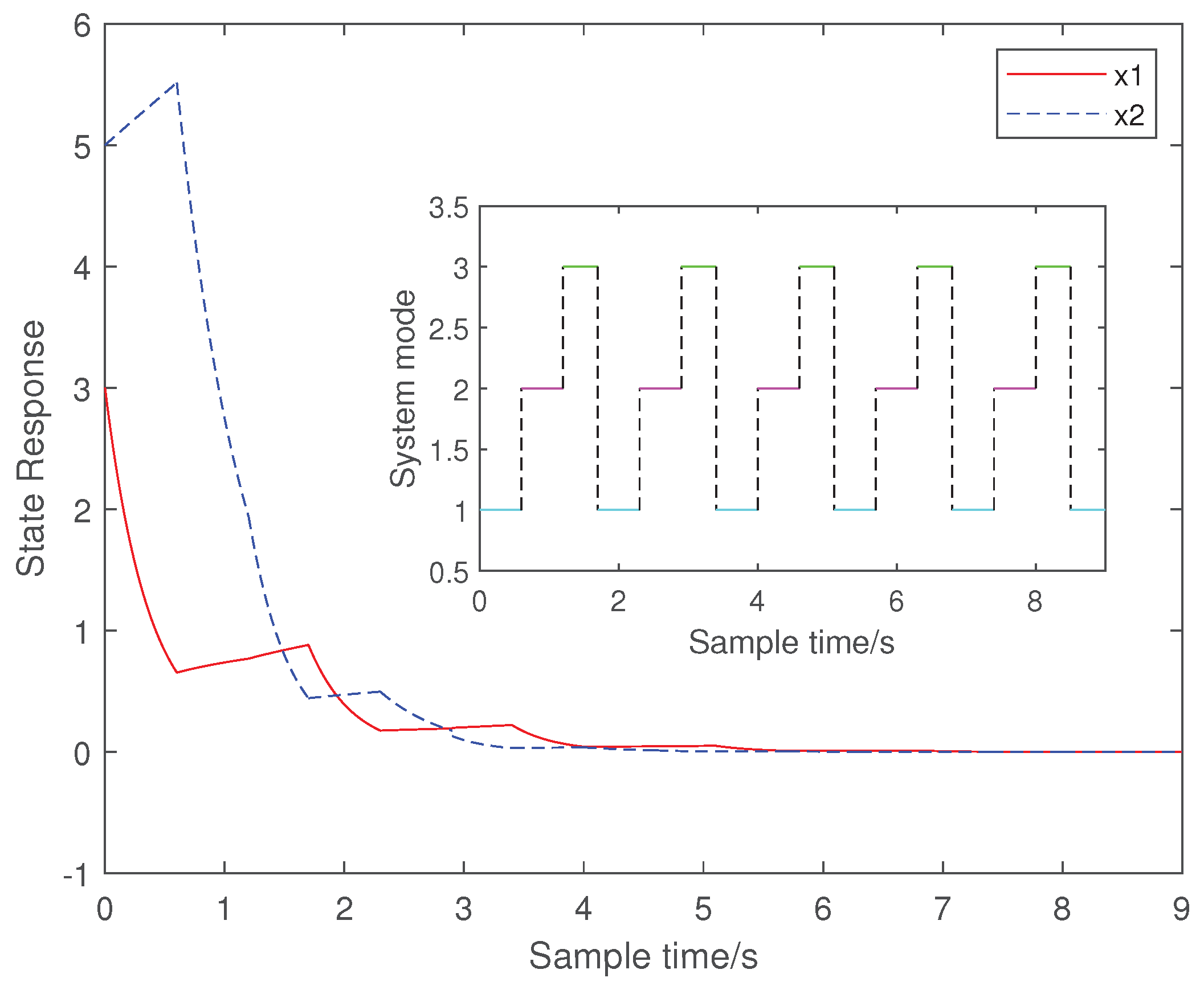
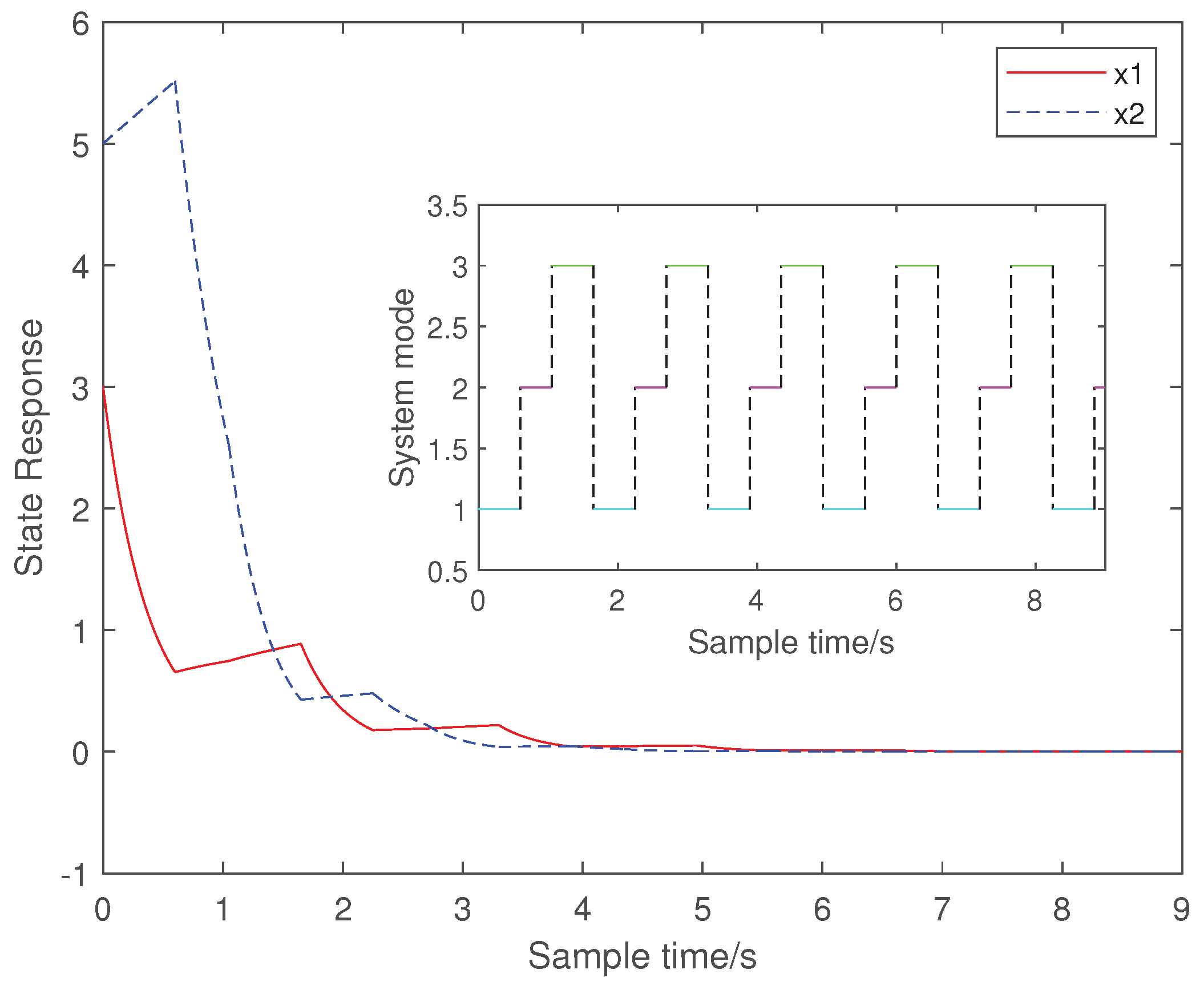
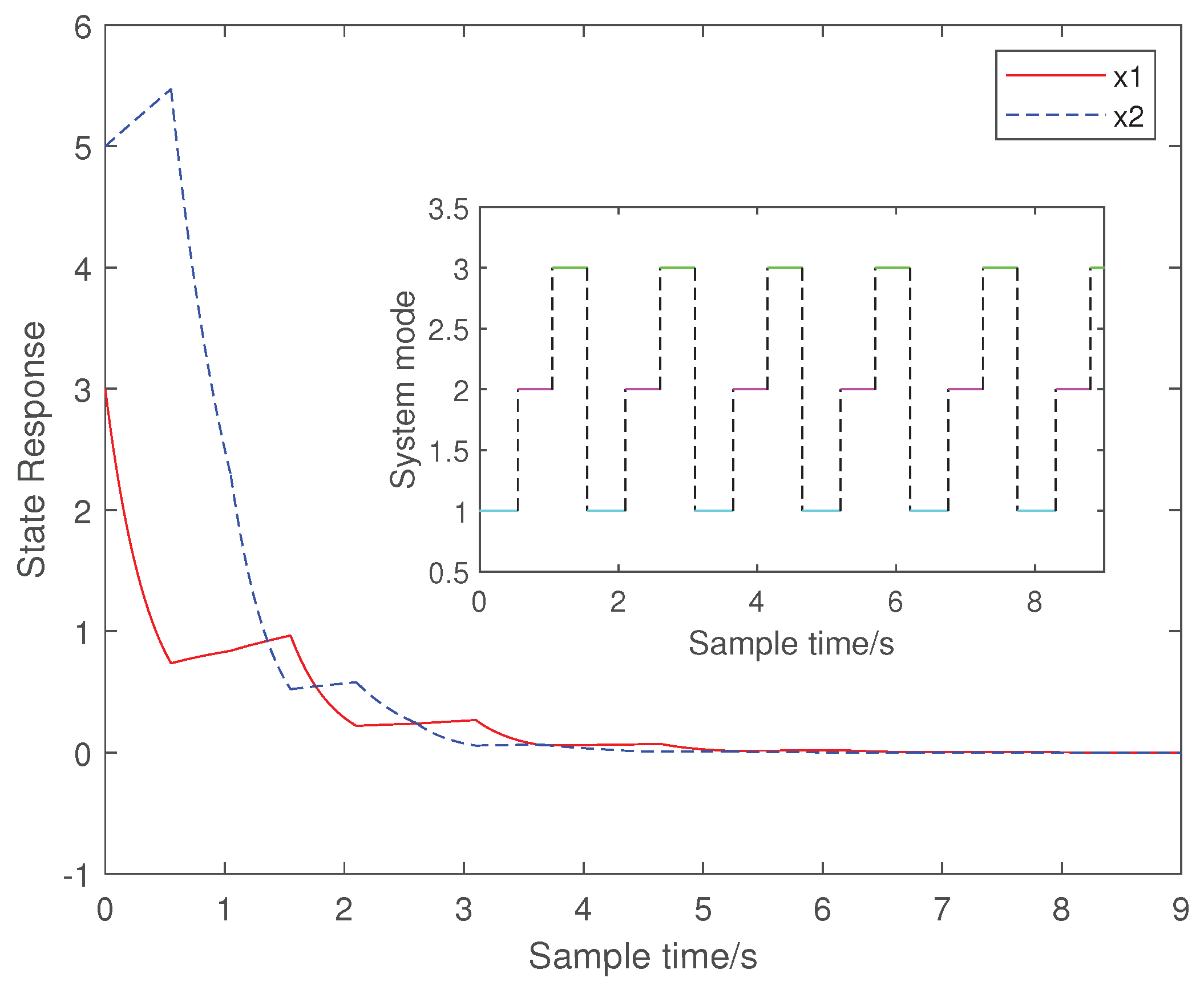
4. Illustrative Example
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jin, J.; Kim, Y.; Wee, S.; Lee, D.; Gans, N. A stable switched-system approach to collision-free wheeled mobile robot navigation. J. Intell. Robot. Syst. Theory Appl. 2017, 86, 599–616. [Google Scholar] [CrossRef]
- Maia, C.A.; Goncalves, M.M. Application of switched adaptive system to load forecasting. Electr. Power Syst. Res. 2008, 78, 721–727. [Google Scholar] [CrossRef]
- Nilsson, P.; Ozay, N.; Topcu, U.; Murray, R.M. Temporal logic control of switched affine systems with an application in fuel balancing. In Proceedings of the 2012 American Control Conference (ACC), Montreal, QC, Canada, 27–29 June 2012; pp. 5302–5309. [Google Scholar]
- Fornasini, E.; Valcher, M.E. Asymptotic stability and stabilizability of special classes of discrete-time positive switched systems. Linear Algebra Appl. 2013, 438, 1814–1831. [Google Scholar] [CrossRef]
- Blanchini, F.; Colaneri, P.; Valcher, M.E. Co-positive Lyapunov functions for the stabilization of positive switched systems. IEEE Trans. Autom. Control 2012, 57, 3038–3050. [Google Scholar] [CrossRef]
- Fornasini, E.; Valcher, M.E. On the stability of continuous-time positive switched systems. In Proceedings of the American Control Conference, Baltimore, MD, USA, 30 June–2 July 2010; pp. 225–6230. [Google Scholar]
- Gurvits, L.; Shorten, R.; Mason, O. On the stability of switched positive linear systems. IEEE Trans. Autom. Control 2007, 52, 1099–1103. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, Y.Z.; Zhao, G.D. Exponential stability for positive switched linear systems with delays and impulses. In Proceedings of the 33rd Chinese Control Conference, Nanjing, China, 28–30 July 2014; pp. 2469–2474. [Google Scholar]
- Ding, X.Y.; Shu, L.; Wang, Z.H. On stability for switched linear positive systems. Math. Comput. Model. 2011, 53, 1044–1055. [Google Scholar] [CrossRef]
- Zhao, X.D.; Zhang, L.X.; Shi, P. Stability of a class of switched positive linear time-delay systems. Int. J. Robust Nonlinear Control 2013, 23, 578–589. [Google Scholar] [CrossRef]
- Zhao, X.D.; Zhang, L.X.; Shi, P.; Karimi, H.R. Stabilization of a class of slowly switched positive linear systems: State-feedback control. In Proceedings of the American Control Conference (ACC), Montreal, QC, Canada, 27–29 June 2012; pp. 5282–5286. [Google Scholar]
- Mason, O.; Shorten, R. On linear copositive Lyapunov functions and the stability of switched positive linear systems. IEEE Trans. Autom. Control 2007, 52, 1346–1349. [Google Scholar] [CrossRef]
- Liu, X.W. Stability analysis of switched positive systems: A switched linear copositive Lyapunov function methed. IEEE Trans. Circuits Syst. II Express Briefs 2009, 56, 414–418. [Google Scholar]
- Zhao, Z.D.; Zhang, L.X.; Shi, P.; Liu, M. Stability of switched positive linear systems with average dwell time switching. Automatica 2012, 48, 1132–1137. [Google Scholar] [CrossRef]
- Yang, H.; Cocquempot, V.; Jiang, B. On stabilization of switched nonlinear systems with unstable modes. Syst. Control Lett. 2009, 58, 703–708. [Google Scholar] [CrossRef]
- Lian, J.; Liu, J. New results on stability of switched positive systems: An average dwell-time approach. IET Control Theory Appl. 2013, 7, 1651–1658. [Google Scholar] [CrossRef]
- Shi, R.Q.; Tan, X.M.; Zhao, X.D.; Zheng, X.L. Stability and l1-gain analysis for switched delay positive systems with stable and unstable subsystems. Circuits Syst. Signal Process. 2015, 34, 1683–1696. [Google Scholar] [CrossRef]
- Xiang, W.M.; Xiao, J. Stabilization of switched continuous-time systems with all modes unstable via dwell time switching. Automatica 2014, 50, 940–945. [Google Scholar] [CrossRef]
- Allerhand, L.; Shaked, U. Robust stability and stabilization of linear switched systems with dwell time. IEEE Trans. Autom. Control 2011, 56, 381–386. [Google Scholar] [CrossRef]
- Liu, J.J.; Zhang, K.J.; Wei, H.K. Robust stability of positive switched systems with dwell time. Int. J. Syst. Sci. 2016, 47, 2553–2562. [Google Scholar] [CrossRef]
- Zhang, J.F.; Han, Z.Z.; Wu, H.; Huang, J. Robust stabilization of discrete-time positive switched systems with uncertainties and average dwell time switching. Circuits Syst. Signal Process. 2014, 33, 71–95. [Google Scholar] [CrossRef]
- Feng, S.X.; Wang, J.; Zhao, J. Stability and robust stability of switched positive linear systems with all modes unstable. IEEE/CAA J. Autom. Sin. 2019, 6, 170–179. [Google Scholar] [CrossRef]
- Yu, Q.; Zhai, G.S. Stability analysis of switched systems under Φ-dependent average dwell time approach. IEEE Access 2020, 8, 30655–30663. [Google Scholar] [CrossRef]
- Zhang, J.F.; Zhang, W.; Cai, X.S.; Han, Z.Z. Stability and stabilization of positive switched systems under asynchronous switching. In Proceedings of the 2014 International Conference on Mechatronics and Control (ICMC), Jinzhou, China, 3–5 July 2014; pp. 347–352. [Google Scholar]
- Rami, M.A.; Bokharaie, V.S.; Mason, O.; Wirth, F.R. Stability criteria for sis epidemiological models under switching policies. Discret. Contin. Dyn. Syst.—Ser. B 2013, 19, 2865–2887. [Google Scholar]

| The set of real numbers | |
| The set of n-dimensional real vectors | |
| The space of real matrices | |
| () | The set of nonnegative (positive) integers |
| The transpose of a matrix | |
| Each component of vector is nonnegative (positive) | |
| The minimal (maximal) components of vector | |
| Each component of matrix is nonnegative (positive) | |
| 1-norm of ; i.e., | |
| Euclidean vector norm of ; i.e., | |
| ⟺ | If and only if |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Q.; Jiang, X. Robust Stability of Switched-Interval Positive Linear Systems with All Modes Unstable Using the Φ-Dependent Dwell Time Technique. Axioms 2023, 12, 686. https://doi.org/10.3390/axioms12070686
Yu Q, Jiang X. Robust Stability of Switched-Interval Positive Linear Systems with All Modes Unstable Using the Φ-Dependent Dwell Time Technique. Axioms. 2023; 12(7):686. https://doi.org/10.3390/axioms12070686
Chicago/Turabian StyleYu, Qiang, and Xiujuan Jiang. 2023. "Robust Stability of Switched-Interval Positive Linear Systems with All Modes Unstable Using the Φ-Dependent Dwell Time Technique" Axioms 12, no. 7: 686. https://doi.org/10.3390/axioms12070686
APA StyleYu, Q., & Jiang, X. (2023). Robust Stability of Switched-Interval Positive Linear Systems with All Modes Unstable Using the Φ-Dependent Dwell Time Technique. Axioms, 12(7), 686. https://doi.org/10.3390/axioms12070686






