1. Introduction
Differential equations are used in the mathematical modelling of physical phenomena based on rate of change of quantities [
1,
2,
3,
4] and very few of them can be solved analytically. Usually, in the absence of known analytical solutions, approximations of the true solution are obtained by applying an appropriate numerical method according to the nature of the given problem. Non-linear partial differential equations (PDEs) are considered a class of challenging problems, and researchers have tried to develop numerical codes to solve them accurately and efficiently. Obtaining accurate and efficient numerical approximations of the true solution of a given differential equation is an ongoing research topic. In this regard, the development and modification of new and existing efficient numerical code has been considered to provide accurate numerical approximations of the true solution. Many different approaches, including finite difference, finite element, finite volume and spectral method, are available to numerically solve a given PDE. However, the finite difference approach is still considered a fundamental approach for solving PDEs which occur frequently in various physical fields, such as fluid mechanics, quantum mechanics, electromagnetism, etc. In order to obtain high-order accurate approximations using the finite difference approach, a stencil based on a large number of grid points is required. A common drawback of this approach is the need to include more equations for grid points near and at the boundaries. An alternative approach to overcome this difficulty, that is, not to enlarge the stencil size is the compact finite difference approach. In this approach, values of the derivative of a function at some grid points are used where the function has already been evaluated. It seems that these type of methods have been known for almost 55 years. For instance, some of the these types of code were reported by Collatz [
5]. In this article, we proposed a non-standard combined numerical scheme based on the compact finite difference method, possessing some additional advantages for integrating the well-known non-linear Burgers’ equation given by
subject to the following initial and boundary conditions, respectively,
and
where
and
v are the velocity, spatial coordinate, time and kinematic viscosity, respectively, whereas
,
are known functions. Equation (
1) shows that convection-, diffusion- and time-dependent terms are present in the equation.
Equation (
1) was first introduced by H. Bateman in 1915 [
6] and later in 1948 by a Dutch physicist J M Burgers to mathematically model turbulence [
7]. Burgers was an active researcher in the field of fluid mechanics and to honour his contributions, this equation was termed the Burgers’ equation. The first analytical treatment of the one-dimensional Burgers’ equation was proposed by Bateman [
6]. In general, for specific initial and boundary conditions, the problem in eq1 can be treated analytically. More precisely, in the gas dynamics Holf [
8] and Cole [
9] proved independently that for any initial condition the problem can be reduced to form of a linear homogeneous heat equation that can be treated analytically. Thus, the exact solution of Burgers’ equation can be written in the form of a Fourier series which is difficult to handle analytically; therefore, it is important to have stable and efficient numerical methods to deal with these types of problems. For a good reference to the exact solution of the one-dimensional Burgers’ equation, one can consult the survey by Benton et al. [
10].
Many researchers have proposed numerical schemes to numerically solve (
1). Zhang et al. [
11] discussed its numerical solution by applying two-step predictor–corrector method, known as MacCormack method in combination with compact finite difference method for spatial discretization. M. Sari et al. [
12] numerically solved the one-dimensional Burgers’ equation using a sixth-order compact finite difference method in combination with low storage third-order total variation diminishing Runge–Kutta scheme. Gao et al. [
13] proposed a numerical scheme by employing a high-accuracy mutli-quadratic quasi-interpolation to approximate the spatial derivatives and a first-order accurate forward difference method for the temporal derivative. For more details on existing numerical schemes to solve Burgers’ equation, one can consult [
14,
15,
16,
17,
18,
19,
20] and the references cited therein. In the scientific literature, other types of Burgers’ equation have been addressed, for instance, Hadhoud et al. [
21] proposed non-polynomial B-spline and shifted Jacobi spectral collocation methods for solving time-fractional non-linear coupled Burgers’ equations, and the local fractional two-dimensional Burgers-type equations was addressed in Yang et al. [
22].
To obtain the numerical solution to Equation (
1) using a compact finite difference approach, researchers have used explicit Runge–Kutta-type or linear multi-step methods for advancing the integration process in the time direction. Due to the small stability region of explicit RK methods, a small step size is required to obtain a reliable approximation to the true solution. This increases the computational cost and in some cases due to the very small grid size, round off errors may arise resulting in an unrealistic solution. In order to use linear multi-step methods, the user needs the starting values to begin the integration process. For this, the user needs to use appropriate codes as starters; for instance, RK methods. To obtain a reliable approximation to the true solution of a given problem with a large grid size, an implicit RK method could be sufficient. However, when using implicit RK methods, the user needs to solve a system of equations at each step of the integration due to the implicit nature of the code. To provide an alternative to these codes, we propose a class of unconventional explicit unconditionally stable numerical codes to accurately and efficiently integrate a given problem. Many authors have proposed these type of methods [
23,
24,
25,
26,
27]. In this regard, we first present the derivation of the family of non-standard methods to integrate systems of ODEs arising in the semi-discretization of initial-boundary values of a Burgers’ equation and analyse its basic characteristics, i.e., order of accuracy and linear stability analysis. Then, by considering this family of methods and coupling them with a fourth-order compact finite difference scheme, a novel combined compact numerical scheme is obtained to deal with the initial-boundary value problem given in Equation (
1).
The rest of the article is organized as follows:
Section 2 concerns the development of a class of time marching numerical schemes and the basic characteristics. In
Section 3, we recall the fourth-order compact finite difference method used with an explicit numerical time marching scheme presented in
Section 4 as a combined numerical scheme. The Von Neumann stability analysis of the scheme is carried out in
Section 5.
Section 6 discusses the numerical experiments carried out by applying the combined numerical scheme, while
Section 7 draws some conclusions of the present work.
2. Development of the Explicit Numerical Schemes
The semi-discretization of Equation (
1) with regard to the space variable results in a system of first-order ODEs can be written as
where
In order to develop the numerical schemes, we consider the scalar case of the above system,
for
.
Firstly, the interval
was discretize into
k subintervals of equal length as
. We termed the step size
and the approximate solution at
as
. Let us assume the following approximation to the true solution to the problem at point
as
where
and
are arbitrary constants. The type of methods was initially proposed by J.D. Lambert [
28]. A difference operator is linked with Equation (
6)
Using the Taylor series to expand
around the point
, we obtain
To obtain the first-order numerical schemes and avoid the second derivative, the coefficient of
in (8) must vanish. Equating the coefficient of
equal to zero, we obtain
given by
provided that
Using
and taking
arbitrarily in Equation (
6), a one-parameter family of numerical schemes is obtained as
This is a new one-parameter class of explicit numerical schemes for solving the system (
5).
2.1. Special Cases
By putting different values for the free parameter , we obtain a different numerical scheme.
If we let
, the well-known method of Fatunla [
29] is obtained as a special case
Let
, we obtain
Let
, we obtain
2.2. Basic Characteristics of the Numerical Scheme
This section concerns the basic characteristics [
30,
31,
32] of the proposed scheme (9), including the order of accuracy and linear stability analysis. Note from the derivation that the scheme has first-order accuracy and its linear stability analysis was carried out by applying it to the Dahlquist test equation given by
Theorem 1. The numerical scheme given in (9) is -stable [33] for Proof. Considering the proposed numerical scheme (9) and applying it to the Dahlquist test equation, we obtain
which may be simplified as
Now consider the stability function
where
For a stable numerical scheme, we must have
It is easy to verify that for
and
, the numerical scheme (9) is
-stable [
33]. Further, we also note that
Hence, the proposed class of methods is also
-stable [
33] for
□
3. Compact Finite Difference Method
Compact finite differences have additional advantages over conventional finite differences as they provide higher accuracy in the approximation with a smaller stencil size. In the present article, we considered fourth-order compact finite differences in order to approximate the spatial derivatives from Equation (
1). Here we recall a fourth-order compact finite difference method and for more details on this type of method, one can consult [
34,
35,
36,
37] and the references cited therein.
The space variable is discretized into N equal subintervals of equal length , where
Consider a fourth-order compact finite difference scheme for discretizing the first spacial derivative from (
1) at the interior nodes
where the prime mark denotes the derivative with respect to the space variable and the following one-sided boundary scheme to obtain approximations at boundary points given by
for
, and
for
.
The above equations can be written in matrix form
Solving the above system of equations, we can obtain approximations to the first-order space derivatives at the discrete points of interest.
Similarly, in order to approximate the second spacial derivative appearing in the equation, we consider the following fourth-order compact finite difference scheme given by:
For interior nodes
For boundary points we have:
for
and
for
.
The complete matrix system for a tri-diagonal fourth-order compact scheme to approximate the second derivative can be written as follows
Solving the above system of equations, one can obtain an approximation to the second-order space derivatives from the equation at the discrete points of interest.
6. Numerical Experiments
In this section, we evaluated the performance of the proposed combined numerical scheme (
21) for solving the Burgers’ equation (
1), subject to the following initial, boundary conditions and exact solution [
11].
Consider the problem
subject to the initial and boundary conditions given by
The exact solution of the problem is given by
The
Table 1 represents the different notations denote the numerical schemes considered in this article.
In
Table 2, the absolute errors are presented for
by applying the new scheme for
and
. This demonstrates that the new scheme accurately integrates the given problem.
Table 3 presents a comparison of the absolute errors produced by the new scheme and conventional scheme 1 for
and
. It is evident from the data that the new scheme performs better in terms of accuracy.
As the new scheme has first-order accuracy in time, in
Table 4 we present a comparison of the numerical results obtained from the new scheme and classical scheme for the values
and
. The numerical data given in
Table 4 shows the good performance of the new scheme compared to classical scheme.
Table 5 shows the rate of convergence (ROC) of the new scheme in the space direction for the values
and
.
Table 5 shows that the ROC agrees with the theoretical order of convergence of the new scheme in the space direction.
In
Table 6, we have presented a comparison of the new scheme with existing approaches [
16,
38] in terms of
-error for values
,
,
,
,
, and
. The data given in
Table 6 demonstrate the better performance of the new scheme.
Next,
Table 7 displays the better performance of the new scheme in comparison with an existing scheme [
39] in terms of accuracy using values
.
Table 8 presents the numerical data concerning
-error and
-error for different values of
v for
,
,
and
.
Table 8 shows that the new scheme produces very accurate approximations to the true solution of the given test problem.
Table 9 shows a comparison between the absolute errors in the application of the new scheme and conventional scheme 2. The numerical data support the better performance of the new scheme. Note that the new scheme integrates the given problem with fewer function evaluations than the conventional scheme 2.
Table 10 shows the ROC of the new scheme in the time direction for values
and
.
Table 10 shows that the ROC agrees with the theoretical order of convergence of the new scheme in the time direction.
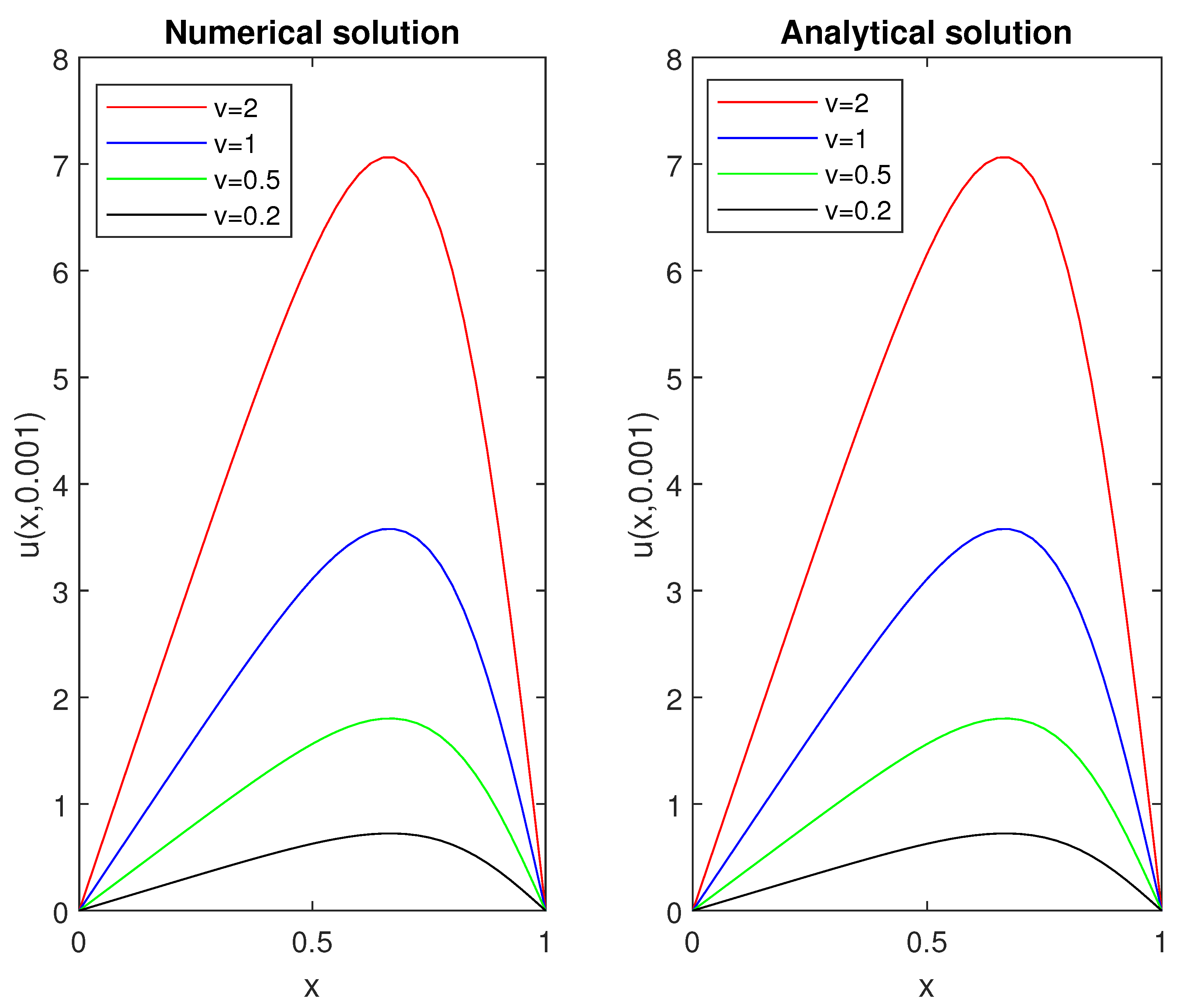
The
Figure 1 shows the numerical and analytical solutions for different values of
v using values
,
and
. It is evident that the physical behaviour of the numerical solution agrees with the physical behaviour of the analytical solution for various values of parameter
v.
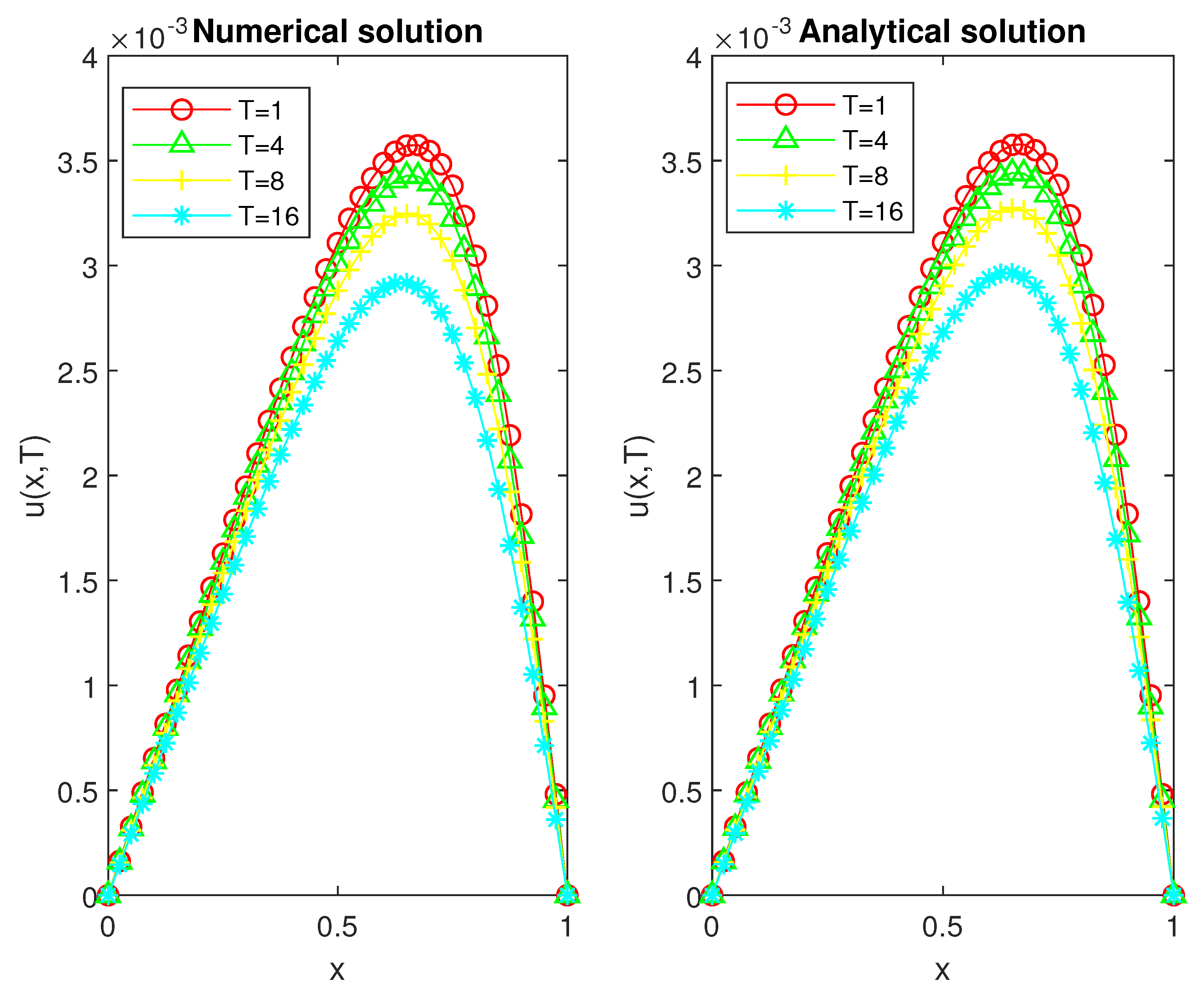
The numerical solution is plotted against the exact solution for different values of
T. The
Figure 2 shows that the physical behaviour of both solutions is similar. The plot is drawn using the values
,
,
and
.