A Time-Varying Coefficient Double Threshold GARCH Model with Explanatory Variables
Abstract
1. Introduction
2. Model and Estimation
3. Simulation Studies
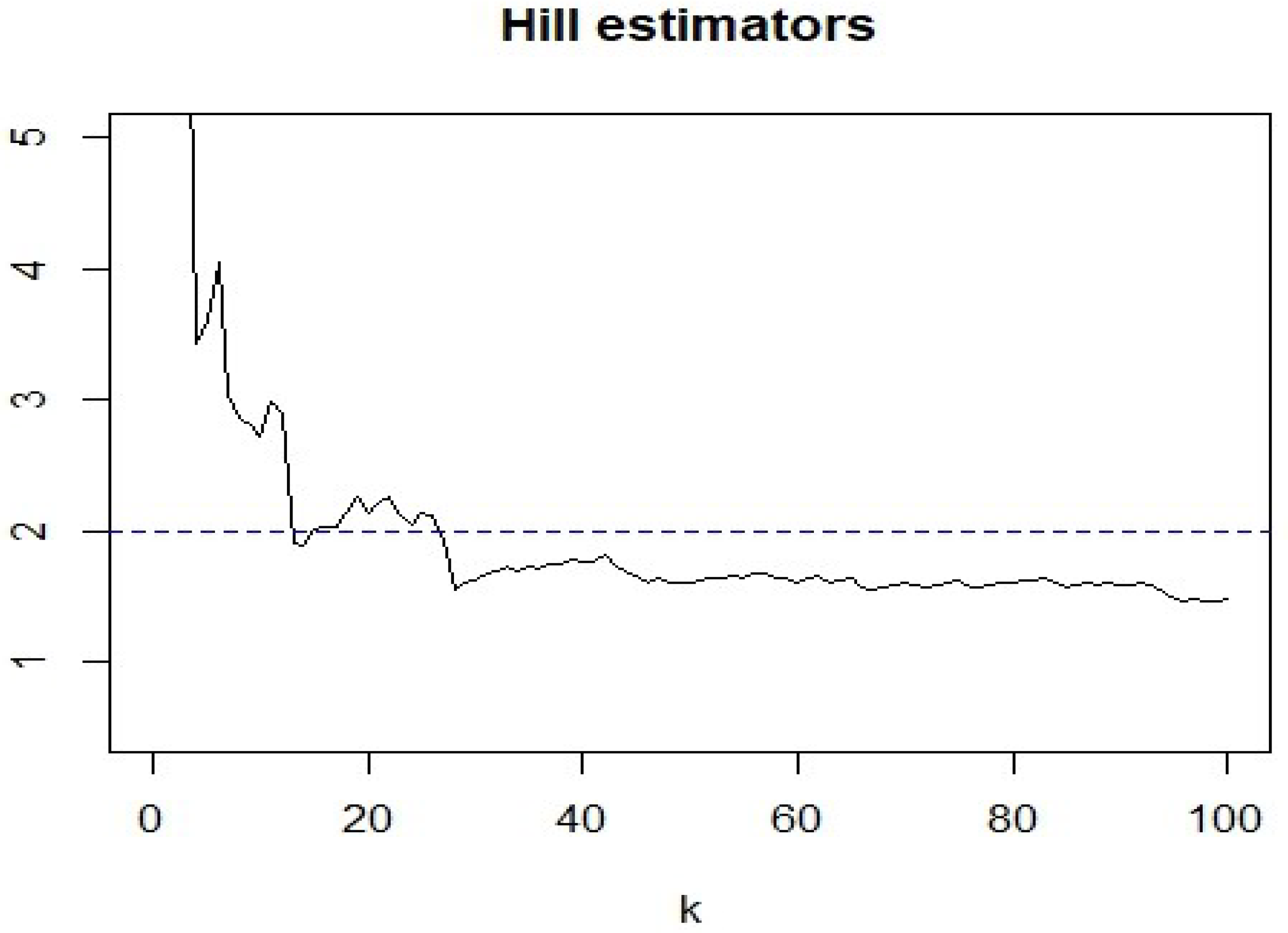
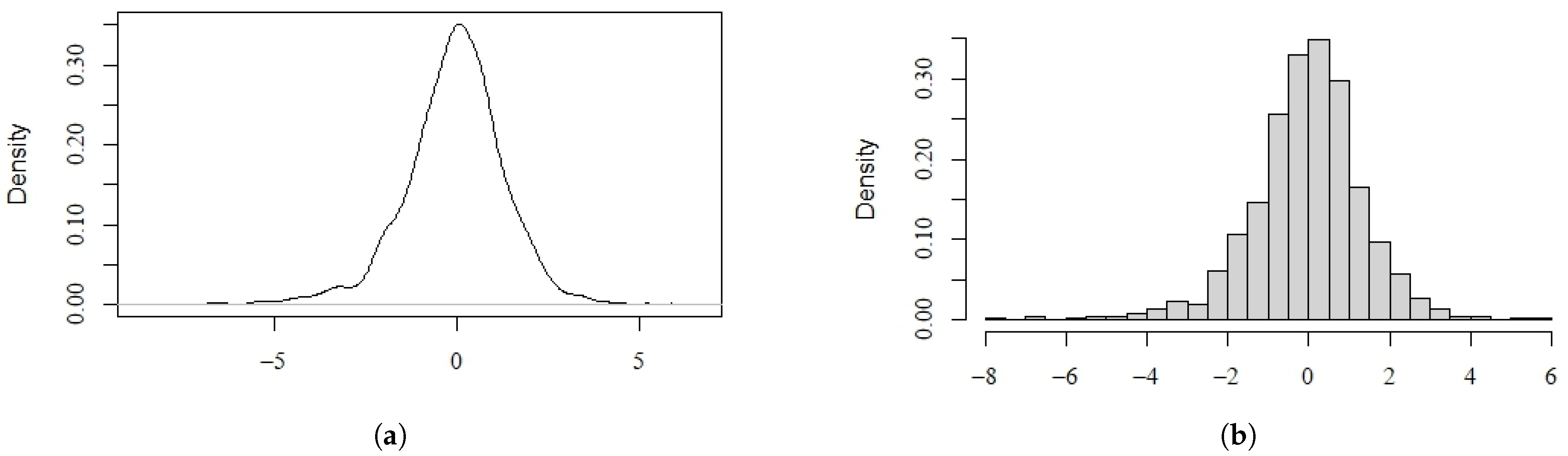
4. Real Data Example
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ACF | Autocorrelation Function Plot |
| PACF | Partial Autocorrelation Function Plot |
Appendix A
References
- Nicholls, D.F.; Quinn, B.G. Random Coefficient Autoregressive Models: An Introduction; Springer: New York, NY, USA, 1982. [Google Scholar]
- Tjostheim, D. Estimation in nonlinear time series models. Stoch. Process. Their Appl. 1986, 21, 251–273. [Google Scholar] [CrossRef]
- Kim, Y.W.; Basawa, I.V. Empirical Bayes estimation for first order autoregressive processes. Aust. J. Stat. 1992, 34, 105–114. [Google Scholar] [CrossRef]
- Hwang, S.Y.; Basawa, I.V. Asymptotic optimal inference for a class of nonlinear time series models. Stoch. Process. Their Appl. 1993, 46, 91–113. [Google Scholar] [CrossRef]
- Hwang, S.Y.; Basawa, I.V. Large sample inference for conditional exponential families with applications to nonlinear time series. J. Stat. Plan. Inference 1994, 38, 141–157. [Google Scholar] [CrossRef]
- Hwang, S.Y.; Basawa, I.V. Parameter estimation for generalized random coefficient autoregressive processes. J. Stat. Plan. Inference 1998, 68, 323–337. [Google Scholar] [CrossRef]
- Aue, A. Strong approximation for RCA(1) time series with applications. Stat. Probab. Lett. 2004, 68, 369–382. [Google Scholar] [CrossRef]
- Aue, A.; Horvath, L.; Steinebach, J. Estimation in Random Coefficient Autoregressive Models. J. Time Ser. Anal. 2006, 27, 61–76. [Google Scholar] [CrossRef]
- Thavaneswaran, A.; Appadoo, S.S.; Ghahramani, M. RCA models with GARCH innovations. Appl. Math. Lett. 2009, 22, 110–114. [Google Scholar] [CrossRef]
- Tong, H. On a Threshold Model; Sijhoff & Noordhoff: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Tong, H.; Lim, K.S. Threshold autoregression, limit cycles and cyclical data. J. R. Stat. Soc. Ser. B (Methodol.) 1980, 42, 245–268. [Google Scholar] [CrossRef]
- Engle, R.F. Autoregressive Conditional Heteroscedasticity with Estimates of the Variance of United Kingdom Inflation. Econometrica 1982, 50, 987–1007. [Google Scholar] [CrossRef]
- Bollerslev, T. Generalized autoregressive conditional heteroskedasticity. J. Econom. 1986, 31, 307–327. [Google Scholar] [CrossRef]
- Karpoff, J.M. The Relation between Price Changes and Trading Volume: A Survey. J. Financ. Quant. Anal. 1987, 22, 109–126. [Google Scholar] [CrossRef]
- Tong, H. Non-Linear Time Series: A Dynamical System Approach; Oxford University Press: Oxford, UK, 1990. [Google Scholar]
- Li, C.W.; Li, W.K. On a Double-Threshold Autoregressive Heteroscedastic Time Series Model. J. Appl. Econometr. 1996, 11, 253–274. [Google Scholar] [CrossRef]
- Brooks, C. A Double-threshold GARCH Model for the French Franc/Deutschmark exchange rate. J. Forecast. 2001, 20, 135–143. [Google Scholar] [CrossRef]
- Chen, C.W.S.; Yang, M.J.; Gerlach, R.; Jim, L.H. The asymmetric reactions of mean and volatility of stock returns to domestic and international information based on a four-regime double-threshold GARCH model. Phys. Stat. Mech. Appl. 2006, 366, 401–418. [Google Scholar] [CrossRef]
- Yang, Y.L.; Chang, C.L. A double-threshold GARCH model of stock market and currency shocks on stock returns. Math. Comput. Simul. 2008, 79, 458–474. [Google Scholar] [CrossRef]
- Chen, C.W.S.; Gerlach, R. Semi-parametric quantile estimation for double threshold autoregressive models with heteroskedasticity. Comput. Stat. 2013, 28, 1103–1131. [Google Scholar] [CrossRef]
- Chen, C.W.S.; Chen, M.; Chen, H. Pairs Trading via Three-Regime Threshold Autoregressive GARCH Models. In Proceedings of the 7th International Conference of the Thailand Econometric Society, TES 2014, Chiang Mai, Thailand, 8–10 January 2014; pp. 127–140. [Google Scholar]
- Chen, C.W.S.; So, M.K.P.; Chiang, T.C. Evidence of Stock Returns and Abnormal Trading Volume: A Threshold Quantile Regression Approach. Jpn. Econ. Rev. 2016, 67, 96–124. [Google Scholar] [CrossRef]
- Luukkonen, R.; Saikkonen, P.; Teräsvirta, T. Testing linearity against smooth transition autoregressive models. Biometrika 1988, 75, 491–499. [Google Scholar] [CrossRef]
- Teräsvirta, T. Specification, estimation, and evaluation of smooth transition autoregressive models. J. Am. Stat. Assoc. 1994, 89, 208–218. [Google Scholar]
- Zheng, H.T.; Basawa, I.V. First-order observation-driven integer-valued autoregressive processes. Stat. Probab. Lett. 2008, 78, 1–9. [Google Scholar] [CrossRef]
- Yu, M.J.; Wang, D.H.; Yang, K. A class of observation-driven random coefficient INAR(1) processes based on negative binomial thinning. J. Korean Stat. Soc. 2019, 48, 248–264. [Google Scholar] [CrossRef]
- Yang, K.; Li, H.; Wang, D.H.; Zhang, C.H. Random coefficients integer-valued threshold autoregressive processes driven by logistic regression. AStA Adv. Stat. Anal. 2021, 105, 533–557. [Google Scholar] [CrossRef]
- Hwang, S.Y.; Basawa, I.V. Explosive Random Coefficient AR(1) Processes and Related Asymptotics for Least Squares Estimation. J. Time Ser. Anal. 2005, 26, 807–824. [Google Scholar] [CrossRef]
- Berkes, I.; Horvath, L.; Ling, S.Q. Estimation in nonstationary random coefficient autoregressive models. J. Time Ser. Anal. 2009, 30, 395–416. [Google Scholar] [CrossRef]
- Aue, A.; Horvath, L. Quasi-likelihood estimation in stationary and nonstationary autoregressive models with random coefficients. Stat. Sin. 2011, 21, 973–999. [Google Scholar]
- Weiss, A. Asymptotic theory for ARCH models: Estimation and testing. Econom. Theory 1986, 2, 107–131. [Google Scholar] [CrossRef]
- Engle, R.F.; Bollerslev, T. Modelling the persistence of conditional variances. Econom. Rev. 1986, 5, 1–50. [Google Scholar] [CrossRef]
- Berkes, I.; Horvath, L.; Kokoszka, P. GARCH processes: Structure and estimation. Bernoulli 2003, 9, 201–227. [Google Scholar] [CrossRef]
- Francq, C.; Zokaian, J.M. Maximum Likelihood Estimation of Pure GARCH and ARMA-GARCH Processes. Bernoulli 2004, 10, 605–637. [Google Scholar] [CrossRef]
- Hall, P.; Yao, Q. Inference in ARCH and GARCH models with heavy-tailed errors. Econometrica 2003, 71, 285–317. [Google Scholar] [CrossRef]
- Zhu, K.; Ling, S.Q. Global self-weighted and local quasi-maximum exponential likelihood estimators for ARMA-GARCH/IGARCH models. Ann. Stat. 2011, 39, 2131–2163. [Google Scholar] [CrossRef]
- Tsay, R.S. Testing and modeling threshold autoregressive processes. J. Am. Stat. Assoc. 1989, 84, 231–240. [Google Scholar] [CrossRef]
- Li, D.; Ling, S.Q. On the least squares estimation of multiple-regime threshold autoregressive models. J. Econom. 2012, 167, 240–253. [Google Scholar] [CrossRef]
- Yu, P. Likelihood estimation and inference in threshold regression. J. Econom. 2012, 167, 274–294. [Google Scholar] [CrossRef]
- Li, D.; Tong, H. Nested sub-sample search algorithm for estimation of threshold models. Stat. Sin. 2016, 26, 1543–1554. [Google Scholar] [CrossRef]
- Zhang, T.W.; Wang, D.H.; Yang, K. Quasi-maximum exponential likelihood estimation for double-threshold GARCH models. Can. J. Stat. 2021, 49, 1152–1178. [Google Scholar] [CrossRef]
- Sheng, D.S.; Wang, D.H.; Kang, Y. A new RCAR(1) model based on explanatory variables and observations. Commun. Stat.-Theory Methods 2022. [Google Scholar] [CrossRef]
- Li, G.D.; Guan, B.; Li, W.K.; Yu, P.L.H. Hysteretic autoregressive time series models. Biometrika 2015, 102, 717–723. [Google Scholar] [CrossRef]
- Silverman, B.W. Density Estimation for Statistics and Data Analysis; Chapman and Hall: New York, NY, USA, 1986. [Google Scholar]
- Ling, S.Q. Self-weighted and local quasi-maximum likelihood estimators for ARMA-GARCH/IGARCH models. J. Econom. 2007, 140, 849–873. [Google Scholar] [CrossRef]


| Parameters | r | r | ||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| n | True Value | 0.8 | 0.3 | 0.2 | 0.4 | 0.3 | 0.1 | 0.3 | 0.5 | 1 | 0.8 | 0.3 | 0.2 | 0.4 | 0.3 | 0.1 | 0.3 | 0.5 | 1 | |
| QMELE | Standard QMLE | |||||||||||||||||||
| 300 | Bias | 0.1007 | 0.0481 | 0.0039 | −0.0457 | −0.0236 | 0.0365 | −0.0077 | −0.0184 | 0.0210 | −0.0183 | 0.1870 | 0.1013 | 0.0089 | −0.0263 | 0.0828 | 0.1431 | −0.0126 | −0.1083 | |
| SD | 0.4195 | 0.1691 | 0.1211 | 0.2341 | 0.1584 | 0.1879 | 0.1231 | 0.1877 | 0.1306 | 0.3206 | 0.2072 | 0.1571 | 0.1966 | 0.1139 | 0.2533 | 0.1643 | 0.1717 | 0.1589 | ||
| ASD | 0.7650 | 0.1517 | 0.1401 | 0.1895 | 0.2446 | 0.1621 | 0.1020 | 0.1707 | – | 0.2697 | 0.1919 | 0.1278 | 0.1654 | 0.0588 | 0.2241 | 0.1436 | 0.1638 | – | ||
| 600 | Bias | 0.0586 | 0.0395 | 0.0152 | −0.0461 | −0.0147 | 0.0258 | −0.0075 | −0.0136 | 0.0294 | −0.0408 | 0.1681 | 0.1019 | 0.0171 | −0.0134 | 0.0728 | 0.1464 | −0.0036 | −0.1193 | |
| SD | 0.2860 | 0.1220 | 0.0784 | 0.1454 | 0.0955 | 0.1199 | 0.0837 | 0.1093 | 0.1049 | 0.2673 | 0.1523 | 0.1185 | 0.1424 | 0.0791 | 0.1588 | 0.1068 | 0.1061 | 0.1491 | ||
| ASD | 0.4960 | 0.1008 | 0.0682 | 0.1289 | 0.1112 | 0.1026 | 0.0733 | 0.1144 | – | 0.1953 | 0.1420 | 0.0965 | 0.1258 | 0.0407 | 0.1416 | 0.1034 | 0.1098 | – | ||
| 900 | Bias | 0.0564 | 0.0328 | 0.0153 | −0.0403 | −0.0039 | 0.0219 | −0.0008 | −0.0176 | 0.0177 | −0.0600 | 0.1651 | 0.1013 | 0.0205 | −0.0103 | 0.0679 | 0.1444 | 0.0036 | −0.1163 | |
| SD | 0.2456 | 0.1135 | 0.0665 | 0.1201 | 0.0750 | 0.0933 | 0.0626 | 0.0864 | 0.0945 | 0.1840 | 0.1405 | 0.0916 | 0.1132 | 0.0694 | 0.1139 | 0.0867 | 0.0798 | 0.1219 | ||
| ASD | 0.4346 | 0.0893 | 0.0636 | 0.1130 | 0.0798 | 0.0764 | 0.0596 | 0.0893 | – | 0.1569 | 0.1229 | 0.0821 | 0.1050 | 0.0345 | 0.1081 | 0.0838 | 0.0867 | – | ||
| 300 | Bias | 0.0879 | 0.0315 | 0.0132 | −0.0071 | 0.0027 | 0.0324 | −0.0120 | 0.-0029 | 0.0238 | −0.1411 | 0.2665 | 0.1933 | 0.0543 | −0.0094 | 0.1366 | 0.2501 | −0.0133 | -0.2345 | |
| SD | 0.4255 | 0.3210 | 0.1480 | 0.2814 | 0.2419 | 0.1818 | 0.1322 | 0.1676 | 0.1953 | 0.3770 | 0.3246 | 0.2838 | 0.2625 | 0.1409 | 0.2843 | 0.2467 | 0.1624 | 0.2522 | ||
| ASD | 0.9172 | 0.2465 | 0.1407 | 0.2424 | 0.1672 | 0.1560 | 0.1086 | 0.1498 | – | 0.4344 | 0.3131 | 0.2361 | 0.1998 | 0.0905 | 0.2697 | 0.1977 | 0.1553 | – | ||
| 600 | Bias | 0.0266 | 0.0169 | 0.0379 | 0.0037 | 0.0044 | 0.0171 | −0.0085 | −0.0025 | 0.0140 | −0.1510 | 0.2746 | 0.1852 | 0.0438 | −0.0050 | 0.1284 | 0.2671 | −0.0070 | −0.2326 | |
| SD | 0.3477 | 0.1625 | 0.1254 | 0.2269 | 0.1197 | 0.0923 | 0.0790 | 0.0923 | 0.1361 | 0.2698 | 0.2885 | 0.2609 | 0.1927 | 0.1019 | 0.1769 | 0.1781 | 0.1063 | 0.2089 | ||
| ASD | 0.7976 | 0.1744 | 0.1417 | 0.1869 | 0.1223 | 0.0803 | 0.0754 | 0.0923 | – | 0.4236 | 0.3202 | 0.5972 | 0.1711 | 0.0677 | 0.1664 | 0.1524 | 0.1054 | – | ||
| 900 | Bias | 0.0503 | 0.0134 | 0.0131 | 0.0007 | 0.0017 | 0.0142 | −0.0017 | −0.0073 | 0.0145 | −0.1730 | 0.2729 | 0.1861 | 0.0388 | 0.0040 | 0.1163 | 0.2783 | −0.0051 | −0.2459 | |
| SD | 0.3070 | 0.1306 | 0.1145 | 0.1761 | 0.0893 | 0.0684 | 0.0631 | 0.0674 | 0.1015 | 0.2359 | 0.2300 | 0.1948 | 0.1692 | 0.0856 | 0.1308 | 0.1371 | 0.0798 | 0.1986 | ||
| ASD | 0.4979 | 0.1286 | 0.0985 | 0.1458 | 0.0854 | 0.0574 | 0.0616 | 0.0719 | – | 0.2980 | 0.2428 | 0.1846 | 0.1643 | 0.0551 | 0.1198 | 0.1261 | 0.0810 | – | ||
| 300 | Bias | 0.0460 | 0.0254 | 0.0361 | 0.0062 | 0.0094 | 0.0353 | −0.0110 | −0.0175 | 0.0181 | −0.2024 | 0.2828 | 0.2106 | 0.1221 | 0.0182 | 0.1552 | 0.3058 | −0.0198 | -0.2972 | |
| SD | 0.3874 | 0.2762 | 0.2336 | 0.3134 | 0.1696 | 0.1785 | 0.1516 | 0.1837 | 0.1897 | 0.5492 | 0.5434 | 0.4623 | 0.3884 | 0.3693 | 0.3681 | 0.4019 | 0.1990 | 0.4593 | ||
| ASD | 0.9868 | 0.2649 | 0.3477 | 0.2615 | 0.2322 | 0.1526 | 0.1204 | 0.1602 | – | 0.5655 | 0.4292 | 0.3356 | 0.2399 | 0.1469 | 0.3580 | 0.2790 | 0.1928 | – | ||
| 600 | Bias | 0.0744 | 0.0221 | 0.0321 | −0.0030 | 0.0076 | 0.0197 | 0.0061 | −0.0095 | 0.0157 | −0.2257 | 0.3067 | 0.2134 | 0.1114 | 0.0139 | 0.1650 | 0.3656 | −0.0168 | −0.3080 | |
| SD | 0.3170 | 0.1761 | 0.2035 | 0.2539 | 0.1337 | 0.0953 | 0.0971 | 0.1008 | 0.1807 | 0.3958 | 0.3814 | 0.4269 | 0.3802 | 0.2285 | 0.2714 | 0.3876 | 0.1416 | 0.3693 | ||
| ASD | 0.7293 | 0.2212 | 0.1850 | 0.2129 | 0.1535 | 0.0796 | 0.0887 | 0.1002 | – | 0.5430 | 0.4499 | 0.3654 | 0.2585 | 0.1619 | 0.2369 | 0.2451 | 0.1423 | – | ||
| 900 | Bias | 0.0520 | 0.0206 | 0.0228 | −0.0016 | 0.0017 | 0.0129 | 0.0004 | −0.0094 | 0.0061 | −0.2230 | 0.2876 | 0.2341 | 0.1072 | 0.0171 | 0.1515 | 0.3606 | −0.0065 | −0.2269 | |
| SD | 0.2963 | 0.1592 | 0.1857 | 0.1934 | 0.1109 | 0.0695 | 0.0752 | 0.0756 | 0.1606 | 0.3703 | 0.3629 | 0.3925 | 0.2976 | 0.1730 | 0.1937 | 0.3382 | 0.1224 | 0.3537 | ||
| ASD | 0.6693 | 0.1832 | 0.1587 | 0.1825 | 0.0965 | 0.0558 | 0.0732 | 0.0921 | – | 0.5329 | 0.3752 | 0.3568 | 0.2486 | 0.1112 | 0.1798 | 0.2185 | 0.1162 | – | ||
| Parameters | r | r | ||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| n | True Value | 0.7 | 0.2 | 0.1 | 0.5 | 0.3 | 0.3 | 0.5 | 0.1 | 0.2 | 0.6 | 0 | 0.7 | 0.2 | 0.1 | 0.5 | 0.3 | 0.3 | 0.5 | 0.1 | 0.2 | 0.6 | 0 | |
| QMELE | Standard QMLE | |||||||||||||||||||||||
| 300 | Bias | 0.0359 | −0.0137 | 0.0481 | −0.0328 | 0.0155 | −0.0096 | 0.0320 | 0.0422 | −0.0195 | −0.0178 | −0.0383 | −0.0959 | −0.0917 | 0.1259 | 0.2274 | 0.0363 | −0.0223 | −0.0126 | 0.1800 | 0.0783 | 0.0138 | −0.2076 | |
| SD | 0.5409 | 0.5306 | 0.0847 | 0.2338 | 0.1485 | 0.2010 | 0.3838 | 0.1125 | 0.0926 | 0.1354 | 0.3876 | 0.2759 | 0.2987 | 0.1185 | 0.2273 | 0.1475 | 0.1171 | 0.2492 | 0.1677 | 0.1213 | 0.1296 | 0.3679 | ||
| ASD | 1.4229 | 1.1241 | 0.1953 | 0.1500 | 0.2043 | 0.1847 | 0.5038 | 0.0768 | 0.0908 | 0.1192 | – | 0.3105 | 0.3780 | 0.3915 | 0.2390 | 0.2067 | 0.0621 | 0.1651 | 0.1051 | 0.1172 | 0.1092 | – | ||
| 600 | Bias | 0.0198 | −0.0096 | 0.0458 | −0.0116 | 0.0102 | −0.0006 | 0.0146 | 0.0295 | −0.0191 | −0.0035 | −0.0181 | −0.1068 | −0.0656 | 0.1197 | 0.2145 | 0.0495 | −0.0100 | −0.0167 | 0.1522 | 0.0097 | 0.0237 | −0.1926 | |
| SD | 0.2824 | 0.3030 | 0.0940 | 0.1476 | 0.1451 | 0.1057 | 0.2527 | 0.0700 | 0.0667 | 0.0818 | 0.3152 | 0.1887 | 0.2099 | 0.0820 | 0.1782 | 0.1074 | 0.0769 | 0.1600 | 0.1049 | 0.0972 | 0.0845 | 0.2622 | ||
| ASD | 0.5658 | 0.5857 | 0.0770 | 0.1148 | 0.1335 | 0.1799 | 0.3272 | 0.0526 | 0.0638 | 0.0826 | − | 0.2092 | 0.2739 | 0.1385 | 0.1560 | 0.1255 | 0.0389 | 0.1155 | 0.0706 | 0.0833 | 0.0748 | − | ||
| 900 | Bias | 0.0046 | −0.0109 | 0.0426 | −0.0187 | 0.0185 | 0.0059 | 0.0153 | 0.0198 | −0.0166 | 0.0019 | 0.0009 | −0.1023 | −0.0685 | 0.1242 | 0.2012 | 0.0554 | −0.0090 | −0.0188 | 0.1448 | 0.0912 | 0.0307 | −0.1842 | |
| SD | 0.3039 | 0.2515 | 0.0648 | 0.1971 | 0.1064 | 0.1343 | 0.2373 | 0.0542 | 0.0551 | 0.0674 | 0.2467 | 0.1693 | 0.1691 | 0.0824 | 0.1452 | 0.1005 | 0.0593 | 0.1332 | 0.0848 | 0.0834 | 0.0676 | 0.2431 | ||
| ASD | 0.6637 | 0.5310 | 0.0552 | 0.0906 | 0.0916 | 0.2017 | 0.2595 | 0.0421 | 0.0524 | 0.0671 | − | 0.1756 | 0.1883 | 0.1045 | 0.1291 | 0.1011 | 0.0329 | 0.0982 | 0.0574 | 0.0681 | 0.0608 | − | ||
| 300 | Bias | 0.0313 | −0.0120 | 0.0486 | 0.0056 | 0.0191 | 0.0014 | 0.0238 | 0.0368 | −0.0076 | −0.0164 | −0.0621 | −0.1206 | −0.1771 | 0.1924 | 0.4032 | 0.0585 | 0.0047 | −0.0641 | 0.2774 | 0.1512 | 0.0214 | −0.3335 | |
| SD | 0.3193 | 0.3897 | 0.1095 | 0.2640 | 0.2000 | 0.1172 | 0.2773 | 0.1165 | 0.1037 | 0.1224 | 0.4687 | 0.4313 | 0.4547 | 0.2527 | 0.4925 | 0.2187 | 0.1716 | 0.3933 | 0.2575 | 0.1904 | 0.1513 | 0.5577 | ||
| ASD | 1.3226 | 1.5866 | 0.3796 | 0.2624 | 0.3878 | 0.1525 | 0.4386 | 0.0834 | 0.1069 | 0.1185 | − | 1.1642 | 1.1136 | 1.2507 | 0.5134 | 0.4134 | 0.1286 | 0.3395 | 0.1632 | 0.1804 | 0.1230 | − | ||
| 600 | Bias | 0.0017 | −0.0226 | 0.0514 | 0.0135 | 0.0135 | 0.0002 | 0.0154 | 0.0266 | -0.0064 | -0.0123 | −0.0289 | −0.1515 | −0.1625 | 0.1936 | 0.3808 | 0.0636 | 0.0094 | −0.0631 | 0.2444 | 0.1841 | 0.0100 | 0.4300 | |
| SD | 0.3227 | 0.3890 | 0.1052 | 0.2181 | 0.1335 | 0.0716 | 0.2301 | 0.0723 | 0.0705 | 0.0805 | 0.3505 | 0.3311 | 0.3739 | 0.1699 | 0.3582 | 0.2084 | 0.1188 | 0.3481 | 0.1662 | 0.1664 | 0.0978 | 0.4905 | ||
| ASD | 0.8516 | 0.9170 | 0.1992 | 0.1811 | 0.2317 | 0.0831 | 0.2878 | 0.0570 | 0.0753 | 0.0816 | − | 0.6877 | 0.9354 | 1.0392 | 0.4891 | 0.6041 | 0.1211 | 0.2533 | 0.1125 | 0.1332 | 0.0848 | − | ||
| 900 | Bias | 0.0030 | −0.0069 | 0.0504 | 0.0061 | 0.0173 | 0.0056 | 0.0215 | 0.0192 | −0.0101 | −0.0014 | −0.0393 | −0.1409 | −0.1552 | 0.1997 | 0.3613 | 0.0740 | 0.0146 | −0.0530 | 0.2273 | 0.1980 | 0.0318 | −0.3594 | |
| SD | 0.2428 | 0.2748 | 0.0846 | 0.1918 | 0.1305 | 0.0869 | 0.1885 | 0.0550 | 0.0593 | 0.0623 | 0.3283 | 0.3440 | 0.3244 | 0.1704 | 0.2986 | 0.1644 | 0.1552 | 0.3282 | 0.1290 | 0.1459 | 0.0834 | 0.4708 | ||
| ASD | 0.5947 | 0.6394 | 0.1459 | 0.1683 | 0.1894 | 0.1004 | 0.2974 | 0.0452 | 0.0592 | 0.0651 | – | 0.8345 | 1.0395 | 0.8602 | 0.3704 | 0.2656 | 0.0864 | 0.2290 | 0.0915 | 0.1107 | 0.0691 | – | ||
| 300 | Bias | 0.0433 | −0.0023 | 0.0626 | 0.0229 | 0.0030 | 0.0154 | 0.0112 | 0.0438 | −0.0093 | −0.0229 | −0.0537 | −0.1922 | −0.2268 | 0.2413 | 0.4413 | 0.0825 | 0.0270 | −0.0813 | 0.3328 | 0.1905 | 0.0208 | −0.3948 | |
| SD | 0.3766 | 0.3797 | 0.1479 | 0.3230 | 0.2228 | 0.2949 | 0.3053 | 0.1284 | 0.1131 | 0.1362 | 0.4103 | 0.5678 | 0.6324 | 0.3059 | 0.6310 | 0.2967 | 0.2549 | 0.5479 | 0.3934 | 0.3245 | 0.1924 | 0.7518 | ||
| ASD | 1.6808 | 1.3841 | 0.3948 | 0.3133 | 0.4711 | 0.1528 | 0.4242 | 0.0921 | 0.1236 | 0.1341 | – | 2.1121 | 3.5392 | 4.8341 | 1.2046 | 0.6966 | 0.3020 | 0.7381 | 0.2889 | 0.3147 | 0.1813 | – | ||
| 600 | Bias | 0.0107 | −0.0194 | 0.0550 | 0.0115 | 0.0276 | 0.0079 | −0.0195 | 0.0282 | −0.0095 | -0.0107 | -0.0467 | -0.1745 | -0.2191 | 0.2423 | 0.4505 | 0.0855 | 0.0376 | -0.1391 | 0.3091 | 0.2309 | 0.0266 | -0.4178 | |
| SD | 0.3062 | 0.5962 | 0.1808 | 1.3040 | 0.1933 | 0.1042 | 0.4318 | 0.0865 | 0.0850 | 0.0948 | 0.4613 | 0.4070 | 0.5125 | 0.3649 | 0.6025 | 0.2615 | 0.2436 | 0.5146 | 0.3219 | 0.2968 | 0.1420 | 0.6121 | ||
| ASD | 0.7485 | 0.8655 | 0.3999 | 0.2782 | 0.2164 | 0.1242 | 0.4406 | 0.0638 | 0.0879 | 0.0947 | – | 1.9790 | 2.8621 | 4.0155 | 1.3184 | 0.7723 | 0.5350 | 0.8205 | 0.2876 | 0.2960 | 0.1757 | – | ||
| 900 | Bias | 0.0111 | -0.0158 | 0.0544 | 0.0121 | 0.0280 | 0.0124 | 0.0082 | 0.0208 | -0.0076 | -0.0082 | -0.0466 | -0.1752 | -0.1976 | 0.2217 | 0.4025 | 0.0938 | 0.0281 | -0.1299 | 0.3051 | 0.2509 | 0.0400 | -0.3946 | |
| SD | 0.3003 | 0.3518 | 0.1512 | 0.2689 | 0.1855 | 0.1521 | 0.2643 | 0.0634 | 0.0707 | 0.0719 | 0.3832 | 0.3332 | 0.4359 | 0.2450 | 0.4416 | 0.2084 | 0.1844 | 0.4231 | 0.3072 | 0.2867 | 0.1272 | 0.5482 | ||
| ASD | 0.8089 | 0.8741 | 0.1964 | 0.2315 | 0.1794 | 0.1236 | 0.3430 | 0.0511 | 0.0719 | 0.0767 | − | 1.4053 | 1.8931 | 4.5213 | 0.9127 | 0.5223 | 0.2029 | 0.4696 | 0.1930 | 0.2297 | 0.1177 | − | ||
| TVCDT-GARCH | DT-GARCH | |
|---|---|---|
| AIC | 1922.522 | 1925.186 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Fu, L.; Wang, D.; Yu, Z. A Time-Varying Coefficient Double Threshold GARCH Model with Explanatory Variables. Axioms 2023, 12, 476. https://doi.org/10.3390/axioms12050476
Zhang T, Fu L, Wang D, Yu Z. A Time-Varying Coefficient Double Threshold GARCH Model with Explanatory Variables. Axioms. 2023; 12(5):476. https://doi.org/10.3390/axioms12050476
Chicago/Turabian StyleZhang, Tongwei, Lianyan Fu, Dehui Wang, and Zhuoxi Yu. 2023. "A Time-Varying Coefficient Double Threshold GARCH Model with Explanatory Variables" Axioms 12, no. 5: 476. https://doi.org/10.3390/axioms12050476
APA StyleZhang, T., Fu, L., Wang, D., & Yu, Z. (2023). A Time-Varying Coefficient Double Threshold GARCH Model with Explanatory Variables. Axioms, 12(5), 476. https://doi.org/10.3390/axioms12050476






