An Analytic Solution for 2D Heat Conduction Problems with General Dirichlet Boundary Conditions
Abstract
1. Introduction
- (1)
- Lee and colleagues [10,11,12,13,14,15,16] used the shifting function method to derive an analytic solution for the heat conduction with time-dependent boundary conditions. They also performed an inverse estimation of a heat treatment problem with unknown time-dependent boundary conditions. However, their research is limited to the scope of one-dimensional heat conduction problems. The greatest contribution of this work is the first investigation of the analytic solution to 2D heat conduction problems with the general Dirichlet boundary conditions by using the proposed method, combining the shifting function method with the expansion theorem method. The applicability of the present method is in solving the heat conduction problems of a rectangular cross-section of an infinite rod with specified space–time-dependent dependent boundary conditions at the four edges of the rectangular region;
- (2)
- Some advanced heat conduction books [17,18,19] proposed some classical techniques such as the Laplace transform, Duhamel’s theorem, and Green’s function to solve the heat conduction problem. However, they are limited to the integration situation during the solution process. The correctness of the solution in this study is verified by comparing it with the results of Young et al. [27]. To the best of the authors’ knowledge, the other cases in this paper have never been presented in past studies. Although the number of series expansion terms determines the accuracy of the solution, the case study shows that the proposed method has good convergence to the solution using series expansion and can quickly reach a convergence value. The influence of the parameters of the time-dependent boundary function on the temperature variation is also studied.
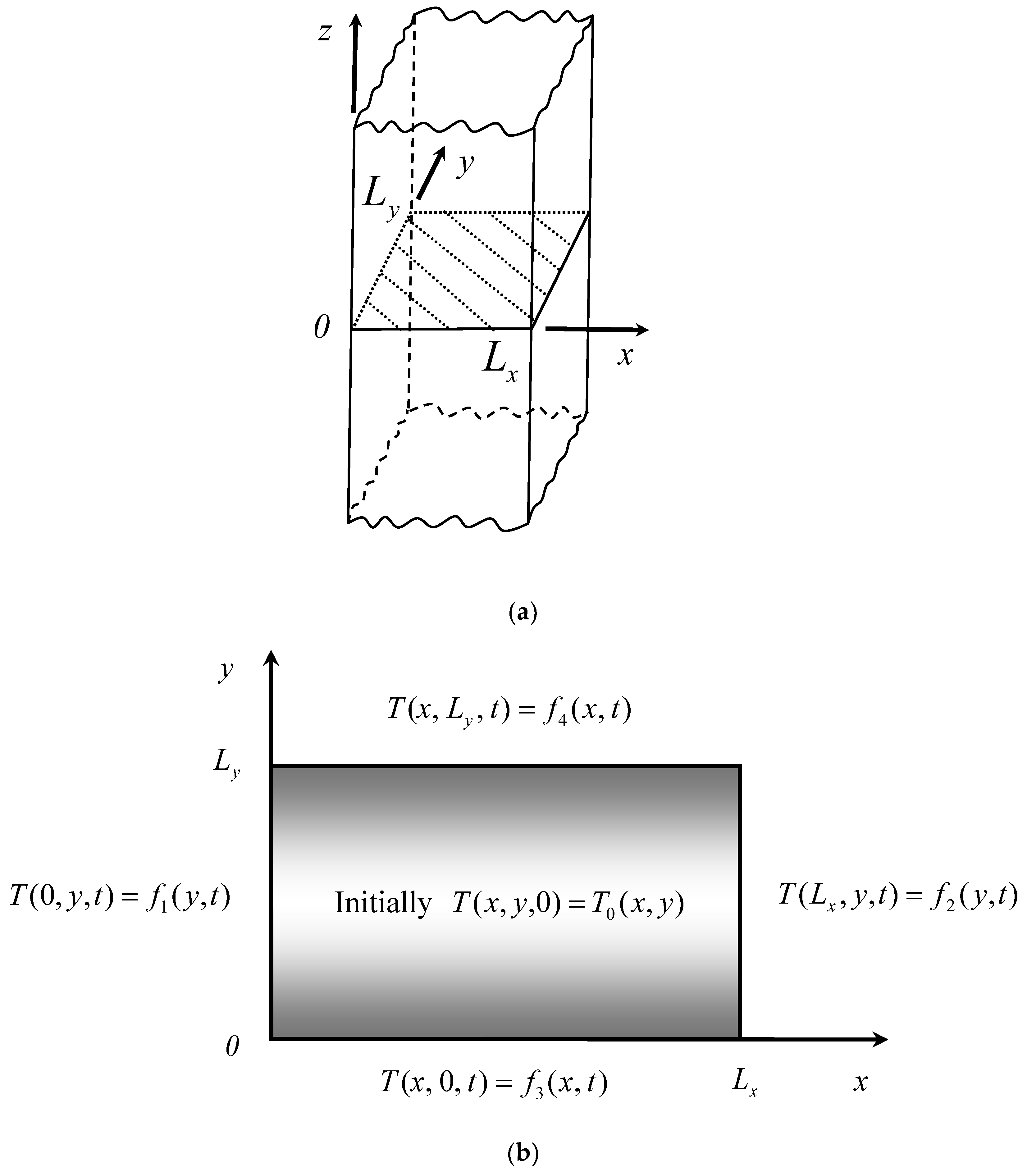
2. Mathematical Modeling
3. The Solution Methodology
3.1. The Dimensionless Form of Physical System
3.2. Principle of Superposition
3.3. Reduced to One-Dimensional Problem
3.4. The Shifting Function Method
3.4.1. Change of Variable
3.4.2. The Shifting Functions
3.4.3. The Eigenfunction Expansion Theorem
3.5. The Analytic Solution
4. Examples and Verification
4.1. The Space-Dependent Boundary Conditions of Periodical Type
4.2. The Space-Dependent Boundary Conditions of Parabolic Type
5. Conclusions
- (1)
- The proposed approach combining the shifting function method and the expansion theorem method can derive an analytic solution for the 2D heat conduction in a rectangular cross-section of an infinite bar with the general Dirichlet boundary conditions specifying space–time-dependent boundary conditions at the four edges of the rectangular region;
- (2)
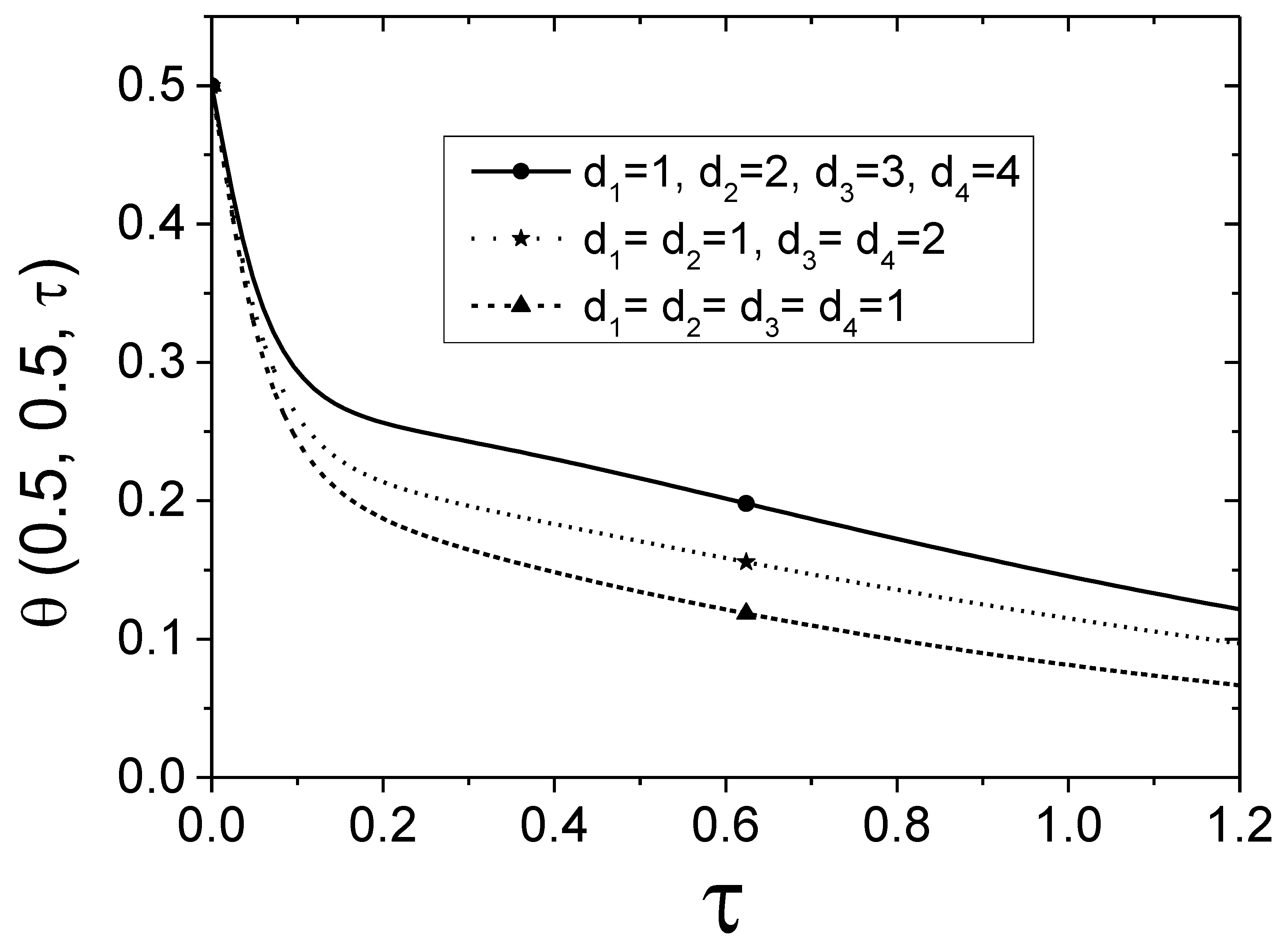
- The series expansion derived from the proposed method has a good convergence to reach the convergence values. For space-dependent boundary with the parabolic-type case, one can take five terms of the series to obtain the series solutions within 1% error;
- (3)
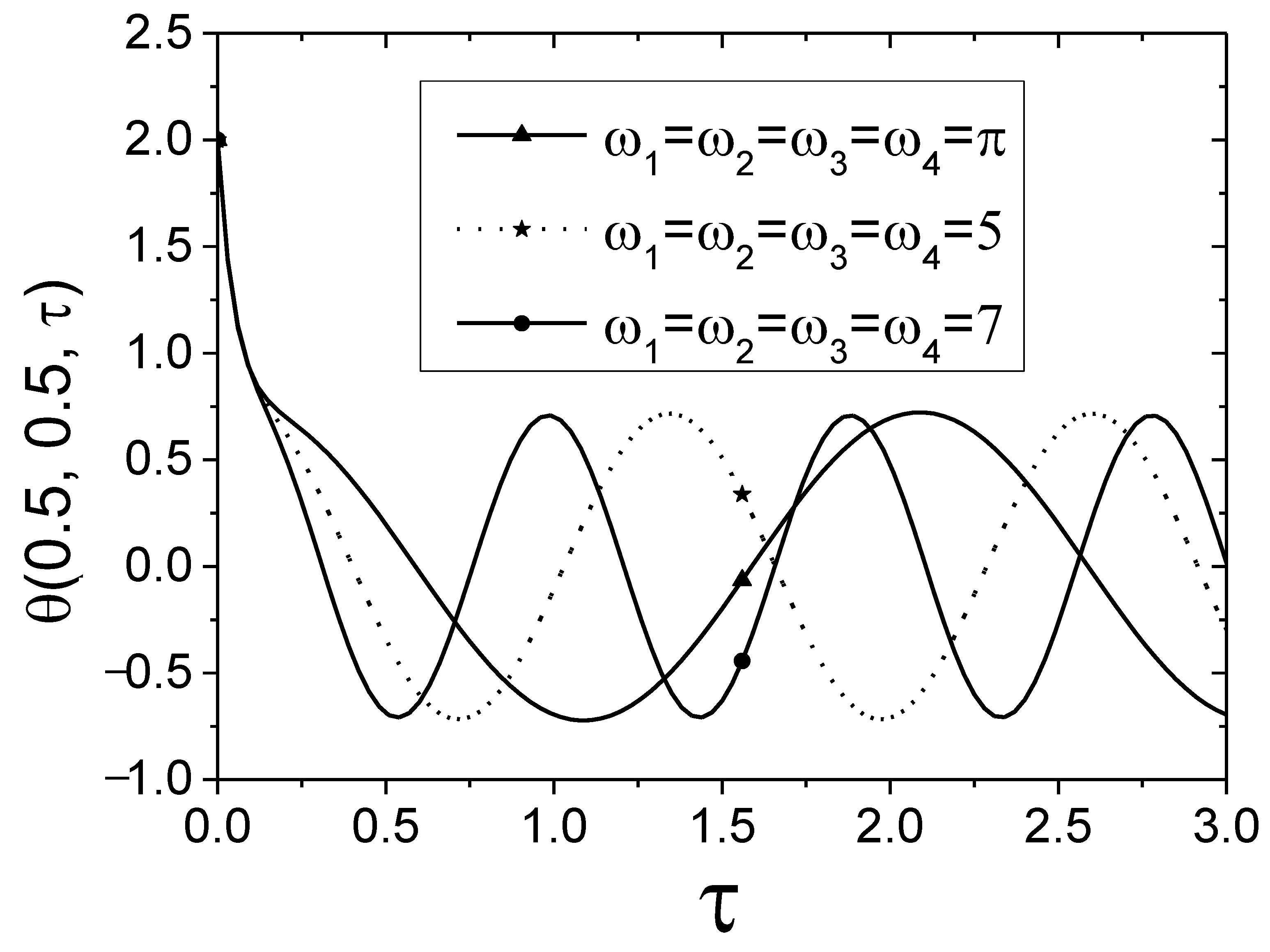
- When considering the time-dependent boundary of harmonic function, the fluctuation of the temperature variation increases as the frequency of the harmonic function increases. When considering the time-dependent boundary of exponential function, , a smaller coefficient will result in a lower and faster drop in temperature.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| two subsystems | |
| specific heat (°C) | |
| four arbitrary constants | |
| temperatures along the surface at the left end and the right end of the rectangular region | |
| temperatures along the surface at the bottom end and the top end of the rectangular region | |
| dimensionless quantity defined in Equation (8) | |
| dimensionless quantity defined in Equation (8) | |
| dimensionless quantity defined in Equation (31) | |
| dimensionless quantity defined in Equation (A7) | |
| shifting function | |
| shifting function | |
| nonhomogeneous term in the differential equation of the transformed system defined in Equation (43) | |
| thermal conductivity (°C) | |
| aspect ratio, defined in Equation (8) | |
| thickness of the two-dimensional rectangular region at x- and y- directions (m) | |
| temperature function (°C) | |
| dimensionless time variable of the transformed function defined in Equations (53) and (A22) | |
| reference temperature (°C) | |
| initial temperature (°C) | |
| time variable (s) | |
| space variable in x-direction of a rectangular region (m) | |
| dimensionless space variable in x-direction of a rectangular region | |
| space variable in y-direction of a rectangular region (m) | |
| dimensionless space variable in y-direction of a rectangular region | |
| thermal diffusivity () | |
| auxiliary integration variable | |
| dimensionless quantity defined in Equations (55) and (A24) | |
| time-dependent boundary condition | |
| n-th eigenvalues depend on defined in Equations (54) and (A23) | |
| dimensionless temperature | |
| dimensionless initial temperature | |
| dimensionless temperatures for subsystems A and B | |
| generalized Fourier coefficient defined in Equation (29) | |
| transformed function defined in Equation (36) | |
| n-th eigenfunction of the transformed function defined in Equation (47) | |
| density () | |
| dimensionless time | |
| n-th eigenvalue for Sturm–Liouville problem defined in Equation (48). | |
| Subscripts | |
| described in the article |
Appendix A. Analytic Solution of the Subsystem B
References
- Perakis, N.; Haidna, O.J.; Ihme, M. Heat transfer augmentation by recombination reactions in turbulent reacting boundary layers at elevated pressures. Int. J. Heat Mass Transf. 2021, 178, 121628. [Google Scholar] [CrossRef]
- Hassan, M.A.S.M.; Razlan, Z.M.; Bakar, S.A.; Rahman, A.A.; Rojan, M.A.; Wan, W.K.; Ibrahim, Z.; Ishak, A.A.; Ridzuan, M.J.M. Derivation and validation of heat transfer model for Spark-Ignition engine cylinder head. Appl. Therm. Eng. 2023, 225, 120240. [Google Scholar] [CrossRef]
- Ivanov, V.; Salomatov, V. On the calculation of the temperature field in solids with variable heat-transfer coefficients. J. Eng. Phys. Thermophys. 1965, 72, 63–64. [Google Scholar] [CrossRef]
- Ivanov, V.; Salomatov, V. Unsteady temperature field in solid bodies with variable heat transfer coefficient. J. Eng. Phys. Thermophys. 1966, 11, 151–152. [Google Scholar] [CrossRef]
- Postol’Nik, Y.S. One-dimensional convective heating with a time-dependent heat-transfer coefficient. J. Eng. Phys. Thermophys. 1970, 18, 233–238. [Google Scholar] [CrossRef]
- Kozlov, V. Solution of heat-conduction problem with variable heat-exchange coefficient. J. Eng. Phys. Thermophys. 1970, 18, 100–104. [Google Scholar] [CrossRef]
- Holy, Z. Temperature and stresses in reactor fuel elements due to time-and space-dependent heat-transfer coefficients. Nucl. Eng. Des. 1972, 18, 145–197. [Google Scholar] [CrossRef][Green Version]
- Özişik, M.N.; Murray, R. On the solution of linear diffusion problems with variable boundary condition parameters. J. Heat Transf. 1974, 96, 48–51. [Google Scholar] [CrossRef]
- Moitsheki, R.J. Transient heat diffusion with temperature-dependent conductivity and time-dependent heat transfer coefficient. Math. Probl. Eng. 2008, 9, 41–58. [Google Scholar] [CrossRef][Green Version]
- Chen, H.T.; Sun, S.L.; Huang, H.C.; Lee, S.Y. Analytic closed solution for the heat conduction with time dependent heat convection coefficient at one boundary. Comput. Model. Eng. Sci. 2010, 59, 107–126. [Google Scholar]
- Lee, S.Y.; Huang, C.C. Analytic solutions for heat conduction in functionally graded circular hollow cylinders with time-dependent boundary conditions. Math. Probl. Eng. 2013, 5, 816385. [Google Scholar] [CrossRef][Green Version]
- Lee, S.Y.; Tu, T.W. Unsteady temperature field in slabs with different kinds of time-dependent boundary conditions. Acta Mech. 2015, 226, 3597–3609. [Google Scholar] [CrossRef]
- Lee, S.Y.; Lin, S.M. Dynamic analysis of nonuniform beams with time-dependent elastic boundary conditions. J. Appl. Mech. 1996, 63, 474–478. [Google Scholar] [CrossRef][Green Version]
- Lee, S.Y.; Huang, T.W. A method for inverse analysis of laser surface heating with experimental data. Int. J. Heat Mass Transf. 2014, 72, 299–307. [Google Scholar] [CrossRef]
- Lee, S.Y.; Huang, T.W. Inverse analysis of spray cooling on a hot surface with experimental data. Int. J. Therm. Sci. 2016, 100, 145–154. [Google Scholar] [CrossRef]
- Lee, S.Y.; Yan, Q.Z. Inverse analysis of heat conduction problems with relatively long heat treatment. Int. J. Heat Mass Transf. 2017, 105, 401–410. [Google Scholar] [CrossRef]
- Carslaw, H.; Jaeger, J. Heat in Solids, 2nd ed.; Clarendon Press: Oxford, UK, 1959. [Google Scholar]
- Özışık, M.N. Heat Conduction; John Wiley & Sons: New York, USA, 1993. [Google Scholar]
- Cole, K.D.; Beck, J.V.; Haji-Sheikh, A.; Litkouhi, B. Heat Conduction Using Green’s Functions; Taylor & Francis: Boca Raton, FL, USA, 2010. [Google Scholar]
- Zhu, S.P. Solving transient diffusion problems: Time-dependent fundamental solution approaches versus LTDRM approaches. Eng. Anal. Bound. Elem. 1998, 21, 87–90. [Google Scholar] [CrossRef]
- Zhu, S.P.; Liu, H.W.; Lu, X.P. A combination of LTDRM and ATPS in solving diffusion problems. Eng. Anal. Bound. Elem. 1998, 21, 285–289. [Google Scholar] [CrossRef]
- Sutradhar, A.; Paulino, G.H.; Gray, L. Transient heat conduction in homogeneous and non-homogeneous materials by the Laplace transform Galerkin boundary element method. Eng. Anal. Bound. Elem. 2002, 26, 119–132. [Google Scholar] [CrossRef]
- Bulgakov, V.; Šarler, B.; Kuhn, G. Iterative solution of systems of equations in the dual reciprocity boundary element method for the diffusion equation. Int. J. Numer. Methods Eng. 1998, 43, 713–732. [Google Scholar] [CrossRef]
- Walker, S. Diffusion problems using transient discrete source superposition. Int. J. Numer. Methods Eng. 1992, 35, 165–178. [Google Scholar] [CrossRef]
- Chen, C.; Golberg, M.; Hon, Y. The method of fundamental solutions and quasi-Monte-Carlo method for diffusion equations. Int. J. Numer. Methods Eng. 1998, 43, 1421–1435. [Google Scholar] [CrossRef]
- Burgess, G.; Mahajerin, E. Transient heat flow analysis using the fundamental collocation method. Appl. Therm. Eng. 2003, 23, 893–904. [Google Scholar] [CrossRef]
- Young, D.; Tsai, C.; Murugesan, K.; Fan, C.; Chen, C. Time-dependent fundamental solutions for homogeneous diffusion problems. Eng. Anal. Bound. Elem. 2004, 28, 1463–1473. [Google Scholar] [CrossRef]
- Cole, K.D.; Yen, D.H. Green’s functions, temperature and heat flux in the rectangle. Int. J. Heat Mass Transf. 2001, 44, 3883–3894. [Google Scholar] [CrossRef]
- Beck, J.V.; Wright, N.T.; Haji-Sheikh, A. Transient power variation in surface conditions in heat conduction for plates. Int. J. Heat Mass Transf. 2008, 51, 2553–2565. [Google Scholar] [CrossRef]
- Lei, J.; Wang, Q.; Liu, X.; Gu, Y.; Fan, C.-M. A novel space-time generalized FDM for transient heat conduction problems. Eng. Anal. Bound. Elem. 2020, 119, 1–12. [Google Scholar] [CrossRef]
- Alam, M.N.; Tunç, C. New solitary wave structures to the (2 + 1)-dimensional KD and KP equations with spatio-temporal dispersion. J. King Saud Univ. Sci. 2020, 32, 3400–3409. [Google Scholar] [CrossRef]
- Islam, S.; Alam, M.N.; Fayz-Al-Asad, M.; Tunç, C. An analytical technique for solving new computational solutions of the modified Zakharov-Kuznetsov equation arising in electrical engineering. J. Appl. Comput. Mech. 2021, 7, 715–726. [Google Scholar]
- Krishnan, G.; Parhizi, M.; Jain, A. Eigenfunction-based solution for solid-liquid phase change heat transfer problems with time-dependent boundary conditions. Int. J. Heat Mass Transf. 2022, 189, 122693. [Google Scholar] [CrossRef]
- Belekar, V.V.; Murphy, E.J.; Subramaniam, S. Analytical solution to heat transfer in stationary wet granular mixtures with time-varying boundary conditions. Int. Commun. Heat Mass Transf. 2023, 140, 106500. [Google Scholar] [CrossRef]

| 1 | 3 | 5 | 10 | 20 | |
|---|---|---|---|---|---|
| 0 | 0.516 | 0.497 | 0.501 | 0.500 | 0.500 |
| 0.1 | 0.229 | 0.246 | 0.243 | 0.243 | 0.243 |
| 0.2 | 0.174 | 0.189 | 0.187 | 0.187 | 0.187 |
| 0.4 | 0.138 | 0.150 | 0.148 | 0.148 | 0.148 |
| 0.6 | 0.113 | 0.123 | 0.121 | 0.121 | 0.121 |
| 0.8 | 0.0921 | 0.100 | 0.0989 | 0.0994 | 0.0994 |
| 1.0 | 0.0754 | 0.0823 | 0.0810 | 0.0814 | 0.0814 |
| 1.2 | 0.0618 | 0.0674 | 0.0663 | 0.0666 | 0.0666 |
| 1 | 3 | 5 | 10 | 20 | |
|---|---|---|---|---|---|
| 0 | 0.516 | 0.497 | 0.501 | 0.500 | 0.500 |
| 0.1 | 0.249 | 0.263 | 0.261 | 0.261 | 0.261 |
| 0.2 | 0.203 | 0.215 | 0.213 | 0.213 | 0.213 |
| 0.4 | 0.176 | 0.184 | 0.183 | 0.183 | 0.183 |
| 0.6 | 0.154 | 0.159 | 0.159 | 0.159 | 0.159 |
| 0.8 | 0.133 | 0.136 | 0.136 | 0.136 | 0.136 |
| 1.0 | 0.113 | 0.115 | 0.115 | 0.115 | 0.115 |
| 1.2 | 0.0954 | 0.0969 | 0.0967 | 0.0968 | 0.0968 |
| 1 | 3 | 5 | 10 | 20 | |
|---|---|---|---|---|---|
| 0 | 0.516 | 0.497 | 0.501 | 0.500 | 0.500 |
| 0.1 | 0.285 | 0.295 | 0.293 | 0.293 | 0.293 |
| 0.2 | 0.251 | 0.257 | 0.256 | 0.256 | 0.256 |
| 0.4 | 0.229 | 0.230 | 0.230 | 0.230 | 0.230 |
| 0.6 | 0.202 | 0.201 | 0.202 | 0.202 | 0.202 |
| 0.8 | 0.174 | 0.172 | 0.172 | 0.172 | 0.172 |
| 1.0 | 0.147 | 0.145 | 0.146 | 0.146 | 0.146 |
| 1.2 | 0.123 | 0.121 | 0.122 | 0.122 | 0.122 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hsu, H.-P.; Tu, T.-W.; Chang, J.-R. An Analytic Solution for 2D Heat Conduction Problems with General Dirichlet Boundary Conditions. Axioms 2023, 12, 416. https://doi.org/10.3390/axioms12050416
Hsu H-P, Tu T-W, Chang J-R. An Analytic Solution for 2D Heat Conduction Problems with General Dirichlet Boundary Conditions. Axioms. 2023; 12(5):416. https://doi.org/10.3390/axioms12050416
Chicago/Turabian StyleHsu, Heng-Pin, Te-Wen Tu, and Jer-Rong Chang. 2023. "An Analytic Solution for 2D Heat Conduction Problems with General Dirichlet Boundary Conditions" Axioms 12, no. 5: 416. https://doi.org/10.3390/axioms12050416
APA StyleHsu, H.-P., Tu, T.-W., & Chang, J.-R. (2023). An Analytic Solution for 2D Heat Conduction Problems with General Dirichlet Boundary Conditions. Axioms, 12(5), 416. https://doi.org/10.3390/axioms12050416







