Schröder-Based Inverse Function Approximation
Abstract
:1. Introduction
1.1. Background Result
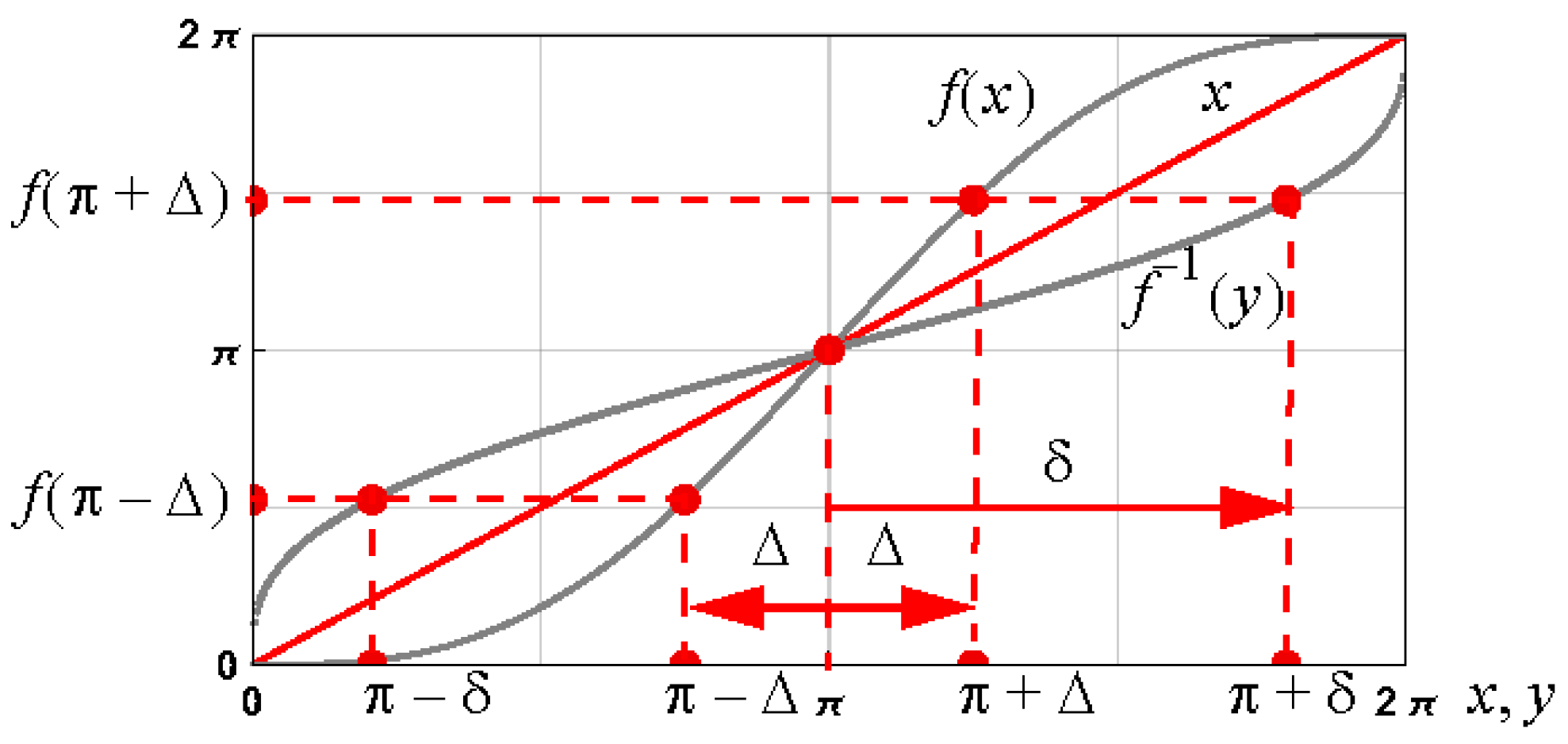
1.2. Assumptions and Notation
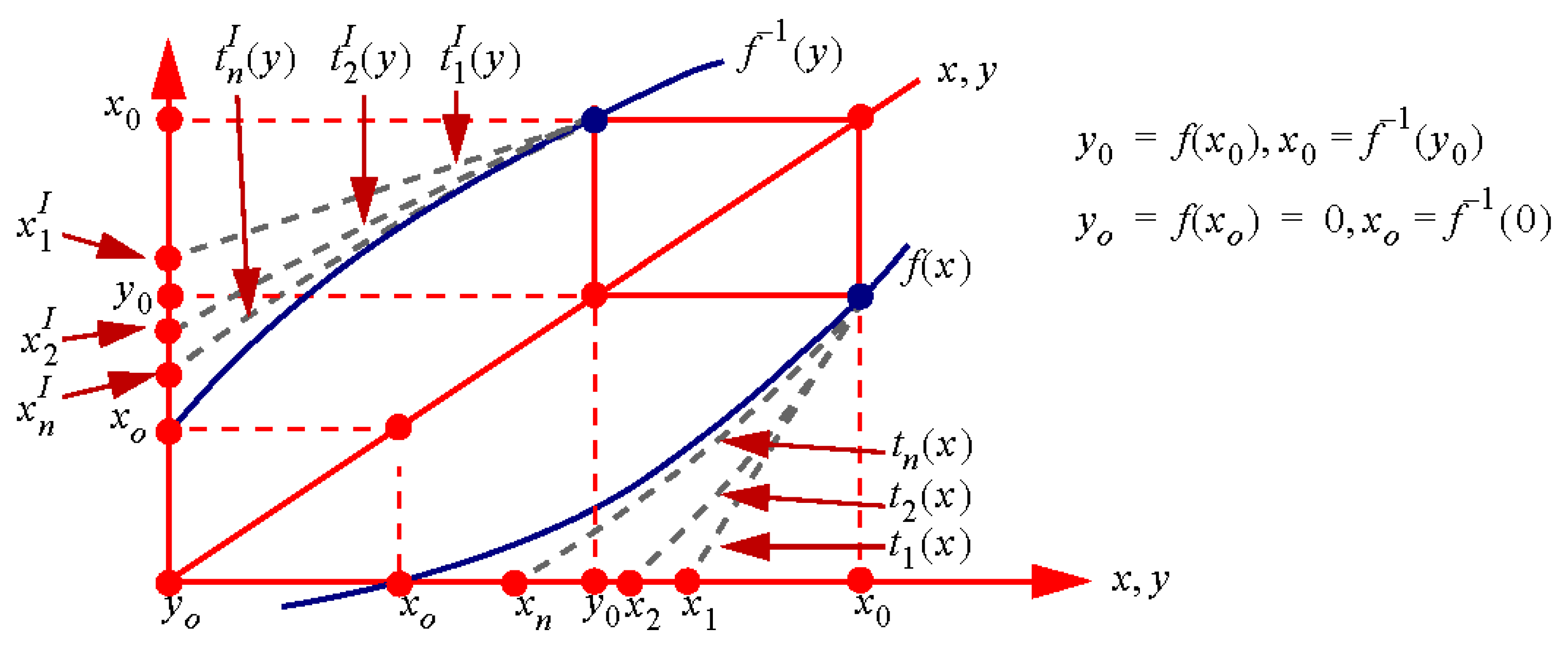
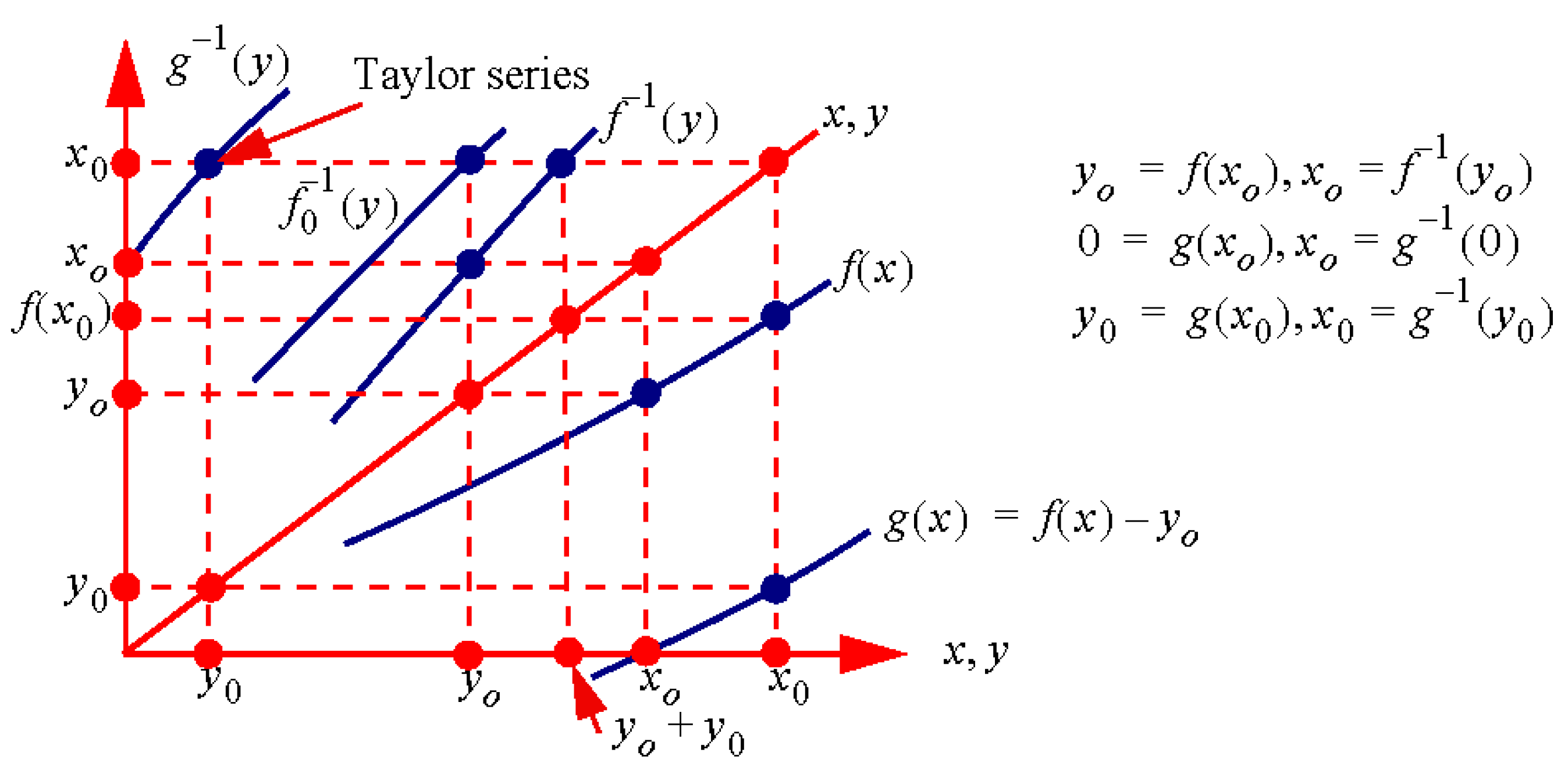
2. Schröder’s Approximations of the First Kind
2.1. Schröder’s Approximations of the First Kind
Notes
2.2. Inverse Function Approximation
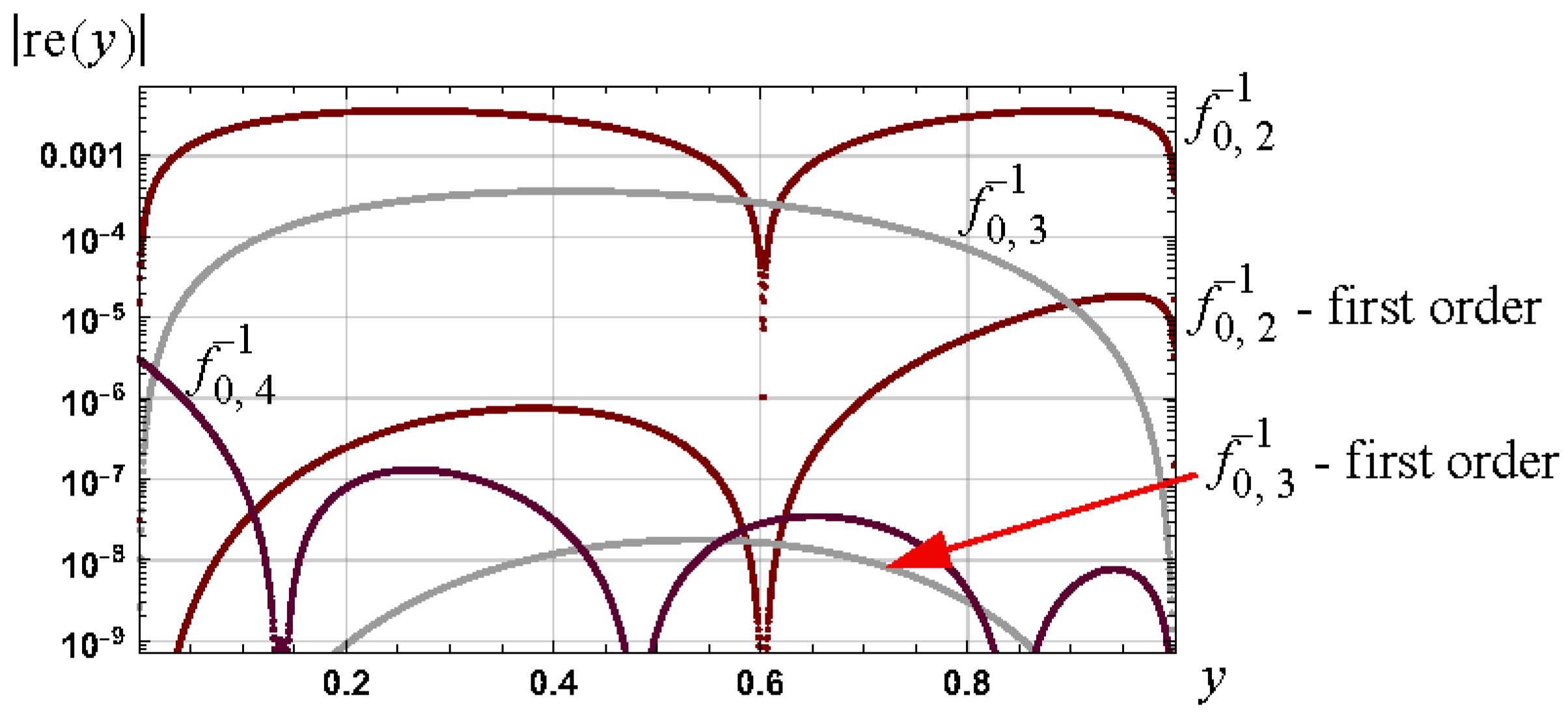
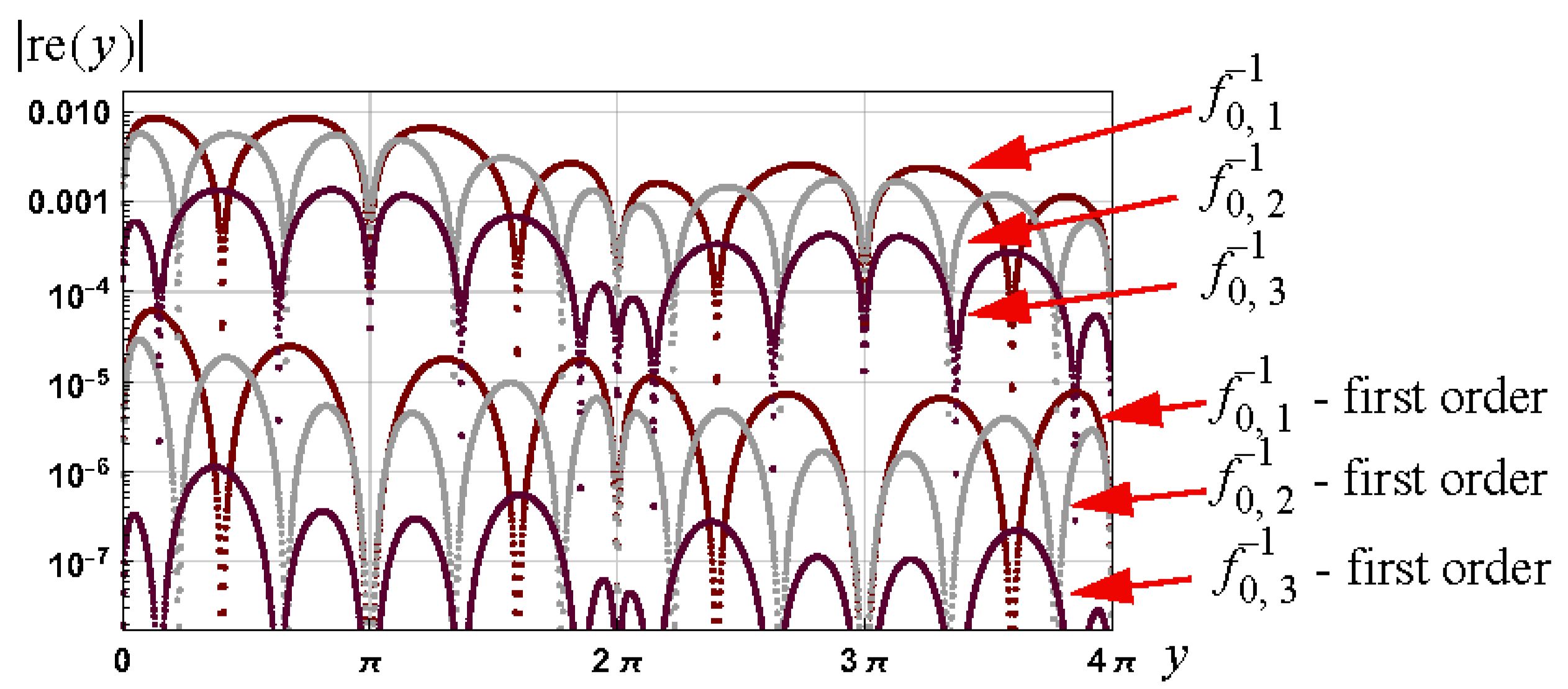
2.3. Notes
2.4. Notes on Convergence
2.4.1. Convergence of Schröder Approximations
2.4.2. Relative Error Bound for First-Order Approximation
2.5. Special Case: Ratio of Two Functions
Approximations for the Inverse of
2.6. Newton–Raphson Iteration
2.7. Notes
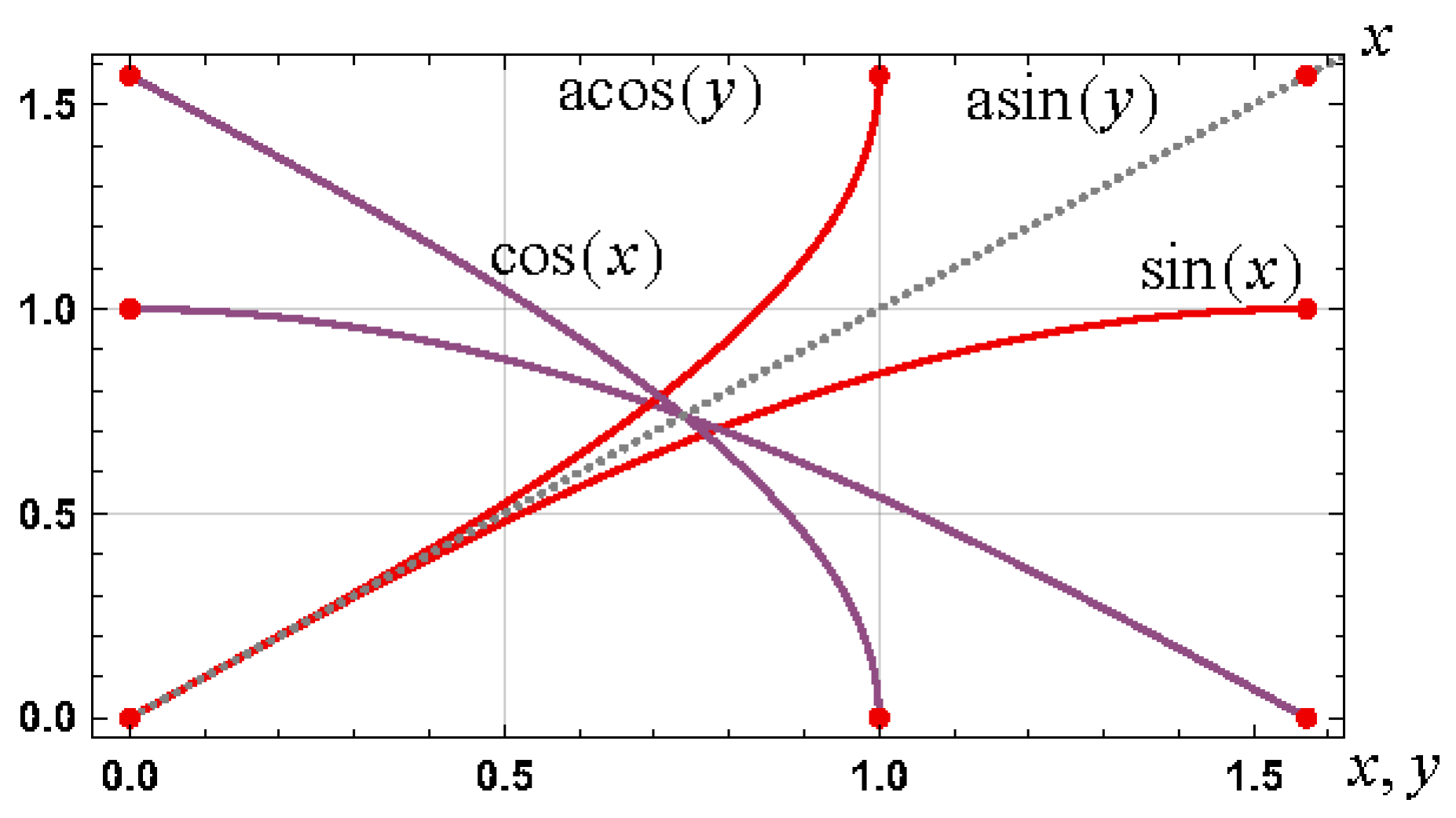
3. Example I: Analytical Approximations for Arcsine
3.1. General Schröder-Based Approximations
3.1.1. Initial Approximations
3.1.2. Explicit Approximations
3.1.3. Results
3.2. Newton–Raphson Iteration
3.3. Hybrid Approximation
3.4. Applications
3.4.1. Lower Bound
3.4.2. Integral
4. Example II: Analytical Approximations for Inverse of x − Sin(x)
4.1. Initial Approximation for
4.2. General Schröder-Based Approximations
Examples
4.3. Newton–Raphson Iteration
4.4. Results
4.5. Applications
5. Example III: Analytical Approximations for Inverse Langevin Function
5.1. Approximations
5.2. General Schröder-Based Approximations
5.3. Results
5.4. Newton–Raphson Iteration
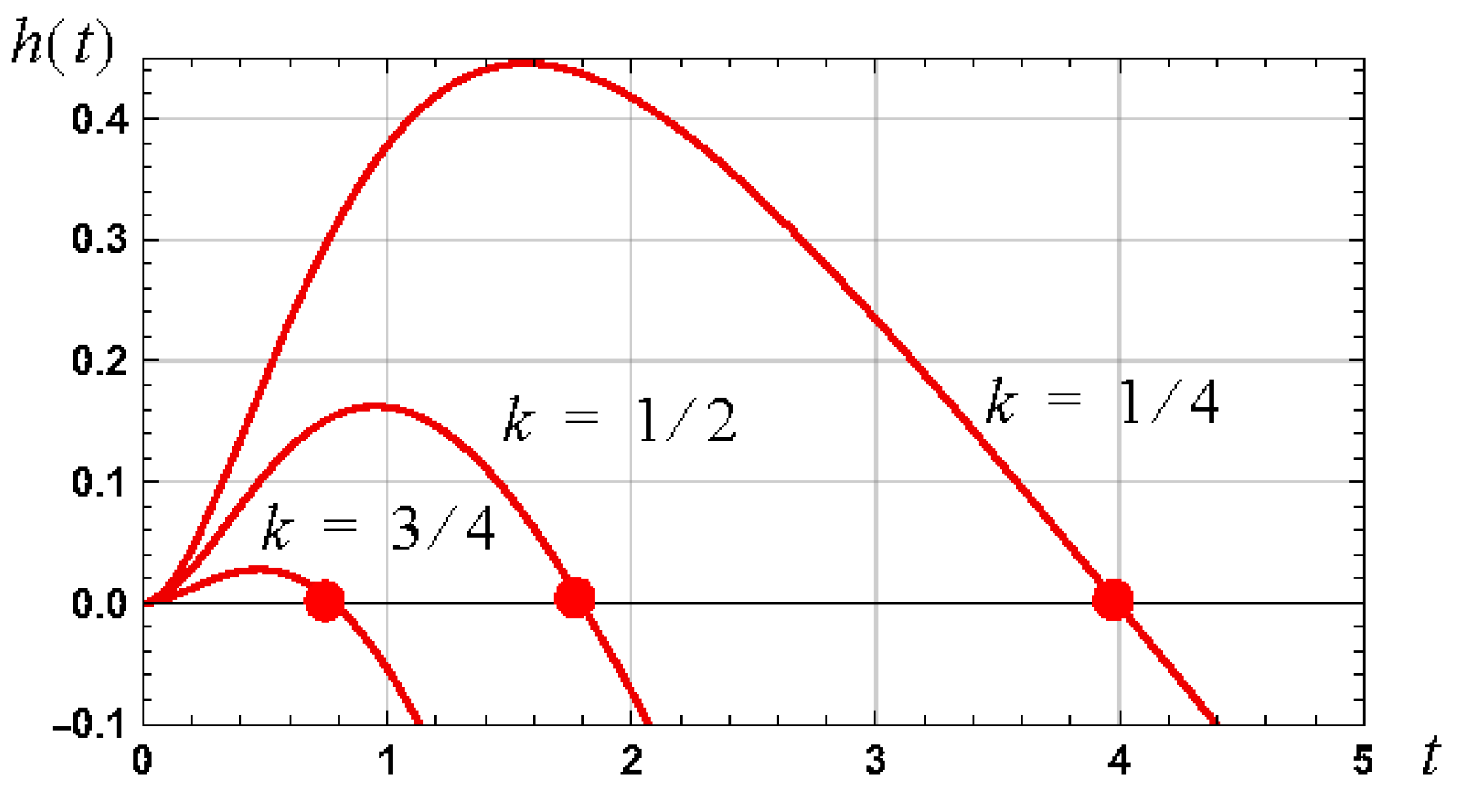
5.5. Applications
Inverse Langevin Function as Zero Crossing Time of an Impulse Response
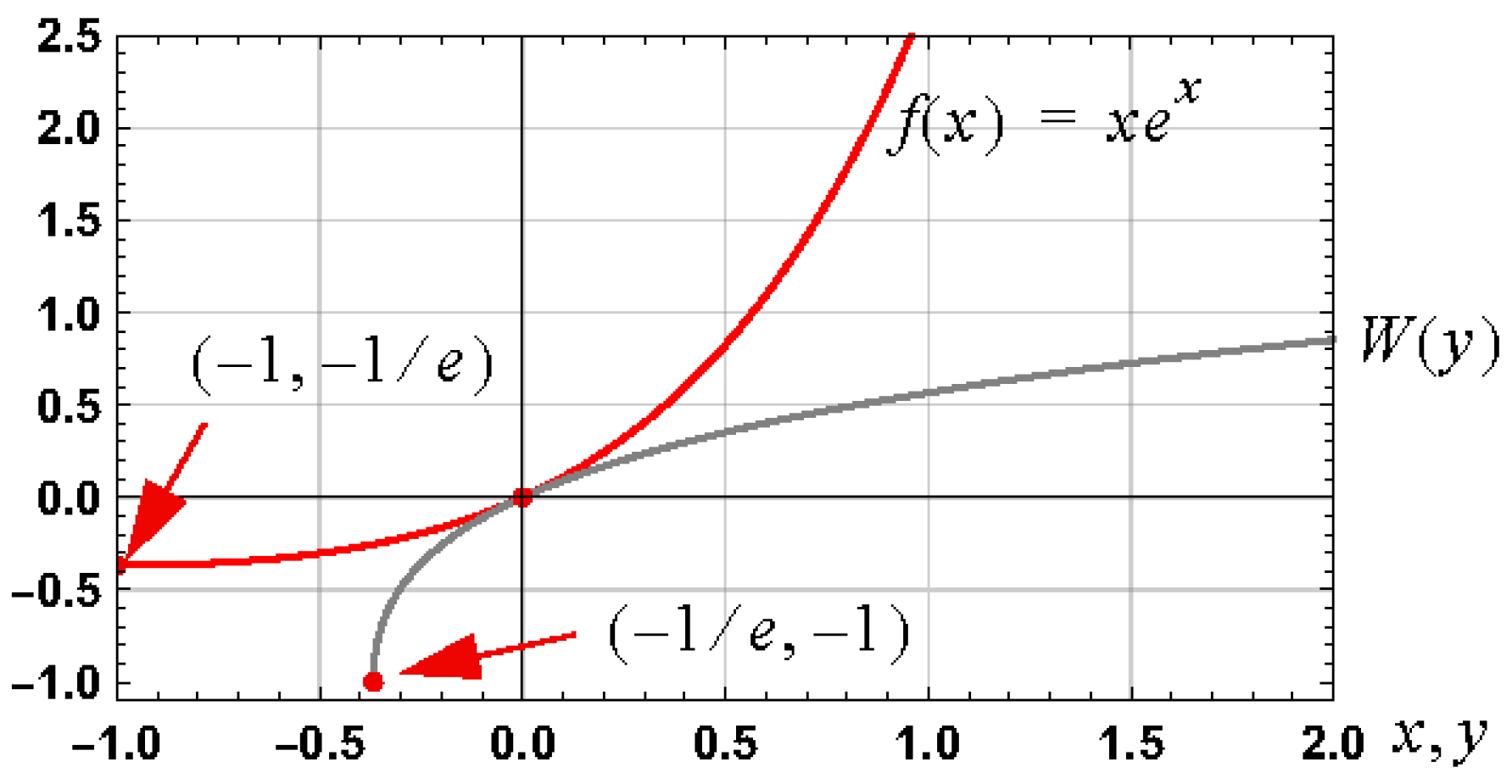
6. Example IV: Analytical Approximations for Lambert Function
6.1. Approximations
6.2. General Schröder-Based Approximations
6.2.1. Special Form
6.2.2. Explicit Approximation
6.3. Hybrid Approximations
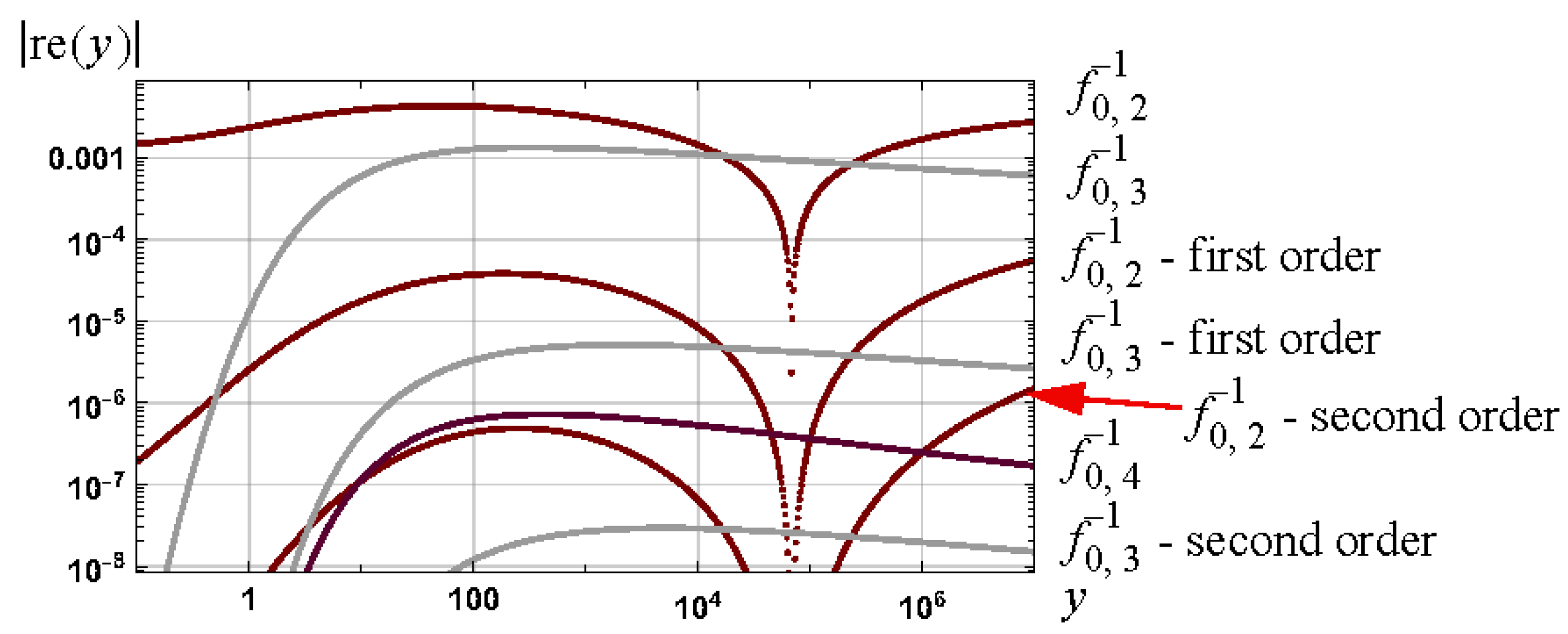
6.4. Results
6.5. Applications
7. Conclusions
Further Research
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Theorem 1
Appendix B. Proof of Lemma 1
Appendix C. Derivative of for the Case of
Appendix D. Inverse of x-Sin(x): Use of Periodicity and Anti-Symmetry
References
- Jedynak, R. New facts concerning the approximation of the inverse Langevin function. J. Non-Newtonian Fluid Mech. 2017, 249, 8–25. [Google Scholar] [CrossRef]
- Jedynak, R. A comprehensive study of the mathematical methods used to approximate the inverse Langevin function. Math. Mech. Solids 2018, 24, 1992–2016. [Google Scholar] [CrossRef]
- Gdawiec, K.; Kotarski, W.; Lisowska, A. Polynomiography based on the nonstandard Newton-like root finding methods. Abstr. Appl. Anal. 2015, 2015, 797594. [Google Scholar] [CrossRef]
- Ypma, T.J. Historical development of the Newton–Raphson method. SIAM Rev. 1995, 37, 531–551. [Google Scholar] [CrossRef]
- Kalantari, B.; Kalantari, I.; Zaare-Nahandi, R. A basic family of iteration functions for polynomial root finding and its characterizations. J. Comput. Appl. Math. 1997, 80, 209–226. [Google Scholar] [CrossRef]
- Petković, M.; Herceg, D. On rediscovered iteration methods for solving equations. J. Comput. Appl. Math. 1999, 107, 275–284. [Google Scholar] [CrossRef]
- Amat, S.; Busquier, S.; Gutiérrez, J.M. Geometric constructions of iterative functions to solve nonlinear equations. J. Comput. Appl. Math. 2003, 157, 197–205. [Google Scholar] [CrossRef]
- Abbasbandy, S. Improving Newton–Raphson method for nonlinear equations by modified Adomian decomposition method. Appl. Math. Comput. 2003, 145, 887–893. [Google Scholar] [CrossRef]
- Chun, C. Iterative methods improving Newton’s method by the decomposition method. Comput. Math. Appl. 2005, 50, 1559–1568. [Google Scholar] [CrossRef]
- Noor, M.A.; Gupta, V. Modified Householder iterative method free from second derivatives for nonlinear equations. Appl. Math. Comput. 2007, 190, 1701–1706. [Google Scholar] [CrossRef]
- Dubeau, F. Polynomial and rational approximations and the link between Schröder’s processes of the first and second kind. Abstr. Appl. Anal. 2014, 2014, 719846. [Google Scholar] [CrossRef]
- Schröder, E. Über unendlich viele Algorithmen zur Auflösung der Gleichungen. Math. Ann. 1870, 2, 317–365. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables; Dover: Mineola, NY, USA, 1964. [Google Scholar]
- Copson, E.T. An Introduction to the Theory of Functions of a Complex Variable; Oxford University Press: Oxford, UK, 1935; pp. 121–123. [Google Scholar]
- Howard, R.M. Radial Based Approximations for Arcsine, Arccosine, Arctangent and Applications. AppliedMath 2023, 3, 343–394. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Tables of Integrals, Series and Products, 7th ed.; Jeffery, A., Zwillinger, D., Eds.; Academic Press: Cambridge, MA, USA, 2007. [Google Scholar]
- Fink, A.M. Two inequalities. Univ. Beograd. Publ. Elektrotehn. Fak. Ser. Mat. 1995, 6, 49–50. [Google Scholar]
- Howard, R.M. Arbitrarily accurate analytical approximations for the Error function. Math. Comput. Appl. 2022, 27, 14. [Google Scholar] [CrossRef]
- Itskov, M.; Dargazany, R.; Hornes, K. Taylor expansion of the inverse function with application to the Langevin function. Math. Mech. Solids 2011, 17, 693–701. [Google Scholar] [CrossRef]
- Howard, R.M. Analytical approximations for the inverse Langevin function via linearization, error approximation and iteration. Rheol. Acta 2020, 59, 521–544. [Google Scholar] [CrossRef]
- Petrosyan, R. Improved approximations for some polymer extension models. Rheol. Acta 2017, 56, 21–26. [Google Scholar] [CrossRef]
- Nguessong, A.N.; Beda, T.; Peyraut, F. A new based error approach to approximate the inverse Langevin function. Rheol. Acta 2014, 53, 585–591. [Google Scholar] [CrossRef]
- Kröger, M. Simple, admissible, and accurate approximants of the inverse Langevin and Brillouin functions, relevant for strong polymer deformations and flows. J. Non-Newton. Fluid Mech. 2015, 223, 77–87. [Google Scholar] [CrossRef]
- Marchi, B.C.; Arruda, E.M. Generalized error-minimizing, rational inverse Langevin approximations. Math. Mech. Solids 2019, 24, 1630–1647. [Google Scholar] [CrossRef]
- Veberič, D. Lambert W function for applications in physics. Comput. Phys. Commun. 2012, 183, 2622–2628. [Google Scholar] [CrossRef]
- Howard, R.M. Analytical approximations for the principal branch of the Lambert W function. Eur. J. Math. Anal. 2022, 2, 14. [Google Scholar] [CrossRef]
- Lóczi, L. Guaranteed-and high-precision evaluation of the Lambert W function. Appl. Math. Comput. 2022, 433, 127406. [Google Scholar] [CrossRef]
- Banwell, T.C. Bipolar transistor circuit analysis using the Lambert W-function. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2000, 47, 1621–1633. [Google Scholar] [CrossRef]
- Visser, M. Primes and the Lambert W function. Mathematics 2018, 6, 56. [Google Scholar] [CrossRef]
- Barry, D.A.; Parlange, J.Y.; Li, L.; Prommer, H.; Cunningham, C.J.; Stagnitti, F. Analytical approximations for real values of the Lambert W-function. Math. Comput. Simul. 2000, 53, 95–103. [Google Scholar] [CrossRef]
- Iacono, R.; Boyd, J.P. New approximations to the principal real-valued branch of the Lambert W-function. Adv. Comput. Math. 2017, 43, 1403–1436. [Google Scholar] [CrossRef]
- Goličnik, M. On the Lambert W function and its utility in biochemical kinetics. Biochem. Eng. J. 2012, 63, 116–123. [Google Scholar] [CrossRef]
- Dargazany, R.; Hörnes, K.; Itskov, M. A simple algorithm for the fast calculation of higher order derivatives of the inverse function. Appl. Math. Comput. 2013, 221, 833–838. [Google Scholar] [CrossRef]
- Craik, A.D. Prehistory of Faà di Bruno’s formula. Am. Math. Mon. 2005, 112, 119–130. [Google Scholar]
- Leslie, R.A. How not to repeatedly differentiate a reciprocal. Am. Math. Mon. 1991, 98, 732–735. [Google Scholar] [CrossRef]



| Approximation | ||||
|---|---|---|---|---|
| Original approximation | ||||
| 1st order: (42) | ||||
| 2nd order: (43) | ||||
| 3rd order: (44) | ||||
| 4th order: (45) | ||||
| 5th order | ||||
| NR—1st iteration: (57) | ||||
| NR—2nd iteration: (58) | ||||
| NR—3rd iteration: (59) | ||||
| NR—4th iteration |
| Approximation | |||
|---|---|---|---|
| Original approximation | |||
| 1st order: (72) | |||
| 2nd order: (73) | |||
| 3rd order: (74) | |||
| 4th order: (75) | |||
| 5th order | |||
| NR—1st iteration: (72) | |||
| NR—2nd iteration: (78) | |||
| NR—3rd iteration | |||
| NR—4th iteration |
| Approximation | |||
|---|---|---|---|
| Original approximation | |||
| 1st order: (94) | |||
| 2nd order: (95) | |||
| 3rd order | |||
| 4th order | |||
| 5th order | |||
| NR—1st iteration | |||
| NR—2nd iteration | |||
| NR—3rd iteration | |||
| NR—4th iteration |
| Approximation | ||||
|---|---|---|---|---|
| Original approximation | 1.96 × 10−3 | 4.53 × 10−3 | 1.33 × 10−3 | 7.23 × 10−7 |
| 1st order: (109) or (114) | 1.60 × 10−5 | 3.02 × 10−4 | 5.12 × 10−6 | 1.49 × 10−12 |
| 2nd order: (110) or (115) | 2.96 × 10−7 | 2.92 × 10−5 | 2.93 × 10−8 | 4.31 × 10−18 |
| 3rd order: (111) | 7.45 × 10−9 | 3.23 × 10−6 | 1.94 × 10−10 | 1.43 × 10−25 |
| 4th order: (112) | 2.02 × 10−10 | 3.86 × 10−7 | 1.39 × 10−12 | 5.06 × 10−29 |
| 5th order | 5.70 × 10−12 | 4.82 × 10−8 | 1.05 × 10−14 | 1.88 × 10−34 |
| NR—1st iteration: (109) | 1.60 × 10−5 | 3.02 × 10−4 | 5.12 × 10−6 | 1.49 × 10−12 |
| NR—2nd iteration | 3.66 × 10−9 | 1.49 × 10−6 | 9.61 × 10−11 | 6.98 × 10−24 |
| NR—3rd iteration | 2.89 × 10−16 | 3.92 × 10−11 | 3.91 × 10−20 | 1.62 × 10−46 |
| NR—4th iteration | 1.81 × 10−30 | 2.79 × 10−20 | 7.08 × 10−39 | 9.04 × 10−92 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Howard, R.M. Schröder-Based Inverse Function Approximation. Axioms 2023, 12, 1042. https://doi.org/10.3390/axioms12111042
Howard RM. Schröder-Based Inverse Function Approximation. Axioms. 2023; 12(11):1042. https://doi.org/10.3390/axioms12111042
Chicago/Turabian StyleHoward, Roy M. 2023. "Schröder-Based Inverse Function Approximation" Axioms 12, no. 11: 1042. https://doi.org/10.3390/axioms12111042
APA StyleHoward, R. M. (2023). Schröder-Based Inverse Function Approximation. Axioms, 12(11), 1042. https://doi.org/10.3390/axioms12111042







