Geometrically Non-Linear Plane Elasticity Problem in the Area of an Angular Boundary Cut-Out
Abstract
:1. Introduction
- (1)
- To consider the geometrical relationships between deformations and displacements in the curvilinear orthogonal coordinate system, taking into account finite deformations and to derive the geometrical relationships in a polar coordinate system for the plane strain state in generalized strains;
- (2)
- To derive the equilibrium equations in the curvilinear orthogonal coordinate system and to derive the equilibrium equations in the polar coordinate system for the plane strain state in generalized strains;
- (3)
- To formulate the plane elasticity problem in the area of the domain boundary angular cut-out, taking into account the geometrical non-linearity, which is physically linear under the action of forced deformations or free thermal strains.
2. Materials and Methods
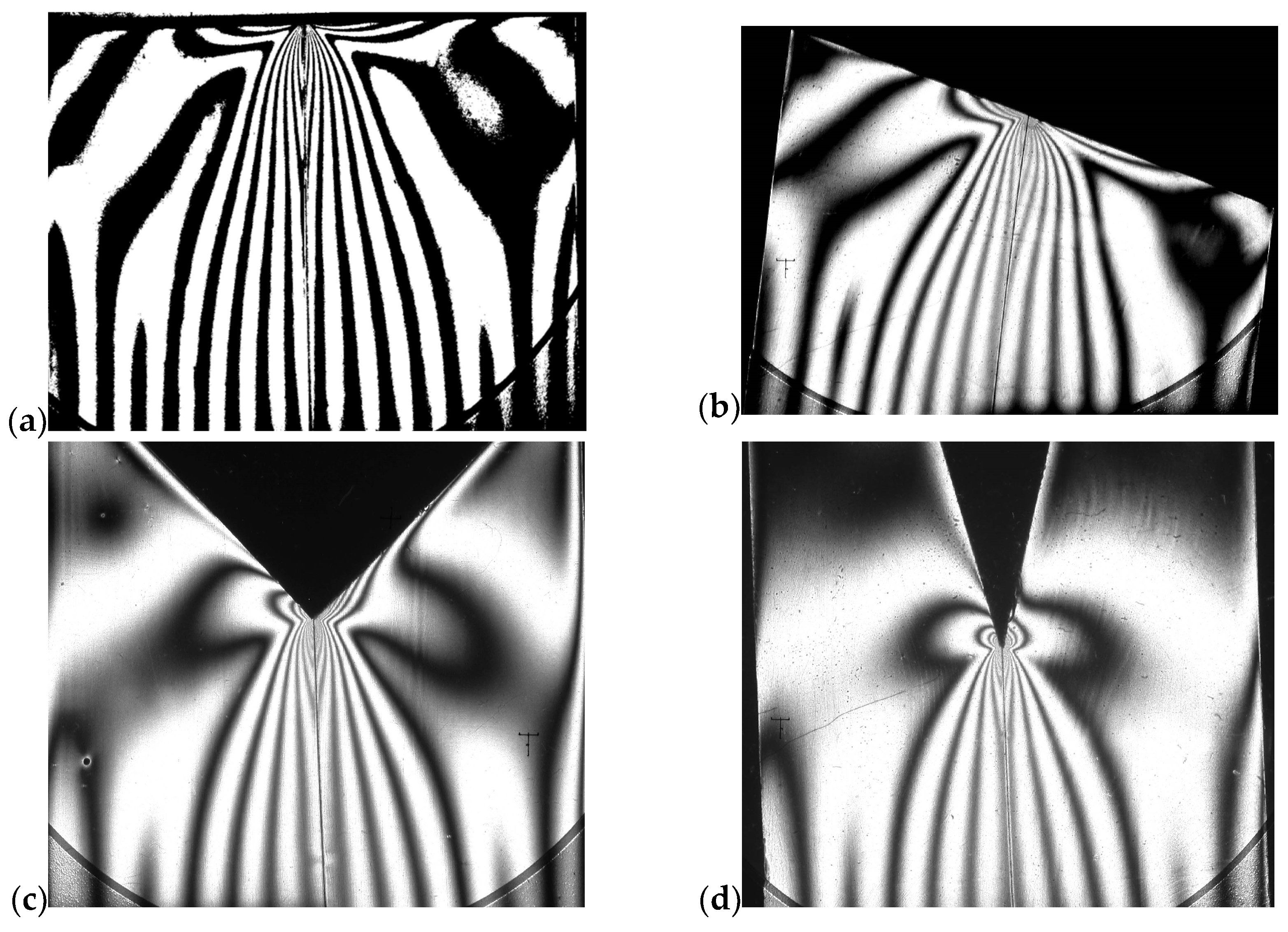
2.1. Experimental Modeling: Problem Statement
2.2. Object of Study
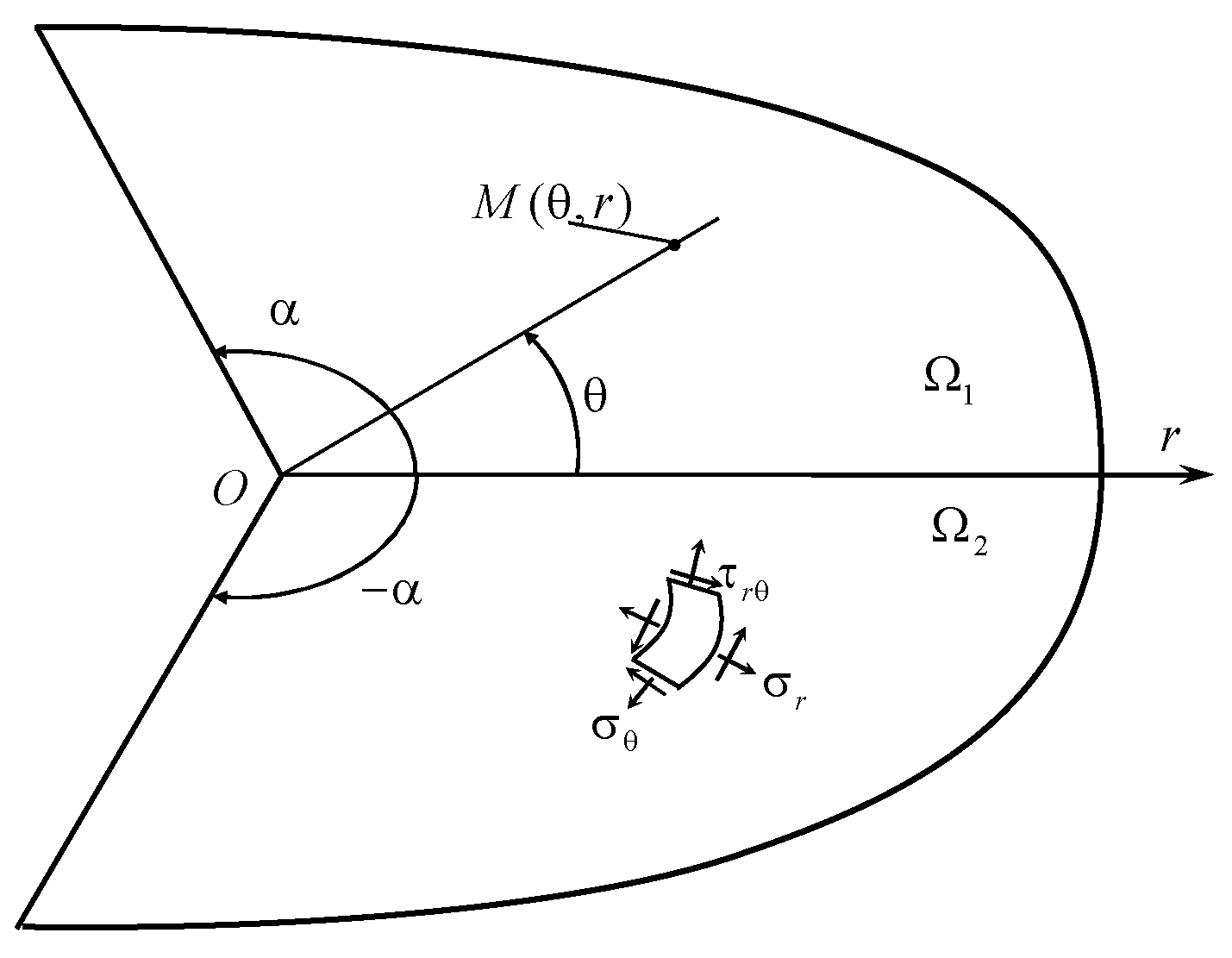
2.3. Taking Finite Deformations into Account for the Spatial Curvilinear Orthogonal and Polar Coordinate Systems
2.4. Ratios for Generalized Stresses and Non-Linear Deformations
2.5. Equilibrium Equations
3. Results
- (1)
- Geometrical relationships between deformations and displacements were provided in a curvilinear orthogonal coordinate system taking into account finite deformations (6); geometrical relationships were derived in the polar coordinate system for the plane deformation state taking into account non-linear deformations (9) and displacements (10);
- (2)
- Equations of equilibrium in the curvilinear orthogonal coordinate system (22), (23), and (24) were provided; equations of equilibrium in the polar coordinate system were derived for the plane deformation state in generalized stresses (25), (26), (27), and (28) and strains (33) and (34) under the action of forced temperature deformations .
- (3)
- The formulation of the plane elasticity problem in the angular boundary cut-out domain taking into account geometric non-linearity, physically linear under the action of forced deformations or free temperature deformations for the plane deformation state includes the following system of equations: geometrical relations (9), equilibrium equations (33) and (34), physical relations (19) and (20), homogeneous boundary conditions (39), and displacement and stress continuity conditions along the contact line of the composite domain in the presence of a finite discontinuity of the given forced deformations on the line of the domain contact. The continuity conditions for deformations are provided in [30].
4. Discussion
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Parton, V.; Perlin, P. Methods of Mathematical Elasticity; Nauka: Moscow, Russia, 1981; 688p. [Google Scholar]
- Love, A.E.H. A Treatise on the Mathematical Theory of Elasticity; New York Public Library: New York, NY, USA, 1944; 643p. [Google Scholar]
- Timoshenko, S.P.; Goodiear, J.N. Theory of Elasticity; McGRAW-HILL BOOK: New York, NY, USA; Toronto, ON, Canada; London, UK, 1951; 522p. [Google Scholar]
- Cherepanov, G. Brittle Destruction Mechanics; Nauka: Moscow, Russia, 1974; 640p. [Google Scholar]
- Cherepanov, G. On singular solutions in elasticity theory. In Mechanics of a Solid Deformed Body; Sudostroyenie: Leningrad, Russia, 1970; pp. 17–32. [Google Scholar]
- Lourier, A. Elasticity Theory; Nauka: Moscow, Russia, 1970; 940p. [Google Scholar]
- Kondratyev, V. Boundary value problems for elliptic equations in domains with conical or corner points. In Transactions of the Moscow Mathematical Society; MSU: Moscow, Russia, 1967; Volume 16, pp. 209–292. [Google Scholar]
- Rabotnov, Y. Mechanics of Deformable Solids; Nauka: Moscow, Russia, 1979; 744p. [Google Scholar]
- Kuliev, V. Singular Boundary Value Problems; Nauka: Moscow, Russia, 2005; 719p. [Google Scholar]
- Aksentjan, O. Features of the stress-strain state of the plate in the neighborhood of the edge. Appl. Math. Mech. 1967, 31, 178–186. [Google Scholar]
- Geen, A.E.; Rivlin, R.S. General Theory of Small Elastic Deformations. Proc. R. Soc. Lond. Ser. A 1952, 211, 128–154. [Google Scholar]
- Rivlin, R.S. The Solution of Problems in Second Order Elasticity Theory. J. Rat. Mech. Anal. 1953, 2, 53–81. [Google Scholar] [CrossRef]
- Toupin, R.A.; Rivlin, R.S. Dimensional Change in Crystals Caused by Dislocation. J. Math. Phys. 1960, 1, 8–15. [Google Scholar] [CrossRef]
- Adkins, J.E.; Geen, A.E.; Nicholas, G.C. Two–dimensional Theory of Elasticity for Finite deformations. Philos. Trans. R. Soc. Lond. Ser. A 1954, 247, 279–306. [Google Scholar]
- Makhutov, N.; Moskvichev, V.; Morozov, E.; Goldstein, R. Unification of computation and experimental methods of testing for crack resistance: Development of the fracture mechanics and new goals. Ind. Lab. Diagn. Mater. 2017, 83, 55–64. [Google Scholar] [CrossRef]
- Morozov, E.; Nikishkov, G. Finite Elements Method in Fracture Mechanics; Knizhny dom Librokom: Moscow, Russia, 2017; 256p. [Google Scholar]
- Kogaev, V.; Makhutov, N.; Gusenkov, A. Calculations of Machine Parts and Structures for Strength and Durability: Guidebook; Mashinostroyenie: Moscow, Russia, 1985; 224p. [Google Scholar]
- Matviyenko, Y. Fracture Mechanics Models and Criteria; Fizmatlit: Moscow, Russia, 2006; 328p. [Google Scholar]
- Frishter, L. Stress-strain state in structure angular zone taking into account differences between stress and deformation intensity factors. Adv. Intell. Syst. Comput. 2020, 982, 352–362. [Google Scholar] [CrossRef]
- Razumovskij, I. Interference-Optical Methods of Deformable Solid Mechanics; Bauman MSTU: Moscow, Russia, 2007; 240p. [Google Scholar]
- Hesin, G. The Photoelasticity Method; Stroyizdat: Moscow, Russia, 1975; Volume 3, 311p. [Google Scholar]
- Kobayashy, A.S.; Dally, J.W.; Atluri, S.N.; Riley, W.F.; Sirkis, J.S.; MCConnell, K.G.; Burger, C.P.; Parks, V.J.; Post, D.; Ranson, W.F.; et al. Handbook on Experimental Mechanics; Albert, S., Kobayashy, A.S., Eds.; University of Washington: Seattle, WA, USA, 1987; Volume 1. [Google Scholar]
- Kobayashy, A.S. (Ed.) Experimental Techniques in Fracture Mechanics 1 and 2; Iowa State University Press: Ames, IA, USA, 1975. [Google Scholar]
- Gourgiotis, P.A.; Georgiadis, H.G. The problem of sharp notch in couple-stress elasticity. Int. J. Solids Struct. 2011, 48, 2630–2641. [Google Scholar] [CrossRef]
- Sinclair, G.B. Stress singularities in classical elasticity. II: Asymptotic identification. Appl. Mech. Rev. 2004, 57, 385–439. [Google Scholar] [CrossRef]
- Pestrenin, V.; Pestrenina, I.; Landik, L.; Vestnik, T.G.U. Mathematics and Mechanics; TGU: Tomsk, Russia, 2013; Volume 4, pp. 80–87. [Google Scholar]
- Frishter, L. Photoelasticity-based study of stress-strain state in the area of the plain domain boundary cut-out area vertex. In Proceedings of the 19th International Scientific Conference Energy Management of Municipal Transportation Facilities and Transport EMMFT 2017, Khabarovsk, Russia, 10–13 April 2017; Advances in Intelligent Systems and Computing. Murgul, V., Popovic, Z., Eds.; Springer: Cham, Switzerland, 2017; Volume 692, pp. 836–844. [Google Scholar] [CrossRef]
- Tihomirov, V. Determination of stress intensity factors by the method of photoelasticity in three-dimensional problems of fracture mechanics. In Development of Methods of Experimental Mechanics; Makhutova, N., Ed.; IMASH RAN: Moscow, Russia, 2003; pp. 103–112. [Google Scholar]
- Kasatkin, B.; Kudrin, A.; Lobanov, L.M. Experimental Methods of Investigating Stresses and Strains; Reference Manual; Kasatkin, B., Ed.; Naukova Dumka: Kiyiv, Ukraine, 1981; 584p. [Google Scholar]
- Novozhilov, V.V. Theory of Elasticity; Pergamon Press: London, UK, 1961; 448p. [Google Scholar]
- Sedov, L. Continuum Mechanics, 6th ed.; Lan: Moscow, Russia, 2004; Volume 1, 528p, Volume 2, 560p. [Google Scholar]
- Bakushev, S. Geometrically and Physically Nonlinear Continuum Mechanics: The Plane Problem; Book House LIBROKOM: Moscow, Russia, 2013; 321p. [Google Scholar]
- Green, A.; Adkins, J. Large Elastic Deformations and Nonlinear Continuum Mechanics; Mir: Moscow, Russia, 1965; 456p. [Google Scholar]
- Vardanyan, G.; Andreev, V.; Atarov, N.; Gorshkov, A. Resistance of Materials with Fundamentals of Theory of Elasticity and Plasticity; ACB Publishers: Moscow, Russia, 1995; 568p. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frishter, L. Geometrically Non-Linear Plane Elasticity Problem in the Area of an Angular Boundary Cut-Out. Axioms 2023, 12, 1030. https://doi.org/10.3390/axioms12111030
Frishter L. Geometrically Non-Linear Plane Elasticity Problem in the Area of an Angular Boundary Cut-Out. Axioms. 2023; 12(11):1030. https://doi.org/10.3390/axioms12111030
Chicago/Turabian StyleFrishter, Lyudmila. 2023. "Geometrically Non-Linear Plane Elasticity Problem in the Area of an Angular Boundary Cut-Out" Axioms 12, no. 11: 1030. https://doi.org/10.3390/axioms12111030
APA StyleFrishter, L. (2023). Geometrically Non-Linear Plane Elasticity Problem in the Area of an Angular Boundary Cut-Out. Axioms, 12(11), 1030. https://doi.org/10.3390/axioms12111030





