A Certain Class of Equi-Statistical Convergence in the Sense of the Deferred Power-Series Method
Abstract
:1. Introduction, Preliminaries and Motivation
- (a)
- If, for each and for everywherethen is deferred statistically point-wise convergent to f on I. We write
- (b)
- If, for each ,then is equi-statistically convergent to f on I. We write
- (c)
- If, for eachwherethen is statistically uniformly convergent to f on I. We write
2. A Korovkin-Type Approximation Theorem
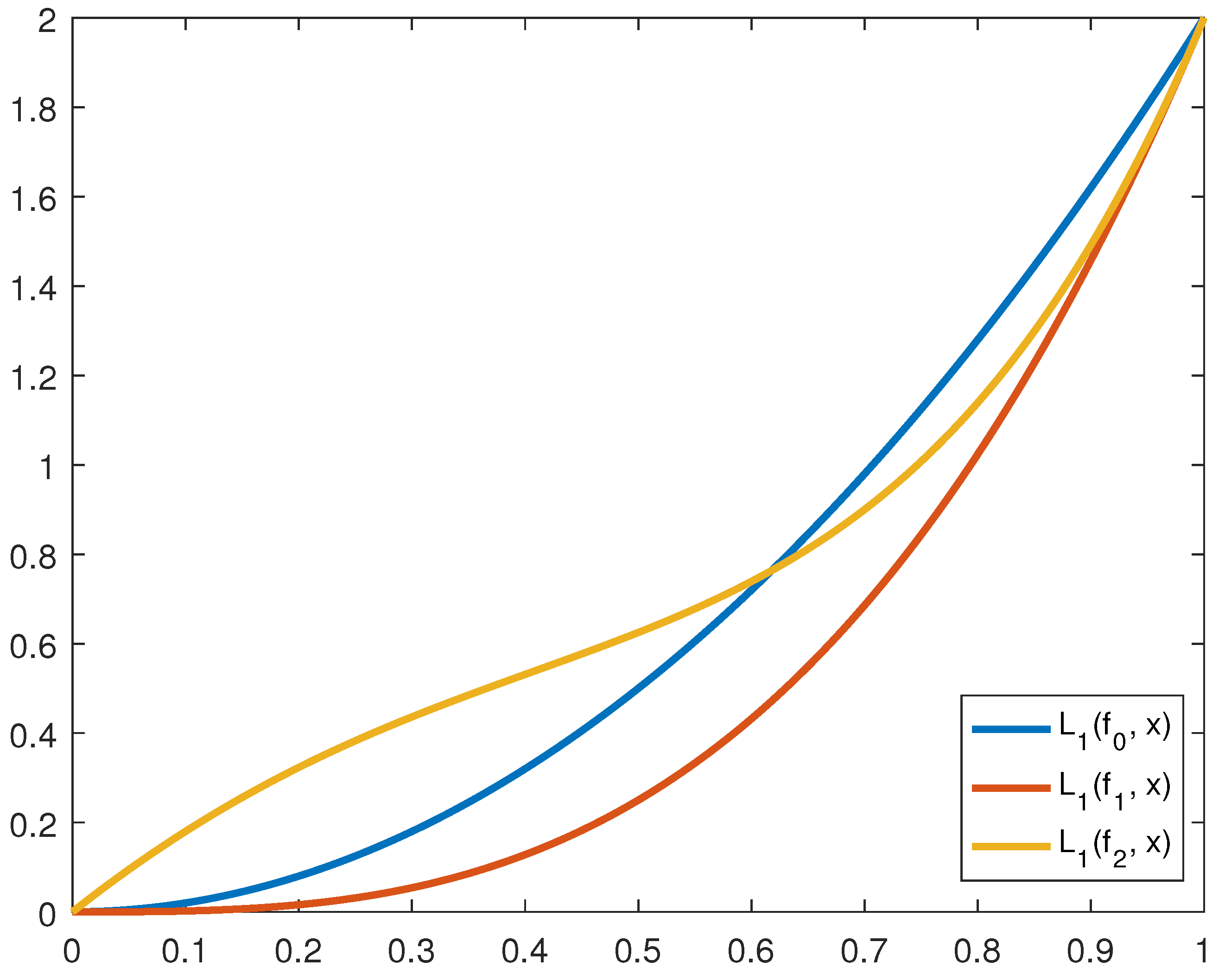
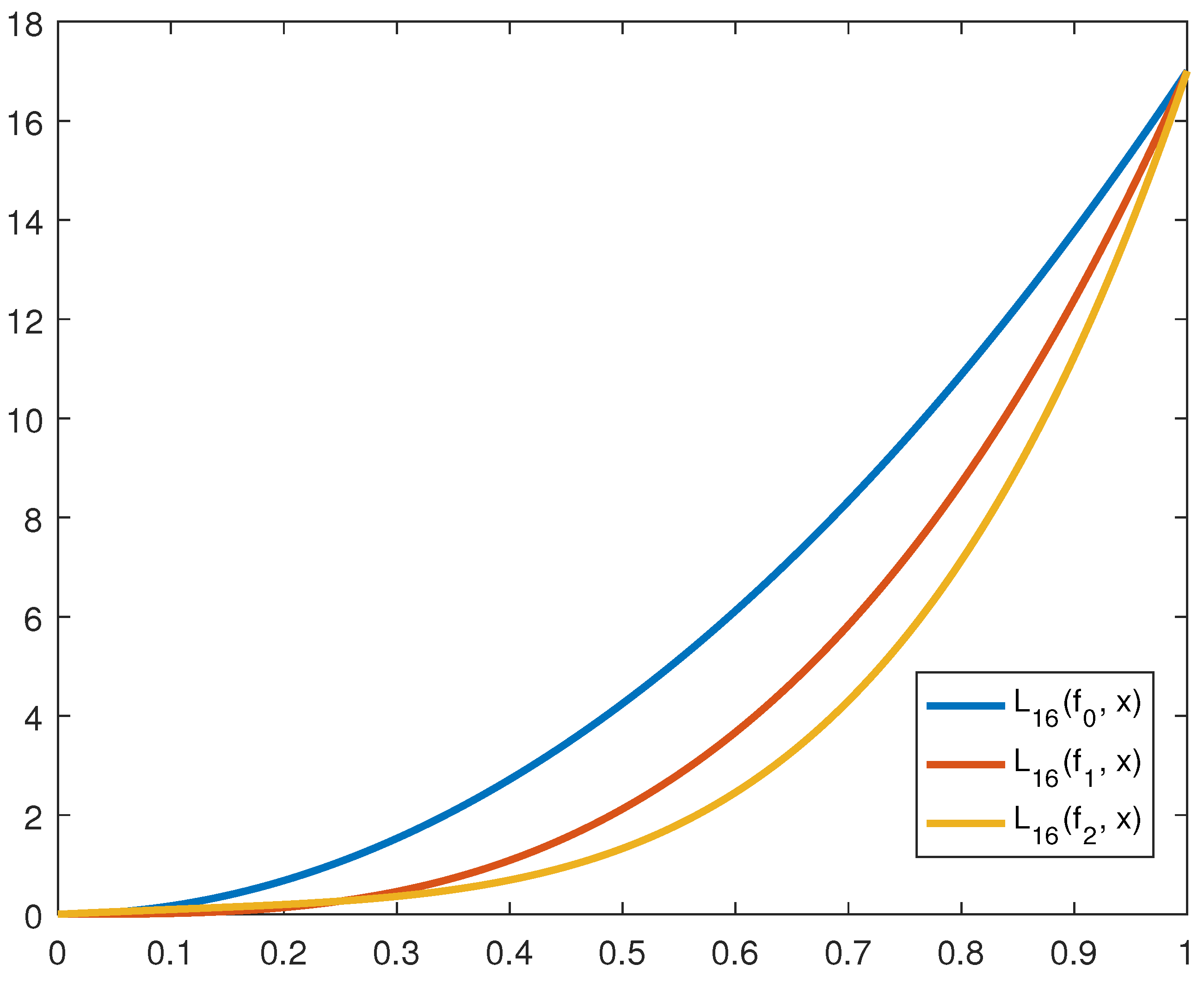
3. Geometrical View of Theorem 4
4. Rate of DP-Equi-Statistical Convergence
- (i)
- on I
- (ii)
- (iii)
- for any scalar
- (iv)
- ,
- (i)
- (ii)
5. Concluding Remarks and Observations
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zygmund, A. Trigonometric Series; Cambridge University Press: Cambridge, UK, 1959. [Google Scholar]
- Fast, H. Sur la convergence statistique. Colloq. Math. 1951, 2, 241–244. [Google Scholar] [CrossRef]
- Steinhaus, H. Sur la convergence ordinaire et la convergence asymtotique. Colloq. Math. 1951, 2, 73–74. [Google Scholar]
- Jena, B.B.; Paikray, S.K. Product of deferred Cesàro and deferred weighted statistical probability convergence and its applications to Korovkin-type theorems. Univ. Sci. 2020, 25, 409–433. [Google Scholar] [CrossRef]
- Jena, B.B.; Paikray, S.K.; Dutta, H. A new approach to Korovkin-type approximation via deferred Cesàro statistical measurable convergence. Chaos Solitons Fractals 2021, 148, 111016. [Google Scholar] [CrossRef]
- Agnew, R.P. On deferred Cesàro means. Ann. Math. 1932, 33, 413–421. [Google Scholar] [CrossRef]
- Jena, B.B.; Paikray, S.K.; Misra, U.K. Statistical deferred Cesàro summability and its applications to approximation theorems. Filomat 2018, 32, 2307–2319. [Google Scholar] [CrossRef]
- Boos, J. Classical and Modern Methods in Summability; Clarendon (Oxford University) Press: Oxford/London, UK; New York, NY, USA, 2000. [Google Scholar]
- Šalát, T. On statistically convergent sequences of real numbers. Math. Slovaca 1980, 30, 139–150. [Google Scholar]
- Maddox, I.J. Statistical convergence in a locally convex space. Math. Proc. Camb. Phil. Soc. 1988, 104, 141–145. [Google Scholar] [CrossRef]
- Fridy, J.A.; Orhan, C. Lacunary statistical summability. J. Math. Anal. Appl. 1993, 173, 497–504. [Google Scholar] [CrossRef]
- Móricz, F. Tauberian conditions under which statistical convergence follows from statistical summability (C, 1). J. Math. Anal. Appl. 2002, 275, 277–287. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Alotaibi, A.; Mursaleen, M. Statistical summability (C, 1) and a Korovkin-type approximation theorem. J. Inequal. Appl. 2012, 2012, 172. [Google Scholar] [CrossRef]
- Karakaya, V.; Chishti, T.A. Weighted statistical convergence. Iran. J. Sci. Technol. Trans. A Sci. 2009, 33, 219–223. [Google Scholar]
- Mursaleen, M.; Karakaya, V.; Ertürk, M.; Gxuxrsoy, F. Weighted statistical convergence and its application to Korovkin-type approximation theorem. Appl. Math. Comput. 2012, 218, 9132–9137. [Google Scholar] [CrossRef]
- Baliarsingh, P. On statistical deferred A-convergence of uncertain sequences. Int. Uncertain. Fuzziness-Knowl.-Based Syst. 2021, 29, 499–515. [Google Scholar] [CrossRef]
- Saini, K.; Raj, K.; Mursaleen, M. Deferred Cesàro and deferred Euler equi-statistical convergence and its applications to Korovkin-type approximation theorem. Int. J. Gen. Syst. 2021, 50, 567–579. [Google Scholar] [CrossRef]
- Saini, K.; Raj, K. Applications of statistical convergence in complex uncertain sequences via deferred Riesz mean. Int. J. Uncertain. Fuzziness-Knowl.-Based Syst. 2021, 29, 337–351. [Google Scholar] [CrossRef]
- Sharma, S.; Singh, U.P.; Raj, K. Applications of deferred Cesàro statistical convergence of sequences of fuzzy numbers of order (ξ,ω). J. Intell. Fuzzy Syst. 2021, 41, 7363–7372. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K.; Misra, U.K. Generalized equi-statistical convergence of the deferred Nörlund summability and its applications to associated approximation theorems. Rev. Real Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. (RACSAM) 2018, 112, 1487–1501. [Google Scholar] [CrossRef]
- Parida, P.; Paikray, S.K.; Jena, B.B. Generalized deferred Cesàro equi-statistical convergence and analogous approximation theorems. Proyecciones J. Math. 2020, 39, 307–331. [Google Scholar] [CrossRef]
- Demirci, K.; Dirik, F.; Yıldız, S. Approximation via equi-statistical convergence in the sense of power series method. Rev. Real Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. 2022, 116, 65. [Google Scholar] [CrossRef]
- Ünver, M.; Orhan, C. Statistical convergence with respect to power series methods and applications to approximation theory. Numer. Funct. Anal. Optim. 2019, 40, 535–547. [Google Scholar] [CrossRef]
- Korovkin, P.P. Convergence of linear positive operators in the spaces of continuous functions. Doklady Akad. Nauk. SSSR 1953, 90, 961–964. (In Russian) [Google Scholar]
- Altomare, F.; Campiti, M. Korovkin-type Approximation Theory and Its Applications. In De Gruyter Studies in Mathematics; Walter de Gruyter: Berlin, Germany, 1994; Volume 17. [Google Scholar]
- Demirci, K.; Orhan, S.; Kolay, B. Statistical relative A-summation process for double sequences on modular spaces. Rev. Real Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. 2018, 112, 1249–1264. [Google Scholar] [CrossRef]
- Jena, B.B.; Paikray, S.K. Product of statistical probability convergence and its applications to Korovkin-type theorem. Miskolc Math. Notes 2019, 20, 969–984. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K. Deferred Cesàro statistical convergence of martingale sequence and Korovkin-type approximation theorems. Miskolc Math. Notes 2022, 23, 443–456. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K. Statistical product convergence of martingale sequences and its applications to Korovkin-type approximation theorems. Math. Methods Appl. Sci. 2021, 44, 9600–9610. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K. Statistical Riemann and Lebesgue integrable sequence of functions with Korovkin-type approximation theorems. Axioms 2021, 10, 229. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K. Statistical deferred Nörlund summability and Korovkin-type approximation theorem. Mathematics 2020, 8, 636. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Jena, B.B.; Paikray, S.K. Deferred Cesàro statistical probability convergence and its applications to approximation theorems. J. Nonlinear Convex Anal. 2019, 20, 1777–1792. [Google Scholar]
- Balcerzak, M.; Dems, K.; Komisarski, A. Statistical convergence and ideal convergence for sequences of functions. J. Math. Anal. Appl. 2007, 328, 715–729. [Google Scholar] [CrossRef]
- Paikray, S.K.; Parida, P.; Mohiuddine, S.A. A certain class of relatively equi-statistical fuzzy approximation theorems. Eur. J. Pure Appl. Math. 2020, 13, 1212–1230. [Google Scholar] [CrossRef]
- Akdağ, S. Weighted equi-statistical convergence of the Korovkin-type approximation theorems. Results Math. 2017, 72, 1073–1085. [Google Scholar] [CrossRef]
- Dirik, F.; Demirci, K. Equi-ideal convergence of positive linear operators for analytic p-ideals. Math. Commun. 2011, 16, 169–178. [Google Scholar]
- Karakuş, S.; Demirci, K.; Duman, O. Equi-statistical convergence of positive linear operators. J. Math. Anal. Appl. 2008, 339, 1065–1072. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Alamri, B.A. Generalization of equi-statistical convergence via weighted lacunary sequence with associated Korovkin and Voronovskaya type approximation theorems. Rev. Real Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. 2019, 113, 1955–1973. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Srivastava, H.M.; Jena, B.B.; Paikray, S.K. A Certain Class of Equi-Statistical Convergence in the Sense of the Deferred Power-Series Method. Axioms 2023, 12, 964. https://doi.org/10.3390/axioms12100964
Srivastava HM, Jena BB, Paikray SK. A Certain Class of Equi-Statistical Convergence in the Sense of the Deferred Power-Series Method. Axioms. 2023; 12(10):964. https://doi.org/10.3390/axioms12100964
Chicago/Turabian StyleSrivastava, Hari Mohan, Bidu Bhusan Jena, and Susanta Kumar Paikray. 2023. "A Certain Class of Equi-Statistical Convergence in the Sense of the Deferred Power-Series Method" Axioms 12, no. 10: 964. https://doi.org/10.3390/axioms12100964
APA StyleSrivastava, H. M., Jena, B. B., & Paikray, S. K. (2023). A Certain Class of Equi-Statistical Convergence in the Sense of the Deferred Power-Series Method. Axioms, 12(10), 964. https://doi.org/10.3390/axioms12100964






