On the Distribution of Kurepa’s Function
Abstract
1. Introduction
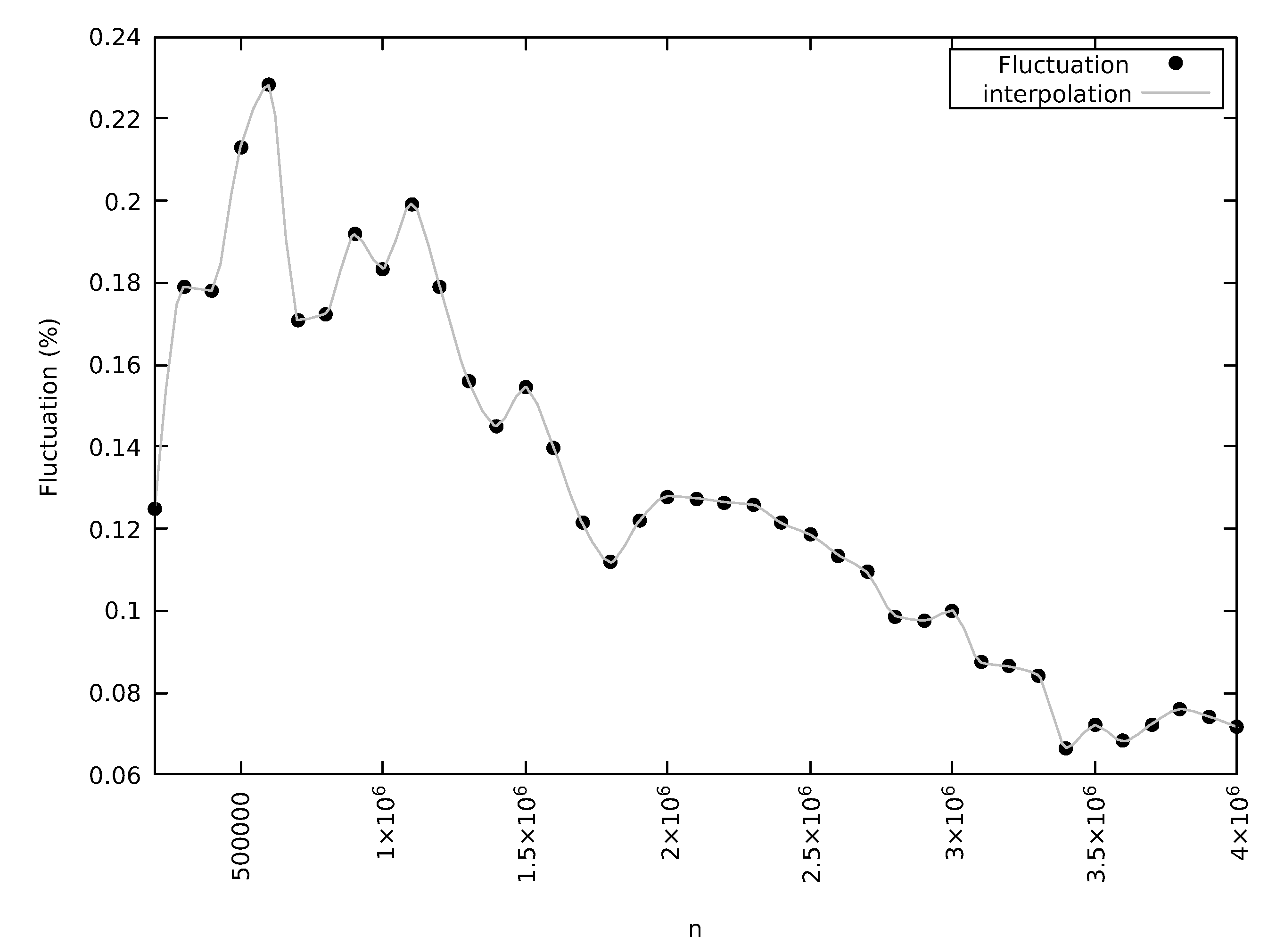
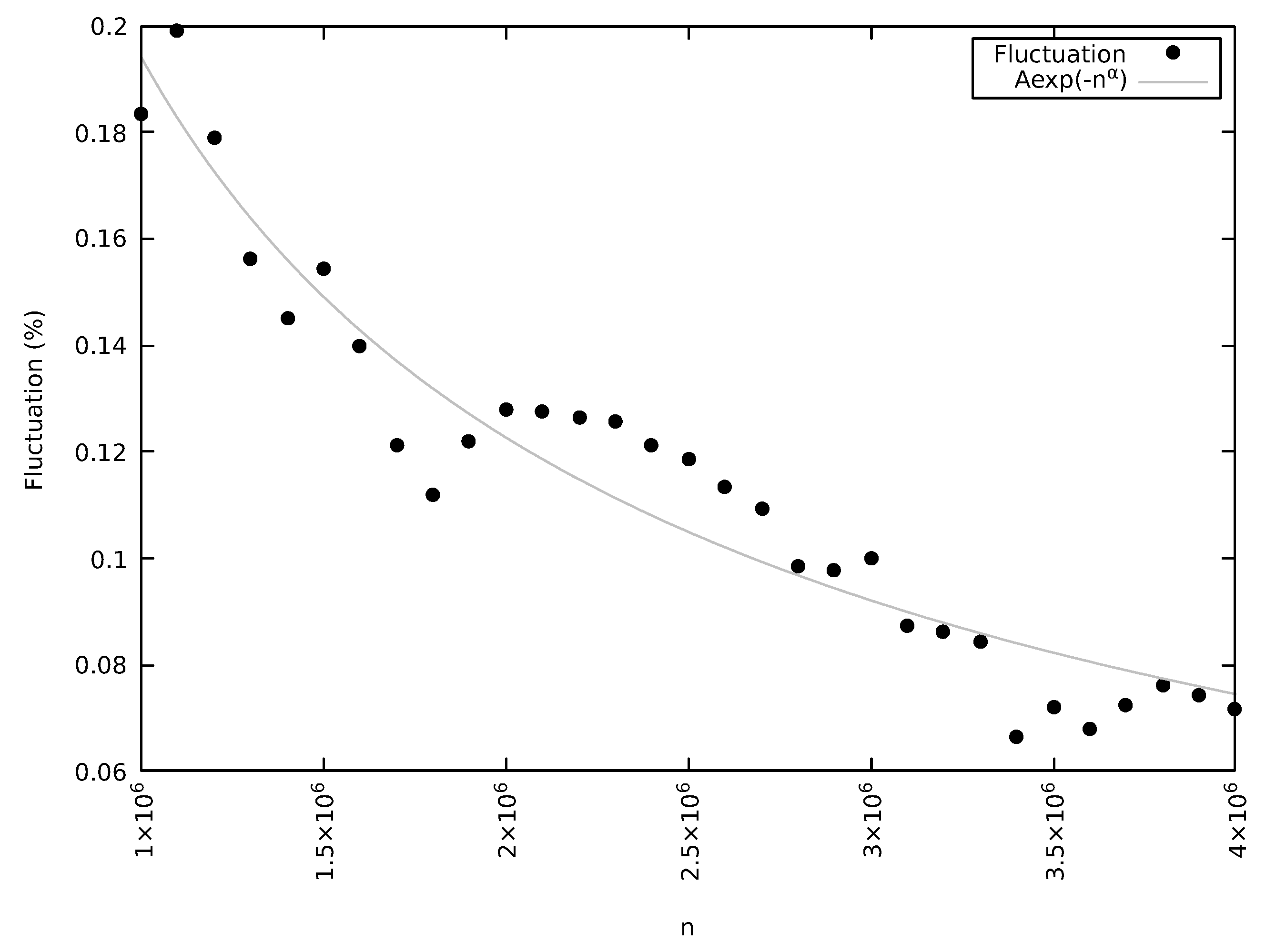
2. The Distribution Conjecture
3. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kurepa, Đ. On the left factorial function !N. Math. Balk. 1971, 1, 147–153. [Google Scholar]
- Kurepa, Đ. O faktorijelima konačnih i beskonačnih brojeva. Rad. Jugosl. Akad. Znan. Umjet. 1953, 296, 105–112. [Google Scholar]
- Kurepa, Đ. Uber die Faktoriellen der endlichen und unendlichen Zahlen. Ac. Sci. Yougosl. Cl. Math. 1954, 4, 51–64. [Google Scholar]
- Kurepa, Đ. Factorials of cardinal numbers and trees. Glasnik Mat. Fiz. Astr. 1964, 19, 7–21. [Google Scholar]
- Kurepa, Đ. Left factorial function in complex domain. Math. Balk. 1973, 3, 297–307. [Google Scholar]
- Ivić, A.; Mijajlovixcx, Ž. On Kurepa’s problems in number theory. Publ. Inst. Math. Beogr. 1995, 57, 19–28. [Google Scholar]
- Kurepa, Đ. Selected Papers of Đuro Kurepa; Ivić, A., Mamuzić, Z., Mijajlović, Ž., Todorčević, S., Eds.; Matematički Institut SANU: Belgrade, Serbia, 1996; Section F; p. 555. Available online: http://www.mi.sanu.ac.rs/novi_sajt/publications/data/ZarkoMijajlovicSelectedPapersOfDjuroKurepa.pdf (accessed on 23 June 2022).
- Mijajlović, Ž. Fifty Years of Kurepa’s !n Hypothesis; Bulletin T.CLIV de l’Académie Serbe des Sciences et des Arts—2021, Classe des Sciences Mathématiques et Naturelles, Sciences Mathématiques, No. 46; Académie Serbe des Sciences et des Arts: Belgrade, Serbia, 2021; pp. 169–181. Available online: http://elib.mi.sanu.ac.rs/files/journals/bltn/46/bltnn46p169-181.pdf (accessed on 23 June 2022).
- Fabiano, N.; Mirkov, N.; Mitrović, Z.D.; Radenović, S. On some new observations on Kurepa’s left factorial. Math. Anal. Contemp. Appl. 2022, 4, 1–8. [Google Scholar] [CrossRef]
- Fabiano, N.; Mirkov, N.; Mitrović, Z.D.; Radenović, S. A discussion on two old standing number theory problems: Collatz hypothesis, together with its relation to Planck’s black body radiation, and Kurepa’s conjecture on left factorial function. In Advances in Applied Analysis and Number Theory; Springer: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Barsky, D.; Benzaghou, B. Nombres de Bell et somme de factorielles. J. Theor. Nr. Bordx. 2004, 16, 1–17. [Google Scholar] [CrossRef]
- Barsky, D.; Benzaghou, B. Erratum a l’article Nombres de Bell et somme de factorielles. J. Theor. Nr. Bordx. 2011, 23, 527. [Google Scholar] [CrossRef]
- Andrejić, V.; Tatarević, M. Searching for a counterexample to Kurepa’s conjecture. Math. Comput. 2016, 85, 3061–3068. [Google Scholar] [CrossRef][Green Version]
- The PARI Group. PARI/GP Version 2.13.4, Univ. Bordeaux. 2022. Available online: http://pari.math.u-bordeaux.fr/ (accessed on 23 June 2022).

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fabiano, N.; Gardašević-Filipović, M.; Mirkov, N.; Todorčević, V.; Radenović, S. On the Distribution of Kurepa’s Function. Axioms 2022, 11, 388. https://doi.org/10.3390/axioms11080388
Fabiano N, Gardašević-Filipović M, Mirkov N, Todorčević V, Radenović S. On the Distribution of Kurepa’s Function. Axioms. 2022; 11(8):388. https://doi.org/10.3390/axioms11080388
Chicago/Turabian StyleFabiano, Nicola, Milanka Gardašević-Filipović, Nikola Mirkov, Vesna Todorčević, and Stojan Radenović. 2022. "On the Distribution of Kurepa’s Function" Axioms 11, no. 8: 388. https://doi.org/10.3390/axioms11080388
APA StyleFabiano, N., Gardašević-Filipović, M., Mirkov, N., Todorčević, V., & Radenović, S. (2022). On the Distribution of Kurepa’s Function. Axioms, 11(8), 388. https://doi.org/10.3390/axioms11080388






