Exact Solutions of Nonlinear Partial Differential Equations via the New Double Integral Transform Combined with Iterative Method

Abstract

This article demonstrates how the new Double Laplace–Sumudu transform (DLST) is successfully implemented in combination with the iterative method to obtain the exact solutions of nonlinear partial differential equations (NLPDEs) by considering specified conditions. The solutions of nonlinear terms of these equations were determined by using the successive iterative procedure. The proposed technique has the advantage of generating exact solutions, and it is easy to apply analytically on the given problems. In addition, the theorems handling the mode properties of the DLST have been proved. To prove the usability and effectiveness of this method, examples have been given. The results show that the presented method holds promise for solving other types of NLPDEs.

1. Introduction

Many nonlinear phenomena are an essential part of applied science and engineering. Nonlinear equations are observed in different types of physics-related problems: fluid dynamics, plasma physics, solid mechanics, quantum field theory, wave propagation in shallow water, and many others. The models are controlled in their range of validity by partial differential equations. The widespread use of these equations is the main reason why mathematicians have become aware of them. Nevertheless, it is neither numerically nor theoretically easy to find a solution to these mathematical problems. In the latest studies, much attention has been paid to obtaining exact or approximate solutions to these types of equations.

It is, therefore, becoming familiar with all analytical and numerical methods and the newly developed methods for solving nonlinear partial differential equations is increasingly important: for example, the Adomian decomposition method [1,2,3,4,5,6,7], the variational iteration method [8,9], the homotopy perturbation method [10], and the reduced differential transform method [11,12,13,14,15,16,17,18,19] and others [19,20,21,22].

Recently, a new double integral transform called DLST has been successfully implemented to solve some integral and partial differential equations [23,24,25,26]. Furthermore, in [27,28,29,30,31,32,33] an attractive formula for the DLST of the Caputo fractional derivative was obtained and used to construct a series for some families of linear fractional differential equations. Unfortunately, this transformation does not solve nonlinear problems or many complex mathematical models like other integral transforms. As a result, some researchers have combined these integral transforms with other methods such as the differential transform method, the homotopy perturbation method, the Adomian decomposition method, and the variational iteration method [34,35,36,37,38,39,40] for solving many nonlinear differential equations.

In the present study, we consider the general nonlinear partial differential equation, which covers the majority of the nonlinear partial differential equations solved in [30,32,33,35,36], of the following form:

$${\displaystyle \sum }_{n=0}^N{c}_n\frac{{\partial }^n\psi \left(y,t\right)}{\partial t^n}+{\displaystyle \sum }_{m=1}^M{d}_m\frac{{\partial }^m\psi \left(y,t\right)}{\partial y^m}+\mathcal{N}\left[\psi \left(y,t\right)\right]=g\left(y,t\right), \left(y,t\right)\in {ℝ}_{+}^2,$$

(1)

with the initial conditions (ICs)

$$\frac{{\partial }^n\psi \left(y,0\right)}{\partial t^n}=f_n\left(y\right),n=0,1,\dots ,N-1,y\in {ℝ}_{+},$$

(2)

and the conditions

$$\frac{{\partial }^m\psi \left(0,t\right)}{\partial y^m}=h_m\left(t\right),m=0,1,\dots ,M-1,t\in {ℝ}_{+},$$

(3)

where $c_n,0\le n\le N$ and $d_m,1\le m\le M$ are the given coefficients and $N\mathrm{and} M$ are positive integers. $\mathcal{N}\left[\psi \left(y,t\right)\right]$ is nonlinear term, and $g\left(x,t\right)$ is the source term in the following form $g\left(x,t\right)=g_1\left(x,t\right)+g_2\left(x,t\right)$.

The main aim of this study is to use the DLST method, including the new iterative method (NIM) planned by Daftardar-Gejji and Jafari in [39], to seek out an exact solution of the nonlinear partial differential equations of type (1) subject to ICs (2) and the conditions (3). The new iterative method (NIM) has been extensively employed by several researchers for the treatment of linear and nonlinear ordinary and partial differential equations of integer and fractional order (see [41,42,43,44,45]). The advantage of the DLST method coupled with the iterative method is that, compared to other known methods (see [45,46]), it provides fast convergence of the exact solution without any restrictive assumptions about the solution. The aim of this study is to present a faster method to find the exact solution of nonlinear PDE via DLST.

The following things will be discussed in the remaining parts of this paper: Section 2 includes basic definitions, properties, and theorems of the DLST. Section 3 of this paper provides the description of the model and the method for obtaining exact analytical solutions of the given nonlinear PDEs using the DLST coupled with an iterative method. Section 4 shows the application of the proposed method to six illustrative examples in order to show its liability, convergence, and efficiency. Finally, Section 5 contains concluding remarks.

2. Basic Definitions and Theorems

This section covers basic definitions of the DLST for some functions of two variables, the existence and the uniqueness conditions for the DLST, and some properties of the DLST for derivatives. For more details about DLST, see [24,25,26].

Definition 1.

Let$\psi \left(y,t\right)$a piecewise function defined on a region$\left[0,A\right]\times \left[0,B\right]$, then the DLST of$\psi \left(y,t\right)$is denoted by$L_y{S}_t \left[\psi \left(y,t\right)\right]=\tilde{\psi }\left(\upsilon ,\omega \right)$and is defined as follows:

$$L_y{S}_t\left[\psi \left(y,t\right)\right]=\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\psi \left(y,t\right)dydt,$$

(4)

where $y,t\ge 0$, and $v$and $\omega$ are complex variables, provided that the integral exists.

The relationship below clearly demonstrates the linearity of DLST:

$$\begin{array}{cc}\hfill L_y{S}_t[\lambda \psi \left(y,t\right)+& \mu \zeta \left(y,t\right)]=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\left[\lambda \psi \left(y,t\right)+\mu \zeta \left(y,t\right)\right]dydt\hfill \\ \hfill & =\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\lambda \psi \left(y,t\right)dydt+\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\mu \zeta \left(y,t\right)dydt\hfill \\ \hfill & =\frac{\lambda }{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\psi \left(y,t\right)dydt+\frac{\mu }{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\zeta \left(y,t\right)dydt\hfill \\ \hfill & =\lambda L_y{S}_t\left[\psi \left(y,t\right)\right]+\mu L_y{S}_t\left[\zeta \left(y,t\right)\right].\hfill \end{array}$$

(5)

where $\lambda$and $\mu$ are nonzero constants.

Definition 2.

The inverse DLST$L_y^{-1} S_t^{-1} \left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]=\psi \left(y,t\right)$is defined by the following.

$$L_y^{-1}{S}_t^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]=\psi \left(y,t\right)=\frac{1}{2\pi i}{{\displaystyle \int }}_{\gamma -i\infty }^{\gamma +i\infty }{e}^{\upsilon y}d\upsilon \frac{1}{2\pi i}{{\displaystyle \int }}_{\eta -i\infty }^{\eta +i\infty }\frac{1}{\omega }{e}^{\frac{t}{\omega }}\tilde{\psi }\left(\upsilon ,\omega \right)d\omega .$$

(6)

2.1. Fundamental Properties of the DLST

In

Table 1. The DLST for some functions of two variables [24,25].

$\psi \left(y,t\right)$	$L_y{S}_t\left[\psi \left(y,t\right)\right]=\tilde{\psi }\left(\upsilon ,\omega \right)$
$1$	$\frac{1}{\upsilon }$
$y^c{t}^d$, if $c$ and $d$ are positive integral	$\frac{c!d!}{{\upsilon }^{c+1}}{\omega }^d$
$e^{cy+dt}$	$\frac{1}{\left(\upsilon -c\right)\left(1-d\omega \right)}$
$\sin (cy+dt)$	$\frac{c+d\upsilon \omega }{\left({\upsilon }^2+c^2\right)\left(1+d^2{\omega }^2\right)}$
$\cos (cy+dt)$	$\frac{\upsilon -cd\omega }{\left({\upsilon }^2+c^2\right)\left(1+d^2{\omega }^2\right)}$
$\sin \mathrm{h}(cy+dt)$	$\frac{c+d\upsilon \omega }{\left({\upsilon }^2-c^2\right)\left(1-d^2{\omega }^2\right)}$
$\cos \mathrm{h}(cy+dt)$	$\frac{\upsilon +cd\omega }{\left({\upsilon }^2-c^2\right)\left(1-d^2{\omega }^2\right)}$
$J_0\left(c\sqrt{yt}\right)$, where $J_0\left(y\right)$ is the modified Bessel function of order zero	$\frac{4}{4\upsilon +\omega c^2}$
$f\left(y\right)g\left(t\right)$	$L_y\left[f\left(y\right)\right]S_t\left[g\left(t\right)\right]$

, we introduce the DLST for some functions of two variables, which can be found in [24,25].

2.2. Existence and Uniqueness Conditions for the DLST

If function $\psi \left(y,t\right)$ is of exponential order $c$ and $d$ at $y\to \infty$ and $t\to \infty$, then there exist a nonnegative constant $K$ such that $\forall y>Y$ and $t>T$; we have the following:

$$\left|\psi \left(y,t\right)\right|\le K{e}^{cy+dt},$$

and we write the following:

$$\psi \left(y,t\right)=O\left(e^{cy+dt}\right)$$

as $y \mathrm{and} t$ tend to infinity or the following is obtained.

$$\underset{y\to \infty ,t\to \infty }{\lim }{e}^{-\upsilon y-\frac{t}{\omega }}\left|\psi \left(y,t\right)\right|=K\underset{y\to \infty ,t\to \infty }{\lim }{e}^{-\left(\upsilon -c\right)y-\left(\frac{1}{\omega }-d\right)t}=0,\upsilon >c,\frac{1}{\omega }>d.$$

Then, $\psi$ is the exponential order as $y \mathrm{and}t$ tend to infinity.

Theorem 1.

(Existence). Let$\psi \left(y,t\right)$be defined on region$\left(0,Y\right)\times \left(0,T\right)$of exponential order$c$and$d$, then the DLST of$\psi \left(y,t\right)$defined for all$\upsilon$ and $\frac{1}{\omega }$ supplied $Re[\upsilon ]>c$ and $Re\left[\frac{1}{\omega }\right]>d$.

Proof of Theorem 1.

We find, from definition 1, the following.

$$\begin{array}{c}\left|\tilde{\psi }\left(\upsilon ,\omega \right)\right|=\left|\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\psi \left(y,t\right)dydt\right|\le K\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\left(\upsilon -c\right)y}dy\underset{0}{\overset{\infty }{{\displaystyle \int }}}\frac{1}{\omega }{e}^{-\left(\frac{1}{\omega }-d\right)t}dt\\ =\frac{K}{\left(\upsilon -c\right)\left(1-d\omega \right)},Re[\upsilon ]>c,Re\left[\frac{1}{\omega }\right]>d.\end{array}$$

(7)

Thus, from Equation (7), we obtain the following.

$$\underset{y\to \infty ,t\to \infty }{\lim }\left|\tilde{\psi }\left(\upsilon ,\omega \right)\right|=0, \mathrm{or} \underset{y\to \infty ,t\to \infty }{\lim }\tilde{\psi }\left(\upsilon ,\omega \right)=0.$$

□

Theorem 2.

(Uniqueness). Let${\tilde{\varphi }}_1\left(\upsilon ,\omega \right)$and${\tilde{\varphi }}_2\left(\upsilon ,\omega \right)$be the DLST of the continuous functions${\varphi }_1\left(y,t\right)$and${\varphi }_2\left(y,t\right)$defined for$y$, $t\ge 0$, respectively. If${\tilde{\varphi }}_1\left(\upsilon ,\omega \right)={\tilde{\varphi }}_2\left(\upsilon ,\omega \right)$, then${\varphi }_1\left(y,t\right)={\varphi }_2\left(y,t\right) .$

In the following arguments, we present some properties of the DLST for derivatives.

2.3. Properties of Derivatives

Let $\tilde{\psi }\left(\upsilon ,\omega \right)=L_y{S}_t\left[\psi \left(y,t\right)\right]$, then the following is the case.

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial y}\right]=\upsilon \tilde{\psi }\left(\upsilon ,\omega \right)-S\left[\psi \left(0,t\right)\right].$$

(8)

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial t}\right]=\frac{1}{\omega }\tilde{\psi }\left(\upsilon ,\omega \right)-\frac{1}{\omega }L\left(\psi \left(y,0\right)\right)$$

(9)

$$L_y{S}_t\left[\frac{{\partial }^2\psi \left(y,t\right)}{\partial y^2}\right]={\upsilon }^2\tilde{\psi }\left(\upsilon ,\omega \right)-\upsilon S\left(\psi \left(0,t\right)\right)-S\left({\psi }_y\left(0,t\right)\right)$$

(10)

$$L_y{S}_t\left[\frac{{\partial }^2\psi \left(y,t\right)}{\partial t^2}\right]=\frac{1}{{\omega }^2}\tilde{\psi }\left(\upsilon ,\omega \right)-\frac{1}{{\omega }^2}L\left(\psi \left(y,0\right)\right)-\frac{1}{\omega }L\left({\psi }_t\left(y,0\right)\right).$$

(11)

Proof. 

In order to prove Equation (8), consider the following.

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial y}\right]=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\frac{\partial \psi \left(y,t\right)}{\partial y}dydt=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\frac{t}{\omega }}dt\left\{\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y}\frac{\partial \psi \left(y,t\right)}{\partial y}dy\right\}.$$

Let $u=e^{-\upsilon y},dv=\frac{\partial \psi \left(y,t\right)}{\partial y}dy$; thus, we have the following.

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial y}\right]=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\frac{t}{\omega }}dt\left\{-\psi \left(0,t\right)+\upsilon \underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y}\psi \left(y,t\right)dy\right\}=\upsilon \tilde{\psi }\left(\upsilon ,\omega \right)-S\left(\psi \left(0,t\right)\right).$$

For Equation (9), we obtain the following.

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial t}\right]=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y-\frac{t}{\omega }}\frac{\partial \psi \left(y,t\right)}{\partial t}dydt=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y}dy\left\{\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\frac{t}{\omega }}\frac{\partial \psi \left(y,t\right)}{\partial t}dt\right\}.$$

Let $u=e^{-\frac{t}{\omega }},dv=\frac{\partial \psi \left(y,t\right)}{\partial t}dt,$ then

$$L_y{S}_t\left[\frac{\partial \psi \left(y,t\right)}{\partial t}\right]=\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\upsilon y}dy\left\{-\psi \left(y,0\right)+\frac{1}{\omega }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\frac{t}{\omega }}\psi \left(y,t\right)dt\right\}=\frac{1}{\omega }\tilde{\psi }\left(\upsilon ,\omega \right)-\frac{1}{\omega }L\left(\psi \left(y,0\right)\right).$$

It is simple to demonstrate Equations (10) and (11).

In general, the above results can be extended as follows, and the proof can be shown by mathematical induction.

$$L_y{S}_t\left[\frac{{\partial }^n\psi \left(y,t\right)}{\partial t^n}\right]={\omega }^{-n}\tilde{\psi }\left(\upsilon ,\omega \right)-{\displaystyle \sum }_{j=0}^{n-1}{\omega }^{-n+j}{L}_y\left[\frac{{\partial }^j\psi \left(y,0\right)}{\partial t^j}\right].$$

(12)

$$L_y{S}_t\left[\frac{{\partial }^m\psi \left(y,t\right)}{\partial y^m}\right]={\upsilon }^m\tilde{\psi }\left(\upsilon ,\omega \right)-{\displaystyle \sum }_{k=0}^{m-1}{\upsilon }^{m-1-k}{S}_t\left[\frac{{\partial }^k\psi \left(0,t\right)}{\partial y^k}\right].$$

(13)

□

The following results are some properties of the DLST and that can be found in [14,15,16].

Property 1.

(Changing of scale property). If$\tilde{\psi }\left(\upsilon ,\omega \right)=L_y{S}_t\left[\psi \left(y,t\right)\right]$, then we have the following.

$$L_y{S}_t\left[\psi \left(cy,dt\right)\right]=\frac{1}{cd}\tilde{\psi }\left(\frac{\upsilon }{c},\frac{\omega }{d}\right).$$

(14)

Proof. 

According to definition 1, the following is the case.

$$L_y{S}_t\left[\psi \left(cy,dt\right)\right]=\frac{1}{\omega }{{\displaystyle \int }}_0^{\infty }{{\displaystyle \int }}_0^{\infty }{e}^{-\upsilon y-\frac{t}{\omega }}\psi \left(cy,dt\right)dydt.$$

(15)

Suppose $x=cy$ and $z=dt$ in Equation (15), we obtain the following.

$$L_y{S}_t\left[\psi \left(cy,dt\right)\right]=\frac{1}{cd}{{\displaystyle \int }}_0^{\infty }{{\displaystyle \int }}_0^{\infty }\frac{1}{\omega }{e}^{-\upsilon \frac{x}{c}-\frac{z}{d\omega }}\psi \left(x,z\right)dxdz=\frac{1}{cd}\tilde{\psi }\left(\frac{\upsilon }{c},\frac{\omega }{d}\right).$$

□

Property 2.

(First shifting property). If$\tilde{\psi }\left(\upsilon ,\omega \right)=L_y{S}_t\left[\psi \left(y,t\right)\right]$, then the following is obtained.

$$L_y{S}_t\left[e^{cy+dt}\psi \left(y,t\right)\right]=\frac{1}{1-d\omega }\tilde{\psi }\left(\upsilon -c,\frac{\omega }{1-d\omega }\right).$$

(16)

For proof, see [24].

Property 3.

(Second shifting property). If $\tilde{\psi }\left(\upsilon ,\omega \right)=L_y{S}_t\left[\psi \left(y,t\right)\right]$, then the following is obtained.

$$L_y{S}_t\left[\psi \left(y-\delta ,t-\epsilon \right)H\left(y-\delta ,t-\epsilon \right)\right]=e^{-\upsilon \delta -\frac{\epsilon }{\omega }}\tilde{\psi }\left(\upsilon ,\omega \right),$$

(17)

$$H\left(y-\delta ,t-\epsilon \right)=\left\{\begin{array}{c}1,y>\delta ,t>\epsilon \\ 0,otherwise\end{array}\right\}.$$

(18)

For the proof, see [24,25].

Property 4.

(Convolution).

If$L_y{S}_t\left[\varphi \left(y,t\right)\right]=\tilde{\varphi }\left(\upsilon ,\omega \right)$ and  $L_y{S}_t\left[\psi \left(y,t\right)\right]=\tilde{\psi }\left(\upsilon ,\omega \right)$, then the following is the case:

$$L_y{S}_t\left[\left(\varphi \ast \ast \psi \right)\left(y,t\right)\right]=\omega \tilde{\varphi }\left(\upsilon ,\omega \right)\tilde{\psi }\left(\upsilon ,\omega \right).$$

(19)

where the following is obtained.

$$\left(\varphi \ast \ast \psi \right)\left(y,t\right)=\underset{0}{\overset{y}{{\displaystyle \int }}}\underset{0}{\overset{t}{{\displaystyle \int }}}\varphi \left(y-\delta ,t-\epsilon \right)\psi \left(\delta ,\epsilon \right)d\delta d\epsilon .$$

(20)

For the proof, see [14,15,17].

3. Principle of the DLST-Iterative (DLST-I) Method

In this section, we introduce a new approach for solving PDEs, which is the DLST-I method. The main idea of this technique is to apply DLST on the given PDE to obtain the equation in a new space. Then, we use the iterative method to decompose nonlinear terms and solve the equation.

Finally, we apply the inverse DLAT to obtain the solution of the target equation in the original space.

Applying DLST on Equation (1), we obtain the following.

$$\begin{array}{cc}\hfill {\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\displaystyle [}{\omega }^{-n}\tilde{\psi }\left(\upsilon ,\omega \right)& -{\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\omega }^{-n+j}{L}_y\left[\frac{{\partial }^j\psi \left(y,0\right)}{\partial t^j}\right]]+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left[{\upsilon }^m\tilde{\psi }\left(\upsilon ,\omega \right)-{\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}{S}_t\left[\frac{{\partial }^k\psi \left(0,t\right)}{\partial y^k}\right]\right]\hfill \\ \hfill & +L_y{S}_t\left[N\psi \left(y,t\right)\right]=\widetilde{g_1}\left(\upsilon ,\omega \right)+L_y{S}_t\left[g_2\left(y,t\right)\right].\hfill \end{array}$$

(21)

Using the single (LT) for ICs (2) and the single (ST) for the conditions in Equation (3), we obtain the following.

$$\begin{array}{cc}\hfill L_y\left[\frac{{\partial }^n\psi \left(y,0\right)}{\partial t^n}\right]=& \overline{f_n}\left(\upsilon \right),n=0,1,\dots ,N-1,S_t\left[\frac{{\partial }^m\psi \left(0,t\right)}{\partial y^m}\right]=\overline{h_m}\left(\omega \right),\hfill \\ \hfill & m=0,1,\dots ,M-1.\hfill \end{array}$$

(22)

By substituting Equation (22) in Equation (21), we have the following.

$$\begin{array}{cc}\hfill {\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n[{\omega }^{-n}\tilde{\psi }\left(\upsilon ,\omega \right)& -{\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\omega }^{-n+j}\overline{f_j}\left(\upsilon \right)]\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left[{\upsilon }^m\tilde{\psi }\left(\upsilon ,\omega \right)-{\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right]\hfill \\ & =\widetilde{g_1}\left(\upsilon ,\omega \right)+L_y{S}_t\left[g_2\left(y,t\right)-\mathcal{N}\left[\psi \left(y,t\right)\right]\right].\hfill \end{array}$$

(23)

Simplifying Equation (23), we obtain the following.

$$\begin{array}{cc}\hfill \tilde{\psi }\left(\upsilon ,\omega \right)& ={\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}\{{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n\left({\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\sigma }^{-n+j}\overline{f_j}\left(\upsilon \right)\right)\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left({\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right)+\widetilde{g_1}\left(\upsilon ,\omega \right)\}\hfill \\ & +{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}{L}_y{S}_t\left[g_2\left(y,t\right)-\mathcal{N}\left[\psi \left(y,t\right)\right]\right].\hfill \end{array}$$

(24)

Taking the inverse LSTD $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ of Equation (24), we obtain the following.

$$\begin{array}{cc}\hfill \psi \left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}& [{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}\{{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n\left({\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\sigma }^{-n+j}\overline{f_j}\left(\upsilon \right)\right)\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left({\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right)+\widetilde{g_1}\left(\upsilon ,\omega \right)\}].\hfill \\ & +L_y{}^{-1}{S}_t{}^{-1}\left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}{L}_y{S}_t\left[g_2\left(y,t\right)-\mathcal{N}\left[\psi \left(y,t\right)\right]\right]\right].\hfill \end{array}$$

(25)

Now, use the iterative approach by assuming the following.

$$\psi \left(y,t\right)={\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}{\psi }_i\left(y,t\right).$$

(26)

Substituting Equation (26) in Equation (25), we obtain the following.

$$\begin{array}{cc}\hfill {\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}{\psi }_i\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}& \left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}\right\{{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n\left({\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\sigma }^{-n+j}\overline{f_j}\left(\upsilon \right)\right)\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left({\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right)+\widetilde{g_1}\left(\upsilon ,\omega \right)\left\}\right]\hfill \\ & +L_y{}^{-1}{S}_t{}^{-1}\left({\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}{L}_y{S}_t\left[g_2\left(y,t\right)-\mathcal{N}\left[\psi \left(y,t\right)\right]\right]\right).\hfill \end{array}$$

(27)

$\mathcal{N}\left[\psi \left(y,t\right)\right]$ is a nonlinear term that can be decomposed into the following.

$$\mathcal{N}\left[{\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}{\psi }_i\left(y,t\right)\right]=\mathcal{N}\left[{\psi }_0\left(y,t\right)\right]+{\displaystyle {\displaystyle \sum }_{i=1}^{\infty }}\left(\mathcal{N}\left[{\displaystyle {\displaystyle \sum }_{k=0}^i}{\psi }_k\left(y,t\right)\right]-\mathcal{N}\left[{\displaystyle {\displaystyle \sum }_{k=0}^{i-1}}{\psi }_k\left(y,t\right)\right]\right).$$

(28)

Substituting Equation (28) in Equation (27), we obtain the following.

$$\begin{array}{cc}\hfill {\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}{\psi }_i\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}& \left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}\right\{{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n\left({\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\sigma }^{-n+j}\overline{f_j}\left(\upsilon \right)\right)\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left({\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right)+\widetilde{g_1}\left(\upsilon ,\omega \right)\left\}\right]\hfill \\ & +L_y{}^{-1}{S}_t{}^{-1}\left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}{L}_y{S}_t\left[g_2\left(y,t\right)-\mathcal{N}\left({\varphi }_0\left(y,t\right)\right)\right]\right]\hfill \\ & -L_y{}^{-1}{S}_t{}^{-1}[[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m{]}^{-1}{L}_y{S}_t\left[{\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}\left[N\left({\displaystyle {\displaystyle \sum }_{k=0}^i}{\psi }_k\left(y,t\right)\right)-\mathcal{N}\left({\displaystyle {\displaystyle \sum }_{k=0}^{i-1}}{\psi }_k\left(y,t\right)\right)\right]\right]].\hfill \end{array}$$

(29)

Following that, we obtain the recurrence relations as follows.

$$\begin{array}{cc}\hfill {\psi }_0\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}& \left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}\right\{{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n\left({\displaystyle {\displaystyle \sum }_{j=0}^{n-1}}{\sigma }^{-n+j}\overline{f_j}\left(\upsilon \right)\right)\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m\left({\displaystyle {\displaystyle \sum }_{k=0}^{m-1}}{\upsilon }^{m-1-k}\overline{h_k}\left(\omega \right)\right)+\widetilde{g_1}\left(\upsilon ,\omega \right)\left\}\right],\hfill \end{array}$$

(30)

$$\begin{array}{cc}\hfill {\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}& \left[{\left[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}+{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m\right]}^{-1}{L}_y{S}_t\right[g_2\left(y,t\right)-\mathcal{N}\left({\varphi }_0\left(y,t\right)\right)\left]\right],\hfill \end{array}$$

(31)

$$\begin{array}{cc}\hfill {\varphi }_{r+1}\left(x,t\right)& =-L_y{}^{-1}{S}_t{}^{-1}\left[\right[{\displaystyle {\displaystyle \sum }_{n=0}^N}{c}_n{\omega }^{-n}\hfill \\ & +{\displaystyle {\displaystyle \sum }_{m=1}^M}{d}_m{\upsilon }^m{]}^{-1}{L}_y{S}_t\left[{\displaystyle {\displaystyle \sum }_{i=0}^{\infty }}\right[\mathcal{N}\left({\displaystyle {\displaystyle \sum }_{k=0}^i}{\psi }_k\left(y,t\right)\right)\hfill \\ & -\mathcal{N}\left({\displaystyle {\displaystyle \sum }_{k=0}^{i-1}}{\psi }_k\left(y,t\right)\right)\left]\right]],r\ge 1.\hfill \end{array}$$

(32)

As a result, we have the series solution of Equation (1), which is stated as follows.

$$\psi \left(y,t\right)={\psi }_0\left(y,t\right)+{\psi }_1\left(y,t\right)+{\psi }_2\left(y,t\right)+\cdots .$$

(33)

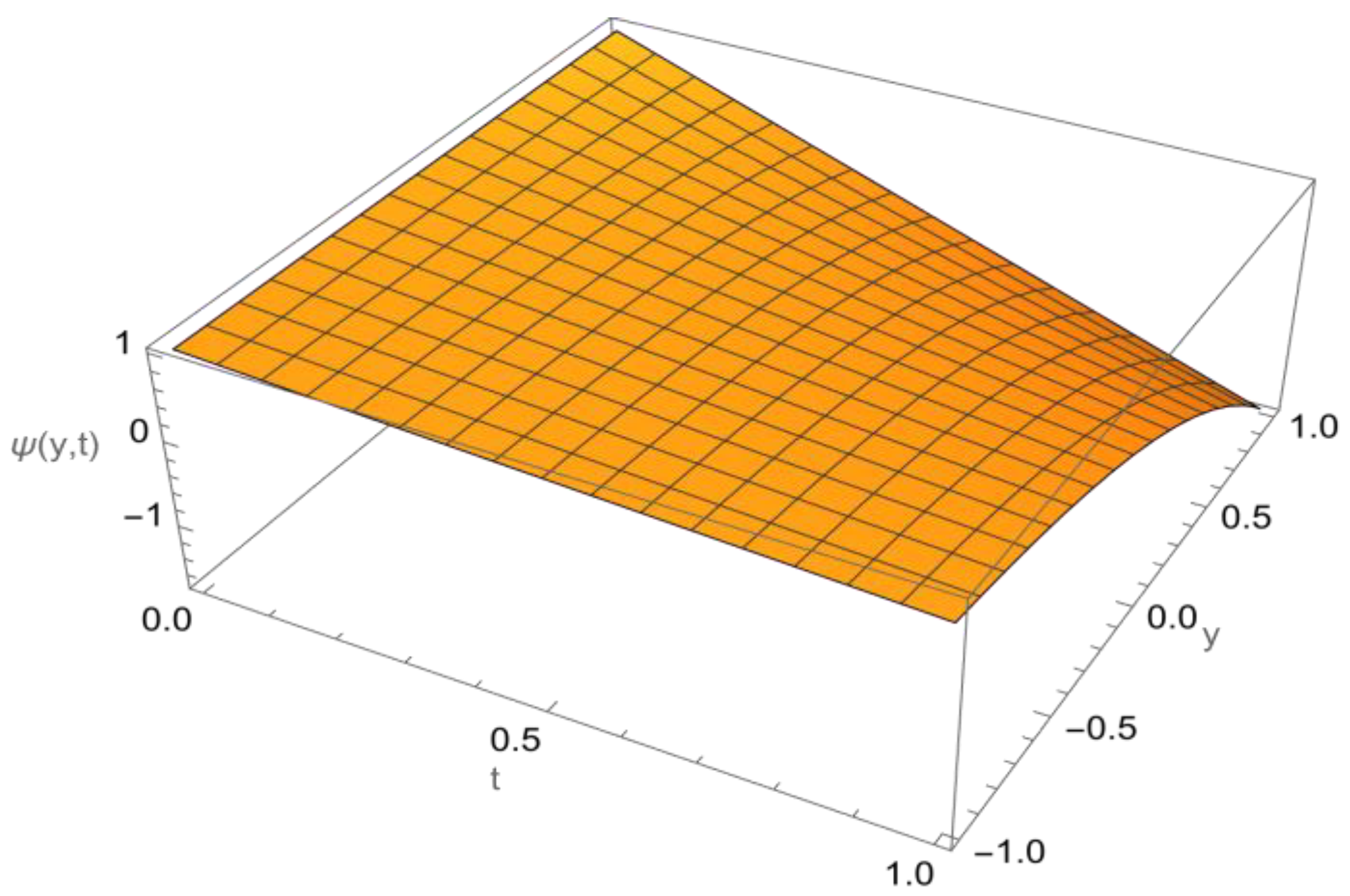
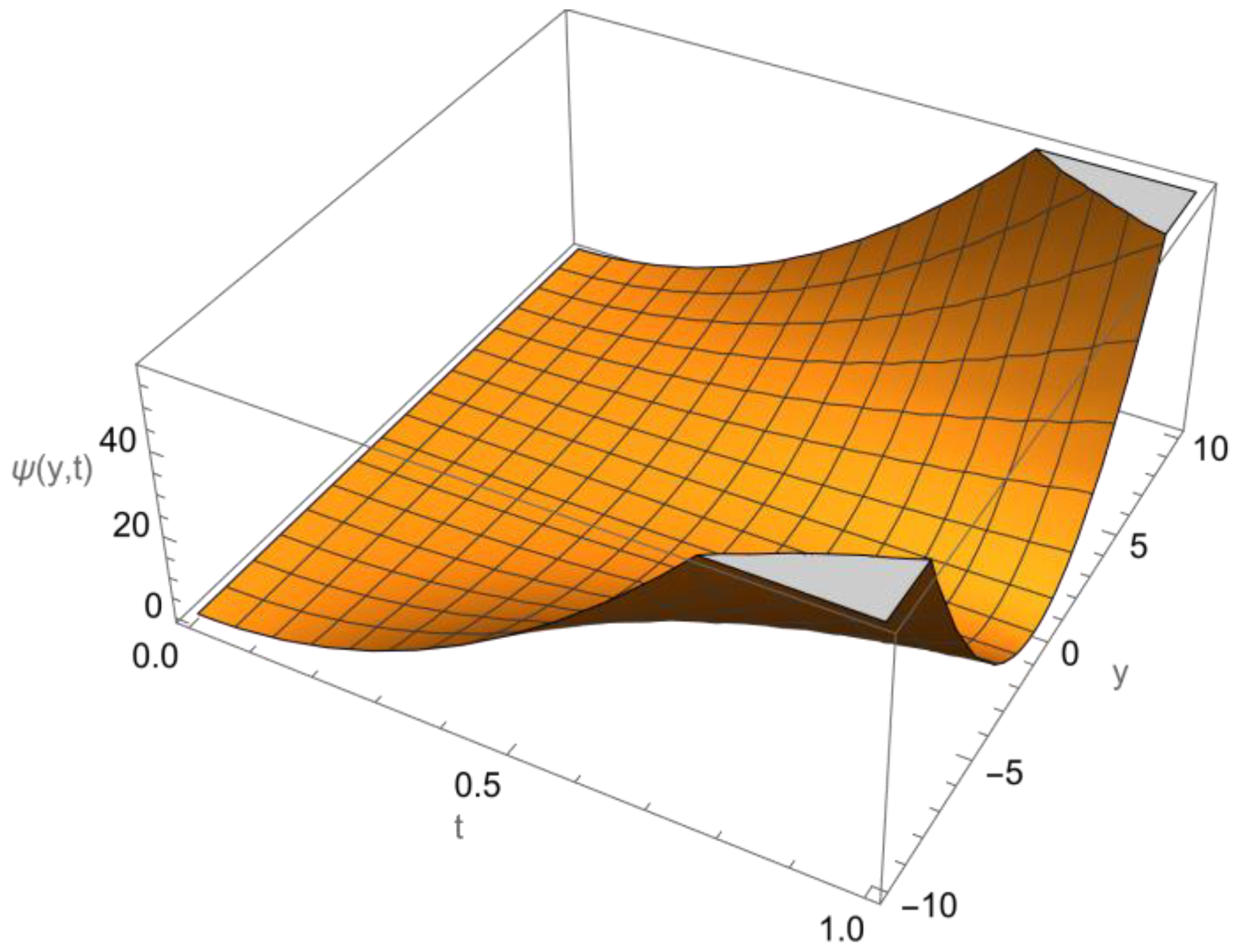
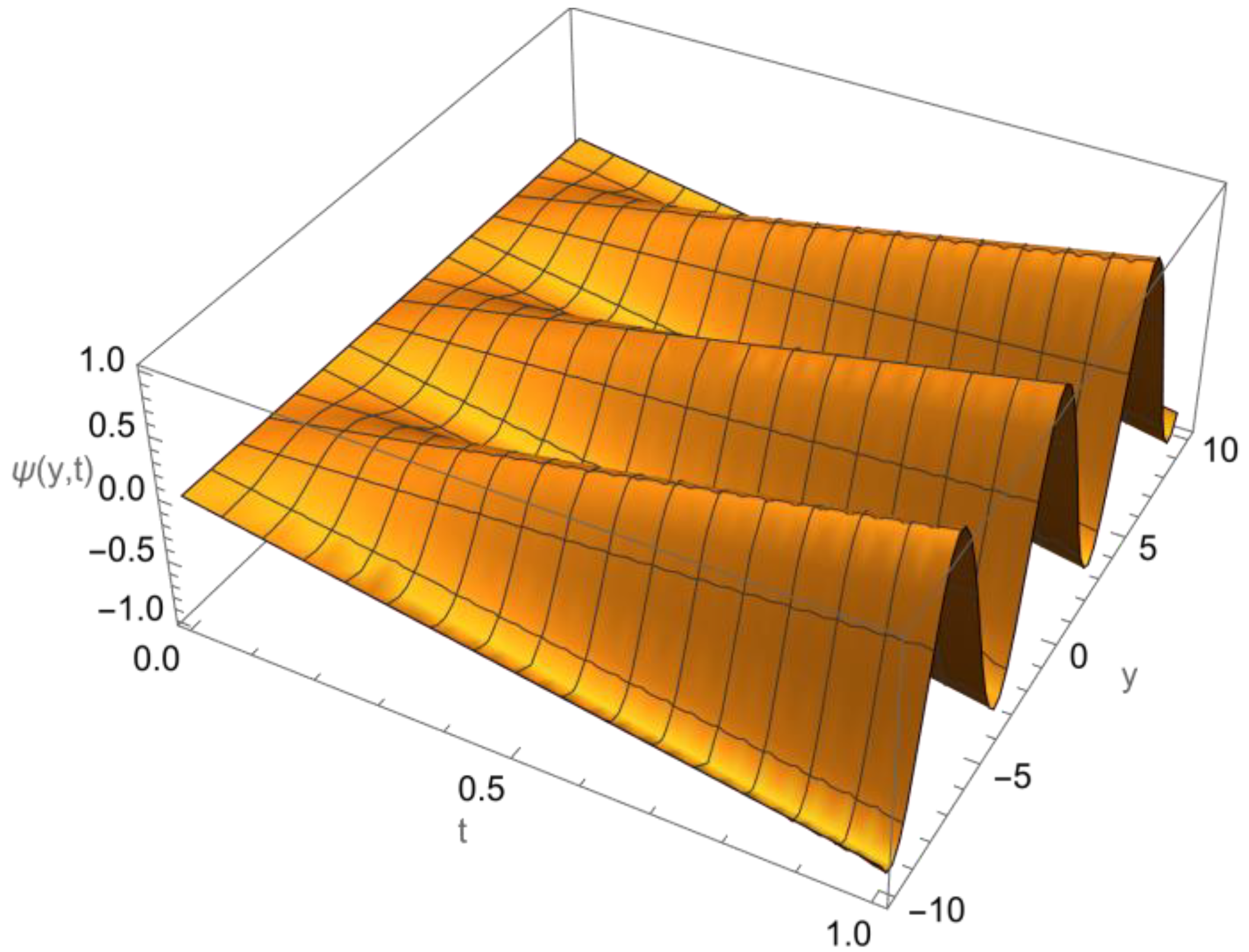
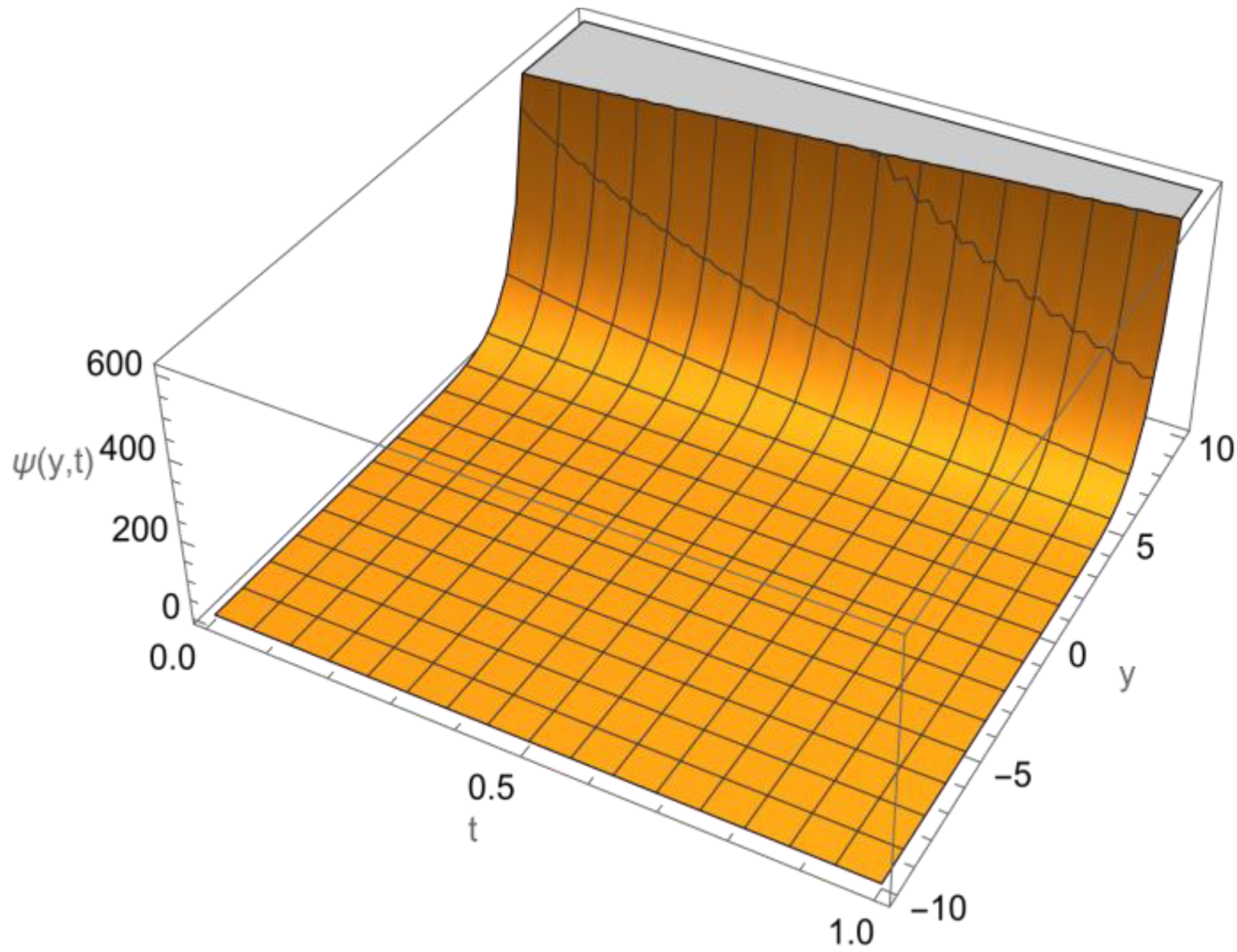
4. Elucidative Examples

In this section, six interesting examples of nonlinear partial differential equations are solved to demonstrate the performance and efficiency of the DLST-I method.

Example 1.

Consider the nonlinear Dissipative wave equation [1]:

$$\frac{{\partial }^2\psi }{\partial t^2}-\frac{{\partial }^2\psi }{\partial y^2}+\frac{\partial }{\partial t}\left(\psi {\psi }_y\right)=2e^{-t}\sin y-2e^{-2t}\sin y\cos y,$$

(34)

with ICs

$$\psi \left(y,0\right)=\sin y,{\psi }_t\left(y,0\right)=-\sin y,$$

(35)

and the following conditions.

$$\psi \left(0,t\right)=0,{\psi }_y\left(0,t\right)=e^{-t}.$$

(36)

Solution. 

Applying the DLST on Equation (34) and the single (LT) on Equation (35) and the single (ST) on Equation (36), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{1}{\left({\upsilon }^2+1\right)\left(1+\omega \right)}-\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[2e^{-2t}\sin y\cos y+\frac{\partial }{\partial t}\left(\psi {\psi }_y\right)\right].$$

(37)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (37), we obtain the following.

$$\psi \left(y,t\right)=e^{-t}\sin y-L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[2e^{-2t}\sin y\cos y+\frac{\partial }{\partial t}\left(\psi {\psi }_y\right)\right]\right].$$

(38)

Now, using the iterative approach, substitute Equation (26) in Equation (38) and use the formulas in Equations (30)–(32); we obtain the following solution components.

$${\psi }_0\left(y,t\right)=e^{-t}\sin y,$$

(39)

$${\psi }_1\left(y,t\right)=-L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[2e^{-2t}\sin y\cos y+\frac{\partial }{\partial t}\left[\left({\psi }_0\right){\left({\psi }_0\right)}_y\right]\right]\right]=0,$$

(40)

$${\psi }_2\left(y,t\right)=-L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[\frac{\partial }{\partial t}\left[\left({\psi }_0+{\psi }_1\right){\left({\psi }_0+{\psi }_1\right)}_y-\frac{\partial }{\partial t}\left[\left({\psi }_0\right){\left({\psi }_0\right)}_y\right]\right]\right]\right]=0.$$

(41)

As a result, we have the solution of Equation (34) as follows.

$$\psi \left(y,t\right)=e^{-t}\sin y.$$

(42)

Figure 1 below, shows the solution of the initial value problem (34) and (35). □

Example 2.

Consider the following nonhomogeneous KdV equation [5]:

$$\frac{\partial \psi }{\partial t}-\psi \frac{\partial \psi }{\partial y}+\frac{{\partial }^3\psi }{\partial y^3}=-e^y\left(t+1\right)+t{e}^y\left(-t{e}^y+1\right),$$

(43)

with IC

$$\psi \left(y,0\right)=1.$$

(44)

and the following conditions.

$$\psi \left(0,t\right)=1-t, {\psi }_y\left(0,t\right)={\psi }_{yy}\left(0,t\right)=-t.$$

(45)

Solution. 

Running the DLST on Equation (43) and the single (LT) on Equation (44) and the single (ST) on Equation (45), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{1}{\upsilon }-\frac{\omega }{\left(\upsilon -1\right)}+\frac{\omega }{\left(1+\omega {\upsilon }^3\right)}{L}_y{S}_t\left[t{e}^y\left(-t{e}^y+1\right)+\psi \frac{\partial \psi }{\partial y}\right].$$

(46)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (46), we obtain the following.

$$\psi \left(y,t\right)=1-t{e}^y+L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{\left(1+\omega {\upsilon }^3\right)}{L}_y{S}_t\left[t{e}^y\left(-t{e}^y+1\right)+\psi \frac{\partial \psi }{\partial y}\right]\right].$$

(47)

Using the iterative approach, substitute Equation (26) in Equation (47) and use Formulas (30)–(32); we obtain the following solution components.

$${\psi }_0\left(y,t\right)=1-t{e}^y,$$

(48)

$${\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{\left(1+\omega {\upsilon }^3\right)}{L}_y{S}_t\left[t{e}^y\left(-t{e}^y+1\right)+{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0,$$

(49)

$${\psi }_2\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{\left(1+\omega {\upsilon }^3\right)}{L}_y{S}_t\left[\left({\psi }_0+{\psi }_1\right)\frac{\partial \left({\psi }_0+{\psi }_1\right)}{\partial y}-{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0,$$

(50)

As a result, we have the solution of Equation (43) as follows.

$$\psi \left(y,t\right)=1-t{e}^y.$$

(51)

Figure 2 below, shows the solution of initial value problem (43) and (44). □

Example 3.

Consider the non-homogeneous advection problem [35]:

$$\frac{\partial \psi }{\partial t}+\psi \frac{\partial \psi }{\partial y}=2t+y+t^3+y{t}^2,$$

(52)

with IC.

$$\psi \left(y,0\right)=0.$$

(53)

Solution. 

Taking the DLST on Equation (52) and the single (LT) on Equation (53), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{2{\omega }^2}{\upsilon }+\frac{\omega }{{\upsilon }^2}+\omega L_y{S}_t\left[t^3+y{t}^2-\psi \frac{\partial \psi }{\partial y}\right].$$

(54)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (54), we obtain the following.

$$\psi \left(y,t\right)=t^2+yt+L_y{}^{-1}{S}_t{}^{-1}\left[\omega L_y{S}_t\left[t^3+y{t}^2-\psi \frac{\partial \psi }{\partial y}\right]\right].$$

(55)

Now, using the iterative approach, substitute Equation (26) in Equation (55) and use Formulas (30)–(32); we obtain the following solution components.

$${\psi }_0\left(y,t\right)=t^2+yt,$$

(56)

$${\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\omega L_y{S}_t\left[t^3+y{t}^2-{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0.$$

(57)

$${\psi }_2\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\omega L_y{S}_t\left[\left({\psi }_0+{\psi }_1\right)\frac{\partial \left({\psi }_0+{\psi }_1\right)}{\partial y}-{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0.$$

(58)

As a result, we have the solution of Equation (52) as follows.

$$\psi \left(y,t\right)=t^2+yt.$$

(59)

Figure 3 below, shows the solution of initial value problem (52) and (53). □

Example 4.

Consider the nonlinear Klein–Gordon equation [46]:

$$\frac{{\partial }^2\psi }{\partial t^2}-\frac{{\partial }^2\psi }{\partial y^2}+{\psi }^2=2y^2-2t^2+y^4{t}^4,$$

(60)

with the ICs

$$\psi \left(y,0\right)=0, {\psi }_t\left(y,0\right)=0,$$

(61)

and the conditions.

$$\psi \left(0,t\right)=0, {\psi }_y\left(0,t\right)=0.$$

(62)

Solution. 

Running DLST on Equation (60) and the single (LT) on Equation (61) and the single (ST) on Equation (62), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{4{\omega }^2}{{\upsilon }^3}+\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[y^4{t}^4-{\psi }^2\right].$$

(63)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (63), we obtain the following.

$$\psi \left(y,t\right)=y^2{t}^2+L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[y^4{t}^4-{\psi }^2\right]\right].$$

(64)

Using the iterative approach, substitute Equation (26) in Equation (64) and use Formulas (30)–(32); we obtain the following solution components.

$${\psi }_0\left(y,t\right)=y^2{t}^2,$$

(65)

$${\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[y^4{t}^4-{\left({\psi }_0\right)}^2\right]\right]=0,$$

(66)

$${\psi }_2\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{1-{\upsilon }^2{\omega }^2}{L}_y{S}_t\left[{\left({\psi }_0+{\psi }_1\right)}^2-{\left({\psi }_0\right)}^2\right]\right]=0.$$

(67)

As a result, we have the solution of Equation (60) as follows.

$$\psi \left(y,t\right)=y^2{t}^2.$$

(68)

Figure 4 below, shows the solution of initial value problem (60) and (61). □

Example 5.

Consider the nonhomogeneous fifth order KdV equation [6]:

$$\frac{\partial \psi }{\partial t}-\psi \frac{\partial \psi }{\partial y}+\frac{{\partial }^3\psi }{\partial y^3}-\frac{{\partial }^5\psi }{\partial y^5}=\cos y+2t\sin y+\frac{t^2}{2}\sin 2y,$$

(69)

with the IC

$$\psi \left(y,0\right)=0.$$

(70)

and the conditions.

$$\psi \left(0,t\right)=t, {\psi }_y\left(0,t\right)=0, {\psi }_{yy}\left(0,t\right)=-t, {\psi }_{yyy}\left(0,t\right)=0, {\psi }_{yyyy}\left(0,t\right)=t.$$

(71)

Solution. 

Running the DLST on Equation (69) and the single (LT) on Equation (70) and the single (ST) on Equation (71), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{\omega \upsilon }{\left({\upsilon }^2+1\right)}+\frac{\omega }{1+\omega {\upsilon }^3-\omega {\upsilon }^5}{L}_y{S}_t\left[\frac{t^2}{2}\sin 2y+\psi \frac{\partial \psi }{\partial y}\right].$$

(72)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (72), we obtain the following.

$$\psi \left(y,t\right)=t\cos y+L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{1+\omega {\upsilon }^3-\omega {\upsilon }^5}{L}_y{S}_t\left[\frac{t^2}{2}\sin 2y+\psi \frac{\partial \psi }{\partial y}\right]\right].$$

(73)

Using the iterative approach, substitute Equation (26) in Equation (73) and use Formulas (30)–(32), we obtain the following solution components.

$${\psi }_0\left(y,t\right)=t\cos y,$$

(74)

$${\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{1+\omega {\upsilon }^3-\omega {\upsilon }^5}{L}_y{S}_t\left[\frac{t^2}{2}\sin 2y+{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0,$$

(75)

$${\psi }_2\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{\omega }{1+\omega {\upsilon }^3-\omega {\upsilon }^5}{L}_y{S}_t\left[\left({\psi }_0+{\psi }_1\right)\frac{\partial \left({\psi }_0+{\psi }_1\right)}{\partial y}-{\psi }_0\frac{\partial {\psi }_0}{\partial y}\right]\right]=0.$$

(76)

As a result, we have the solution of Equation (69) as follows

$$\psi \left(y,t\right)=t\cos y$$

(77)

Figure 5 below, shows the solution of initial value problem (69) and (70). □

Example 6.

Consider the following non-linear telegraph equation [45]:

$$\frac{{\partial }^2\psi }{\partial y^2}=\frac{{\partial }^2\psi }{\partial t^2}+2\frac{\partial \psi }{\partial t}+{\psi }^2+e^{y-2t}-e^{2y-4t},$$

(78)

with the ICs

$$\psi \left(y,0\right)=e^y,{\psi }_t\left(y,0\right)=-2e^y,$$

(79)

and the conditions.

$$\psi \left(0,t\right)={\psi }_y\left(0,t\right)=e^{-2t}.$$

(80)

Solution. 

Applying the DLST on Equation (78) and the single (LT) on Equation (79) and the single (ST) on Equation (80), we obtain the following.

$$\tilde{\psi }\left(\upsilon ,\omega \right)=\frac{1}{\left(\upsilon -1\right)\left(1+2\omega \right)}+\frac{{\omega }^2}{\left({\omega }^2{\upsilon }^2-2\omega -1\right)}{L}_y{S}_t\left[{\psi }^2-e^{2y-4t}\right].$$

(81)

Taking the inverse DLST $L_y{}^{-1}{S}_t{}^{-1}\left[\tilde{\psi }\left(\upsilon ,\omega \right)\right]$ on Equation (81), we obtain the following.

$$\psi \left(y,t\right)=e^{y-2t}+L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{\left({\omega }^2{\upsilon }^2-2\omega -1\right)}{L}_y{S}_t\left[{\psi }^2-e^{2y-4t}\right]\right].$$

(82)

Using the iterative approach substitute Equation (26) in Equation (82) and Formulas (30)–(32), we obtain the following solution components.

$${\psi }_0\left(y,t\right)=e^{y-2t},$$

(83)

$${\psi }_1\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{\left({\omega }^2{\upsilon }^2-2\omega -1\right)}{L}_y{S}_t\left[{\left({\psi }_0\right)}^2-e^{2y-4t}\right]\right]=0,$$

(84)

$${\psi }_2\left(y,t\right)=L_y{}^{-1}{S}_t{}^{-1}\left[\frac{{\omega }^2}{\left({\omega }^2{\upsilon }^2-2\omega -1\right)}{L}_y{S}_t\left[{\left({\psi }_0+{\psi }_1\right)}^2-{\left({\psi }_0\right)}^2\right]\right]=0.$$

(85)

As a result, we have the solution of Equation (78) as follows.

$$\psi \left(y,t\right)=e^{y-2t}.$$

(86)

Figure 6 below, shows the solution of initial value problem (78) and (79). □

5. Conclusions

In this paper, we presented a new method—that is, a combination of the double Laplace–Sumudu transform and a numerical method, which is known as an iterative method, to obtain the exact solutions of the nonlinear partial differential equations with initial conditions, which are widely used in mathematical physics. Six examples are given to demonstrate the applicability of the method under consideration. The suggested method’s answers to Examples 1, 2, 3, 4, 5, and 6 are in good agreement with the same problem examined in [40,42,45,46], and nontrivial problems treated using earlier approaches become trivial in the sense that the following decomposition:

$$\psi \left(y,t\right)={\psi }_0\left(y,t\right)+{\psi }_1\left(y,t\right)+{\psi }_2\left(y,t\right)+\dots +{\psi }_r\left(y,t\right).$$

consists of a single term, i.e., $\psi \left(y,t\right)={\psi }_0\left(y,t\right)$. We concluded from our research that using the double Laplace–Sumudu transform in combination with the iterative method yields very practical analytical findings with less computational work.