Hidden Dynamics and Hybrid Synchronization of Fractional-Order Memristive Systems
Abstract
1. Introduction
2. Preliminaries
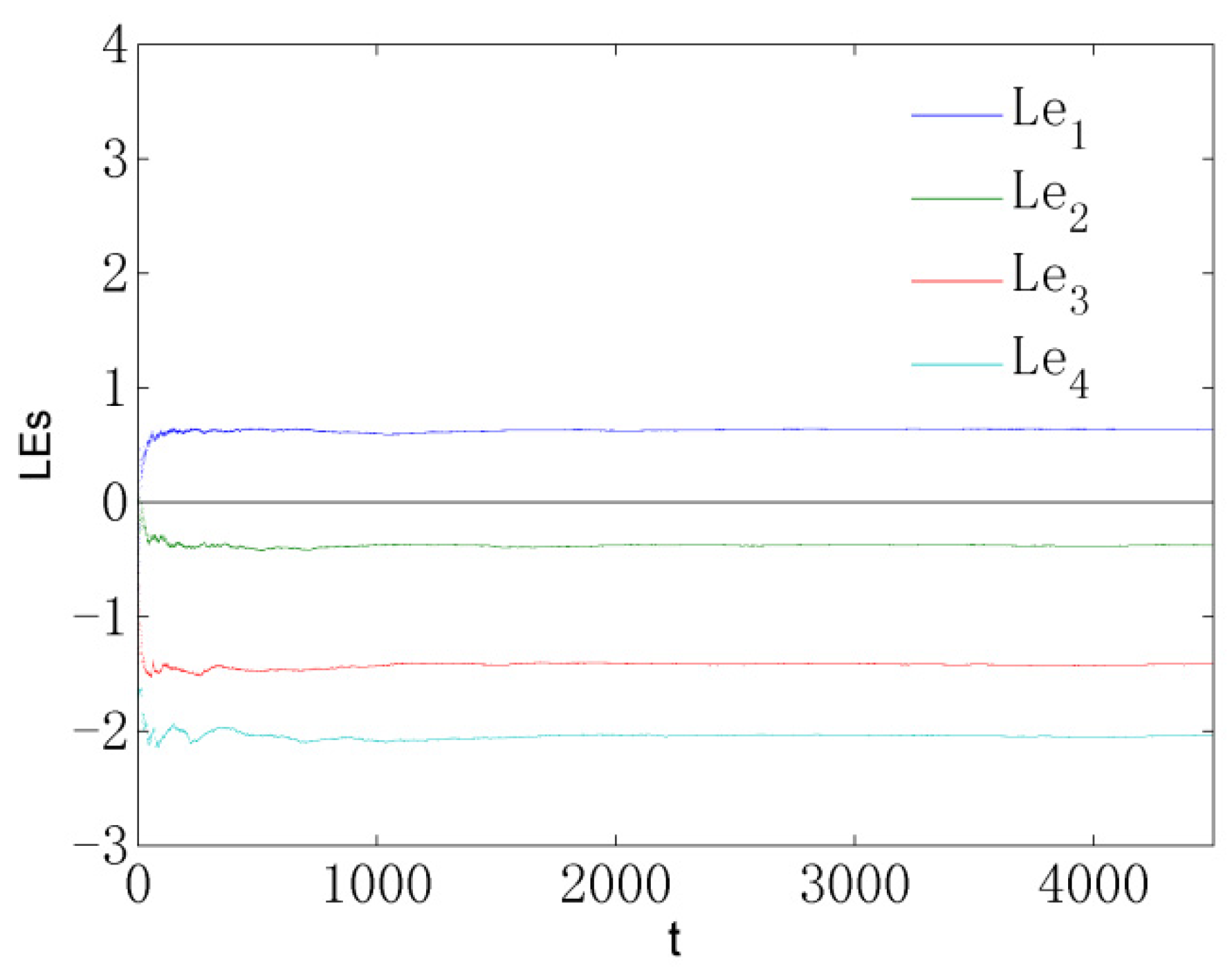
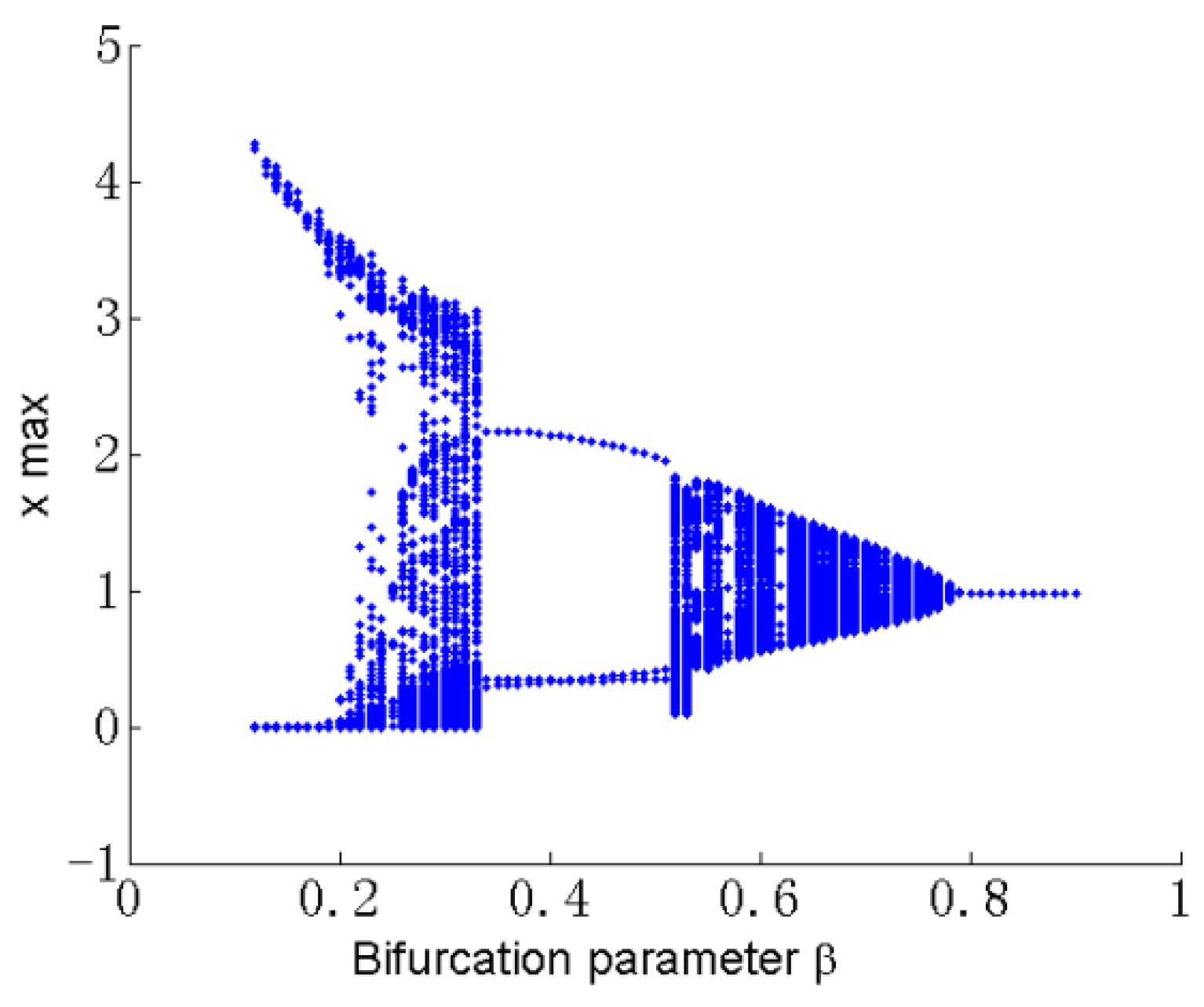
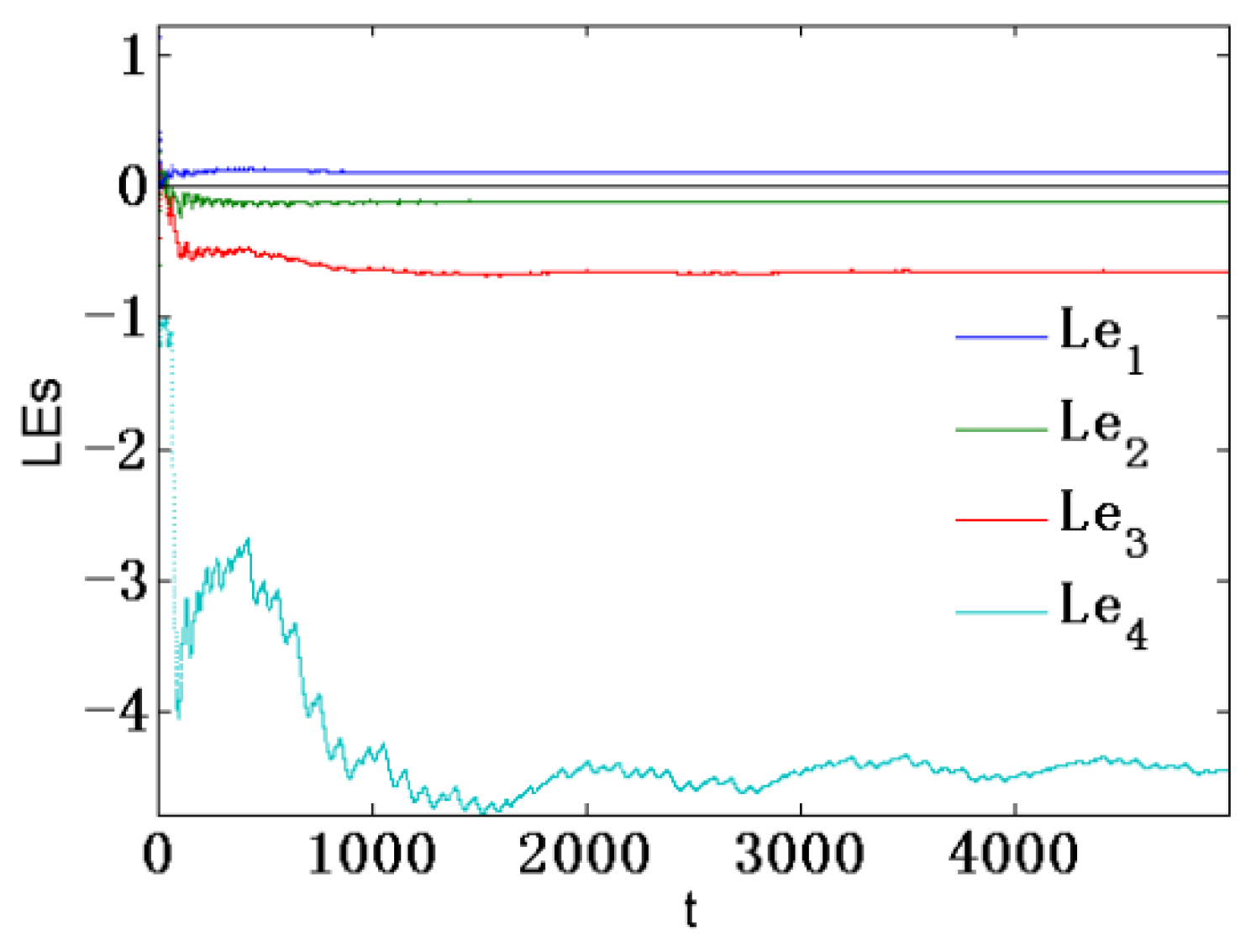
3. System Description and Its Dynamical Behaviors
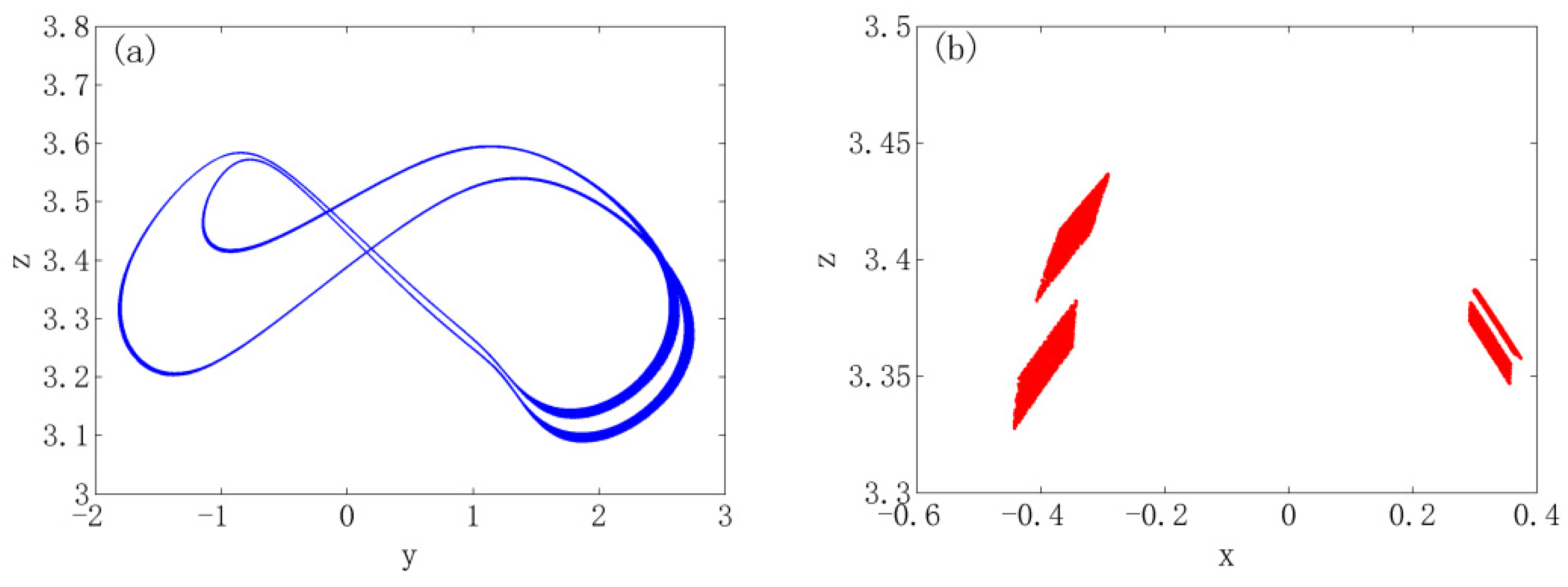
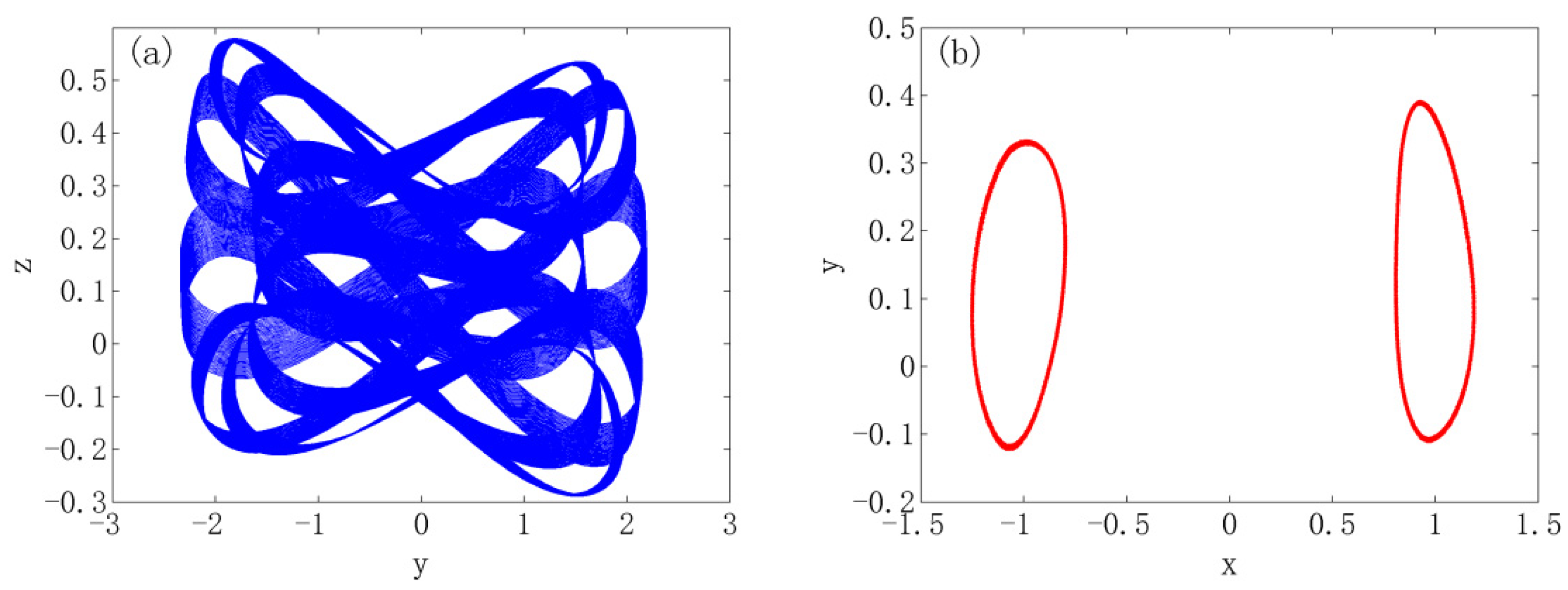
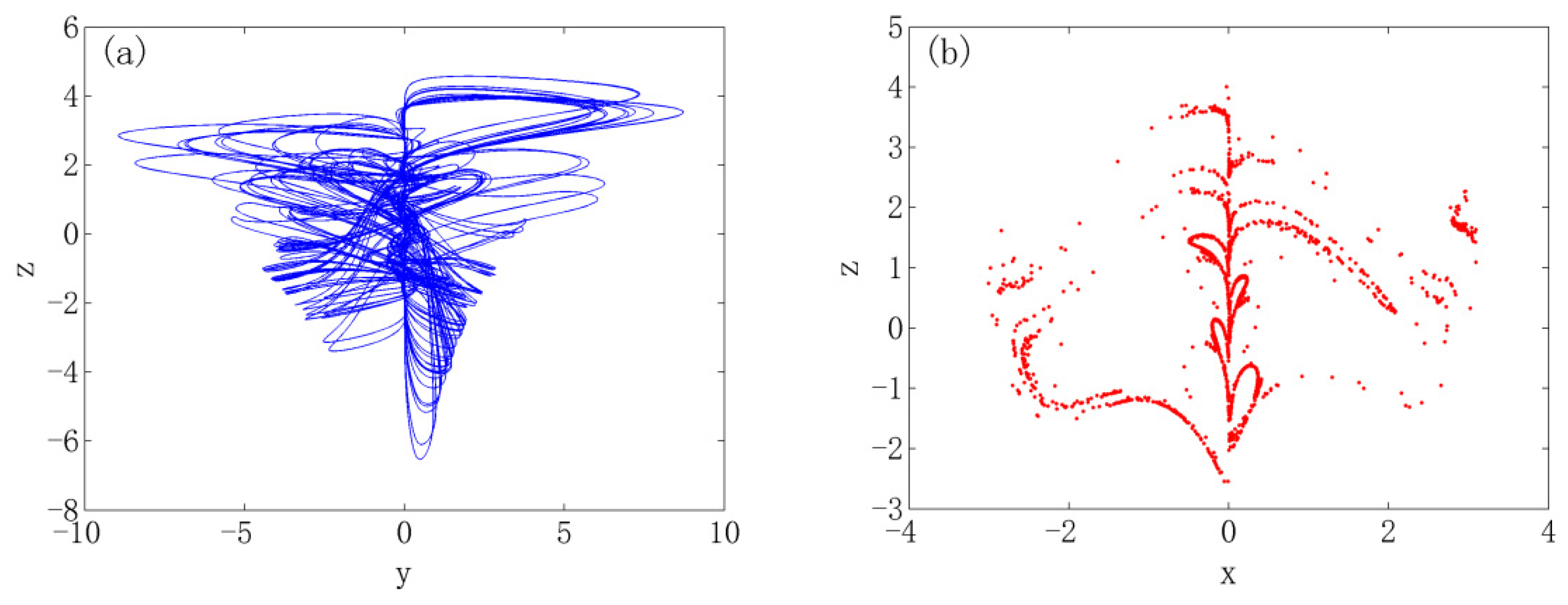
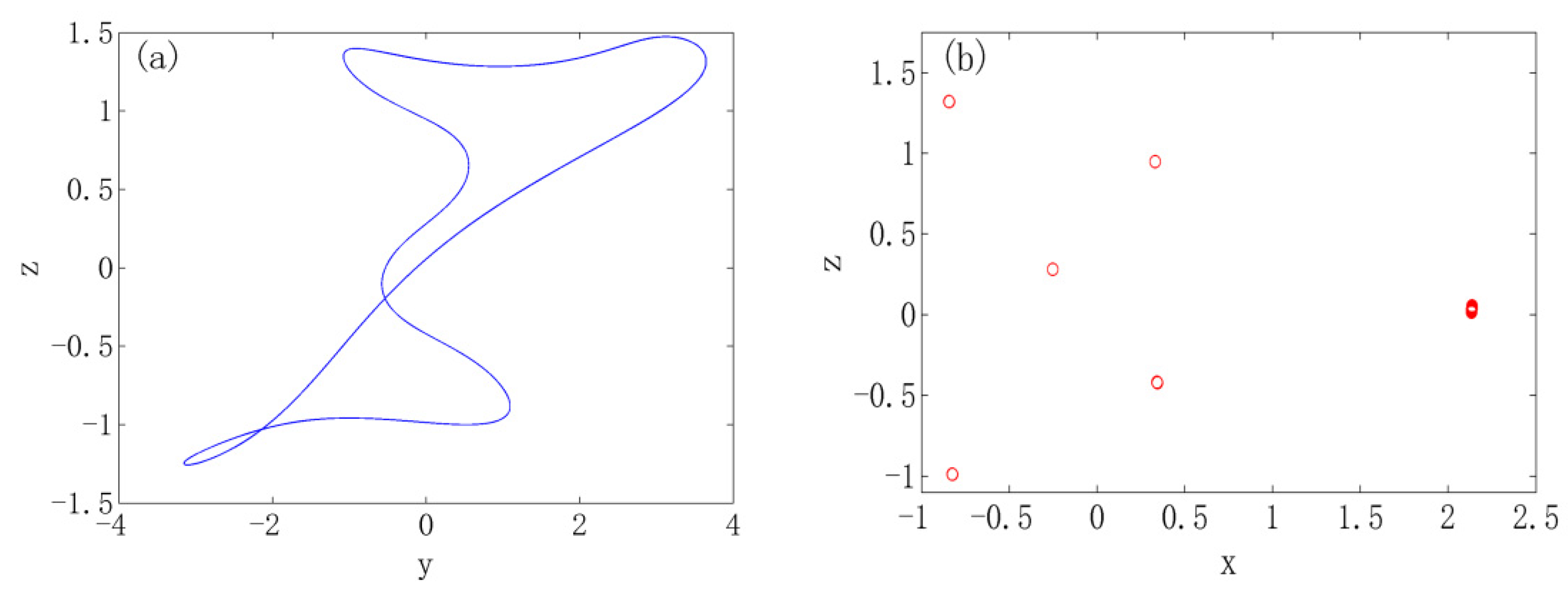
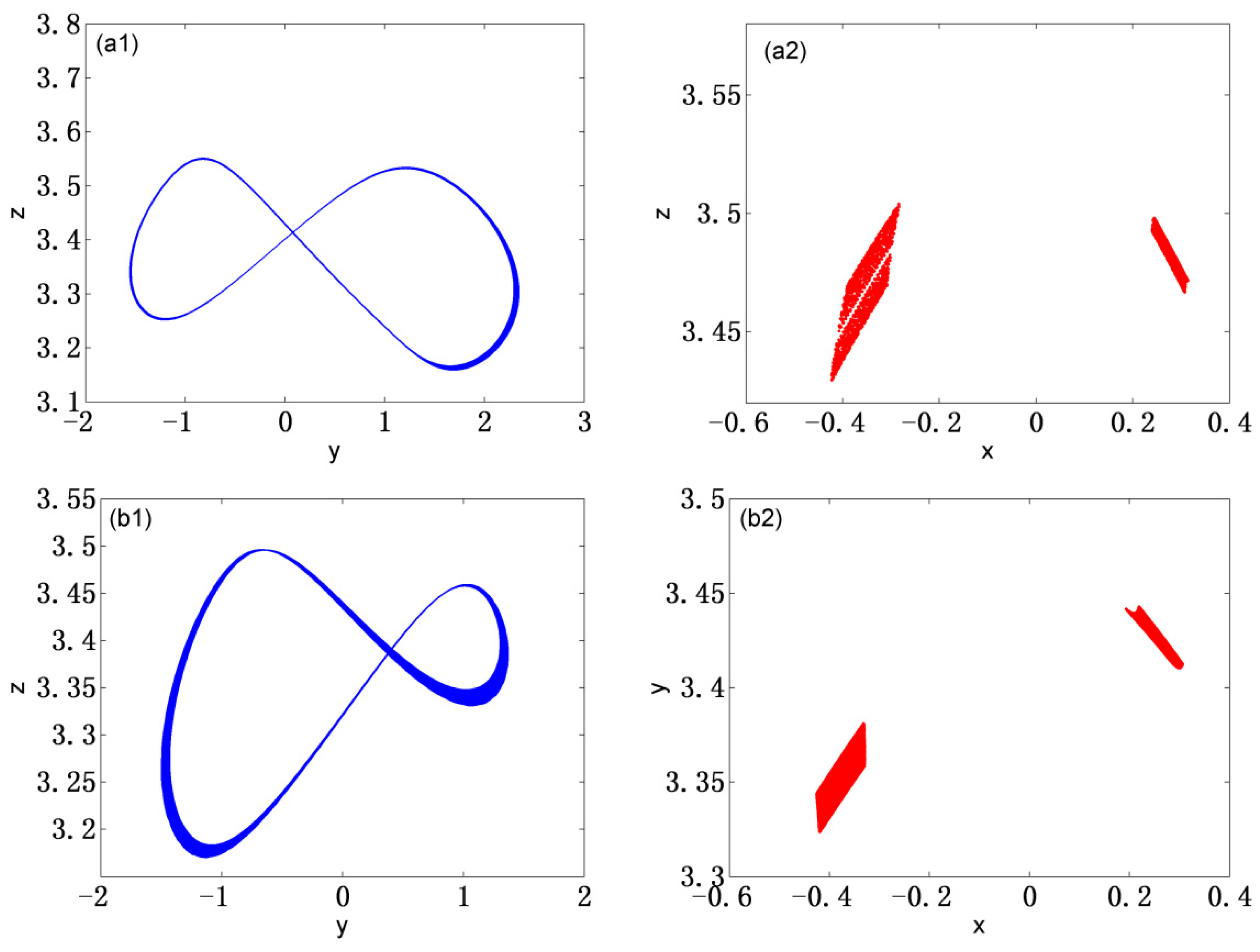
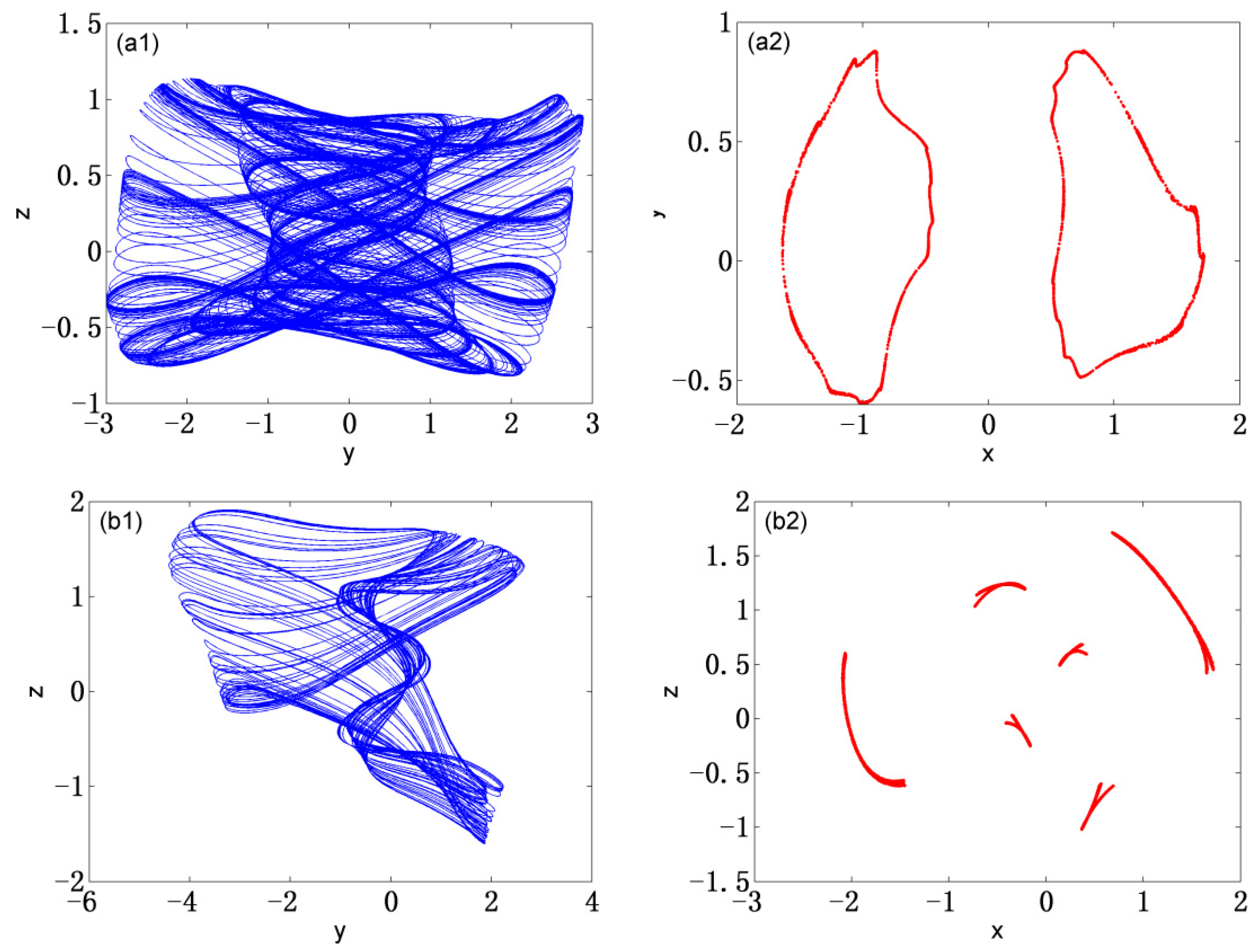
3.1. Hidden Attractors in the Proposed System
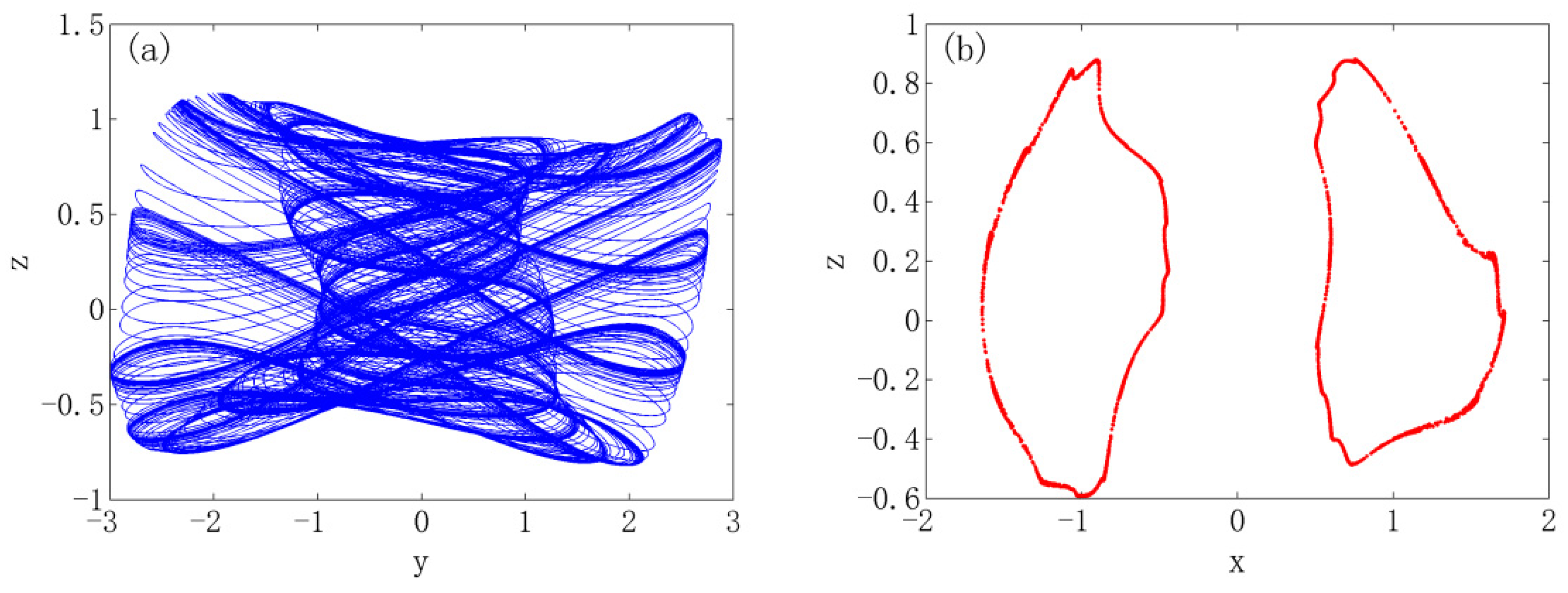
3.2. Coexistence of Different Hidden Attractors
4. Hybrid Synchronization Scheme
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Akgul, A.; Hussain, S.; Pehlivan, I. A new three-dimensional chaotic system, its dynamical analysis and electronic circuit applications. Optik 2016, 127, 7062–7071. [Google Scholar] [CrossRef]
- Dudkowski, D.; Jafari, S.; Kapitaniak, T.; Kuznetsov, N.V.; Leonov, G.A.; Prasad, A. Hidden attractors in dynamical systems. Phys. Rep. 2016, 637, 1–50. [Google Scholar] [CrossRef]
- Zhu, M.; Wang, C. A novel parallel chaotic system with greatly improved Lyapunov exponent and chaotic range. Int. J. Mod. Phys. B 2020, 34, 2050048. [Google Scholar] [CrossRef]
- Mobayen, S.; Fekih, A.; Vaidyanathan, S.; Sambas, A. Chameleon Chaotic Systems with Quadratic Nonlinearities: An Adaptive Finite-Time Sliding Mode Control Approach and Circuit Simulation. IEEE Access 2021, 9, 64558–64573. [Google Scholar] [CrossRef]
- Min, F.H.; Li, C.; Zhang, L.; Li, C.B. Initial value-related dynamical analysis of the memristor-based system with reduced dimensions and its chaotic synchronization via adaptive sliding mode control method. Chin. J. Phys. 2019, 58, 117–131. [Google Scholar] [CrossRef]
- Xuan, L.; Ahmad, S.; Ullah, A.; Saifullah, S.; Akgül, A.; Qu, H. Bifurcations, stability analysis and complex dynamics of Caputo fractal-fractional cancer model. Chaos Solitons Fractals 2022, 159, 112113. [Google Scholar] [CrossRef]
- Dong, E.; Zhang, Z.; Yuan, M.; Ji, Y.; Zhou, X.; Wang, Z. Ultimate boundary estimation and topological horseshoe analysis on a parallel 4D hyperchaotic system with any number of attractors and its multi-scroll. Nonlinear Dyn. 2019, 95, 3219–3236. [Google Scholar] [CrossRef]
- Capligins, F.; Litvinenko, A.; Aboltins, A.; Austrums, E.; Rusins, A. Chaotic jerk circuit usage in communication systems. In Proceedings of the 2020 IEEE Workshop on Microwave Theory and Techniques in Wireless Communications (MTTW’20), Riga, Latvia, 1–2 October 2020; pp. 227–230. [Google Scholar]
- Can, E.; Kocamaz, U.E.; Uyaroglu, Y. A new six-term 3d unified chaotic system. Iran. J. Sci. Technol.-Trans. Electr. Eng. 2020, 44, 1593–1604. [Google Scholar] [CrossRef]
- Hua, Z.; Zhou, Y. Exponential Chaotic Model for Generating Robust Chaos. IEEE Trans. Syst. Man, Cybern. Syst. 2021, 51, 3713–3724. [Google Scholar] [CrossRef]
- Butzer, P.L.; Westphal, U. An Introduction to Fractional Calculus; World Scientific: Singapore, 2000; pp. 1–85. [Google Scholar] [CrossRef]
- Chai, X.H.; Cao, H.; Zhang, B.; Jiao, M.; Zhang, C.J. Research on analysis and control performance of full fractional-order boost converter system. J. Power Suppl. 2020, 17, 27–33. [Google Scholar]
- El-Saka, H.A.A.; Lee, S.; Jang, B. Dynamic analysis of fractional-order predator–prey biological economic system with Holling type II functional response. Nonlinear Dyn. 2019, 96, 407–416. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, F. A fractional-order CNN hyperchaotic system for image encryption algorithm. Phys. Scr. 2021, 96, 035209. [Google Scholar] [CrossRef]
- Dong, J.; Xiao, Y.; Ma, H.; Zhang, G.J. Dynamic characteristics analysis of time-delay fractional order dynamic system. In Proceedings of the 2020 3rd International Conference on Computer Information Science and Application Technology (CISAT), Dali, China, 17–19 July 2020; Volume 1634, p. 012105. [Google Scholar]
- Li, R. Effects of system parameter and fractional order on dynamic behavior evolution in fractional-order Genesio-Tesi system. Optik 2016, 127, 6695–6709. [Google Scholar] [CrossRef]
- Tacha, O.I.; Munoz-Pacheco, J.M.; Zambrano-Serrano, E.; Stouboulos, I.N.; Pham, V.-T. Determining the chaotic behavior in a fractional-order finance system with negative parameters. Nonlinear Dyn. 2018, 94, 1303–1317. [Google Scholar] [CrossRef]
- Yang, Z.; Liang, D.; Ding, D.; Hu, Y. Dynamic behavior of fractional-order memristive time-delay system and image encryption application. Mod. Phys. Lett. B 2021, 35, 2150271. [Google Scholar] [CrossRef]
- Wu, J.; Wang, G.; Iu, H.H.-C.; Shen, Y.; Zhou, W. A Nonvolatile Fractional Order Memristor Model and Its Complex Dynamics. Entropy 2019, 21, 955. [Google Scholar] [CrossRef]
- Chen, M.; Yu, J.; Bao, B. Finding hidden attractors in improved memristor-based Chua’s circuit. Circuits Syst. 2015, 51, 462–464. [Google Scholar] [CrossRef]
- Zhou, P.; Zhu, W. Function projective synchronization for fractional-order chaotic systems. Nonlinear Anal. Real World Appl. 2011, 12, 811–816. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Hu, Z.-W.; Luo, C. Generalized Synchronization of Nonidentical Fractional-Order Chaotic Systems. Int. J. Mod. Phys. B 2013, 27, 1350195. [Google Scholar] [CrossRef]
- Khan, A.; Bhat, M.A. Multiswitching combination synchronization of non-identical fractional-order chaotic systems. Pramana-J. Phys. 2018, 90, 73. [Google Scholar] [CrossRef]
- Meng, X.L.; Mao, B.X. Sliding mode synchronization of fractional-order T chaotic systems with logarithmic. J. Shandong Univ. Eng. Sci. 2020, 50, 7–12. [Google Scholar]
- Guo, R.; Zhang, Y.; Jiang, C. Synchronization of Fractional-Order Chaotic Systems with Model Uncertainty and External Disturbance. Mathematics 2021, 9, 877. [Google Scholar] [CrossRef]
- Udhayakumar, K.; Rihan, F.A.; Rakkiyappan, R.; Cao, J. Fractional-order discontinuous systems with indefinite LKFs: An application to fractional-order neural networks with time delays. Neural Netw. 2021, 145, 319–330. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.L.; Liu, X.G.; Li, X.M. Finite-time synchronization of delayed fractional-order coupled neural networks. Int. J. Syst. Sci. 2022, 53, 2597–2611. [Google Scholar] [CrossRef]
- Liu, D.; Li, T.; Wang, Y. Adaptive Dual Synchronization of Fractional-Order Chaotic System with Uncertain Parameters. Mathematics 2022, 10, 470. [Google Scholar] [CrossRef]
- Gorenflo, R.; Mainardi, F. Fractional calculus: Integral and differential equations of fractional order. arXiv 2008, arXiv:0805.3823. [Google Scholar]
- Bao, H.; Bao, B.C.; Lin, Y.; Wang, J.; Wu, H.G. Hidden attractor and its dynamical characteristic in memristive self-oscillating system. Acta Phys. Sin. 2016, 65, 180501. [Google Scholar]
- Pulido-Luna, J.R.; López-Rentería, J.A.; Cazarez-Castro, N.R.; Campos, E. A two-directional grid multiscroll hidden attractor based on piecewise linear system and its application in pseudo-random bit generator. Integration 2021, 81, 34–42. [Google Scholar] [CrossRef]
- Awrejcewicz, J. Chapter 3: Generation of self-excited and hidden multiscroll attractors in multistable systems. In Recent Trends in Chaotic, Nonlinear and Complex Dynamics, Chapter 3; World Scientific: Singapore, 2021; pp. 40–78. [Google Scholar]
- Escalante-González, R.J.; Campos, E. Multistable systems with hidden and self-excited scroll attractors generated via piecewise linear systems. Complexity 2020, 2020, 7832489. [Google Scholar] [CrossRef]
- Kuznetsov, N.V.; Leonov, A. Hidden attractor in dynamical systems: Systems with no equilibria, multistability and coexisting attractors. IFAC Proc. Vol. 2014, 47, 5445–5454. [Google Scholar] [CrossRef]
- Hu, J.B.; Han, Y.; Zhao, L.D. A stability theorem about fractional systems and synchronizing fractional unified chaotic systems based on the theorem. Acta Phys. Sin. 2009, 58, 4402–4407. [Google Scholar]
- Hu, J.B.; Han, Y.L.; Zhao, D. A novel stability theorem for fractional systems and its applying in synchronizing fractional chaotic system based on backstepping approach. Acta Phys. Sin. 2009, 58, 2235–2239. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, H.; Zhuang, L.; Chen, C.; Wang, Z. Hidden Dynamics and Hybrid Synchronization of Fractional-Order Memristive Systems. Axioms 2022, 11, 645. https://doi.org/10.3390/axioms11110645
Jiang H, Zhuang L, Chen C, Wang Z. Hidden Dynamics and Hybrid Synchronization of Fractional-Order Memristive Systems. Axioms. 2022; 11(11):645. https://doi.org/10.3390/axioms11110645
Chicago/Turabian StyleJiang, Haipeng, Lizhou Zhuang, Cheng Chen, and Zuolei Wang. 2022. "Hidden Dynamics and Hybrid Synchronization of Fractional-Order Memristive Systems" Axioms 11, no. 11: 645. https://doi.org/10.3390/axioms11110645
APA StyleJiang, H., Zhuang, L., Chen, C., & Wang, Z. (2022). Hidden Dynamics and Hybrid Synchronization of Fractional-Order Memristive Systems. Axioms, 11(11), 645. https://doi.org/10.3390/axioms11110645





