Oscillatory Behavior of Third-Order Quasi-Linear Neutral Differential Equations
Abstract
1. Introduction
- (I1)
- is positive and where
- (I2)
- q is non-negative and does not eventually vanish (i.e., is not eventually zero on any half line for );
- (I3)
- and
- (I4)
- and satisfieswhere is the quotient of odd positive integers.
2. Auxiliary Results
- (a)
- and is decreasing, and ,
- (b)
- and is increasing.
3. Main Results
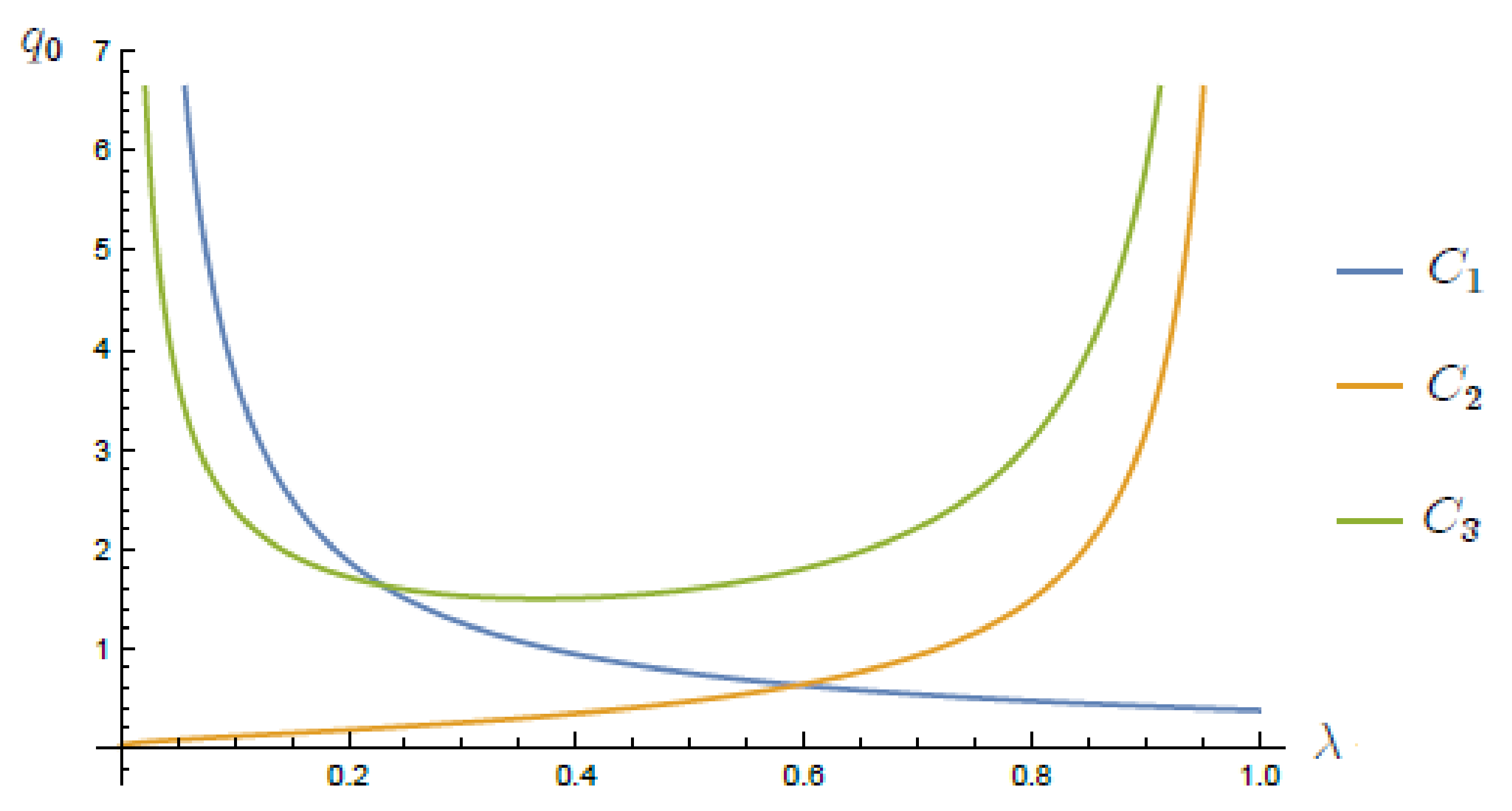
4. Applications
4.1. Asymptotic Properties
4.2. Oscillation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Liu, M.; Dassios, I.; Tzounas, G.; Milano, F. Stability Analysis of Power Systems with Inclusion of Realistic-Modeling of WAMS Delays. IEEE Trans. Power Syst. 2019, 34, 627–636. [Google Scholar] [CrossRef]
- Milano, F.; Dassios, I. Small-Signal Stability Analysis for Non-Index 1 Hessenberg Form Systems of Delay Differential-Algebraic Equations. IEEE Trans. Circuits Syst. Regul. Pap. 2016, 63, 1521–1530. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Berezansky, L.; Braverman, E.; Domoshnitsky, A. Nonoscillation Theory of Functional Differential Equations with Applications; Springer: Berlin/Heidelberg, Germany, 2012; 520p, ISBN 978-1-4614-3454-2. [Google Scholar]
- Hale, J.K. Theory of Functional Differential Equations; Springer: Berlin/Heidelberg, Germany, 1977. [Google Scholar]
- Moaaz, O.; Elabbasy, E.M.; Qaraad, B. An improved approach for studying oscillation of generalized Emden–Fowler neutral differential equation. J. Inequal. Appl. 2020, 2020, 69. [Google Scholar] [CrossRef]
- Chatzarakis, G.E.; Moaaz, O.; Li, T.; Qaraad, B. Some oscillation theorems for nonlinear second-order differential equations with an advanced argument. Adv. Differ. Equ. 2020, 160. [Google Scholar] [CrossRef]
- Bohner, M.; Grace, S.R.; Sager, I.; Tunc, E. Oscillation of third-order nonlinear damped delay differential equations. Appl. Math. Comput. 2016, 278, 21–32. [Google Scholar] [CrossRef]
- Chatzarakis, G.E.; Grace, S.R.; Jadlovska, I.; Li, T.; Tunc, E. Oscillation criteria for third-order Emden–Fowler differential equations with unbounded neutral coefficients. Complexity 2019, 2019, 5691758. [Google Scholar] [CrossRef]
- Grace, S.R.; Graef, J.R.; Tunc, E. Oscillatory behaviour of third order nonlinear differential equations with a nonlinear nonpositive neutral term. J. Taibah Univ. Sci. 2019, 13, 704–710. [Google Scholar] [CrossRef]
- Grace, S.R.; Jadlovska, I.; Tunc, E. Oscillatory and asymptotic behavior of third-order nonlinear differential equations with a superlinear neutral term. Turk. J. Math. 2020, 44, 1317–1329. [Google Scholar] [CrossRef]
- Moaaz, O.; Qaraad, B.; El-Nabulsi, R.; Bazighifan, O. New Results for Kneser Solutions of Third-Order Nonlinear Neutral Differential Equations. Mathematics 2020, 8, 686. [Google Scholar] [CrossRef]
- Elabbasy, E.M.; Qaraad, B.; Abdeljawad, T.; Moaaz, O. Oscillation Criteria for a Class of Third-Order Damped Neutral Differential Equations. Symmetry 2020, 12, 1988. [Google Scholar] [CrossRef]
- Philos, C. On the existence of nonoscillatory solutions tending to zero at ∞ for differential equations with positive delays. Arch. Math. 1981, 36, 168–178. [Google Scholar] [CrossRef]
- Chatzarakis, G.E.; Dzurina, J.; Jadlovsk, I. Oscillatory and asymptotic properties of third-order quasilinear delay differential equations. J. Inequal. Appl. 2019, 23. [Google Scholar] [CrossRef]
- Li, T.; Zhang, C.; Baculikova, B.; Dzurina, J. On the oscillation of third-order quasi-linear delay differential equations. Tatra Mt. Math. Publ. 2011, 48, 117–123. [Google Scholar] [CrossRef]
- Dzurina, J.; Thapani, E.; Tamilvanan, S. Oscillation of solutions to third-order half-linear neutral differential equations. Electron. J. Differ. Equ. 2012, 29, 1–9. [Google Scholar]
- Graef, J.; Tunc, E.; Grace, S. Oscillatory and asymptotic behavior of a third-order nonlinear neutral differential equation. Opuscula Math. 2017, 37, 839–852. [Google Scholar] [CrossRef]
- Santra, S.S.; Ghosh, A.; Bazighifan, O.; Khedher, K.M.; Nofal, T.A. Second-order impulsive differential systems with mixed and several delays. Adv. Differ. Equ. 2021, 1, 1–12. [Google Scholar] [CrossRef]
- Santra, S.S.; Baleanu, D.; Khedher, K.M.; Moaaz, O. First-order impulsive differential systems: Sufficient and necessary conditions for oscillatory or asymptotic behavior. Adv. Differ. Equ. 2021, 1, 1–20. [Google Scholar] [CrossRef]
- Santra, S.S.; Tripathy, A.K. On oscillatory first order nonlinear neutral differential equations with nonlinear impulses. J. Appl. Math. Comput. 2019, 59, 257–270. [Google Scholar] [CrossRef]
- Ruggieri, M.; Santra, S.S.; Scapellato, A. On nonlinear impulsive differential systems with canonical and non-canonical operators. Appl. Anal. 2021. [Google Scholar] [CrossRef]
- Moaaz, O. Oscillation Theorems for Cartain Second-Order Differential Equations; Lambert Academic Publishing: Saarbrücken, Germany, 2014. [Google Scholar]
- Tunc, E. Oscillatory and asymptotic behavior of third-order neutral differential equations with distributed deviating arguments. Electron. J. Differ. Equ. 2017. [Google Scholar] [CrossRef][Green Version]
- Tiryaki, A.; Aktas, M.F. Oscillation criteria of a certain class of third-order nonlinear delay differential equations with damping. J. Math. Anal. Appl. 2007, 325, 54–68. [Google Scholar] [CrossRef]
- Baculikova, B.; Dzurina, J. Oscillation of third-order functional differential equations. Electron. J. Qual. Theory of Diff. Equ. 2010, 43, 1–10. [Google Scholar] [CrossRef]
- Baculikova, B.; Dzurina, J. Oscillation of third-order nonlinear differential equations. Appl. Math. Lett. 2011, 24, 466–470. [Google Scholar] [CrossRef]
- Grace, S.R.; Agarwal, R.P.; Pavani, R.; Thapani, E. On the oscillation of certain third order nonlinear functional differential equations. Appl. Math. Comput. 2008, 202, 102–112. [Google Scholar] [CrossRef]
- Saker, S.H.; Dzurina, J. On the oscillation of certain class of third-order nonlinear delay differential equations. Math. Bohem. 2010, 135, 225–237. [Google Scholar] [CrossRef]
- Ravi, P.; Agarwal, R.P.; Bohner, M.; Li, T.; Zhang, C. Oscillation of Third-Order Nonlinear Delay Differential Equations. Taiwan. J. Math. 2013, 17, 545–558. [Google Scholar]
- Sidorov, N.A.; Trufanov, A.N. Nonlinear operator equations with a functional perturbation of the argument of neutral type. Differ. Equ. 2009, 45, 1840–1844. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Wang, Q. Oscillation of Second-Order Nonlinear Neutral Dynamic Equations on Time Scales. Appl. Math. Comput. 2010, 216, 2837–2848. [Google Scholar] [CrossRef]
- Thapani, E.; Li, T. On the oscillation of third-order quasi-linear neutral functional differential equations. Arch. Math. 2011, 47, 181–199. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qaraad, B.; Moaaz, O.; Santra, S.S.; Noeiaghdam, S.; Sidorov, D.; Elabbasy, E.M. Oscillatory Behavior of Third-Order Quasi-Linear Neutral Differential Equations. Axioms 2021, 10, 346. https://doi.org/10.3390/axioms10040346
Qaraad B, Moaaz O, Santra SS, Noeiaghdam S, Sidorov D, Elabbasy EM. Oscillatory Behavior of Third-Order Quasi-Linear Neutral Differential Equations. Axioms. 2021; 10(4):346. https://doi.org/10.3390/axioms10040346
Chicago/Turabian StyleQaraad, Belgees, Osama Moaaz, Shyam Sundar Santra, Samad Noeiaghdam, Denis Sidorov, and Elmetwally M. Elabbasy. 2021. "Oscillatory Behavior of Third-Order Quasi-Linear Neutral Differential Equations" Axioms 10, no. 4: 346. https://doi.org/10.3390/axioms10040346
APA StyleQaraad, B., Moaaz, O., Santra, S. S., Noeiaghdam, S., Sidorov, D., & Elabbasy, E. M. (2021). Oscillatory Behavior of Third-Order Quasi-Linear Neutral Differential Equations. Axioms, 10(4), 346. https://doi.org/10.3390/axioms10040346









