Economical Feasibility of Rare Earth Mining outside China
Abstract
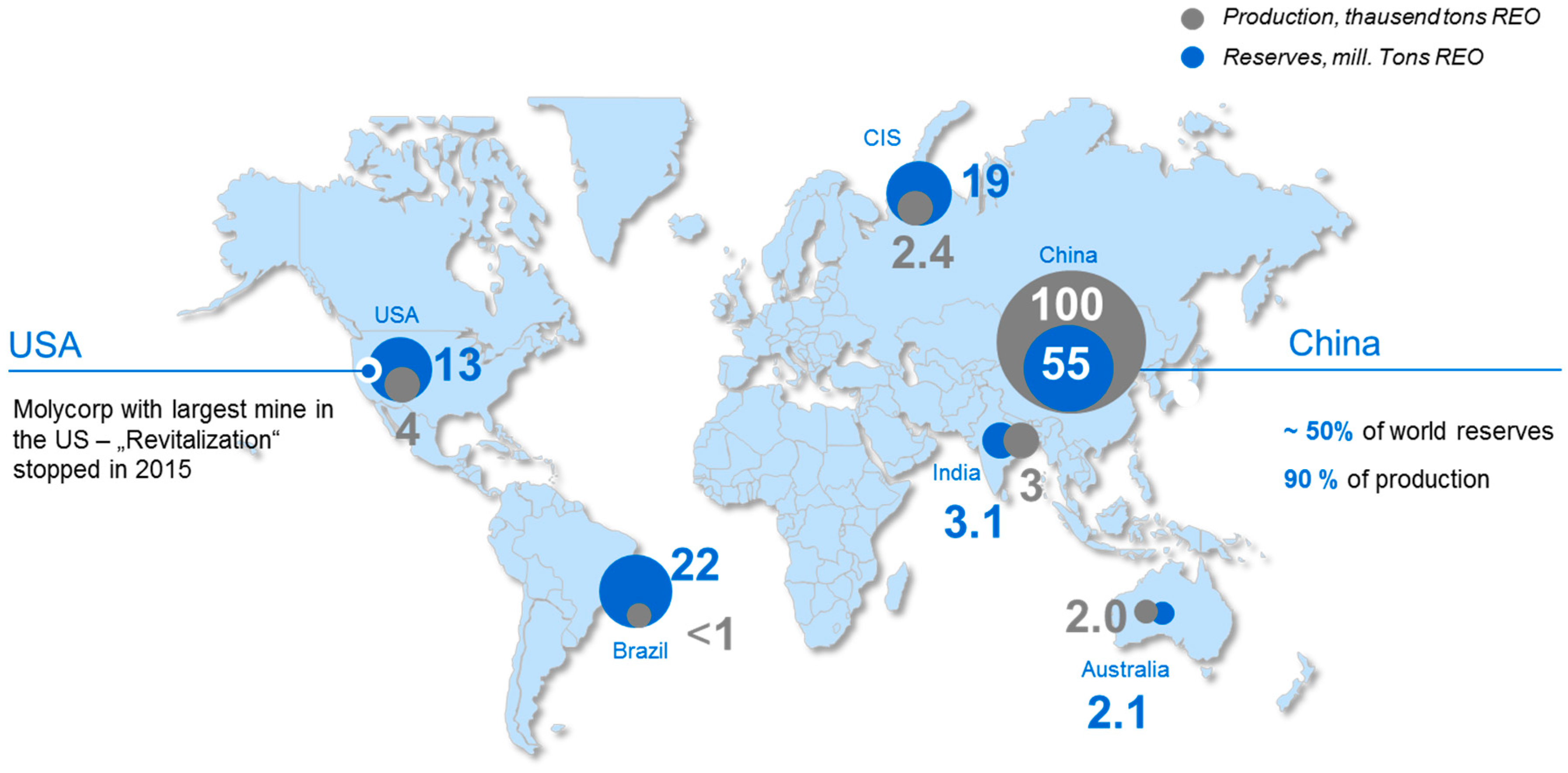
:1. Introduction
2. Criticality of REE and DCF Model
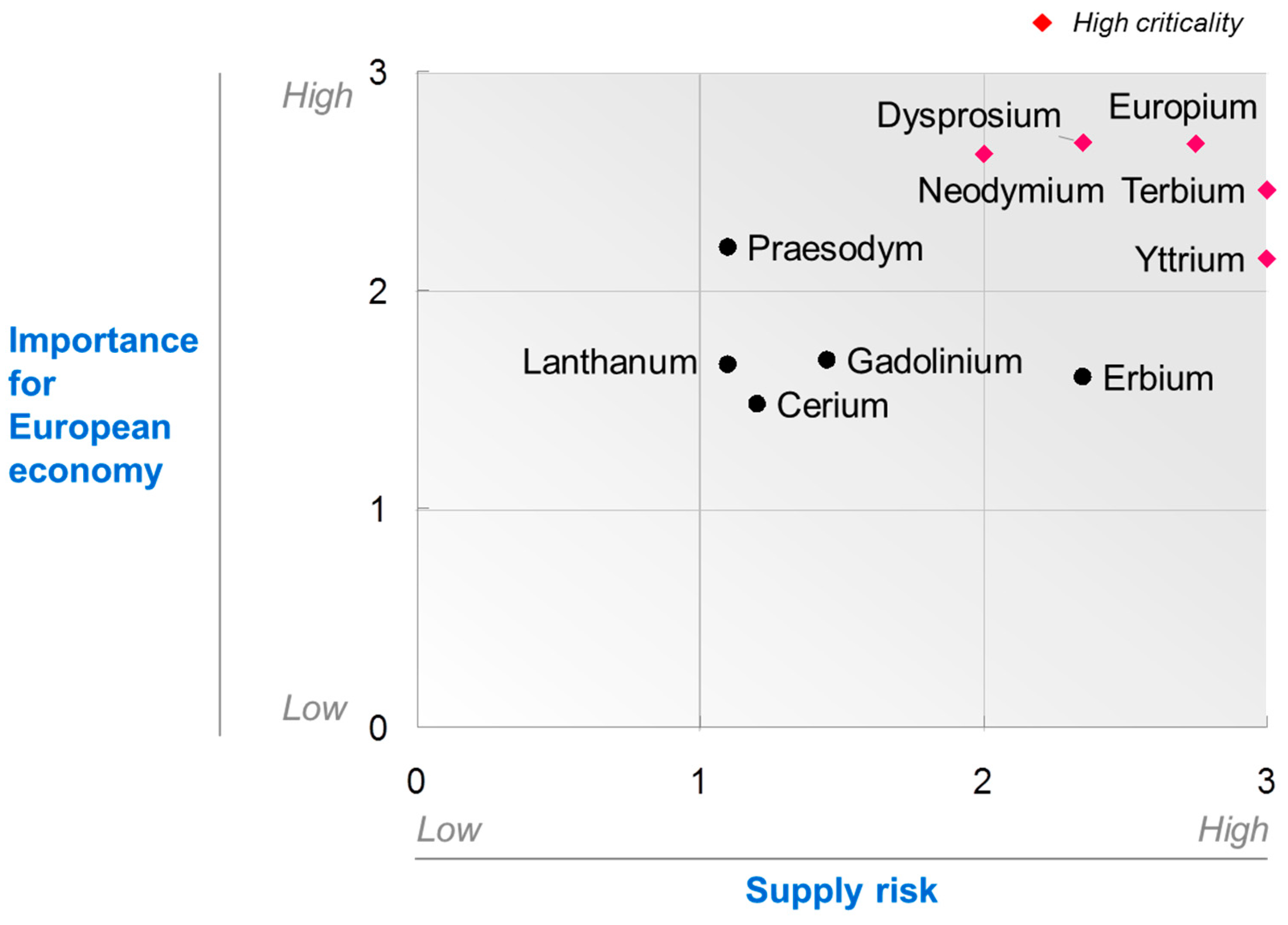
2.1. Determining the Criticality of REE
2.2. Structure of the Model
2.2.1. Selection of Potential REE Projects
2.2.2. Expenditure
2.2.3. Revenues
2.3. DCF Model (Method)
3. Results and Discussion
3.1. Results
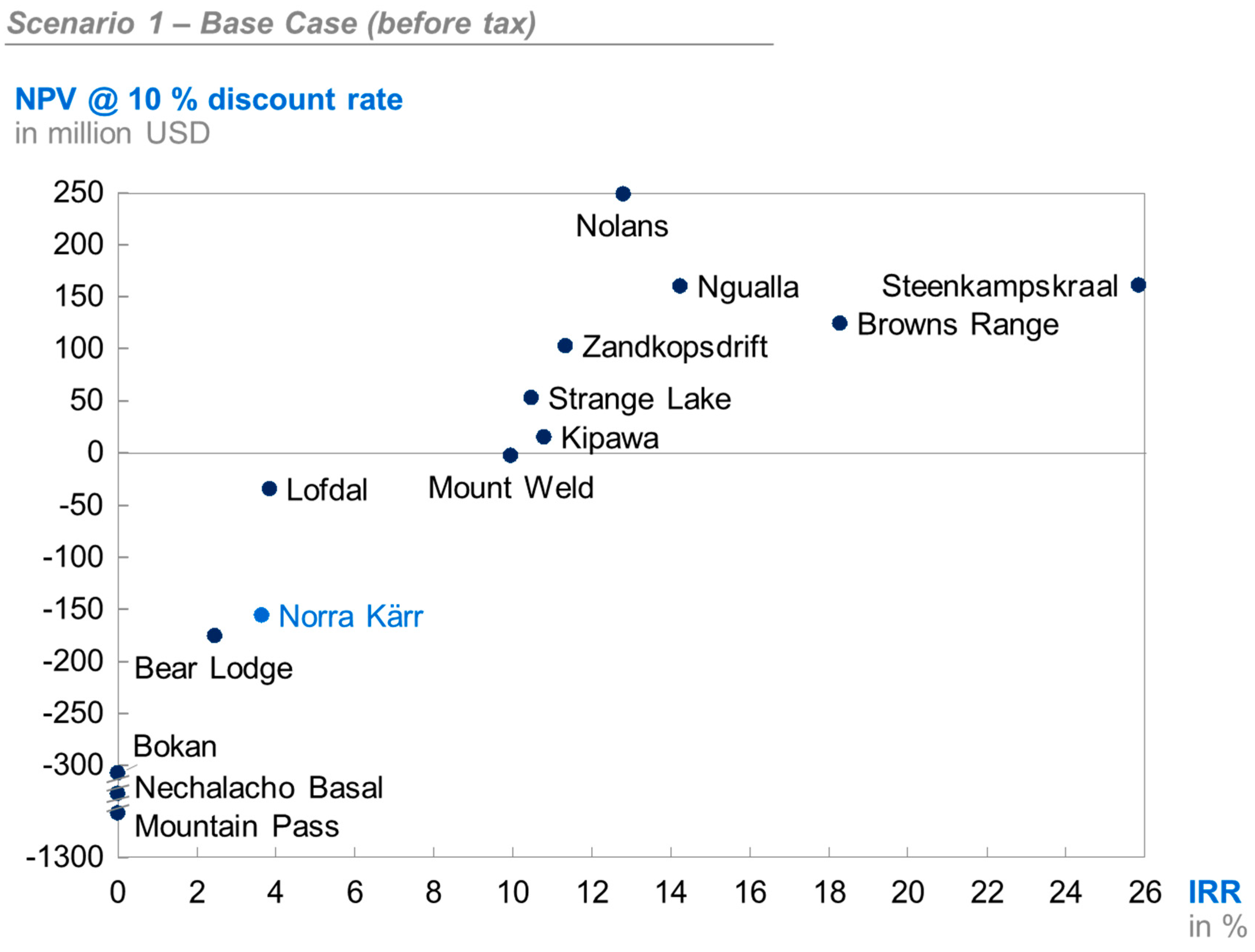
3.1.1. Scenario 1—Base Case
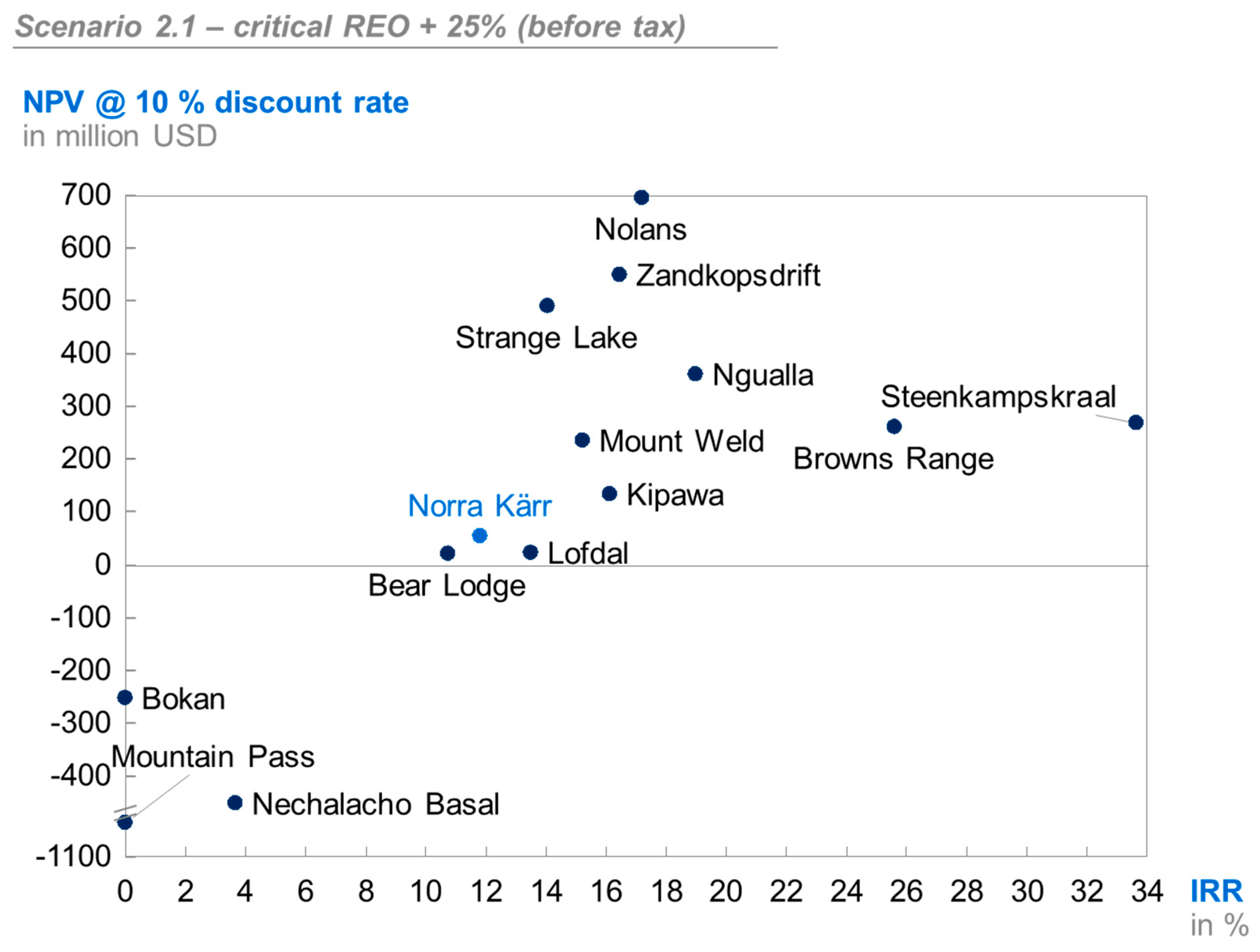
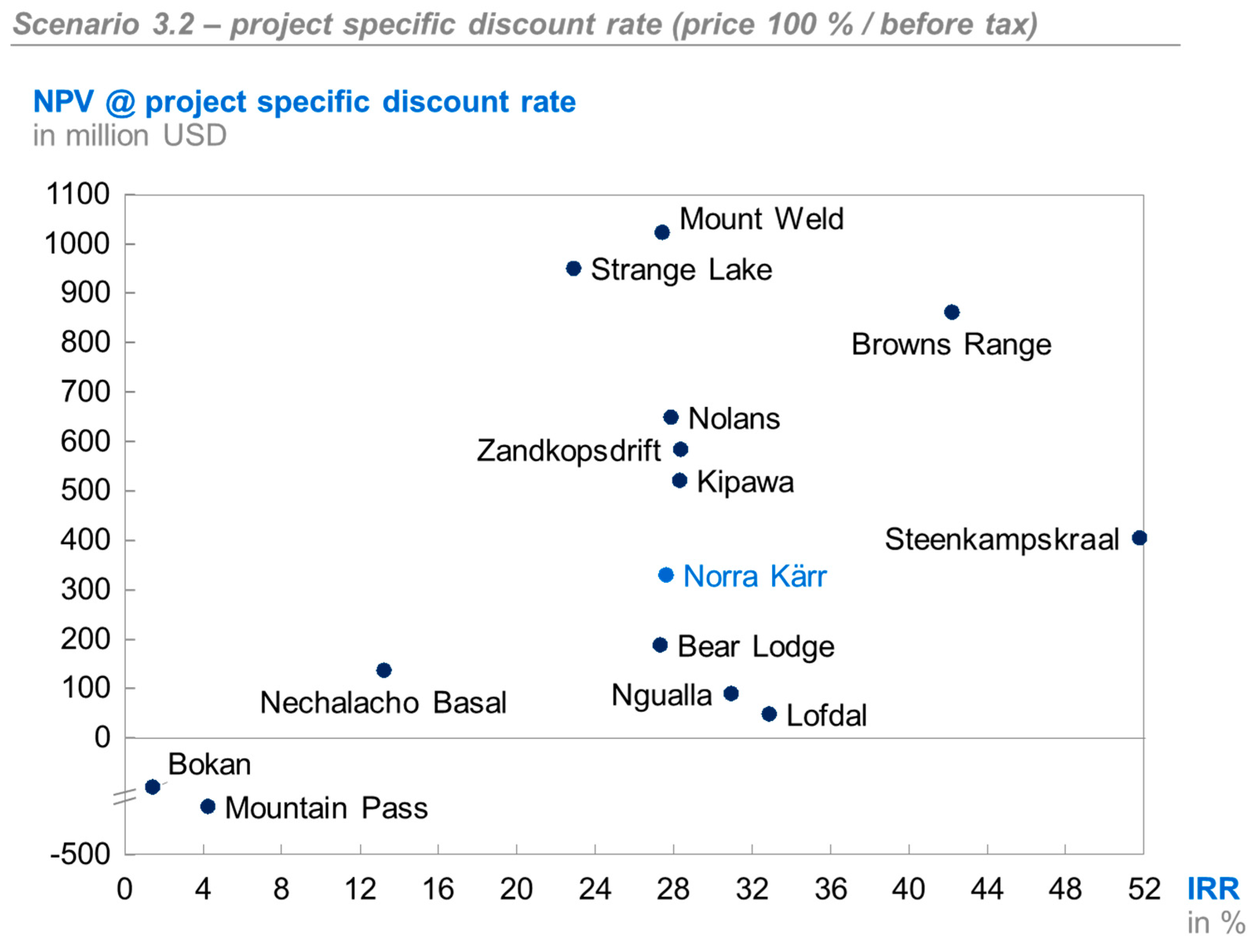
3.1.2. Scenario 2—Disproportionately Impact Price Development for Critical REO
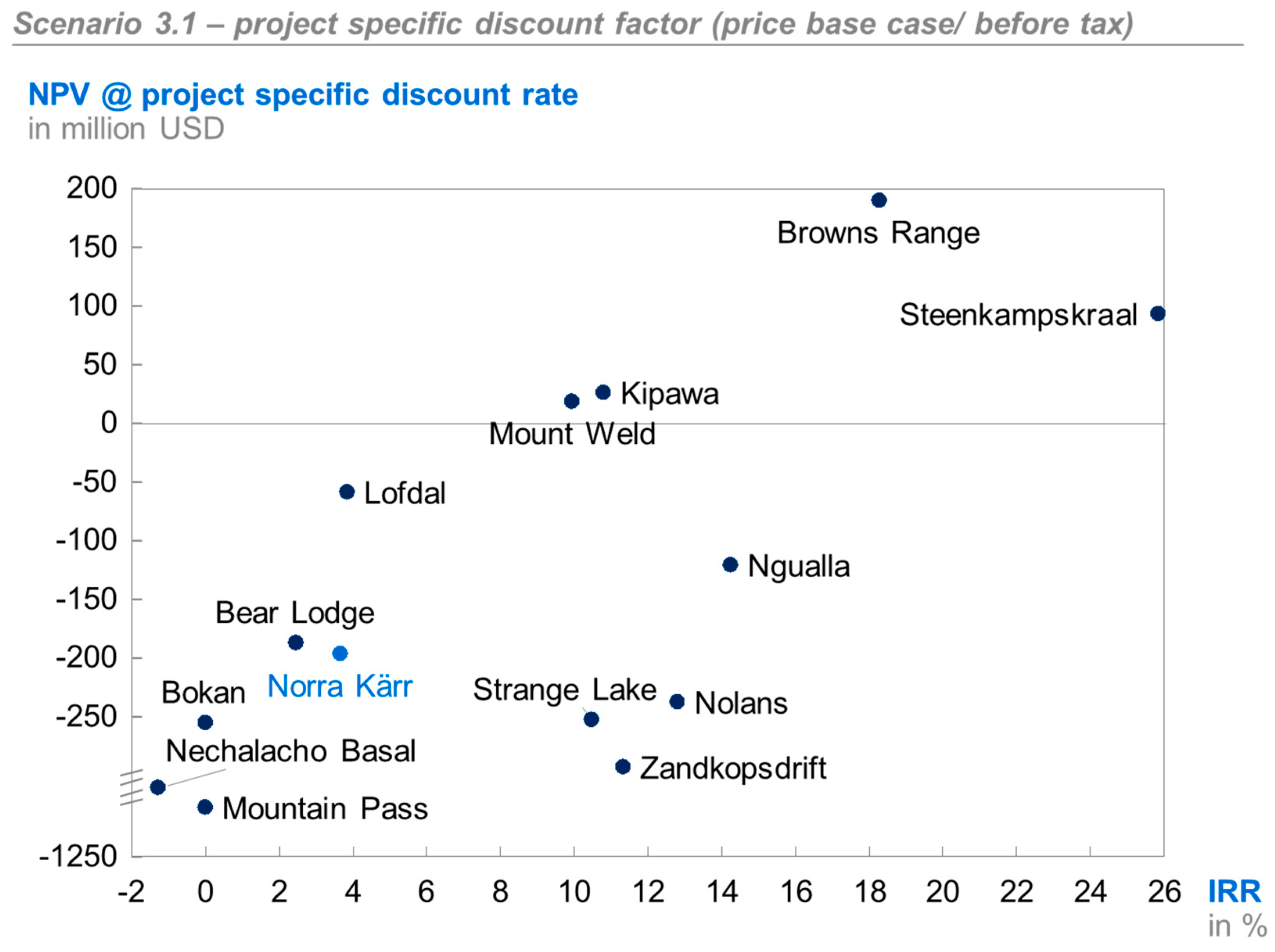
3.1.3. Scenario 3—Project-Specific Discount Rate
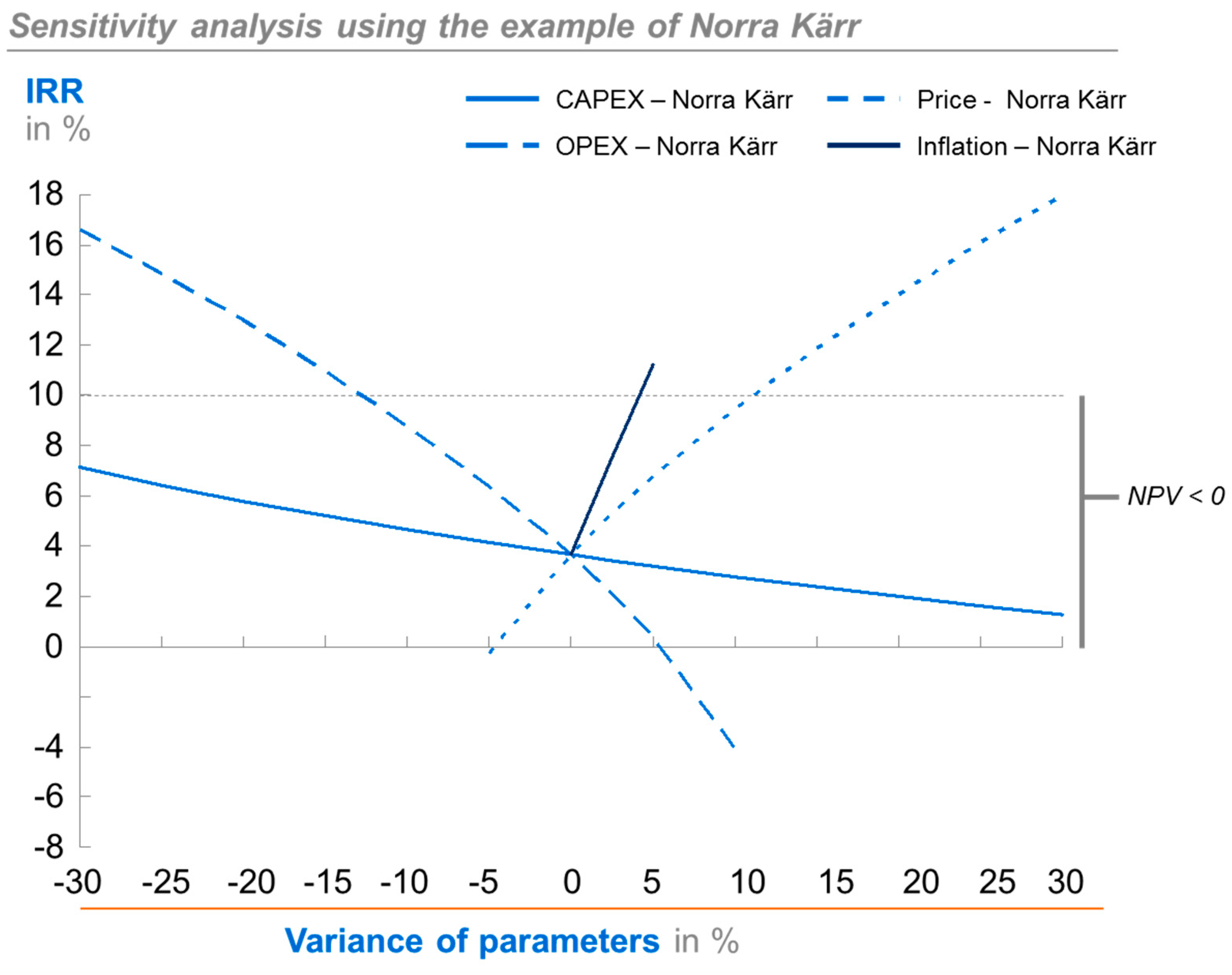
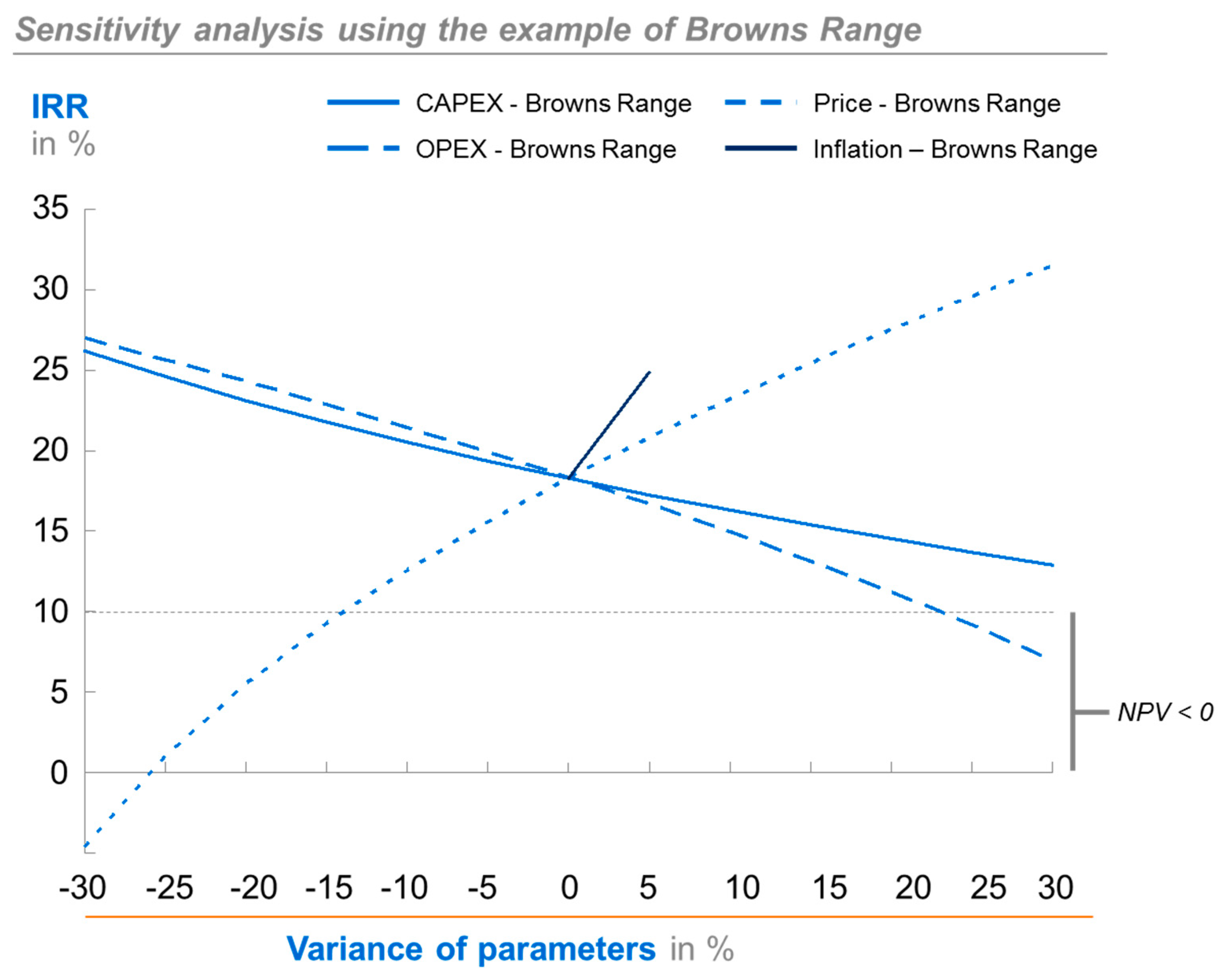
3.1.4. Sensitivity Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- U.S. Geological Survey. Mineral Commodity Summaries Rare Earth in the United States: U.S. Geological Survey Data Series. 2014. Available online: http://minerals.usgs.gov/minerals/pubs/commodity/rare_earths/mcs-2014-raree.pdf (accessed on 22 November 2016).
- MIDAS Research. Industry Report Seltene Metalle und Seltene Erden—Rohstoffe für das 21; Jahrhundert: Mannheim, Germany, 2011. [Google Scholar]
- Humphries, M. Rare Earth Elements: The Global Supply Chain; Congressional Research Service: Washington, DC, USA, 2013. [Google Scholar]
- Kramer, D. Concern grows over China’s dominance of rare-earth metals. Phys. Today 2010, 63, 22. [Google Scholar] [CrossRef]
- Stone, R. RAs China’s Rare Earth R&D Becomes Ever More Rarefied, Others Tremble. Science 2009, 325, 1336–1337. [Google Scholar] [PubMed]
- U.S. Department of Energy. Critical Materials Strategy. 2011. Available online: http://energy.gov/sites/prod/files/DOE_CMS2011_FINAL_Full.pdf (accessed on 22 November 2016).
- Report on Critical Raw Materials for the EU—Critical Raw Materials Profiles; European Commission: Brussels, Belgium, 2014; Available online: http://ec.europa.eu/DocsRoom/documents/11911/attachments/1/translations (accessed on 21 September 2019).
- Thiess, G. Minerals policy in Europe: Some recent developments. Resour. Policy 2010, 35, 190–198. [Google Scholar] [CrossRef]
- Massari, S.; Ruberti, M. Rare earth elements as critical raw materials: Focus on international markets and future strategies. Resour. Policy 2013, 38, 36–43. [Google Scholar] [CrossRef]
- Arafura Resource Limited. Nolans Development Report. 2014. Available online: https://www.arultd.com/images/150826_Mining_the_Territory_FINAL.pdf (accessed on 21 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 31 March 2014. Available online: https://www.lynascorp.com/wp-content/uploads/2019/04/140430-Q1-2014-Quarterly-Report-1324839.pdf (accessed on 19 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 30 June 2014. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/140929-Lynas-Financial-Report-2014.pdf (accessed on 20 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 30 September 2014. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/141015-Quarterly-Report-1373317.pdf (accessed on 20 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 31 December 2014. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/150313-Lynas-Interim-Report-31-Dec-14-1419268.pdf (accessed on 20 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 31 March 2015. Available online: https://www.lynascorp.com/wp-content/uploads/2019/04/150424-Quarterly-Activities-Report-1430062.pdf (accessed on 20 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 30 June 2015. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/150921-Financial-Report-year-ended-30-June-2015_1475481.pdf (accessed on 20 September 2019).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 30 September 2015. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/151014-Lynas-Quarterly-Activities-Report.pdf (accessed on 20 September).
- Lynas Coproration Ltd. Quarterly Report—Period Ending 31 December 2015. Available online: https://www.lynascorp.com/wp-content/uploads/2019/05/160310-Half-Year-Accounts-31-Dec-2015-1534548.pdf (accessed on 20 September 2019).
- Ciuculescu, T.; Foo, B.; Gowans, R.; Hawton, K.; Jacobs, C.; Spooner, J. Technical Report Disclosing the Results of the Feasibility Study on the Nechalacho Rare Earth Elements Project; Micon International Limited for Quest Rare Minerals: Toronto, ON, Canada, 2013. [Google Scholar]
- Micon International Limited; Quest Rare Minerals Ltd. NI 43-101 Technical Report on the Preliminary Economic Assessment (PEA) for the Strange Lake property Quebec, Canada; Micon International Limited for Quest Rare Minerals: Toronto, ON, Canada, 2014. [Google Scholar]
- Molycorp. Annual Report 2013. 2014. Available online: https://sec.report/Document/0001489137-14-000014/ (accessed on 21 September 2019).
- Molycorp. Molycorp Reports Fourth Quarter and Full Year 2014 Financial Results. 2015. Available online: https://sec.report/Document/0001489137-15-000007/ (accessed on 21 September 2019).
- Molycorp. Molycorp Reports Third Quarter 2014 Financial Results. 2015. Available online: https://sec.report/Document/0001489137-14-000140/ (accessed on 21 September 2019).
- Molycorp. Molycorp Reports Second Quarter 2014 Financial Results. 2015. Available online: https://sec.report/Document/0001489137-14-000117/ (accessed on 21 September 2019).
- Molycorp. Molycorp Reports First Quarter 2014 Financial Results. 2015. Available online: https://sec.report/Document/0001489137-14-000058/ (accessed on 21 September 2019).
- Northern Minerals. Browns Range Project Definitive Feasibility Study (Presentation). 2015. Available online: http://northernminerals.com.au/wp-content/uploads/2015/02/1503-02-Browns-Range-Definitive-Feasibility-Study-Presentation-.pdf (accessed on 15 November 2016).
- Press Release Namibia Rare Earth Inc. Namibia Rare Earth Files 43-101 Preliminary Economic Assessment Report for Heavy Rare Earth Mine at Lofdal. 2014. Available online: http://www.namibiarareearths.com/lofdal.asp (accessed on 6 November 2016).
- Bentzen, E.H.; Hassan, G.; Galbraith, L.; Hammen, R.F.; Robinson, R.J.; Hafez, S.A.; Annavarapu, S. Preliminary Economic Assessment on the Bokan Mountain Rare Earth Element Project near ketchikan, Alaska; Tetra Tech: Vancouver, BC, Canada, 2013. [Google Scholar]
- Dahlberg, P.S.; Noble, A.; Pickarts, J.T.; Rose, W.L.; Jaacks, J. Bear Lodge Project Canadian NI 43-10 Pre-Feasibility Study Report—Technical Report on the Mineral Reserves and Development of the Bull Hill Mine, Wyoming; Roche Engineering, Rare Element Resources Ltd.: Littleton, CO, USA, 2014. [Google Scholar]
- Roche Engineering, Matamec Explorations Inc. NI 43-101 Report—Feasibility Study for the Kipawa Project. 2013. Available online: http://matamec.com/vns-site/uploads/documents/061623-003-finrep_matamec-n143-101-20131017-001-appen.pdf (accessed on 22 November 2016).
- Peak Resource Limited. Ngualla Rare Earth Project—Preliminary Feasibility Study, Executive Summary. 2014. Available online: https://www.peakresources.com.au/ (accessed on 22 November 2016).
- Venmyn Rand; Frontier Rare Earths Limited. Amended Independent Technical Report on the Results of a Preliminary Economic Assessment of Frontier Rare Earth Limited’s Zandkopsdrift Rare Earth Project. 2012. Available online: http://www.frontierrareearths.com/projects.php (accessed on 22 November 2016).
- Venmyn Deloitte; South Africa for Great Western Minerals Group Ltd. National Instrument 43-101 Independent Technical Report on the Results of a Feasibility Study for the Steenkampskraal Rare Earth Element Project in the Western Cape. 2014. Available online: https://www.steenkampskraal.com (accessed on 22 April 2017).
- Legislative Counsel. Strategic and Critical Materials Stock Piling Act; 1939; Volume 50, p. 98. Available online: https://legcounsel.house.gov/Comps/Strategic%20And%20Critical%20Materials%20Stock%20Piling%20Act.pdf (accessed on 17 February 2017).
- Mason, E. An american view of raw materials problems: The report of the president’s materials policy commission. J. Ind. Econ. 1952, 1, 1–20. Available online: http://www.jstor.org/stable/2097676 (accessed on 17 February 2017). [CrossRef]
- Achzet, B. Empirische Analyse von Preis-Und Verfügbarkeitsbeeinflussenden Indikatoren Unter Berücksichtigung der Kritikalität von Rohstoffen; Disserta Verlag: Hamburg, Germany, 2012; ISBN 978-3954250929. [Google Scholar]
- Kausch, P.; Bertau, M.; Gutzmer, J.; Matschullat, J. Strategische Rohstoffe—Risikovorsorge; Springer Spektrum: Berlin/Heidelberg, Germany, 2014; ISBN 978-3-642-39704-2. [Google Scholar]
- Report on Critical Raw Materials for the EU; European Commission: Brussels, Belgium, 2014; Available online: http://ec.europa.eu/DocsRoom/documents/10010/attachments/1/translations (accessed on 21 September 2019).
- Jaroni, M.; Friedrich, B.; Letmathe, P. Criticality of Rare Earth Metals: A European Analysis; Working Paper; RWTH Aachen University: Aachen, Germany, 2017. [Google Scholar]
- Short, M.; Apelt, T.; Moseley, G.; Mounde, M.; Touche, G.D. Prefeasibility Study-NI 43-101-Technical Report for the Norra Kärr Rare Earth Element Deposit; GBM Minerals Engineering Consultants Ltd.: Twickenham, UK, 2015. [Google Scholar]
- Thomson Reuters Datastream, Rare Earth Prices February 2015, Datastream Professional Version 5.1. Available online: https://www.thomsonreuters.com/en.html (accessed on 23 August 2015).
- Thomson Reuters Datastream. Rare Earth Prices June 2015 Datastream Professional Version 5.1. Available online: https://www.thomsonreuters.com/en.html (accessed on 23 August 2015).
- Thomson Reuters Datastream. Rare Earth Prices August 2015 Datastream Professional Version 5.1. Available online: https://www.thomsonreuters.com/en.html (accessed on 23 August 2015).
- Kruschwitz, L. Investitionsrechnung; De Gruyter: Oldenbourg, Germany, 2014; ISBN 978-3110371734. [Google Scholar]
- Smith, L.D. Discounted Cash Flow Analysis—Methodology and Discount Rate. Available online: http://citeseerx.ist.psu.edu/viewdoc/download;jsessionid=543F8857AD3C3993C03674199C662AA9?doi=10.1.1.201.2710&rep=rep1&type=pdf (accessed on 19 September 2019).
- Baurens, S. Valuation of Metals and Mining Companies. 2010. Available online: http://www.basinvest.ch/upload/pdf/Valuation_of_Metals_and_Mining_Companies.pdf (accessed on 25 November 2016).
- Boerse.de. Konjunkturdaten. 2016. Available online: http://www.boerse.de/konjunkturdaten/staatsanleihen (accessed on 3 May 2016).
- Schacht, U.; Fackler, M. Praxishandbuch Unternehmensbewertung: Grundlagen, Methoden, Fallbeispiele; Gabler Verlag: Wiesbaden, Germany, 2015; ISBN 978-3409126984. [Google Scholar]

| Project | Country | Company | Capacity | SOP | LOM | Mining |
|---|---|---|---|---|---|---|
| in mt/years | Planned | in Years | ||||
| Steenkampskraal | ZAF | Great Western Minerals Group Ltd. | 2 | NA | 13 | UG |
| Mount Weld Phase 1 | AUS | Lynas Corporation Ltd. | 11 | 2013 | 25 | OP |
| Mountain Pass | USA | Molycorp Inc. | 20 | 2013 | OP | |
| Ngualla | TZA | Peak Resources Ltd. | 10 | 2017 | 58 | OP |
| Bear Lodge | USA | Rare Element Resources Ltd. | 7.5 | 2016 | 45 | OP |
| Nolans | AUS | Arafura Resources Ltd. | 20 | 2018 | 23 | OP |
| Zandkopsdrift (JV) | ZAF | Frontier Rare Earths Ltd. | 20 | NA | 20 | OP |
| Nechalacho Basal | CAN | Avalon Rare Metals Inc. | 7 | 2018 | 20 | UG |
| Strange Lake | CAN | Quest Rare Minerals Ltd. | 10 | 2019 | 30 | OP |
| Brwons Range | AUS | Northern Minerals Limited | 3 | 2017 | 11 | OP |
| Lofdal | NAM | Namibia Rare Earths Inc. | 1.5 | NA | 7.25 | OP |
| Bokan | USA | Ucore Rare Metals Inc. | 1.8 | NA | 11 | UG |
| Norra Kärr | SWE | Tasman Metals Ltd. | 4.8 | NA | 20 | OP |
| Kipawa | CAN | Matamec Explorations Inc. | 3.6 | NA | 15.25 | OP |
| Project | Basket Price in USD/kg | REO Content in Ore | Thereof Critical REO 1 Content | |||
|---|---|---|---|---|---|---|
| Base Case | + 25% | + 50% | + 100% | In % w/w | In % | |
| Steenkampskraal | 61.89 | 72.99 | 84.09 | 106.30 | 4.7 | 70 |
| Mount Weld CLD Phase 1 | 21.54 | 24.96 | 28.39 | 35.24 | 9.7 | 20 |
| Mountain Pass | 14.69 | 16.58 | 18.47 | 22.24 | 6.6 | 12 |
| Ngualla | 18.87 | 21.74 | 24.62 | 30.36 | 4.2 | 17 |
| Bear Lodge | 22.95 | 26.76 | 30.56 | 38.16 | 2.7 | 21 |
| Nolans | 22.73 | 26.35 | 29.97 | 37.22 | 2.6 | 23 |
| Zandkopsdrift (JV) | 22.53 | 26.33 | 30.13 | 37.74 | 2.2 | 22 |
| Nechalacho Basal | 36.42 | 42.41 | 48.40 | 60.37 | 1.4 | 33 |
| Strange Lake | 48.40 | 55.07 | 61.74 | 75.08 | 1.4 | 48 |
| Brwons Range | 68.21 | 78.72 | 89.23 | 110.25 | 0.7 | 70 |
| Lofdal | 70.63 | 81.40 | 92.16 | 113.70 | 0.6 | 70 |
| Bokan | 42.93 | 50.00 | 57.06 | 71.18 | 0.6 | 45 |
| Norra Kärr | 46.69 | 53.67 | 60.65 | 74.61 | 0.6 | 51 |
| Kipawa | 40.07 | 46.17 | 52.27 | 64.47 | 0.4 | 40 |
| Project | Basketprice | LOM | NPV @ 10% | IRR |
|---|---|---|---|---|
| in USD/kg | in Years | in USD | in % | |
| Steenkampskraal | 61.89 | 13.00 | 161 | 25.8 |
| Mount Weld CLD Phase 1 | 21.54 | 25.00 | −249 | 9.9 |
| Mountain Pass | 14.69 | −1257 | - | |
| Ngualla | 18.87 | 58.00 | 161 | 14.2 |
| Bear Lodge | 22.95 | 45.00 | −175 | 2.5 |
| Nolans | 22.73 | 23.00 | 250 | 12.8 |
| Zandkopsdrift (JV) | 22.53 | 20.00 | 103 | 11.3 |
| Nechalacho Basal | 36.42 | 20.00 | −698 | −1.3 |
| Strange Lake | 48.40 | 30.00 | 53 | 10.5 |
| Brwons Range | 68.21 | 11.00 | 125 | 18.3 |
| Lofdal | 70.63 | 7.25 | −34 | 3.8 |
| Bokan | 42.93 | 11.00 | −308 | - |
| Norra Kärr | 46.69 | 20.00 | −155 | 3.7 |
| Kipawa | 40.07 | 15.20 | 15 | 10.8 |
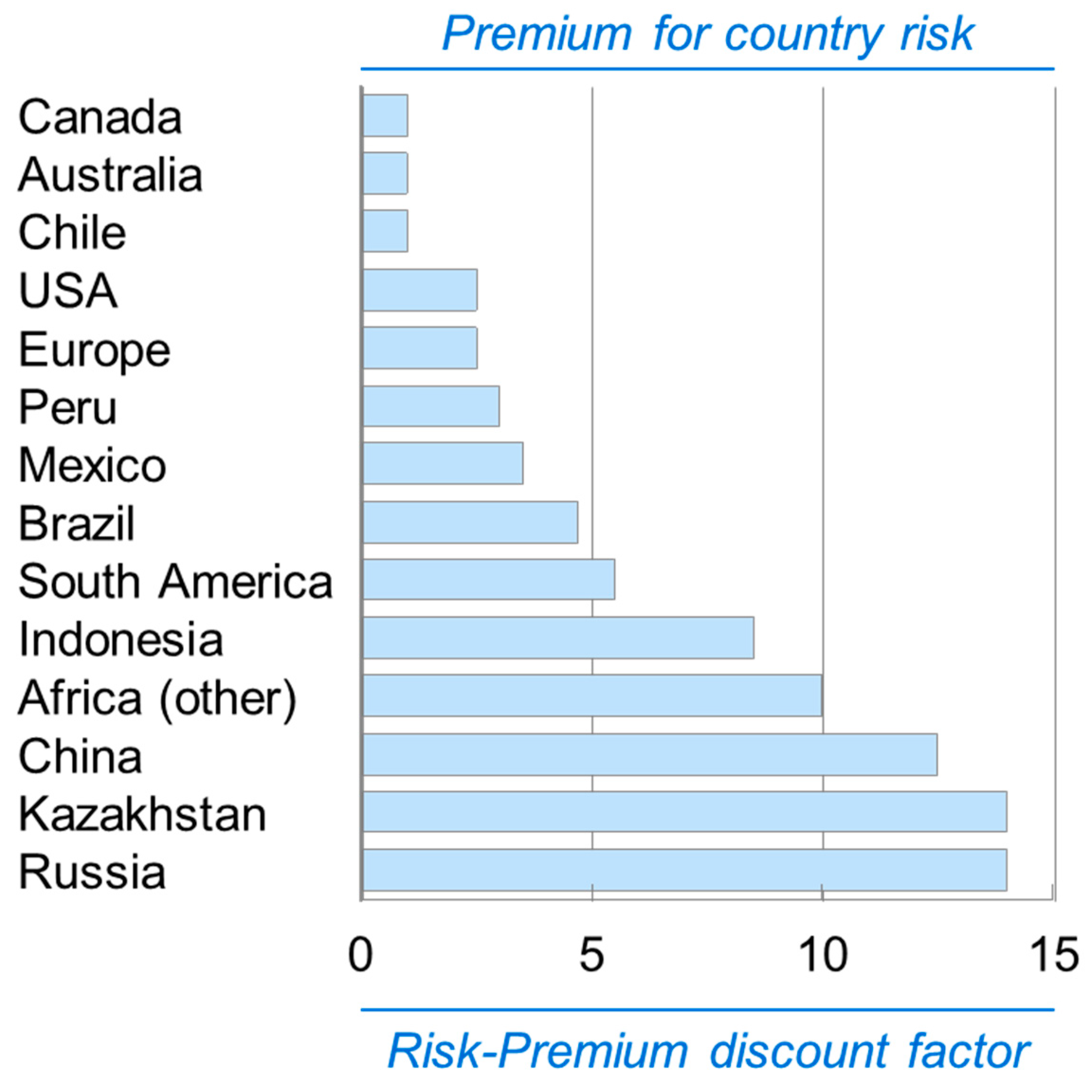
| Project | Stage | Premium | LOM | Premium | Country | Premium | Discount Rate |
|---|---|---|---|---|---|---|---|
| (May 2015) | in % | in Years | in % | in % | in % | ||
| Steenkampskraal | PFS | 4 | 13 | 2 | ZAF | 5.5 | 14.0 |
| Mount Weld CLD Phase 1 | in prod. | 0 | 25 | 6 | AUS | 1.0 | 9.5 |
| Mountain Pass | in prod. | 0 | 6 | USA | 2.5 | 11.0 | |
| Ngualla | PFS 2014 | 4 | 58 | 8 | TZA | 10.0 | 24.5 |
| Bear Lodge | PFS 2014 | 4 | 45 | 8 | USA | 2.5 | 17.0 |
| Nolans | PEA 2013 | 8 | 23 | 6 | AUS | 1.0 | 17.5 |
| Zandkopsdrift (JV) | PFS 2015 | 4 | 20 | 6 | ZAF | 5.5 | 18.0 |
| Nechalacho Basal | FS 2013 | 2 | 20 | 6 | CAN | 1.0 | 11.5 |
| Strange Lake | PFS 2013 | 4 | 30 | 6 | CAN | 1.0 | 13.5 |
| Brwons Range | DFS 2015 | 2 | 11 | 2 | AUS | 1.0 | 7.5 |
| Lofdal | PEA 2014 | 8 | 7.25 | 2 | NAM | 10.0 | 22.5 |
| Bokan | PEA 2013 | 8 | 11 | 2 | USA | 2.5 | 15.0 |
| Norra Kärr | PFS 2014 | 4 | 20 | 6 | SWE | 2.5 | 15.0 |
| Kipawa | DFS 2013 | 2 | 15.25 | 4 | CAN | 1.0 | 9.5 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jaroni, M.S.; Friedrich, B.; Letmathe, P. Economical Feasibility of Rare Earth Mining outside China. Minerals 2019, 9, 576. https://doi.org/10.3390/min9100576
Jaroni MS, Friedrich B, Letmathe P. Economical Feasibility of Rare Earth Mining outside China. Minerals. 2019; 9(10):576. https://doi.org/10.3390/min9100576
Chicago/Turabian StyleJaroni, Marie Sophie, Bernd Friedrich, and Peter Letmathe. 2019. "Economical Feasibility of Rare Earth Mining outside China" Minerals 9, no. 10: 576. https://doi.org/10.3390/min9100576
APA StyleJaroni, M. S., Friedrich, B., & Letmathe, P. (2019). Economical Feasibility of Rare Earth Mining outside China. Minerals, 9(10), 576. https://doi.org/10.3390/min9100576






