Relating Topological and Electrical Properties of Fractured Porous Media: Insights into the Characterization of Rock Fracturing
Abstract
1. Introduction
2. Methodological Background
2.1. Electrical Parameters
2.2. Modeling Approach
2.3. Numerical Simulations
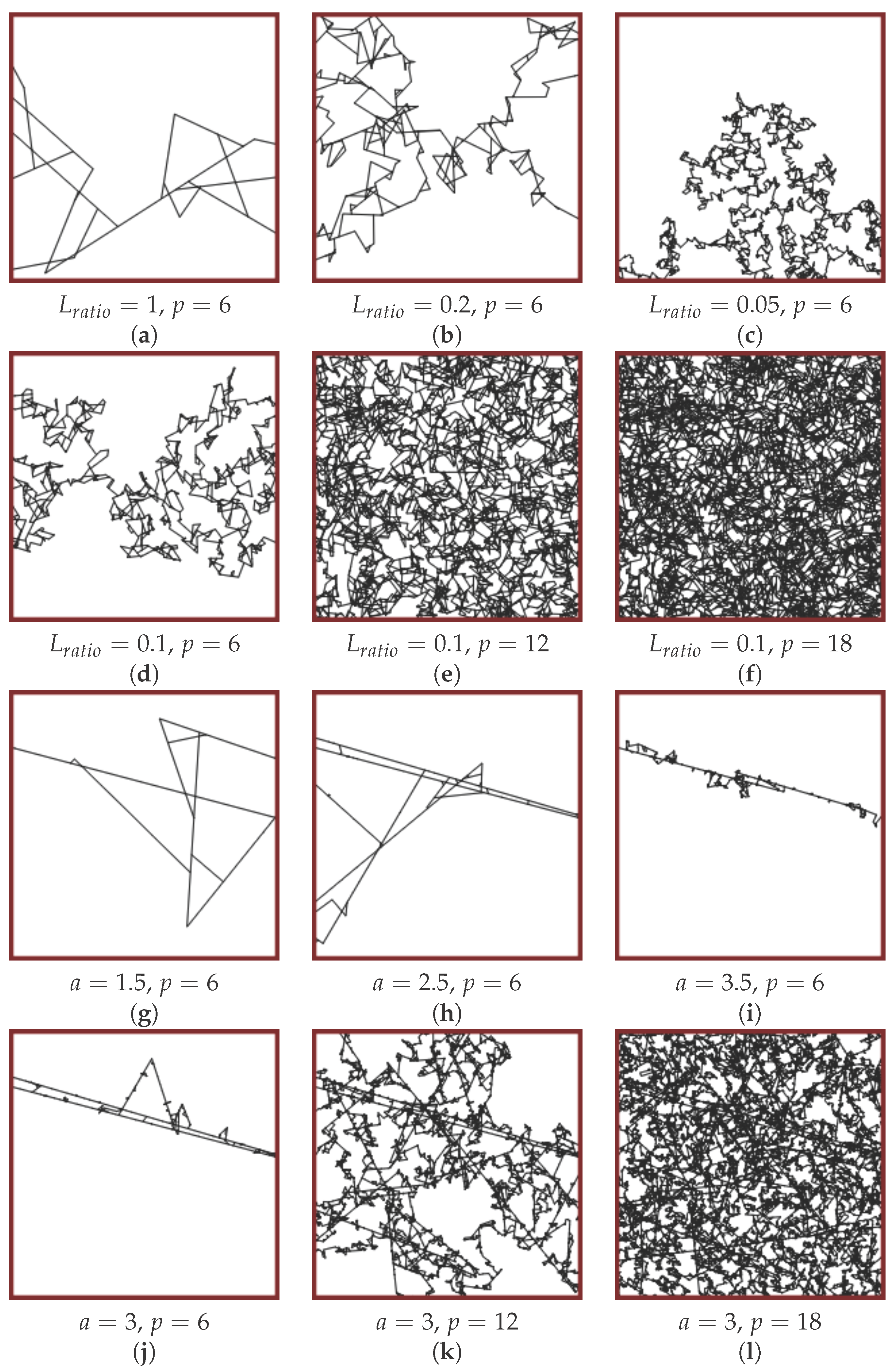
3. Results for Complex Fractured Porous Media
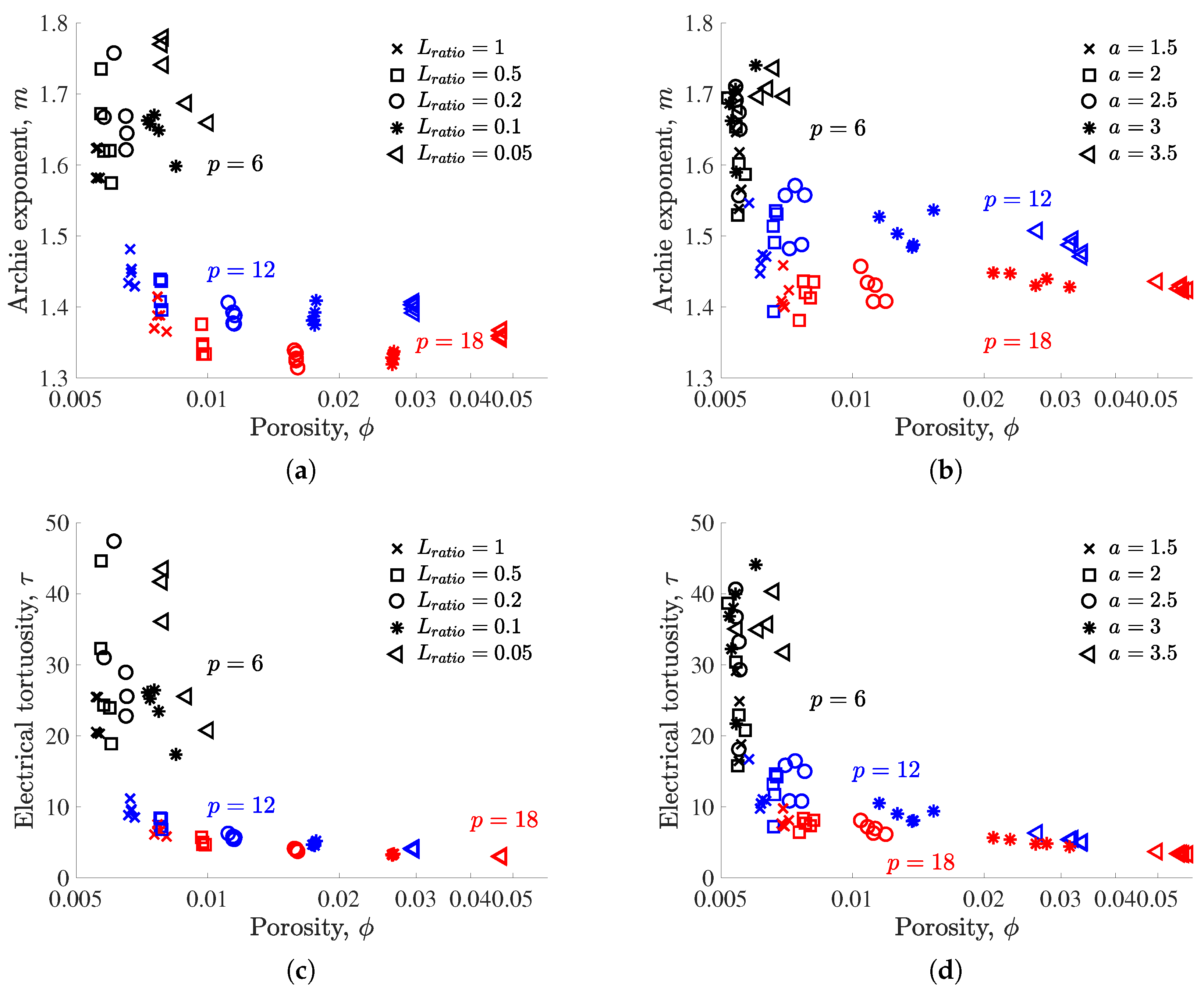
3.1. Results for a Large Range of Fracture Densities
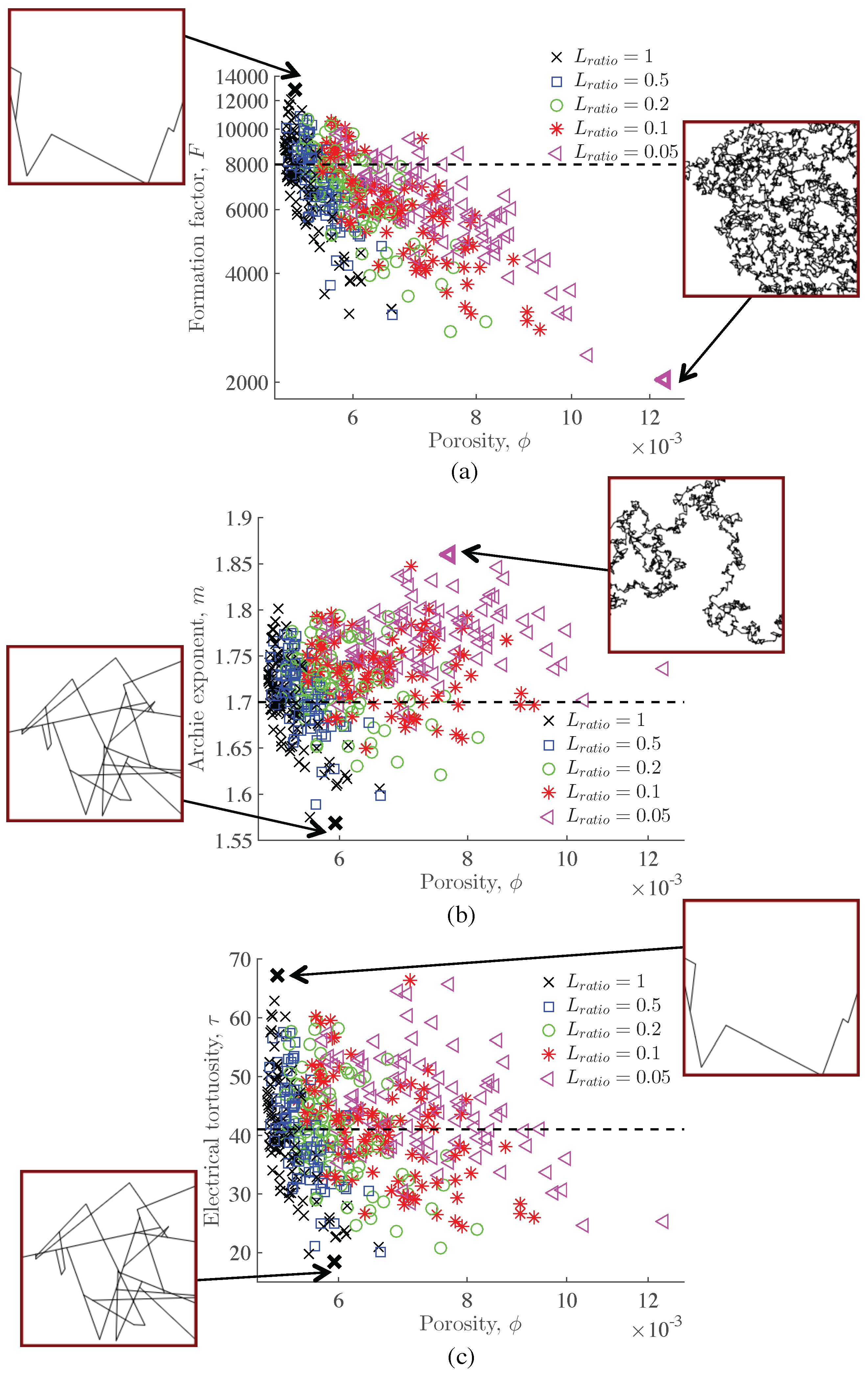
3.2. Results at the Fracture Percolation Threshold
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Garg, S.K.; Pritchett, J.W.; Wannamaker, P.E.; Combs, J. Characterization of geothermal reservoirs with electrical surveys: Beowawe geothermal field. Geothermics 2007, 36, 487–517. [Google Scholar] [CrossRef]
- Kazakis, N.; Vargemezis, G.; Voudouris, K.S. Estimation of hydraulic parameters in a complex porous aquifer system using geoelectrical methods. Sci. Total Environ. 2016, 550, 742–750. [Google Scholar] [CrossRef] [PubMed]
- Priegnitz, M.; Thaler, J.; Spangenberg, E.; Schicks, J.M.; Schroetter, J.; Abendroth, S. Characterizing electrical properties and permeability changes of hydrate bearing sediments using ERT data. Geophys. J. Int. 2015, 202, 1599–1612. [Google Scholar] [CrossRef]
- Carneiro, J.F. Numerical simulations on the influence of matrix diffusion to carbon sequestration in double porosity fissured aquifers. Int. J. Greenh. Gas Control 2009, 3, 431–443. [Google Scholar] [CrossRef]
- Kolditz, O.; Clauser, C. Numerical simulation of flow and heat transfer in fractured crystalline rocks: Application to the Hot Dry Rock site in Rosemanowes (U.K.). Geothermics 1998, 27, 1–23. [Google Scholar] [CrossRef]
- Lee, M.W.; Collett, T.S. Gas hydrate saturations estimated from fractured reservoir at Site NGHP-01-10, Krishna-Godavari Basin, India. J. Geophys. Res. Solid Earth 2009, 114. [Google Scholar] [CrossRef]
- Berkowitz, B. Characterizing flow and transport in fractured geological media: A review. Adv. Water Resour. 2002, 25, 861–884. [Google Scholar] [CrossRef]
- Bonnet, E.; Bour, O.; Odling, N.E.; Davy, P.; Main, I.; Cowie, P.; Berkowitz, B. Scaling of fracture systems in geological media. Rev. Geophys. 2001, 39, 347–383. [Google Scholar] [CrossRef]
- Neuman, S. Trends, prospects and challenges in quantifying flow and transport through fractured rocks. Hydrogeol. J. 2005, 13, 124–147. [Google Scholar] [CrossRef]
- Dorn, C.; Linde, N.; Le Borgne, T.; Bour, O.; de Dreuzy, J.R. Conditioning of stochastic 3-D fracture networks to hydrological and geophysical data. Adv. Water Resour. 2013, 62, 79–89. [Google Scholar] [CrossRef]
- Le Borgne, T.; Bour, O.; Riley, M.S.; Gouze, P.; Pezard, P.A.; Belghoul, A.; Lods, G.; Le Provost, R.; Greswell, R.B.; Ellis, P.A.; et al. Comparison of alternative methodologies for identifying and characterizing preferential flow paths in heterogeneous aquifers. J. Hydrol. 2007, 345, 134–148. [Google Scholar] [CrossRef]
- Lofi, J.; Pezard, P.; Loggia, D.; Garel, E.; Gautier, S.; Merry, C.; Bondabou, K. Geological discontinuities, main flow path and chemical alteration in a marly hill prone to slope instability: Assessment from petrophysical measurements and borehole image analysis. Hydrol. Process. 2012, 26. [Google Scholar] [CrossRef]
- Daily, W.D.; Ramirez, A.L. In situ porosity distribution using geophysical tomography. Geophys. Res. Lett. 1984, 11, 614–616. [Google Scholar] [CrossRef]
- Kazatchenko, E.; Mousatov, A. Primary and Secondary Porosity Estimation of Carbonate Formations Using Total Porosity and the Formation Factor. Soc. Pet. Eng. 2002. [Google Scholar] [CrossRef]
- Whitman, D.; Yeboah-Forson, A. Electrical resistivity and porosity structure of the upper Biscayne Aquifer in Miami-Dade County, Florida. J. Hydrol. 2015, 531, 781–791. [Google Scholar] [CrossRef]
- Archie, G. The electrical resistivity log as an aid in determining some reservoir characteristics. Trans. Am. Inst. Min. Metall. Eng. 1942, 146, 54–61. [Google Scholar] [CrossRef]
- Katsube, T.J.; Hume, J.P. Permeability determination in crystalline rocks by standard geophysical logs. Geophysics 1987, 52, 342–352. [Google Scholar] [CrossRef]
- Pezard, P.A.; Luthi, S.M. Borehole electrical images in the basement of the Cajon Pass Scientific Drillhole, California; Fracture identification and tectonic implications. Geophys. Res. Lett. 1988, 15, 1017–1020. [Google Scholar] [CrossRef]
- Boadu, F.; Gyamfi, J.; Owusu, E. Determining subsurface fracture characteristics from azimuthal resistivity surveys: A case study at Nsawam, Ghana. Geophysics 2005, 70, B35–B42. [Google Scholar] [CrossRef]
- Caballero Sanz, V.; Roubinet, D.; Demirel, S.; Irving, J. 2.5-D discrete-dual-porosity model for simulating geoelectrical experiments in fractured rock. Geophys. J. Int. 2017, 209, 1099–1110. [Google Scholar] [CrossRef]
- Lesparre, N.; Boyle, A.; Grychtol, B.; Cabrera, J.; Marteau, J.; Adler, A. Electrical resistivity imaging in transmission between surface and underground tunnel for fault characterization. J. Appl. Geophys. 2016, 128, 163–178. [Google Scholar] [CrossRef]
- Le Pennec, J.L.; Hermitte, D.; Dana, I.; Pezard, P.; Coulon, C.; Cochemé, J.J.; Mulyadi, E.; Ollagnier, F.; Revest, C. Electrical conductivity and pore-space topology of Merapi Lavas: Implications for the degassing of porphyritic andesite magmas. Geophys. Res. Lett. 2001, 28, 4283–4286. [Google Scholar] [CrossRef]
- Liu, Y.; Xue, Z.; Park, H.; Kiyama, T.; Zhang, Y.; Nishizawa, O.; Chae, K.S. Measurement of electrical impedance of a Berea sandstone core during the displacement of saturated brine by oil and CO2 injections. J. Appl. Geophys. 2015, 123, 50–62. [Google Scholar] [CrossRef]
- Sen, P.N.; Scala, C.; Cohen, M.H. A self-similar model for sedimentary rocks with application to the dielectric constant of fused glass beads. Geophysics 1981, 46, 781–795. [Google Scholar] [CrossRef]
- Wright, H.M.; Cashman, K.V. Compaction and gas loss in welded pyroclastic deposits as revealed by porosity, permeability, and electrical conductivity measurements of the Shevlin Park Tuff. Geol. Soc. Am. Bull. 2014, 126, 234–247. [Google Scholar] [CrossRef]
- Al-Ghamdi, A.; Chen, B.; Behmanesh, H.; Qanbari, F.; Aguilera, R. An Improved Triple-Porosity Model for Evaluation of Naturally Fractured Reservoirs. SPE Reserv. Eval. Eng. 2011, 14, 397–404. [Google Scholar] [CrossRef]
- Bauer, D.; Youssef, S.; Han, M.; Bekri, S.; Rosenberg, E.; Fleury, M.; Vizika, O. From computed microtomography images to resistivity index calculations of heterogeneous carbonates using a dual-porosity pore-network approach: Influence of percolation on the electrical transport properties. Phys. Rev. E 2011, 84, 011133. [Google Scholar] [CrossRef] [PubMed]
- Glover, P.W.J. A generalized Archie’s law for n phases. Geophysics 2010, 75, E247–E265. [Google Scholar] [CrossRef]
- Revil, A.; Cathles, L.M. Permeability of shaly sands. Water Resour. Res. 1999, 35, 651–662. [Google Scholar] [CrossRef]
- Torskaya, T.; Shabro, V.; Torres-Verdín, C.; Salazar-Tio, R.; Revil, A. Grain Shape Effects on Permeability, Formation Factor, and Capillary Pressure from Pore-Scale Modeling. Transp. Porous Media 2013, 102, 71–90. [Google Scholar] [CrossRef]
- Ghanbarian, B.; Hunt, A.G.; Ewing, R.P.; Skinner, T.E. Universal scaling of the formation factor in porous media derived by combining percolation and effective medium theories. Geophys. Res. Lett. 2014, 41, 3884–3890. [Google Scholar] [CrossRef]
- Winsauer, W.O. Resistivity of brine-saturated sands in relation to pore geometry. AAPG Bull. 1952, 36, 253–277. [Google Scholar]
- Ildefonse, B.; Pezard, P. Electrical properties of slow-spreading ridge gabbros from ODP Site 735, Southwest Indian Ridge. Tectonophysics 2001, 330, 69–92. [Google Scholar] [CrossRef]
- Pezard, P.; Ito, H.; Hermitte, D.; Revil, A. Electrical properties and alteration of granodiorites from the GSJ Hirabayashi Hole, Japan. In Proceedings of the International Workshop of the Nojima Fault Core and Borehole Data Analysis, Tsukuba, Japan, 22–23 November 1999; Geological Survey of Japan; Number EQ/00/1 in USGS Open-File Report 000-129. Ito, H., Fujimoto, K., Tanaka, H., Lockner, D., Eds.; pp. 255–262. [Google Scholar]
- Violay, M.; Pezard, P.A.; Ildefonse, B.; Belghoul, A.; Laverne, C. Petrophysical properties of the root zone of sheeted dikes in the ocean crust: A case study from Hole ODP/IODP 1256D, Eastern Equatorial Pacific. Tectonophysics 2010, 493, 139–152. [Google Scholar] [CrossRef]
- Berg, C.R. Dual-Porosity Equations From Effective Medium Theory. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006; Society of Petroleum Engineers: Richardson, TX, USA, 2006. [Google Scholar]
- Long, J.C.S.; Remer, J.S.; Wilson, C.R.; Witherspoon, P.A. Porous media equivalents for networks of discontinuous fractures. Water Resour. Res. 1982, 18, 645–658. [Google Scholar] [CrossRef]
- De Dreuzy, J.R.; Davy, P.; Bour, O. Hydraulic properties of two-dimensional random fracture networks following a power law length distribution: 1. Effective connectivity. Water Resour. Res. 2001, 37, 2065–2078. [Google Scholar] [CrossRef]
- Roubinet, D.; de Dreuzy, J.R.; Davy, P. Connectivity-consistent mapping method for 2-D discrete fracture networks. Water Resour. Res. 2010, 46, W07532. [Google Scholar] [CrossRef]
- De Dreuzy, J.R.; Davy, P.; Bour, O. Hydraulic properties of two-dimensional random fracture networks following a power law length distribution: 2. Permeability of networks based on lognormal distribution of apertures. Water Resour. Res. 2001, 37, 2079–2095. [Google Scholar] [CrossRef]
- Schön, J. Physical Properties of Rocks. In Physical Properties of Rocks-A Workbook; Schön, J., Ed.; Elsevier: Amsterdam, The Netherlands, 2011; Volume 8. [Google Scholar]
- Walsh, J.B.; Brace, W.F. The effect of pressure on porosity and the transport properties of rock. J. Geophys. Res. Solid Earth 1984, 89, 9425–9431. [Google Scholar] [CrossRef]
- Pape, H.; Riepe, L.; Schopper, J.R. Petrophysical Detection Of Microfissures In Granites. In Proceedings of the Society of Petrophysicists and Well-Log Analysts (SPWLA) 26th Annual Logging Symposium, Dallas, TX, USA, 17–20 June 1985. [Google Scholar]
- Selley, R.C.; Sonnenberg, S.A. (Eds.) Chapter 3-Methods of Exploration. In Elements of Petroleum Geology, 3rd ed.; Academic Press: Boston, MA, USA, 2015; pp. 41–152. [Google Scholar]
- Selley, R.C.; Sonnenberg, S.A. (Eds.) Chapter 6-The Reservoir. In Elements of Petroleum Geology, 3rd ed.; Academic Press: Boston, MA, USA, 2015; pp. 255–320. [Google Scholar]
- Wright, H.M.; Cashman, K.V.; Gottesfeld, E.H.; Roberts, J.J. Pore structure of volcanic clasts: Measurements of permeability and electrical conductivity. Earth Planet. Sci. Lett. 2009, 280, 93–104. [Google Scholar] [CrossRef]
- Pezard, P.A. Electrical properties of mid-ocean ridge basalt and implications for the structure of the upper oceanic crust in Hole 504B. J. Geophys. Res. Solid Earth 1990, 95, 9237–9264. [Google Scholar] [CrossRef]
- Sunberg, K. Effect on impregnating waters on electrical conductivity of soils and rocks. Trans. Am. Inst. Min. Metal. Eng. 1932, 79, 367–391. [Google Scholar]
- Brace, W.F.; Orange, A.S.; Madden, T.R. The effect of pressure on the electrical resistivity of water-saturated crystalline rocks. J. Geophys. Res. 1965, 70, 5669–5678. [Google Scholar] [CrossRef]
- Roubinet, D.; Irving, J. Discrete-dual-porosity model for electric current flow in fractured rock. J. Geophys. Res. Solid Earth 2014, 119, 767–786. [Google Scholar] [CrossRef]
- Bour, O.; Davy, P. Connectivity of random fault networks following a power law fault length distribution. Water Resour. Res. 1997, 33, 1567–1583. [Google Scholar] [CrossRef]
- Markov, M.; Mousatov, A.; Kazatchenko, E.; Markova, I. Determination of electrical conductivity of double-porosity formations by using generalized differential effective medium approximation. J. Appl. Geophys. 2014, 108, 104–109. [Google Scholar] [CrossRef]
- Daigle, H.; Ghanbarian, B.; Henry, P.; Conin, M. Universal scaling of the formation factor in clays: Example from the Nankai Trough. J. Geophys. Res. Solid Earth 2015, 120, 7361–7375. [Google Scholar] [CrossRef]
- Faulkner, D.; Jackson, C.; Lunn, R.; Schlische, R.; Shipton, Z.; Wibberley, C.; Withjack, M. A review of recent developments concerning the structure, mechanics and fluid flow properties of fault zones. J. Struct. Geol. 2010, 32, 1557–1575. [Google Scholar] [CrossRef]

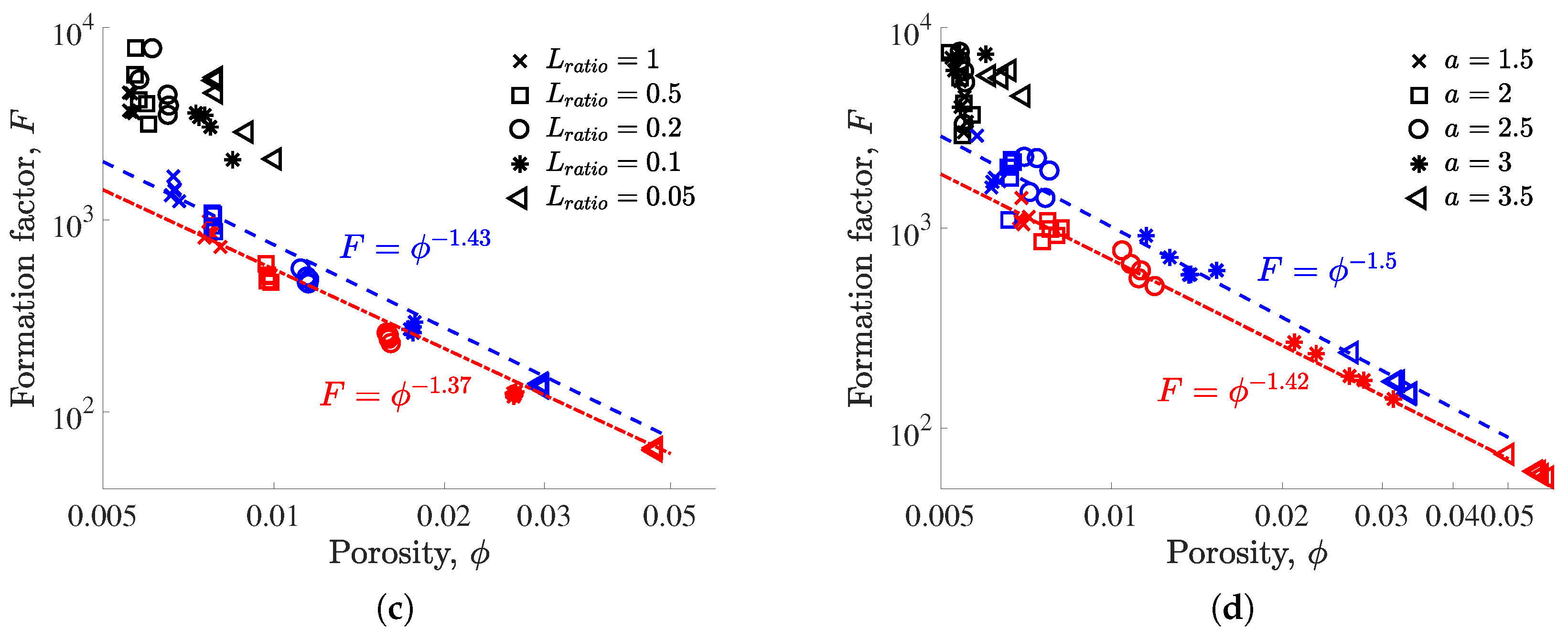


| p (Figure 2c) | 6 | ||||
| 12 | |||||
| 18 | |||||
| p (Figure 2d) | 6 | 0 | |||
| 12 | |||||
| 18 | |||||
| (Figure 6a) | 1 | 33 | ||||||
| 38 | ||||||||
| 38 | ||||||||
| 1.74 | 40 | |||||||
| 1.77 | 0.94 | 42 | 1.77 | 0.94 | ||||
| a (Figure 6b) | 37 | |||||||
| 2 | 35 | |||||||
| 37 | ||||||||
| 3 | 39 | |||||||
| 42 | ||||||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roubinet, D.; Irving, J.; Pezard, P.A. Relating Topological and Electrical Properties of Fractured Porous Media: Insights into the Characterization of Rock Fracturing. Minerals 2018, 8, 14. https://doi.org/10.3390/min8010014
Roubinet D, Irving J, Pezard PA. Relating Topological and Electrical Properties of Fractured Porous Media: Insights into the Characterization of Rock Fracturing. Minerals. 2018; 8(1):14. https://doi.org/10.3390/min8010014
Chicago/Turabian StyleRoubinet, Delphine, James Irving, and Philippe A. Pezard. 2018. "Relating Topological and Electrical Properties of Fractured Porous Media: Insights into the Characterization of Rock Fracturing" Minerals 8, no. 1: 14. https://doi.org/10.3390/min8010014
APA StyleRoubinet, D., Irving, J., & Pezard, P. A. (2018). Relating Topological and Electrical Properties of Fractured Porous Media: Insights into the Characterization of Rock Fracturing. Minerals, 8(1), 14. https://doi.org/10.3390/min8010014





