Extraction Kinetics of Rare Earth Elements from Ion-Adsorbed Underclays
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Preparation and Analysis
2.2. Kinetic Modeling Methods
3. Results and Discussion
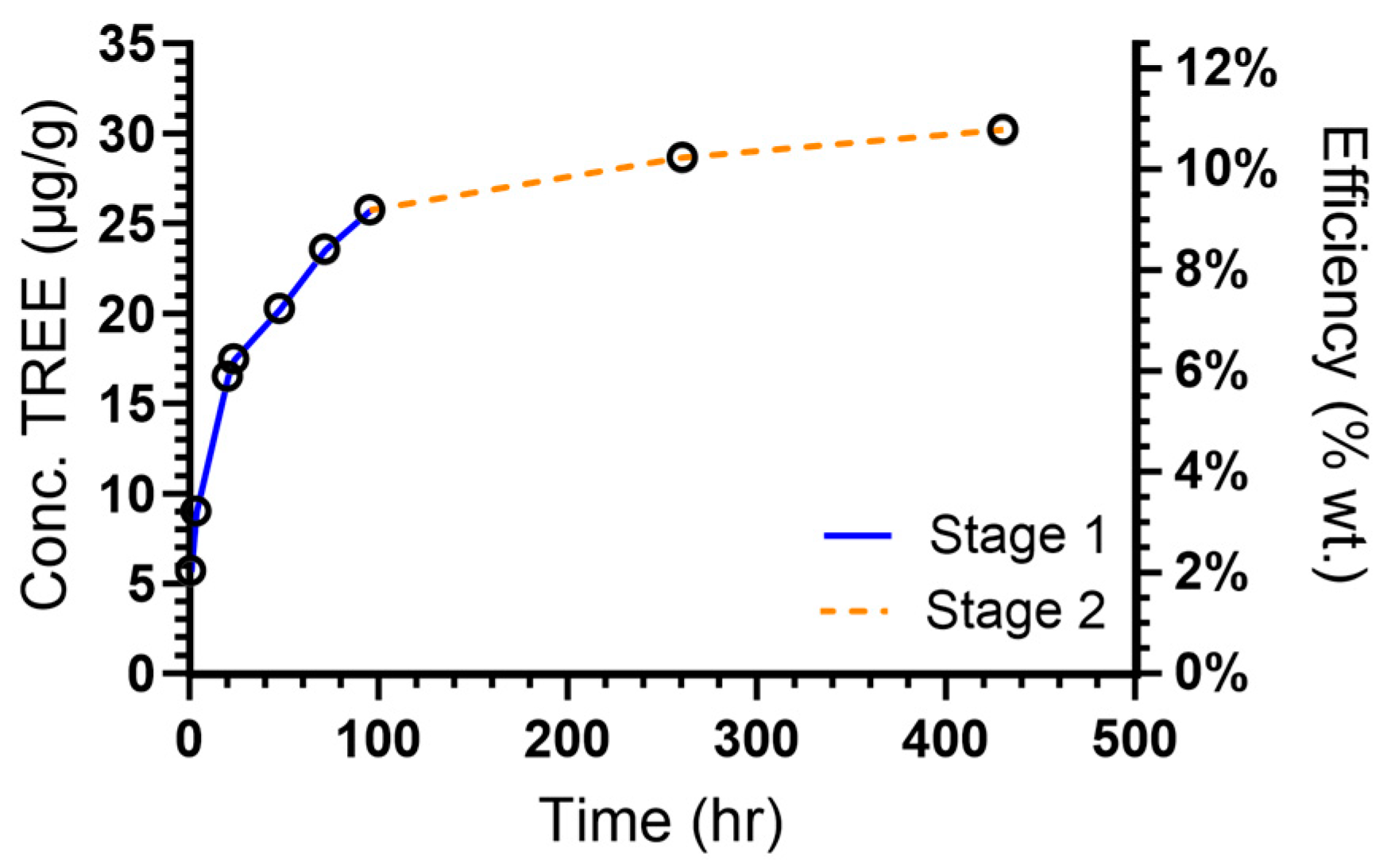
3.1. Extraction Efficiencies
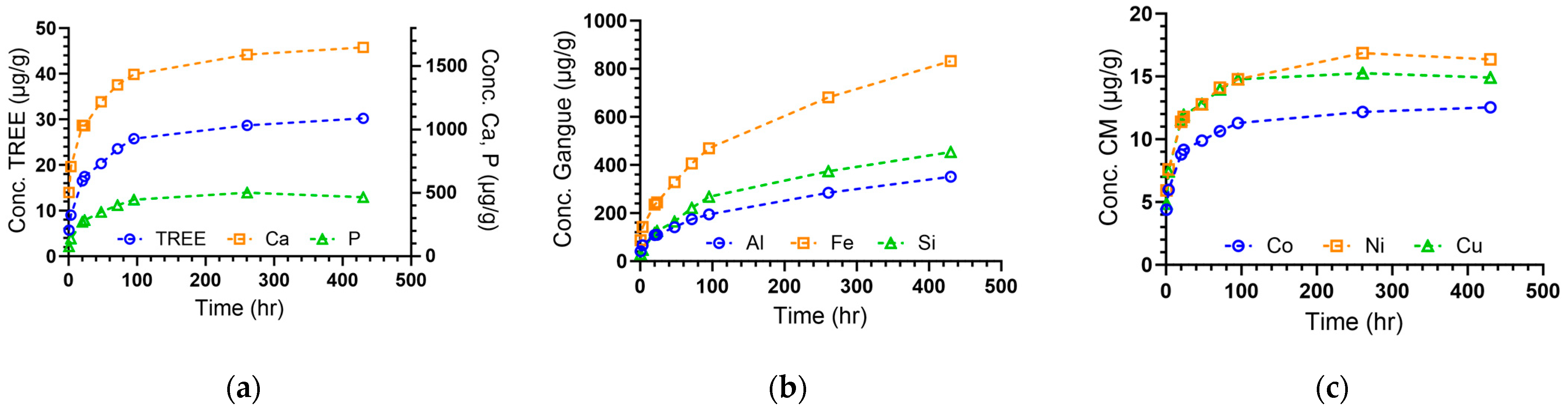
3.2. Leaching Profiles
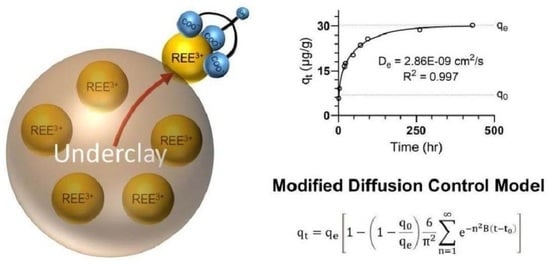
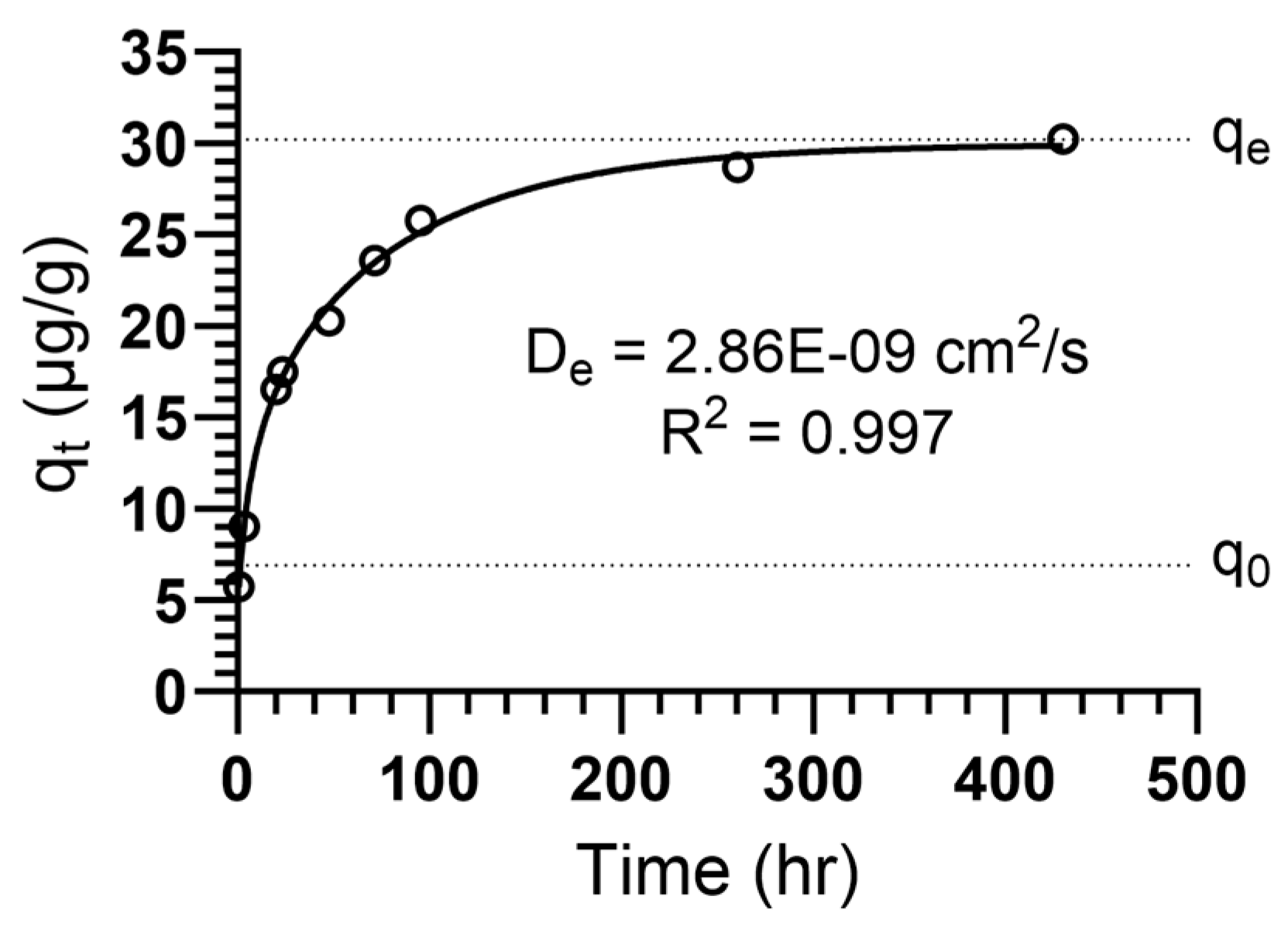
3.3. Rate Determining Mechanism
3.4. Modified Diffusion-Controlled Mechanism
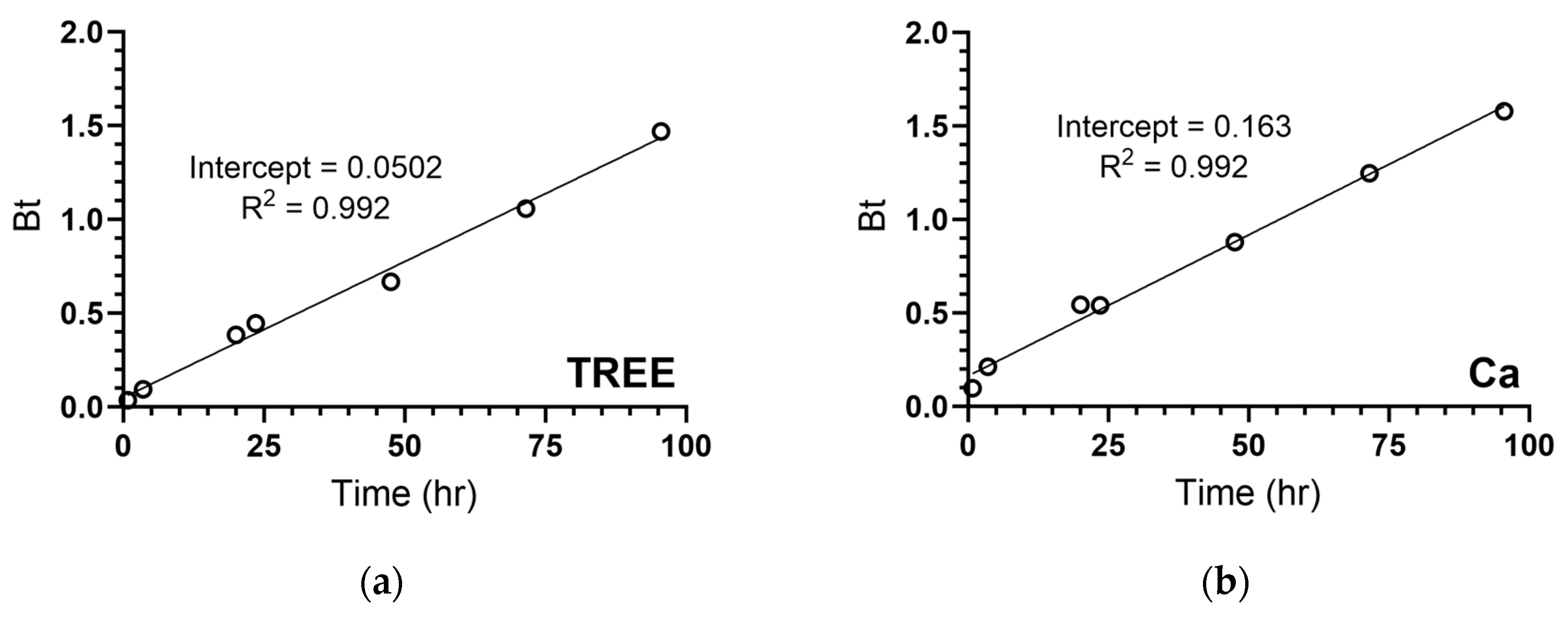
3.5. Determination of the Diffusion-Controlled Mechanism
3.6. Reaction Kinetics
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Haque, N.; Hughes, A.; Lim, S.; Vernon, C. Rare Earth Elements: Overview of Mining, Mineralogy, Uses, Sustainability and Environmental Impact. Resources 2014, 3, 614–635. [Google Scholar] [CrossRef]
- Imholte, D.D.; Nguyen, R.T.; Vedantam, A.; Brown, M.; Iyer, A.; Smith, B.J.; Collins, J.W.; Anderson, C.G.; O’Kelley, B. An assessment of U.S. rare earth availability for supporting U.S. wind energy growth targets. Energy Policy 2018, 113, 294–305. [Google Scholar] [CrossRef]
- U.S. Geological Survery (USGS). Rare Earths Mineral Commodity Summaries. 2021. Available online: https://pubs.usgs.gov/periodicals/mcs2021/mcs2021-rare-earths.pdf (accessed on 4 August 2021).
- Yang, X.J.; Lin, A.; Li, X.-L.; Wu, Y.; Zhou, W.; Chen, Z. China’s ion-adsorption rare earth resources, mining consequences and preservation. Environ. Dev. 2013, 8, 131–136. [Google Scholar] [CrossRef]
- Kanazawa, Y.; Kamitani, M. Rare earth minerals and resources in the world. J. Alloys Compd. 2006, 408–412, 1339–1343. [Google Scholar] [CrossRef]
- Papangelakis, V.G.; Moldoveanu, G. In Recovery of Rare Earth Elements from Clay Minerals, Proceedings of the 1st Rare Earth Resources Conference, Milos, Greece, 4–9 June 2014. pp. 191–202. Available online: https://www.eurare.org/docs/eres2014/fifthSession/VladimirosPapangelakis.pdf (accessed on 4 August 2021).
- Brown, M.A.; Kropf, A.J.; Paulenova, A.; Gelis, A.V. Aqueous complexation of citrate with neodymium(III) and americium(III): A study by potentiometry, absorption spectrophotometry, microcalorimetry, and XAFS. Dalton Trans. 2014, 43, 6446–6454. [Google Scholar] [CrossRef] [PubMed]
- Moldoveanu, G.; Papangelakis, V. Recovery of rare earth elements adsorbed on clay minerals: II. Leaching with ammonium sulfate. Hydrometallurgy 2013, 131–132, 158–166. [Google Scholar] [CrossRef]
- Moldoveanu, G.A.; Papangelakis, V.G. An overview of rare-earth recovery by ion-exchange leaching from ion-adsorption clays of various origins. Mineral. Mag. 2016, 80, 63–76. [Google Scholar] [CrossRef]
- Shan, X.-q.; Lian, J.; Wen, B. Effect of organic acids on adsorption and desorption of rare earth elements. Chemosphere 2002, 47, 701–710. [Google Scholar] [CrossRef]
- Evangelou, M.W.H.; Ebel, M.; Schaeffer, A. Chelate assisted phytoextraction of heavy metals from soil. Effect, mechanism, toxicity, and fate of chelating agents. Chemosphere 2007, 68, 989–1003. [Google Scholar] [CrossRef]
- Wang, L.; Liao, C.; Yang, Y.; Xu, H.; Xiao, Y.; Yan, C. Effects of organic acids on the leaching process of ion-adsorption type rare earth ore. J. Rare Earths 2017, 35, 1233–1238. [Google Scholar] [CrossRef]
- Huang, Y.; Wang, D.; Liu, H.; Fan, G.; Peng, W.; Cao, Y. Selective complexation leaching of copper from copper smelting slag with the alkaline glycine solution: An effective recovery method of copper from secondary resource. Sep. Purif. Technol. 2023, 326, 124619. [Google Scholar] [CrossRef]
- Yang, J.; Montross, S.; Britton, J.; Stuckman, M.; Lopano, C.; Verba, C. Microanalytical Approaches to Characterizing REE in Appalachian Basin Underclays. Minerals 2020, 10, 546. [Google Scholar] [CrossRef]
- Montross, S.N.; Yang, J.; Britton, J.; McKoy, M.; Verba, C. Leaching of Rare Earth Elements from Central Appalachian Coal Seam Underclays. Minerals 2020, 10, 577. [Google Scholar] [CrossRef]
- Yang, J.; Bauer, S.; Verba, C. Strategies to Recover Easily-Extractable Rare Earth Elements and Other Critical Metals from Coal Waste Streams and Adjacent Rock Strata Using Citric Acid; National Energy Technology Laboratory (NETL): Pittsburgh, PA, USA; Morgantown, WV, USA, 2022. [Google Scholar]
- Zhang, W.; Honaker, R. Characterization and recovery of rare earth elements and other critical metals (Co, Cr, Li, Mn, Sr, and V) from the calcination products of a coal refuse sample. Fuel 2020, 267, 117236. [Google Scholar] [CrossRef]
- Bauer, S.; Yang, J.; Stuckman, M.; Verba, C. Rare Earth Element (REE) and Critical Mineral Fractions of Central Appalachian Coal-Related Strata Determined by 7-Step Sequential Extraction. Minerals 2022, 12, 1350. [Google Scholar] [CrossRef]
- Ait Brahim, J.; Ait Hak, S.; Achiou, B.; Boulif, R.; Beniazza, R.; Benhida, R. Kinetics and mechanisms of leaching of rare earth elements from secondary resources. Miner. Eng. 2022, 177, 107351. [Google Scholar] [CrossRef]
- Xiao, Y.; Liu, X.; Feng, Z.; Huang, X.; Huang, L.; Chen, Y.; Wu, W. Role of minerals properties on leaching process of weathered crust elution-deposited rare earth ore. J. Rare Earths 2015, 33, 545–552. [Google Scholar] [CrossRef]
- He, Z.; Zhang, Z.; Yu, J.; Zhou, F.; Xu, Y.; Xu, Z.; Chen, Z.; Chi, R. Kinetics of column leaching of rare earth and aluminum from weathered crust elution-deposited rare earth ore with ammonium salt solutions. Hydrometallurgy 2016, 163, 33–39. [Google Scholar] [CrossRef]
- Safari, V.; Arzpeyma, G.; Rashchi, F.; Mostoufi, N. A shrinking particle—Shrinking core model for leaching of a zinc ore containing silica. Int. J. Miner. Process. 2009, 93, 79–83. [Google Scholar] [CrossRef]
- Faraji, F.; Alizadeh, A.; Rashchi, F.; Mostoufi, N. Kinetics of leaching: A review. Rev. Chem. Eng. 2020, 38, 113–148. [Google Scholar] [CrossRef]
- Jander, W. Reaktionen im festen Zustande bei höheren Temperaturen. Reaktionsgeschwindigkeiten endotherm verlaufender Umsetzungen. Z. Für Anorg. Und Allg. Chem. 1927, 163, 1–30. [Google Scholar] [CrossRef]
- Ginstling, A.; Brounshtein, B. Concerning the diffusion kinetics of reactions in spherical particles. J. Appl. Chem. USSR 1950, 23, 1327–1338. [Google Scholar]
- Pang, Y.; Li, Q. A review on kinetic models and corresponding analysis methods for hydrogen storage materials. Int. J. Hydrog. Energy 2016, 41, 18072–18087. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion, 2nd ed.; Oxford University Press: Oxford, UK, 1975. [Google Scholar]
- Perez, E.E.; Carelli, A.A.; Crapiste, G.H. Temperature-dependent diffusion coefficient of oil from different sunflower seeds during extraction with hexane. J. Food Eng. 2011, 105, 180–185. [Google Scholar] [CrossRef]
- Viegas, R.; Campinas, M.; Costa, H.; Rosa, M. How do the HSDM and Boyd’s model compare for estimating intraparticle diffusion coefficients in adsorption processes. Adsorption 2014, 20, 737–746. [Google Scholar] [CrossRef]
- Plazinski, W.; Rudzinski, W. Kinetics of Adsorption at Solid/Solution Interfaces Controlled by Intraparticle Diffusion: A Theoretical Analysis. J. Phys. Chem. C 2009, 113, 12495–12501. [Google Scholar] [CrossRef]
- Boyd, G.E.; Schubert, J.; Adamson, A.W. The Exchange Adsorption of Ions from Aqueous Solutions by Organic Zeolites. I. Ion-exchange Equilibria1. J. Am. Chem. Soc. 1947, 69, 2818–2829. [Google Scholar] [CrossRef]
- Reichenberg, D. Properties of Ion-Exchange Resins in Relation to their Structure. III. Kinetics of Exchange. J. Am. Chem. Soc. 1953, 75, 589–597. [Google Scholar] [CrossRef]
- Turner, B.D.; Henley, B.J.; Sleap, S.B.; Sloan, S.W. Kinetic model selection and the Hill model in geochemistry. Int. J. Environ. Sci. Technol. 2015, 12, 2545–2558. [Google Scholar] [CrossRef]
- Dada, A.O.; Adekola, F.A.; Odebunmi, E.O. A novel zerovalent manganese for removal of copper ions: Synthesis, characterization and adsorption studies. Appl. Water Sci. 2017, 7, 1409–1427. [Google Scholar] [CrossRef]
- Tsibranska, I.; Hristova, E. Comparison of different kinetic models for adsorption of heavy metals onto activated carbon from apricot stones. Bulg. Chem. Commun. 2011, 43, 370–377. [Google Scholar]
- Silva, A.M.N.; Kong, X.; Hider, R.C. Determination of the pKa value of the hydroxyl group in the α-hydroxycarboxylates citrate, malate and lactate by 13C NMR: Implications for metal coordination in biological systems. BioMetals 2009, 22, 771–778. [Google Scholar] [CrossRef] [PubMed]
- Shi, Q.; Zhao, Y.; Meng, X.; Shen, L.; Qiu, G.; Zhang, X.; Yu, H.; He, X.; He, H.; Zhao, H. Column leaching of ion adsorption rare earth ore at low ammonium concentration. J. Mater. Res. Technol. 2022, 19, 2135–2145. [Google Scholar] [CrossRef]
- Goyne, K.W.; Brantley, S.L.; Chorover, J. Rare earth element release from phosphate minerals in the presence of organic acids. Chem. Geol. 2010, 278, 1–14. [Google Scholar] [CrossRef]
- Bolan, N.S.; Naidu, R.; Mahimairaja, S.; Baskaran, S. Influence of low-molecular-weight organic acids on the solubilization of phosphates. Biol. Fertil. Soils 1994, 18, 311–319. [Google Scholar] [CrossRef]
- Uddin, F. Clays, nanoclays, and montmorillonite minerals. Metall. Mater. Trans. A 2008, 39, 2804–2814. [Google Scholar] [CrossRef]
- Kirby, C.S.; Rimstidt, J.D. Interaction of municipal solid waste ash with water. Environ. Sci. Technol. 1994, 28, 443–451. [Google Scholar] [CrossRef]
- Dijkstra, J.J.; van der Sloot, H.A.; Comans, R.N.J. The leaching of major and trace elements from MSWI bottom ash as a function of pH and time. Appl. Geochem. 2006, 21, 335–351. [Google Scholar] [CrossRef]
- Brady, J.; Cherniak, D. Diffusion in Minerals: An Overview of Published Experimental Diffusion Data. Rev. Mineral. Geochem. 2010, 72, 899–920. [Google Scholar] [CrossRef]
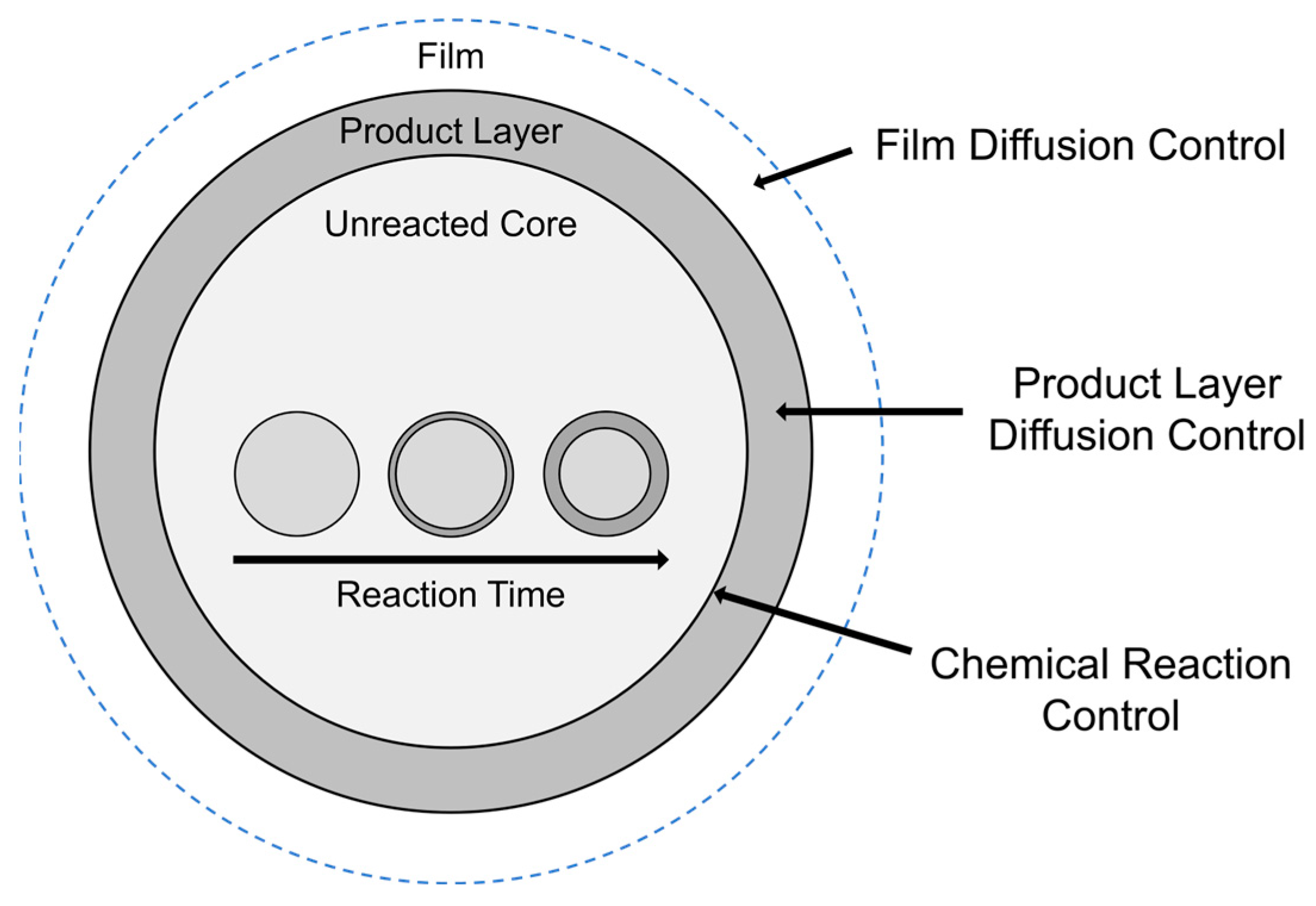


| Geometric Contraction Model | Equation |
|---|---|
| Shrinking Core Model | |
| Liquid Film Diffusion Control | |
| Product Layer Diffusion Control | |
| Chemical Reaction Control | |
| Jander Equation | |
| Ginstling–Brounshtein Model |
| REE | Wt. % | Major Cations | Wt. % |
|---|---|---|---|
| Sc | 7.15 | Mg | 4.03 |
| Y | 18.9 | K | 0.578 |
| La | 1.06 | Ca | 31.0 |
| Ce | 3.08 | Al | 0.275 |
| Pr | 5.18 | Si | 0.146 |
| Nd | 8.56 | P | 26.0 |
| Sm | 23.9 | Mn | 3.26 |
| Eu | 34.1 | Fe | 2.83 |
| Gd | 36.6 | Zn | 24.2 |
| Tb | 34.2 | ||
| Dy | 27.0 | U | 8.61 |
| Ho | 20.0 | Th | 25.0 |
| Er | 15.2 | ||
| Tm | 11.4 | Cr | 0.387 |
| Yb | 9.64 | Co | 28.5 |
| Lu | 8.71 | Ni | 15.3 |
| TREE | 10.8 | Cu | 13.9 |
| REE | qe (µg g−1) | q0 (µg g−1) | B (hr−1) | t0 (hr) | R-Squared | De (cm2 s−1) | Non-Diffusive (%) |
| Sc | 1.79 | 2.52 × 10−1 | 5.70 × 10−3 | 5.60 | 0.996 | 1.38 × 10−9 | 14.1 |
| Y | 10.5 | 2.98 | 1.20 × 10−2 | 3.86 | 0.998 | 2.91 × 10−9 | 28.2 |
| La | 4.24 × 10−1 | 0 | 1.08 × 10−2 | 0 | 0.996 | 2.61 × 10−9 | 0 |
| Ce | 2.57 | 1.55 × 10−1 | 1.12 × 10−2 | 0.965 | 0.995 | 2.72 × 10−9 | 6.01 |
| Pr | 5.24 × 10−1 | 5.76 × 10−2 | 1.12 × 10−2 | 1.96 | 0.996 | 2.72 × 10−9 | 11.0 |
| Nd | 3.48 | 4.41 × 10−1 | 1.16 × 10−2 | 2.07 | 0.996 | 2.81 × 10−9 | 12.7 |
| Sm | 2.17 | 4.57 × 10−1 | 1.14 × 10−2 | 3.38 | 0.996 | 2.78 × 10−9 | 21.0 |
| Eu | 6.79 × 10−1 | 7.96 × 10−2 | 1.47 × 10−2 | 1.14 | 0.994 | 3.58 × 10−9 | 11.7 |
| Gd | 4.42 | 9.84 × 10−1 | 1.23 × 10−2 | 3.12 | 0.997 | 2.98 × 10−9 | 22.3 |
| Tb | 6.48 × 10−1 | 1.79 × 10−1 | 1.10 × 10−2 | 4.49 | 0.997 | 2.67 × 10−9 | 27.6 |
| Dy | 2.78 | 7.74 × 10−1 | 1.14 × 10−2 | 4.20 | 0.997 | 2.77 × 10−9 | 27.8 |
| Ho | 3.96 × 10−1 | 9.12 × 10−2 | 1.35 × 10−2 | 2.65 | 0.997 | 3.29 × 10−9 | 23.0 |
| Er | 8.00 × 10−1 | 2.47 × 10−1 | 1.16 × 10−2 | 4.52 | 0.997 | 2.81 × 10−9 | 30.8 |
| Tm | 8.10 × 10−2 | 2.42 × 10−2 | 9.98 × 10−2 | 4.95 | 0.998 | 2.42 × 10−9 | 29.9 |
| Yb | 4.01 × 10−1 | 9.58 × 10−2 | 1.13 × 10−2 | 3.09 | 0.997 | 2.74 × 10−9 | 23.9 |
| Lu | 5.19 × 10−2 | 1.48 × 10−2 | 1.10 × 10−2 | 4.23 | 0.996 | 2.68 × 10−9 | 28.4 |
| TREE | 30.0 | 6.65 | 1.18 × 10−2 | 3.23 | 0.997 | 2.86 × 10−9 | 22.2 |
| Major Cations | qe (µg g−1) | q0 (µg g−1) | B (hr−1) | t0 (hr) | R-Squared | De (cm2 s−1) | Non-Diffusive (%) |
| Mg | 227 | 105 | 3.97 × 10−3 | 15.4 | 0.985 | 9.64 × 10−10 | 46.2 |
| K | 143 | 80.0 | 1.09 × 10−2 | 5.78 | 0.974 | 2.64 × 10−9 | 56.0 |
| Ca | 1640 | 591 | 1.16 × 10−2 | 3.69 | 0.998 | 2.81 × 10−9 | 36.0 |
| Al | 483 | 17.6 | 1.76 × 10−3 | 3.95 | 0.996 | 4.28 × 10−10 | 3.63 |
| Si | 716 | 104 | 1.06 × 10−3 | 38.3 | 0.997 | 2.57 × 10−10 | 14.5 |
| P | 486 | 22.4 | 1.78 × 10−2 | 0.302 | 0.994 | 4.32 × 10−9 | 4.61 |
| Mn | 5.74 | 2.08 | 3.60 × 10−3 | 14.0 | 0.992 | 8.74 × 10−10 | 36.2 |
| Fe | 1170 | 39.1 | 1.72 × 10−3 | 6.11 | 0.998 | 4.17 × 10−10 | 3.34 |
| Zn | 31.3 | 6.79 | 6.21 × 10−3 | 5.50 | 0.995 | 1.51 × 10−9 | 21.7 |
| U | 5.15 × 10−1 | 1.05 × 10−1 | 1.07 × 10−2 | 2.22 | 0.998 | 2.61 × 10−9 | 20.4 |
| Th | 4.25 | 3.41 × 10−1 | 1.20 × 10−2 | 1.08 | 0.999 | 2.92 × 10−9 | 8.01 |
| Ti | 3.79 | 0 | 9.73 × 10−4 | 7.86 | 0.994 | 2.36 × 10−10 | 0 |
| Cr | 1.64 | 0 | 3.31 × 10−4 | 57.4 | 0.994 | 8.04 × 10−11 | 0 |
| Co | 12.6 | 6.90 | 9.84 × 10−3 | 7.92 | 0.998 | 2.39 × 10−9 | 54.8 |
| Ni | 16.7 | 7.62 | 1.16 × 10−2 | 5.05 | 0.995 | 2.81 × 10−9 | 45.5 |
| Cu | 15.1 | 6.37 | 2.36 × 10−2 | 2.81 | 0.995 | 5.73 × 10−9 | 42.1 |
| REE | Y-Int | R-Squared | Major Cations | Y-Int | R-Squared |
|---|---|---|---|---|---|
| Sc | 6.58 × 10−3 | 0.984 | Mg | 1.74 × 10−1 | 0.938 |
| Y | 8.77 × 10−2 | 0.992 | K | 3.41 × 10−1 | 0.927 |
| La | −2.84 × 10−2 | 0.981 | Ca | 1.63 × 10−1 | 0.992 |
| Ce | −1.04 × 10−2 | 0.985 | Al | 8.80 × 10−3 | 0.996 |
| Pr | 9.72 × 10−4 | 0.987 | Si | −5.65 × 10−3 | 0.975 |
| Nd | 5.15 × 10−3 | 0.985 | P | −2.45 × 10−2 | 0.982 |
| Sm | 3.50 × 10−2 | 0.990 | Mn | 1.01 × 10−1 | 0.968 |
| Eu | 1.86 × 10−2 | 0.981 | Fe | 2.56 × 10−3 | 0.995 |
| Gd | 4.97 × 10−2 | 0.992 | Zn | 4.00 × 10−2 | 0.988 |
| Tb | 6.45 × 10−2 | 0.987 | |||
| Dy | 7.31 × 10−2 | 0.992 | U | 6.14 × 10−2 | 0.991 |
| Ho | 5.99 × 10−2 | 0.992 | Th | 1.16 × 10−2 | 0.995 |
| Er | 8.37 × 10−2 | 0.986 | Ti | 4.23 × 10−4 | 0.966 |
| Tm | 9.01 × 10−2 | 0.987 | Cr | −1.52 × 10−3 | 0.955 |
| Yb | 6.54 × 10−2 | 0.994 | Co | 2.77 × 10−1 | 0.961 |
| Lu | 8.47 × 10−2 | 0.982 | Ni | 2.51 × 10−1 | 0.968 |
| TREE | 5.02 × 10−2 | 0.992 | Cu | 1.81 × 10−1 | 0.967 |
| REE | k (µg g−1 h−n) | n | R-Squared | Major Cations | k (µg g−1 h−n) | n | R-Squared |
|---|---|---|---|---|---|---|---|
| Sc | 2.13 × 10−1 | 0.385 | 0.990 | Al | 39.3 | 0.345 | 0.989 |
| Y | 2.58 | 0.280 | 0.996 | Si | 23.5 | 0.527 | 0.991 |
| La | 5.38 × 10−2 | 0.405 | 0.997 | P | 93.2 | 0.345 | 0.997 |
| Ce | 3.88 × 10−1 | 0.372 | 0.996 | Fe | 75.5 | 0.393 | 0.986 |
| Pr | 8.54 × 10−2 | 0.357 | 0.997 | Zn | 5.62 | 0.305 | 0.993 |
| Nd | 6.10 × 10−1 | 0.347 | 0.996 | ||||
| Sm | 4.35 × 10−1 | 0.319 | 0.996 | U | 1.18 × 10−1 | 0.282 | 0.997 |
| Eu | 1.37 × 10−1 | 0.326 | 0.991 | Th | 7.15 × 10−1 | 0.352 | 0.998 |
| Gd | 9.61 × 10−1 | 0.304 | 0.996 | ||||
| Tb | 1.43 × 10−1 | 0.299 | 0.993 | Ti | 1.62 × 10−1 | 0.413 | 0.970 |
| Dy | 6.43 × 10−1 | 0.290 | 0.995 | Cr | 7.18 × 10−3 | 0.762 | 0.980 |
| Ho | 9.44 × 10−2 | 0.289 | 0.997 | ||||
| Er | 1.96 × 10−1 | 0.279 | 0.993 | ||||
| Tm | 1.92 × 10−2 | 0.278 | 0.995 | ||||
| Yb | 9.34 × 10−2 | 0.284 | 0.998 | ||||
| Lu | 1.24 × 10−2 | 0.282 | 0.992 | ||||
| TREE | 6.43 | 0.305 | 0.996 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prem, P.; Burgess, W.; Yang, J.; Verba, C. Extraction Kinetics of Rare Earth Elements from Ion-Adsorbed Underclays. Minerals 2023, 13, 1503. https://doi.org/10.3390/min13121503
Prem P, Burgess W, Yang J, Verba C. Extraction Kinetics of Rare Earth Elements from Ion-Adsorbed Underclays. Minerals. 2023; 13(12):1503. https://doi.org/10.3390/min13121503
Chicago/Turabian StylePrem, Priscilla, Ward Burgess, Jon Yang, and Circe Verba. 2023. "Extraction Kinetics of Rare Earth Elements from Ion-Adsorbed Underclays" Minerals 13, no. 12: 1503. https://doi.org/10.3390/min13121503
APA StylePrem, P., Burgess, W., Yang, J., & Verba, C. (2023). Extraction Kinetics of Rare Earth Elements from Ion-Adsorbed Underclays. Minerals, 13(12), 1503. https://doi.org/10.3390/min13121503