Study on Fluid–Solid Characteristics of Grouting Filling Similar-Simulation Materials
Abstract
:1. Introduction
2. Experimental Program
2.1. Deduction of the Similarity of Fluid-Solid Materials
2.2. Determination of Preparation Parameters
2.3. Physical and Mechanical Tests
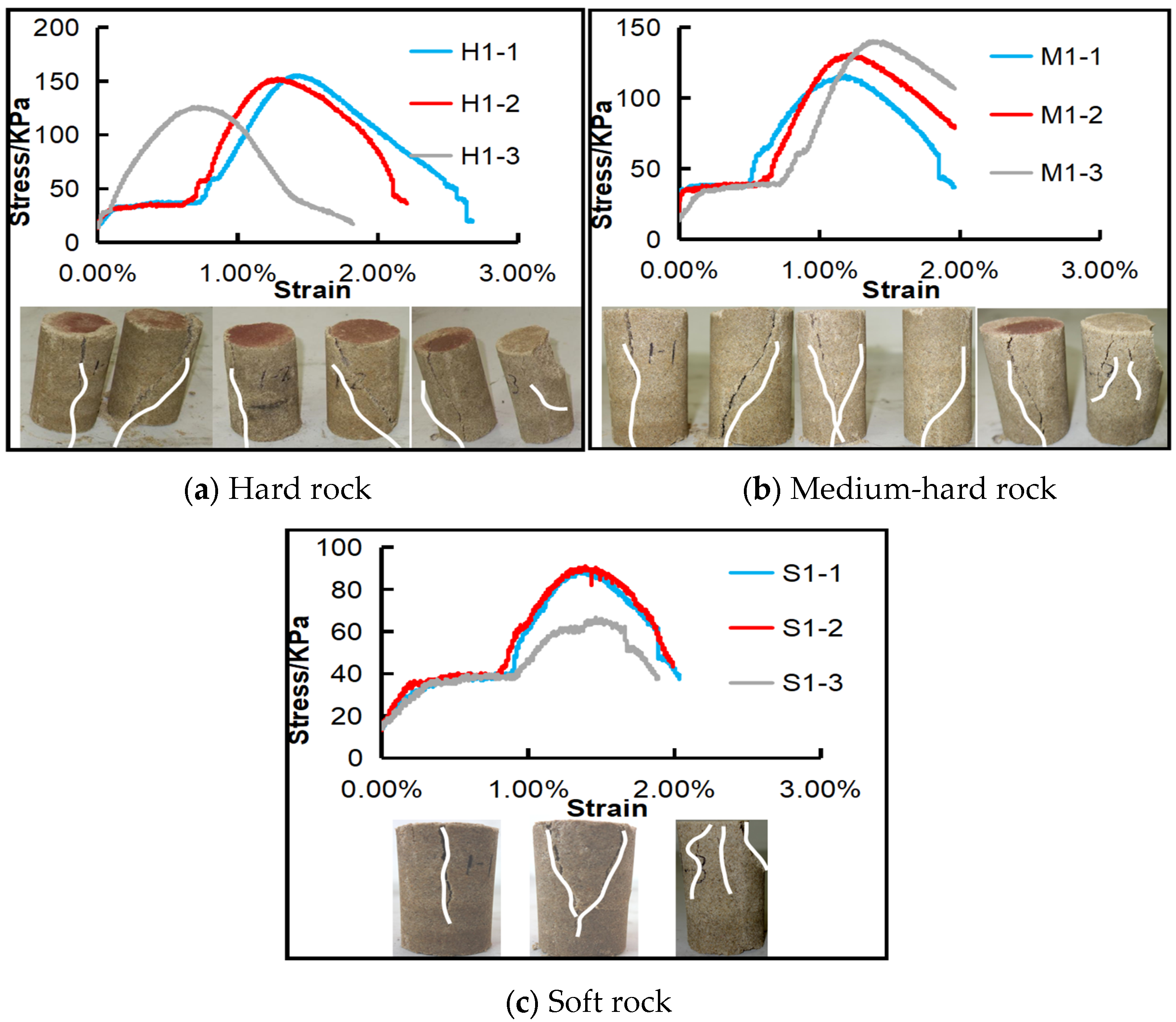
- For the hard rock group (H group), the compressive strength of rock specimens prepared in different batches is different; the maximum compressive strength is 154 KPa, the minimum compressive strength is 129 KPa, and the average compressive strength is 144 KPa, with a deviation of 10.2. The strength deviation of rock specimens in the H1-3 group is relatively large.
- For the medium-hard rock group (M group), the maximum compressive strength of rock specimens is 137 KPa, the minimum compressive strength is 115 KPa, the average compressive strength is 126 KPa, the deviation is 6.6, and the overall data are credible.
- For the soft rock group (S group), the maximum compressive strength of rock specimens is 89 KPa, the minimum compressive strength is 64 KPa, and the average compressive strength is 80 KPa, with a deviation of 11.1. The deviation of rock specimens in the S1-3 group is relatively large.
3. Hydrological Experiments
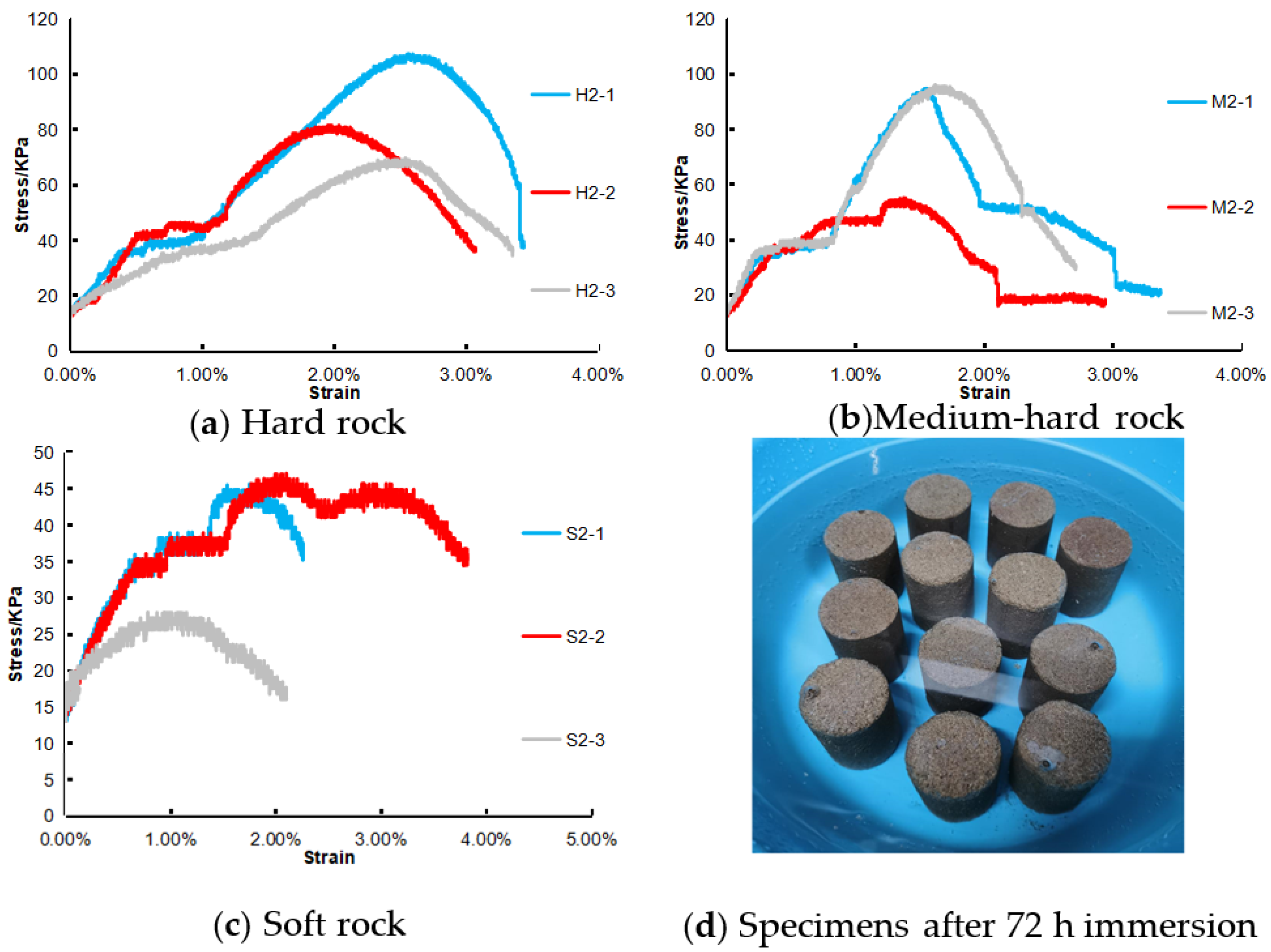
3.1. Water Immersion Experiment
3.2. Water Storage Experiment
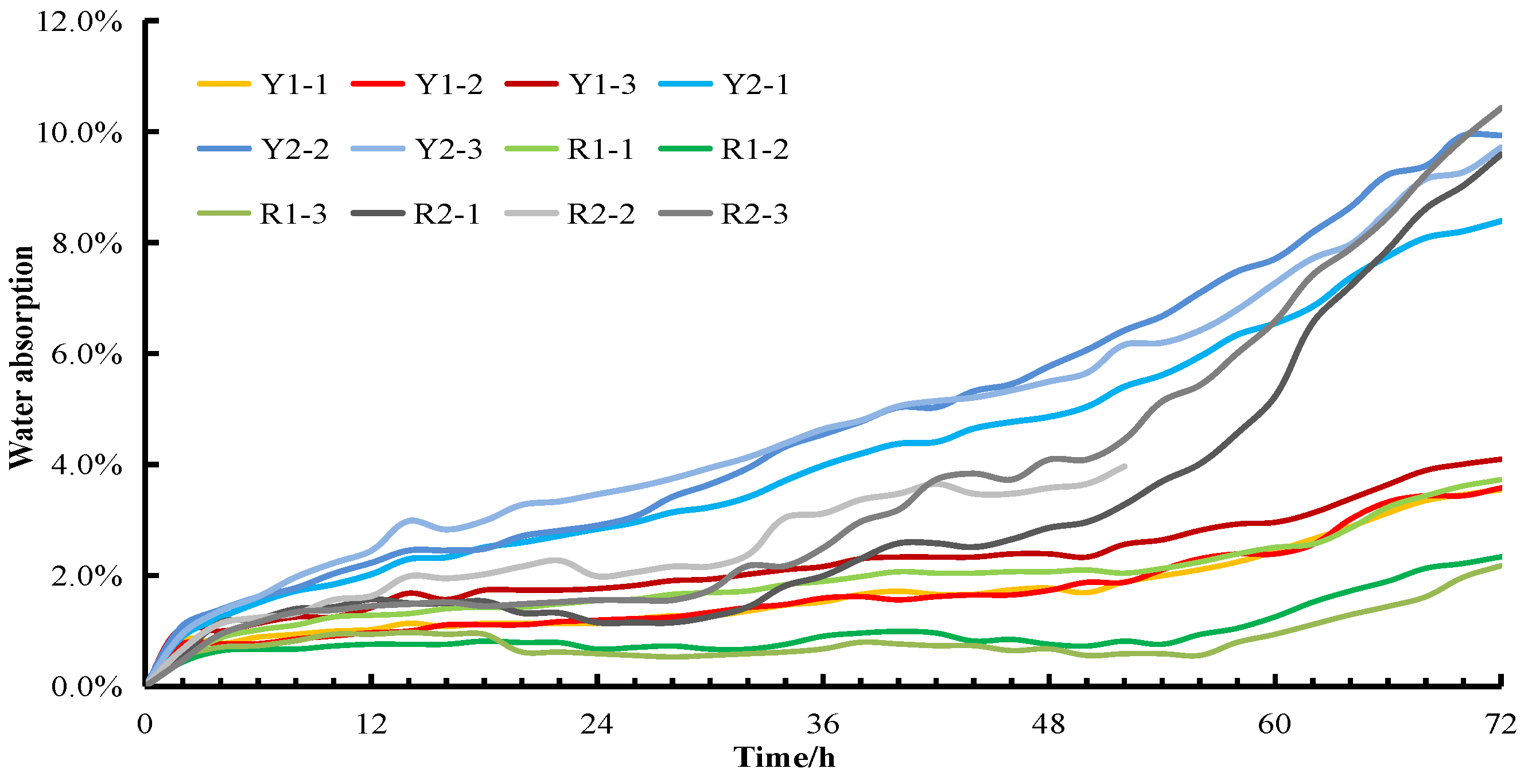
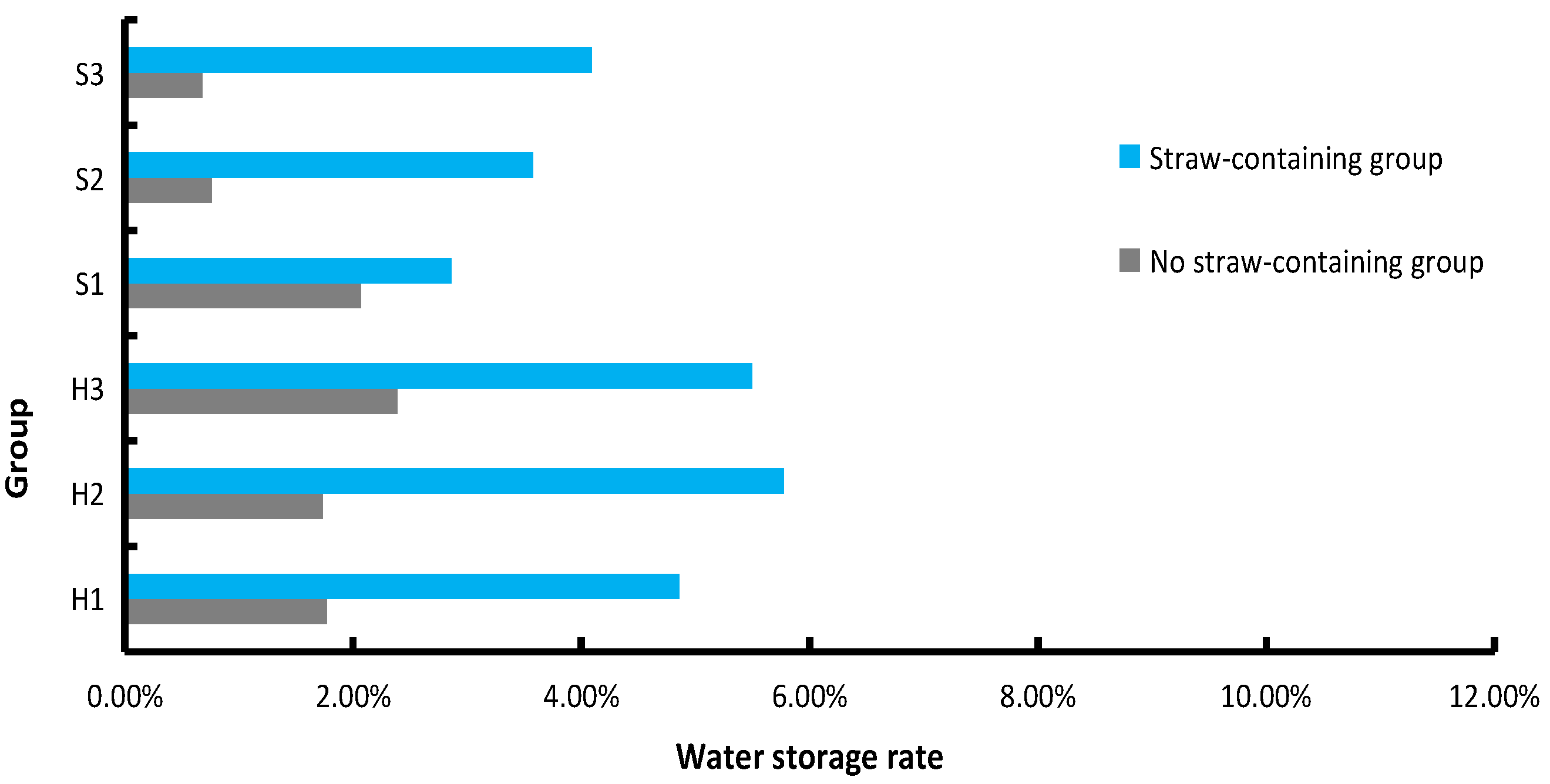
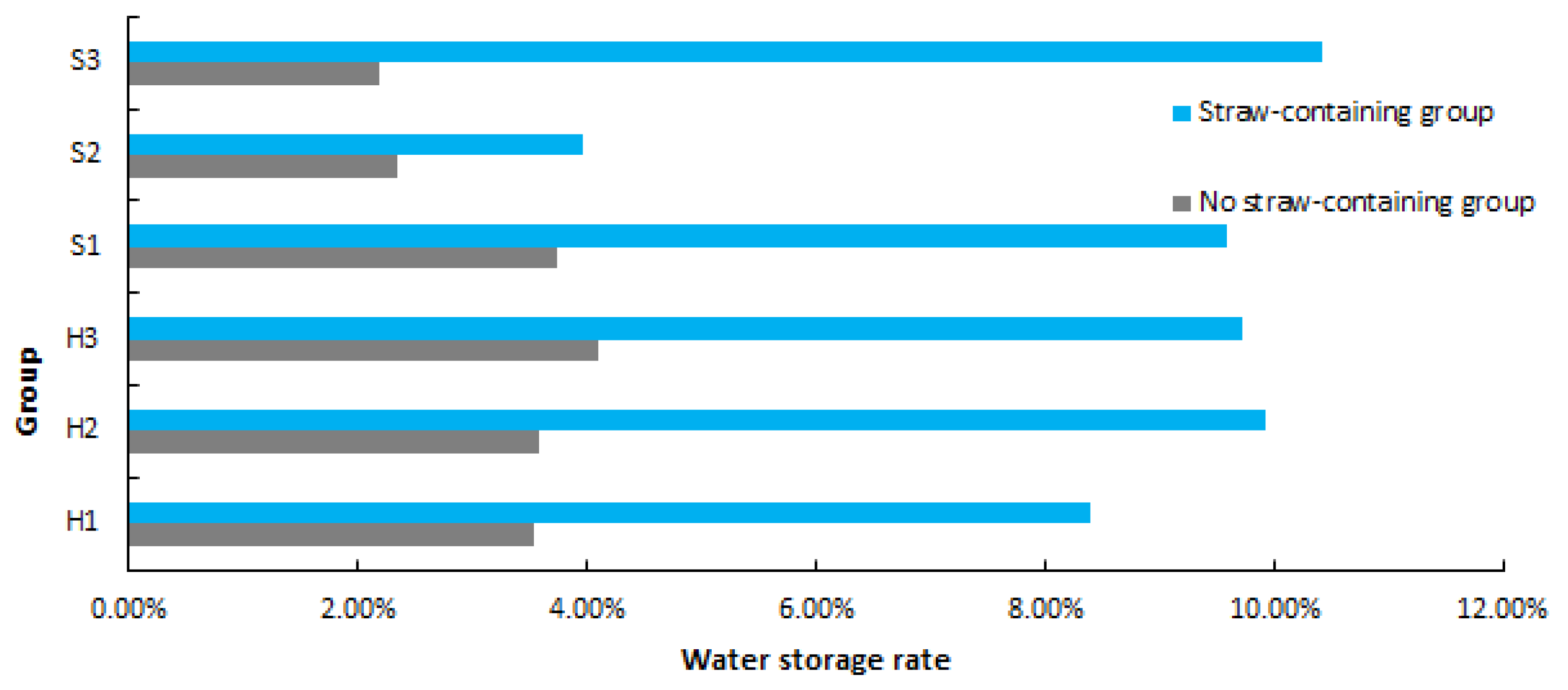
- There is a linear relationship between the time and the water absorption rate of rock specimens in different groups, and the saturation no longer increases after 72 h. The average saturated water storage rate of rock specimens with straw powder is 9.6% and that of rock specimens without straw powder is 3%. Therefore, the straw powder can improve the water storage rate of rock specimens by more than 300%.
- The average water storage rate of rock specimens with straw powder is 2% within 24 h and that of rock specimens without straw powder is less than 1%. After 48 h, the average water storage rate of rock specimens with straw powder is more than 4.5%, while that of rock specimens without straw powder is less than 2%. It can be seen that the water absorption rate of rock specimens with straw powder is relatively slow at the first 24 h and then the water absorption rate significantly increases. This is because the oil film inside the material hinders the formation of the water absorption pathway. However, with the increased time, the oil film continues to seep out and form a pore channel inside the sample. Therefore, the water absorption rate slowly increases first and then increases rapidly.
3.3. Permeation Experiments
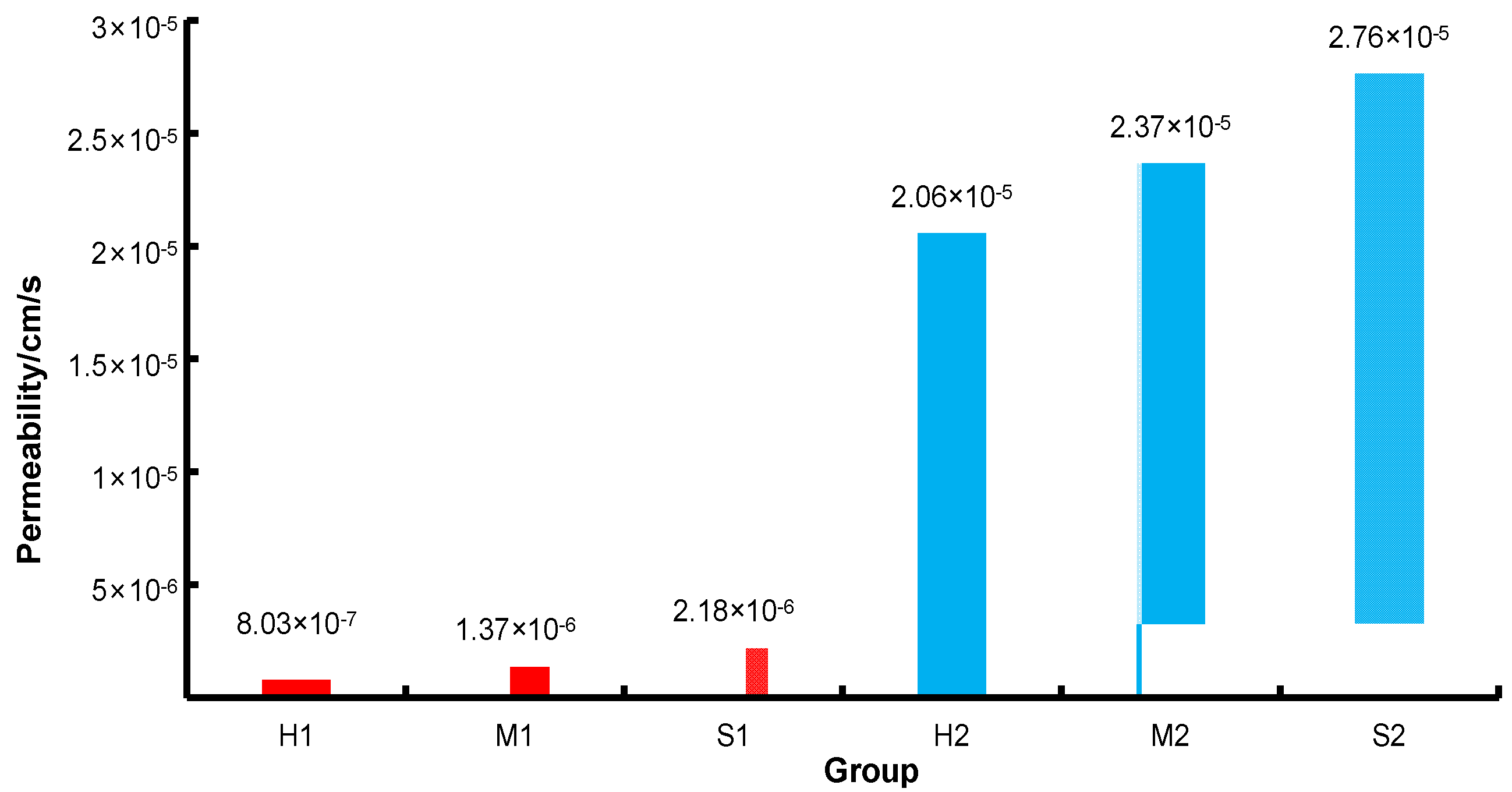
- The permeability difference of rock groups with different lithologies is small and the greater the hardness of rock specimens, the worse the permeability.
- The average permeability of the rock group without PVA is 1.45 × 10−6 cm/s, while the average permeability of the rock group with PVA can reach 2.36 × 10−5 cm/s. Therefore, PVA material significantly improves the permeability of rock materials and the permeability improvement rate reaches 1627%.
4. Application
5. Conclusions
- (1)
- According to the above experimental results, the similarity coefficients of the time similarity ratio, geometric similarity ratio, and permeability similarity ratio are obtained as follows: Π1 = Π2 = gt2/l = 0.025 and Π3 = k/l2 = 1,600,000. Additionally, Π1 and Π2 are defined as the time similarity coefficients of the bed separation grouting simulation and Π3 as the permeability similarity coefficient of the bed separation grouting simulation.These coefficients provide a reference for research and development of similar-simulation materials for the bed separation grouting simulation. According to the experimental needs, these coefficients can be used to adjust the geometric similarity ratio, time similarity ratio, and permeability similarity ratio.
- (2)
- A similar-simulation material with the adjustable strength of 80 KPa–144 KPa was obtained under limited experimental conditions. The experimental results verify that this material does not disintegrate in water and its water storage rate can be adjusted between 3% and 9.6%, and the permeability can reach 2.36 × 10−5 cm/s.
- (3)
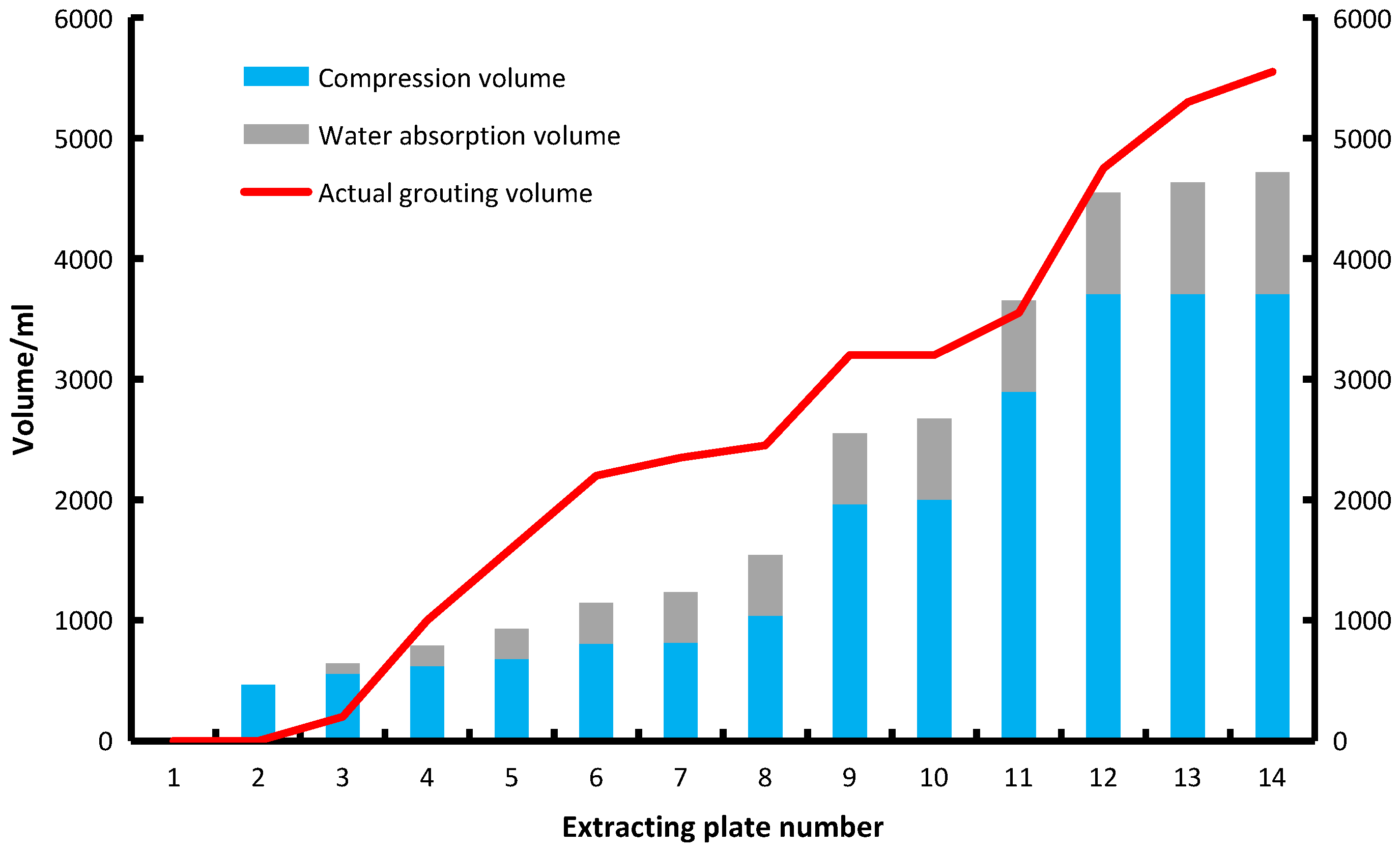
- The similar-simulation experiment of mining-induced bed separation grouting was carried out in a three-dimensional physical experiment system at a similarity ratio of 1:400. The results show that the permeability of the similar-simulation material is strengthened by the generated cracks via the rock strata migration undermining and the volume of the bed separation is smaller than that of the grouting in the grouting process. This indicates that the material can successfully simulate the water loss and consolidation process of slurry and be used for grouting simulation experiments.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Miao, X.X.; Qian, M.G. Research Status and Prospect of Green Exploitation of Coal Resources in China. J. Min. Saf. Eng. 2009, 26, 1–14. [Google Scholar] [CrossRef]
- Qian, M.G.; Xu, J.L.; Wang, J.C. Further on the sustainable mining of coal. J. China Coal Soc. 2018, 43, 1–13. [Google Scholar] [CrossRef]
- Li, J.M.; Huang, Y.L.; Pu, H.; Gao, H.D.; Li, Y.; Ouyang, S.S.; Guo, Y.C. Influence of block shape on macroscopic deformation response and meso-fabric evolution of crushed gangue under the triaxial compression. Powder Technol. 2021, 384, 112–124. [Google Scholar] [CrossRef]
- Syd, S.P. Topical areas of research needs in ground control—A state of the art review on coal mine ground control. Int. J. Min. Sci. Technol. 2015, 25, 1–6. [Google Scholar] [CrossRef]
- Zhou, D.; Wu, K.; Bai, Z.; Hu, Z.; Li, L.; Xu, Y.; Diao, X. Formation and development mechanism of ground crack caused by coal mining: Effects of overlying key strata. B Eng. Geol. Env. 2019, 78, 1025–1044. [Google Scholar] [CrossRef]
- Liu, Z.X.; Cui, B.Q.; Liang, Y.B.; Guo, H.; Li, Y.Y. Study on Foundation Deformation of Buildings in Mining Subsidence Area and Surface Subsidence Prediction. Geotech. Geol. Eng. 2019, 37, 1755–1764. [Google Scholar] [CrossRef]
- Xu, J.L. Research and Progress of Green Mining in Coal Mine in 20 Years. Coal Sci. Technol. 2020, 48, 1–15. [Google Scholar] [CrossRef]
- Xu, J.L.; Xuan, D.; Zhu, W.B.; Wang, X.Z.; Teng, H. Research and Practice of Partial Filling Coal Mining Technology. Coal Sci. Technol. 2015, 40, 1303–1312. [Google Scholar] [CrossRef]
- Xu, J.L.; Xuan, D.; Zhu, W.B.; Wang, X.Z. Partial Backfilling Coal Mining Technology Based on Key Strata Control. J. Min. Strat. Control Eng. 2019, 1, 69–76. [Google Scholar]
- Zhang, L.; Xu, J.L.; Xuan, D.; Gan, M.G. Experiment and application of compression characteristics of overburden isolation grouting filling slurry. J. China Coal Soc. 2017, 42, 1117–1122. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, J.L.; Xuan, D. Experimental study on drainage characteristics of isolated grouting filling slurry. China Coal 2017, 43, 121–124. [Google Scholar] [CrossRef]
- Xuan, D.; Wang, B.; Xu, J.L. A shared borehole approach for coal-bed methane drainage and ground stabilization with grouting. Int. J. Rock Mech. Min. 2016, 86, 235–244. [Google Scholar] [CrossRef]
- Sun, W.B.; Zhou, F.; Shao, J.L.; Du, H.Q.; Xue, Y.C. Development Status and Prospects of Mine Physical Similar Material Simulation Experiments. Geotech. Geol. Eng. 2019, 37, 3025–3036. [Google Scholar] [CrossRef]
- Qing-biao, W.; Zhu, Q.K.; Shao, T.S.; Yu, X.G.; Xu, S.Y.; Zhang, J.J.; Kong, Q.L. The rheological test and application research of glass fiber cement slurry based on plugging mechanism of dynamic water grouting. Constr. Build. Mater. 2018, 189, 119–130. [Google Scholar] [CrossRef]
- Wu, J.; Chen, B.; Chai, S.J.; Kong, W.L. Experimental Study on Compression Characteristics of Gangue Grouting Filling Materials. Geotech. Geol. Eng. 2020, 38, 4557–4565. [Google Scholar] [CrossRef]
- Huang, Q.X.; Zhang, W.Z.; Hou, Z.C. Study on Similar Materials of Waterproof Layer in Solid-liquid Coupling Test. Chin. J. Rock Mech. Eng. 2010, 29, 2813–2818. [Google Scholar]
- Huang, Q.X. Study on water resisting property of subsurface aquiclude in shallow coal seam mining. J. Coal Sci. Eng. 2008, 14, 369–372. [Google Scholar] [CrossRef]
- Zhang, J.; Hou, Z.J. Experimental study on simulation materials for solid-liquid coupling. Chin. J. Rock Mech. Eng. 2004, 23, 3157–3161. [Google Scholar] [CrossRef]
- Sun, W.B.; Zhang, S.C.; LI, Y.Y.; Lu, C. Development application of solid-fluld coupling similar material for floor strata and simulation test of waterinrush in deep mining. Chin. J. Rock Mech. Eng. 2015, 34, 2665–2670. [Google Scholar] [CrossRef]
- Lian, H.Q.; Xia, X.; Ran, W.; Zhao, Q.F. Experimental study on water resistance of new fluid-solid coupling similar simulation materials. Coal Min. Technol. 2015, 20, 12–16. [Google Scholar] [CrossRef]
- Zhang, S.C.; Guo, W.J.; Li, Y.Y.; Sun, W.B.; Yin, D.W. Experimental Simulation of Fault Water Inrush Channel Evolution in a Coal Mine Floor. Mine Water Env. 2017, 36, 443–451. [Google Scholar] [CrossRef]
- Tang, L.; Guo, P. Research and Application of 3D Printing Water Soluble Polyvinyl Alcohol Material. New Chem. Mater. 2019, 47, 221–223. [Google Scholar]
- Lin, F.; Zhao, J.L.; Wu, Q.P. Study on waste-metakaolin composite permeable material. J. Sci. Teach. Coll. Univ. 2017, 37, 56–58. [Google Scholar] [CrossRef]
- Zhao, H.K.; Zhao, P.P.; Bing, S.P.; Chen, J.; Zhang, K.H. Preparation and Analysis of Hydroxypropyl Methyl Cellulose Doped Straw Absorbent Materials. New Chem. Mater. 2020, 48, 249–253. [Google Scholar] [CrossRef]
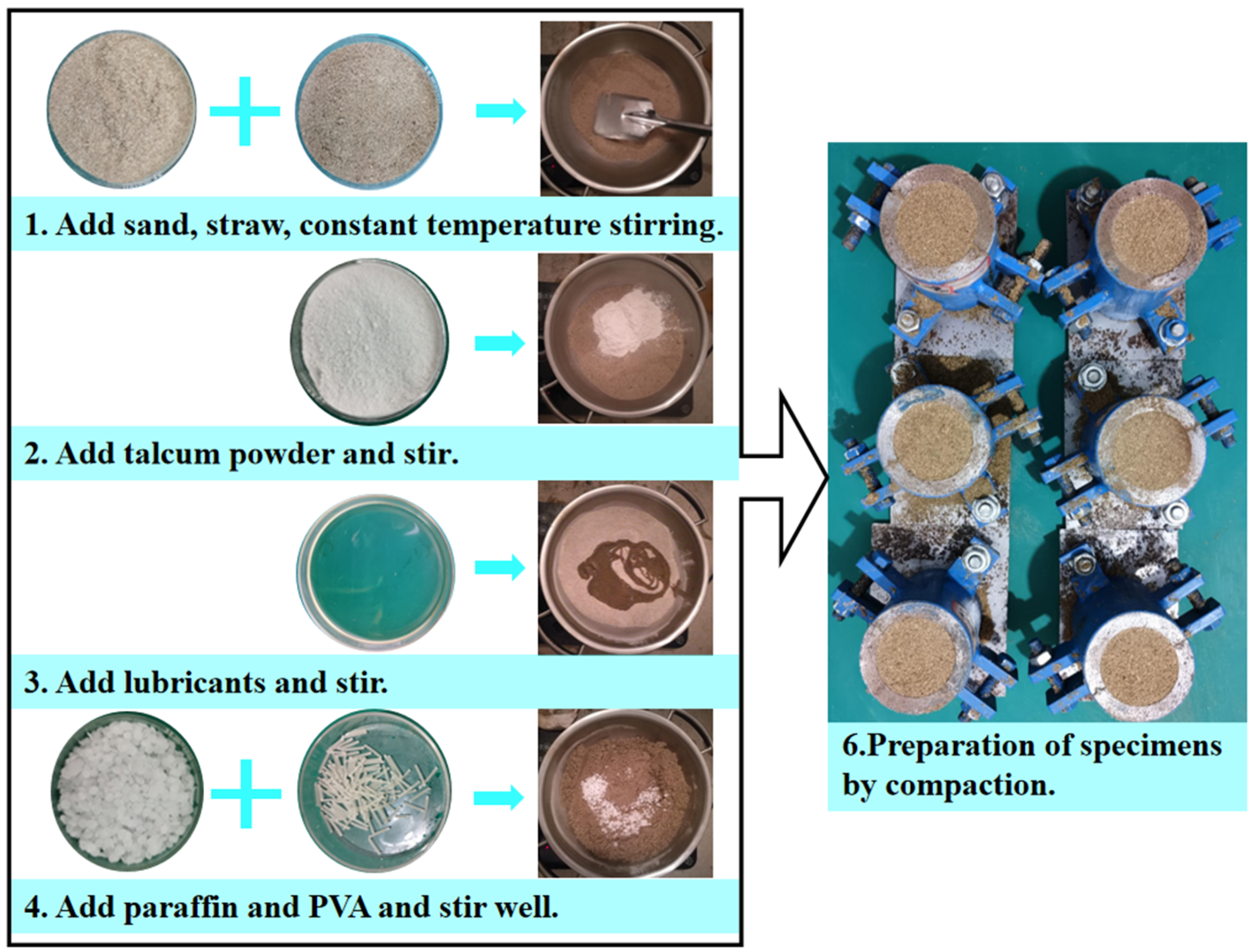



| Simulated Lithology | Paraffin | Lubricating Oil | Talc Powder | Sand | Straw Powder | PVA |
|---|---|---|---|---|---|---|
| Hard rock | 4.5 | 4.5 | 9 | 80 | 2 | 2 |
| Medium-hard rock | 3 | 4.5 | 10.5 | 80 | 2 | 2 |
| Soft rock | 2 | 4.5 | 11.5 | 80 | 2 | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, K.; Xuan, D.; Li, J. Study on Fluid–Solid Characteristics of Grouting Filling Similar-Simulation Materials. Minerals 2022, 12, 502. https://doi.org/10.3390/min12050502
Zheng K, Xuan D, Li J. Study on Fluid–Solid Characteristics of Grouting Filling Similar-Simulation Materials. Minerals. 2022; 12(5):502. https://doi.org/10.3390/min12050502
Chicago/Turabian StyleZheng, Kaidan, Dayang Xuan, and Jian Li. 2022. "Study on Fluid–Solid Characteristics of Grouting Filling Similar-Simulation Materials" Minerals 12, no. 5: 502. https://doi.org/10.3390/min12050502
APA StyleZheng, K., Xuan, D., & Li, J. (2022). Study on Fluid–Solid Characteristics of Grouting Filling Similar-Simulation Materials. Minerals, 12(5), 502. https://doi.org/10.3390/min12050502





