Two Quantum Triatomic Hamiltonians: Applications to Non-Adiabatic Effects in NO₂ Spectroscopy and in Kr + OH(A²Σ⁺) Electronic Quenching

Abstract

This review discusses two triatomic Hamiltonians and their applications to some non-adiabatic spectroscopic and collision problems. Carter and Handy in 1984 presented the first Hamiltonian in bond lengths–bond angle coordinates, that is here applied for studying the NO₂ spectroscopy: vibronic states, internal dynamics, and interaction with the radiation due to the $\tilde{\mathrm{X}}$²A′(A₁)−$\tilde{\mathrm{A}}$²A′(B₂) conical intersection. The second Hamiltonian was reported by Tennyson and Sutcliffe in 1983 in Jacobi coordinates and is here employed in the study of the Kr + OH(A²Σ⁺) electronic quenching due to conical intersection and Renner–Teller interactions among the 1²A′, 2²A′, and 1²A″ electronic species. Within the non-relativistic approximation and the expansion method in diabatic electronic representations, the formalism is exact and allows a unified study of various non-adiabatic interactions between electronic states. The rotation, inversion, and nuclear permutation symmetries are considered for defining rovibronic representations, which are symmetry adapted for ABC and AB₂ molecules, and the matrix elements of the Hamiltonians are then computed.

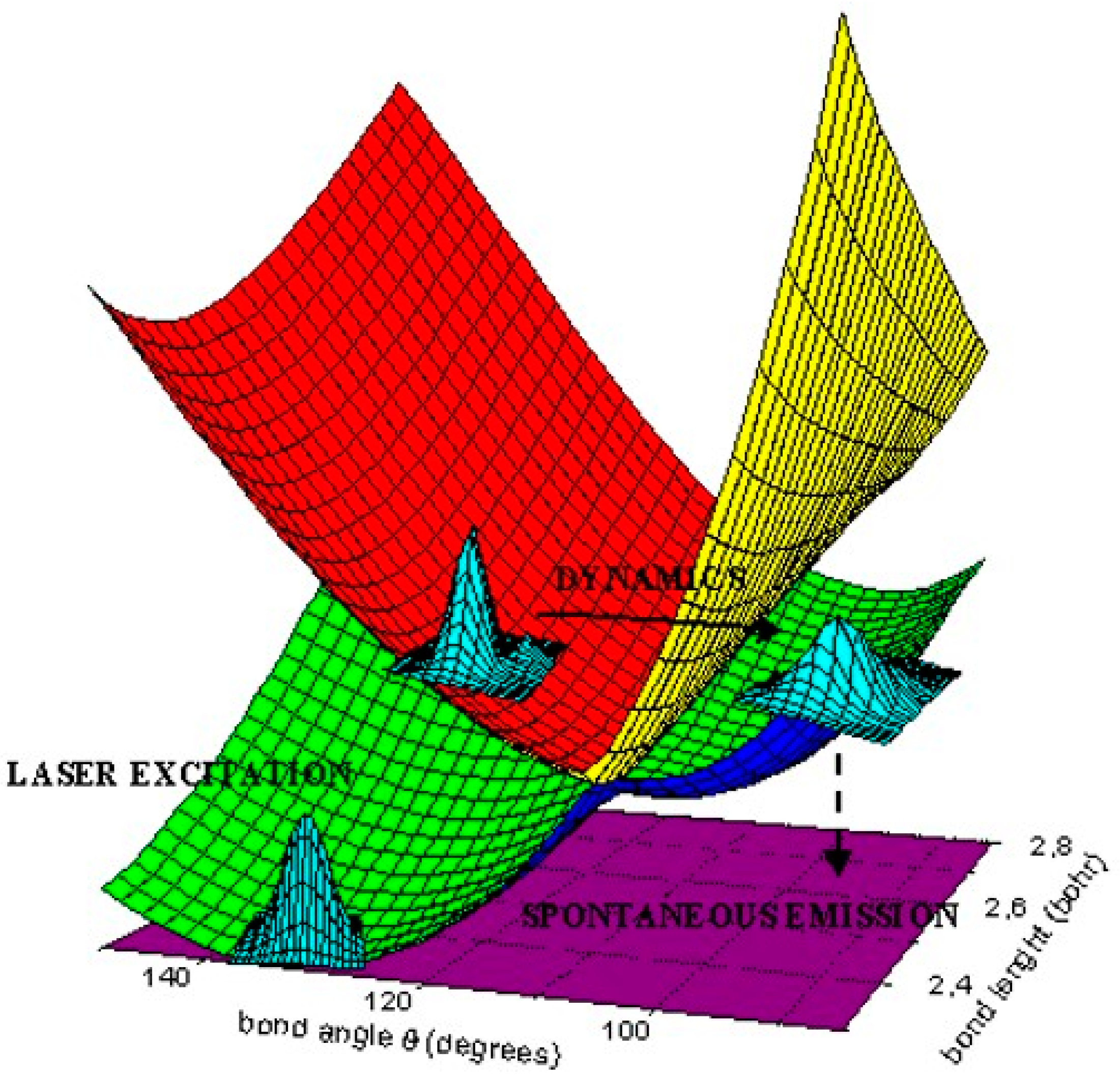
1. Introduction

Let us consider a laboratory-fixed (LF) frame, an isolated and polyatomic molecule, and a space-fixed (SF) frame with the origin at some point of the molecule, e.g., at the nuclear center of mass. The LF, electrostatic, and non-relativistic Hamiltonian is then equal to the translational one plus an internal SF term, with fully separated coordinates. We thus consider only the SF Hamiltonian ${\widehat{H}}^{\mathrm{SF}}$ with an exact separation of the translation.

Because ${\widehat{H}}^{\mathrm{SF}}$ commutes with the total spinless angular momentum $\widehat{J}$, the molecule is referred to as a body-fixed (BF) frame, thus achieving an approximate separation of the rotation. In 1981, Sutcliffe [1] presented a general BF Hamiltonian $\widehat{H}$, equal to the sum of rotational, electronic, and vibrational terms [Equations (3.18) and (3.20)],

$$\widehat{H}\left(\widehat{J},\widehat{L},Q,q\right)={\widehat{T}}^{\mathrm{rot}}\left(\widehat{J},\widehat{L},Q\right)+{\widehat{H}}^{\mathrm{el}}\left(q;Q\right)+{\widehat{T}}^{\mathrm{vib}}\left(Q\right)$$

(1)

where $\widehat{L}$ is the electronic orbital angular momentum, Q are nuclear vibrational coordinates, and q are those electronic and Cartesians. Contrary to [1], and according to [2], here $\widehat{J}$ satisfies non-standard commutation rules $\widehat{J}\times \widehat{J}=-i\widehat{J}$ (a. u. everywhere, unless otherwise specified), whereas $\widehat{L}$ fulfills the standard ones. ${\widehat{H}}^{\mathrm{el}}$ contains the total nuclear mass M_nucl and the polarization term, due to the removal of the translation. Because M_nucl is much larger than the electron mass, it can be safely removed from the electronic Hamiltonian that thus assumes the usual form.

The ${\widehat{T}}^{\mathrm{rot}}$ representation on the electronic states $\left|e\left(q;Q\right)\right.〉$ gives rise to rovibronic non-adiabatic (NA) Renner-Teller (RT) couplings $\left.〈e^{\prime }\right|{\widehat{L}}_a{\left|e\right.〉}_q$, $\left.〈e^{\prime }\right|{\widehat{L}}_{a^{\prime }a}{\left|e\right.〉}_q$, and $\left.〈e^{\prime }\right|{\widehat{L}}_a\partial /\partial Q{\left|e\right.〉}_q$. The Q-depending term that multiplies $\left.〈e^{\prime }\right|{\widehat{L}}_z{\left|e\right.〉}_q$ diverges when the molecule is linear along z, and this singularity must be removed by a suitable rotational representation.

On the other hand, ${\widehat{T}}^{\mathrm{rot}}$ and ${\widehat{T}}^{\mathrm{vib}}$ depend on $\partial /\partial Q$ and ${\partial }^2/\partial Q^{\prime }\partial Q$, and the electronic representation yields vibronic couplings $\left.〈e^{\prime }\right|\partial /\partial Q{\left|e\right.〉}_q$ and $\left.〈e^{\prime }\right|{\partial }^2/\partial Q^{\prime }Q{\left|e\right.〉}_q$ associated with NA conical-intersection (CI) effects. These couplings are very difficult to compute and diverge at the intersection locus of two adiabatic potential energy surfaces (PESs) $\left.〈e^{\prime }\right|{\widehat{H}}^{\mathrm{el}}{\left|e\right.〉}_q$. It is then better a diabatic electronic representation, coupled by ${\widehat{H}}^{\mathrm{el}}$, whose vibronic couplings divided by the reduced masses are negligible with respect to other terms. More in general, a NA coupling is identically equal to zero if it does not contain a vibrational irreducible representation of the permutation-inversion symmetry group.

Non-relativistic effects in any molecule can be investigated with this Hamiltonian, which is however very complicated, and many approximations are necessary, e.g., separation between linear and non-linear molecules, small vibrations with respect to equilibrium nuclear geometries, point-group symmetries, and normal coordinates. This is discussed in detail by Wilson et al. [3], following Kemble [4].

Exact triatomic Hamiltonians, however, have been well known for many years, and two of them will be discussed in the next sections, with emphasis on non-adiabatic interactions among electronic states.

2. Carter-Handy Hamiltonian

Using the general theory of Sutcliffe [1], this Hamiltonian was obtained in 1984 [5]. In the BF frame, an A−B−C molecule is described by the A−B R₁ and B−C R₂ bond lengths, by the A$\hat{\mathrm{B}}$C bond angle θ, and lies in the (z,x) plane with x bisecting θ, and x_A and x_B ≥ 0. ${\widehat{T}}^{\mathrm{rot}}$ is then equal to

$$\begin{array}{l}{\widehat{T}}^{\mathrm{rot}}={\displaystyle \frac{1}{8{\cos }^2\theta /2}}\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}+{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}-{\displaystyle \frac{2}{m_{\mathrm{B}}{R}_1{R}_2}}\right){\left({\widehat{J}}_z-{\widehat{L}}_z\right)}^2\\ \hspace{1em} +{\displaystyle \frac{1}{8{\sin }^2\theta /2}}\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}+{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}-{\displaystyle \frac{2}{m_{\mathrm{B}}{R}_1{R}_2}}\right){\left({\widehat{J}}_x-{\widehat{L}}_x\right)}^2\\ \hspace{1em} +{\displaystyle \frac{1}{8}}\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}+{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}-{\displaystyle \frac{2\cos \theta }{m_{\mathrm{B}}{R}_1{R}_2}}\right){\left({\widehat{J}}_y-{\widehat{L}}_y\right)}^2\\ \hspace{1em} -{\displaystyle \frac{1}{4\sin \theta }}\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}-{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}\right)\left[\left({\widehat{J}}_z-{\widehat{L}}_z\right)\left({\widehat{J}}_x-{\widehat{L}}_x\right)+\left({\widehat{J}}_x-{\widehat{L}}_x\right)\left({\widehat{J}}_z-{\widehat{L}}_z\right)\right]\\ \hspace{1em} +{\displaystyle \frac{1}{2i}}\left[\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}-{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}\right)\left({\displaystyle \frac{\cot \theta }{2}}+{\displaystyle \frac{\partial }{\partial \theta }}\right)-{\displaystyle \frac{\sin \theta }{m_{\mathrm{B}}}}\left({\displaystyle \frac{1}{R_2}}{\displaystyle \frac{\partial }{\partial R_1}}-{\displaystyle \frac{1}{R_1}}{\displaystyle \frac{\partial }{\partial R_2}}\right)\right]\left({\widehat{J}}_y-{\widehat{L}}_y\right),\end{array}$$

(2)

where $\mu$ are reduced masses, and ${\widehat{T}}^{\mathrm{vib}}$ is

$$\begin{array}{l}{\widehat{T}}^{\mathrm{vib}}=-{\displaystyle \frac{1}{4}}\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}+{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}-{\displaystyle \frac{2\cos \theta }{m_{\mathrm{B}}{R}_1{R}_2}}\right)\left({\displaystyle \frac{{\partial }^2}{\partial {\theta }^2}}+\cot \theta {\displaystyle \frac{\partial }{\partial \theta }}\right)\\ \hspace{1em}\hspace{1em} -{\displaystyle \frac{1}{2{\mu }_1}}{\displaystyle \frac{{\partial }^2}{\partial R_1^2}}-{\displaystyle \frac{1}{2{\mu }_2}}{\displaystyle \frac{{\partial }^2}{\partial R_2^2}}-{\displaystyle \frac{1}{4}}\left({\displaystyle \frac{{\partial }^2}{\partial {\theta }^2}}+\cot \theta {\displaystyle \frac{\partial }{\partial \theta }}\right)\left({\displaystyle \frac{1}{{\mu }_1{R}_1^2}}+{\displaystyle \frac{1}{{\mu }_2{R}_2^2}}-{\displaystyle \frac{2\cos \theta }{m_{\mathrm{B}}{R}_1{R}_2}}\right)\\ \hspace{1em}\hspace{1em} -{\displaystyle \frac{\cos \theta }{m_{\mathrm{B}}}}{\displaystyle \frac{{\partial }^2}{\partial R_1\partial R_2}}+{\displaystyle \frac{1}{m_{\mathrm{B}}}}\left({\displaystyle \frac{1}{R_1}}{\displaystyle \frac{\partial }{\partial R_2}}+{\displaystyle \frac{1}{R_2}}{\displaystyle \frac{\partial }{\partial R_1}}\right)\left(\sin \theta {\displaystyle \frac{\partial }{\partial \theta }}+\cos \theta \right).\end{array}$$

(3)

${\widehat{T}}^{\mathrm{rot}}$ is represented on the ${\widehat{J}}_z$ eigenstates $\left|K\right.〉$ labeled by the helicity quantum number. Therefore, in the matrix elements $\left.〈K^{\prime }\right|{\widehat{T}}^{\mathrm{rot}}\left|K\right.〉$, we have K′ = K, K′ = K ± 1, and K′ = K ± 2, that is Coriolis couplings up to ΔK = ±2. ${\widehat{T}}^{\mathrm{vib}}$ contains the cross term ${\partial }^2/\partial R_1\partial R_2$ also, because the coordinates are not orthogonal, but improves the convergence of the levels and their assignments. This Hamiltonian can be easily employed for investigating spectroscopic and internal-dynamics problems, but it is not suitable for studying the collision dynamics.

Using the variational theory, much better than the perturbation one, we have applied the vibrational Hamiltonian for investigating the NO₂ vibronic spectroscopy and the photo-induced dynamics due to the $\tilde{\mathrm{X}}$²A′(A₁)−$\tilde{\mathrm{A}}$²A′(B₂) CI, where A₁ and B₂ denote diabatic electronic states. This Hamiltonian has been previously employed in the spectroscopic study of CO₂ and SO₂ [6], ${\mathrm{CO}}_2^{+}$ [7], and ${\mathrm{CH}}_2^{+}$ [7].

Figure 1 [8] shows the following: (1) a photon absorption by a vibrational-rotation state of the ground species A₁ creates a Franck-Condon (FC) wave-packet (WP) on the excited B₂ PES; (2) the WP propagates in space and time on B₂; (3) a spontaneous emission of one photon brings back the WP on the A₁ PES.

Our work began with the calculation of a diabatic representation [9] and of NA states and levels [10] up to about 19,000 cm⁻¹, by using primitive, optimized, and Born–Oppenheimer (BO) vibrational basis functions. The practical implementation in [9] involved the ab-initio configuration-interaction method, the analytical fitting of the configuration-interaction data, and a diabatization method based on the electronic dipole-moment matrix. In [10], we used primitive, optimized, and Born-Oppenheimer basis functions, and the diabatic potentials were optimized with respect to 191 observed bands up to 10,000 cm⁻¹, thus converging 1060 non-adiabatic levels up 17,000 cm⁻¹, within 4.4 cm⁻¹, with 6117 basis functions per symmetry.

Figure 2 [11] shows the CI onset, between 8500 and 12,500 cm⁻¹. The first and third columns report the vibrational levels of A₁ and B₂, respectively, together their assignments: symmetric stretching, bending, and anti-symmetric stretching. The second column shows the NA levels E_n. For example, the weights of the highest diabatic states A₁ (0,14,1) and B₂ (0,3,0) are 37% and 23% in the associated NA species, and 32% is the B₂ electronic weight of the last. NA-CI effects begin at ~9500 cm⁻¹ with the coupling A₁ (0,11,1) + B₂ (0,0,0) and then increase with the energy: above 16,000 cm⁻¹, they are very strong and yield a nearly chaotic spectrum, as we shall see below.

The agreement with experimental data is good up to 16,000 cm⁻¹ [10], but it worsens at higher energies owing to inaccuracies of the PESs and coupling.

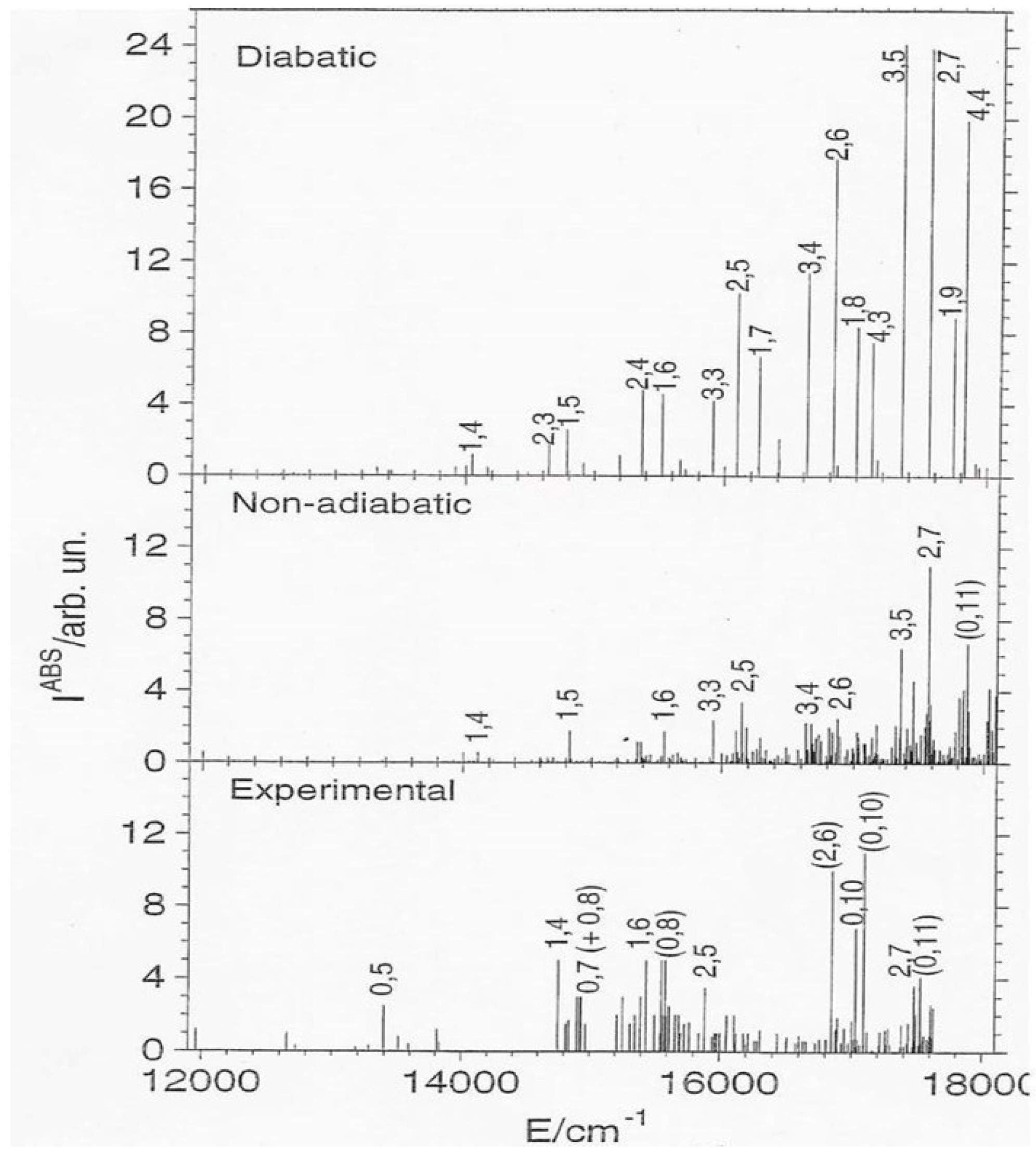
The calculation of the FC absorption spectrum does not present any problem, and Figure 3 thus reports this spectrum [11], showing v₁ and v₂. We pass from a strong and regular diabatic spectrum to a weak and irregular NA one, because the CI coupling mixes dark A₁ and bright B₂ states. These results thus explain the beginning and the development of the CI effects, from the regular far-infrared region up to the chaotic yellow portion of the spectrum. This figure shows also the differences between calculated and observed data.

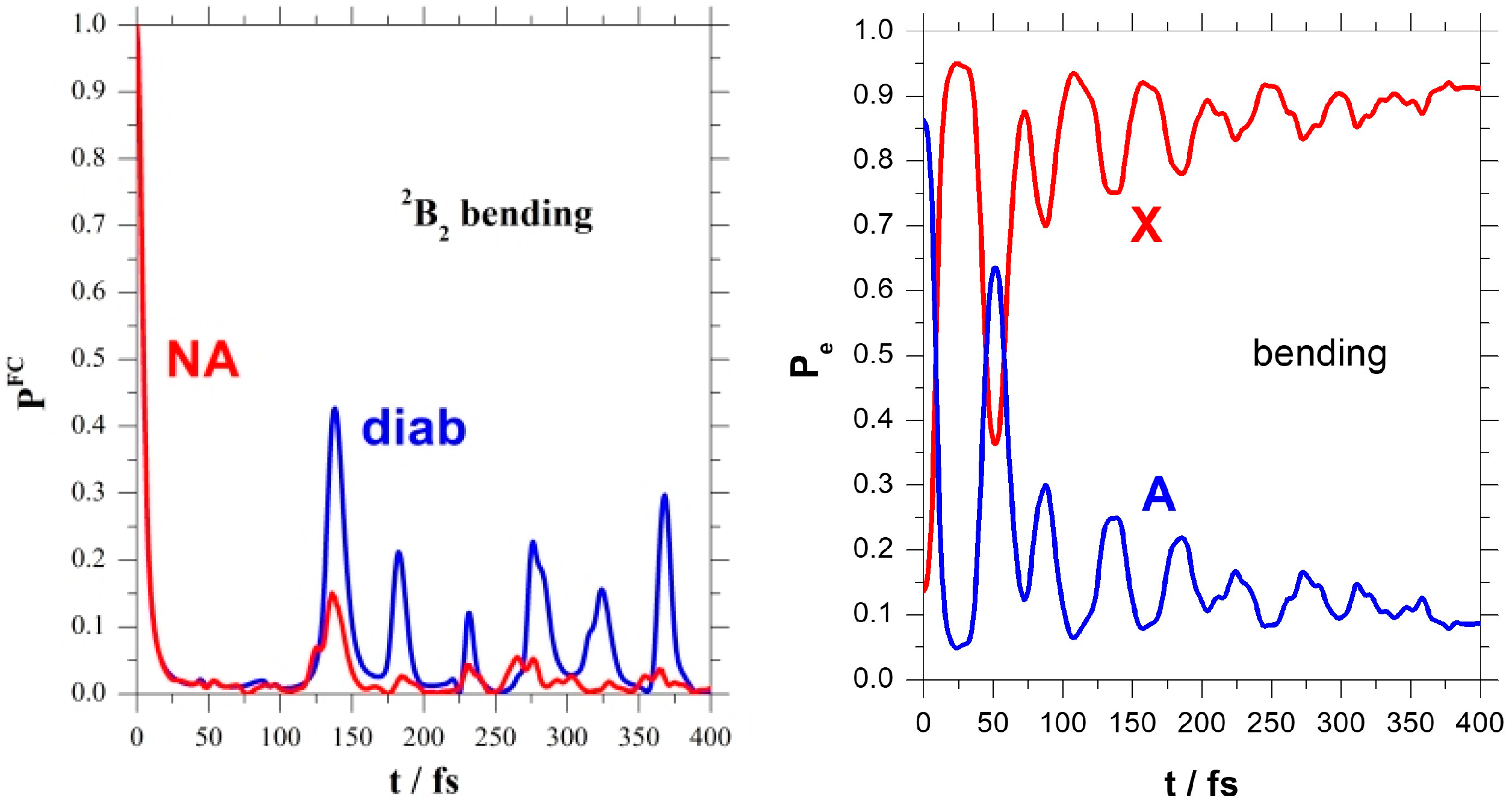
The left panel of Figure 4 confirms these findings, showing the Fourier transformation of the absorption spectrum from energy to the time domain. This is the survival probability of the FC state and points out the differences between the regular B₂ bending recurrences and the irregular NA ones. The electronic populations of the right panel of Figure 4 show again these NA bending recurrences.

Finally, Figure 5 [8] presents six snapshots at different times of the WP photo-induced propagation, from the initial absorption up to the final fluorescence. Here, Q⁺ is the symmetric stretching coordinate and the anti-symmetric stretching is equal to zero. This completes the internal dynamics shown schematically in Figure 1.

3. Tennyson-Sutcliffe Hamiltonian

By the general theory of Sutcliffe [1], this Hamiltonian was obtained in 1983 [12] and then rearranged in [13]. In the BF frame, an A−B−C molecule is described by the Jacobi coordinates R (distance A–BC center of mass), r (distance B–C), and γ (included angle), and lies in the (z,x) plane with z parallel to r. This Hamiltonian is better than the CarterHandy one for investigating A + BC collisions. Following [13], we write

$${\widehat{T}}^{\mathrm{rot}}={\widehat{T}}^{\mathrm{J}}+{\widehat{T}}^{\mathrm{L}}$$

(4)

where the first term includes only $\widehat{J}$ and is equal to

$${\widehat{T}}^{\mathrm{J}}=b\left({\widehat{J}}^2-2{\widehat{J}}_z^2\right)+{\displaystyle \frac{b+B}{{\sin }^2\gamma }}{\widehat{J}}_z^2+b\left[{\widehat{J}}_{+}\left({\widehat{J}}_z\cot \gamma +{\displaystyle \frac{\partial }{\partial \gamma }}\right)+{\widehat{J}}_{-}\left({\widehat{J}}_z\cot \gamma -{\displaystyle \frac{\partial }{\partial \gamma }}\right)\right]$$

(5)

with b = 1/(2μ_rr²) and B = 1/(2μ_RR²). Both $\widehat{L}$ and $\widehat{J}$ are present in the second term,

$$\begin{array}{l}{\widehat{T}}^{\mathrm{L}}=b{\widehat{L}}^2+\left({\displaystyle \frac{b+B}{{\sin }^2\gamma }}-2b\right){\widehat{L}}_z^2+2b{\widehat{L}}_x{\widehat{L}}_z\cot \gamma -2bi{\widehat{L}}_y{\displaystyle \frac{\partial }{\partial \gamma }}+b\left({\widehat{J}}_{+}-{\widehat{J}}_{-}\right)i{\widehat{L}}_y\\ \hspace{1em} -2{\widehat{J}}_z\left[\left({\displaystyle \frac{b+B}{{\sin }^2\gamma }}-b\right){\widehat{L}}_z+b{\widehat{L}}_x\cot \gamma \right]-b\left({\widehat{J}}_{+}+{\widehat{J}}_{-}\right)\left({\widehat{L}}_x+{\widehat{L}}_z\cot \gamma \right),\end{array}$$

(6)

and we have ΔK = ±1 only.

The vibrational Hamiltonian is simply equal to

$${\widehat{T}}^{\mathrm{vib}}=-b{\partial }^2/\partial r^2-B{\partial }^2/\partial R^2-\left(b+B\right)\left({\displaystyle \frac{{\partial }^2}{\partial {\gamma }^2}}+\cot \gamma {\displaystyle \frac{\partial }{\partial \gamma }}\right)$$

(7)

because the Jacobi coordinates are orthogonal. The $1/{\sin }^2\gamma$ and $\cot \gamma$ factors in these equations imply that this Hamiltonian is very large near the linearity.

This Hamiltonian is simpler than the previous one and can be easily applied to collision-dynamics problems, but presents many difficulties in spectroscopy. Therefore, it was employed in many atom + diatom collisions, in particular for investigating the NA quenching Kr + OH(A²Σ⁺) → Kr + OH(X²Π) due to CI and RT interactions among the 1²A′, 2²A′, and 1²A″ adiabatic electronic species, with OH(A²Σ⁺) in the ground vibrational-rotational state [13]. We again employ the variational theory for computing PESs and NA couplings, the reaction probabilities are obtained via the real WP formalism [14], and the Hamiltonian is used in many works, e.g., in [15].

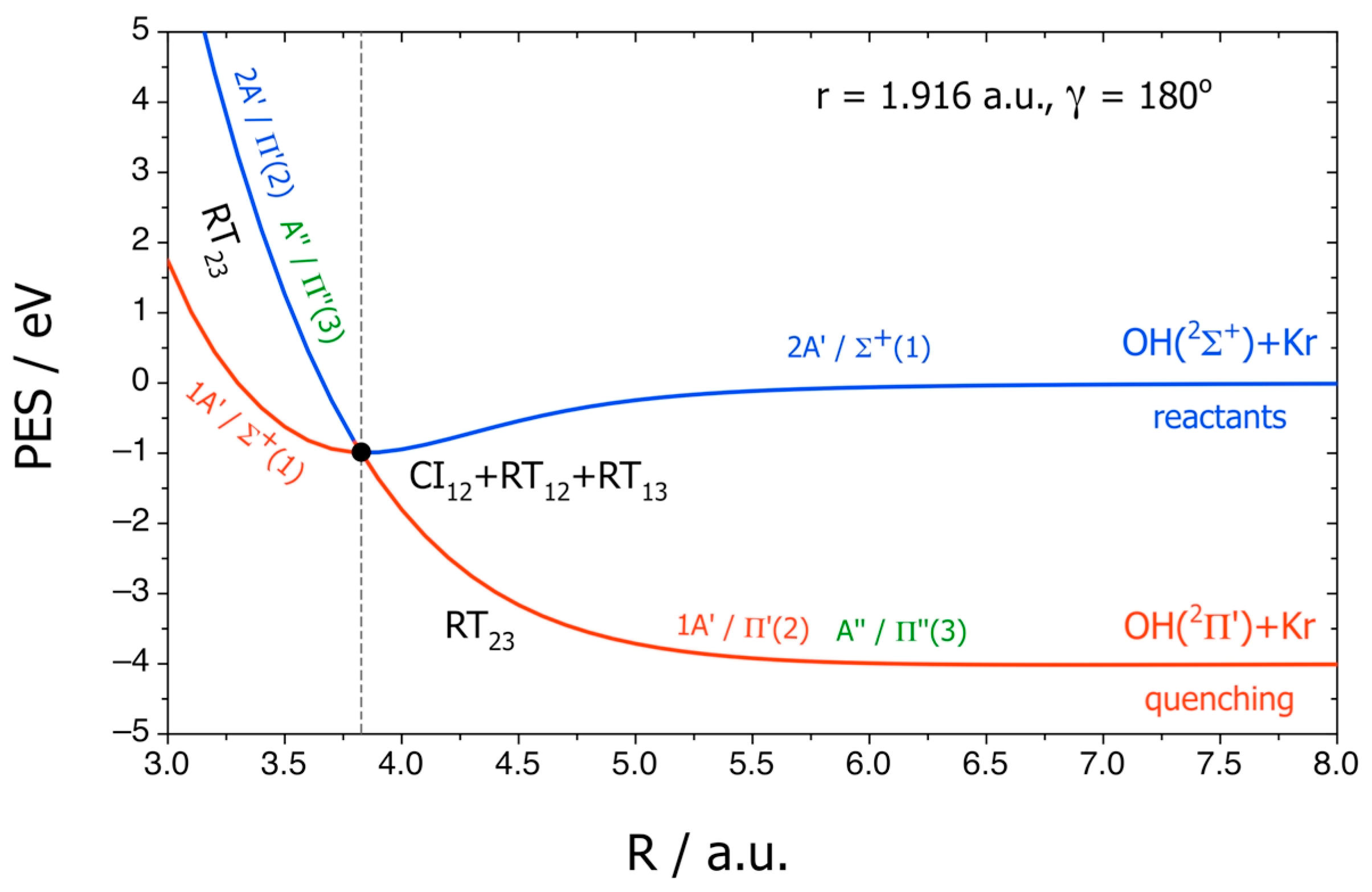
Figure 6 reports R cuts of the adiabatic PESs and of the diabatic ones labeled by Σ⁺(1), Π′(2), and Π″(3), where γ = 180° corresponds to the direction from H to O. The NA couplings CI₁₂, RT₁₂, RT₁₃, and RT₂₃ are shown schematically and yield the electronic quenching, forbidden in the BO approximation.

Three NA couplings are plotted in Figure 7 at r = 1.916: CI₁₂ = $\left.〈{\Sigma }^{+}\right|{\widehat{H}}^{\mathrm{el}}\left|{\Pi }^{\prime }\right.〉$,

$${\mathrm{RT}}_{13}=-iB{\left[2J\left(J+1\right)\right]}^{1/2}\left.〈{\Sigma }^{+}\right|{\widehat{L}}_x+\cot \gamma {\widehat{L}}_z\left|{\Pi }^{″}\right.〉$$

(8)

and

$${\mathrm{RT}}_{23}=-iB{\left[2J\left(J+1\right)\right]}^{1/2}\left.〈{\Pi }^{\prime }\right|{\widehat{L}}_x+\cot \gamma {\widehat{L}}_z\left|{\Pi }^{″}\right.〉$$

(9)

with the z axis along R and at J = 70.

We see that CI₁₂ is larger than RT₁₃, and RT₂₃ and is much larger than RT₁₂ too. The strength of the NA interactions is thus CI₁₂ >> RT₂₃ > RT₁₃ > RT₁₂ and the quenching should be essentially due to the 1A′–2A′ conical intersection, but symmetry implies that Renner–Teller terms are necessary to populate Π″(3).

Initial-state-resolved quenching probabilities are obtained propagating time-dependent WPs ${\psi }^J\left(t\right)$ up to J = 140, with the formalism of [14] and performing a time-to-energy Fourier transform at the end of the propagation. This is a multi-state approach, requiring about 80,000 s per J on Barcelona computers.

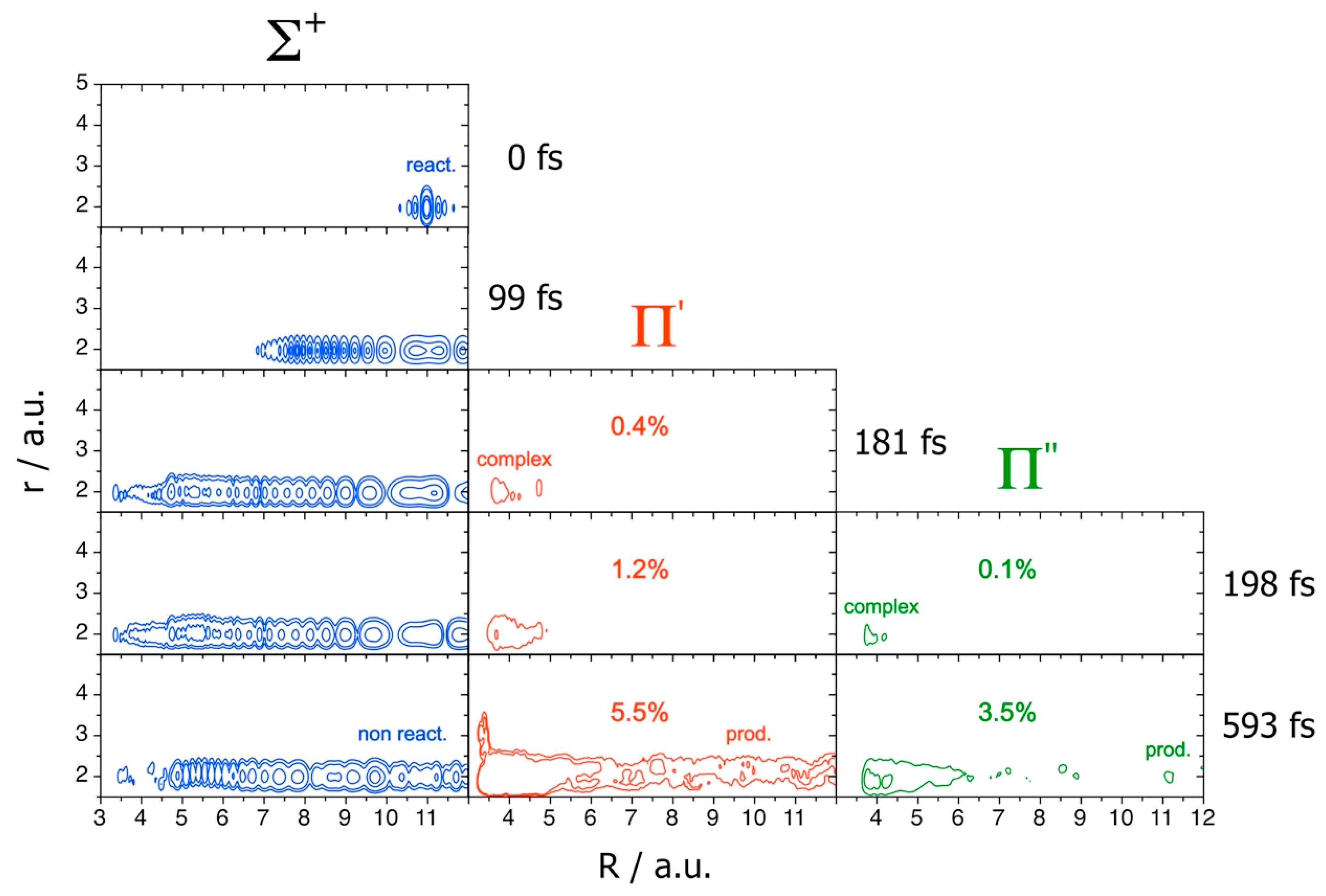
The quenching mechanism on the coupled diabatic PESs $\left|e\right.〉$ is shown in Figure 8, which presents electronic norms and snapshots at five times t of the probability densities $d_{eRr}^J\left(t\right)={{\displaystyle \sum _j\left|〈eRrj|{\psi }^{70}\left(t\right)〉\right|}}^2$ of the WP at J = 70, where j labels the Legendre polynomials.

At the beginning, the WP remains on the Σ⁺ PES and the quenching is closed. At ~180 fs, part of the WP jumps on the Π′ PES owing to the Σ⁺-Π′ conical intersection, forming KrOH metastable complexes. At ~200 fs, also the Π″ surface is populated, and the complexes reveal their full NA nature, spreading on all the PESs. At larger times, we finally see both the non-reactive WP on Σ⁺, due to recrossing of the CI seam, and the reactive densities on Π′ and Π″, together with the formation of the quenching products resolved on these final states.

Quenching probabilities, cross sections, and rate constants confirm the above findings, based on the analysis of the couplings and of the WP. Figure 9 reports CI, RT, and CI + RT probabilities $\left(P_{{\Pi }^{\prime }}^{70}+P_{\Pi ″}^{70}\right)$ versus the collision energy E_col, for the formation of both Π states at J = 70: CI and CI + RT results are nearly equal, whereas those RT are much smaller.

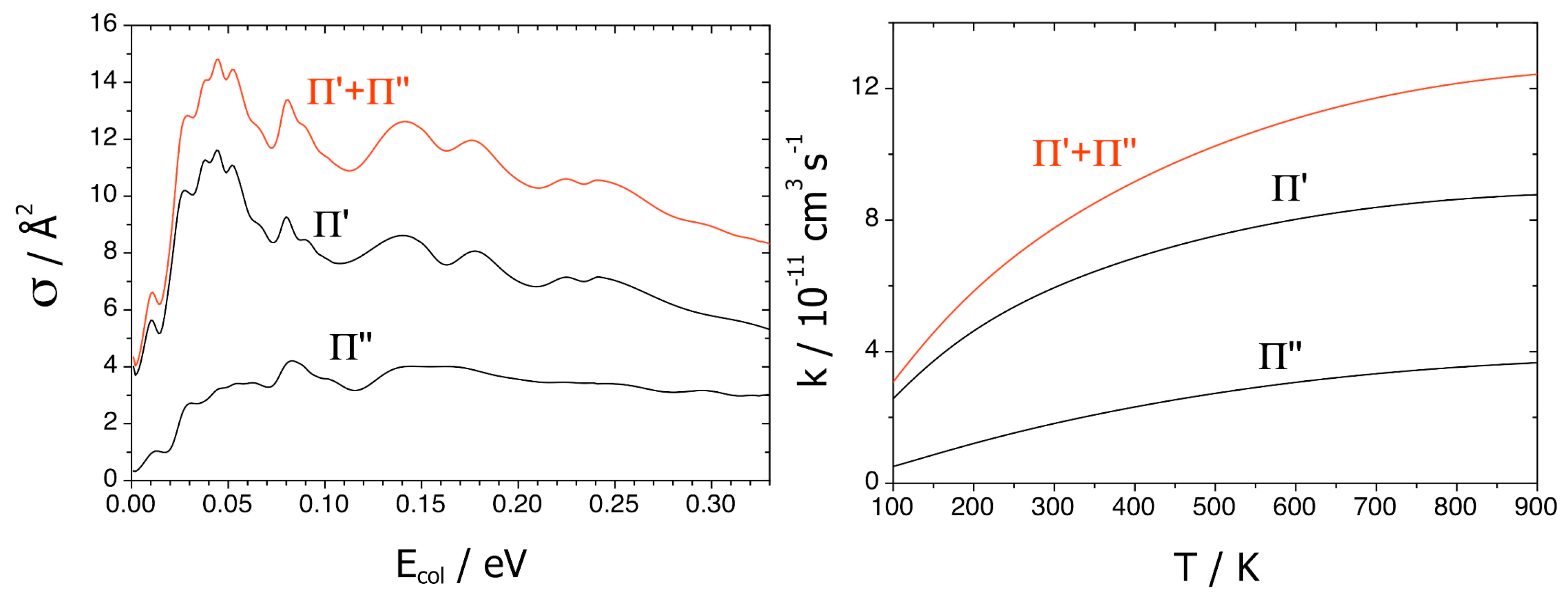
The cross sections σ of Figure 10 point out a strongly preferred Π′ channel at low E_col, in agreement with the CI₁₂-induced quenching and the reaction probabilities. Larger energies then enhance the Π″ weight owing to the RT₁₃ and RT₂₃ couplings. The total σ(E_col = 0.039 eV) is 14.1 Ų, and the thermally averaged <σ(300 K)> is 11.6 Ų, in acceptable agreement with previous experimental results equal to 17.2 ± 0.4 and 10 ± 1 Ų. These differences are due to the limits of the PESs and couplings, as is usual. Finally, the rate constants confirm the cross-section results.

4. Conclusions

The vibrational part of the Carter-Handy Hamiltonian is applied to the spectroscopy of NO₂. With respect to the BO absorption spectrum, the CI line density increases, the NA spectrum is weaker and borrows its intensity from that BO, with many progressions being more irregular. CI effects also extend to the whole spectrum above their beginning.

The Tennyson–Sutcliffe Hamiltonian is applied to the Kr + OH quenching dynamics, finding that CI effects are much larger than those RT which open the Π″(3) channel. In fact, CI is due to the bare electronic Hamiltonian, whereas all RT operators are multiplied by the small factor B = 1/(2μ_RR²) of Equations (8) and (9) depending on the large Kr mass. The different magnitude of these couplings is also due to the different PES regions where NA effects are important: CI in bent geometries and RT near the linearity. CI rules the dynamics that is modulated by RT, these effects are non-additive but in competition, and the former is counterbalanced by the latter. All these findings are similar to those of the H₂O [17,18] and CO₂ [19] photo-dissociations. Finally, CI effects are large in both NO₂ and Kr + OH because the CI minimum geometry is near to that of the B₂ and Σ⁺ PES, respectively.