The Effect of Fractional Order of Time Phase Delay via a Mixed Integral Equation in (2 + 1) Dimensions with an Extended Discontinuous Kernel
Abstract
1. Introduction
2. Formulation of the Problem
- (i)
- In the kernel of position satisfies
- (ii)
- The two functions for the two constants satisfy the following:
- (iii)
- The continuous function in time satisfies for a constant
- (iv)
- The normality of the given function in the space satisfies
3. Special Cases from the General Singular Kernel
- Case 1. Weak singular kernels
- (i)
- The Logarithmic kernels forms can take one of the following forms
- (i-1)
- (i-2)
- (ii)
- Carleman functions forms
- (ii-1)
- (ii-2)
- (iii)
- Logarithmic-Carleman kernels forms
- (iii-1)
- (iii-2)
- Case 2. Cauchy singular kernel
- Case 3. Strong singular kernel
4. The Convergence Procedure
5. Existence and Uniqueness Solution of a Phase-Lag Mixed Integral Equation
6. Error Analysis and Stability
7. Technical Method of Separation
8. The Relation Between the Kernel of Position and Its Coefficients of Fractional Time
9. The Product Nystrӧm Method
- (i)
- Logarithmic kernel type:
- (ii)
- Carleman kernel type:
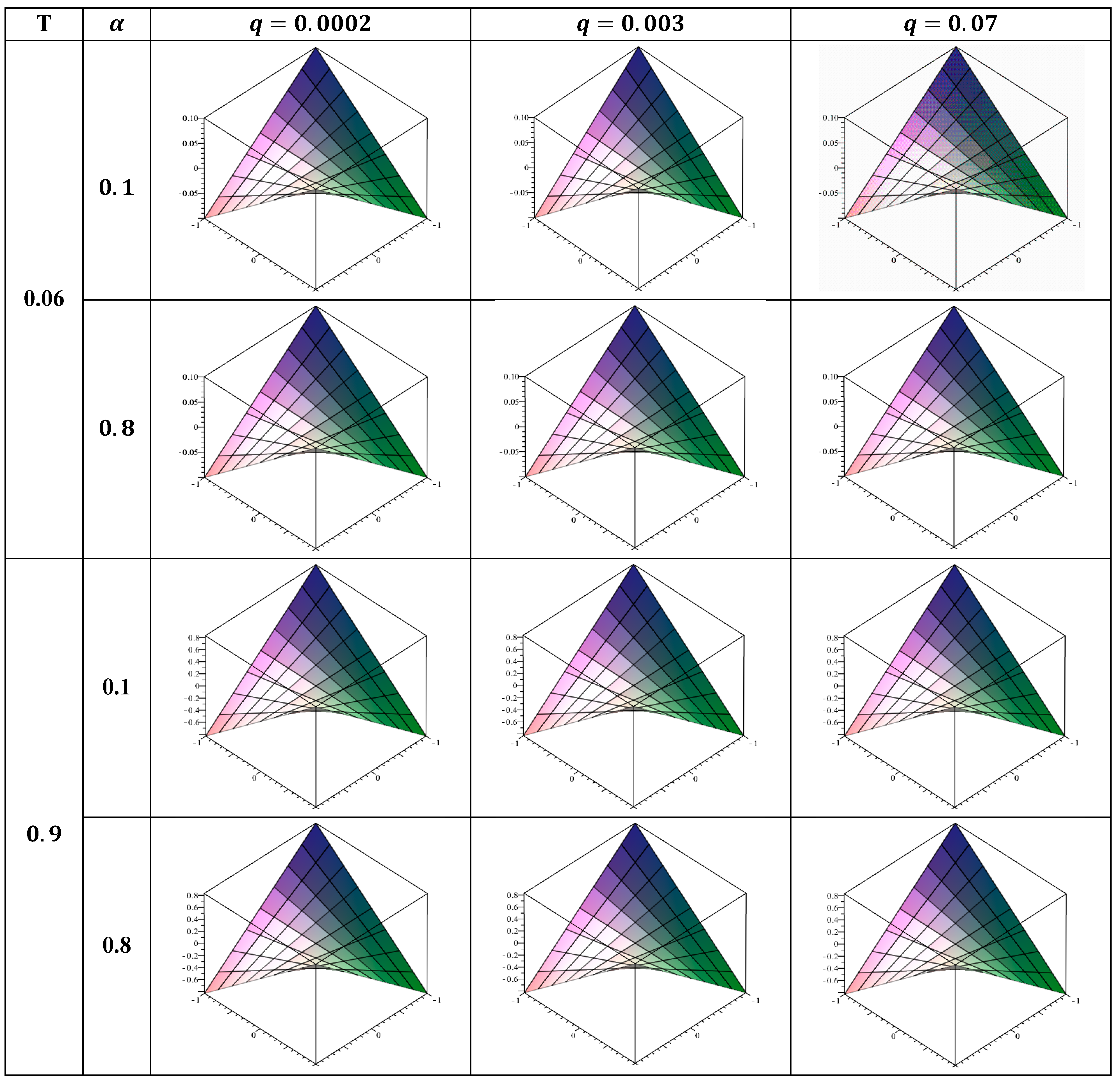
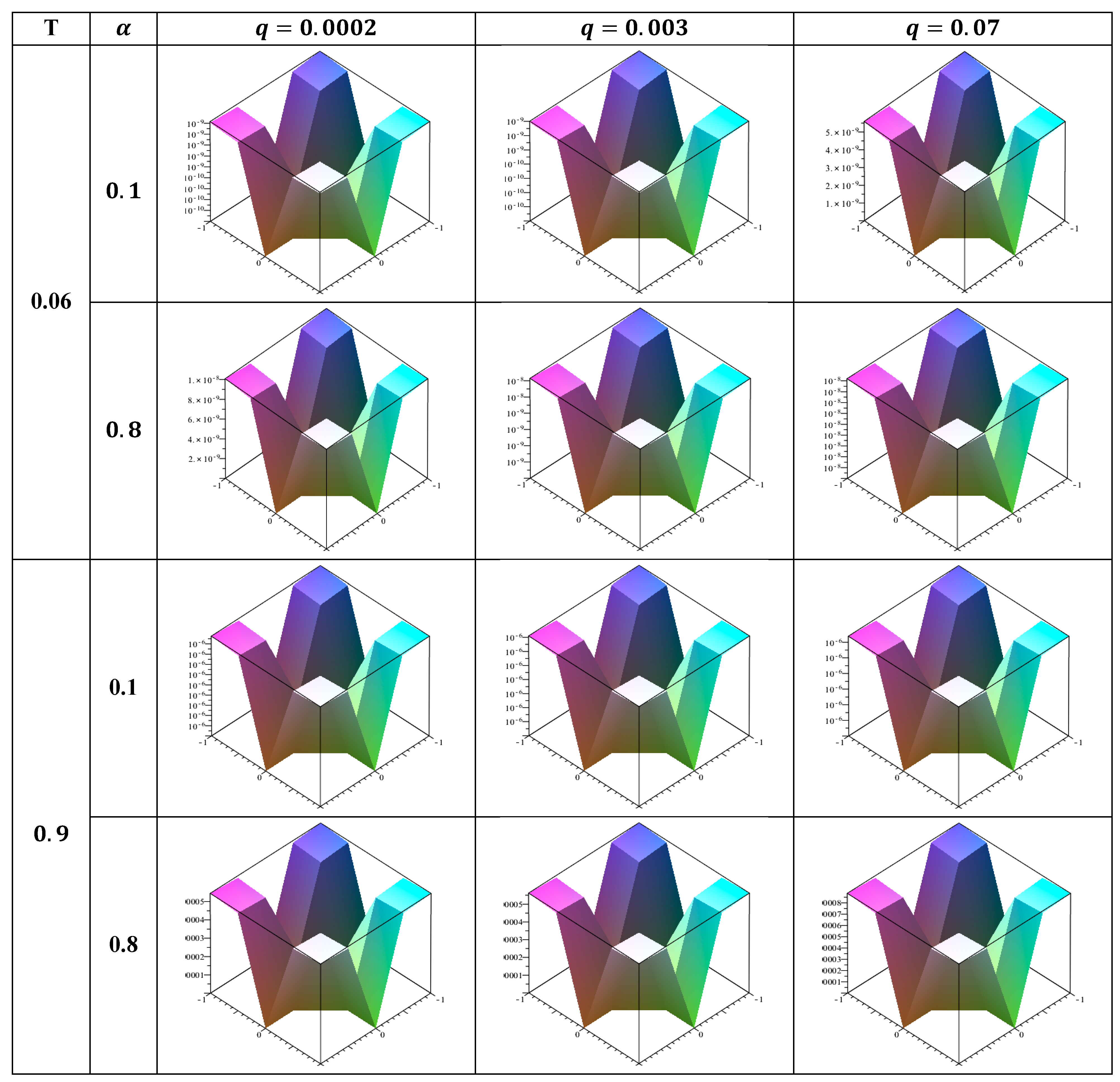
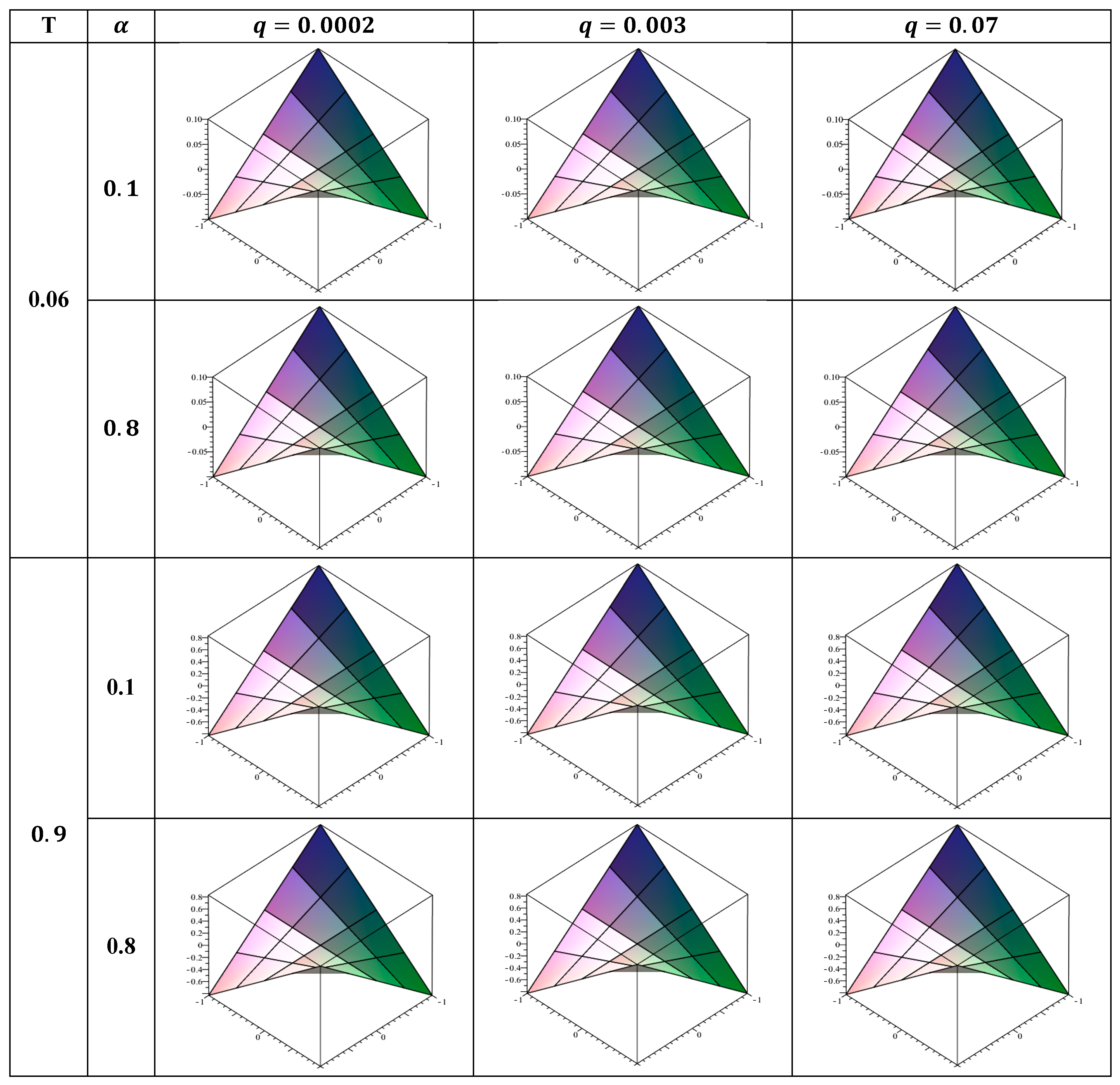
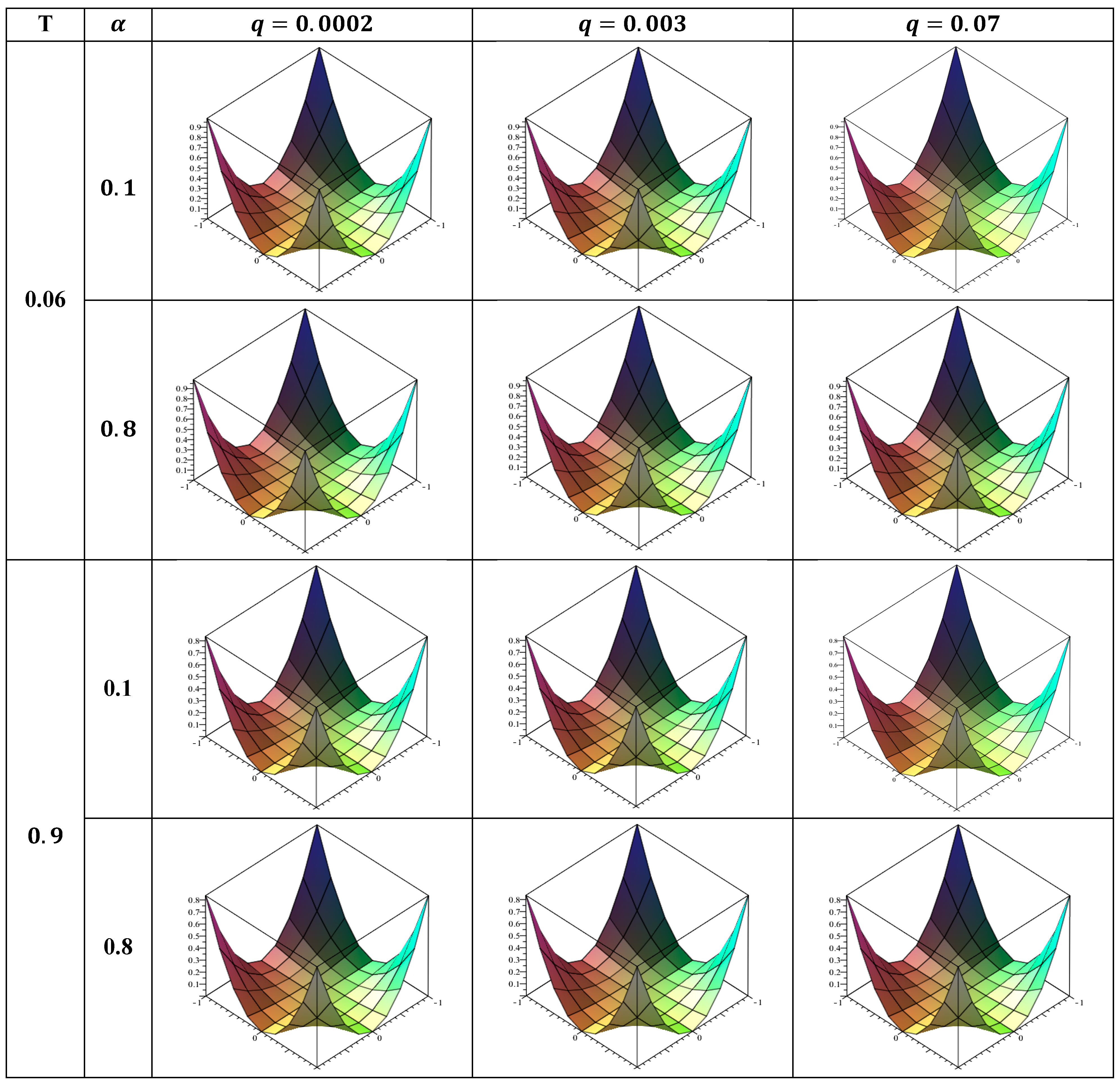
10. Numerical Results and Discussion
11. Discussion
- (1)
- It is apparent that the inaccuracy grows over time, and this makes sense.
- (2)
- Cases where or in applications (1 and 2) all have zero solutions, which is why they are not included in the tables.
- (3)
- The numerical solution is a good approximation of the exact solution, as shown by the extremely small error values.
- (4)
- There seems to be minimal impact on the error values when the fractional power α is altered.
- (5)
- The results presented in the previous tables show how the phase lag’s effect grows over an extended amount of time.
- (6)
- According to the logarithmic kernel results, Application 3’s accuracy is nearly equivalent to that of Application 1.
- (7)
- Application 2 performs better than Application 4 in terms of the Carleman kernel’s results accuracy, indicating that results are impacted by the time function’s status as an exponential function compared to the polynomial.
- (8)
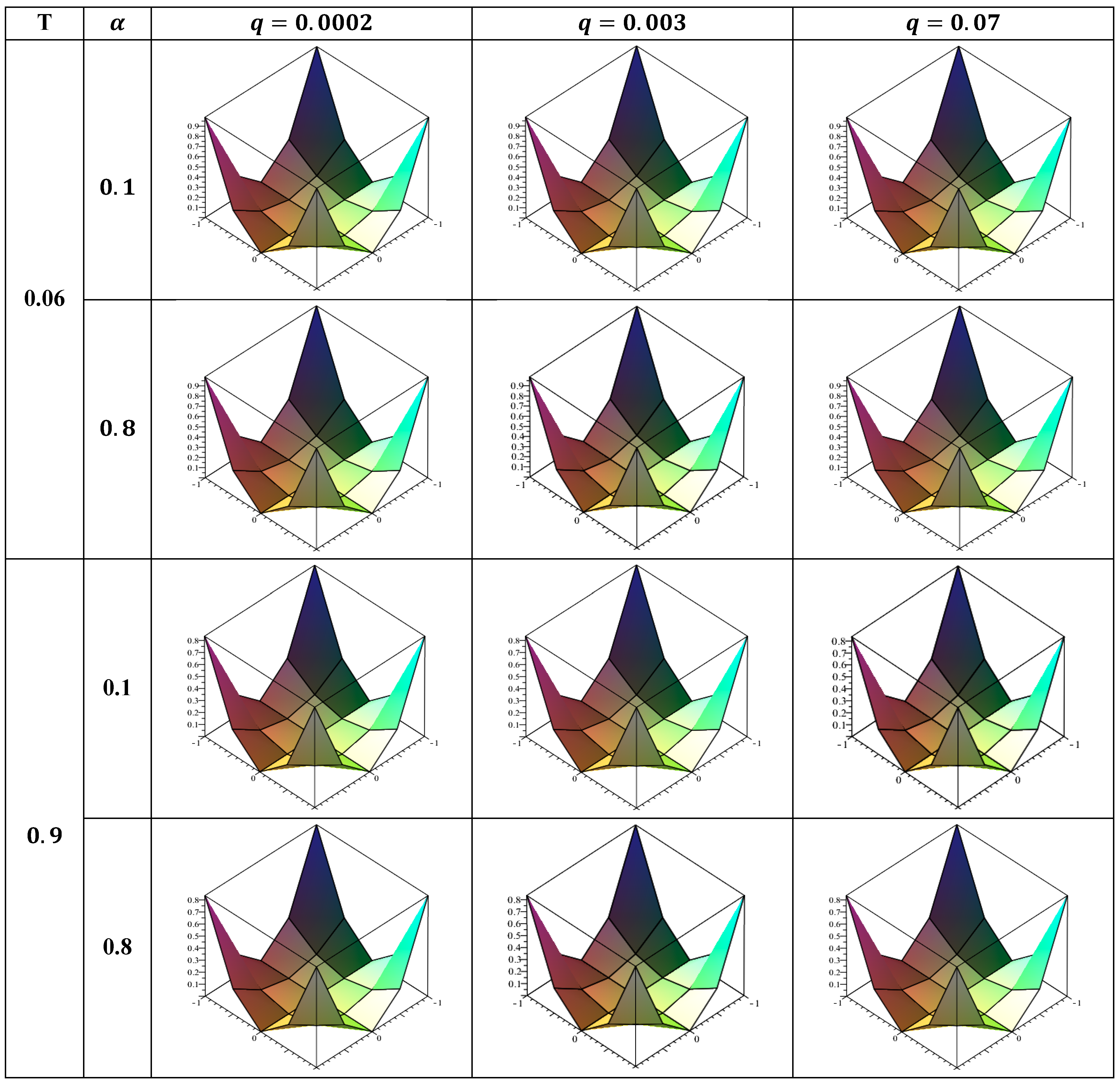
- The numerical results maintain the symmetric property of the exact solution for both variables, and .
- (9)
- The graphs 1–8 verified the effectiveness of the suggested methods.
12. Conclusions
- (1)
- We focused on studying the P-MIE with singular kernels, which has tremendous value in mathematical modeling. After using Taylor’s expansion and then using Riemann-Liouville fractional integral on P-MIE, a MIE in (2 + 1) dimensions in position and time was obtained.
- (2)
- The convergence of the MIE solution was demonstrated by showing that the infinite series of solutions converges uniformly to a continuous function.
- (3)
- The contraction theorem has been used to prove that a solution exists and is unique under certain conditions by proving that the integral operator is bounded and continuous. Error estimation was discussed to prove the convergence of the error and thus the approximate solution.
- (4)
- The separation of variables method is a powerful scheme that allows us to transform a multidimensional MIE that depends on time variable into a set of two-dimensional integral equations in position with time-dependent coefficients.
- (5)
- The connection between position kernels and time-dependent coefficients is investigated. The Nystrӧm method, as an effective numerical method, was applied to the resulting integral equation to achieve a system of linear algebraic equations, which can be easily solved numerically.
- (6)
- PNM was applied to MIE with logarithmic kernel and Carleman function as popular types of singular kernel. Numerical calculations were performed by using Maple 18. The computational results show how effective and applicable the strategies used to solve P-MIE were.
13. Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alhazmi, S.E.; Abdou, M.A.; Basseem, M. Physical phenomena of spectral relationships via quadratic third kind mixed integral equation with discontinuous kernel. AIMS Math. 2023, 8, 24379–24400. [Google Scholar] [CrossRef]
- Basseem, M.; Alalyani, A. On the solution of quadratic nonlinear integral equation with different singular kernels. Math. Probl. Eng. 2020, 1, 7856207. [Google Scholar] [CrossRef]
- Afiatdoust, F.; Heydari, M.H.; Hosseini, M.M. A block-by-block method for nonlinear variable-order fractional quadratic integral equations. Comput. Appl. Math. 2023, 42, 1–38. [Google Scholar] [CrossRef]
- Jan, A.R. An asymptotic model for solving mixed integral equation in position and time. J. Math. 2022, 2022, 8063971. [Google Scholar] [CrossRef]
- Sur, A.; Paul, S.; Kanoria, M. Modeling of memory-dependent derivative in a functionally graded plate. Waves Random Complex Media 2021, 31, 618–638. [Google Scholar] [CrossRef]
- Chiriţă, S. On the time differential dual-phase-lag thermoelastic model. Meccanica 2017, 52, 349–361. [Google Scholar] [CrossRef]
- Farengo, R.; Lee, Y.C.; Guzdar, P.N. An electromagnetic integral equation: Application to microtearing modes. Phys. Fluids 1983, 26, 3515–3523. [Google Scholar] [CrossRef]
- Abdou, M.A.; Nasr, M.E.; Abdel-Aty, M.A. Study of the normality and continuity for the mixed integral equations with phase-lag term. Int. J. Math. Anal. 2017, 11, 787–799. [Google Scholar] [CrossRef]
- Aljawi, S.; Aljohani, S.; Kamran; Ahmed, A.; Mlaiki, N. Numerical Solution of the Linear Fractional Delay Differential Equation Using Gauss–Hermite Quadrature. Symmetry 2024, 16, 721. [Google Scholar] [CrossRef]
- Rostami, Y.; Maleknejad, K. A novel approach to solving system of integral partial differential equations based on hybrid modified block-pulse functions. Math. Methods Appl. Sci. 2024, 47, 5798–5818. [Google Scholar] [CrossRef]
- Nili Ahmadabadi, M. An efficient method for mixed integral equations with phase lag. Int. J. Comput. Math. 2020, 97, 1170–1182. [Google Scholar] [CrossRef]
- Mosa, G.A.; Abdou, M.A.; Rahby, A.S. Numerical solutions for nonlinear Volterra-Fredholm integral equations of the second kind with a phase lag. AIMS Math 2021, 6, 8525–8543. [Google Scholar] [CrossRef]
- Jan, A.R.; Abdou, M.A.; Basseem, B. A Physical Phenomenon for the Fractional Nonlinear Mixed Integro-Differential Equation Using a Quadrature Nystrom Method. Fractal Fract. 2023, 7, 656. [Google Scholar] [CrossRef]
- Xie, J. Numerical computation of fractional partial differential equations with variable coefficients utilizing the modified fractional Legendre wavelets and error analysis. Math. Methods Appl. Sci. 2021, 44, 7150–7164. [Google Scholar] [CrossRef]
- Chandler-Wilde, S.N.; Gover, M.J.C. On the application of a generalization of Toeplitz matrices to the numerical solution of integral equations with weakly singular convolution kernels. J. Numer. Anal. IMAJNA 1989, 9, 525–544. [Google Scholar] [CrossRef]
- Golberg, M.A. Introduction to the numerical solution of Cauchy singular integral equations. In Numerical Solution of Integral Equations; Springer: Berlin/Heidelberg, Germany, 1990; pp. 183–308. [Google Scholar]
- Shpazzadeh, E.; Chu, Y.M.; Hashemi, M.S.; Moharrami, M.; Inc, M. Hermite multiwavelets representation for the sparse solution of nonlinear Abel’s integral equation. Appl. Math. Comput. 2022, 427, 127171. [Google Scholar] [CrossRef]
- Assari, P.; Adibi, H.; Dehghan, M. The numerical solution of weakly singular integral equations based on the meshless product integration (MPI) method with error analysis. Appl. Numer. Math. 2014, 81, 76–93. [Google Scholar] [CrossRef]
- Theocaris, P.S. Numerical solution of singular integral equations: Methods. J. Eng. Mech. Div. 1981, 107, 733–752. [Google Scholar] [CrossRef]
- Raad, S.A.; Abdou, M.A. An Algorithm for the Solution of Integro-Fractional Differential Equations with a Generalized Symmetric Singular Kernel. Fractal Fract. 2024, 8, 644. [Google Scholar] [CrossRef]
- Raad, S.A.; Al-Atawi, M.M. Nyström Method to Solve Two-Dimensional Volterra Integral Equation with Discontinuous Kernel. J. Comput. Theor. Nanosci. 2021, 18, 1177–1184. [Google Scholar] [CrossRef]
- Laguardia, A.L.; Russo, M.G. A Nyström Method for 2D Linear Fredholm Integral Equations on Curvilinear Domains. Mathematics 2023, 11, 4859. [Google Scholar] [CrossRef]



| 0.06 | 0.1 | 0.0002 | 2.24647177 × 10−2 | 1.558214555 × 10−9 | 1.44169605 × 107 |
| 0.003 | 2.92986494 × 10−2 | 1.88027055 × 107 | |||
| 0.07 | 7.32816318 × 10−3 | 4.70292307 × 106 | |||
| 0.5 | 0.0002 | 4.56402136 × 10−3 | 2.060115707 × 10−9 | 2.21541991 × 106 | |
| 0.003 | 1.93917719 × 10−3 | 9.41295281 × 105 | |||
| 0.07 | 1.56621550 × 10−3 | 7.60256084 × 105 | |||
| 0.8 | 0.0002 | 2.93925202 × 10−3 | 1.125466208 × 10−9 | 2.61158620 × 106 | |
| 0.003 | 2.41031307 × 10−3 | 2.14161300 × 106 | |||
| 0.07 | 3.215797908 × 10−4 | 2.85730294 × 105 | |||
| 0.9 | 0.1 | 0.0002 | 0.1102612531 | 4.948630959 × 10−6 | 2.22811630 × 104 |
| 0.003 | 0.1514828497 | 3.06110621 × 104 | |||
| 0.07 | 0.1685235510 | 3.40545804 × 104 | |||
| 0.5 | 0.0002 | 7.68838019 × 10−2 | 1.836458028 × 10−5 | 4.18652649 × 103 | |
| 0.003 | 6.29569392 × 10−2 | 3.42817196 × 104 | |||
| 0.07 | 4.22343163 × 10−3 | 2.29977030 × 103 | |||
| 0.8 | 0.0002 | 8.80384991 × 10−2 | 2.204893242 × 10−5 | 3.99286902 × 103 | |
| 0.003 | 8.52627409 × 10−2 | 3.86697819 × 103 | |||
| 0.07 | 5.41758858 × 10−2 | 2.45707523 × 104 |
| 0.06 | 0.1 | 0.0002 | 2.26357831 × 10−2 | 7.94583098 × 10−11 | 2.84876222 × 108 |
| 0.003 | 2.95336629 × 10−2 | 3.71687530 × 108 | |||
| 0.07 | 6.99334837 × 10−3 | 8.80128006 × 107 | |||
| 0.5 | 0.0002 | 4.60677683 × 10−3 | 1.03030152 × 10−10 | 4.47128993 × 107 | |
| 0.003 | 1.96091517 × 10−3 | 1.90324398 × 107 | |||
| 0.07 | 1.47942490 × 10−3 | 1.43591450 × 107 | |||
| 0.8 | 0.0002 | 2.96781289 × 10−3 | 5.54826340 × 10−11 | 5.34908434 × 107 | |
| 0.003 | 2.43469571 × 10−3 | 4.38821218 × 107 | |||
| 0.07 | 2.92036874 × 10−4 | 5.26357263 × 106 | |||
| 0.9 | 0.1 | 0.0002 | 0.3485415170 | 5.752106720 × 10−6 | 6.05937153 × 104 |
| 0.003 | 0.4845708396 | 8.42423243 × 104 | |||
| 0.07 | 0.6495743338 | 1.12928074 × 105 | |||
| 0.5 | 0.0002 | 0.3424298187 | 2.219335342 × 10−5 | 1.54293861 × 104 | |
| 0.003 | 0.2973429841 | 1.33978394 × 104 | |||
| 0.07 | 7.7707867 × 10−2 | 3.50140268 × 103 | |||
| 0.8 | 0.0002 | 0.4424471260 | 2.705506654 × 10−5 | 1.63535774 × 104 | |
| 0.003 | 0.4334768256 | 1.60220203 × 104 | |||
| 0.07 | 0.3224413618 | 1.19179659 × 104 |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | ±0.100216007 | 6.9774 × 10−9 | ±0.100216005 | 5.34990 × 10−9 | ±0.100215979 | 2.13073 × 10−8 |
| (±0.5, ±1) (±1, ±0.5) | ±0.05010801 | 6.3372 × 10−9 | ±0.05010801 | 4.87618 × 10−9 | ±0.05010798 | 1.94338 × 10−8 | |
| (0.0, ±1) (±1, 0.0) | ±1.15 × 10−20 | 1.1464 × 10−20 | ±6.74 × 10−21 | 6.7399 × 10−21 | ±8.62 × 10−21 | 8.6201 × 10−21 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±5.73 × 10−21 | 5.7321 × 10−21 | ±3.37 × 10−21 | 3.3699 × 10−21 | ±4.31 × 10−21 | 4.3101 × 10−21 | |
| (±0.5, ±0.5) | ±0.025054006 | 5.78279 × 10−9 | ±0.025054004 | 4.44250 × 10−9 | ±0.025053982 | 1.77307 × 10−8 | |
| 0.8 | (±1, ±1) | ±0.100215962 | 3.83557 × 10−8 | ±0.100215953 | 4.67728 × 10−8 | ±0.100215649 | 3.50759 × 10−7 |
| (±0.5, ±1) (±1, ±0.5) | ±0.05010796 | 3.49964 × 10−8 | ±0.05010796 | 5.50074 × 10−8 | ±0.05010768 | 3.19868 × 10−7 | |
| (0.0, ±1) (±1, 0.0) | ±3.51 × 10−21 | 3.5096 × 10−21 | ±5.22 × 10−21 | 5.2189 × 10−21 | ±3.21 × 10−19 | 3.2053 × 10−19 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±1.75 × 10−21 | 1.7548 × 10−21 | ±2.61 × 10−21 | 2.6095 × 10−21 | ±1.60 × 10−19 | 1.6026 × 10−19 | |
| (±0.5, ±0.5) | ±0250539681 | 3.19071 × 10−8 | ±0.02505396 | 5.01720 × 10−8 | ±0.025053708 | 2.91713 × 10−8 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) (−1, −1) | ±0.829037206 | 3.72063 × 10−5 | ±0.829027082 | 2.70817 × 10−5 | ±0.829024343 | 2.43433 × 10−5 |
| (±0.5, ±1) (±1, ±0.5) | ±0.41453393 | 3.39313 × 10−5 | ±0.41452469 | 2.46979 × 10−5 | ±0.41452220 | 2.22005 × 10−5 | |
| (0.0, ±1) (±1, 0.0) | ±6.24 × 10−18 | 6.2399 × 10−18 | ±3.31 × 10−18 | 3.3059 × 10−18 | ±2.67 × 10−18 | 2.6711 × 10−18 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±3.12 × 10−18 | 3.1202 × 10−18 | ±1.65 × 10−18 | 1.6530 × 10−18 | ±1.34 × 10−18 | 1.3356 × 10−18 | |
| (±0.5, ±0.5) | ±0.207280945 | 3.09447 × 10−5 | ±0.207272524 | 2.25239 × 10−5 | ±0.207270246 | 2.02464 × 10−5 | |
| 0.8 | (±1, ±1) | ±0.828792380 | 2.07620 × 10−4 | ±0.828785621 | 2.14379 × 10−4 | ±0.828662607 | 3.37393 × 10−4 |
| (±0.5, ±1) (±1, ±0.5) | ±0.41431065 | 1.89345 × 10−4 | ±0.41430449 | 1.95510 × 10−4 | ±0.41419230 | 3.07696 × 10−4 | |
| (0.0, ±1) (±1, 0.0) | ±1.25 × 10−18 | 1.2482 × 10−18 | ±.1.65 × 10−18 | 1.6530 × 10−18 | ±3.30 × 10−18 | 3.2966 × 10−18 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±6.24 × 10−19 | 6.2410 × 10−19 | ±6.66 × 10−19 | 6.6558 × 10−19 | ±1.65 × 10−18 | 1.6483 × 10−18 | |
| (±0.5, ±0.5) | ±0.207077321 | 1.72679 × 10−4 | ±0.207071699 | 1.78301 × 10−4 | ±0.206969388 | 2.80612 × 10−4 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | ±0.100216002 | 1.8093 × 10−9 | ±0.100216001 | 1.3873 × 10−9 | ±0.100215994 | 5.5875 × 10−9 |
| (±0.5, ±1) (±1, ±0.5) | ±0.05010800 | 1.7763 × 10−9 | ±0.05010800 | 1.3791 × 10−9 | ±0.05010799 | 5.4727 × 10−9 | |
| (0.0, ±1) (±1, 0.0) | ±1.09 × 10−18 | 1.094 × 10−18 | ±6.43 × 10−19 | 6.429 × 10−19 | ±1.32 × 10−18 | 1.318 × 10−18 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±5.47 × 10−18 | 5.468 × 10−18 | ±3.21 × 10−19 | 3.215 × 10−19 | ±6.59 × 10−19 | 6.588 × 10−19 | |
| (±0.5, ±0.5) | ±0.025054002 | 1.7447 × 10−9 | ±0.025054001 | 1.3463 × 10−9 | ±0.025053995 | 5.3689 × 10−9 | |
| 0.8 | (±1, ±1) | ±0.100215990 | 1.0073 × 10−8 | ±0.100215988 | 1.2283 × 10−8 | ±0.100215908 | 9.1971 × 10−8 |
| (±0.5, ±1) (±1, ±0.5) | ±0.05010799 | 9.8341 × 10−9 | ±0.05010799 | 1.1992 × 10−8 | ±0.05010791 | 9.0024 × 10−8 | |
| (0.0, ±1) (±1, 0.0) | ±5.53 × 10−18 | 5.528 × 10−18 | ±8.22 × 10−18 | 8.220 × 10−18 | ±5.24 × 10−17 | 5.237 × 10−17 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±2.76 × 10−18 | 2.7639 × 10−18 | ±4.11 × 10−18 | 4.110 × 10−18 | ±2.62 × 10−17 | 2.6186 × 10−17 | |
| (±0.5, ±0.5) | ±0.025053990 | 9.6324 × 10−9 | ±0.025053988 | 1.1746 × 10−8 | ±0.025053912 | 8.8136 × 10−8 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | ±0.829009757 | 9.7566 × 10−6 | ±0.829007102 | 7.1017 × 10−6 | ±0.829006384 | 6.3836 × 10−6 |
| (±0.5, ±1) (±1, ±0.5) | ±0.41450955 | 9.5511 × 10−6 | ±0.41450695 | 6.9521 × 10−6 | ±0.41450625 | 6.2491 × 10−6 | |
| (0.0, ±1) (±1, 0.0) | ±1.11 × 10−15 | 1.105 × 10−15 | ±5.85 × 10−16 | 5.852 × 10−16 | ±4.73 × 10−16 | 4.729 × 10−16 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±5.52 × 10−15 | 5.523 × 10−15 | ±2.93 × 10−16 | 2.926 × 10−16 | ±2.36 × 10−16 | 2.364 × 10−16 | |
| (±0.5, ±0.5) | ±0.207259350 | 9.3499 × 10−6 | ±0.207256806 | 6.8056 × 10−6 | ±0.207256117 | 6.1174 × 10−6 | |
| 0.8 | (±1, ±1) | ±0.828945556 | 5.4444 × 10−5 | ±0.828943784 | 5.6216 × 10−5 | ±0.828911526 | 8.8474 × 10−5 |
| (±0.5, ±1) (±1, ±0.5) | ±0.41444670 | 5.3297 × 10−5 | ±0.41444497 | 5.5032 × 10−5 | ±0.41441389 | 8.6611 × 10−5 | |
| (0.0, ±1) (±1, 0.0) | ±5.20 × 10−16 | 5.196 × 10−16 | ±5.54 × 10−16 | 5.539 × 10−16 | ±1.37 × 10−15 | 1.371 × 10−15 | |
| (0.0, ±0.5) (±0.5, 0.0) | ±2.60 × 10−16 | 2.598 × 10−16 | ±2.77 × 10−16 | 2.769 × 10−16 | ±6.86 × 10−16 | 6.856 × 10−16 | |
| (±0.5, ±0.5) | ±0.207197825 | 5.2175 × 10−5 | ±0.207196127 | 5.3873 × 10−5 | ±0.207165214 | 8.4786 × 10−6 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | 0.988071714 | 1.958 × 10−10 | 0.988071713 | 1.501 × 10 | 0.988071713 | 3.513 × 10−10 |
| (±0.5, ±1) (±1, ±0.5) | 0.247017928 | 2.678 × 10−10 | 0.247017928 | 1.501 × 10−10 | 0.247017927 | 7.609 × 10−10 | |
| (0.0, ±1) (±1, 0.0) | 7.381 × 10−11 | 7.381 × 10−11 | 5.65697 × 10−11 | 5.657 × 10−11 | 2.389 × 10−10 | 2.389 × 10−10 | |
| (0.0, ±0.5) (±0.5, 0.0) | 2.746 × 10−10 | 2.746 × 10−10 | 2.10452× 10−10 | 2.105 × 10−10 | 8.888 × 10−10 | 8.888 × 10−10 | |
| (±0.5, ±0.5) | 0.061754483 | 5.471 × 10−10 | 0.061754482 | 4.082 × 10−10 | 0.061754480 | 1.8292 × 10−9 | |
| (0.0, 0.0) | 1.713 × 10−10 | 1.713 × 10−10 | 1.31276 × 10−10 | 1.313 × 10−10 | 5.544 × 10−10 | 5.544 × 10−10 | |
| 0.8 | (±1, ±1) | 0.988071714 | 7.042 × 10−10 | 0.988071714 | 8.342 × 10−10 | 0.988071719 | 5.8033 × 10−9 |
| (±0.5, ±1) (±1, ±0.5) | 0.247017927 | 7.0422 × 10−9 | 0.247017927 | 1.5562 × 10−9 | 0.247017916 | 1.2444 × 10−8 | |
| (0.0, ±1) (±1, 0.0) | 3.931 × 10−10 | 3.931 × 10−10 | 4.79153 × 10−10 | 4.791 × 10−10 | 3.9947 × 10−9 | 3.9947 × 10−9 | |
| (0.0, ±0.5) (±0.5, 0.0) | 1.4623 × 10−9 | 1.4623 × 10−9 | 1.782555 × 10−9 | 1.7826 × 10−9 | 1.4861 × 10−8 | 1.4861 × 10−8 | |
| (±0.5, ±0.5) | 0.061754479 | 2.9951 × 10−9 | 0.061754478 | 3.6777 × 10−9 | 0.061754452 | 3.0483 × 10−8 | |
| (0.0, 0.0) | 9.122 × 10−10 | 9.122 × 10−10 | 1.111926 × 10−9 | 1.1119 × 10−9 | 9.2701 × 10−9 | 9.2701 × 10−9 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | 0.835269777 | 4.3446 × 10−7 | 0.835269899 | 3.1250 × 10−7 | 0.835269978 | 2.3311 × 10−7 |
| (±0.5, ±1) (±1, ±0.5) | 0.208818468 | 9.1511 × 10−7 | 0.208818211 | 6.5817 × 10−7 | 0.208818044 | 4.9093 × 10−7 | |
| (0.0, ±1) (±1, 0.0) | 2.9334 × 10−7 | 2.9334 × 10−7 | 2.1099 × 10−7 | 2.1099 × 10−7 | 1.5740 × 10−7 | 1.5740 × 10−7 | |
| (0.0, ±0.5) (±0.5, 0.0) | 1.0913 × 10−6 | 1.0913 × 10−6 | 7.8494 × 10−7 | 7.8494 × 10−7 | 5.8555 × 10−7 | 5.8555 × 10−7 | |
| (±0.5, ±0.5) | 0.052206628 | 2.2398 × 10−6 | 0.052205999 | 1.6110 × 10−6 | 0.052205590 | 1.2018 × 10−6 | |
| (0.0, 0.0) | 6.8072 × 10−7 | 6.8072 × 10−7 | 4.8963 × 10−7 | 4.8963 × 10−7 | 3.6526 × 10−7 | 3.6526 × 10−7 | |
| 0.8 | (±1, ±1) | 0.835271821 | 1.6095 × 10−6 | 0.835271854 | 1.6428 × 10−6 | 0.835272420 | 2.2084 × 10−6 |
| (±0.5, ±1) (±1, ±0.5) | 0.208814162 | 3.3906 × 10−6 | 0.208814092 | 3.4608 × 10−6 | 0.208812900 | 4.6524 × 10−6 | |
| (0.0, ±1) (±1, 0.0) | 1.0869 × 10−6 | 1.0869 × 10−6 | 1.1094 × 10−6 | 1.1094 × 10−6 | 1.4915 × 10−6 | 1.4915 × 10−6 | |
| (0.0, ±0.5) (±0.5, 0.0) | 4.0436 × 10−6 | 4.0436 × 10−6 | 4.1273 × 10−6 | 4.1273 × 10−6 | 5.5486 × 10−6 | 5.5486 × 10−6 | |
| (±0.5, ±0.5) | 0.052196089 | 8.2990 × 10−5 | 0.052195917 | 8.4708 × 10−6 | 0.052193000 | 1.1388 × 10−5 | |
| (0.0, 0.0) | 2.5223 × 10−6 | 2.5223 × 10−6 | 2.5745 × 10−6 | 2.5745 × 10−6 | 3.4611 × 10−6 | 3.4611 × 10−6 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | 0.988071720 | 6.7307 × 10−9 | 0.988071718 | 5.1587 × 10−9 | 0.988071691 | 2.1927 × 10−8 |
| (±0.5, ±1) (±1, ±0.5) | 0.247017935 | 6.8470 × 10−9 | 0.247017933 | 5.2369 × 10−9 | 0.247017906 | 2.1950 × 10−8 | |
| (0.0, ±1) (±1, 0.0) | 7.9725 × 10−9 | 7.9725 × 10−9 | 6.1104 × 10−9 | 6.1104 × 10−9 | 2.5805 × 10−8 | 2.5805 × 10−8 | |
| (0.0, ±0.5) (±0.5, 0.0) | 3.7631 × 10−9 | 3.7631 × 10−9 | 2.8842 × 10−9 | 2.8842 × 10−9 | 1.2180 × 10−8 | 1.2180 × 10−8 | |
| (±0.5, ±0.5) | 0.061754486 | 3.8397 × 10−9 | 0.061754485 | 2.9318 × 10−9 | 0.061754470 | 1.2474 × 10−8 | |
| (0.0, 0.0) | 1.9756 × 10−9 | 1.9756 × 10−9 | 1.5142 × 10−9 | 1.5142 × 10−9 | 6.3946 × 10−9 | 6.3946 × 10−9 | |
| 0.8 | (±1, ±1) | 0.988071677 | 3.5915 × 10−8 | 0.988071669 | 4.3780 × 10−8 | 0.988071347 | 3.6567 × 10−7 |
| (±0.5, ±1) (±1, ±0.5) | 0.247017892 | 3.5980 × 10−8 | 0.247017884 | 4.3843 × 10−8 | 0.247017562 | 3.6604 × 10−7 | |
| (0.0, ±1) (±1, 0.0) | 4.2459 × 10−8 | 4.2459 × 10−8 | 5.1756 × 10−8 | 5.1756 × 10−8 | 4.3149 × 10−7 | 4.3149 × 10−7 | |
| (0.0, ±0.5) (±0.5, 0.0) | 2.0041 × 10−8 | 2.0041 × 10−8 | 2.4429 × 10−8 | 2.4429 × 10−8 | 2.0367 × 10−7 | 2.0367 × 10−7 | |
| (±0.5, ±0.5) | 0.061754462 | 2.0464 × 10−8 | 0.061754457 | 2.4972 × 10−8 | 0.061754274 | 2.0837 × 10−7 | |
| (0.0, 0.0) | 1.0521 × 10−8 | 1.0521 × 10−8 | 1.2825 × 10−8 | 1.2825 × 10−8 | 1.0692 × 10−7 | 1.0692 × 10−7 | |
| α | (x, y) | q = 0.0002 | q = 0.003 | q = 0.07 | |||
|---|---|---|---|---|---|---|---|
| Num. Sol. | Error | Num. Sol. | Error | Num. Sol. | Error | ||
| 0.1 | (±1, ±1) | 0.835297081 | 2.6870 × 10−5 | 0.835289538 | 1.9327 × 10−5 | 0.835284629 | 1.4417 × 10−5 |
| (±0.5, ±1) (±1, ±0.5) | 0.208844433 | 2.6880 × 10−5 | 0.208836887 | 1.9334 × 10−5 | 0.208831976 | 1.4423 × 10−5 | |
| (0.0, ±1) (±1, 0.0) | 3.1687 × 10−5 | 3.1687 × 10−5 | 2.2791 × 10−5 | 2.2791 × 10−5 | 1.7002 × 10−5 | 1.7002 × 10−5 | |
| (0.0, ±0.5) (±0.5, 0.0) | 1.4956 × 10−5 | 1.4956 × 10−5 | 1.0758 × 10−5 | 1.0758 × 10−5 | 8.0250 × 10−6 | 8.0250 × 10−6 | |
| (±0.5, ±0.5) | 0.052219691 | 1.5303 × 10−5 | 0.052215395 | 1.1007 × 10−5 | 0.052212599 | 8.2108 × 10−5 | |
| (0.0, 0.0) | 7.8521 × 10−6 | 7.8521 × 10−6 | 5.6478 × 10−6 | 5.6478 × 10−6 | 4.2131 × 10−6 | 4.2131 × 10−6 | |
| 0.8 | (±1, ±1) | 0.835170662 | 9.9549 × 10−5 | 0.835168602 | 1.0161 × 10−4 | 0.835133616 | 1.3660 × 10−4 |
| (±0.5, ±1) (±1, ±0.5) | 0.208717965 | 9.9587 × 10−5 | 0.208715905 | 1.0165 × 10−4 | 0.208680905 | 1.3665 × 10−4 | |
| (0.0, ±1) (±1, 0.0) | 1.1739 × 10−4 | 1.1739 × 10−4 | 1.1982 × 10−4 | 1.1982 × 10−4 | 1.6107 × 10−4 | 1.6107 × 10−4 | |
| (0.0, ±0.5) (±0.5, 0.0) | 5.5406 × 10−5 | 5.5406 × 10−5 | 5.6553 × 10−5 | 5.6553 × 10−5 | 7.6023 × 10−5 | 7.6023 × 10−5 | |
| (±0.5, ±0.5) | 0.052147694 | 5.6694 × 10−5 | 0.052146521 | 5.786723E-5 | 0.052126596 | 7.7792 × 10−5 | |
| (0.0, 0.0) | 2.9088 × 10−5 | 2.9088 × 10−5 | 2.9689 × 10−5 | 2.9689 × 10−5 | 3.9911 × 10−5 | 3.9911 × 10−5 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raad, S.A.; Abdou, M.A. The Effect of Fractional Order of Time Phase Delay via a Mixed Integral Equation in (2 + 1) Dimensions with an Extended Discontinuous Kernel. Symmetry 2025, 17, 36. https://doi.org/10.3390/sym17010036
Raad SA, Abdou MA. The Effect of Fractional Order of Time Phase Delay via a Mixed Integral Equation in (2 + 1) Dimensions with an Extended Discontinuous Kernel. Symmetry. 2025; 17(1):36. https://doi.org/10.3390/sym17010036
Chicago/Turabian StyleRaad, Sameeha A., and Mohammed A. Abdou. 2025. "The Effect of Fractional Order of Time Phase Delay via a Mixed Integral Equation in (2 + 1) Dimensions with an Extended Discontinuous Kernel" Symmetry 17, no. 1: 36. https://doi.org/10.3390/sym17010036
APA StyleRaad, S. A., & Abdou, M. A. (2025). The Effect of Fractional Order of Time Phase Delay via a Mixed Integral Equation in (2 + 1) Dimensions with an Extended Discontinuous Kernel. Symmetry, 17(1), 36. https://doi.org/10.3390/sym17010036







