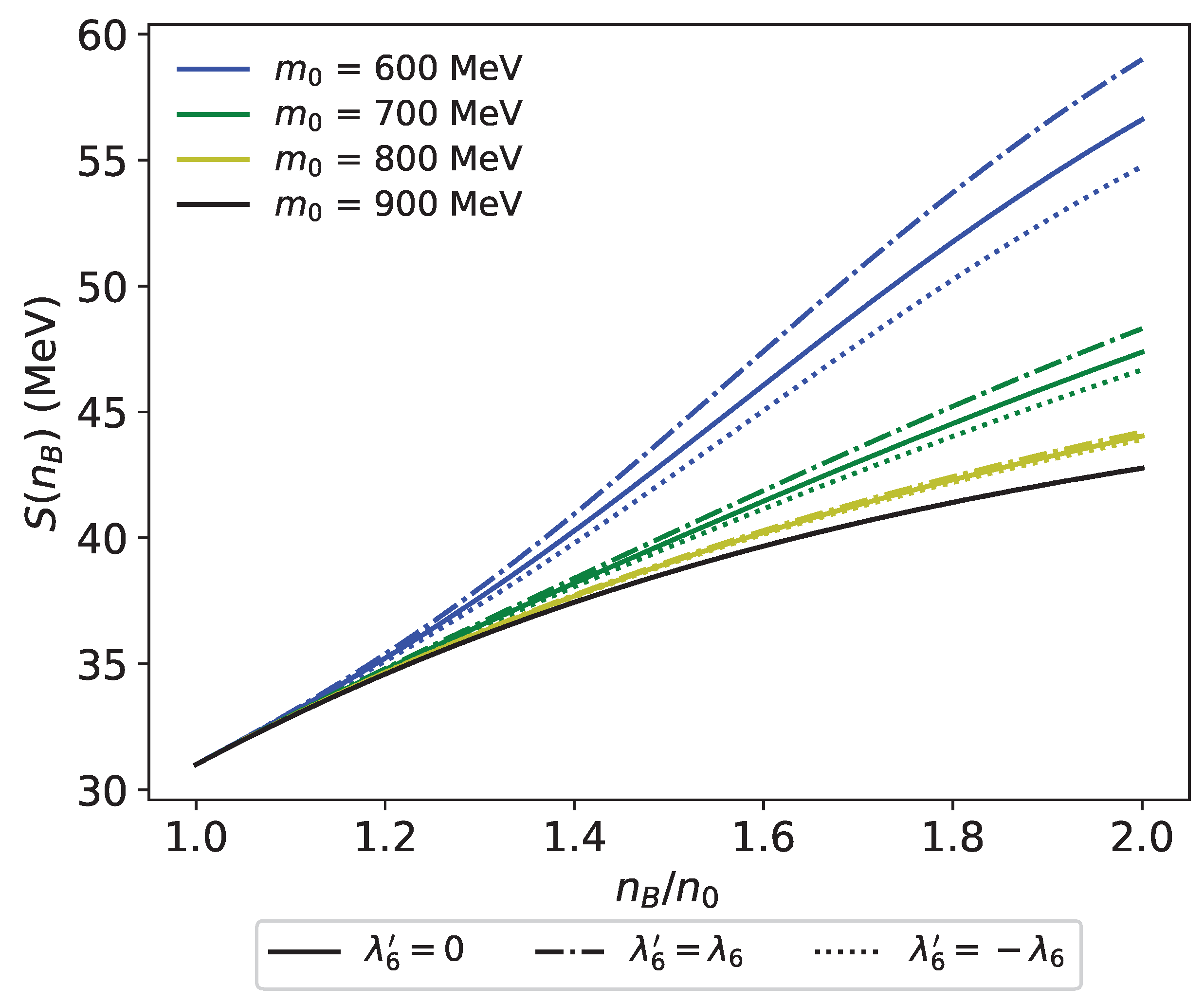Figure 1.
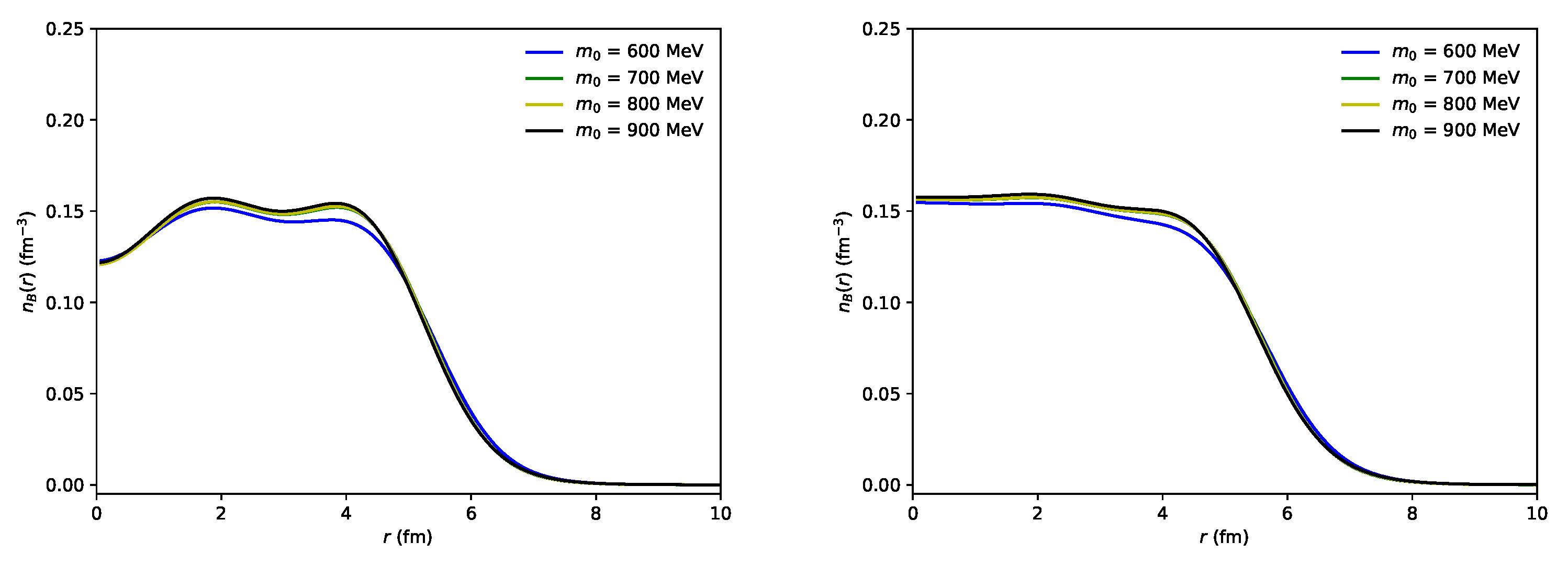
Nucleon density profile in 112Sn (left) and 126Sn (right) calculated with MeV.
Figure 1.
Nucleon density profile in 112Sn (left) and 126Sn (right) calculated with MeV.
Figure 2.
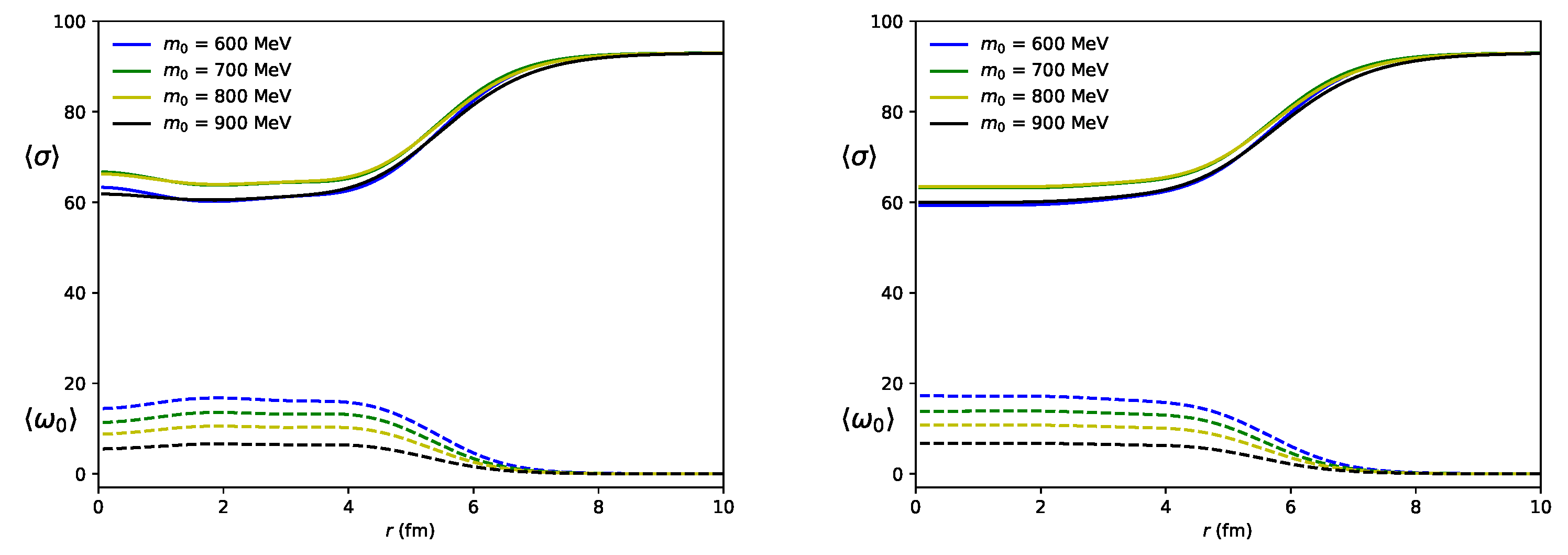
and in 112Sn (left) and 126Sn (right) with MeV.
Figure 2.
and in 112Sn (left) and 126Sn (right) with MeV.
Figure 3.
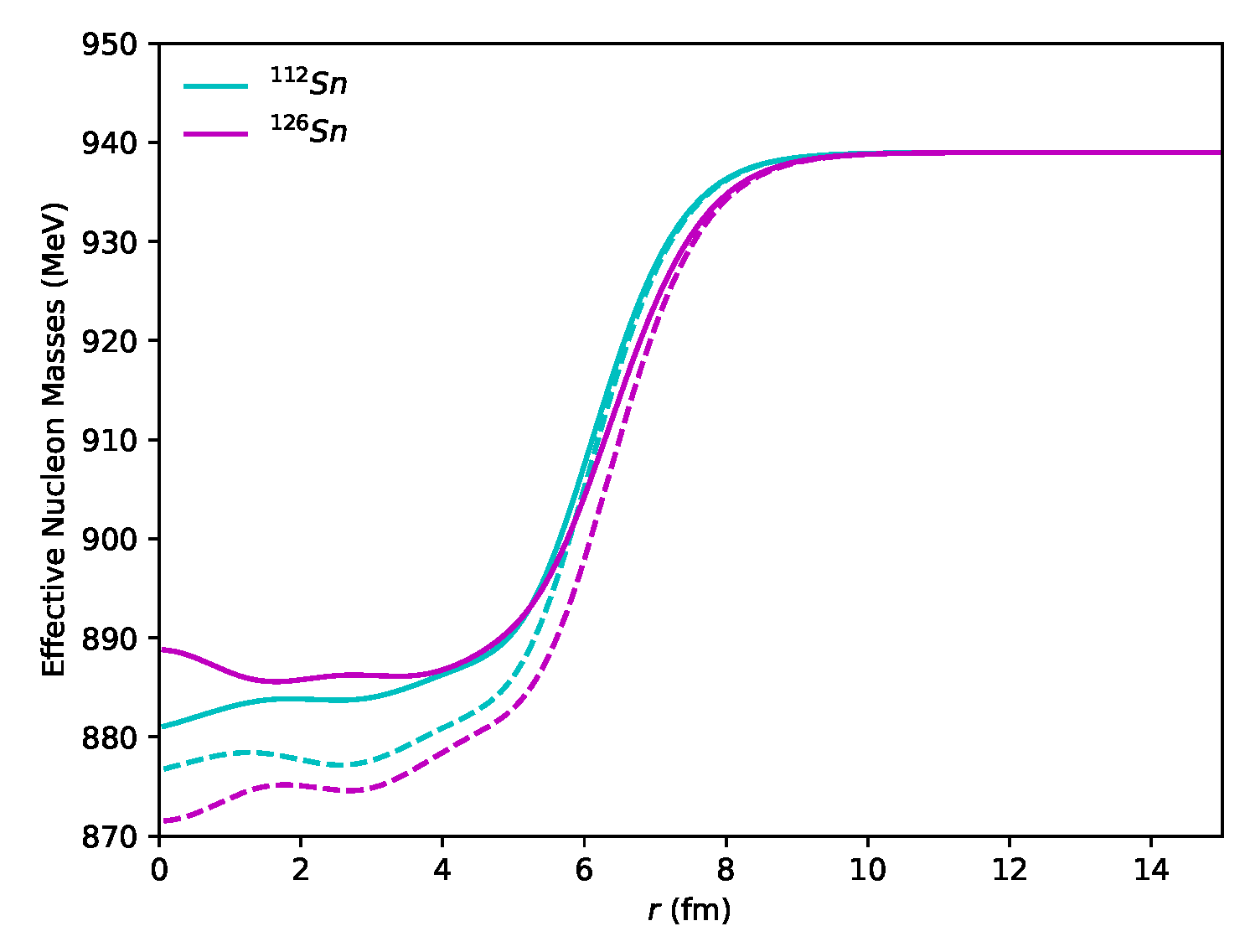
Neutron and proton effective masses in 112Sn and 126Sn with MeV. Here, solid (dashed) lines are for the neutron (proton).
Figure 3.
Neutron and proton effective masses in 112Sn and 126Sn with MeV. Here, solid (dashed) lines are for the neutron (proton).
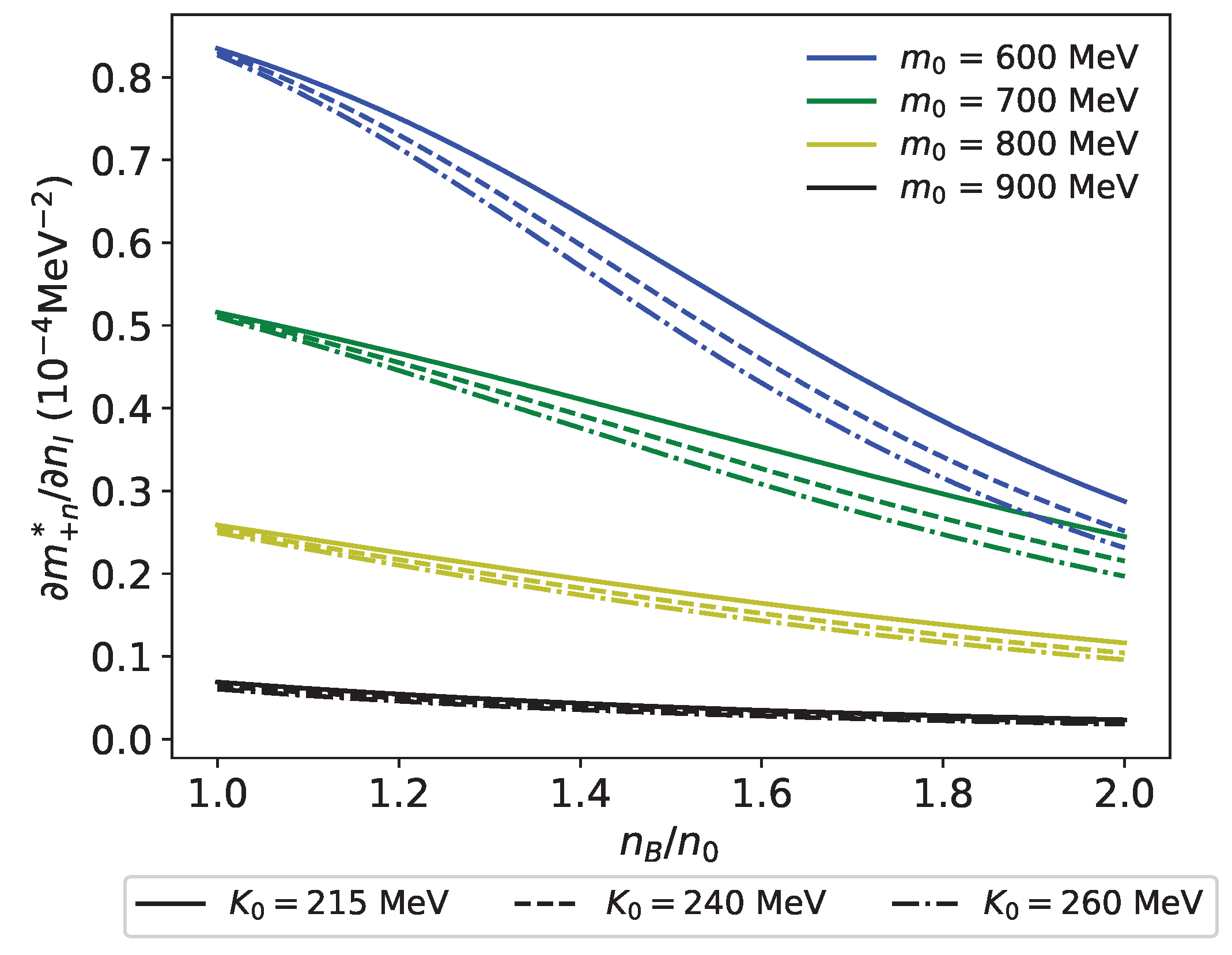
Figure 4.
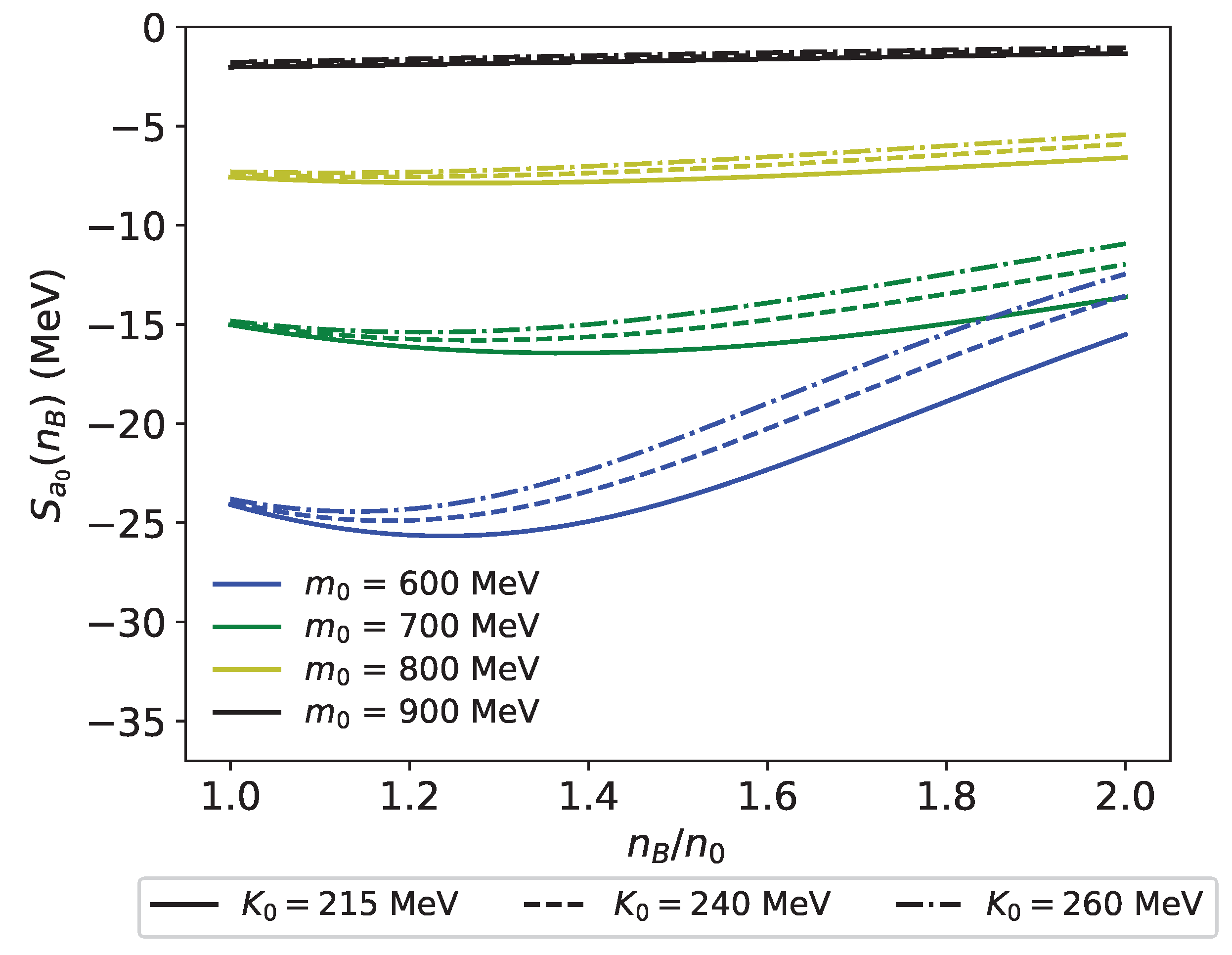
Nucleon contribution for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 4.
Nucleon contribution for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 5.
meson contribution to the symmetry energy for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 5.
meson contribution to the symmetry energy for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 6.
for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 6.
for –900 MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 7.
meson contribution in model for –900 MeV and . Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 7.
meson contribution in model for –900 MeV and . Solid, dashed, and dash-dot curves represent with MeV, respectively.
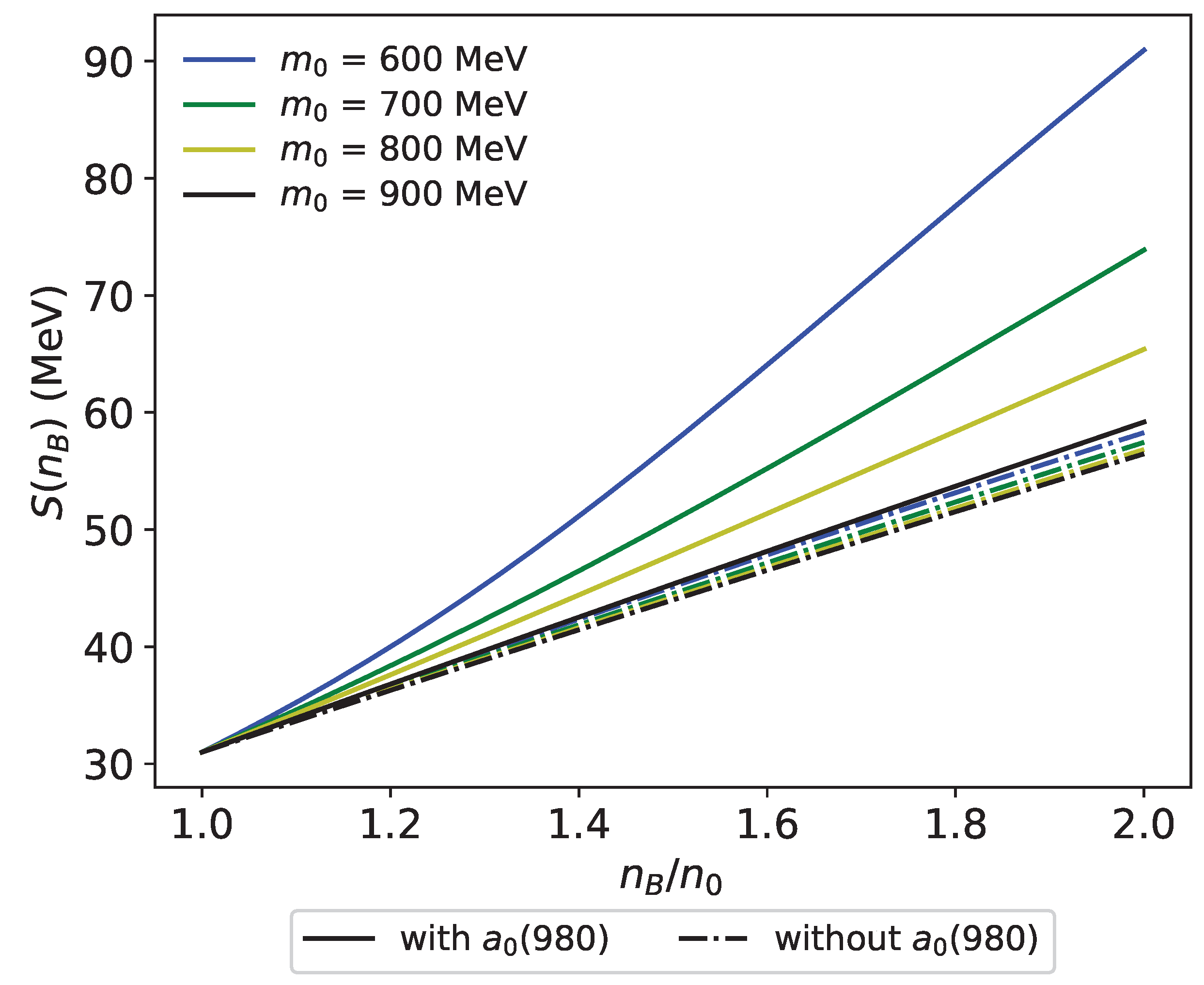
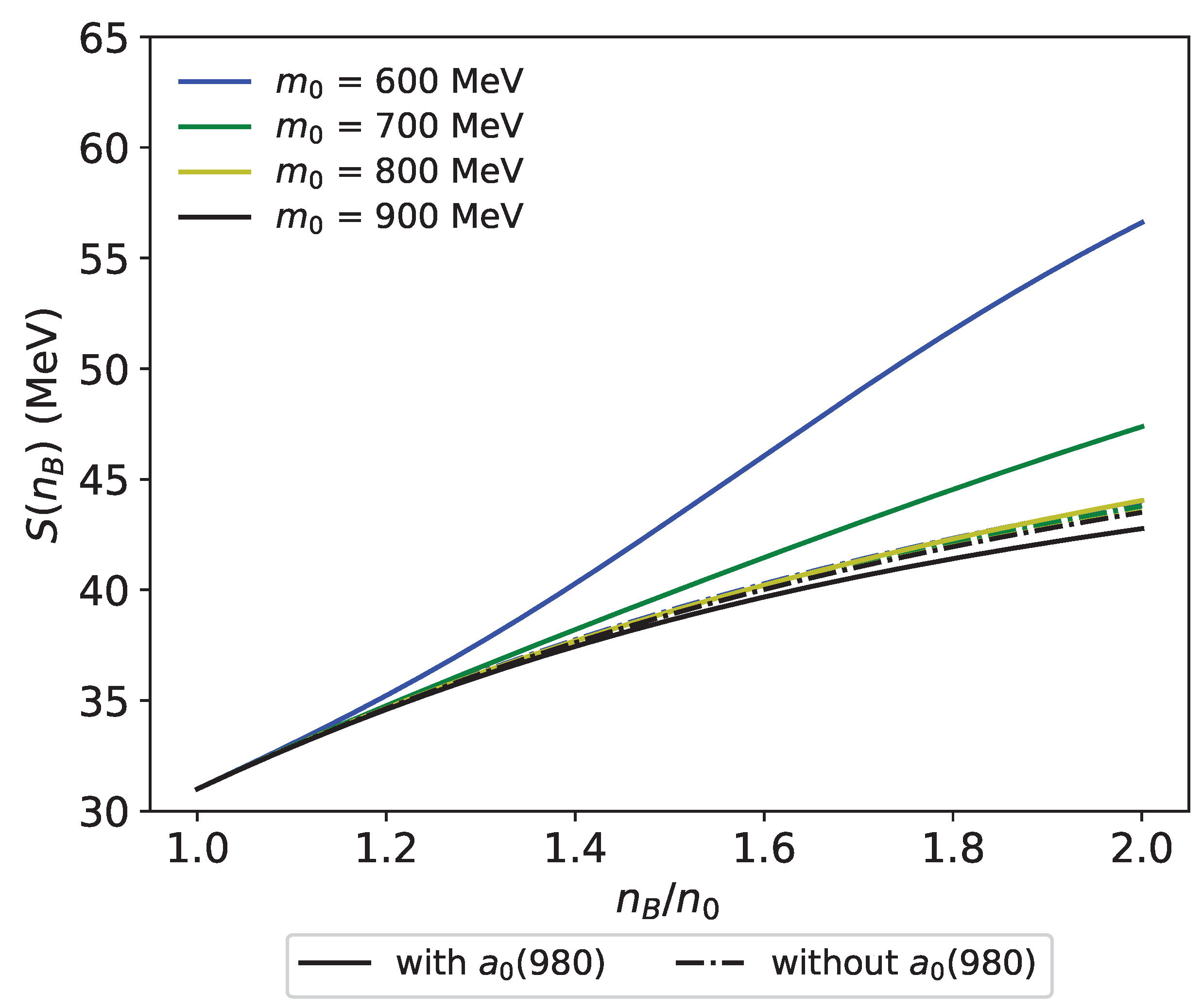
Figure 8.
Symmetry energy for –900 MeV, MeV, and . Solid curves represent the of the model, including with = 0, while the dash-dot curves show the results of the model without .
Figure 8.
Symmetry energy for –900 MeV, MeV, and . Solid curves represent the of the model, including with = 0, while the dash-dot curves show the results of the model without .
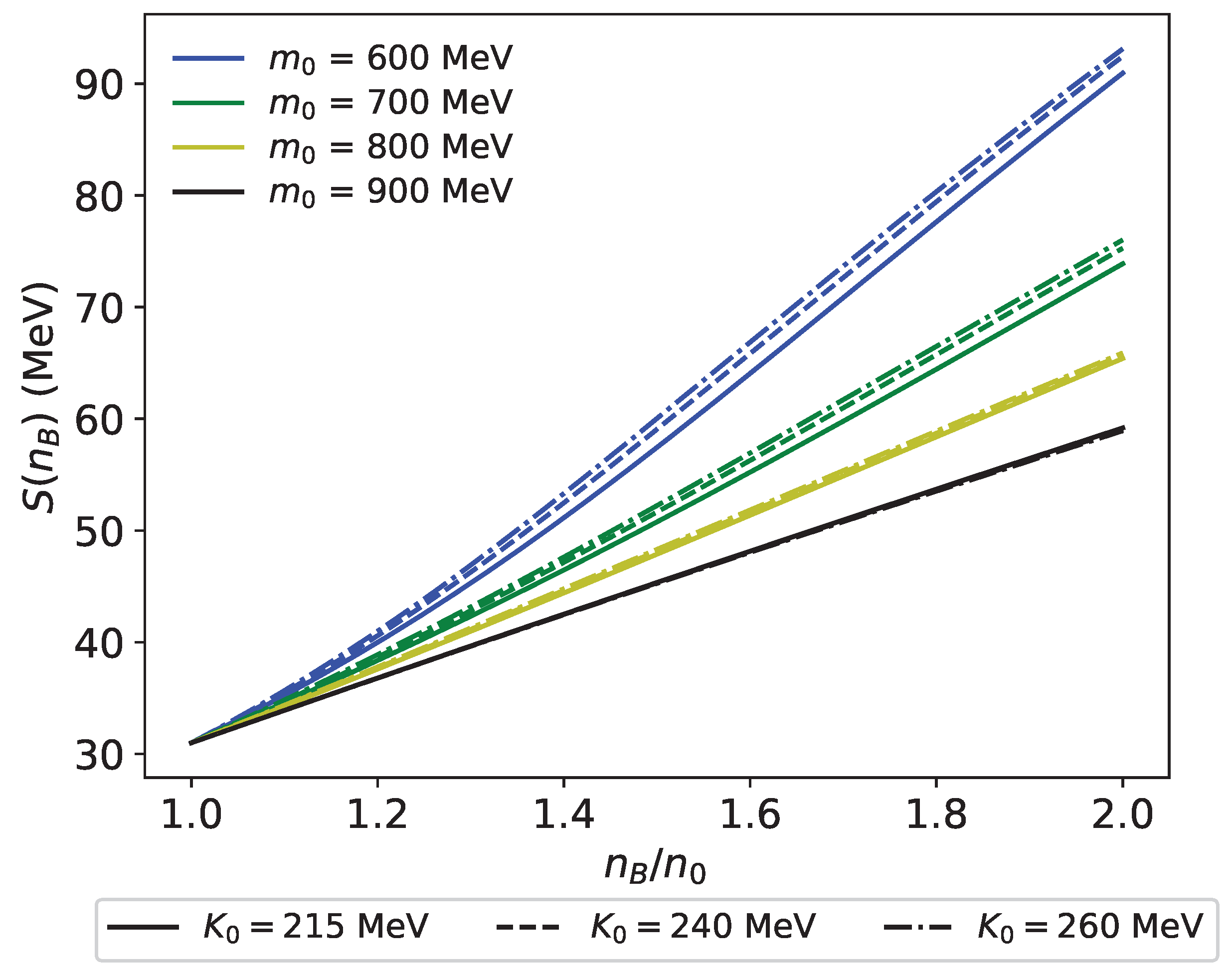
Figure 9.
Symmetry energy for –900 MeV, , with different choices of compared. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 9.
Symmetry energy for –900 MeV, , with different choices of compared. Solid, dashed, and dash-dot curves represent with MeV, respectively.
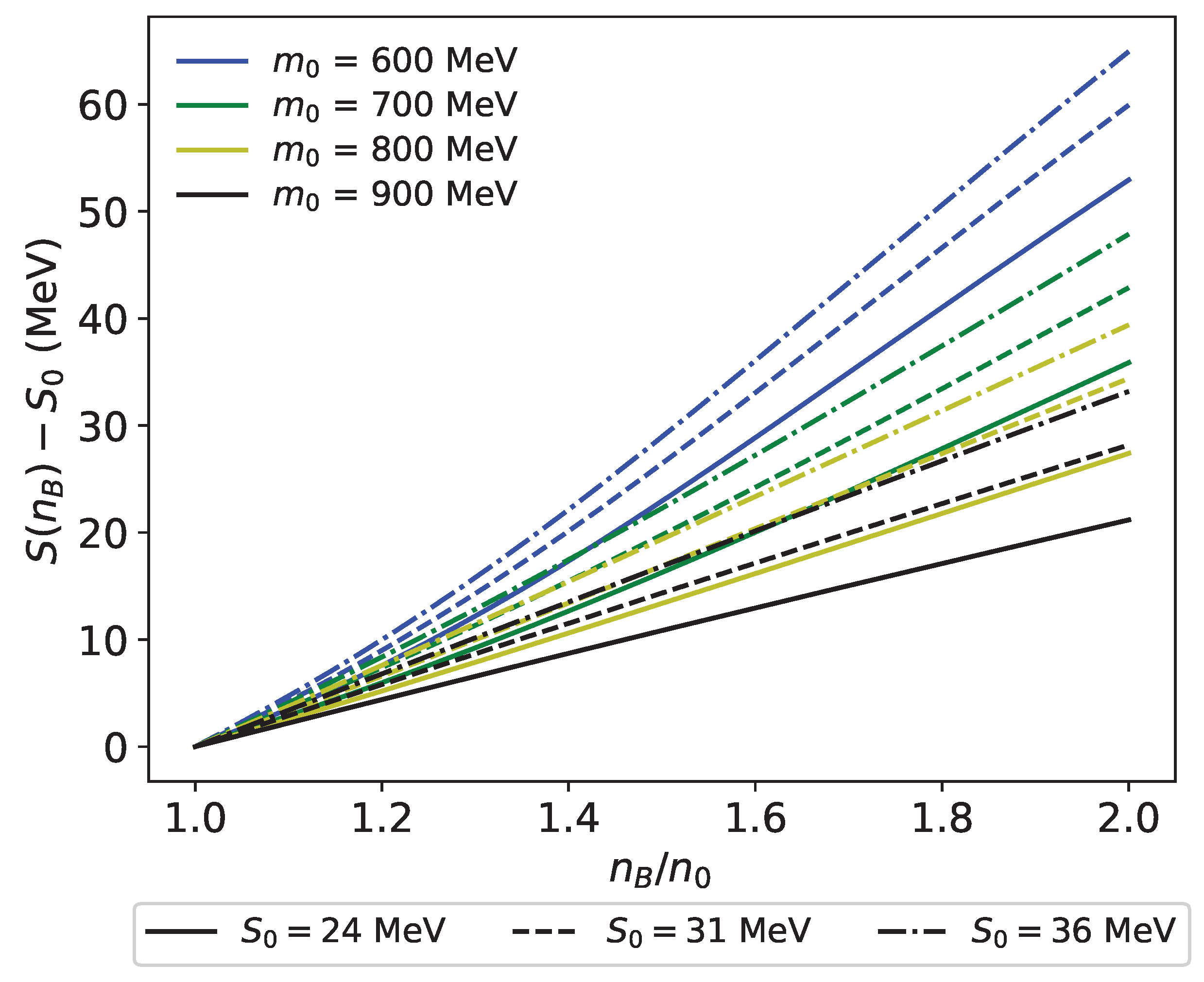
Figure 10.
Difference of the symmetry energy in model for –900 MeV and . MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 10.
Difference of the symmetry energy in model for –900 MeV and . MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
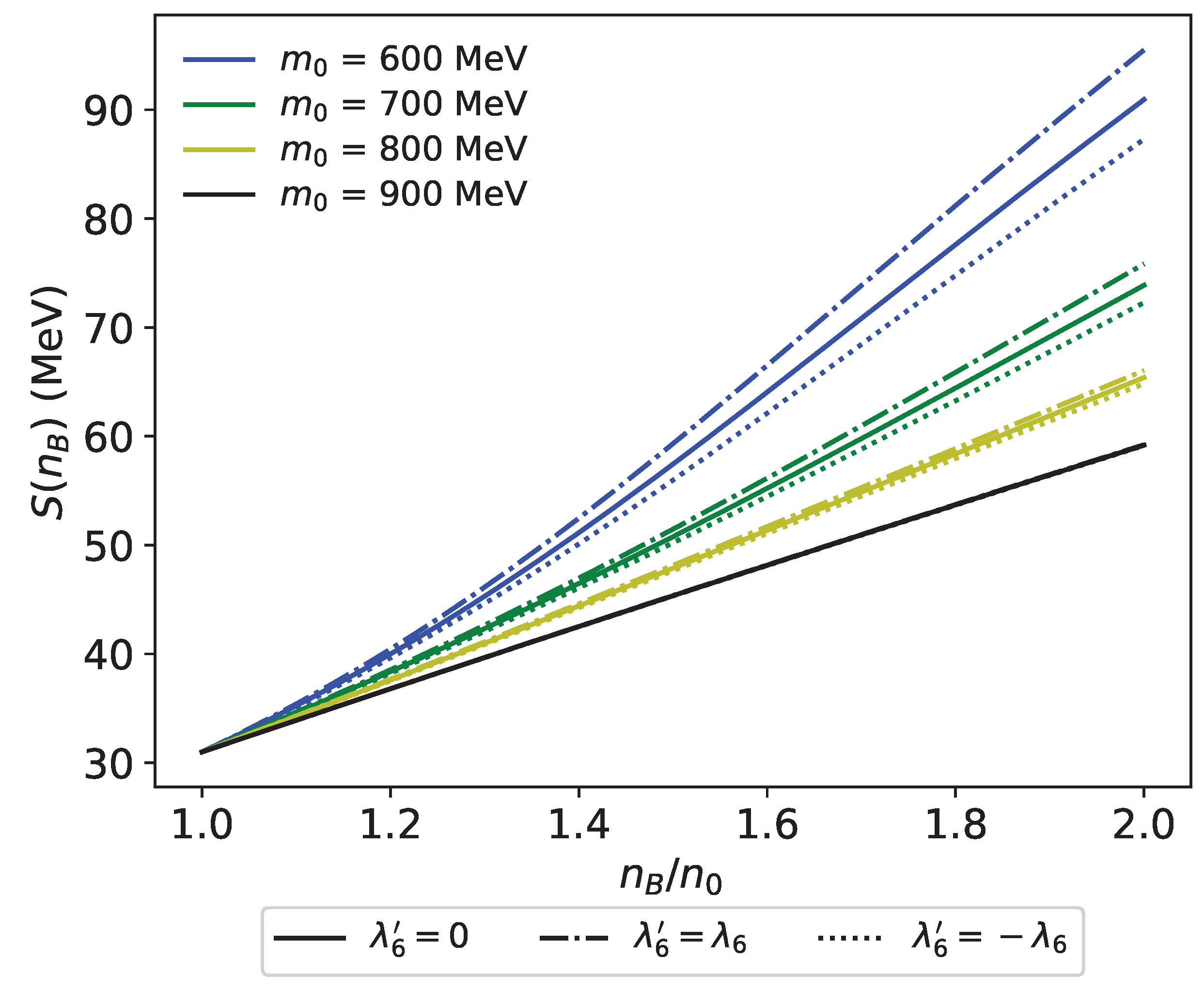
Figure 11.
Symmetry energy for –900 MeV and MeV, with the effect of compared. Solid, dash-dot, and dotted curves show the with , and , respectively.
Figure 11.
Symmetry energy for –900 MeV and MeV, with the effect of compared. Solid, dash-dot, and dotted curves show the with , and , respectively.
Figure 12.
meson contribution in model for –900 MeV, , and MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 12.
meson contribution in model for –900 MeV, , and MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
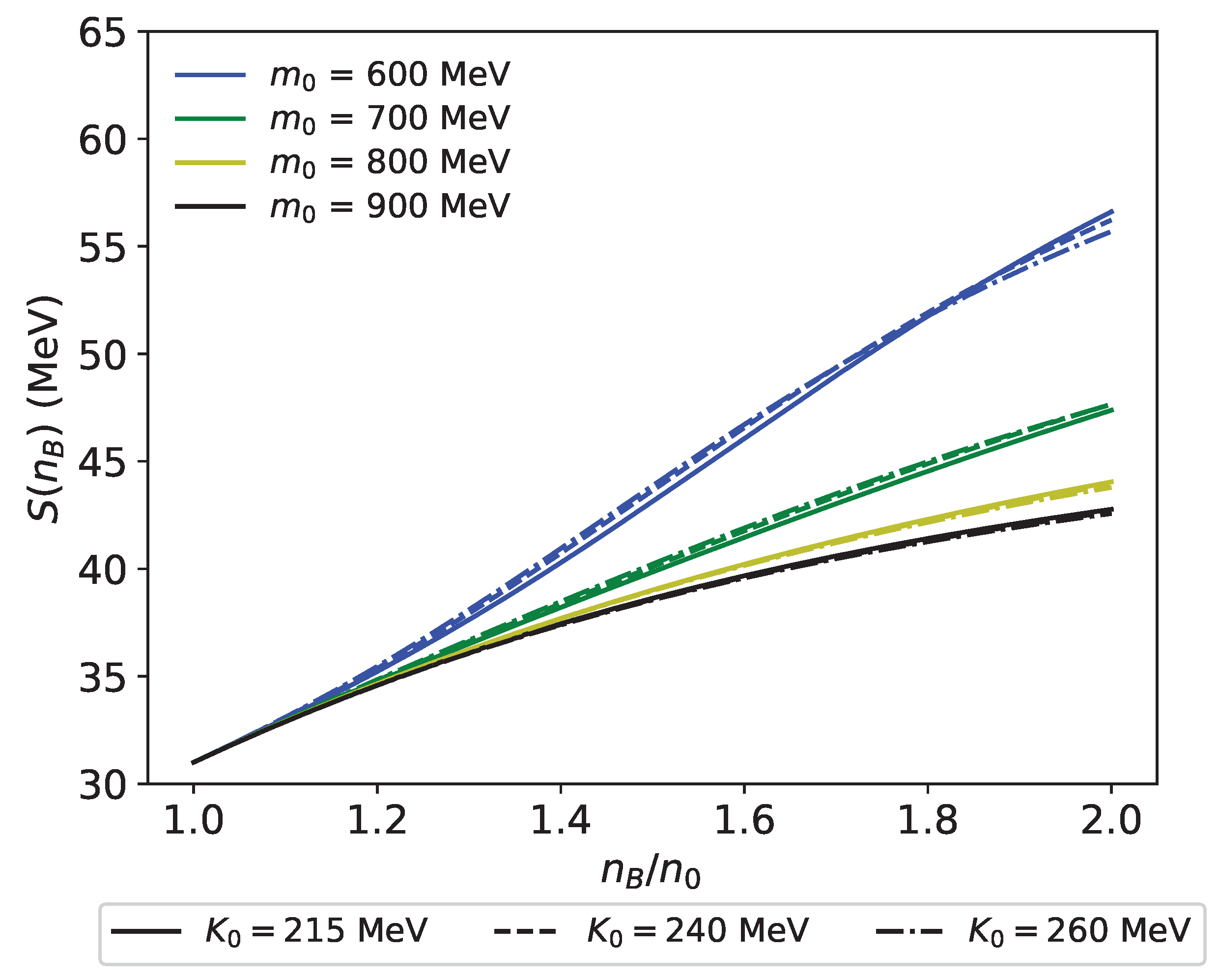
Figure 13.
Symmetry energy in model with vector meson mixing for –900 MeV, , MeV, and MeV. Solid curves represent the of the model, including with = 0, while the dash-dot curves show the results of the model without .
Figure 13.
Symmetry energy in model with vector meson mixing for –900 MeV, , MeV, and MeV. Solid curves represent the of the model, including with = 0, while the dash-dot curves show the results of the model without .
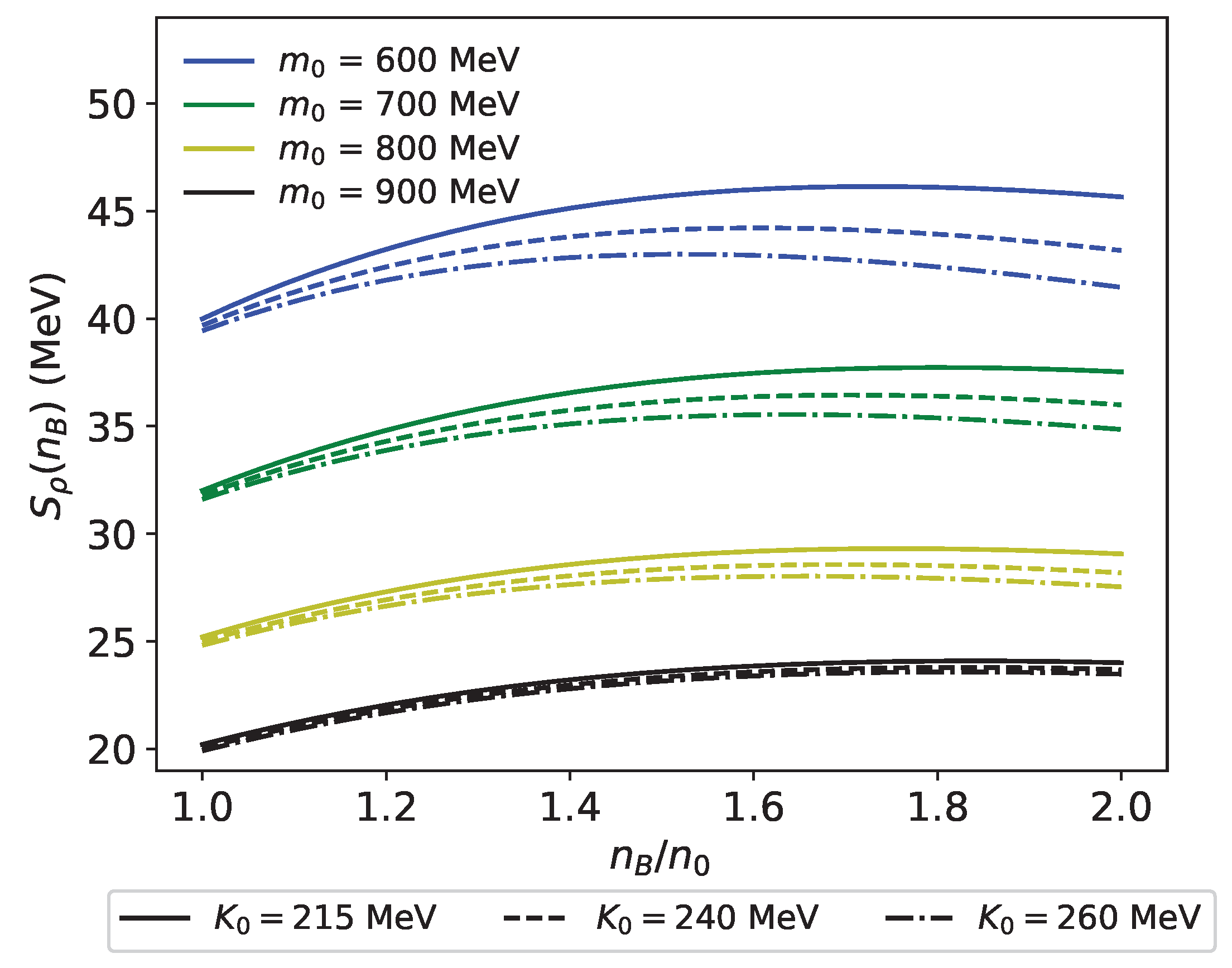
Figure 14.
dependence of the symmetry energy in the model with vector meson mixing for , and MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
Figure 14.
dependence of the symmetry energy in the model with vector meson mixing for , and MeV. Solid, dashed, and dash-dot curves represent with MeV, respectively.
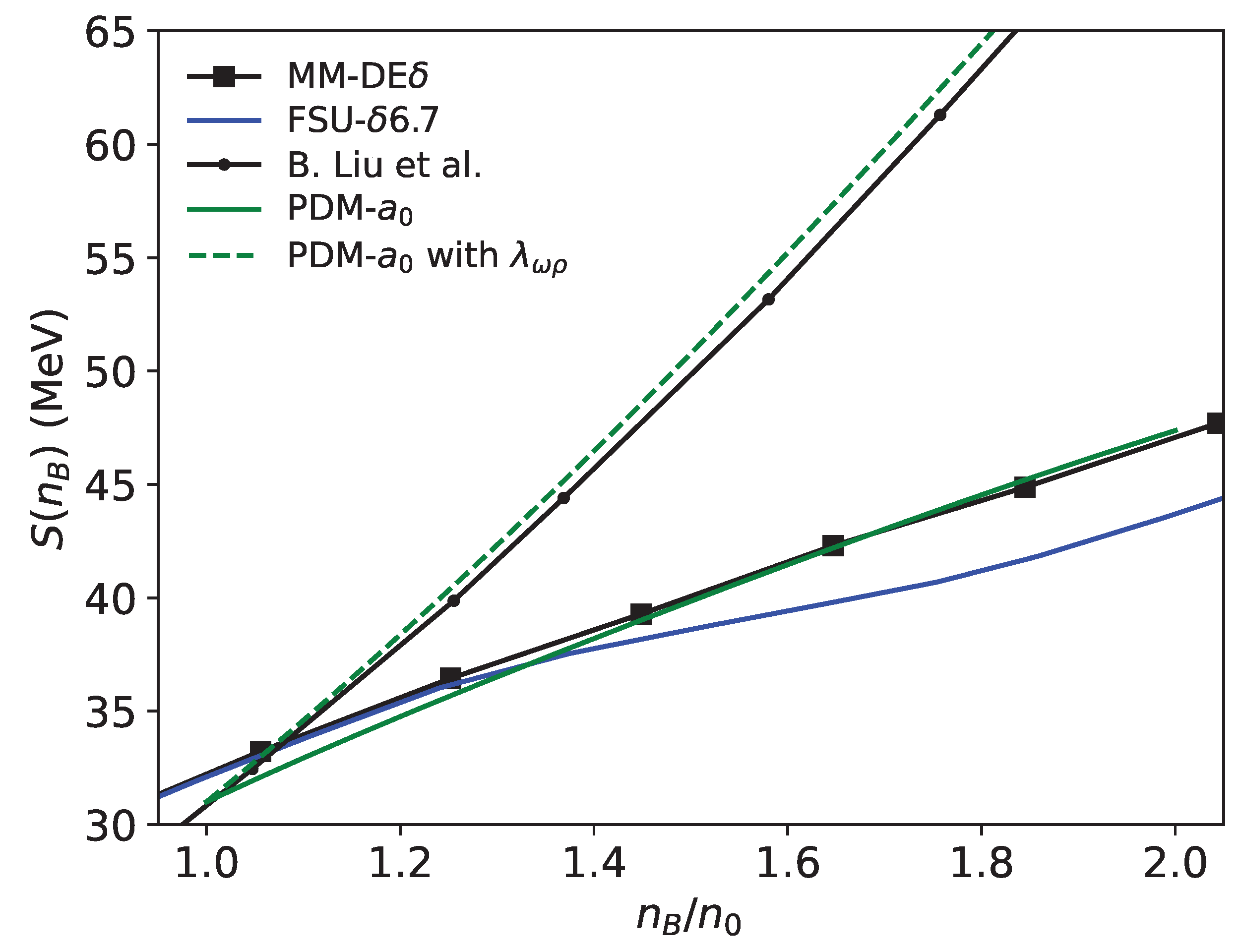
Figure 15.
Comparison of the symmetry energy
of the present model with the ones of other models. The results from density dependent RMF model DD-ME
[
65] and RMF model from B. Liu et al. [
63] and FSU-
6.7 [
56] are compared. The results from present model shown in the figure takes the typical value of
MeV,
,
MeV, and
MeV for result with vector meson mixing.
Figure 15.
Comparison of the symmetry energy
of the present model with the ones of other models. The results from density dependent RMF model DD-ME
[
65] and RMF model from B. Liu et al. [
63] and FSU-
6.7 [
56] are compared. The results from present model shown in the figure takes the typical value of
MeV,
,
MeV, and
MeV for result with vector meson mixing.
Figure 16.
Symmetry energy for –900 MeV, MeV, and MeV with the effect of compared. Solid, dash-dot, and dotted curves show the with .
Figure 16.
Symmetry energy for –900 MeV, MeV, and MeV with the effect of compared. Solid, dash-dot, and dotted curves show the with .
Table 1.
Saturation properties that are used to determine the model parameters: saturation density , binding energy , incompressibility , and symmetry energy .
Table 1.
Saturation properties that are used to determine the model parameters: saturation density , binding energy , incompressibility , and symmetry energy .
| [fm−3] | [MeV] | [MeV] | [MeV] |
|---|
| 0.16 | 16 | 215, 240 | 31 |
Table 2.
Values of meson masses and pion decay constant in the vacuum in unit of MeV.
Table 2.
Values of meson masses and pion decay constant in the vacuum in unit of MeV.
| | | |
|---|
| 140 | 783 | 776 | 93 |
Table 3.
Values of , , for = 600–900 MeV, MeV.
Table 3.
Values of , , for = 600–900 MeV, MeV.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | | 8.427 | 7.762 | 6.941 | 5.921 |
| 14.836 | 14.171 | 13.349 | 12.329 |
| 23.377 | 20.979 | 13.346 | 2.502 |
| 42.368 | 38.92 | 26.128 | 6.673 |
| 16.79 | 15.739 | 10.58 | 1.969 |
| 8.902 | 7.055 | 5.471 | 3.389 |
| 7.896 | 8.16 | 8.314 | 8.442 |
| MeV | | 8.427 | 7.762 | 6.941 | 5.921 |
| 14.836 | 14.171 | 13.349 | 12.329 |
| 21.821 | 18.842 | 11.692 | 1.537 |
| 39.367 | 34.583 | 22.577 | 4.388 |
| 15.344 | 13.54 | 8.683 | 0.649 |
| 9.132 | 7.305 | 5.66 | 3.522 |
| 7.854 | 8.13 | 8.298 | 8.436 |
Table 4.
Slope parameter computed as a output from the model with MeV. MeV.
Table 4.
Slope parameter computed as a output from the model with MeV. MeV.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | [MeV] | 85.91 | 82.87 | 81.32 | 80.15 |
| MeV | [MeV] | 86.25 | 83.04 | 81.33 | 80.08 |
| MeV | [MeV] | 86.45 | 83.14 | 81.33 | 80.03 |
Table 5.
Slope parameter with MeV. MeV.
Table 5.
Slope parameter with MeV. MeV.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | [MeV] | 64.91 | 61.87 | 60.32 | 59.15 |
| MeV | [MeV] | 85.91 | 82.87 | 81.32 | 80.15 |
| MeV | [MeV] | 100.91 | 97.87 | 96.32 | 95.15 |
Table 6.
Binding energy per nucleon and the charge radius (
) with the parameter set in
Table 3 for
MeV. The table is taken from Ref. [
67].
Table 6.
Binding energy per nucleon and the charge radius (
) with the parameter set in
Table 3 for
MeV. The table is taken from Ref. [
67].
| (MeV) | | 600 | 700 | 800 | 900 | Exp. |
|---|
| BE/A (MeV) | 16O | | | | | |
| 40Ca | | | | | |
| 48Ca | | | | | |
| 58Ni | | | | | |
| 70Ge | | | | | |
| 82Se | | | | | |
| 92Mo | | | | | |
| 112Sn | | | | | |
| 126Sn | | | | | |
| 138Ba | | | | | |
| 154Sm | | | | | |
| 170Er | | | | | |
| 182W | | | | | |
| 202Pb | | | | | |
| 208Pb | | | | | |
| RMS deviation | | | | | | − |
| (fm) | 16O | | | | | |
| 40Ca | | | | | |
| 48Ca | | | | | |
| 58Ni | | | | | |
| 70Ge | | | | | |
| 82Se | | | | | |
| 92Mo | | | | | |
| 112Sn | | | | | |
| 126Sn | | | | | |
| 138Ba | | | | | |
| 154Sm | | | | | |
| 170Er | | | | | |
| 182W | | | | | |
| 202Pb | | | | | |
| 208Pb | | | | | |
| RMS deviation | | | | | | − |
Table 7.
Binding energy per nucleon and the charge radius (
) with the parameter set in
Table 3 for
MeV. The table is taken from Ref. [
67].
Table 7.
Binding energy per nucleon and the charge radius (
) with the parameter set in
Table 3 for
MeV. The table is taken from Ref. [
67].
| (MeV) | | 600 | 700 | 800 | 900 | Exp. |
|---|
| BE/A (MeV) | 16O | | | | | |
| 40Ca | | | | | |
| 48Ca | | | | | |
| 58Ni | | | | | |
| 70Ge | | | | | |
| 82Se | | | | | |
| 92Mo | | | | | |
| 112Sn | | | | | |
| 126Sn | | | | | |
| 138Ba | | | | | |
| 154Sm | | | | | |
| 170Er | | | | | |
| 182W | | | | | |
| 202Pb | | | | | |
| 208Pb | | | | | |
| RMS deviation | | | | | | − |
| (fm) | 16O | | | | | |
| 40Ca | | | | | |
| 48Ca | | | | | |
| 58Ni | | | | | |
| 70Ge | | | | | |
| 82Se | | | | | |
| 92Mo | | | | | |
| 112Sn | | | | | |
| 126Sn | | | | | |
| 138Ba | | | | | |
| 154Sm | | | | | |
| 170Er | | | | | |
| 182W | | | | | |
| 202Pb | | | | | |
| 208Pb | | | | | |
| RMS deviation | | | | | | − |
Table 8.
Parameter set with the inputs in Equation (
70). All the parameters are dimensionless except
.
Table 8.
Parameter set with the inputs in Equation (
70). All the parameters are dimensionless except
.
| | | | | | | [MeV] |
|---|
| | | | | | | |
Table 9.
Binding energy per nucleon and the charge radius (
) with the parameters in
Table 8.
Table 9.
Binding energy per nucleon and the charge radius (
) with the parameters in
Table 8.
| | BE/A [MeV] | [fm] |
|---|
| | PDM | RCHB | Exp. | PDM | RCHB | Exp. |
| 16O | | | | | | |
| 40Ca | | | | | | |
| 48Ca | | | | | | |
| 58Ni | | | | | | |
| 70Ge | | | | | | |
| 82Se | | | | | | |
| 92Mo | | | | | | |
| 112Sn | | | | | | |
| 126Sn | | | | | | |
| 138Ba | | | | | | |
| 154Sm | | | | | | |
| 170Er | | | | | | |
| 182W | | | | | | |
| 202Pb | | | | | | |
| 208Pb | | | | | | |
| RMS deviation | | | − | | | − |
Table 10.
Values of masses of and mesons in unit of MeV.
Table 10.
Values of masses of and mesons in unit of MeV.
Table 11.
Values of parameters and for several choices of and with .
Table 11.
Values of parameters and for several choices of and with .
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | | −9.40 | −11.79 | −19.43 | −30.27 |
| 185.59 | 177.94 | 144.51 | 90.62 |
| MeV | | −10.95 | −13.93 | −21.08 | −31.24 |
| 176.81 | 164.81 | 133.38 | 83.05 |
Table 12.
The values of and slope parameter in the model without vector meson mixing, for several choices of and with . MeV.
Table 12.
The values of and slope parameter in the model without vector meson mixing, for several choices of and with . MeV.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | | 12.52 | 11.20 | 9.94 | 8.90 |
| [MeV] | 120.14 | 105.21 | 97.05 | 87.65 |
| MeV | | 12.47 | 11.16 | 9.90 | 8.86 |
| [MeV] | 126.58 | 108.78 | 98.67 | 87.75 |
| MeV | | 12.43 | 11.13 | 9.86 | 8.83 |
| [MeV] | 131.19 | 111.45 | 99.86 | 87.75 |
Table 13.
Values of and slope parameter in the model without vector meson mixing, for several choices of and with . MeV.
Table 13.
Values of and slope parameter in the model without vector meson mixing, for several choices of and with . MeV.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | | 11.37 | 9.9 | 8.45 | 7.2 |
| [MeV] | 99.14 | 84.21 | 76.05 | 66.65 |
| MeV | | 12.52 | 11.2 | 9.94 | 8.9 |
| [MeV] | 120.14 | 105.21 | 97.05 | 87.65 |
| MeV | | 13.28 | 12.04 | 10.88 | 9.94 |
| [MeV] | 135.14 | 120.21 | 112.05 | 102.65 |
Table 14.
Values of for several choices of , , with = 0. Vector meson mixing is considered.
Table 14.
Values of for several choices of , , with = 0. Vector meson mixing is considered.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | MeV | 15.34 | 13.78 | 12.59 | 11.42 |
| MeV | 14.88 | 13.27 | 11.97 | 10.72 |
| MeV | 14.46 | 12.81 | 11.44 | 10.13 |
| MeV | 14.08 | 12.39 | 10.97 | 9.63 |
| MeV | 13.72 | 12.02 | 10.55 | 9.19 |
| MeV | MeV | 15.63 | 13.96 | 12.68 | 11.41 |
| MeV | 15.14 | 13.42 | 12.04 | 10.7 |
| MeV | 14.69 | 12.94 | 11.49 | 10.11 |
| MeV | 14.28 | 12.51 | 11.0 | 9.6 |
| MeV | 13.9 | 12.11 | 10.58 | 9.16 |
Table 15.
Values of for several choices of , , with = 0. Vector meson mixing is considered.
Table 15.
Values of for several choices of , , with = 0. Vector meson mixing is considered.
| | [MeV] | 600 | 700 | 800 | 900 |
|---|
| MeV | MeV | 0.0254 | 0.0818 | 0.3191 | 2.8164 |
| MeV | 0.0222 | 0.0693 | 0.2632 | 2.2253 |
| MeV | 0.0191 | 0.0567 | 0.2072 | 1.6342 |
| MeV | 0.0159 | 0.0442 | 0.1513 | 1.0431 |
| MeV | 0.0127 | 0.0316 | 0.0954 | 0.4519 |
| MeV | MeV | 0.0252 | 0.0761 | 0.2914 | 2.4593 |
| MeV | 0.0223 | 0.065 | 0.2418 | 1.9443 |
| MeV | 0.0194 | 0.054 | 0.1921 | 1.4293 |
| MeV | 0.0165 | 0.0429 | 0.1424 | 0.9142 |
| MeV | 0.0135 | 0.0318 | 0.0927 | 0.3992 |