Analytic Theory of Seven Classes of Fractional Vibrations Based on Elementary Functions: A Tutorial Review
Abstract
1. Introduction
1.1. Class I Fractional Vibration
1.2. Class II Fractional Vibration
1.3. Class III Fractional Vibration
1.4. Class IV Fractional Vibration
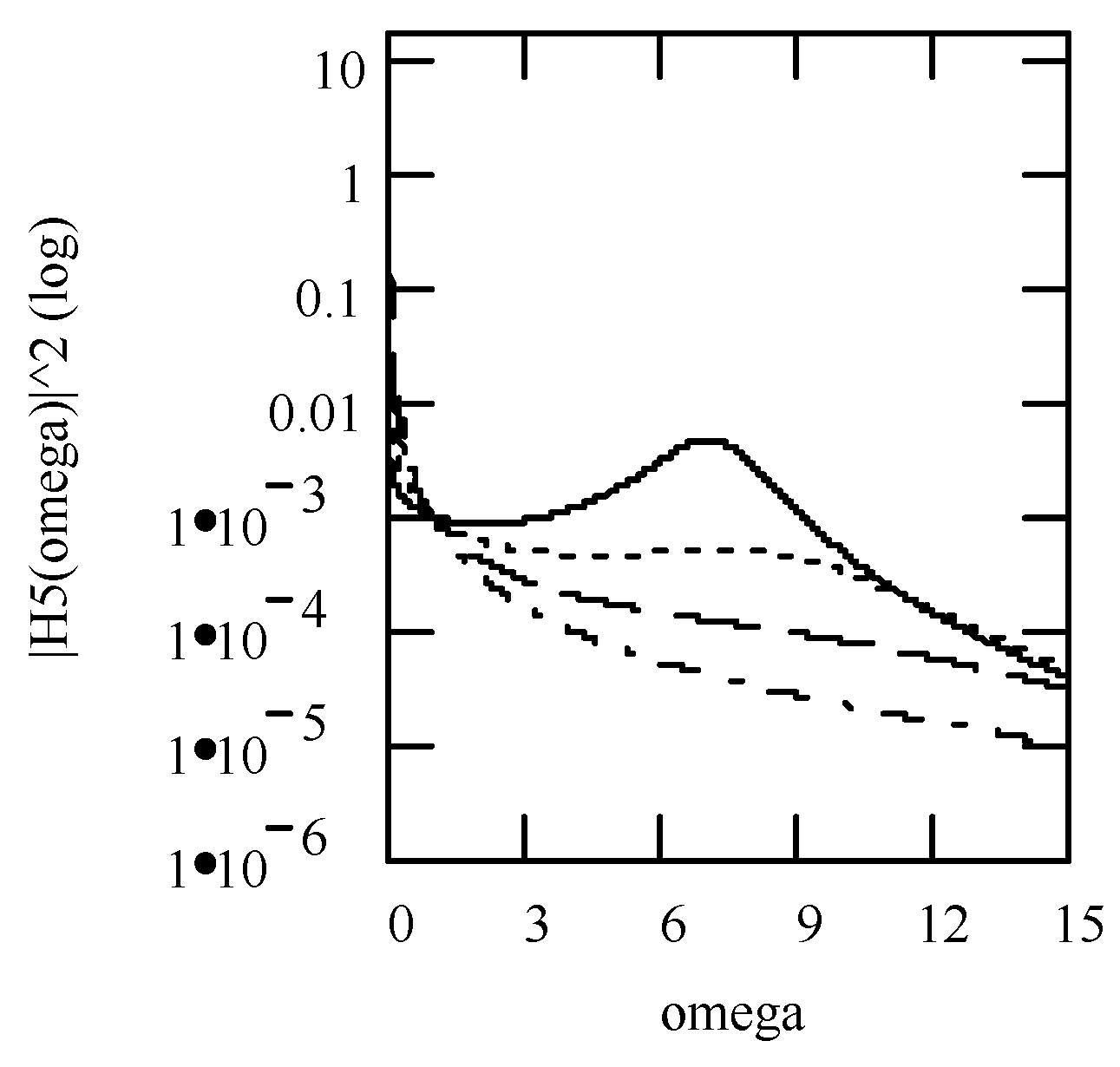
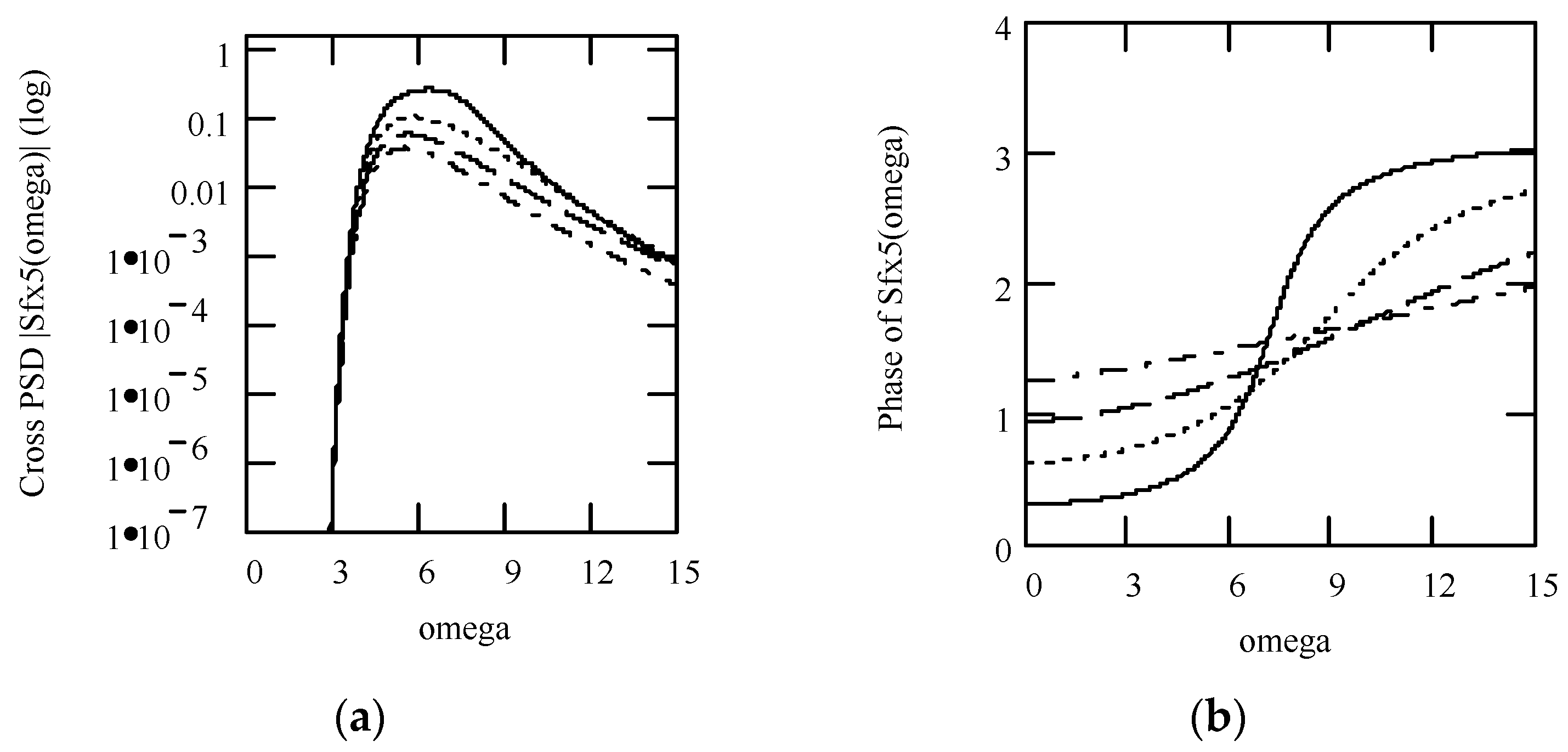
1.5. Class V Fractional Vibration
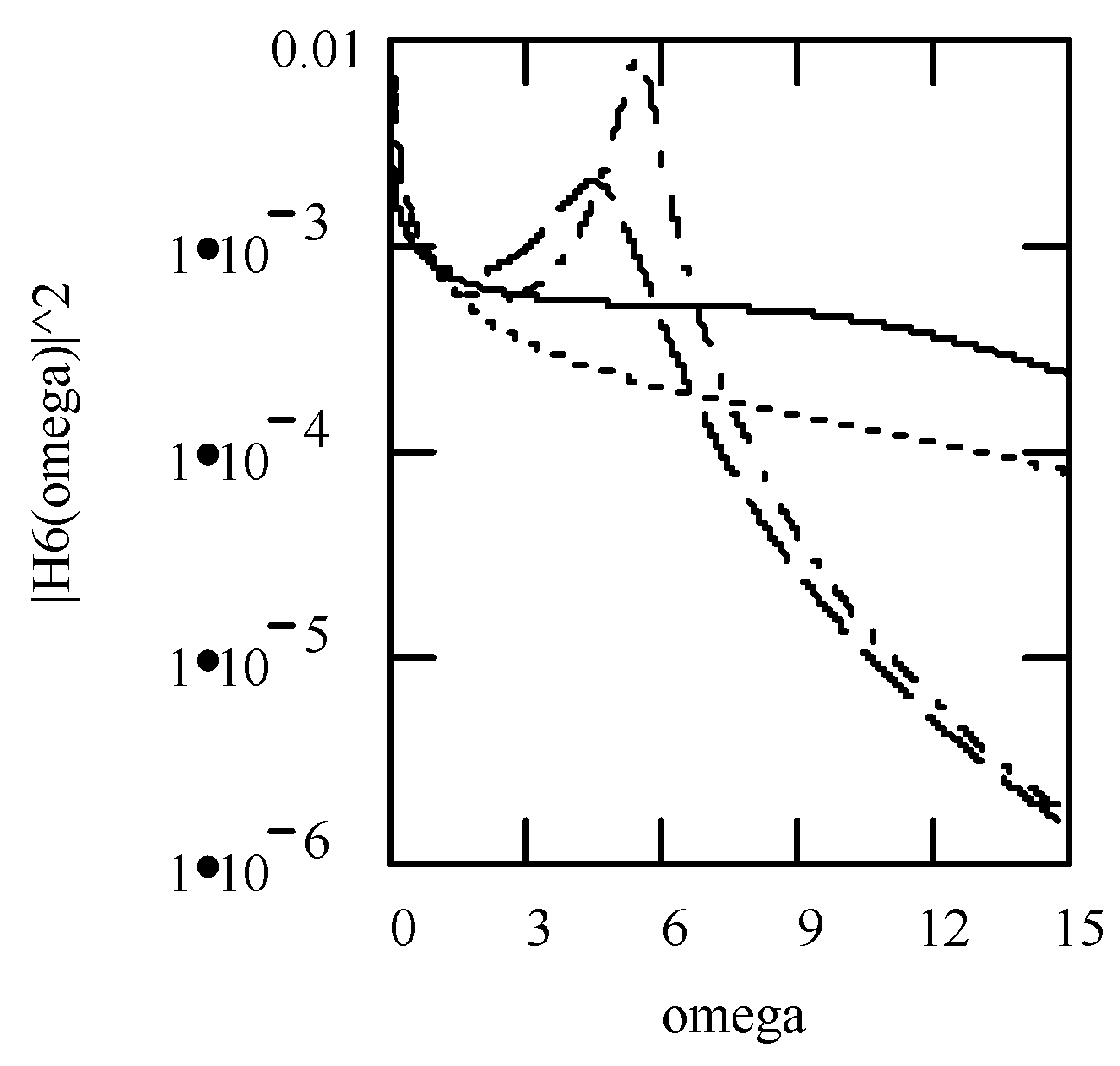
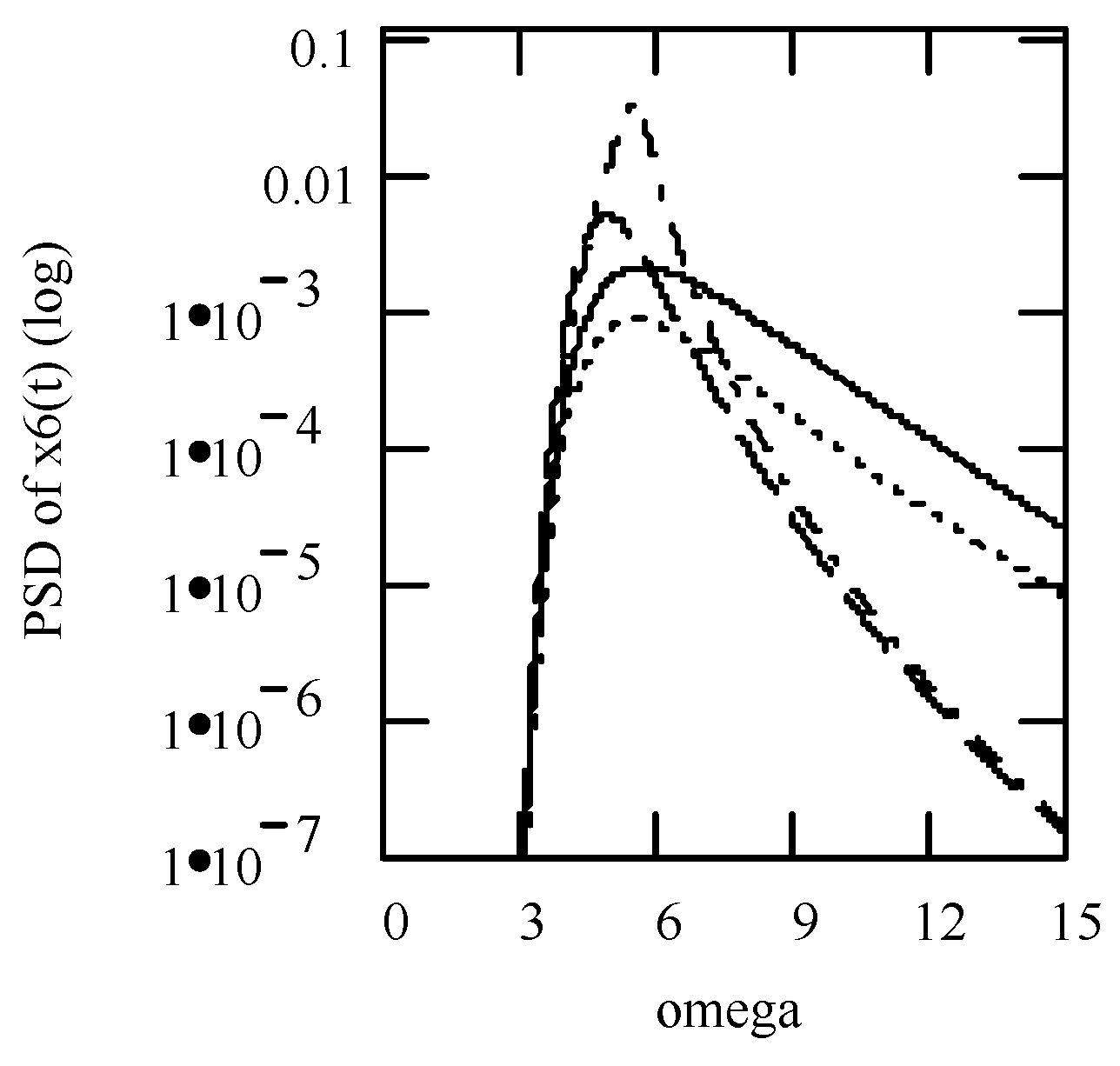
1.6. Class VI Fractional Vibration
1.7. Class VII Fractional Vibration
1.8. Problem Statements
- (1)
- From the perspective of vibration engineering, people are interested in its equivalent mass or damping or stiffness (Harris [71], Palley et al. [72]). However, analytical expressions of equivalent mass, damping, or stiffness with respect to fractional vibrations of classes I–VII are rarely reported. What they are is a problem.
- (2)
- Equivalent damping-free and damped natural angular frequencies play a key role in vibration engineering. However, their analytical expressions are seldom seen. What they are for class I–VII fractional vibrators is the second problem.
- (3)
- From an engineering point of view, the logarithmic decrement in a vibration system is essential. However, analytical expressions of equivalent logarithmic decrements in class I–VII fractional vibrators are rarely reported. What they are is a problem.
- (4)
- The quantity called the Q factor of a vibration system plays a role in engineering. Nonetheless, analytical expressions of Q factors for fractional vibrators of classes I–VII are rarely seen. What Q factors are for class I–VII fractional vibrators is a problem.
- (5)
- Let B0.707 be the frequency bandwidth of a vibration system. It is a crucial parameter in describing a vibration system. Nevertheless, analytical expressions of equivalent frequency bandwidths of fractional vibrators of classes I–VII are seldom seen. What they are is a problem
1.9. Paper Organization
2. Analytic Theory of Class VI Fractional Vibrators
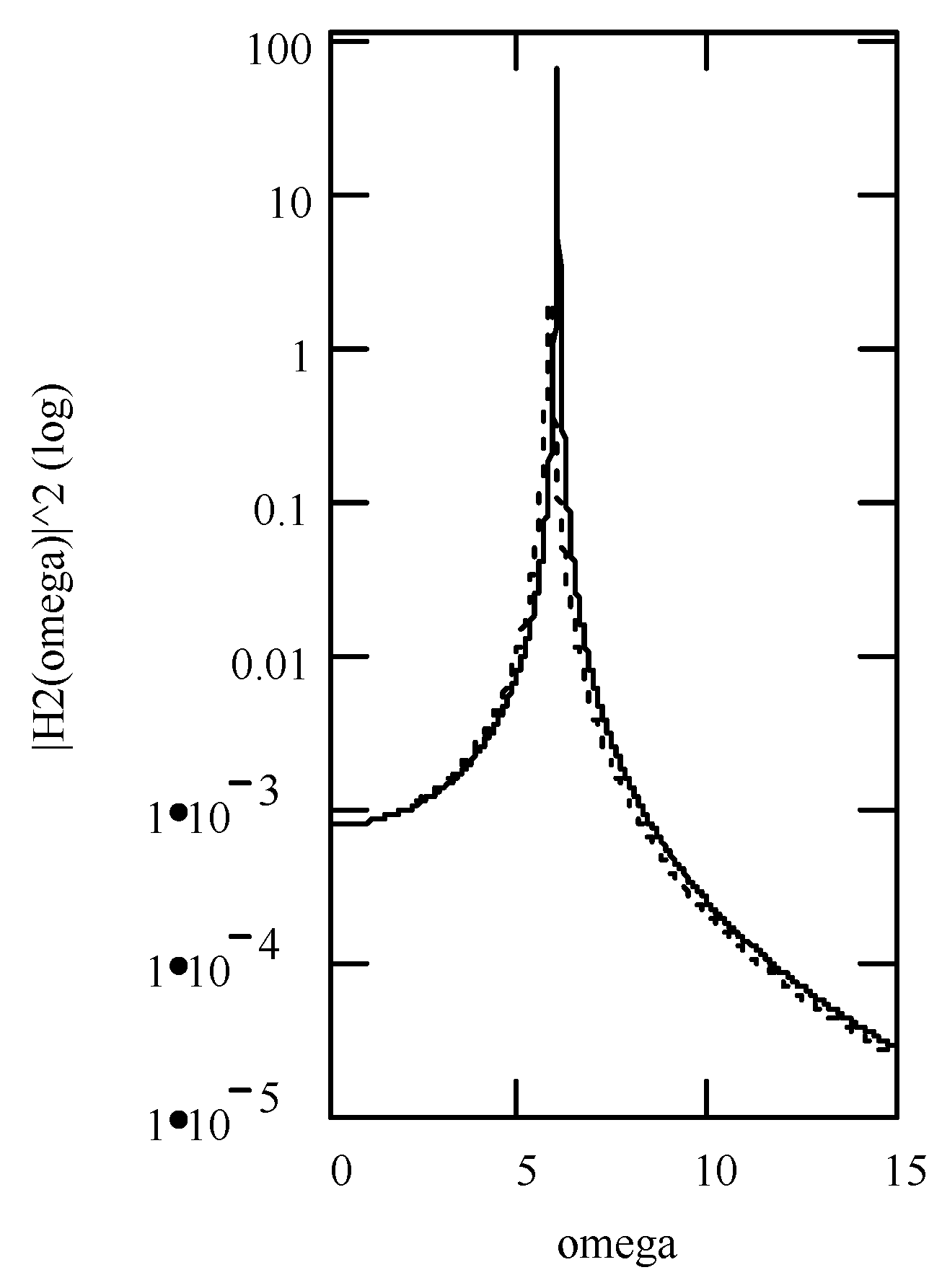
2.1. Frequency Transfer Function of Class VI Fractional Vibrator
2.2. Equivalent Motion Equation of Class VI Fractional Vibrator
2.3. Equivalent Parameters of Class VI Fractional Vibrator
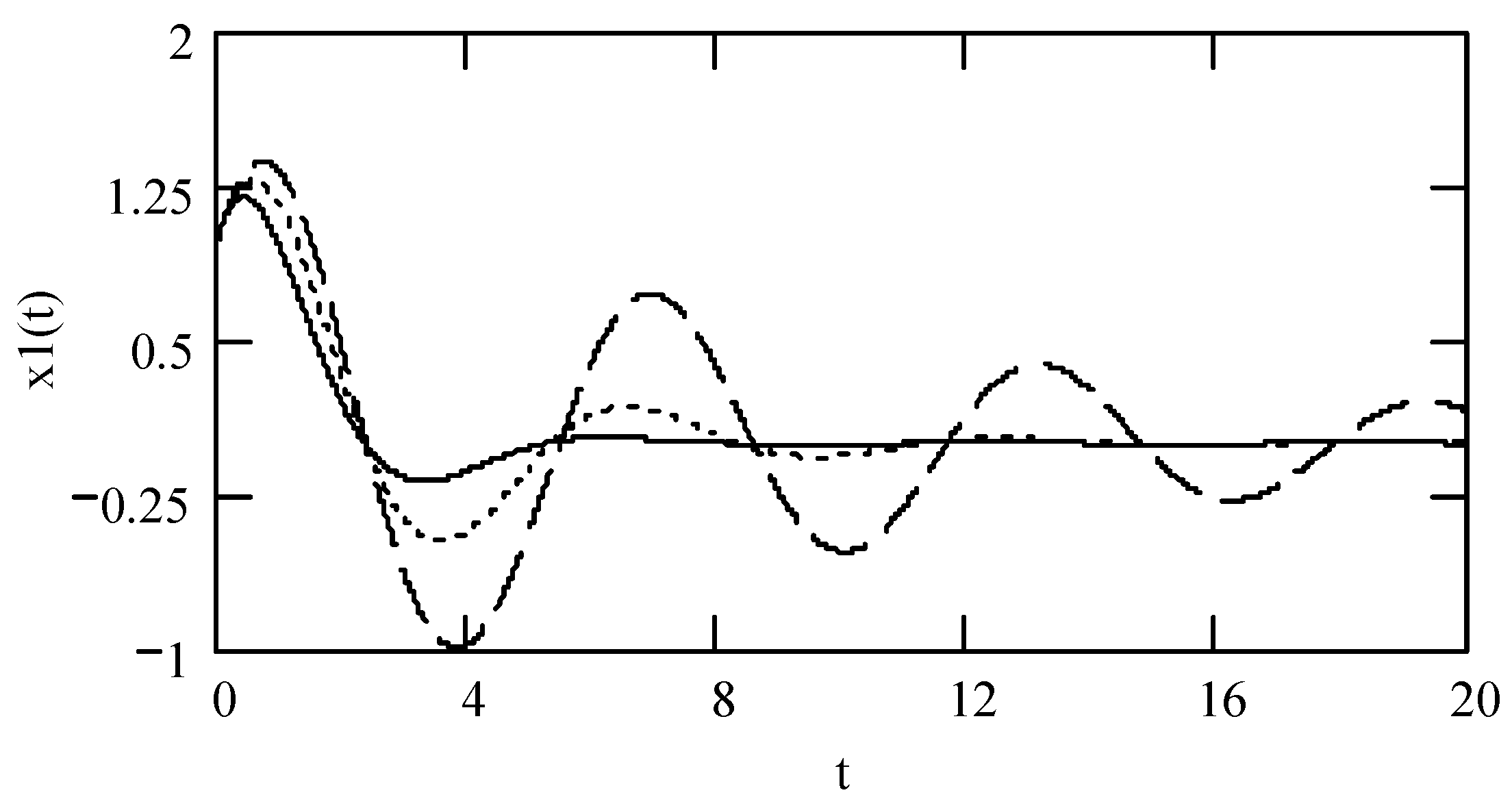
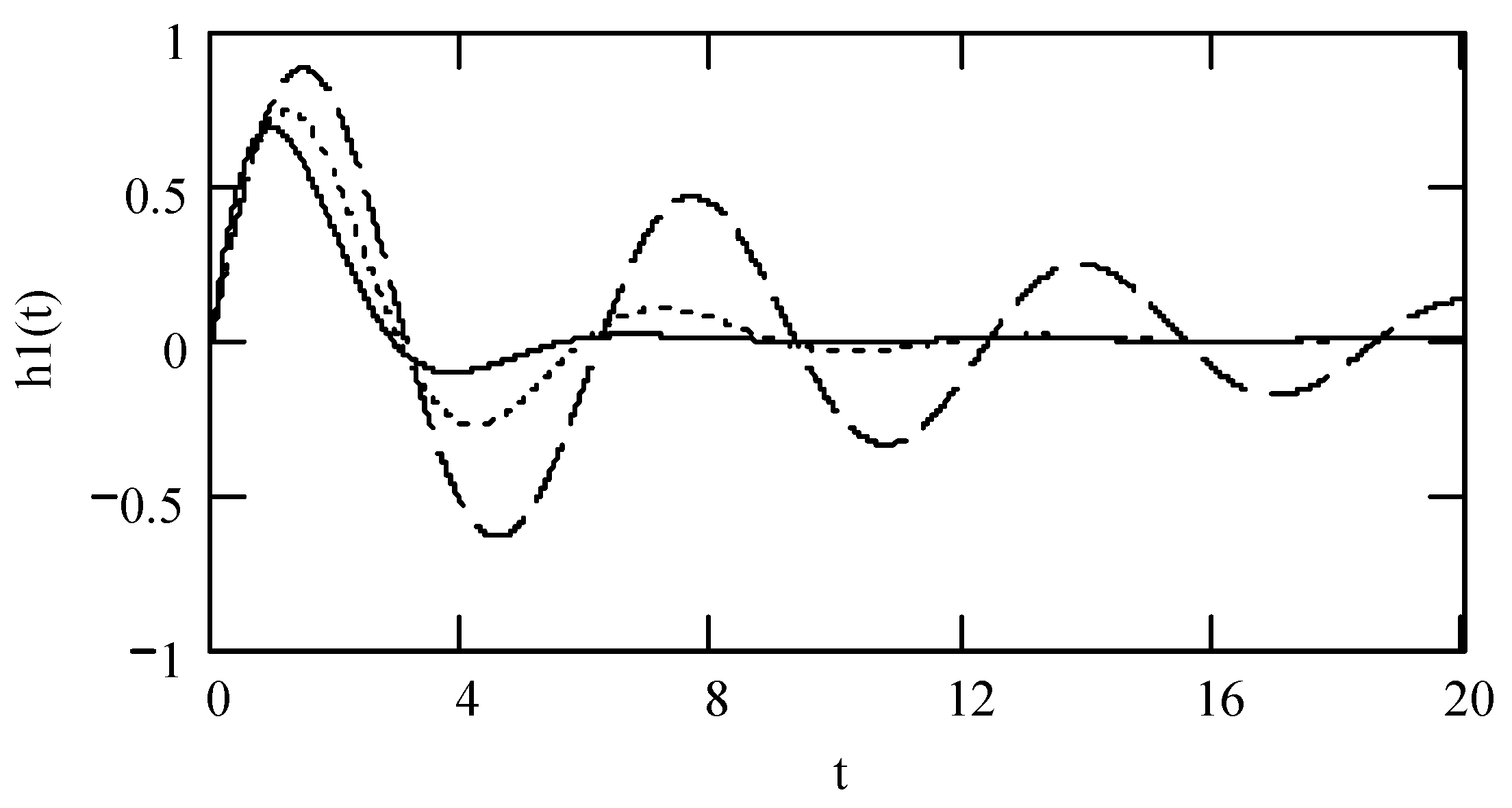
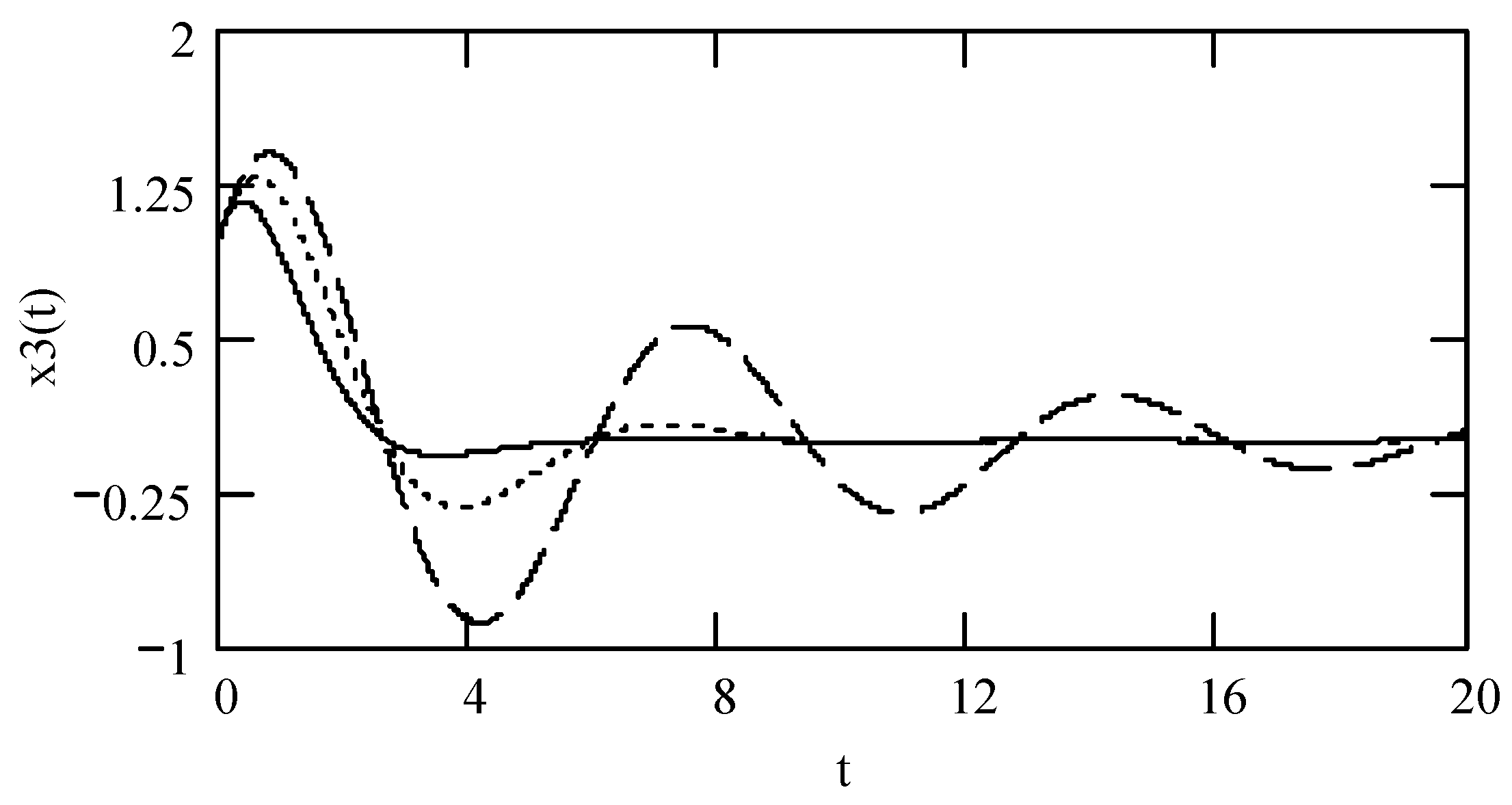
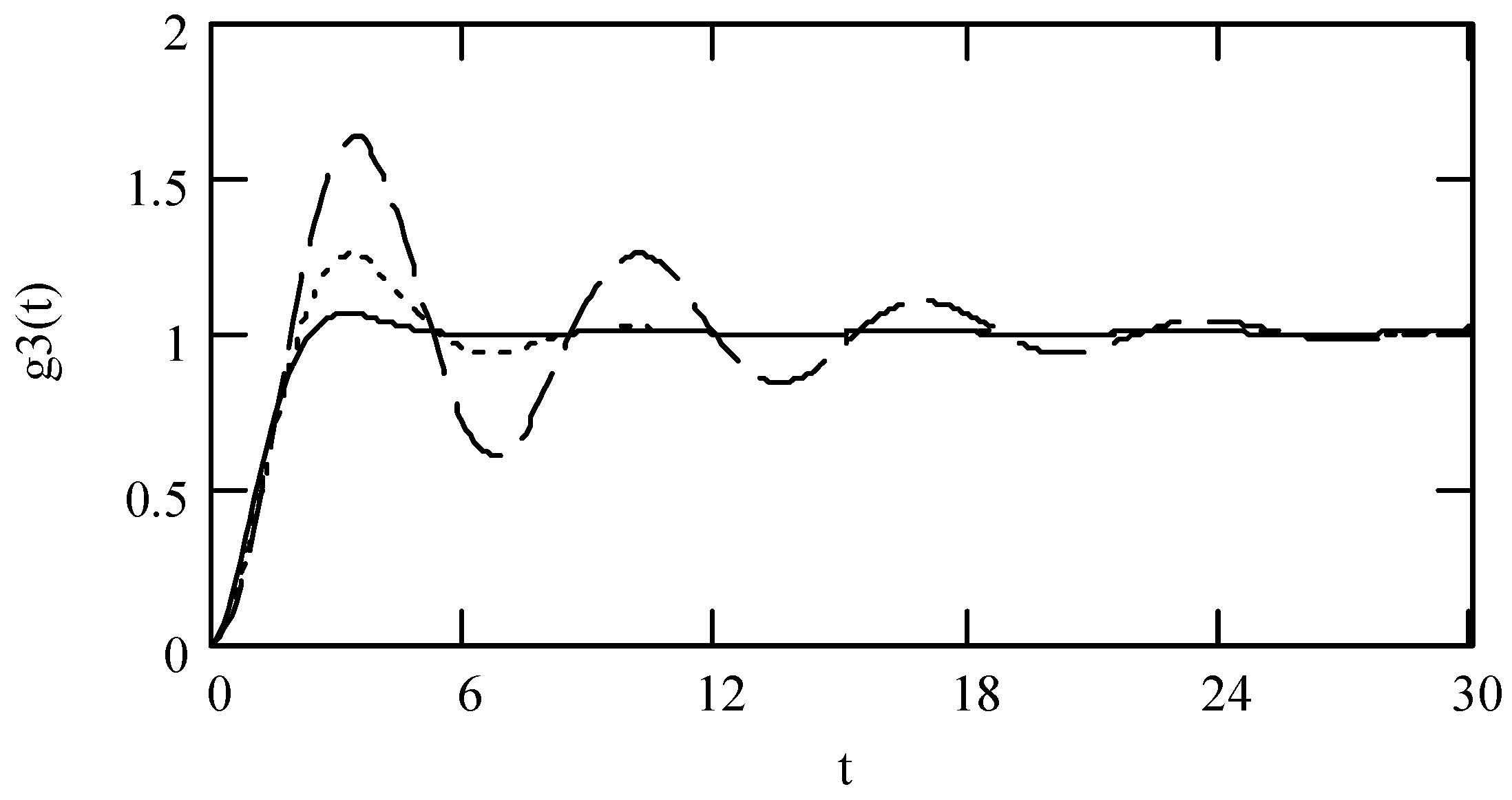
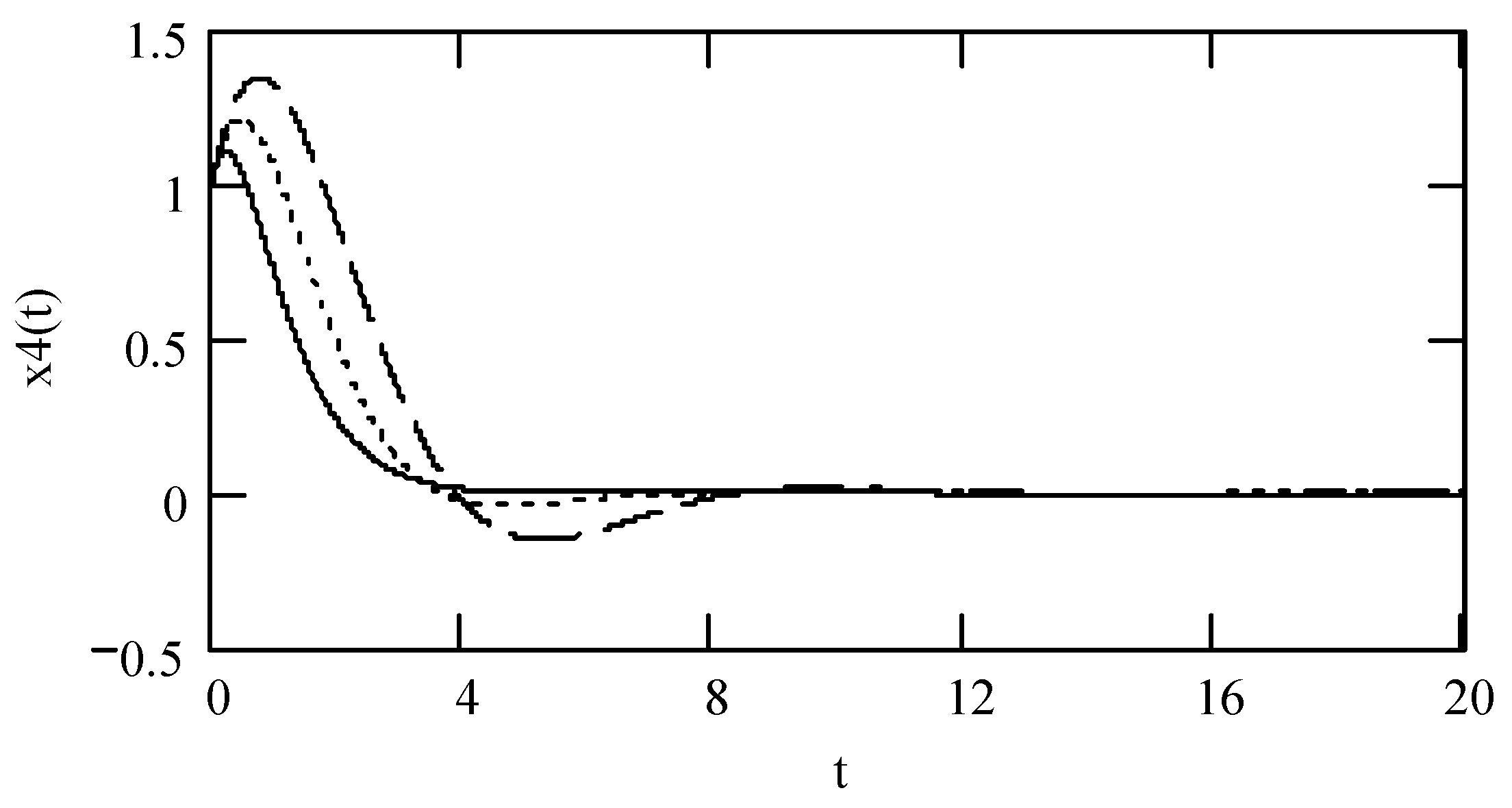
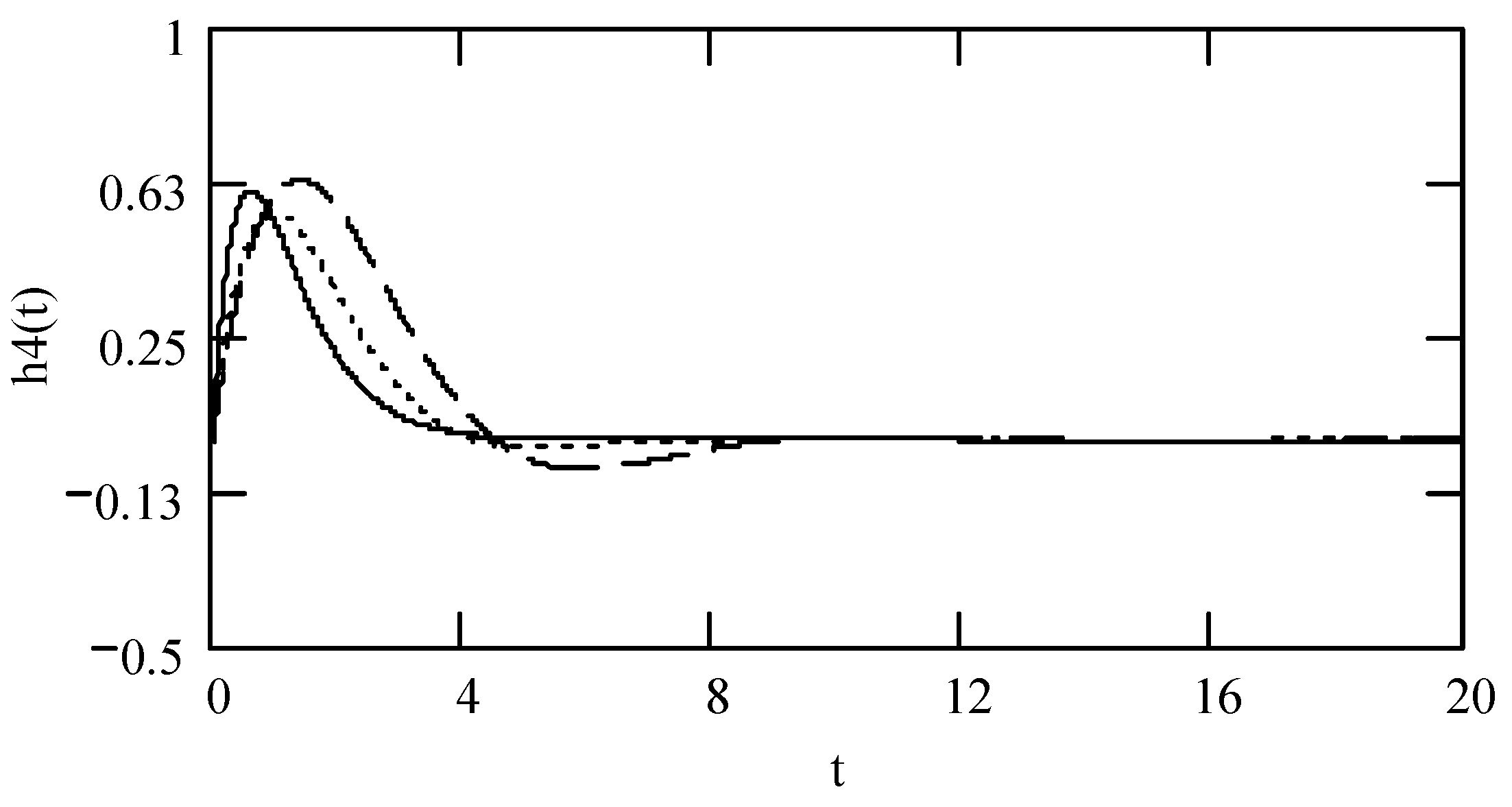
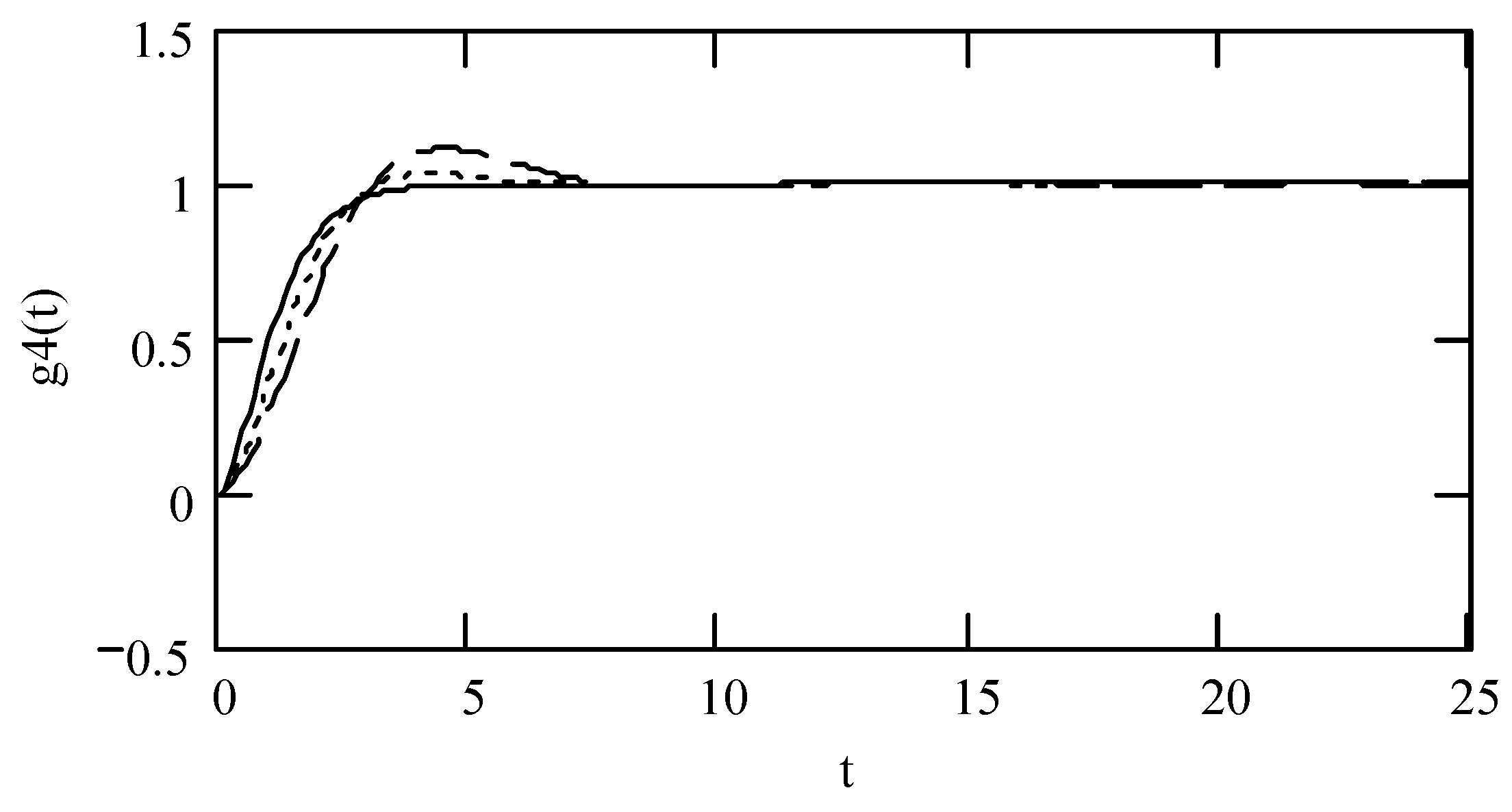
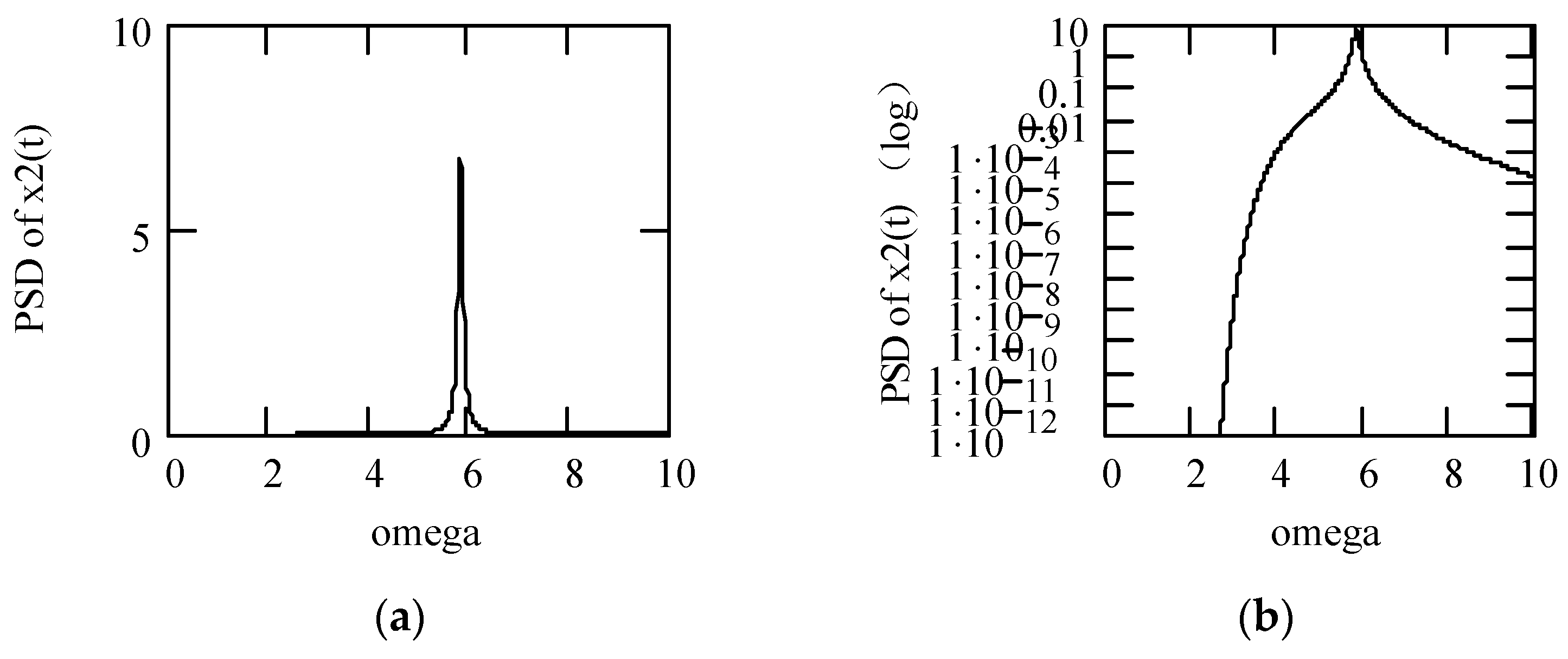
2.4. Responses of Class VI Fractional Vibrator
2.5. Equivalent Logarithmic Decrement in Class VI Fractional Vibrators
2.6. Quality Factor of Class VI Fractional Vibrators
2.7. Equivalent Frequency Bandwidth of Class VI Fractional Vibrators
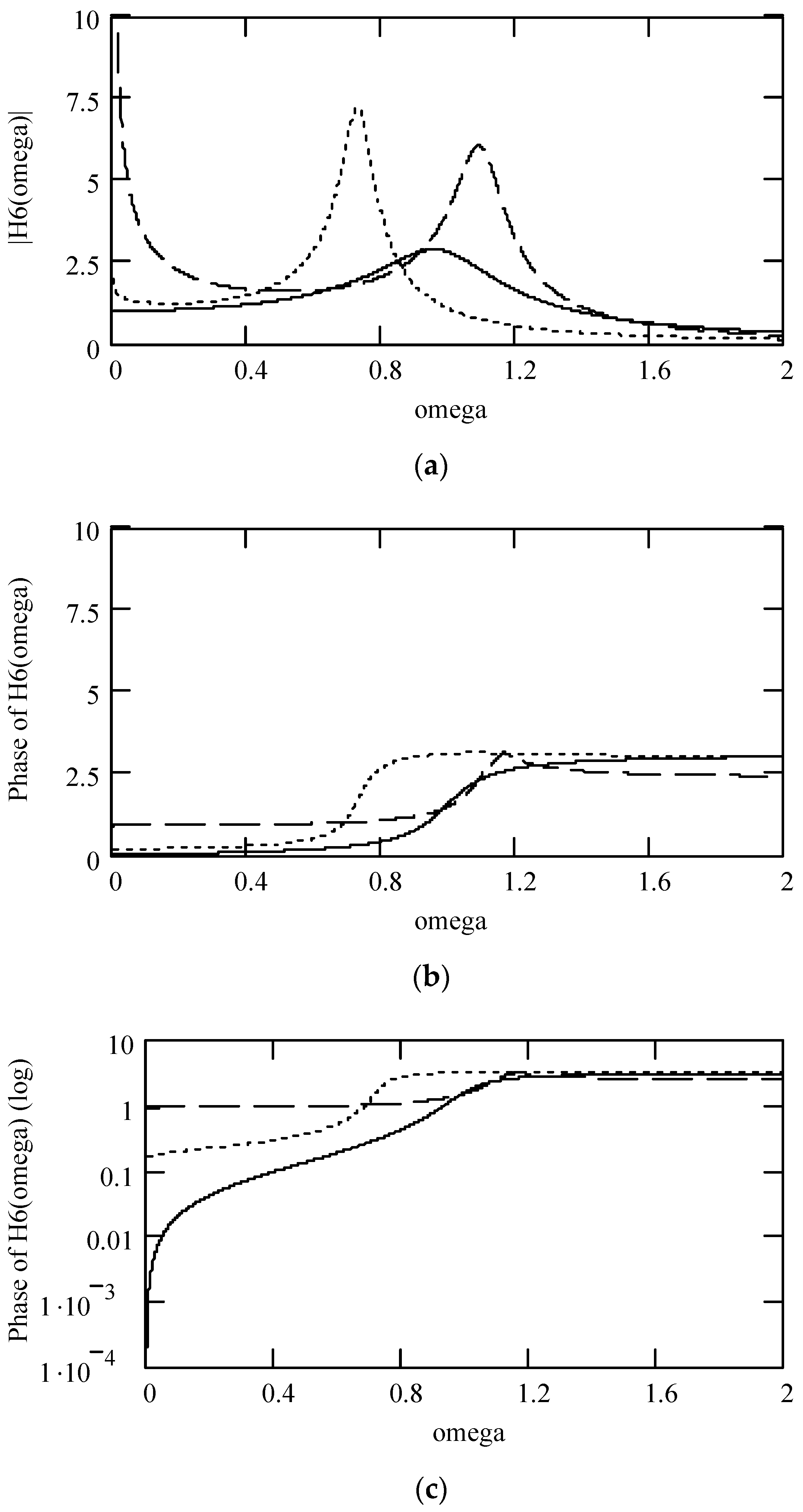
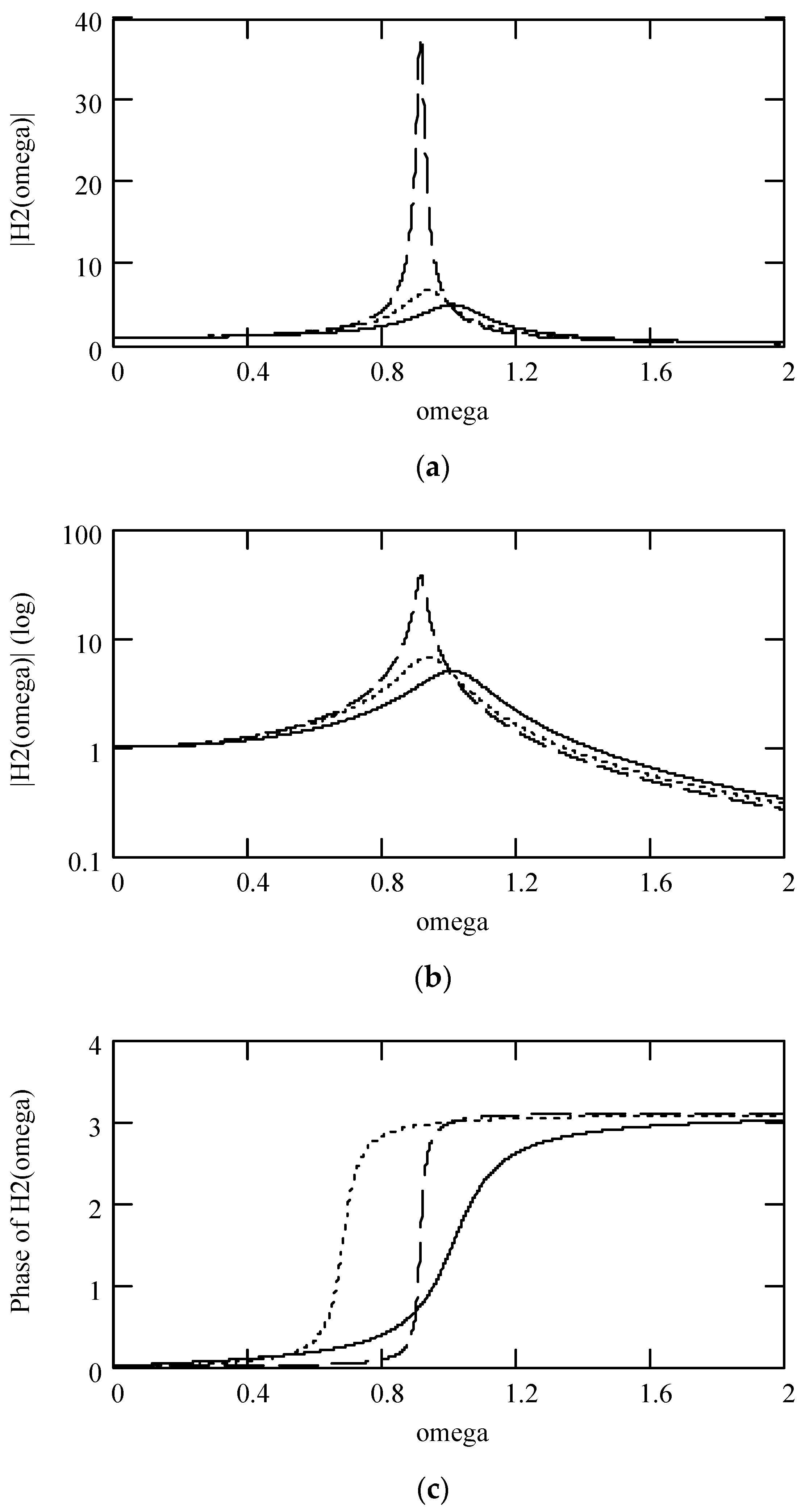
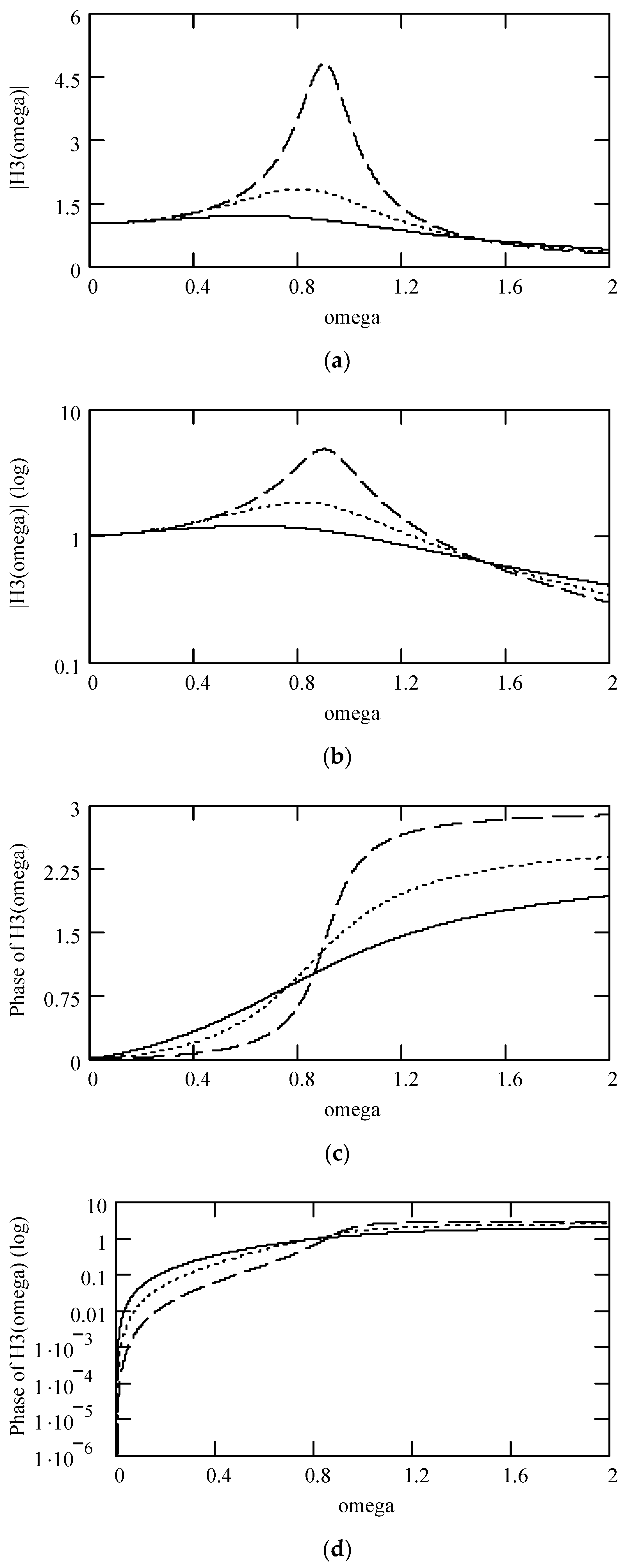
2.8. More about Frequency Transfer Function of Class VI Fractional Vibrations
3. Representing Analytic Theory of Class I Fractional Vibrators
3.1. Frequency Transfer Function of Class I Fractional Vibrator
3.2. Equivalent Motion Equation of Class I Fractional Vibrator
3.3. Equivalent Parameters of Class I Fractional Vibrator
3.4. Responses of Class I Fractional Vibrator
3.5. Equivalent Logarithmic Decrement in Class I Fractional Vibrators
3.6. Quality Factor of Class I Fractional Vibrators
3.7. Equivalent Frequency Bandwidth of Class I Fractional Vibrators
3.8. More about Frequency Transfer Function of Class I Fractional Vibrators
4. Analytic Theory of Class II Fractional Vibrators
4.1. Frequency Transfer Function of Class II Fractional Vibrator
4.2. Equivalent Motion Equation of Class II Fractional Vibrator
4.3. Equivalent Parameters of Class II Fractional Vibrator
4.4. Responses of Class II Fractional Vibrator
4.5. Equivalent Logarithmic Decrement in Class II Fractional Vibrators
4.6. Quality Factor of Class II Fractional Vibrators
4.7. Equivalent Frequency Bandwidth of Class II Fractional Vibrators
4.8. More about Frequency Transfer Function of Class II Fractional Vibrators
5. Representation of Analytic Theory of Class III Fractional Vibrators
5.1. Frequency Transfer Function of Class III Fractional Vibrator
5.2. Equivalent Motion Equation of Class III Fractional Vibrator
5.3. Equivalent Parameters of Class III Fractional Vibrator
5.4. Responses of Class III Fractional Vibrator
5.5. Equivalent Logarithmic Decrement in Class III Fractional Vibrators
5.6. Quality Factor of Class III Fractional Vibrators
5.7. Equivalent Frequency Bandwidth of Class III Fractional Vibrators
5.8. More about Frequency Transfer Function of Class III Fractional Vibrators
6. Analytic Theory of Class IV Fractional Vibrators
6.1. Frequency Transfer Function of Class IV Fractional Vibrator
6.2. Equivalent Motion Equation of Class IV Fractional Vibrator
6.3. Equivalent Parameters of Class IV Fractional Vibrator
6.4. Responses of Class IV Fractional Vibrator
6.5. Equivalent Logarithmic Decrement in Class IV Fractional Vibrators
6.6. Quality Factor of Class IV Fractional Vibrators
6.7. Equivalent Frequency Bandwidth of Class IV Fractional Vibrators
6.8. More about Frequency Transfer Function of Class IV Fractional Vibrators
7. Analytic Theory of Class V Fractional Vibrators
7.1. Frequency Transfer Function of Class V Fractional Vibrator
7.2. Equivalent Motion Equation of Class V Fractional Vibrator
7.3. Equivalent Parameters of Class V Fractional Vibrator
7.4. Responses of Class V Fractional Vibrator
7.5. Equivalent Logarithmic Decrement in Class V Fractional Vibrators
7.6. Quality Factor of Class V Fractional Vibrators
7.7. Equivalent Frequency Bandwidth of Class V Fractional Vibrators
7.8. More about Frequency Transfer Function of Class V Fractional Vibrators
8. Analytic Theory of Class VII Fractional Vibrators
8.1. Frequency Transfer Function of Class VII Fractional Vibrator
8.2. Equivalent Motion Equation of Class VII Fractional Vibrator
8.3. Equivalent Parameters of Class VII Fractional Vibrator
8.4. Responses of Class VII Fractional Vibrator
8.5. Equivalent Logarithmic Decrement in Class VII Fractional Vibrators
8.6. Quality Factor of Class VII Fractional Vibrators
8.7. Equivalent Frequency Bandwidth of Class VII Fractional Vibrators
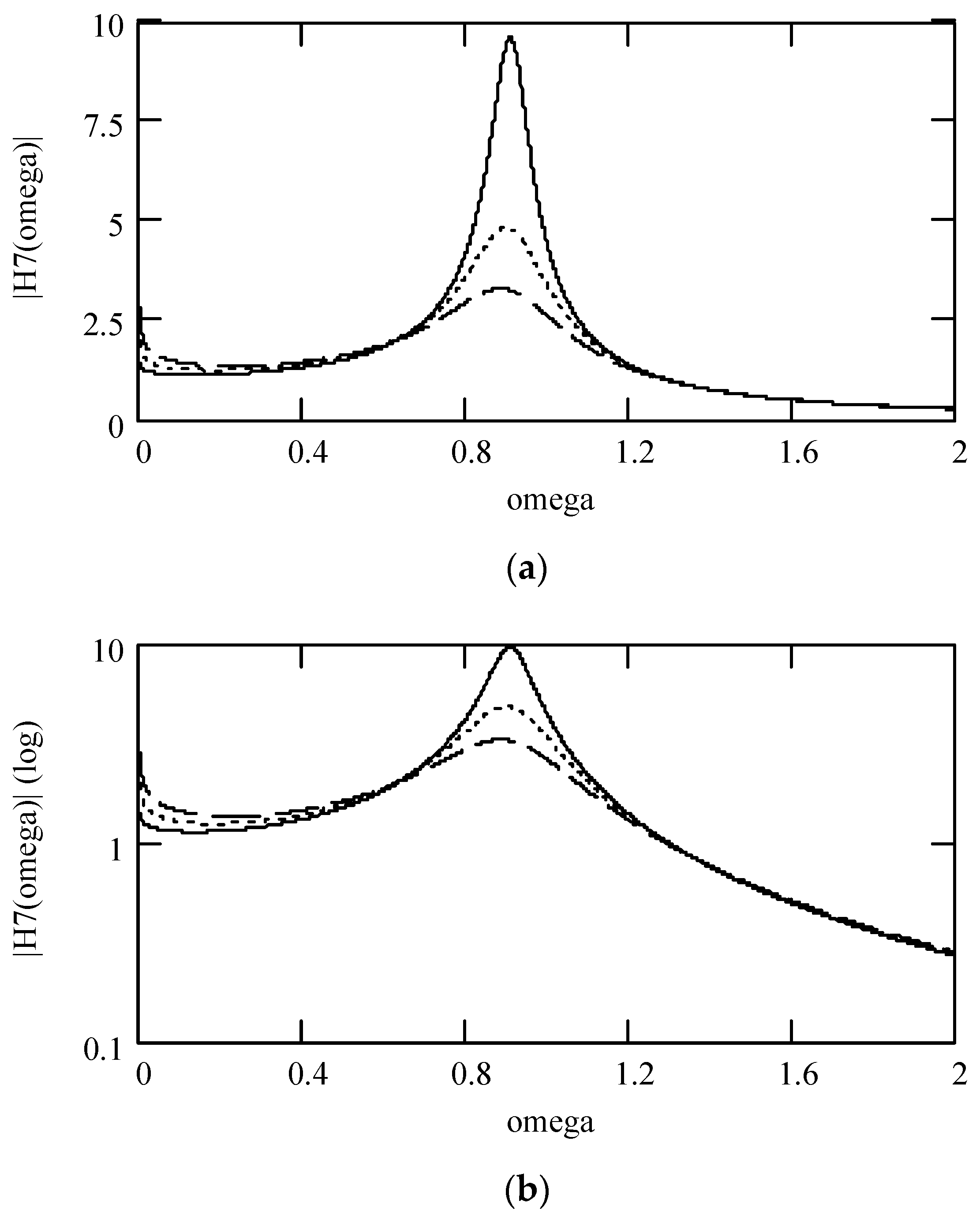
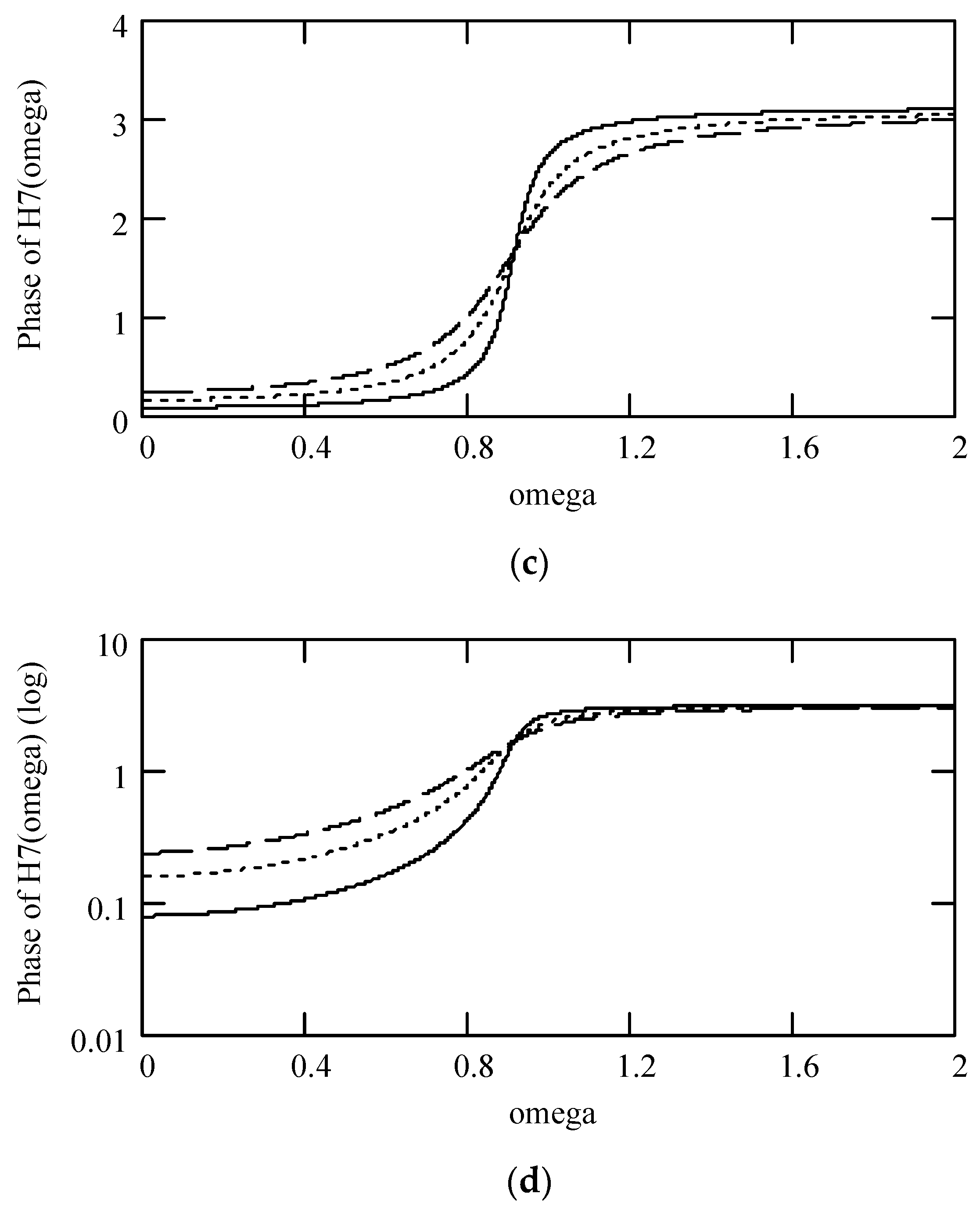
8.8. More about Frequency Transfer Function of Class VII Fractional Vibrators
9. Application: Analytical Expression of Forced Response to Multi-Fractional Damped Euler–Bernoulli Beam
9.1. Multi-Fractional Damped Euler–Bernoulli Beam
9.2. Closed Form Forced Response
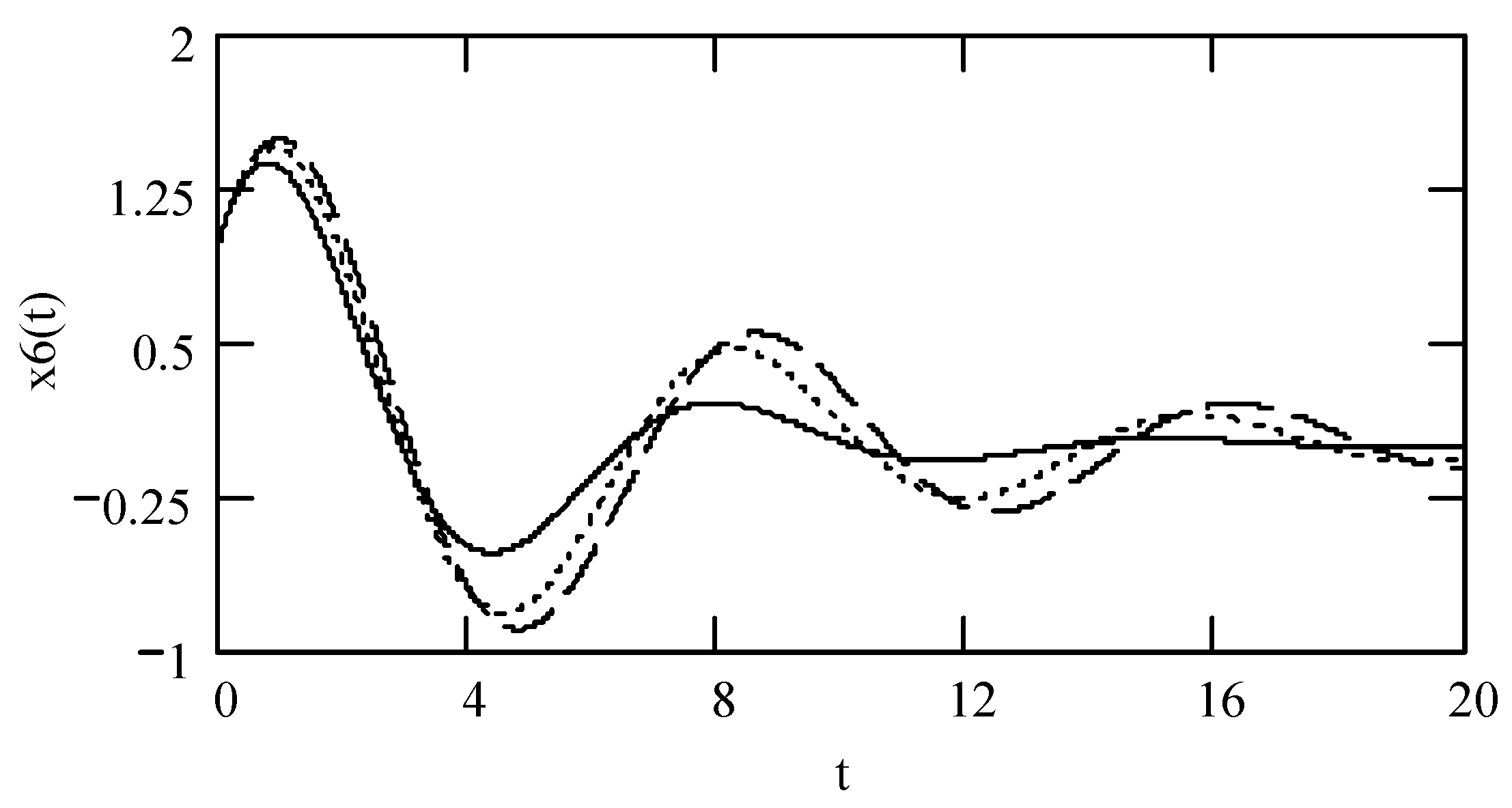
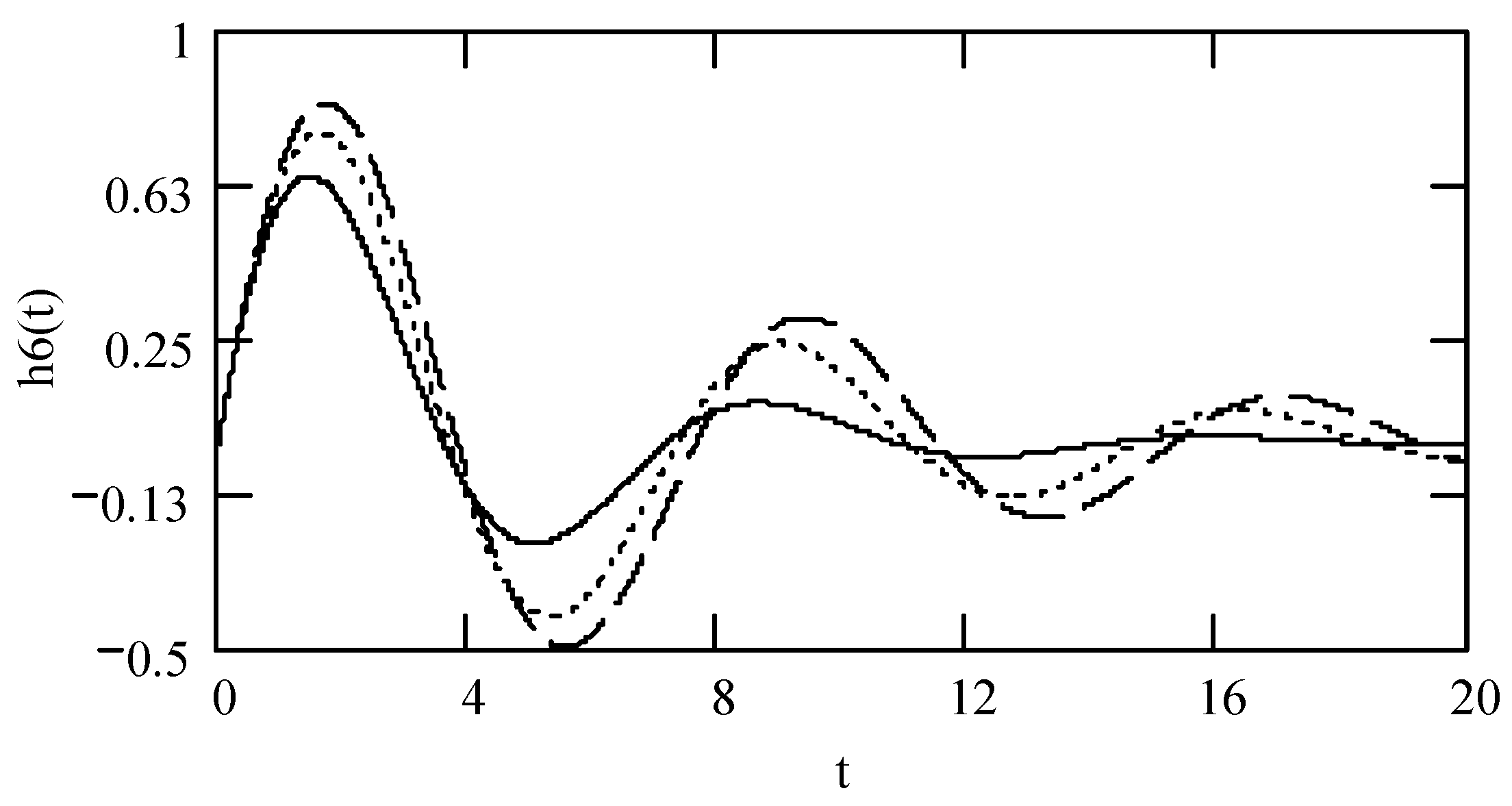
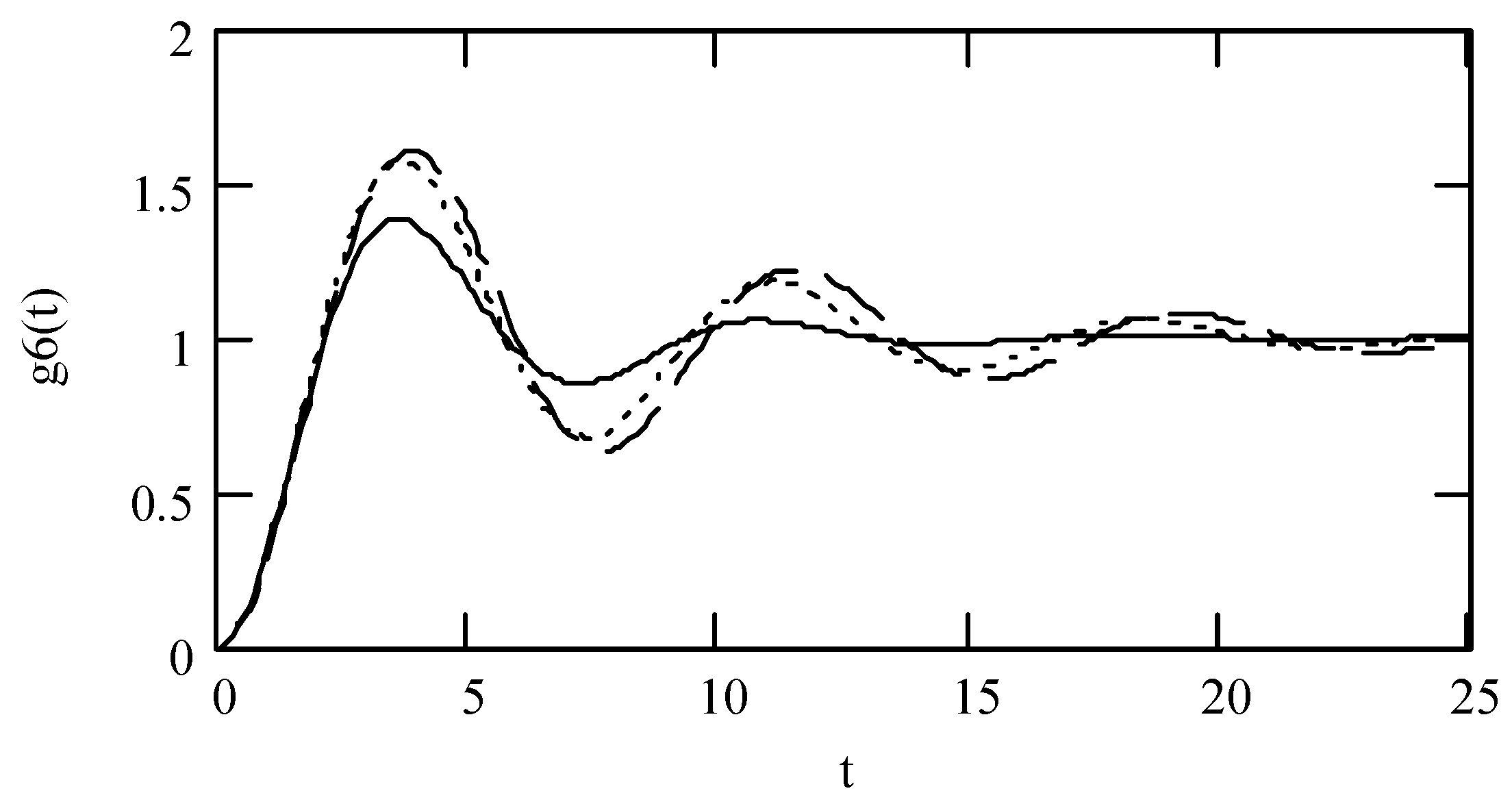
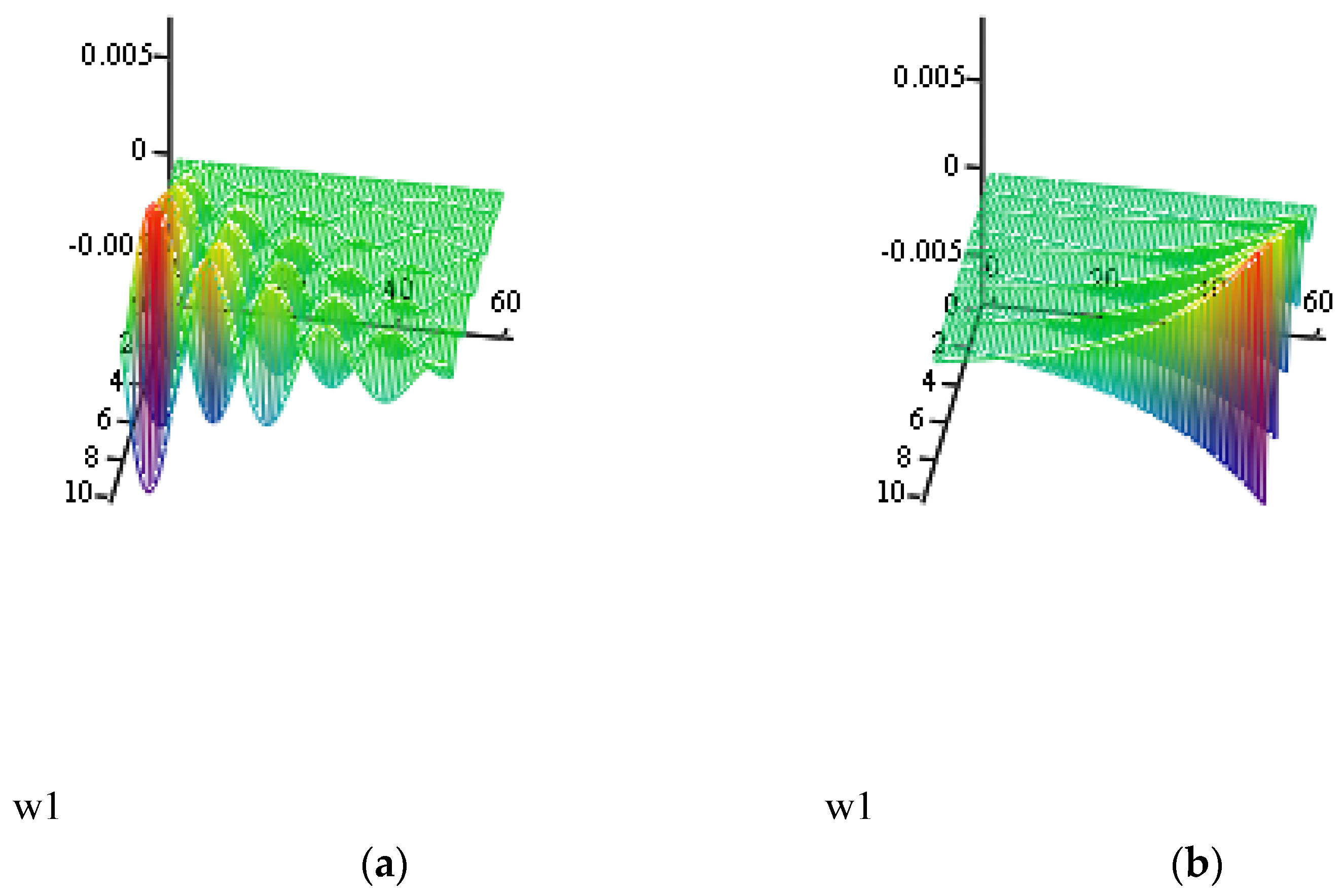
9.3. Demonstration
10. Application: Seven Classes of Fractionally Random Vibrations Driven by P-M Spectrum
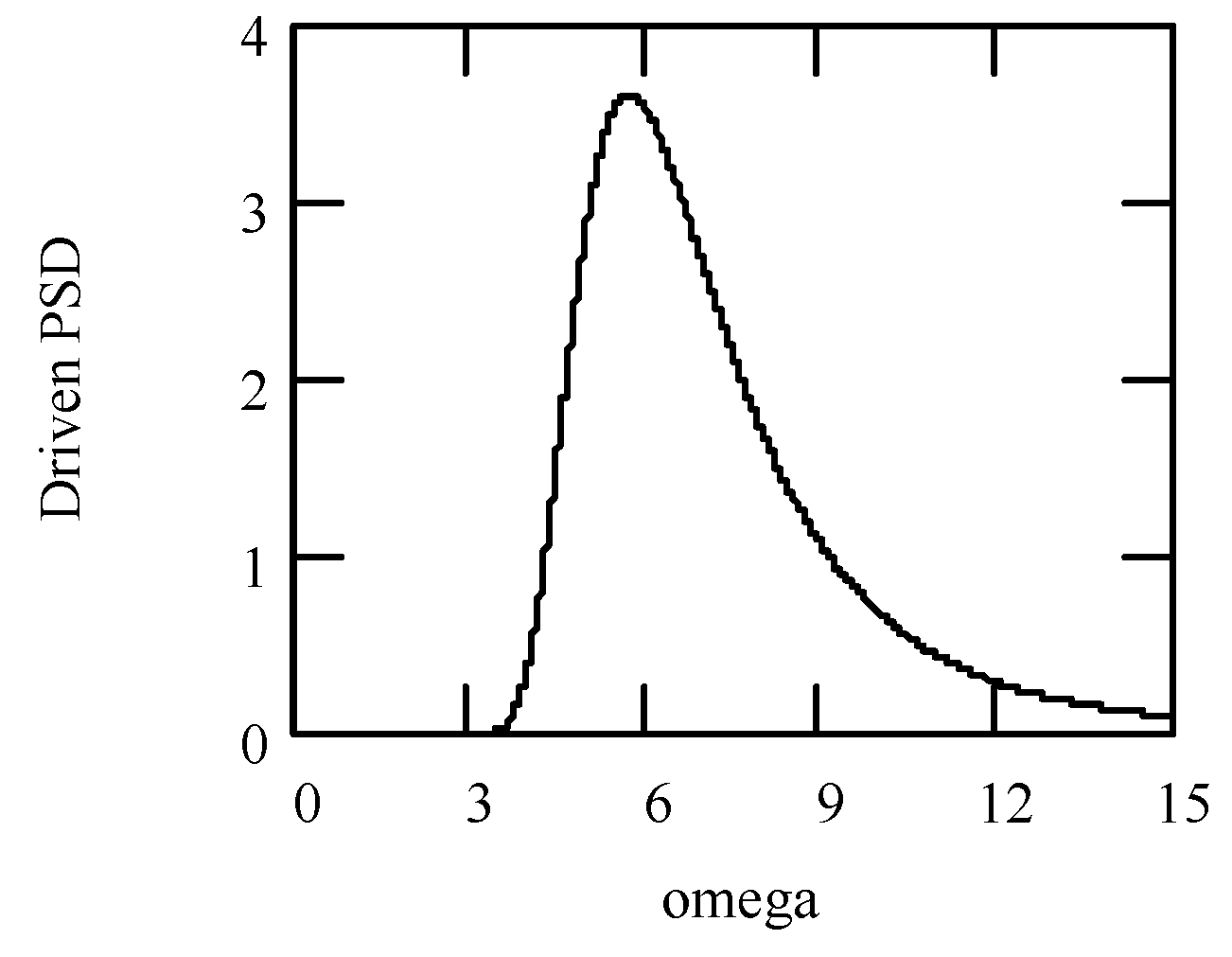
10.1. Background
10.2. Responses of Class I Fractional Vibrators Driven by P-M Spectrum
10.2.1. Computations
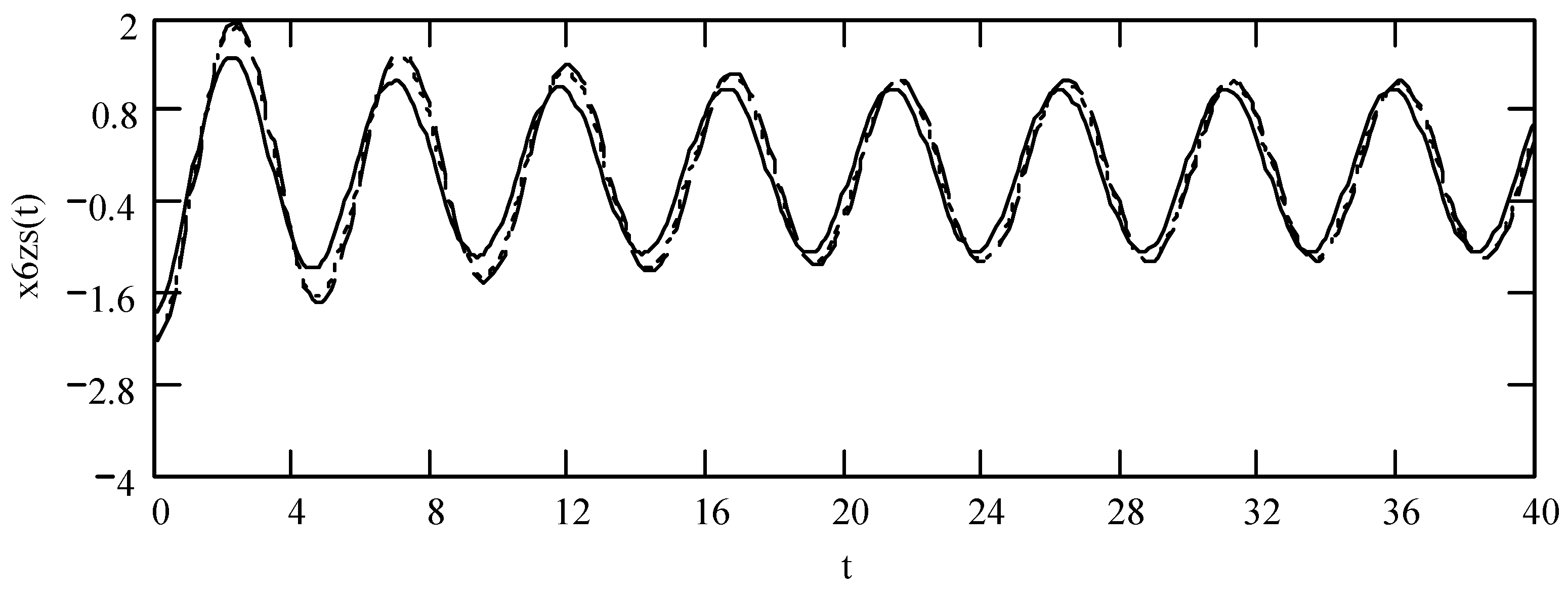
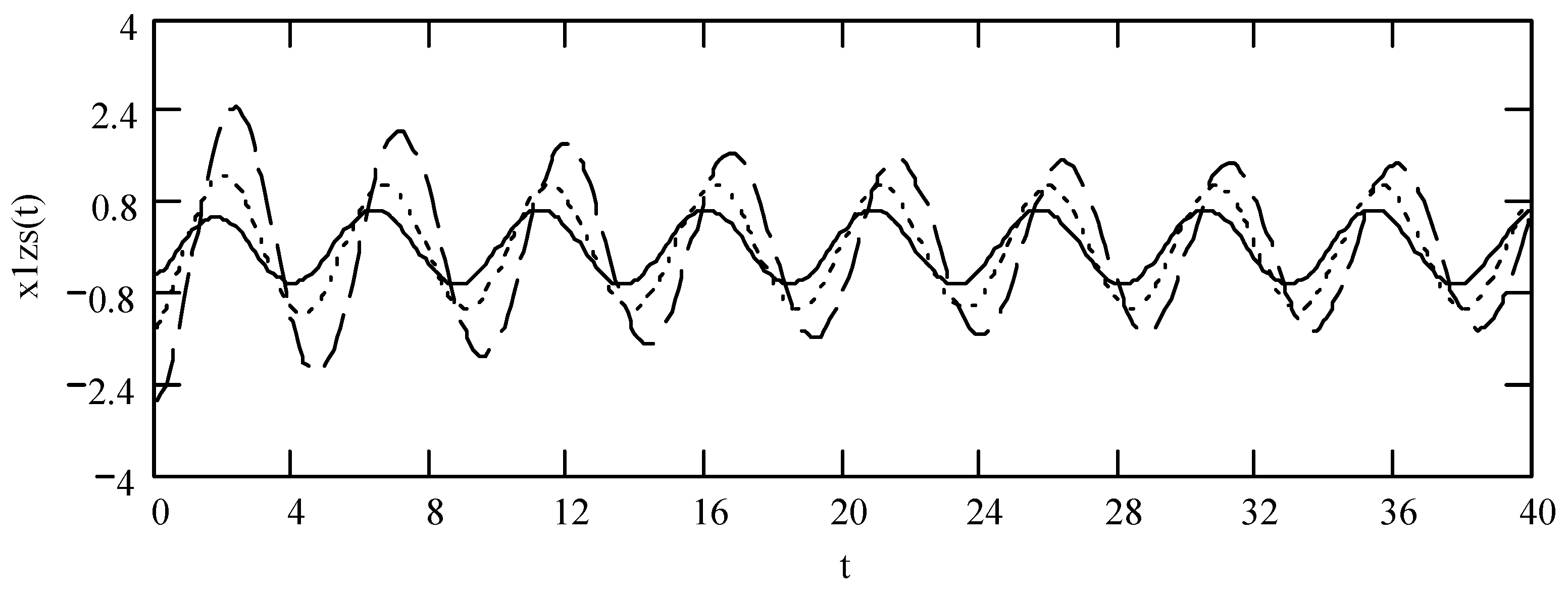
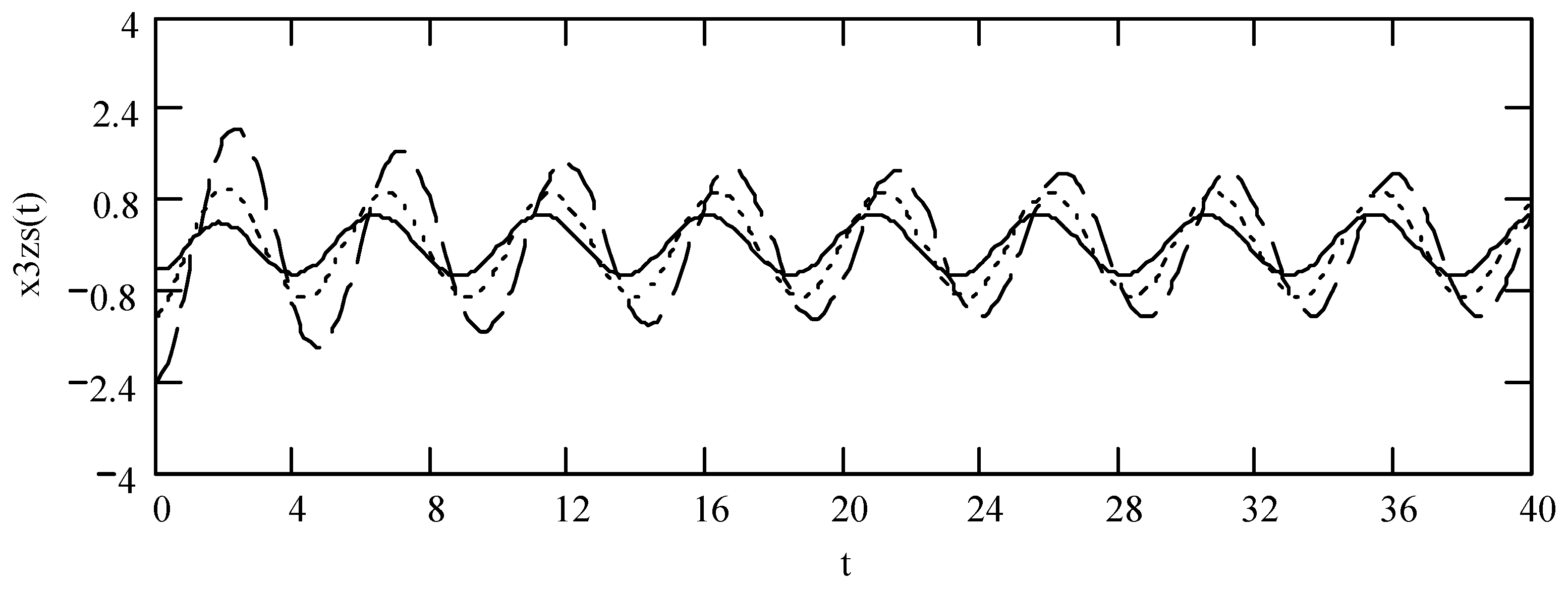
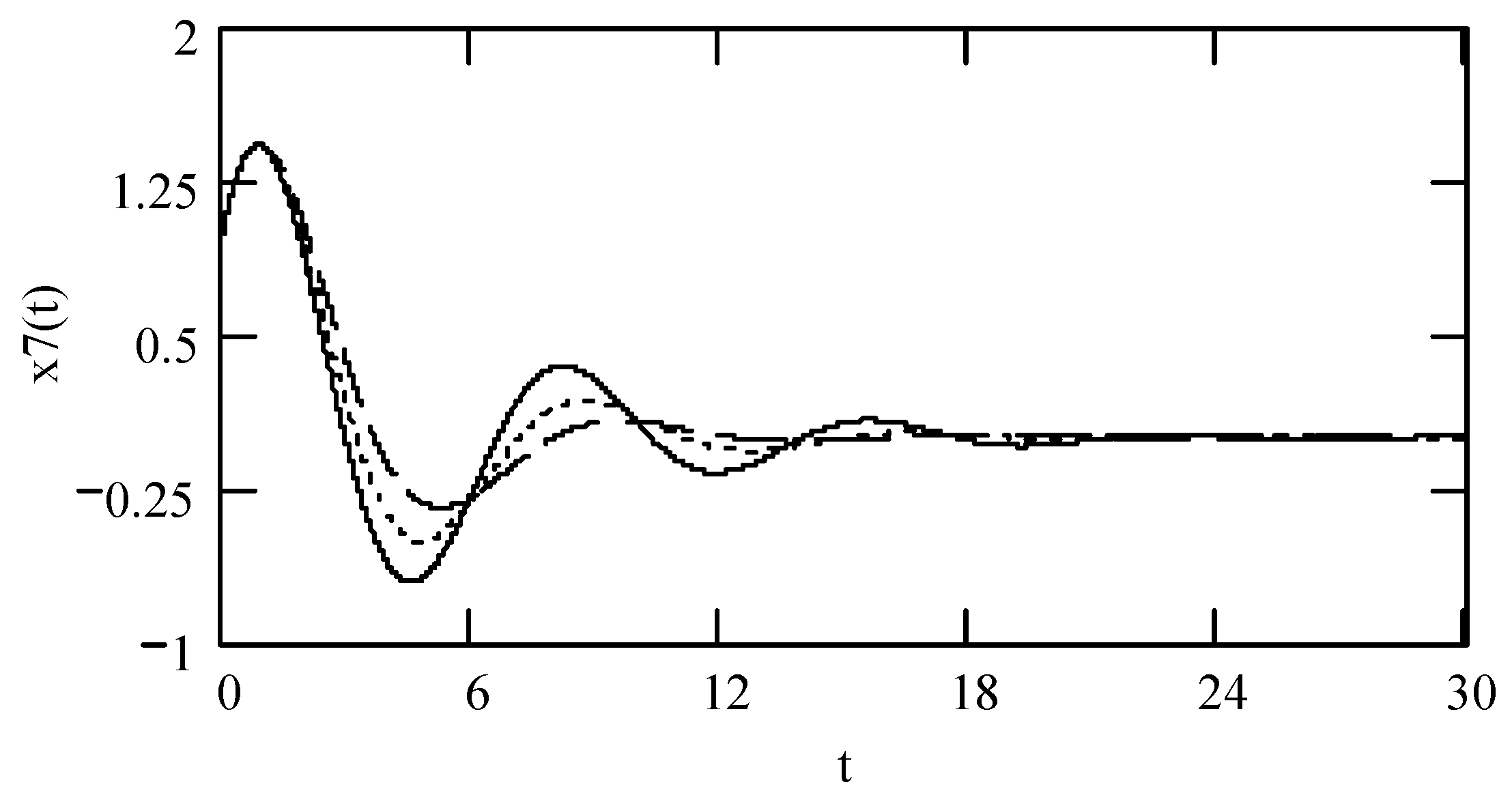
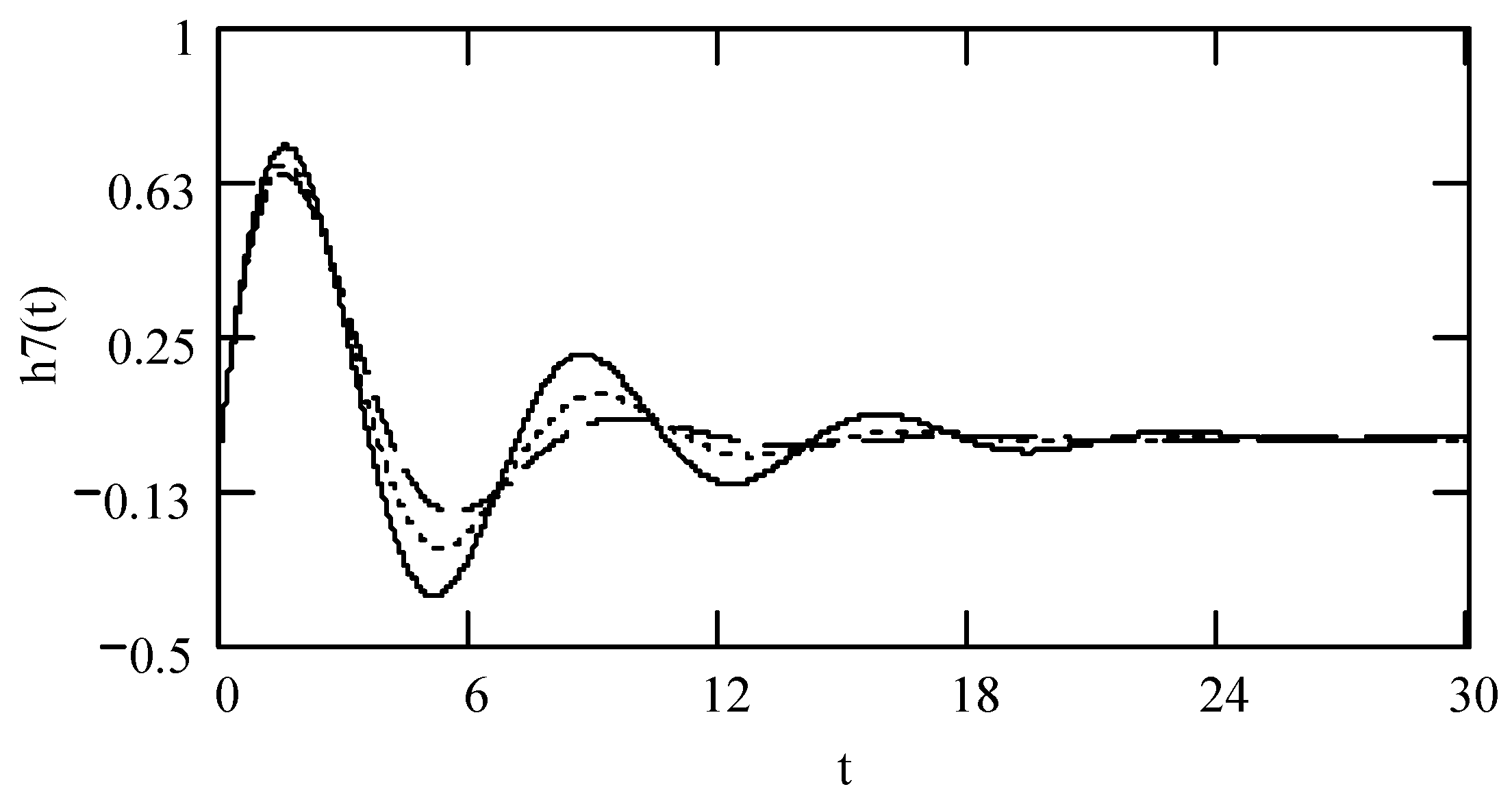
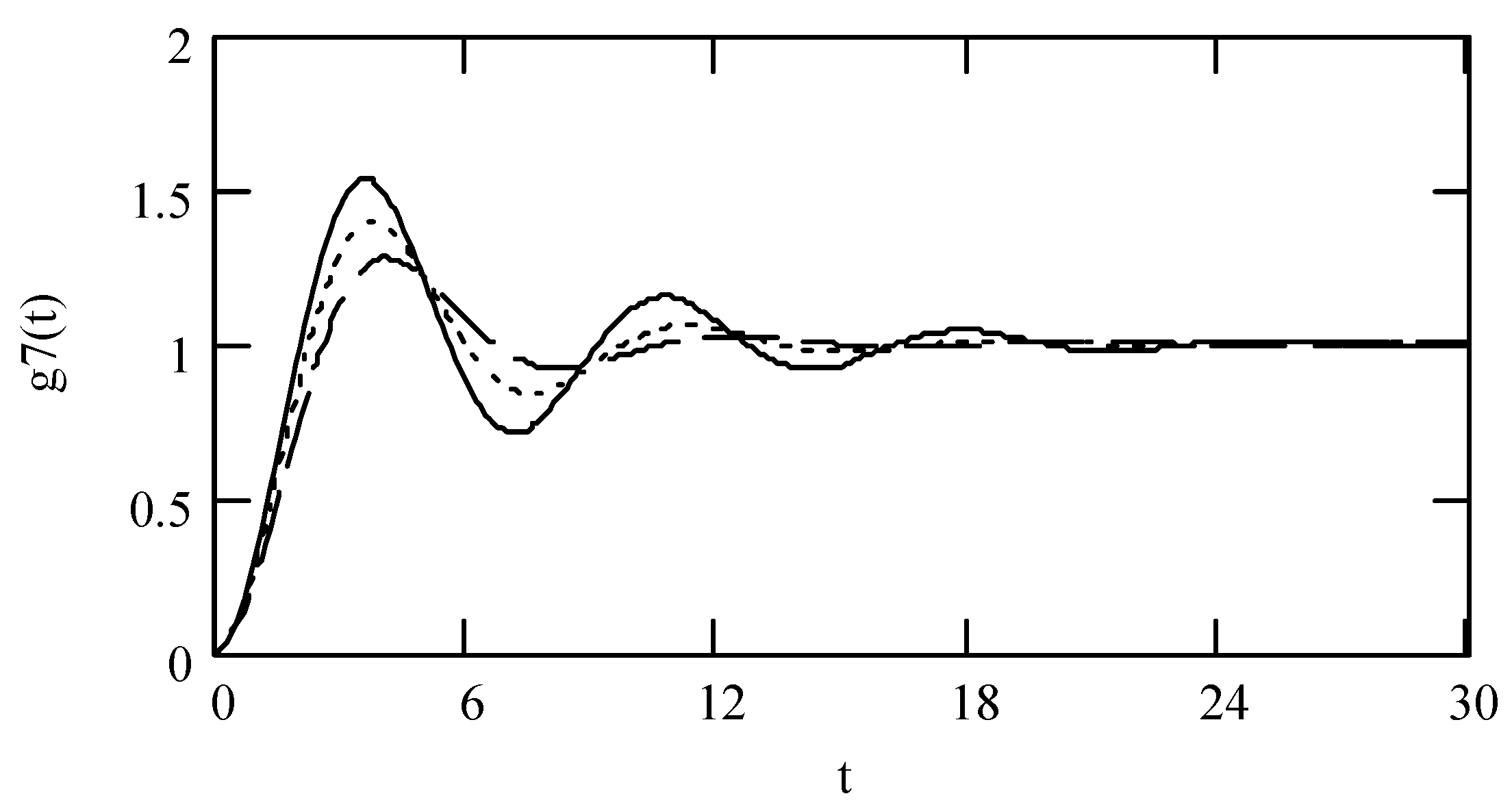
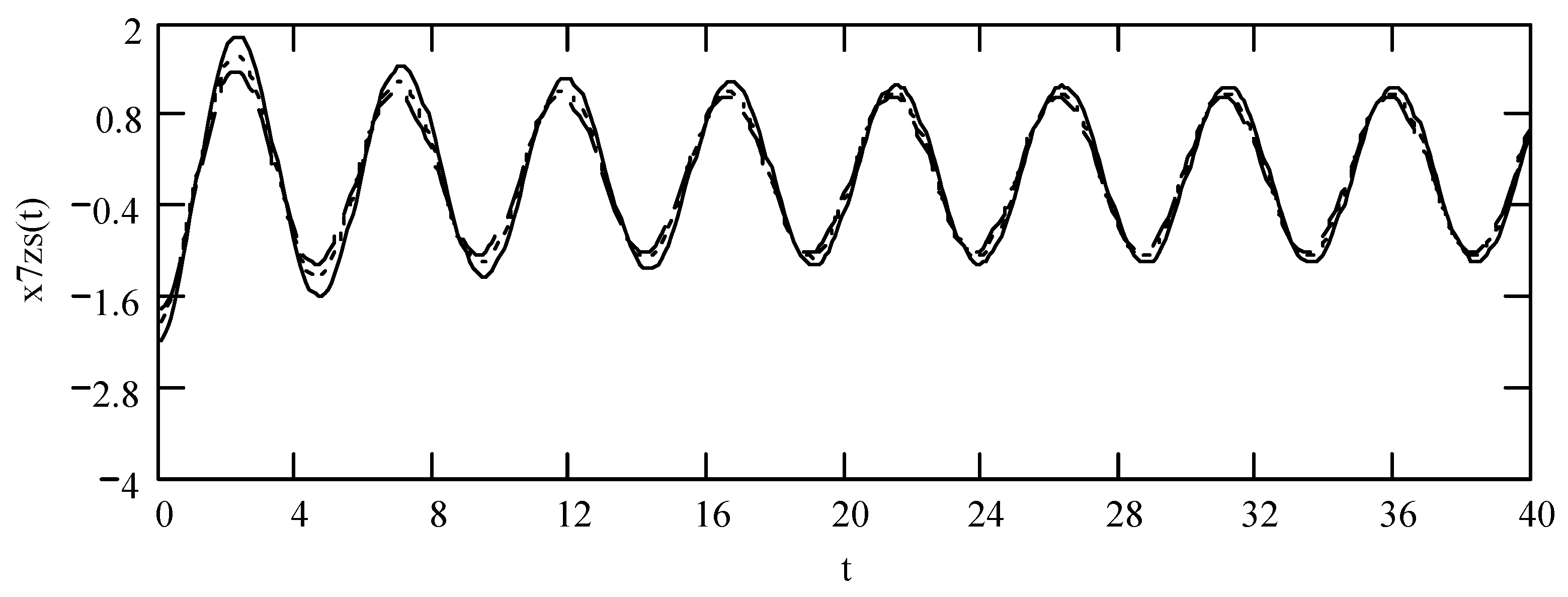
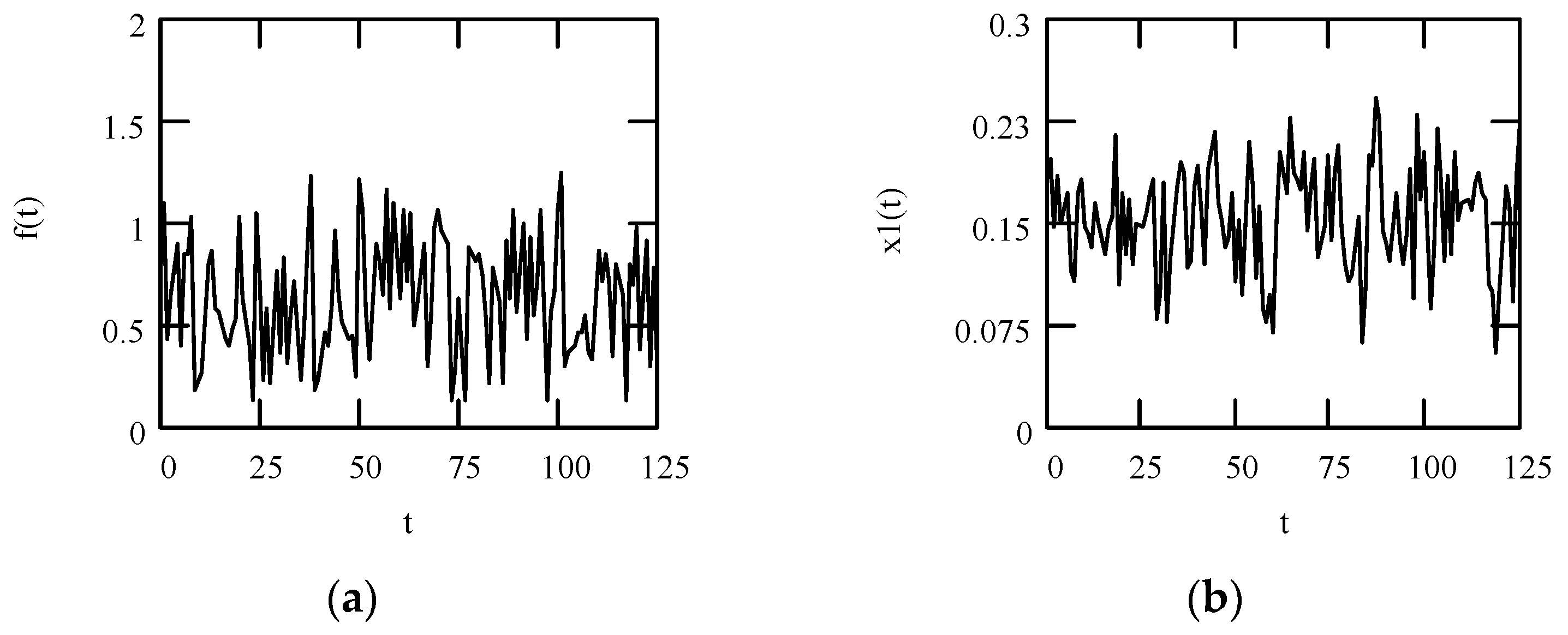
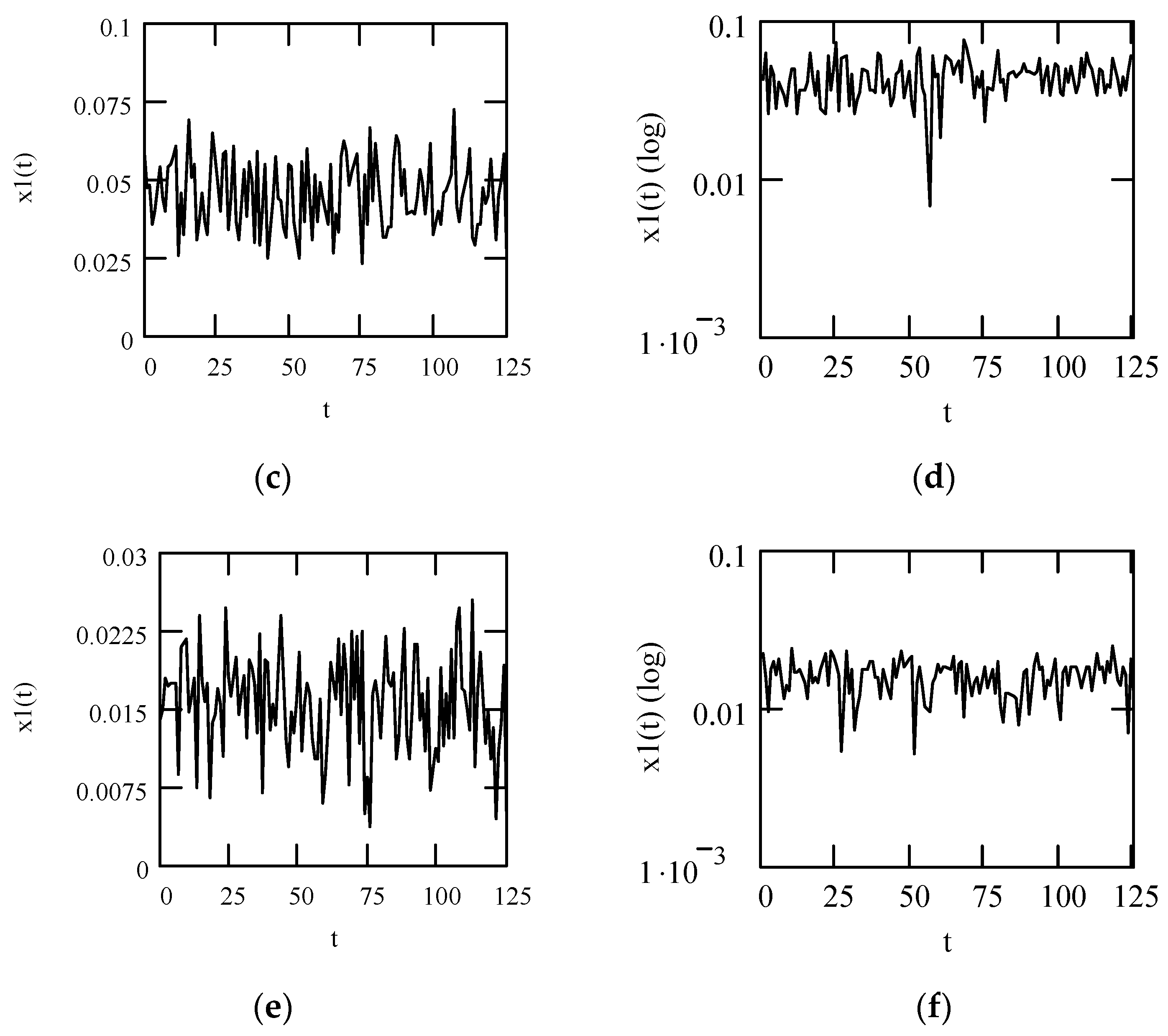
10.2.2. Effects of α on Responses
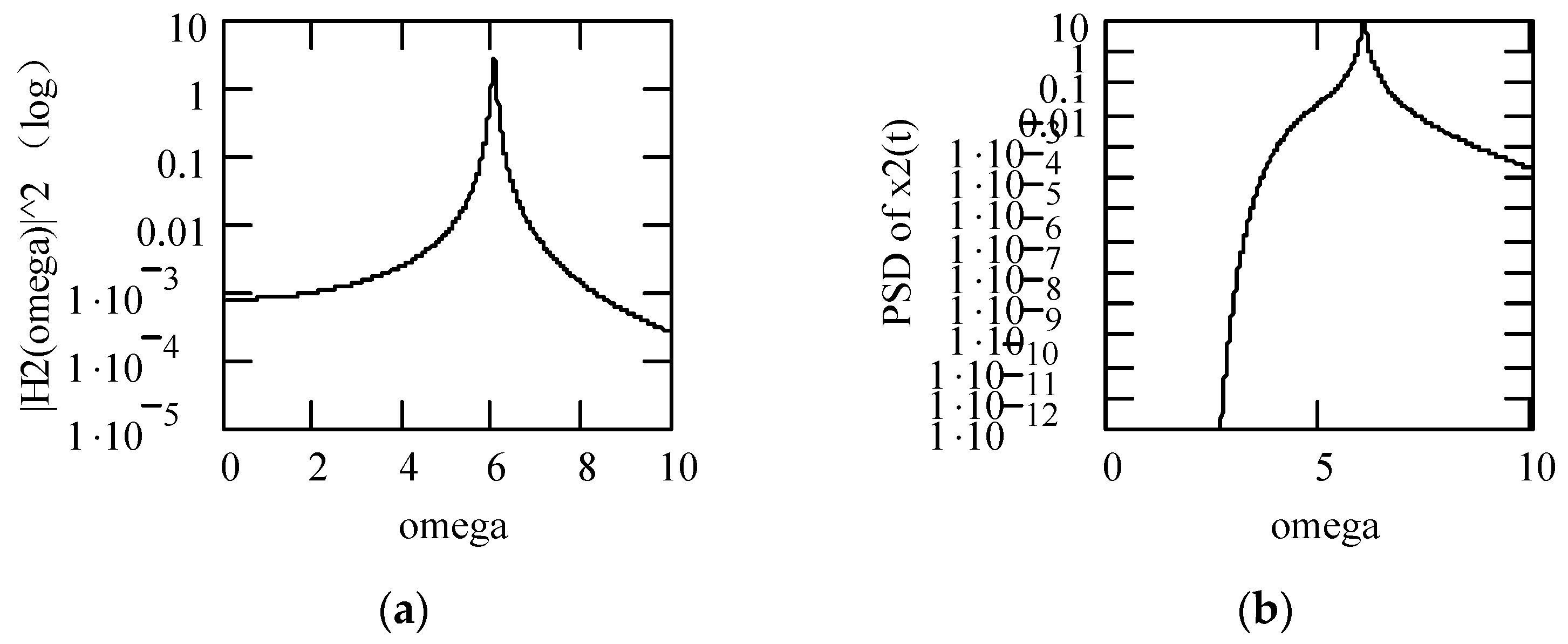
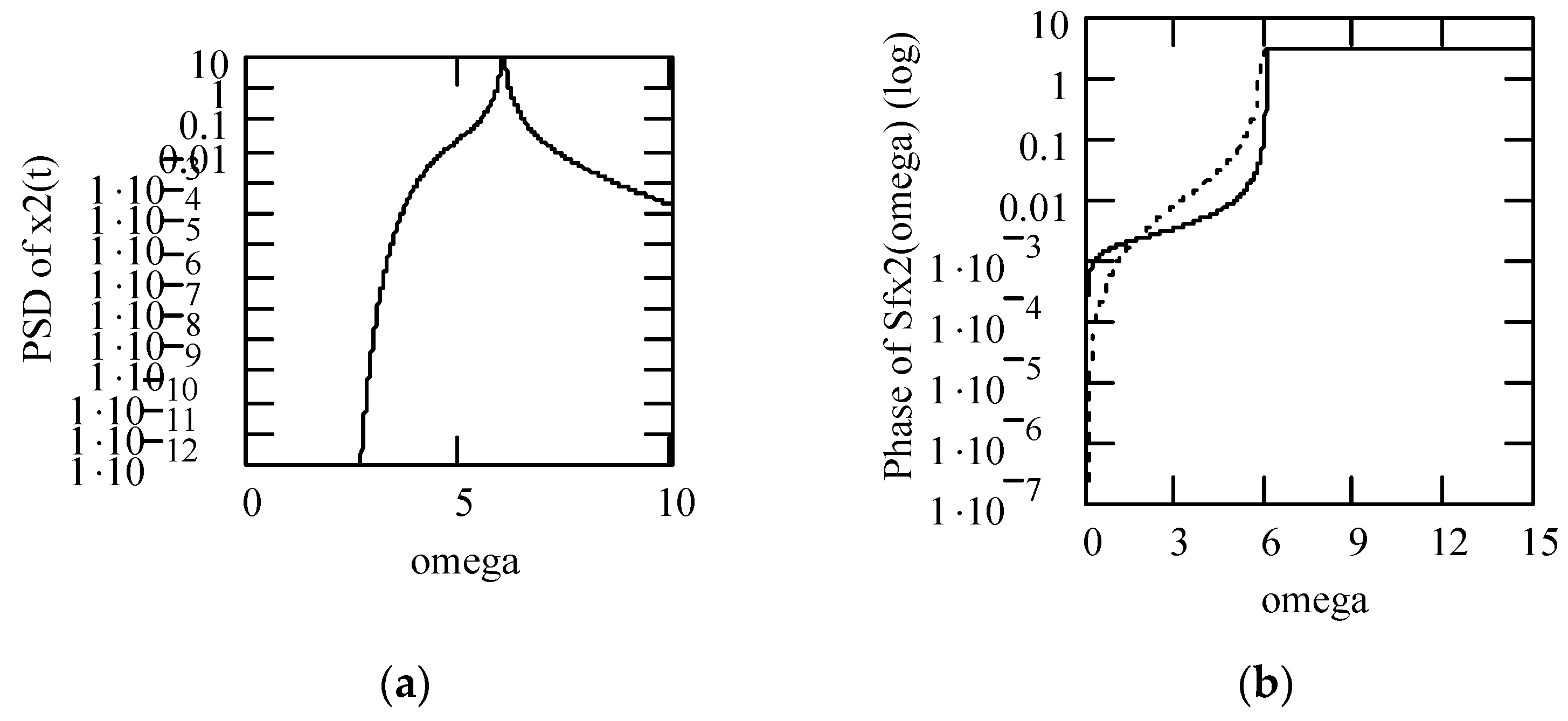
10.3. Responses of Class II Fractional Vibrators Driven by P-M Spectrum
10.3.1. Computation Methods
10.3.2. Effects of β on Responses
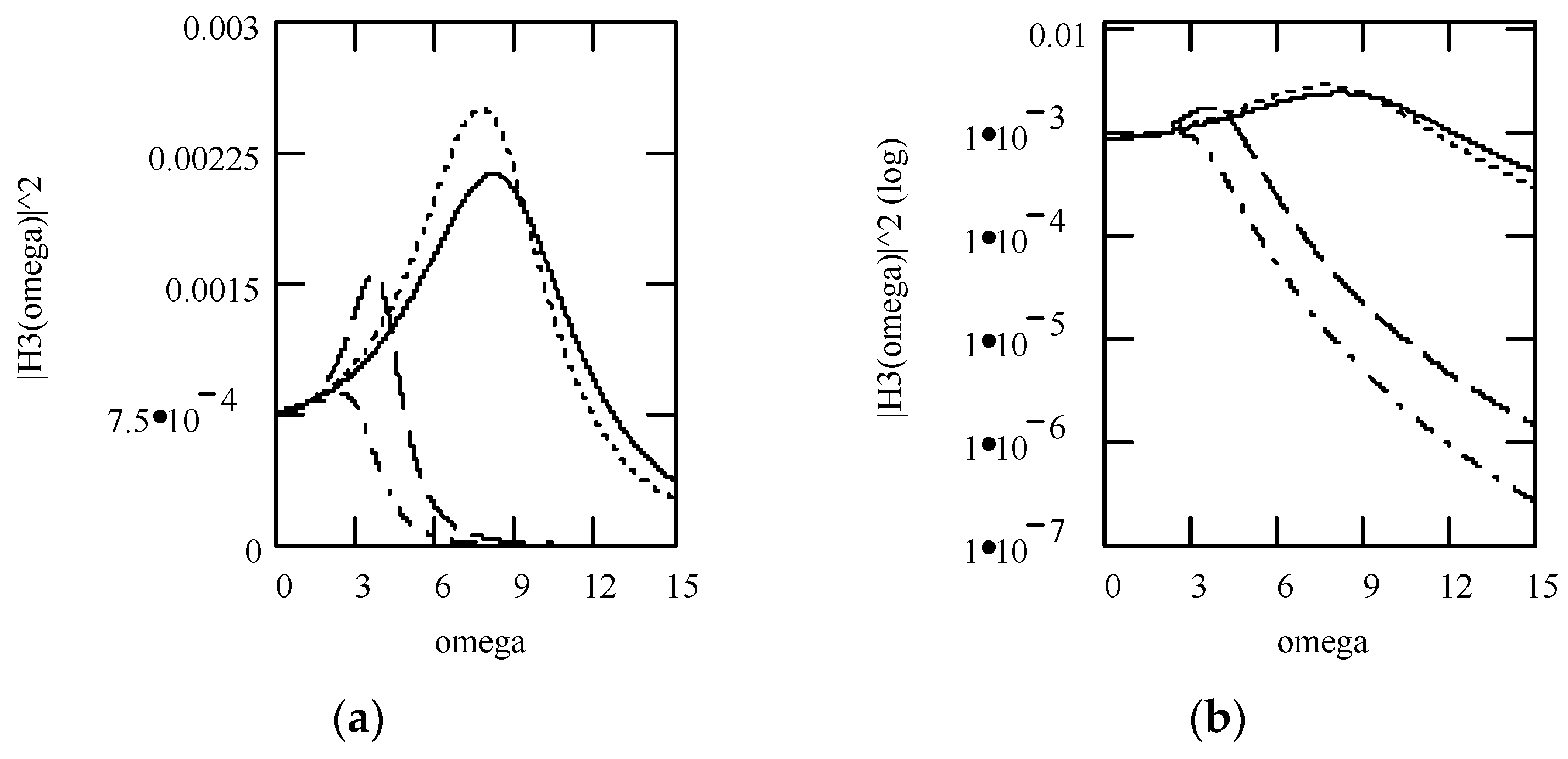
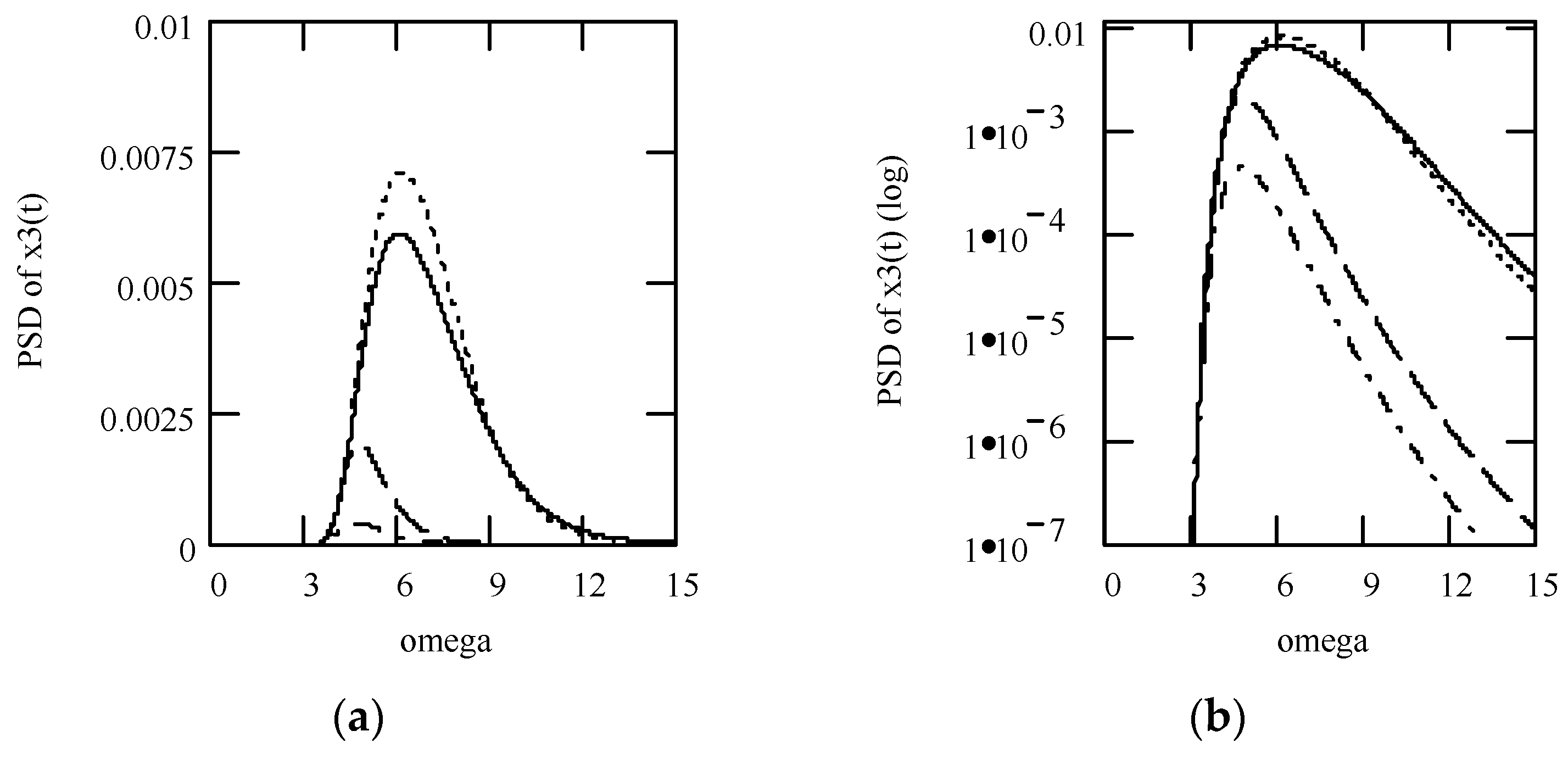
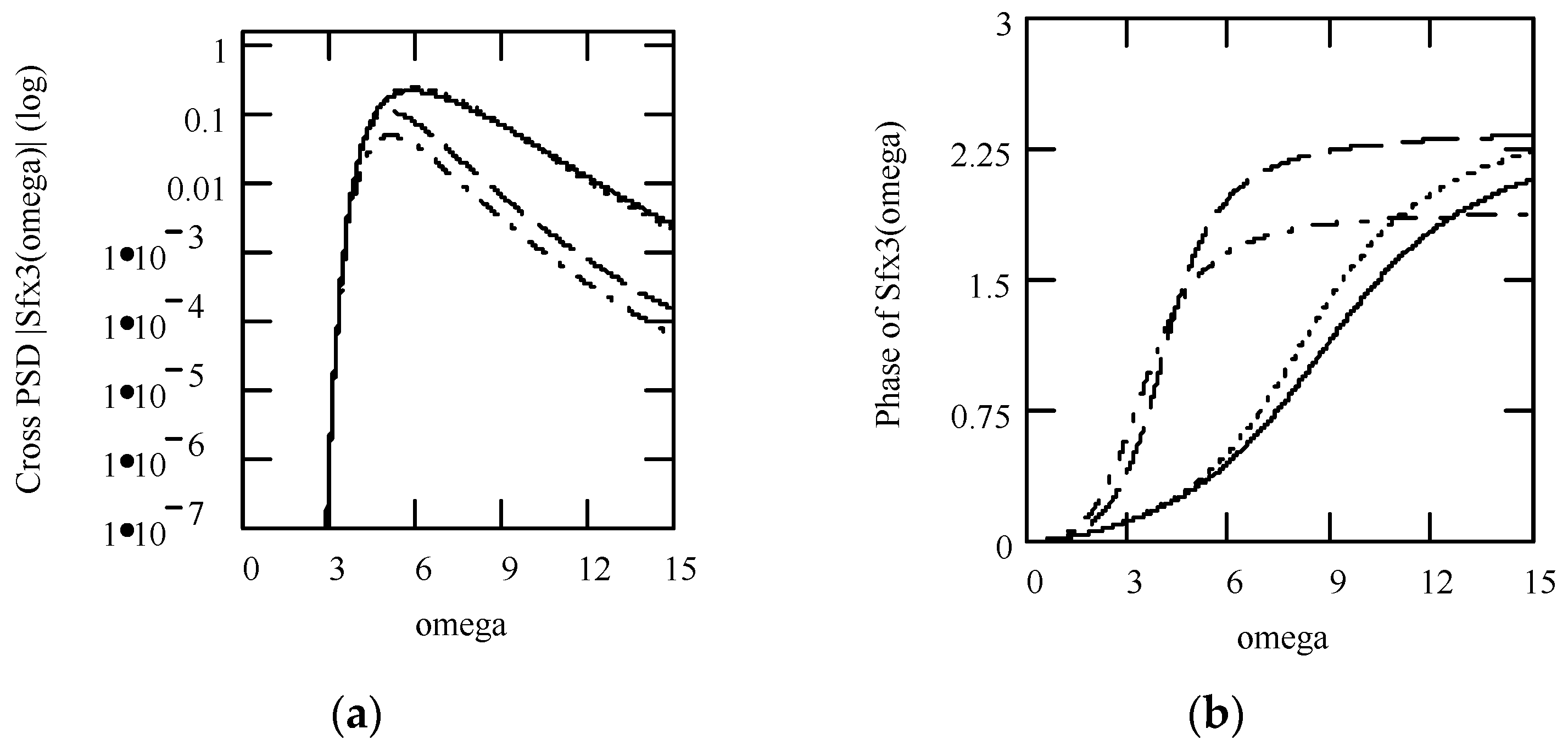
10.4. Responses of Class III Fractional Vibrators Driven by P-M Spectrum
10.4.1. Computations
10.4.2. Effects of α and β on Responses
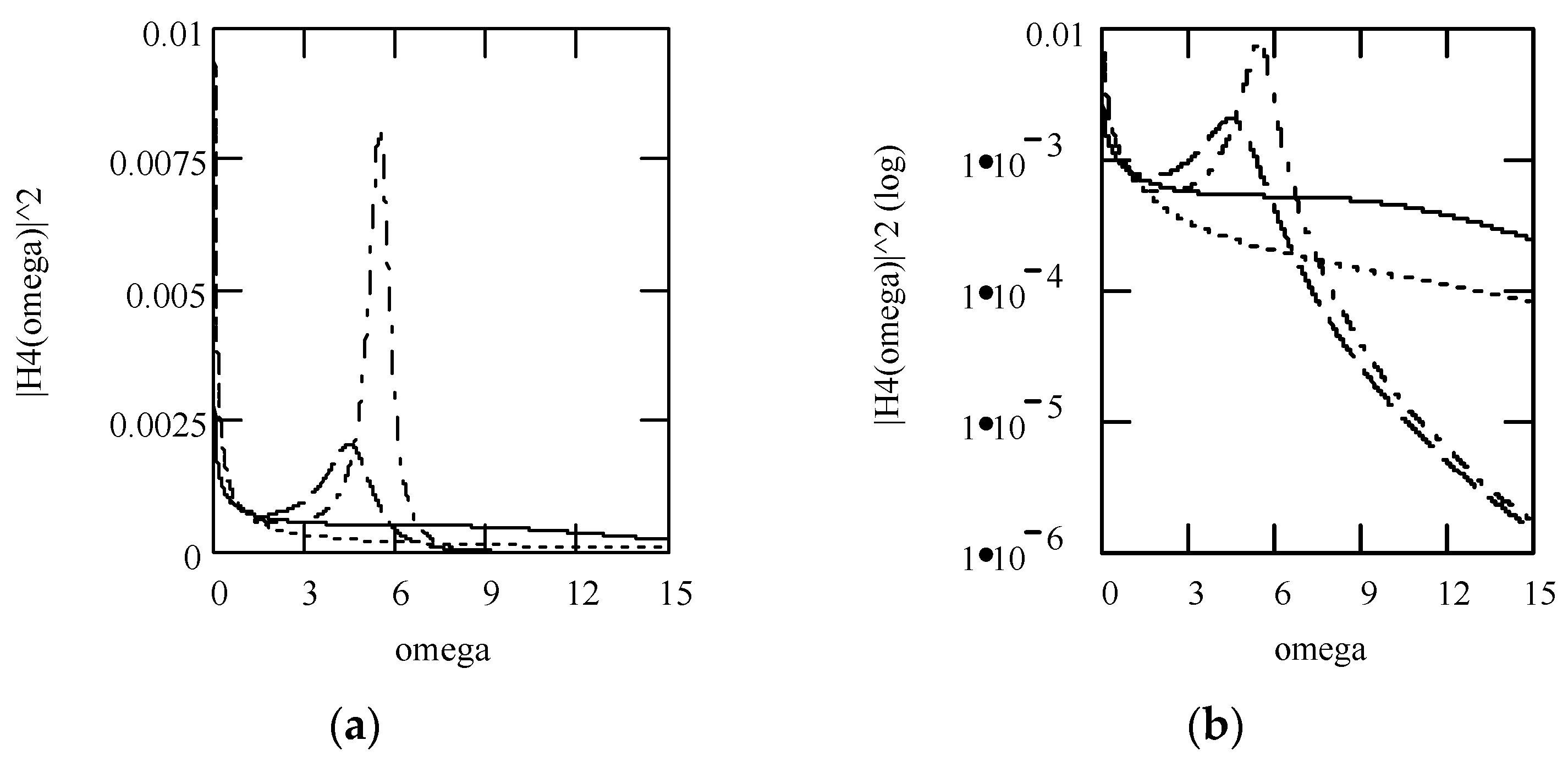
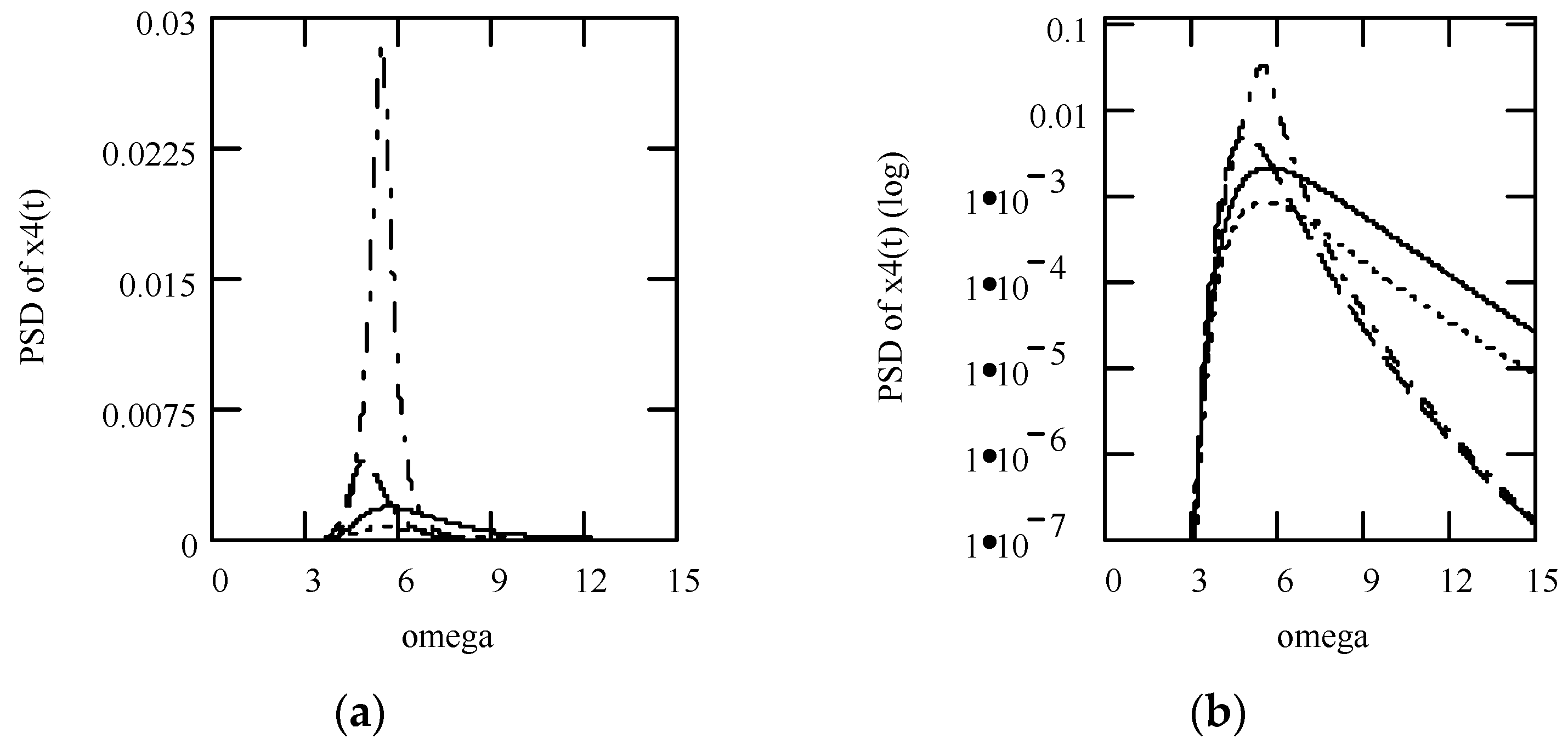
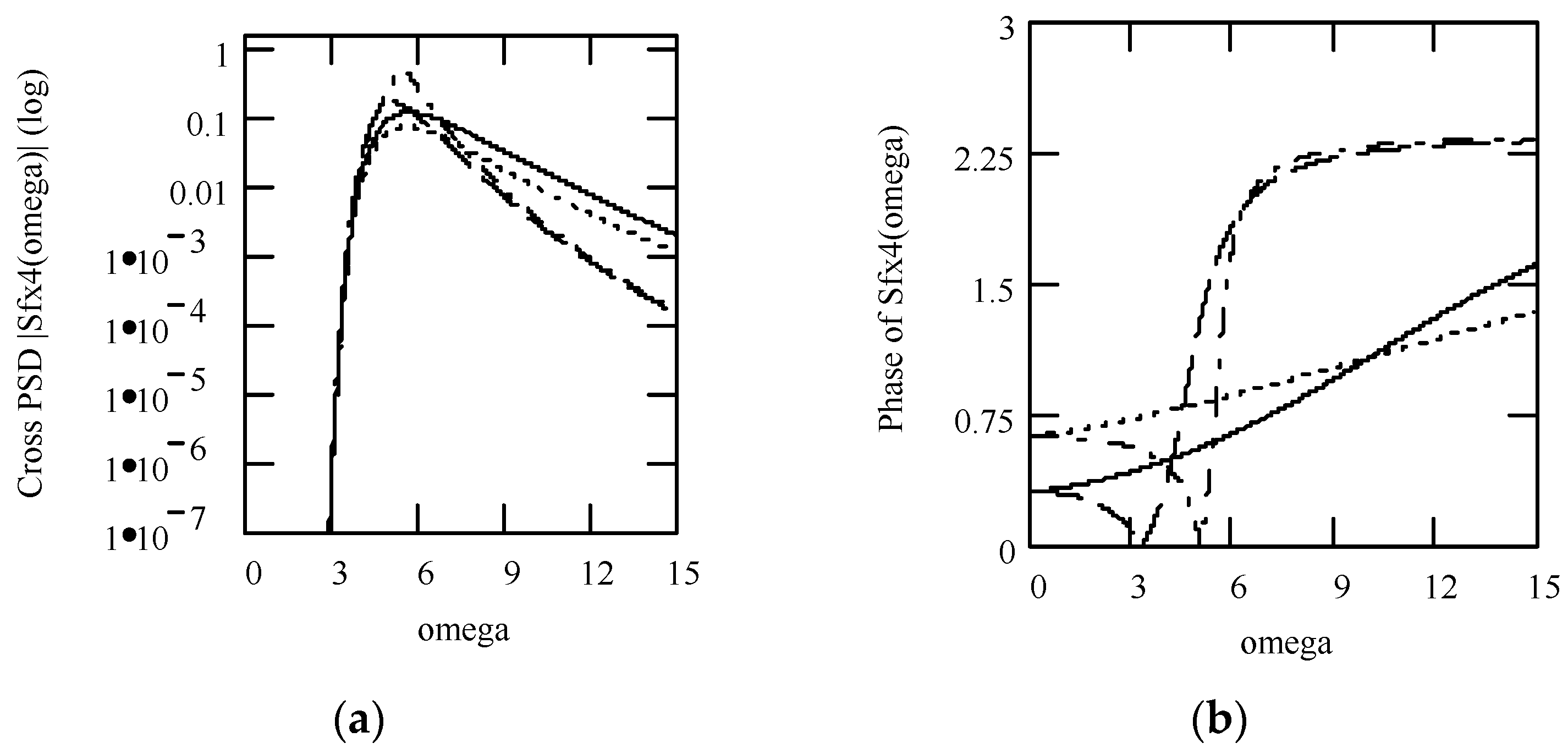
10.5. Responses of Class IV Fractional Vibrator Driven by P-M Spectrum
10.5.1. Computations
10.5.2. Effects of α and λ on Responses
10.6. Responses of Class V Fractional Vibrators Driven by P-M Spectrum
10.6.1. Computations
10.6.2. Effects of λ on Responses
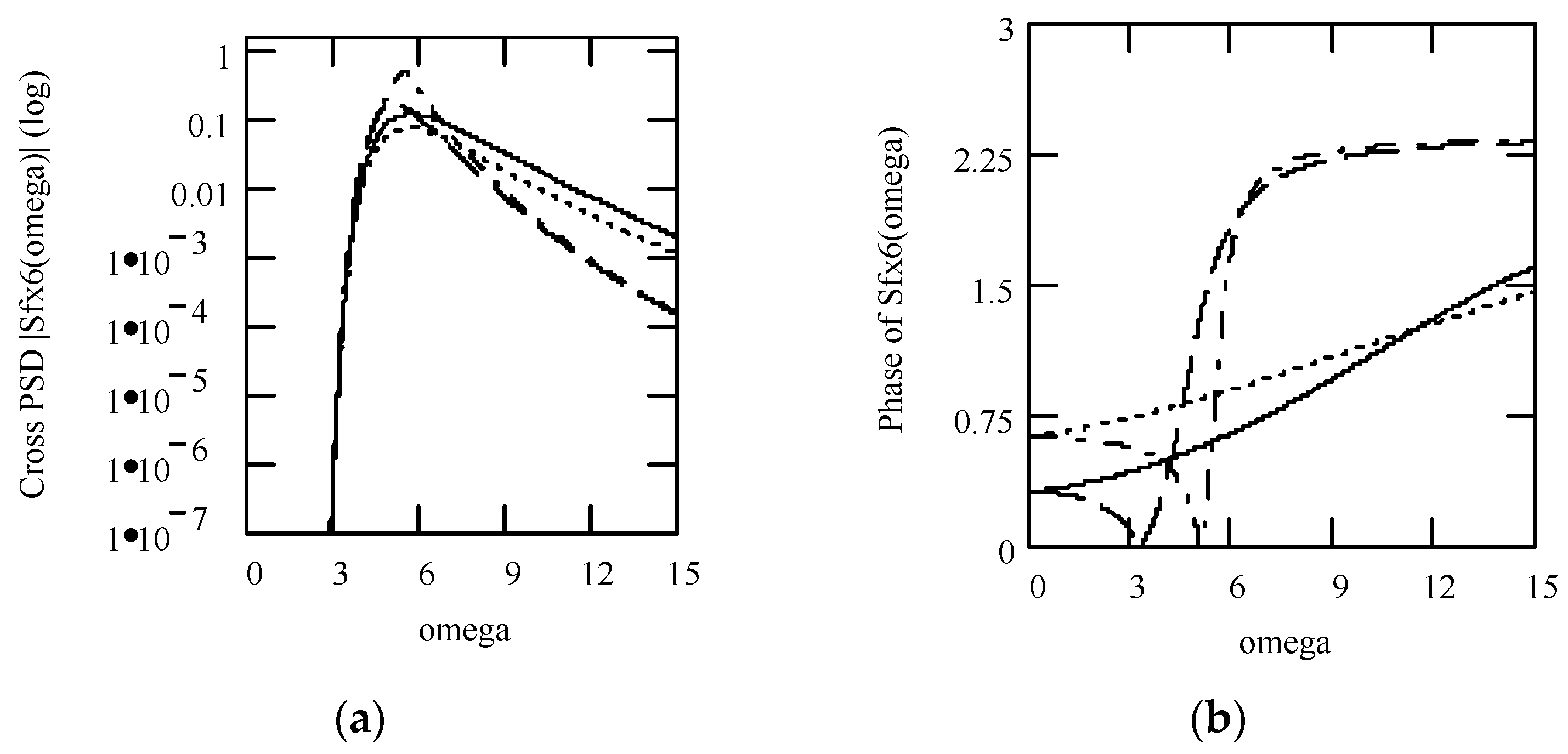
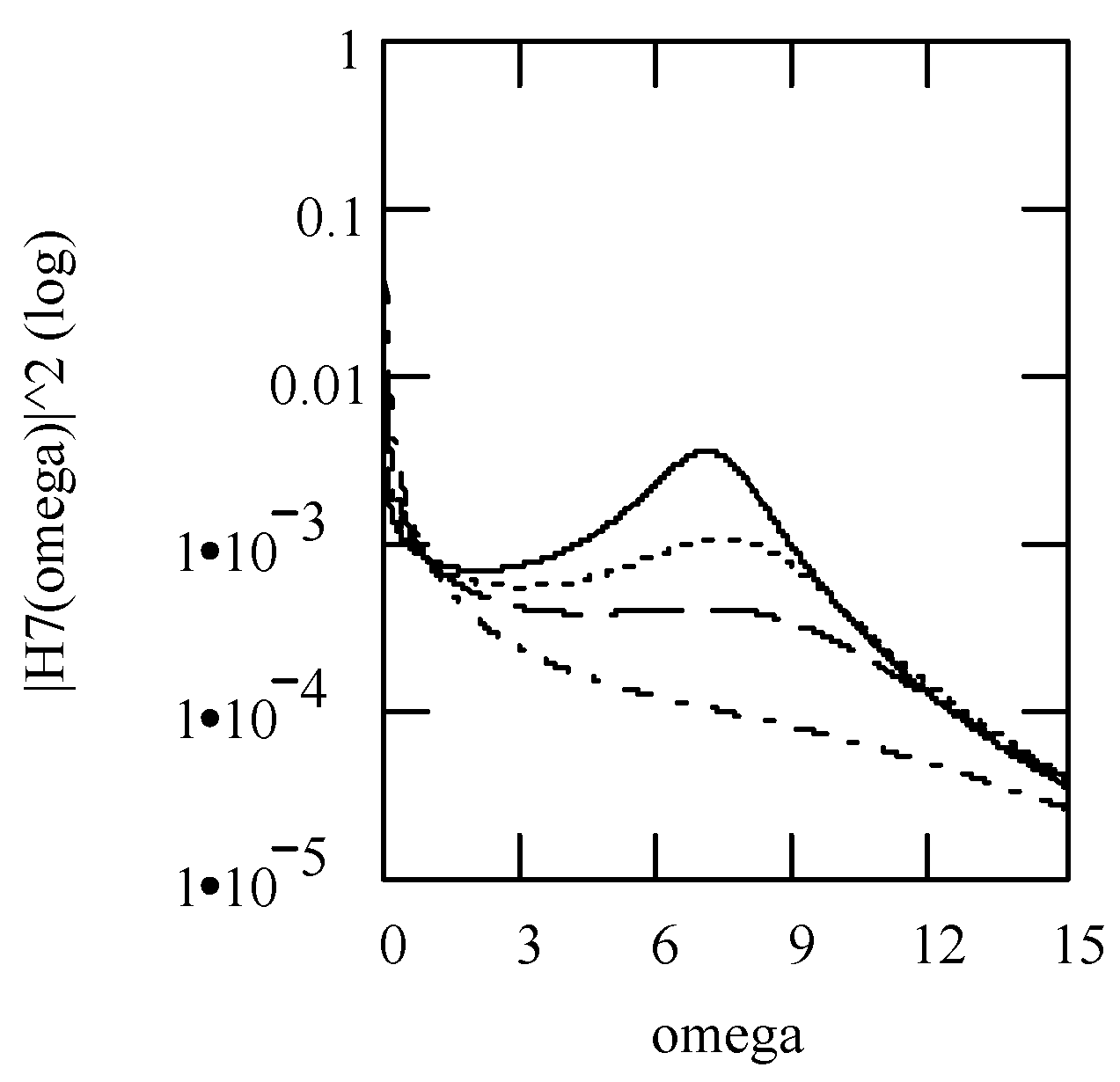
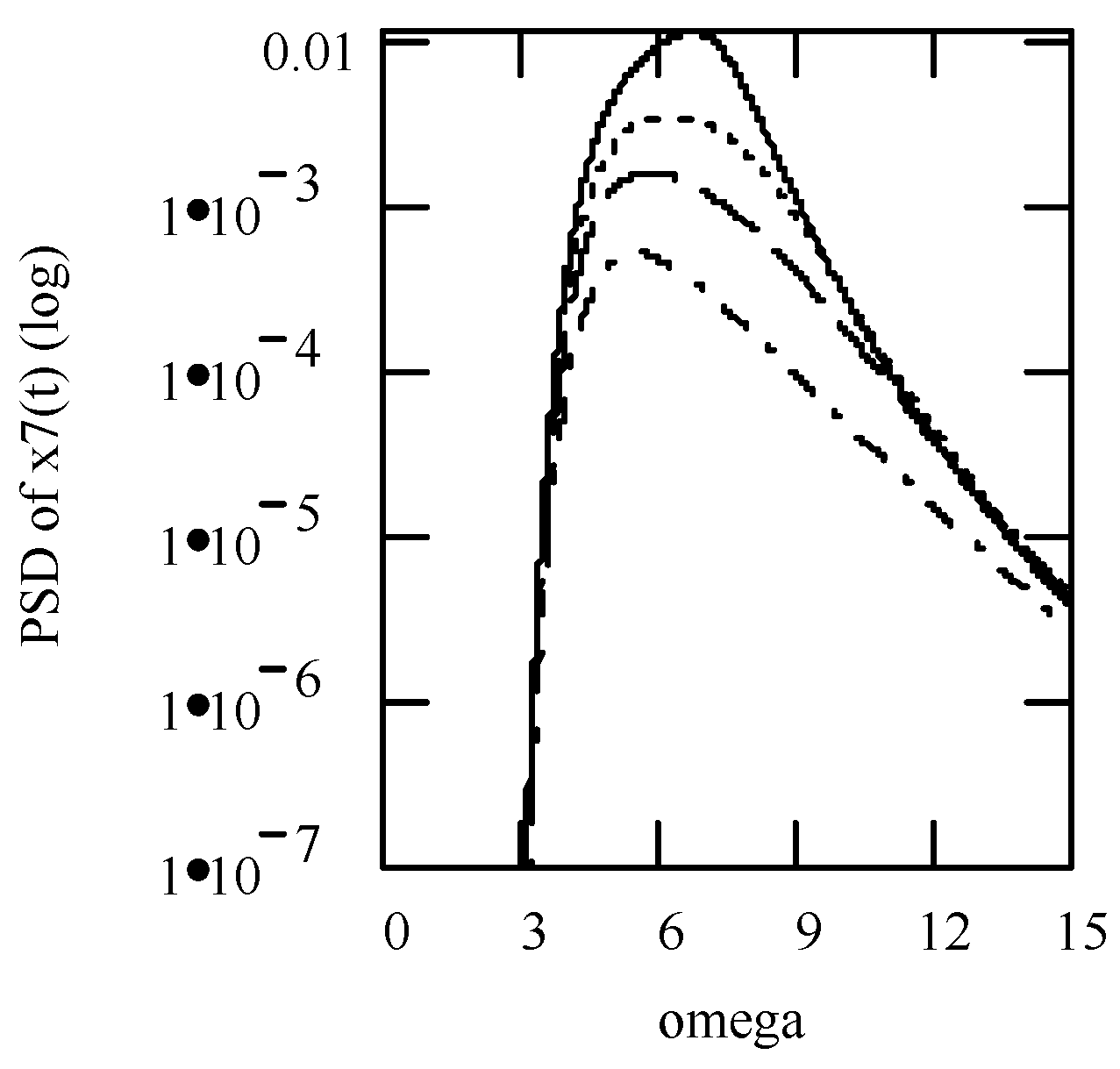
10.7. Responses of Class VI Fractional Vibrators Driven by P-M Spectrum
10.7.1. Computations
10.7.2. Effects of α, β, and λ on Responses
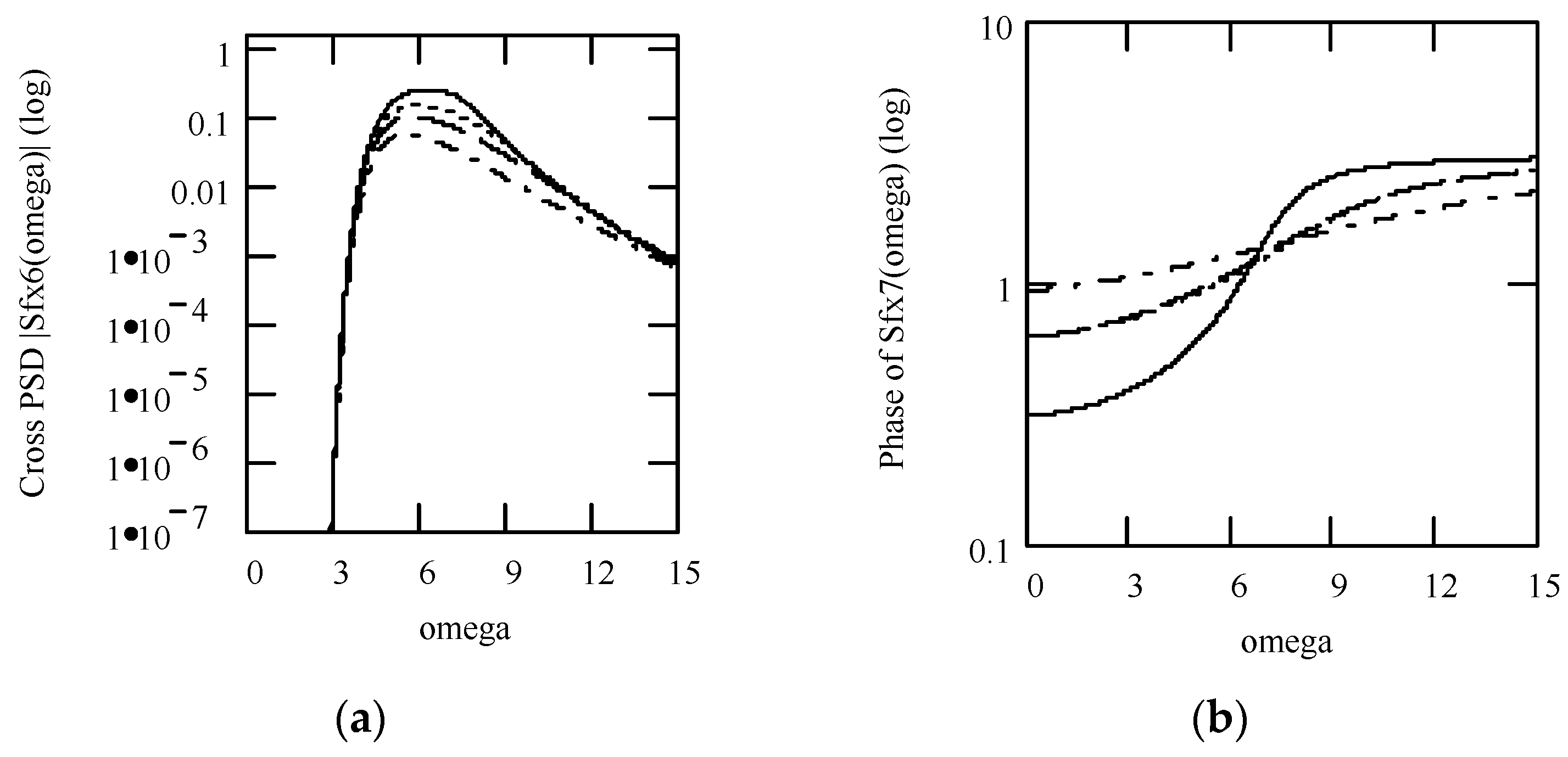
10.8. Responses of Class VII Fractional Vibrators Driven by P-M Spectrum
10.8.1. Computations
10.8.2. Effects of β and λ on Responses
10.9. Summary
11. Application: Mathematical Explanation of Rayleigh Damping Assumption
11.1. Mathematical Explanation
11.2. Frequency Dependency of Elements: A View from Rayleigh Damping Assumption
12. Summary and Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Duan, J.-S. The periodic solution of fractional oscillation equation with periodic input. Adv. Math. Phys. 2013, 2013, 869484. [Google Scholar] [CrossRef]
- Duan, J.-S.; Wang, Z.; Liu, Y.-L.; Qiu, X. Eigenvalue problems for fractional ordinary differential equations. Chaos Solitons Fractals 2013, 46, 46–53. [Google Scholar] [CrossRef]
- Zurigat, M. Solving fractional oscillators using Laplace homotopy analysis method. Ann. Univ. Craiova Math. Comput. Sci. Ser. 2011, 38, 1–11. [Google Scholar]
- Blaszczyk, T.; Ciesielski, M. Fractional oscillator equation–Transformation into integral equation and numerical solution. Appl. Math. Comput. 2015, 257, 428–435. [Google Scholar] [CrossRef]
- Blaszczyk, T.; Ciesielski, M.; Klimek, M.; Leszczynski, J. Numerical solution of fractional oscillator equation. Appl. Math. Comput. 2011, 218, 2480–2488. [Google Scholar]
- Blaszczyk, T. A numerical solution of a fractional oscillator equation in a non-resisting medium with natural boundary conditions. Rom. Rep. Phys. 2015, 67, 350–358. [Google Scholar]
- Al-Rabtah, A.; Ertürk, V.S.; Momani, S. Solutions of a fractional oscillator by using differential transform method. Comput. Math. Appl. 2010, 59, 1356–1362. [Google Scholar]
- Drozdov, A.D. Fractional oscillator driven by a Gaussian noise. Phys. A 2007, 376, 237–245. [Google Scholar] [CrossRef]
- Stanislavsky, A.A. Fractional oscillator. Phys. Rev. E 2004, 70, 051103. [Google Scholar]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Damping characteristics of a fractional oscillator. Phys. A 2004, 339, 311–319. [Google Scholar]
- Achar, B.N.N.; Hanneken, J.W.; Clarke, T. Response characteristics of a fractional oscillator. Phys. A 2002, 309, 275–288. [Google Scholar] [CrossRef]
- Achar, B.N.N.; Hanneken, J.W.; Enck, T.; Clarke, T. Dynamics of the fractional oscillator. Phys. A 2001, 297, 361–367. [Google Scholar] [CrossRef]
- Tofighi, A. The intrinsic damping of the fractional oscillator. Phys. A 2003, 329, 29–34. [Google Scholar] [CrossRef]
- Ryabov, Y.E.; Puzenko, A. Damped oscillations in view of the fractional oscillator equation. Phys. Rev. B 2002, 66, 184201. [Google Scholar] [CrossRef]
- Ahmad, W.E.; Elwakil, A.S.R. Fractional-order Wien-bridge oscillator. Electron. Lett. 2001, 37, 1110–1112. [Google Scholar] [CrossRef]
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Springer: Berlin/Heidelberg, Germany, 2013; Volume II. [Google Scholar]
- Tavazoei, M.S. Reduction of oscillations via fractional order pre-filtering. Signal Process. 2015, 107, 407–414. [Google Scholar] [CrossRef]
- Sandev, T.; Tomovski, Z. The general time fractional wave equation for a vibrating string. J. Phys. A Math. Theor. 2010, 43, 055204. [Google Scholar] [CrossRef]
- Singh, H.; Srivastava, H.M.; Kumar, D. A reliable numerical algorithm for the fractional vibration equation. Chaos Solitons Fractals 2017, 103, 131–138. [Google Scholar] [CrossRef]
- Rossikhin, Y.A. Reflections on two parallel ways in progress of fractional calculus in mechanics of solids. Appl. Mech. Rev. 2010, 63, 010701. [Google Scholar] [CrossRef]
- Pskhu, A.V.; Rekhviashvili, S.S. Analysis of forced oscillations of a fractional oscillator. Tech. Phys. Lett. 2018, 44, 1218–1221. [Google Scholar] [CrossRef]
- Aghchi, S.; Fazli, H.; Sun, H.G. A numerical approach for solving optimal control problem of fractional order vibration equation of large membranes. Comput. Math. Appl. 2024, 165, 19–27. [Google Scholar] [CrossRef]
- Čermák, J.; Kisela, T. Stabilization and destabilization of fractional oscillators via a delayed feedback control. Commun. Nonlinear Sci. Numer. Simul. 2023, 117, 106960. [Google Scholar] [CrossRef]
- Li, M. Three classes of fractional oscillators. Symmetry 2018, 10, 40. [Google Scholar] [CrossRef]
- Li, M. Theory of Fractional Engineering Vibrations; Walter de Gruyter: Berlin, Germany; Boston, MA, USA, 2021. [Google Scholar]
- Li, M. Fractional Vibrations with Applications to Euler-Bernoulli Beams; CRC Press: Boca Raton, UK, 2023. [Google Scholar]
- Lin, L.-F.; Chen, C.; Zhong, S.-C.; Wang, H.-Q. Stochastic resonance in a fractional oscillator with random mass and random frequency. J. Stat. Phys. 2015, 160, 497–511. [Google Scholar] [CrossRef]
- Hermosillo-Arteaga, A.; Romo, M.P.; Magaña-del-Toro, R. Response spectra generation using a fractional differential model. Soil Dyn. Earthq. Eng. 2018, 115, 719–729. [Google Scholar] [CrossRef]
- Alkhaldi, H.S.; Abu-Alshaikh, I.M.; Al-Rabadi, A.N. Vibration control of fractionally-damped beam subjected to a moving vehicle and attached to fractionally-damped multi-absorbers. Adv. Math. Phys. 2013, 2013, 232160. [Google Scholar] [CrossRef]
- Dai, H.; Zheng, Z.; Wang, W. On generalized fractional vibration equation. Chaos Solitons Fractals 2017, 95, 48–51. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Y.; Liu, D.; Jia, W.; Huang, H. Responses of Duffing oscillator with fractional damping and random phase. Nonlinear Dyn. 2013, 74, 745–753. [Google Scholar] [CrossRef]
- He, G.; Tian, Y.; Wang, Y. Stochastic resonance in a fractional oscillator with random damping strength and random spring stiffness. J. Stat. Mech. Theory Exp. 2013, 2013, P09026. [Google Scholar] [CrossRef]
- Leung, A.Y.T.; Guo, Z.; Yang, H.X. Fractional derivative and time delay damper characteristics in Duffing-van der Pol oscillators. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 2900–2915. [Google Scholar] [CrossRef]
- Chen, L.C.; Zhuang, Q.Q.; Zhu, W.Q. Response of SDOF nonlinear oscillators with lightly fractional derivative damping under real noise excitations. Eur. Phys. J. Spec. Top. 2011, 193, 81–92. [Google Scholar] [CrossRef]
- Deü, J.-F.; Matignon, D. Simulation of fractionally damped mechanical systems by means of a Newmark-diffusive scheme. Comput. Math. Appl. 2010, 59, 1745–1753. [Google Scholar] [CrossRef]
- Drăgănescu, G.E.; Bereteu, L.; Ercuţa, A.; Luca, G. Anharmonic vibrations of a nano-sized oscillator with fractional damping. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 922–926. [Google Scholar] [CrossRef]
- Xie, F.; Lin, X. Asymptotic solution of the van der Pol oscillator with small fractional damping. Phys. Scr. 2009, 2009, 014033. [Google Scholar] [CrossRef]
- Ren, R.; Luo, M.; Deng, K. Stochastic resonance in a fractional oscillator driven by multiplicative quadratic noise. J. Stat. Mech. Theory Exp. 2017, 2017, 023210. [Google Scholar] [CrossRef]
- Ren, R.; Luo, M.; Deng, K. Stochastic resonance in a fractional oscillator subjected to multiplicative trichotomous noise. Nonlinear Dyn. 2017, 90, 379–390. [Google Scholar] [CrossRef]
- Yuan, J.; Zhang, Y.; Liu, J.; Shi, B.; Gai, M.; Yang, S. Mechanical energy and equivalent differential equations of motion for single-degree-of-freedom fractional oscillators. J. Sound Vib. 2017, 397, 192–203. [Google Scholar] [CrossRef]
- Shitikova, M.V. The fractional derivative expansion method in nonlinear dynamic analysis of structures. Nonlinear Dyn. 2020, 99, 109–122. [Google Scholar] [CrossRef]
- Lin, L.-F.; Chen, C.; Wang, H.-Q. Trichotomous noise induced stochastic resonance in a fractional oscillator with random damping and random frequency. J. Stat. Mech. Theory Exp. 2016, 2016, 023201. [Google Scholar] [CrossRef]
- Naranjani, Y.; Sardahi, Y.; Chen, Y.-Q.; Sun, J.-Q. Multi-objective optimization of distributed-order fractional damping. Commun. Nonlinear Sci. Numer. Simul. 2015, 24, 159–168. [Google Scholar] [CrossRef]
- Duan, J.S.; Zhang, Y.Y. Discriminant and root trajectories of characteristic equation of fractional vibration equation and their effects on solution components. Fractal Fract. 2022, 6, 514. [Google Scholar] [CrossRef]
- Di Matteo, A.; Spanos, P.D.; Pirrotta, A. Approximate survival probability determination of hysteretic systems with fractional derivative elements. Probabilistic Eng. Mech. 2018, 54, 138–146. [Google Scholar] [CrossRef]
- Tomovski, Ž.; Sandev, T. Effects of a fractional friction with power-law memory kernel on string vibrations. Comput. Math. Appl. 2011, 62, 1554–1561. [Google Scholar] [CrossRef]
- Zelenev, V.M.; Meshkov, S.I.; Rossikhin, Y.A. Damped vibrations of hereditary-elastic systems with weakly singular kernels. J. Appl. Mech. Tech. Phys. 1970, 11, 290–293. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. New approach for the analysis of damped vibrations of fractional oscillators. Shock. Vib. 2009, 16, 365–387. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Applications of fractional calculus to dynamic problems of linear and nonlinear hereditary mechanics of solids. Appl. Mech. Rev. 1997, 50, 15–67. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Application of fractional calculus for dynamic problems of solid mechanics: Novel trends and recent results. Appl. Mech. Rev. 2010, 63, 010801. [Google Scholar] [CrossRef]
- Bagley, R.; Torvik, P.J. A generalized derivative model for an elastomer damper. Shock Vibr. Bull. 1979, 49, 135–143. [Google Scholar]
- Spanos, P.D.; Malara, G. Nonlinear random vibrations of beams with fractional derivative elements. J. Eng. Mech. 2014, 140, 04014069. [Google Scholar] [CrossRef]
- Spanos, P.D.; Malara, G. Nonlinear vibrations of beams and plates with fractional derivative elements subject to combined harmonic and random excitations. Probabilist. Eng. Mech. 2020, 59, 103043. [Google Scholar] [CrossRef]
- Hu, R.C.; Zhang, D.X.; Deng, Z.C.; Xu, C.H. Stochastic analysis of a nonlinear energy harvester with fractional derivative damping. Nonlinear Dyn. 2022, 108, 1973–1986. [Google Scholar] [CrossRef]
- Cao, Q.Y.; Hu, S.-L.J.; Li, H.J. Frequency/Laplace domain methods for computing transient responses of fractional oscillators. Nonlinear Dyn. 2022, 108, 1509–1523. [Google Scholar] [CrossRef]
- Cao, Q.Y.; Hu, S.-L.J.; Li, H.J. Nonstationary response statistics of fractional oscillators to evolutionary stochastic excitation. Commun. Nonlinear Sci. Numer. Simul. 2021, 103, 105962. [Google Scholar] [CrossRef]
- Kaltenbacher, B.; Schlintl, A. Fractional time stepping and adjoint based gradient computation in an inverse problem for a fractionally damped wave equation. J. Comput. Phys. 2022, 449, 110789. [Google Scholar] [CrossRef]
- Pang, D.H.; Jiang, W.; Liu, S.; Jun, D. Stability analysis for a single degree of freedom fractional oscillator. Phys. A 2019, 523, 498–506. [Google Scholar] [CrossRef]
- Gomez-Aguilar, J.F.; Rosales-Garcia, J.J.; Bernal-Alvarado, J.J.; Cordova-Fraga, T.; Guzman-Cabrera, R. Fractional mechanical oscillators. Rev. Mex. Fis. 2012, 58, 348–352. [Google Scholar]
- Tian, Y.; Zhong, L.-F.; He, G.-T.; Yu, T.; Luo, M.-K.; Stanley, H.E. The resonant behavior in the oscillator with double fractional-order damping under the action of nonlinear multiplicative noise. Phys. A 2018, 490, 845–856. [Google Scholar] [CrossRef]
- Berman, M.; Cederbaum, L.S. Fractional driven-damped oscillator and its general closed form exact solution. Phys. A 2018, 505, 744–762. [Google Scholar] [CrossRef]
- Duan, J.-S.; Li, M.; Wang, Y.; An, Y.-L. Approximate solution of fractional differential equation by quadratic splines. Fractal Fract. 2022, 6, 369. [Google Scholar] [CrossRef]
- Mendiola-Fuentes, J.; Guerrero-Ruiz, E.; Rosales-García, J. Multivariate Mittag-Leffler solution for a forced fractional-order harmonic oscillator. Mathematics 2024, 12, 1502. [Google Scholar] [CrossRef]
- Morales-Delgado, V.F.; Gómez-Aguilar, J.F.; Taneco-Hernández, M.A.; Escobar-Jiménez, R.F. A novel fractional derivative with variable- and constant-order applied to a mass-spring-damper system. Eur. Phys. J. Plus 2018, 133, 78. [Google Scholar] [CrossRef]
- Parovik, R.I. Amplitude-frequency and phase-frequency performances of forced oscillations of a nonlinear fractional oscillator. Tech. Phys. Lett. 2019, 45, 660–663. [Google Scholar] [CrossRef]
- Parovik, R.I. Quality factor of forced oscillations of a linear fractional oscillator. Tech. Phys. 2020, 65, 1015–1019. [Google Scholar] [CrossRef]
- Sene, N.; Aguilar, J.F.G. Fractional mass-spring-damper system described by generalized fractional order derivatives. Fractal Fract. 2019, 3, 39. [Google Scholar] [CrossRef]
- Duan, S.; Zhang, Y.; Qiu, X. Exact solutions of fractional order oscillation equation with two fractional derivative terms. J. Nonlinear Math. Phys. 2023, 30, 531–552. [Google Scholar] [CrossRef]
- Li, M. PSD and cross PSD of responses of seven classes of fractional vibrations driven by fGn, fBm, fractional OU process, and von Kármán process. Symmetry 2024, 16, 635. [Google Scholar] [CrossRef]
- Duan, J.-S. A modified fractional derivative and its application to fractional vibration equation. Appl. Math. Inf. Sci. 2016, 10, 1863–1869. [Google Scholar] [CrossRef]
- Harris, C.M. Shock and Vibration Handbook, 5th ed.; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Palley, O.M.; Bahizov, B.; Voroneysk, E.R. Handbook of Ship Structural Mechanics; Xu, B.H.; Xu, X.; Xu, M.Q., Translators; National Defense Industry Publishing House: Beijing, China, 2002. (In Chinese) [Google Scholar]
- Lewis, E.V. (Ed.) Vol. II: Resistance, Propulsions and Vibration. In Principles of Naval Architecture, 2nd rev. ed.; The Society of Naval Architects and Marine Engineers (SNAME): Alexandria, VA, USA, 1988. [Google Scholar]
- Ouzizi, A.; Abdoun, F.; Azrar, L. Nonlinear dynamics of beams on nonlinear fractional viscoelastic foundation subjected to moving load with variable speed. J. Sound Vib. 2022, 523, 116730. [Google Scholar] [CrossRef]
- Lewandowski, R.; Baum, M. Dynamic characteristics of multilayered beams with viscoelastic layers described by the fractional Zener model. Arch. Appl. Mech. 2015, 82, 1793–1824. [Google Scholar] [CrossRef]
- Nešić, N.; Cajić, M.; Karličić, D.; Obradović, A.; Simonović, J. Nonlinear vibration of a nonlocal functionally graded beam on fractional visco-Pasternak foundation. Nonlinear Dyn. 2022, 107, 2003–2026. [Google Scholar] [CrossRef]
- Rossikhin, Y.A.; Shitikova, M.V. Classical beams and plates in a fractional derivative medium, Impact response. In Encyclopedia of Continuum Mechanics; Springer: Berlin/Heidelberg, Germany, 2020; Volume 1, pp. 294–305. [Google Scholar]
- Freundlich, J. Vibrations of a simply supported beam with a fractional derivative viscoelastic material model-supports movement excitation. Shock. Vib. 2013, 20, 126735. [Google Scholar]
- Freundlich, J. Transient vibrations of a fractional Kelvin-Voigt viscoelastic cantilever beam with a tip mass and subjected to a base excitation. J. Sound Vib. 2019, 438, 99–115. [Google Scholar] [CrossRef]
- Freundlich, J. Dynamic response of a simply supported viscoelastic beam of a fractional derivative type to a moving force load. J. Theor. Appl. Mech. 2016, 54, 1433–1445. [Google Scholar] [CrossRef][Green Version]
- Crandall, S.H.; Mark, W.D. Random Vibration in Mechanical Systems; Elsevier Inc.: New York, NY, USA; London, UK, 1963. [Google Scholar]
- Nakagawa, K.; Ringo, M. Engineering Vibrations; Xia, S.R., Translator; Shanghai Science and Technology Publishing House: Shanghai, China, 1981. (In Chinese) [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock, 2nd ed.; John Wiley & Sons: London, UK, 2009; Volume 3. [Google Scholar]
- Nigam, N.C. Introduction to Random Vibrations; The MIT Press: Cambridge, MA, USA, 1983. [Google Scholar]
- Preumont, A. Random Vibration and Spectral Analysis; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Preumont, A. Twelve Lectures on Structural Dynamics, Solid Mechanics and Its Applications; Springer: New York, NY, USA, 2013; Volume 198. [Google Scholar]
- Soong, T.T.; Grigoriu, M. Random Vibration of Mechanical and Structural Systems; Prentice-Hall: New York, NY, USA, 1992. [Google Scholar]
- Thomson, W.T.; Dahleh, M.D. Theory of Vibration with Applications, 5th ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1998. [Google Scholar]
- Lutes, L.D.; Sarkani, S.; Vibrations, R. Analysis of Structural and Mechanical Systems; Elsevier: Amsterdam, The Netherlands; Butterworth-Heinemann: Oxford, UK, 2004. [Google Scholar]
- Elishakoff, I.; Lyon, R.H. Random Vibration Status and Recent Developments; Elsevier: New York, NY, USA, 1986. [Google Scholar]
- Jensen, J.J. Load and Global Response of Ships; Elsevier: Amsterdam, The Netherlands; Academic Press: Oxford, UK, 2001; Volume 4. [Google Scholar]
- Rothbart, H.A.; Brown, T.H., Jr. Mechanical Design Handbook, 2nd ed.; Measurement, Analysis and Control of Dynamic Systems; McGraw–Hill: New York, NY, USA, 2006. [Google Scholar]
- Simiu, E.; Scanlan, R.H. Wind Effects on Structures: Fundamentals and Applications to Design, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 1996. [Google Scholar]
- Ra, S.S. Mechanical Vibrations, Sixth Edition in SI Units; Pearson Education: Harlow, UK, 2018. [Google Scholar]
- Dhanak, R.; Xiros, I. Springer Handbook of Ocean Engineering; Springer: Dordrecht, The Netherland; Heidelberg, Germany, 2016. [Google Scholar]
- Findeisen, D. System Dynamics and Mechanical Vibrations, An Introduction; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Mukhopadhyay, M. Structural Dynamics: Vibrations and Systems; Springer: Cham, Switzerland, 2021. [Google Scholar]
- Cheli, F.; Diana, G. Advanced Dynamics of Mechanical Systems; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Lewis, E.V. (Ed.) Vol. III: Motions in Waves and Controllability. In Principles of Naval Architecture, 2nd rev. ed.; The Society of Naval Architects and Marine Engineers (SNAME): Alexandria, VA, USA, 1989. [Google Scholar]
- Massel, S.R. Ocean Surface Waves: Their Physics and Prediction; World Scientific: Singapore, 1997. [Google Scholar]
- Chairabarti, S.K. Offshore Structure Modeling; World Scientific: Singapore, 1994. [Google Scholar]
- Li, M. A method for requiring block size for spectrum measurement of ocean surface waves. IEEE Trans. Instrum. Meas. 2006, 55, 2207–2215. [Google Scholar] [CrossRef]
- The Specialist Committee on Waves. Final Report and Recommendations to the 23rd ITTC. In Proceedings of the 23rd ITTC, Venice, Italy,, 8–14 September 2002; Volume II, pp. 497–543.
- Li, M. Generation of teletraffic of generalized Cauchy type. Phys. Scr. 2010, 81, 025007. [Google Scholar] [CrossRef]
- Strutt, J.W.; Rayleigh, J.W.S. The Theory of Sound. Macmillan & Co., Ltd.: London, UK, 1877; Volume 1. [Google Scholar]
- Trombetti, T.; Silvestri, S. On the modal damping ratios of shear-type structures equipped with Rayleigh damping systems. J. Sound Vib. 2006, 292, 21–58. [Google Scholar] [CrossRef]
- Poul, M.K.; Zerva, A. Efficient time-domain deconvolution of seismic ground motions using the equivalent-linear method for soil-structure interaction analyses. Soil Dyn. Earthq. Eng. 2018, 112, 138–151. [Google Scholar] [CrossRef]
- Nåvik, P.; Rønnquist, A.; Stichel, S. Identification of system damping in railway catenary wire systems from full-scale measurements. Eng. Struct. 2016, 113, 71–78. [Google Scholar] [CrossRef]
- Park, S.-K.; Park, H.W.; Shin, S.; Lee, H.S. Detection of abrupt structural damage induced by an earthquake using a moving time window technique. Comput. Struct. 2008, 86, 1253–1265. [Google Scholar] [CrossRef]
- Hussein, M.I.; Biringen, S.; Bilal, O.R.; Kucala, A. Flow stabilization by subsurface phonons. Proc. Math. Phys. Eng. Sci. 2015, 471, 20140928. [Google Scholar] [CrossRef]
- Sigmund, O.; Jensen, J.S. Systematic design of phononic band-gap materials and structures by topology optimization. Philos. Trans. Math. Phys. Eng. Sci. 2003, 361, 1001–1019. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Tan, H.; Chen, J.; Jiao, Z. Influences of earthquake characteristics on seismic performance of anchored sheet pile quay with barrette piles. J. Coast. Res. 2018, 85, 701–705. [Google Scholar] [CrossRef]
- Battisti, D.S.; Hirst, A.C.; Sarachik, E.S. Instability and predictability in coupled atmosphere-ocean models. Philos. Trans. R. Soc. London. Ser. A Math. Phys. Sci. 1989, 329, 237–247. [Google Scholar]
- Cox, D.; Kämpf, J.; Fernandes, M. Dispersion and connectivity of land-based discharges near the mouth of a coastal inlet. J. Coast. Res. 2013, 29, 100–109. [Google Scholar] [CrossRef][Green Version]
- Tisseur, F.; Meerbergen, K. The quadratic eigenvalue problem. SIAM Rev. 2001, 43, 235–286. [Google Scholar] [CrossRef]
- Chu, E.K.-W.; Huang, T.-M.; Lin, W.-W.; Wu, C.-T. Palindromic eigenvalue problems: A brief survey. Taiwan. J. Math. 2010, 14, 743–779. [Google Scholar] [CrossRef]
- Fay, J.P.; Puria, S.; Steele, C.R. The discordant eardrum. Proc. Natl. Acad. Sci. USA 2006, 103, 19743–19748. [Google Scholar] [CrossRef]
- Naderian, H.; Cheung, M.M.S.; Mohammadian, M.; Dragomirescu, E. Integrated finite strip flutter analysis of bridges. Comput. Struct. 2019, 212, 145–161. [Google Scholar] [CrossRef]
- Tian, Z.; Huo, L.; Gao, W.; Li, H.; Song, G. Modeling of the attenuation of stress waves in concrete based on the Rayleigh damping model using time-reversal and PZT transducers. Smart Mater. Struct. 2017, 26, 105030. [Google Scholar] [CrossRef]
- Iovane, G.; Nasedkin, A.V. Finite element dynamic analysis of anisotropic elastic solids with voids. Comput. Struct. 2009, 87, 981–989. [Google Scholar] [CrossRef]
- Kouris, L.A.S.; Penna, A.; Magenes, G. Seismic damage diagnosis of a masonry building using short-term damping measurements. J. Sound Vib. 2017, 394, 366–391. [Google Scholar] [CrossRef]
- Jin, X.D.; Xia, L.J. Ship Hull Vibration; The Press of Shanghai Jiaotong University: Shanghai, China, 2011. (In Chinese) [Google Scholar]
- DeWeaver, E.; Nigam, S. Influence of mountain ranges on the mid-latitude atmospheric response to El Niño events. Nature 1995, 378, 706–708. [Google Scholar] [CrossRef]
- Langley, R.S. A transfer matrix analysis of the energetics of structural wave motion and harmonic vibration. Proc. Math. Phys. Eng. Sci. 1996, 452, 1631–1648. [Google Scholar]
- Bhaskar, A. Taussky’s theorem, symmetrizability and modal analysis revisited. Proc. Math. Phys. Eng. Sci. 2001, 457, 2455–2480. [Google Scholar] [CrossRef]
- Horiuchi, T.; Konno, T. A new method for compensating actuator delay in real-time hybrid experiments. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2001, 359, 1893–1909. [Google Scholar] [CrossRef]
- Mohammad, D.R.A.; Khan, N.U.; Ramamurti, V. On the role of Rayleigh damping. J. Sound Vib. 1995, 185, 207–218. [Google Scholar] [CrossRef]
- Kim, H.-G.; Wiebe, R. Experimental and numerical investigation of nonlinear dynamics and snap-through boundaries of post-buckled laminated composite plates. J. Sound Vib. 2019, 439, 362–387. [Google Scholar] [CrossRef]
- Li, M. Stationary responses of seven classes of fractional vibrations driven by sinusoidal force. Fractal Fract. 2024, 8, 479. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; Al-Khaled, K.; Darweesh, A. Numeric-analytic solutions for nonlinear oscillators via the modified multi-stage decomposition method. Mathematics 2019, 7, 550. [Google Scholar] [CrossRef]
- Ren, Z.; Chen, J.; Wang, T.; Zhang, Z.; Zhao, P.; Liu, X.; Xie, J. Main sub-harmonic joint resonance of fractional quintic van der Pol-Duffing oscillator. Nonlinear Dyn. 2024, 112, 17863–17880. [Google Scholar] [CrossRef]
- El-Dib, Y.O. Criteria of vibration control in delayed third-order critically damped Duffing oscillation. Arch. Appl. Mech. 2022, 92, 1–19. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A.; Anukool, W. A new approach to nonlinear quartic oscillators. Arch. Appl. Mech. 2022, 92, 351–362. [Google Scholar] [CrossRef]



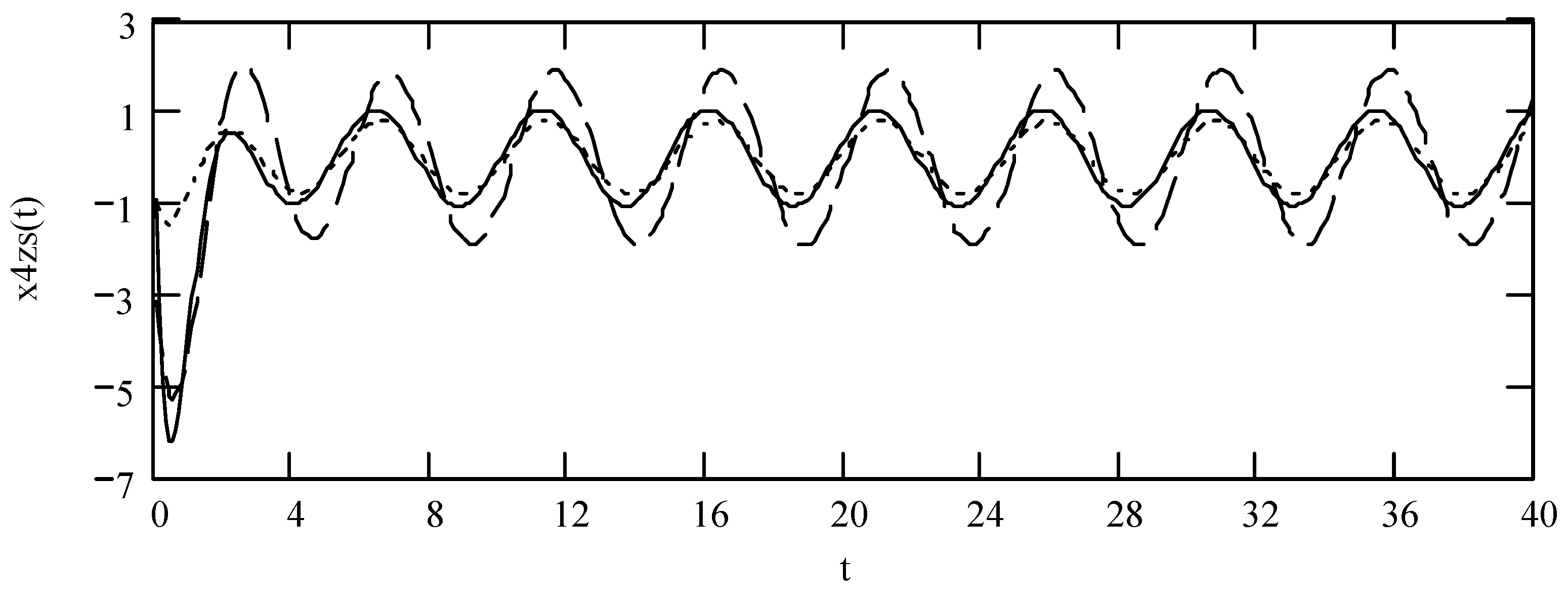
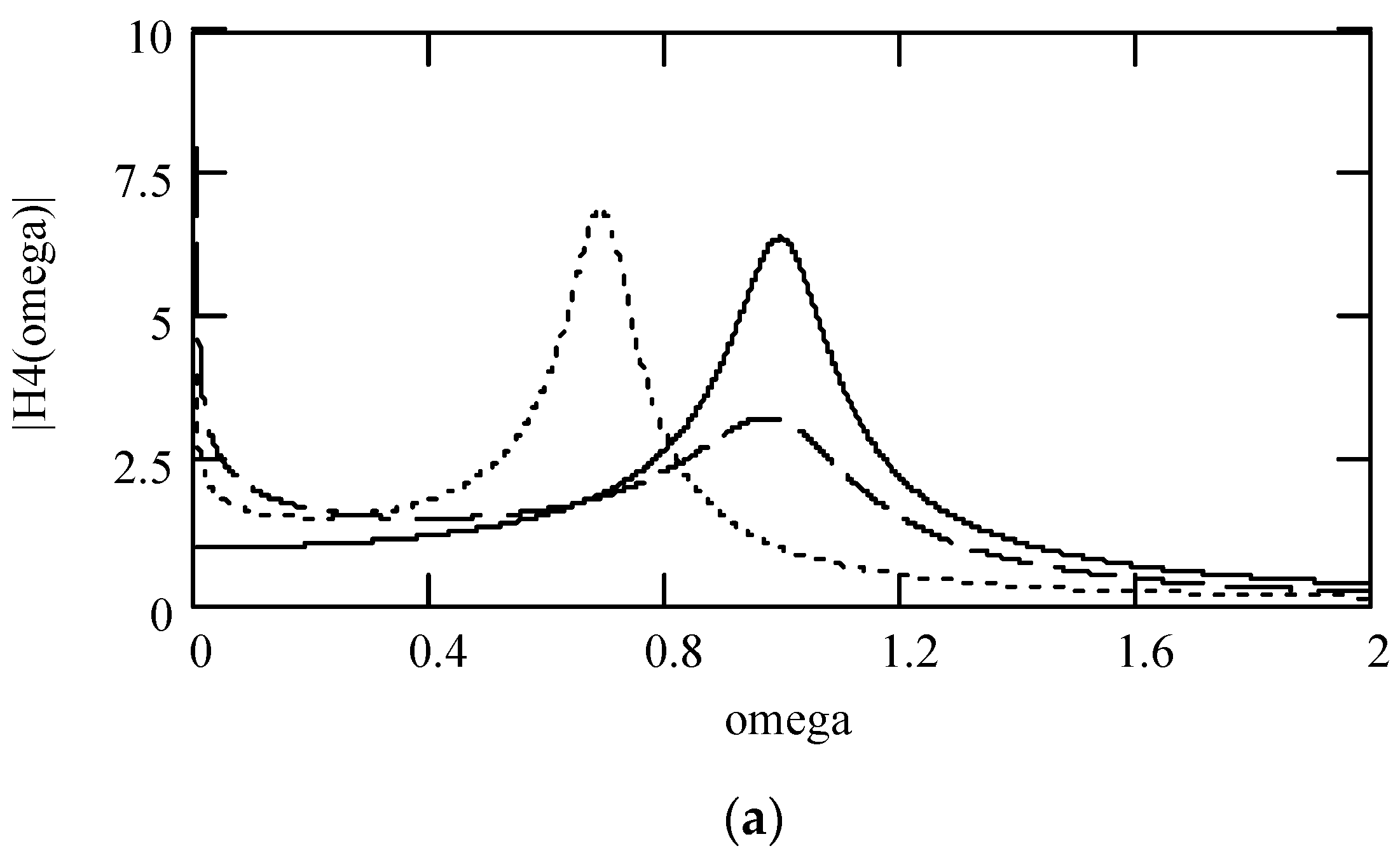
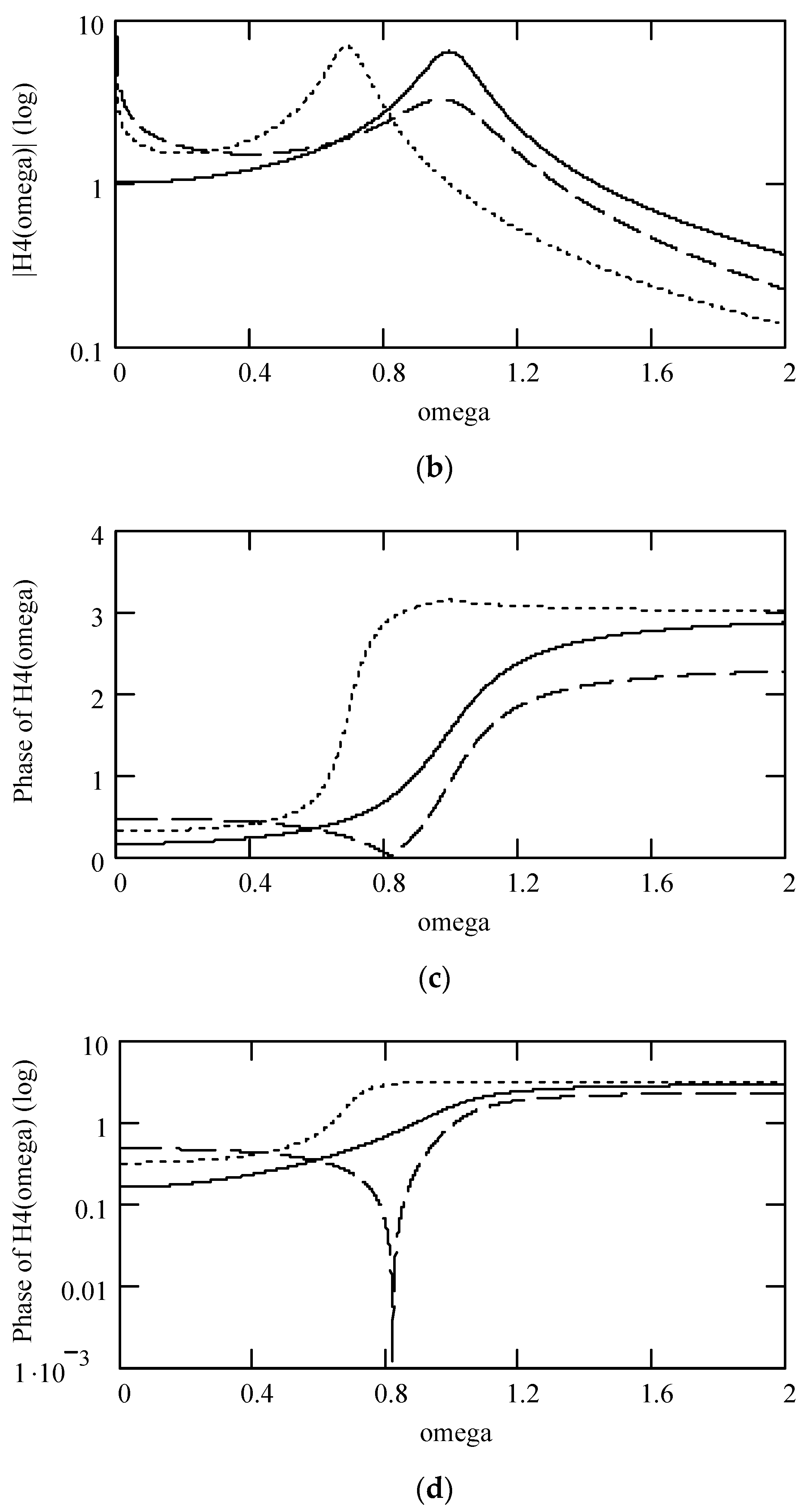
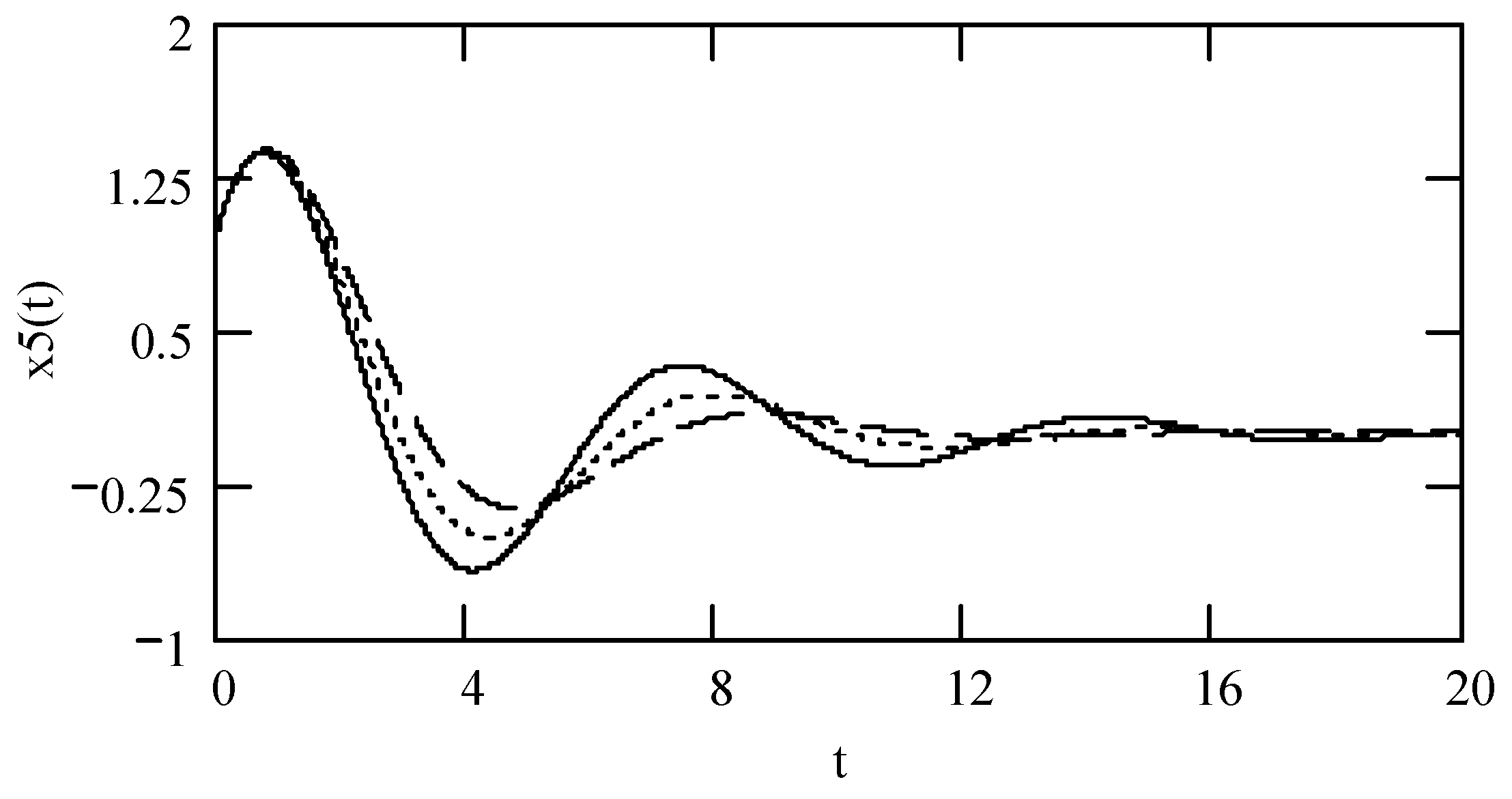
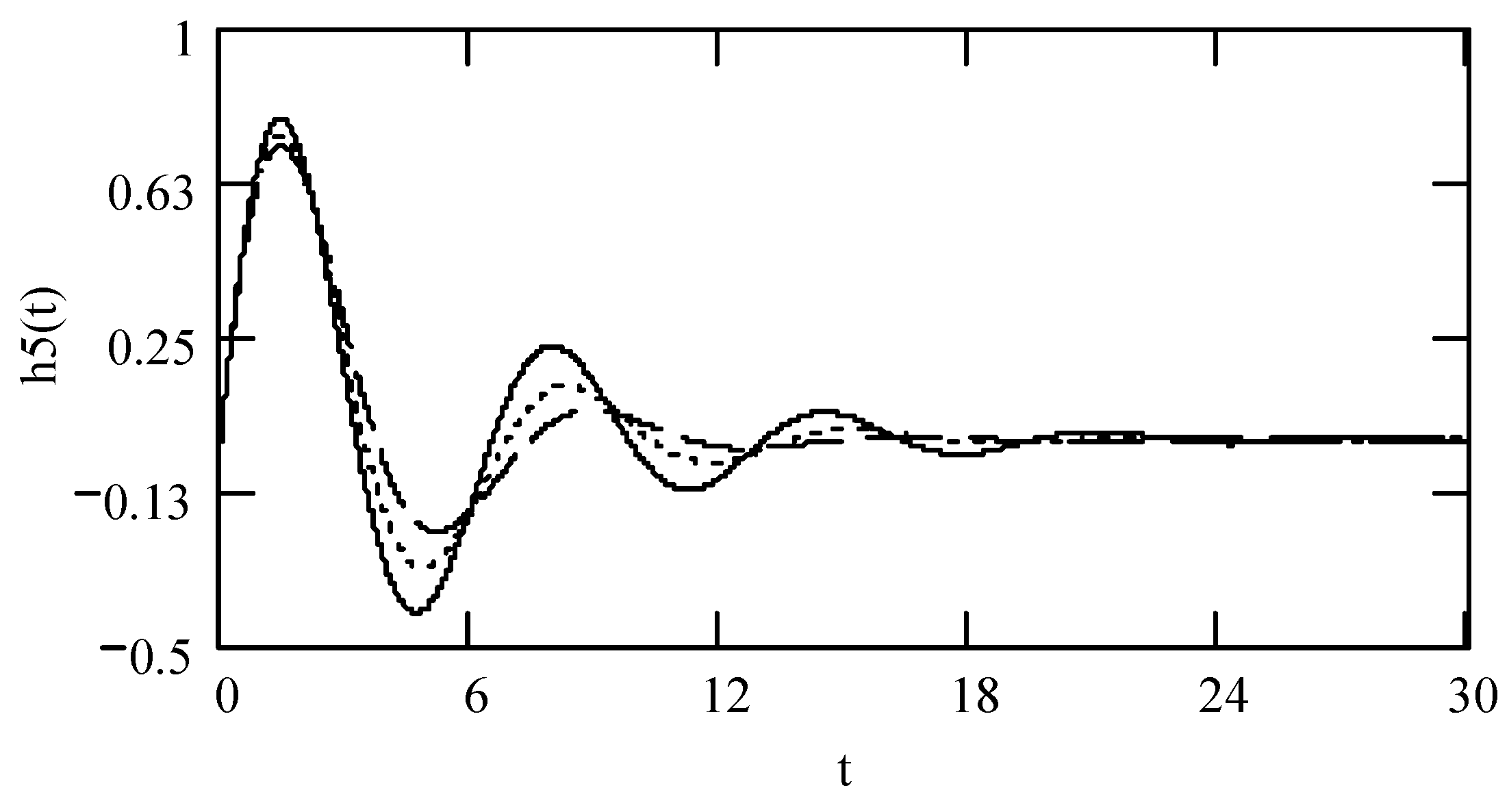
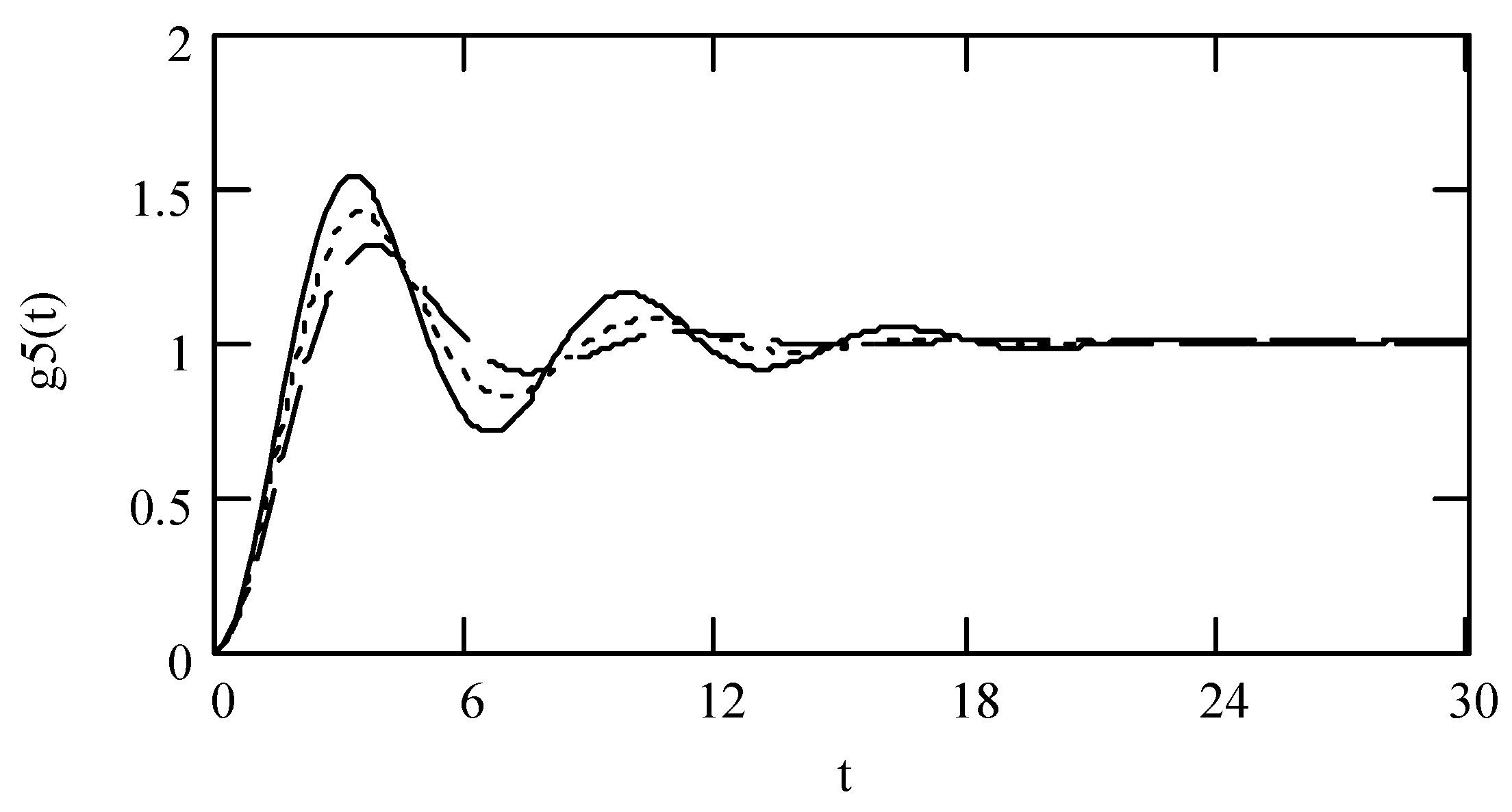
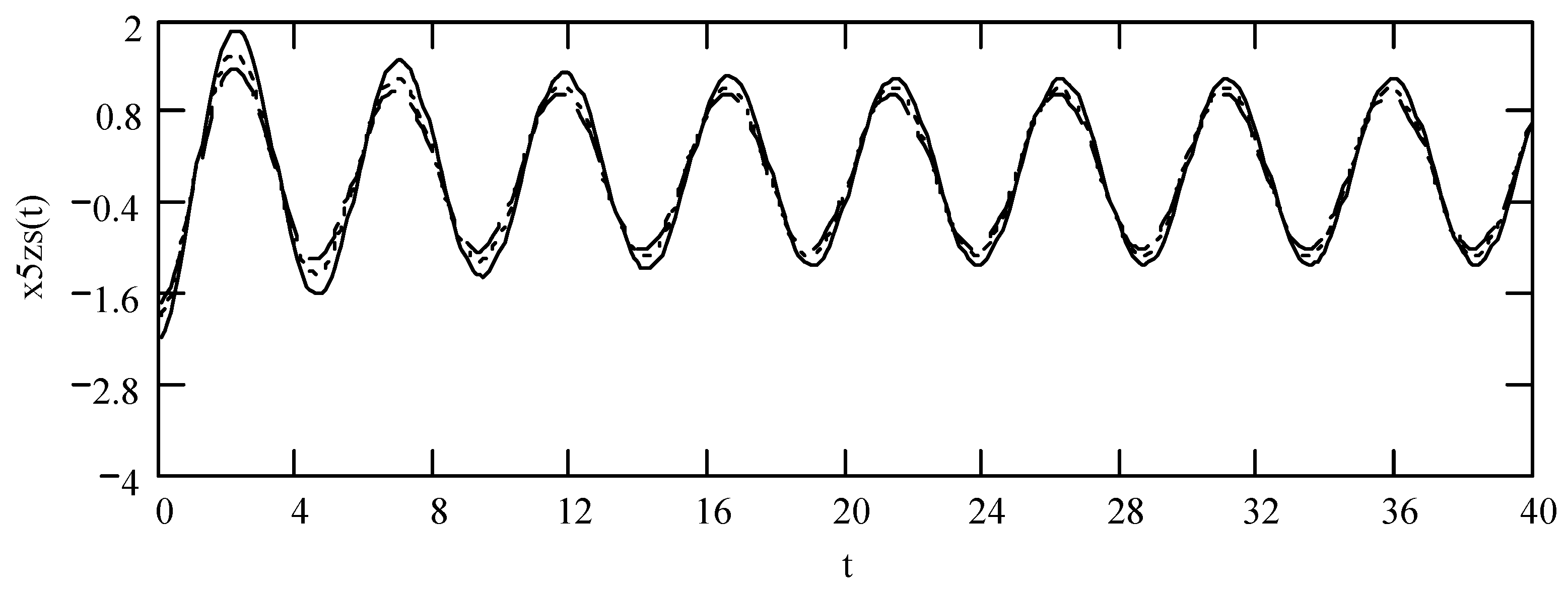

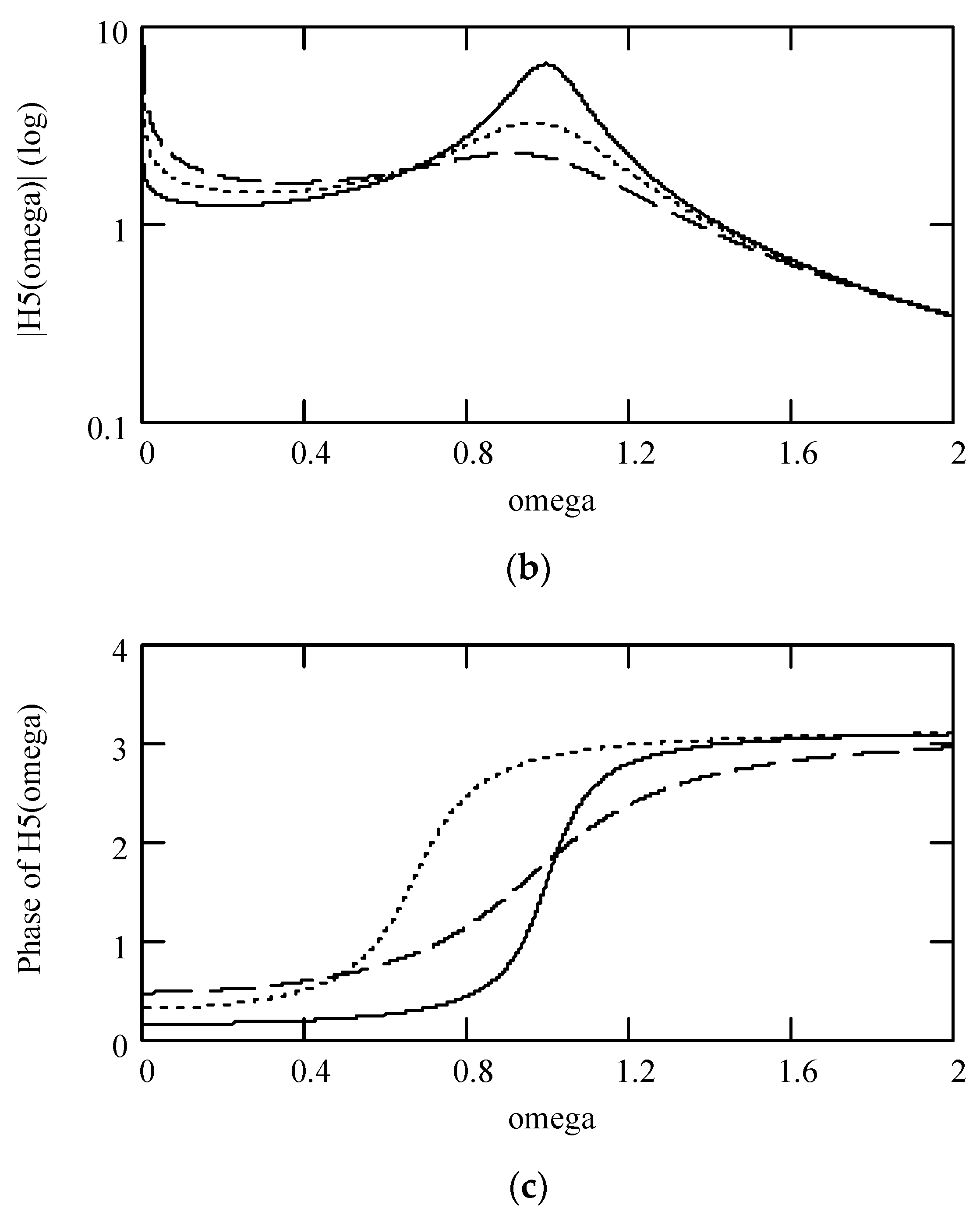






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M. Analytic Theory of Seven Classes of Fractional Vibrations Based on Elementary Functions: A Tutorial Review. Symmetry 2024, 16, 1202. https://doi.org/10.3390/sym16091202
Li M. Analytic Theory of Seven Classes of Fractional Vibrations Based on Elementary Functions: A Tutorial Review. Symmetry. 2024; 16(9):1202. https://doi.org/10.3390/sym16091202
Chicago/Turabian StyleLi, Ming. 2024. "Analytic Theory of Seven Classes of Fractional Vibrations Based on Elementary Functions: A Tutorial Review" Symmetry 16, no. 9: 1202. https://doi.org/10.3390/sym16091202
APA StyleLi, M. (2024). Analytic Theory of Seven Classes of Fractional Vibrations Based on Elementary Functions: A Tutorial Review. Symmetry, 16(9), 1202. https://doi.org/10.3390/sym16091202





