The Well-Posedness of Incommensurate FDEs in the Space of Continuous Functions
Abstract
1. Introduction
2. Generalization of Preliminary Theorems on Cartesian Product Space
2.1. Completeness of and Generalization of Arzelà–Ascoli Theorem for Cartesian Space
2.2. Compactness of Cartesian Product
2.3. Schauder and Banach Fixed-Point Theorems
2.4. Transforming into Incommensurate Weakly Singular Volterra Integral Equation
3. Regularity Analysis with the Schauder Fixed-Point Theorem
4. An Existence Result Using the Banach Fixed-Point Theorem
5. Existence Results with the Weaker Lipschitz Conditions
6. Uniqueness of the Solutions Based on Gronwall Inequality
7. Continuous Dependency to Initial Values
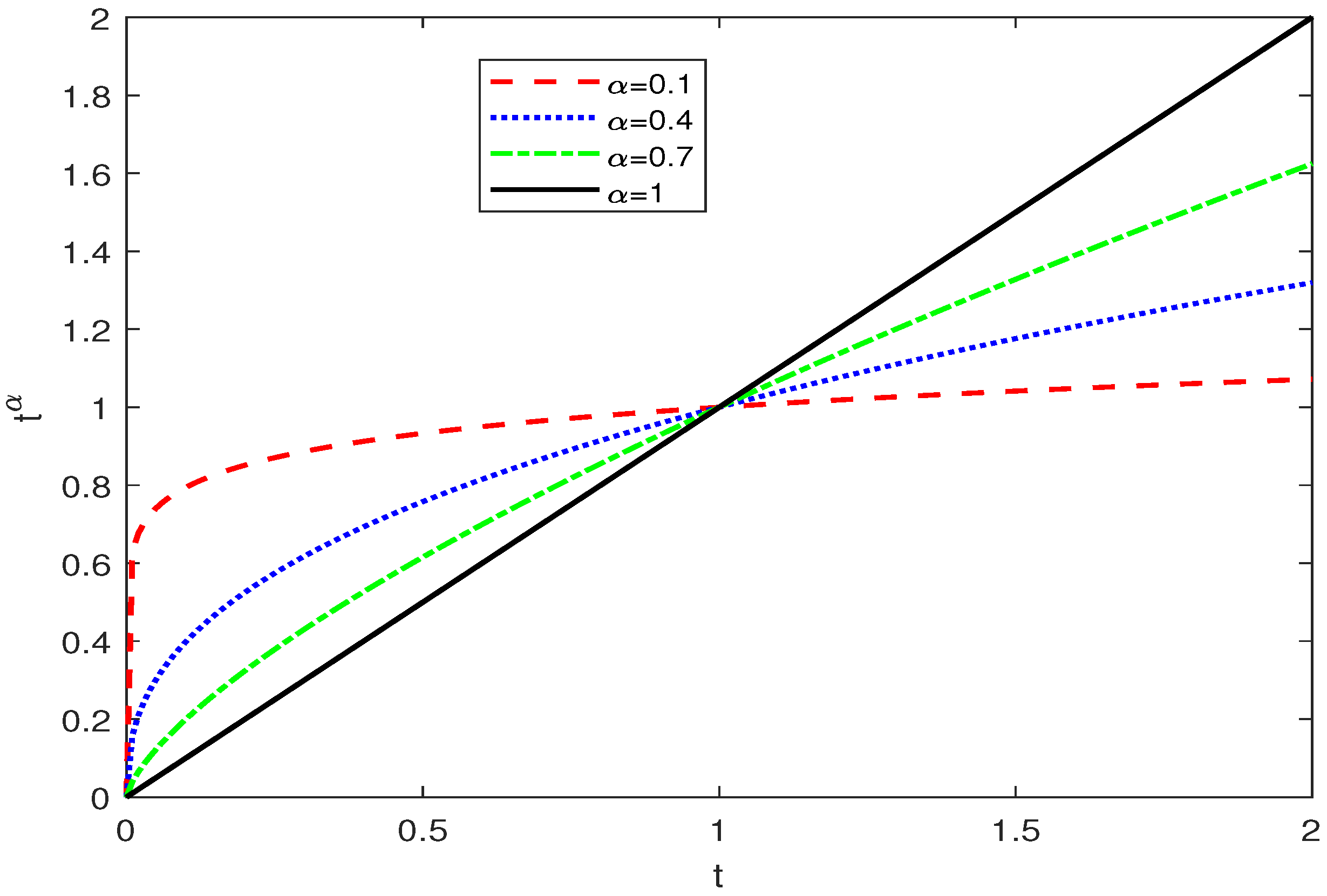
8. An Illustrative Example
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Diethelm, K.; Ford, N.J. The Analysis of Fractional Differential Equations; Lecture Notes in Mathematics; Springer: New York, NY, USA, 2010. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Daftardar-Gejji, V.; Babakhani, A. Analysis of a system of fractional differential equations. J. Math. Anal. Appl. 2004, 293, 511–522. [Google Scholar] [CrossRef]
- Drapaca, C.S. A mathematical investigation of sex differences in Alzheimer’s disease. Fractal Fractional 2022, 6, 457. [Google Scholar] [CrossRef]
- Baleanu, D.; Shiri, B. Generalized fractional differential equations for past dynamic. AIMS Math. 2022, 7, 14394–14418. [Google Scholar] [CrossRef]
- Shiri, B.; Baleanu, D. Numerical solution of some fractional dynamical systems in medicine involving non-singular kernel with vector order. Results Nonlinear Anal. 2019, 2, 160–168. [Google Scholar]
- Das, S.; Gupta, P.K. A mathematical model on fractional Lotka–Volterra equations. J. Theor. Biol. 2011, 277, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Tavazoei, M.; Asemani, M.H. On robust stability of incommensurate fractional-order systems. Commun. Nonlinear Sci. Numer. Simul. 2020, 90, 105–344. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, L.; Huang, J.; Gorbachev, S.; Aravind, R.V. Exploration on bifurcation for an incommensurate five-neuron fractional-order BAM neural network involving multiple delays. Phys. D Nonlinear Phenom. 2024, 460, 134047. [Google Scholar] [CrossRef]
- Yu, C.; Gao, G. Existence of fractional differential equations. J. Math. Anal. Appl. 2005, 310, 26–29. [Google Scholar] [CrossRef]
- Daftardar-Gejji, V.; Jafari, H. Analysis of a system of nonautonomous fractional differential equations involving Caputo derivatives. J. Math. Anal. Appl. 2007, 328, 1026–1033. [Google Scholar] [CrossRef]
- Zhang, S. The existence of a positive solution for a nonlinear fractional differential equation. J. Math. Anal. Appl. 2000, 252, 804–812. [Google Scholar] [CrossRef]
- Sheng, Y.; Zhang, T. The existence theory of solution in Sobolev space for fractional differential equations. Appl. Math. Lett. 2024, 149, 108896. [Google Scholar] [CrossRef]
- Saha, K.K.; Sukavanam, N.; Pan, S. Existence and uniqueness of solutions to fractional differential equations with fractional boundary conditions. Alex. Eng. J. 2023, 72, 147–155. [Google Scholar] [CrossRef]
- Matar, M.M.; Samei, M.E.; Etemad, S.; Amara, A.; Rezapour, S.; Alzabut, J. Stability Analysis and Existence Criteria with Numerical Illustrations to Fractional Jerk Differential System Involving Generalized Caputo Derivative. Qual. Theory Dyn. Syst. 2024, 23, 111. [Google Scholar] [CrossRef]
- Kou, C.; Zhou, H.; Yan, Y. Existence of solutions of initial value problems for nonlinear fractional differential equations on the half-axis. Nonlinear Anal. Theory Methods 2011, 74, 5975–5986. [Google Scholar] [CrossRef]
- Turan, N.; Başarır, M.; Şahin, A. On the solutions of a nonlinear system of q-difference equations. Bound. Value Probl. 2024, 2024, 92. [Google Scholar] [CrossRef]
- Turan, N.; Başarır, M.; Şahin, A. On the solutions of the second-order (p, q)-difference equation with an application to the fixed-point theory. AIMS Math. 2024, 9, 10679–10697. [Google Scholar] [CrossRef]
- Hashemi, M.S.; Baleanu, D. Lie Symmetry Analysis of Fractional Differential Equations; Chapman and Hall/CRC: Boca Raton, FL, USA, 2020. [Google Scholar]
- Paneva-Konovska, J. Series in Mittag-Leffler functions: Inequalities and convergent theorems. Fract. Calc. Appl. Anal.s 2010, 13, 403–414. [Google Scholar]
- Ye, H.; Gao, J.; Ding, Y. A generalized Gronwall inequality and its application to a fractional differential equation. J. Math. Anal. Appl. 2007, 328, 1075–1081. [Google Scholar] [CrossRef]
- Webb, J.R. Weakly singular Gronwall inequalities and applications to fractional differential equations. J. Math. Anal. Appl. 2019, 471, 692–711. [Google Scholar] [CrossRef]
- Kaslik, E.; Sivasundaram, S. Nonlinear dynamics and chaos in fractional-order neural networks. Neural Netw. 2012, 32, 245–256. [Google Scholar] [CrossRef] [PubMed]
- Karoun, R.C.; Ouannas, A.; Horani, M.A.; Grassi, G. The effect of Caputo fractional variable difference operator on a discrete-time hopfield neural network with non-commensurate order. Fractal Fract. 2022, 6, 575. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shiri, B.; Shi, Y.-G.; Baleanu, D. The Well-Posedness of Incommensurate FDEs in the Space of Continuous Functions. Symmetry 2024, 16, 1058. https://doi.org/10.3390/sym16081058
Shiri B, Shi Y-G, Baleanu D. The Well-Posedness of Incommensurate FDEs in the Space of Continuous Functions. Symmetry. 2024; 16(8):1058. https://doi.org/10.3390/sym16081058
Chicago/Turabian StyleShiri, Babak, Yong-Guo Shi, and Dumitru Baleanu. 2024. "The Well-Posedness of Incommensurate FDEs in the Space of Continuous Functions" Symmetry 16, no. 8: 1058. https://doi.org/10.3390/sym16081058
APA StyleShiri, B., Shi, Y.-G., & Baleanu, D. (2024). The Well-Posedness of Incommensurate FDEs in the Space of Continuous Functions. Symmetry, 16(8), 1058. https://doi.org/10.3390/sym16081058






