Analysis of the Surrounding Rock Full-Displacement Variation in Large-Span Mudstone Highway Tunnels
Abstract
1. Introduction
2. Field Monitoring of Tunnel Full Displacement
2.1. Engineering Background
2.2. Monitoring Scheme
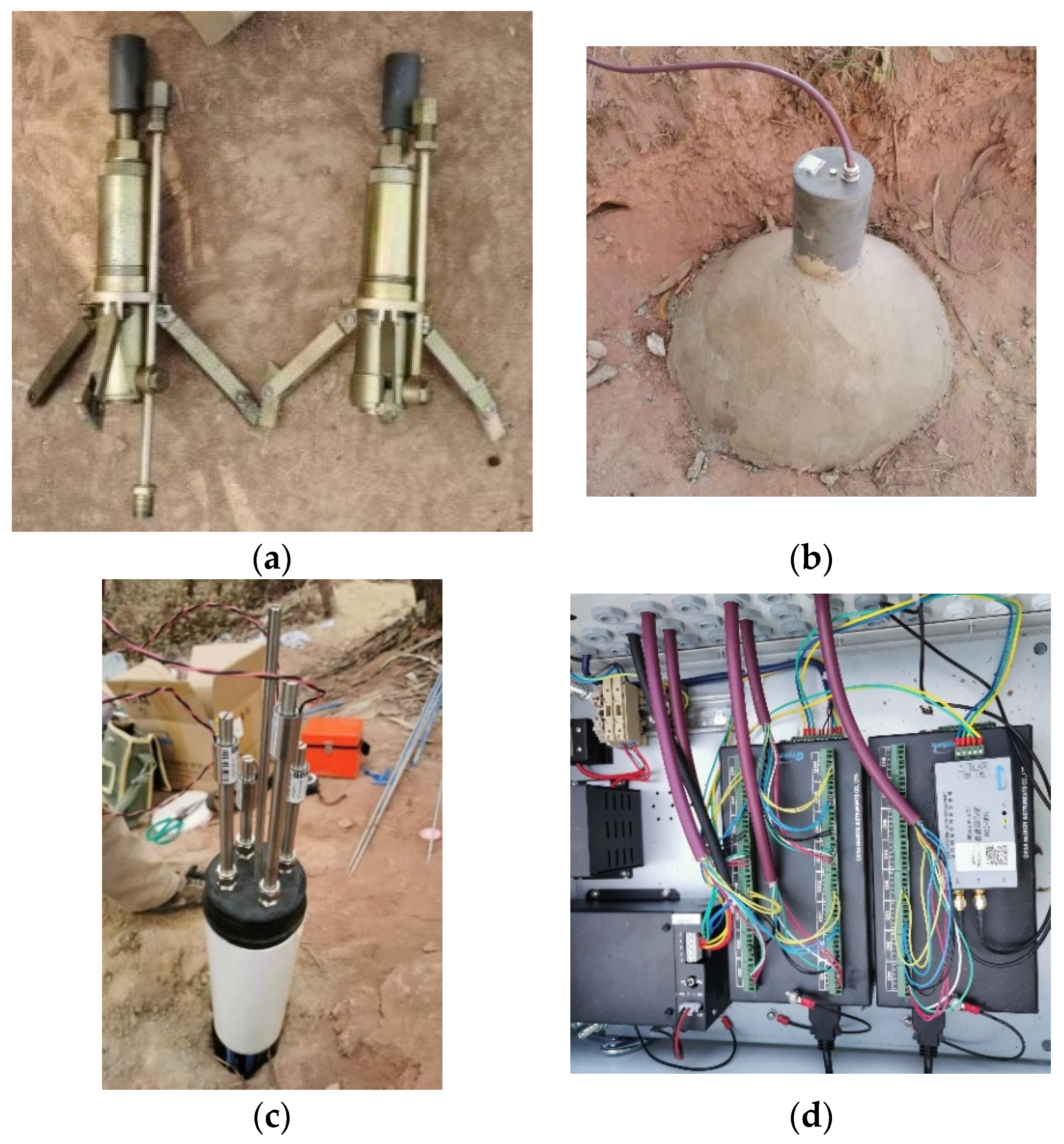
2.3. Installation and Operation of Sensors
- (1)
- After setting a point on the surface using a total station, a borehole is drilled vertically downward from this point. The drilling is stopped when the bottom of the hole is approximately 1 m from the tunnel vault.
- (2)
- The anchor head is connected to the manual hydraulic pump via the hydraulic pipe and manually pressurized until the anchor head is tightened. Note that the hydraulic pipe and anchor head are connected through the snap, and the pressure range of the hydraulic pump is between 3 and 5 MPa.
- (3)
- The anchor head is connected to a stainless steel measuring rod, which is lengthened section by section to put the anchor head at the pre-determined depth.
- (4)
- The hydraulic pump relief valve is opened quickly so that the anchor head instantly pops open, firmly anchored to the borehole wall.
- (5)
- The remaining measurement points are set according to steps 1 to 4.
- (6)
- After completing the setting of the anchor heads at different depths, the measuring rod is inserted into each base. The displacement meter is then connected to the measuring rod.
- (7)
- The multipoint displacement meter base is encased in cement mortar and secured to the surface once it solidifies.
- (8)
- The signal line of each displacement meter is connected to the automatic acquisition box, which is powered by solar energy and a battery, to achieve the timed automatic acquisition.
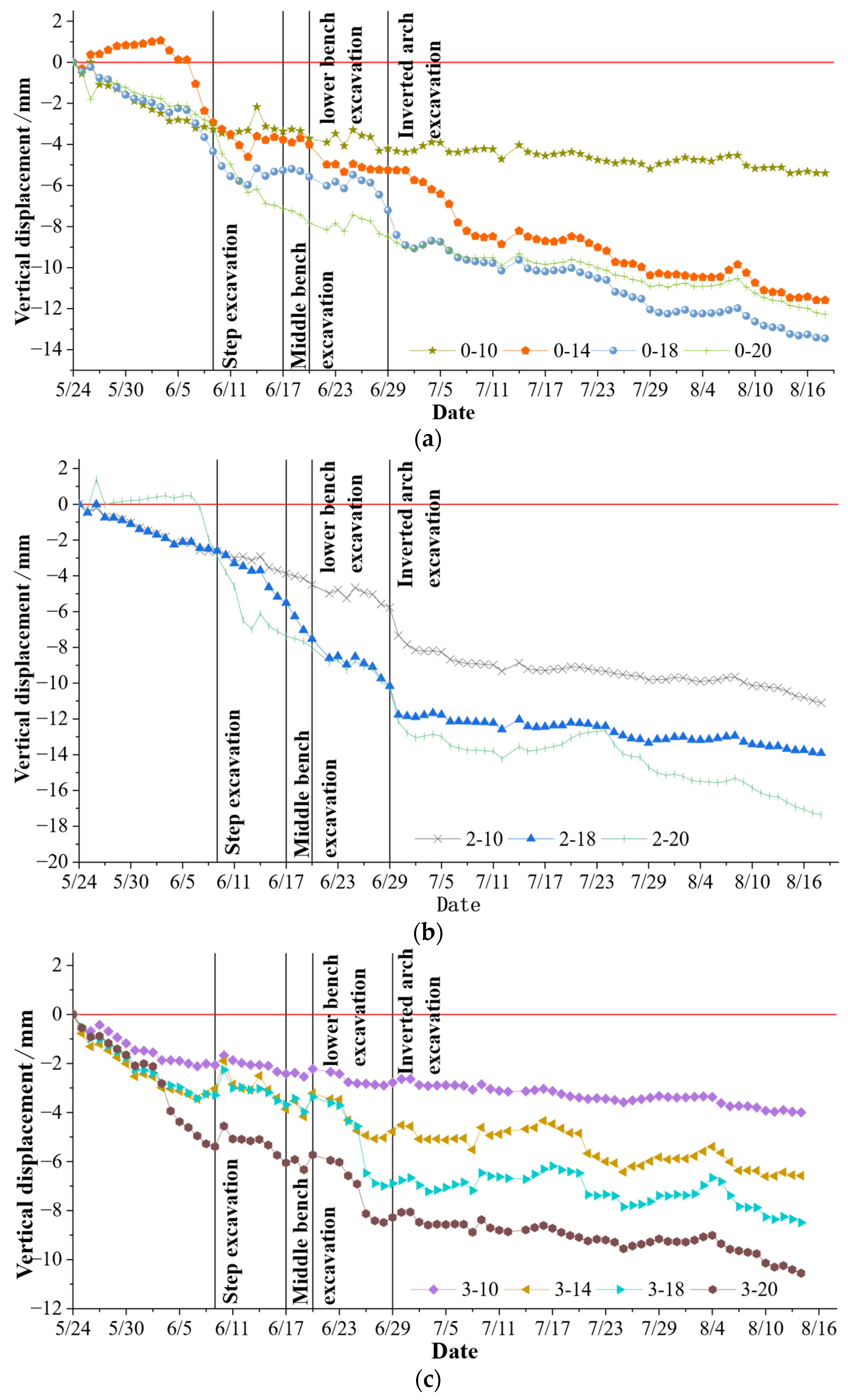
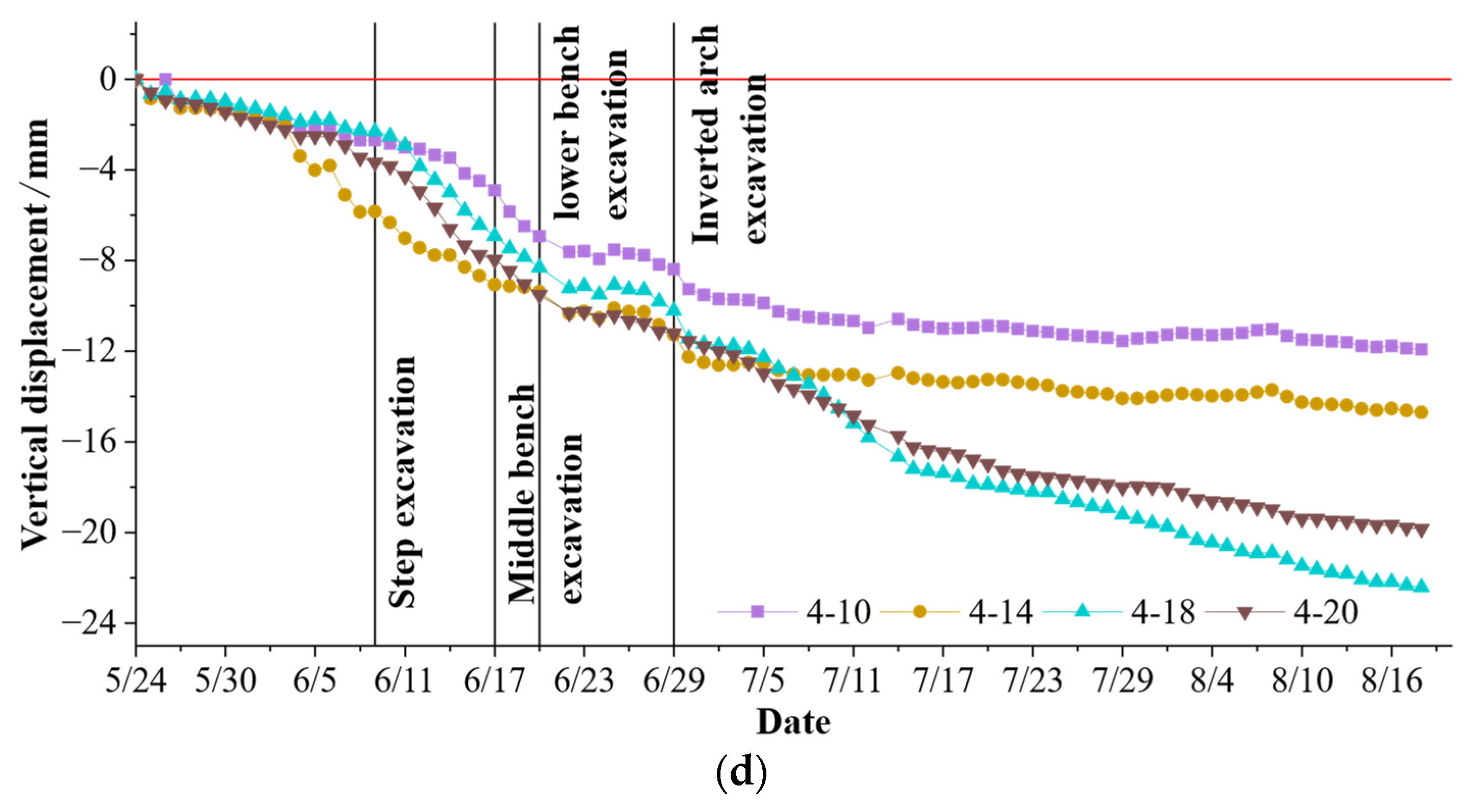
2.4. Full Displacement Detection Results and Analysis
- (1)
- Small settlement area: When the tunnel face is in the range of −1.75~−0.6 times that of the tunnel diameter, the rock layer has a small vertical deformation and the settlement is within 2 mm, mainly due to the blasting excavation of the tunnel face, by the loosening the surrounding rock.
- (2)
- Early slight growth area: When the tunnel face is in the range of −0.6~0 times that of the tunnel diameter, the settlement increases slightly due to the reduced influence of the tunnel-face spatial effect, which accounts for at least 15% of the total settlement value.
- (3)
- Rapid growth area: As the progress of the tunnel excavation face, within the range of 0 to 2 times the diameter of the measuring section after the excavation, monitoring is conducted. The settlement increases sharply because of the excavation of the three steps and the inverted arch, which accounts for about 40~60% of the total settlement.
- (4)
- Slow growth area: After the overall excavation of the tunnel and the construction of the secondary lining are complete, when the construction surface is 2~2.5 times the tunnel diameter from the monitoring section, the supporting structure stress state is gradually completed and the settlement rate gradually slows.
- (5)
- Gradual stable area: After completing the monitoring section, when the construction surface is greater than 2.5 times the tunnel diameter from the monitoring section, the settlement curve gradually tends to be gentle. After about 30 days, the settlement is basically stable.
3. Regression Analysis of Full Displacement of Tunnel Deformation
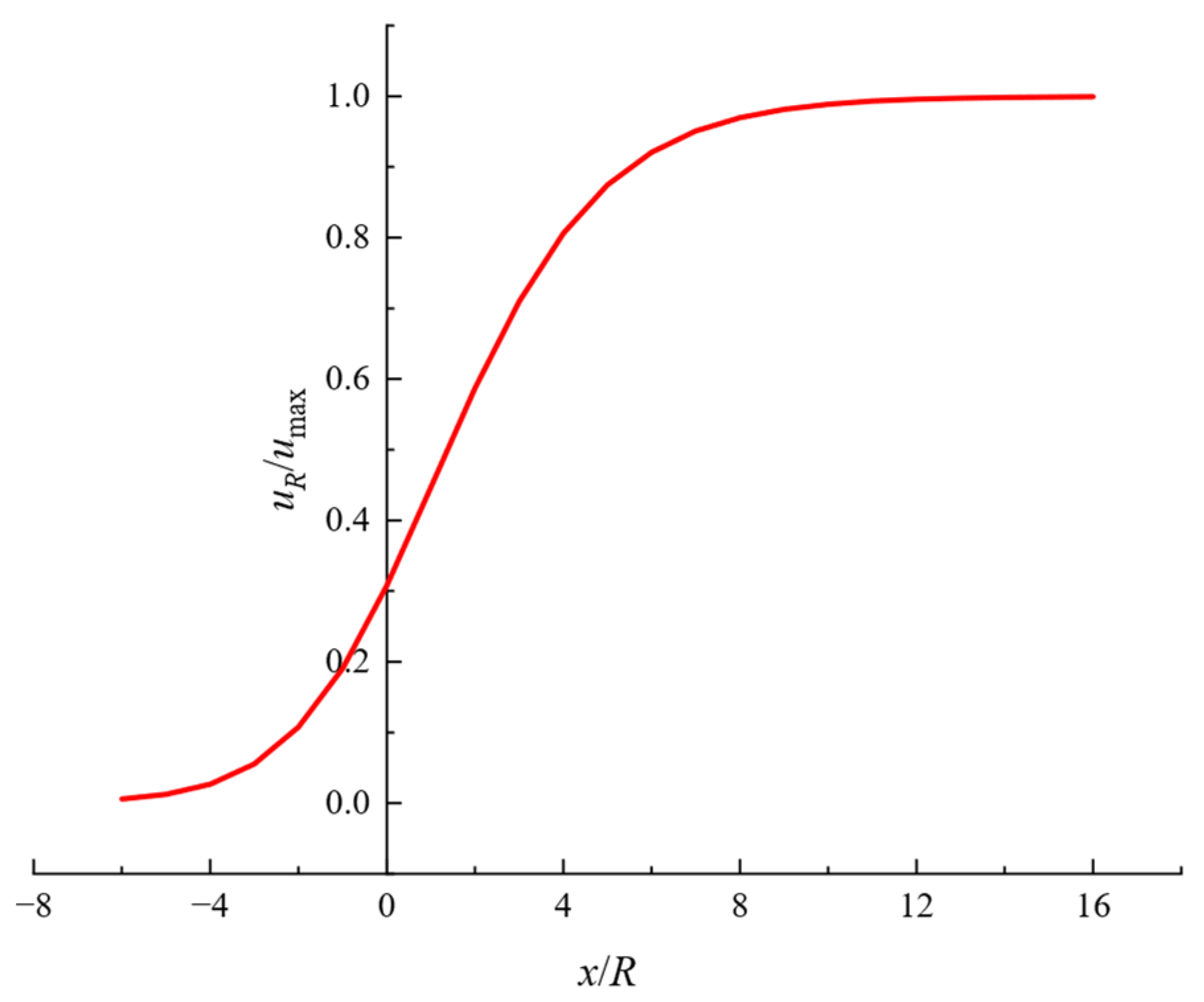
3.1. Tunnel-Face Spatial Effect
3.2. Analysis of the Displacement Stable Convergence Value
3.3. Regression Analysis of the Vertical Displacement Convergence Value
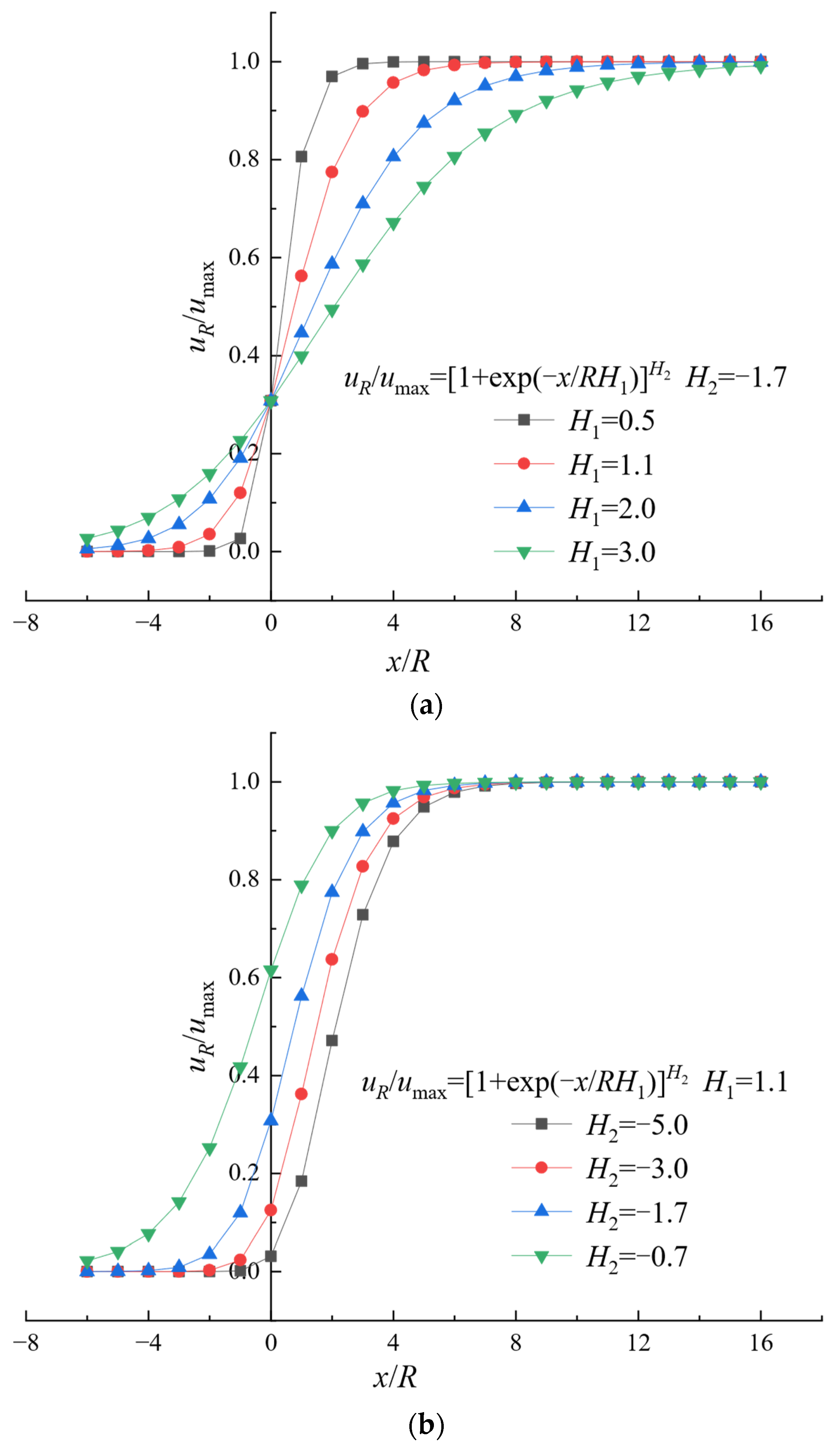
3.4. Parameter Analysis of the Longitudinal Settlement-Displacement Regression Curve Equation
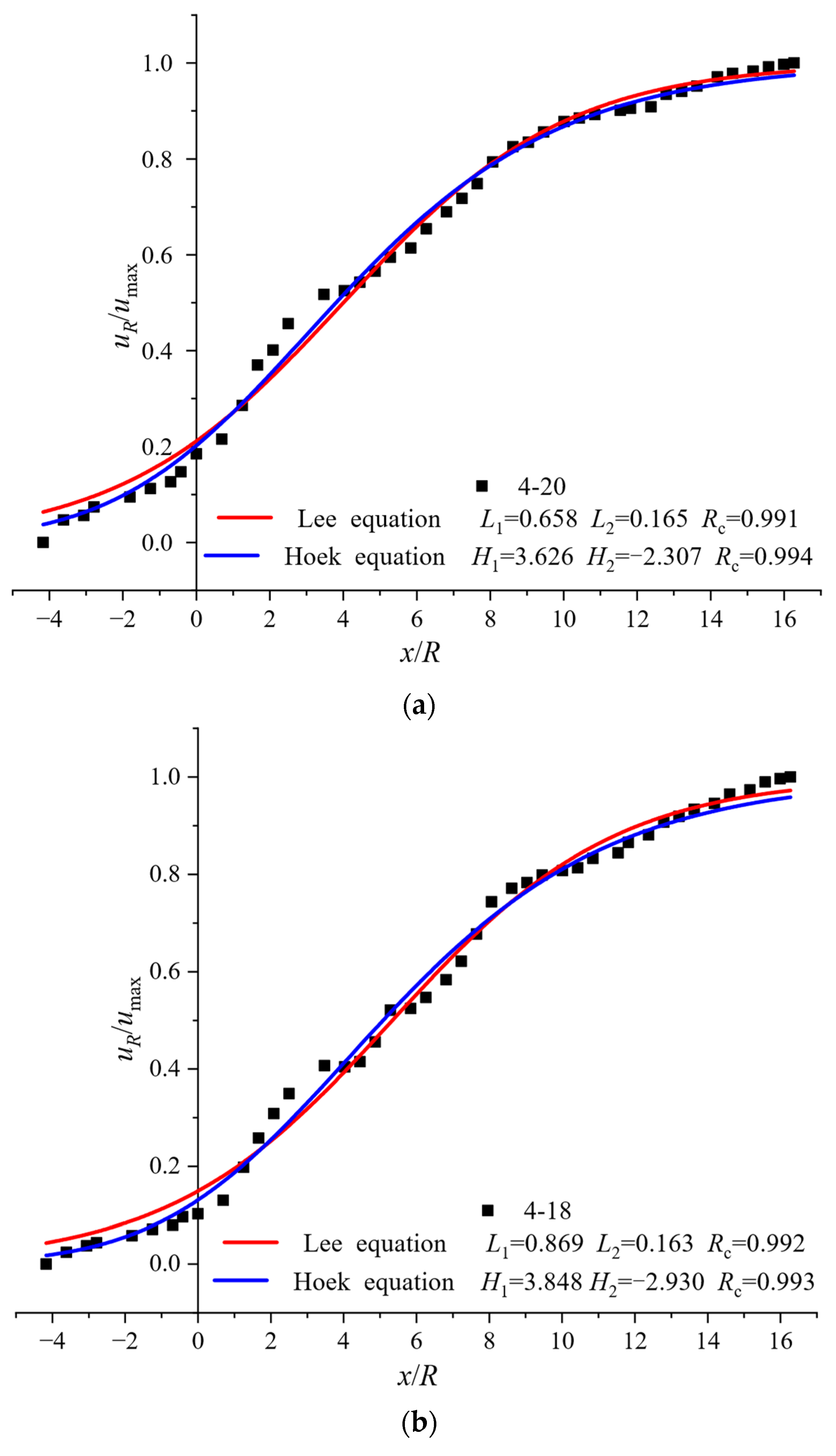
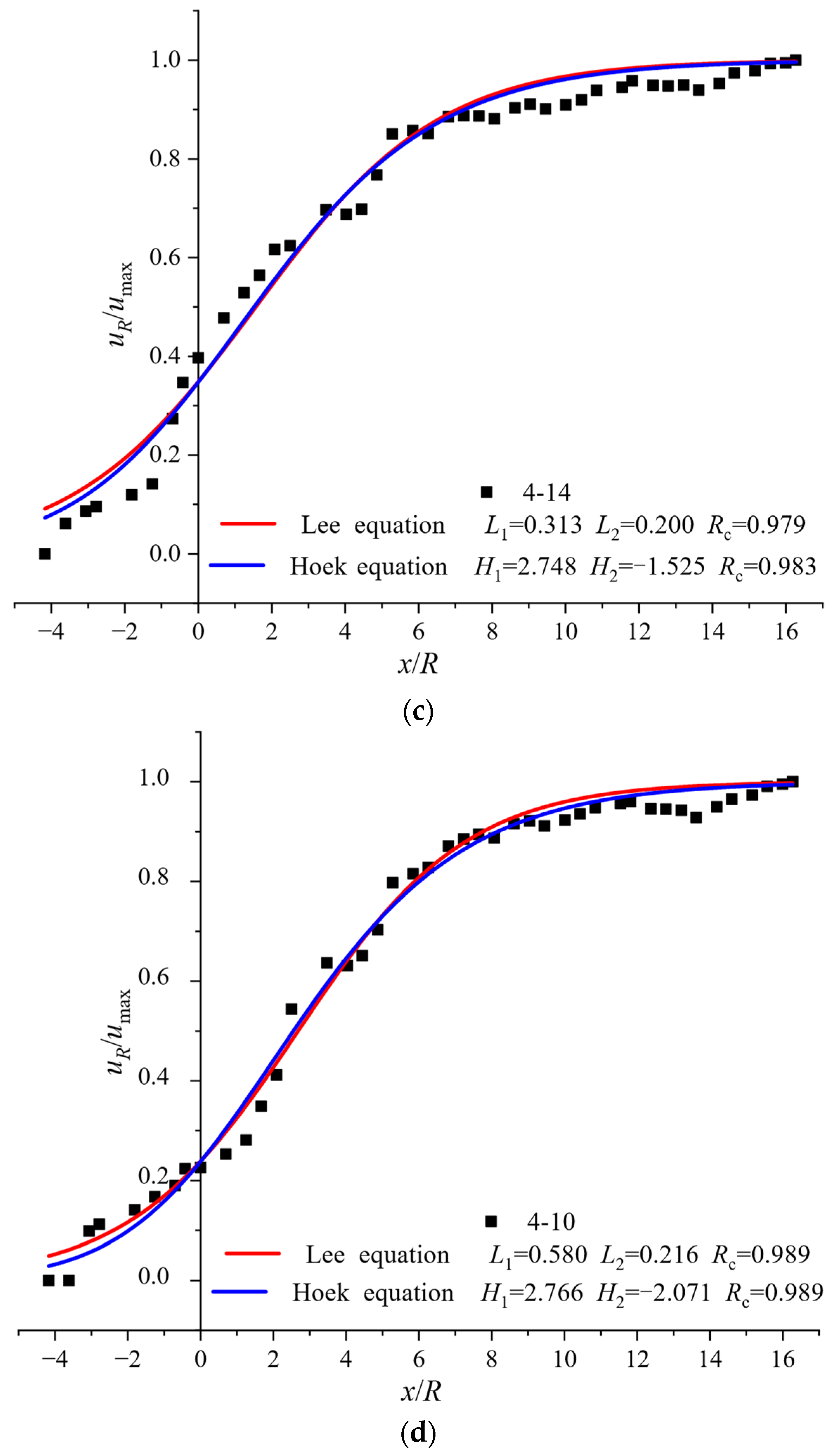
3.5. Fitting Analysis of Field-Measured Data
4. Numerical Simulation of Tunnel Full Displacement
4.1. Fundamental Assumption
4.2. Model Establishment
4.3. Parameter Selection and Boundary Conditions
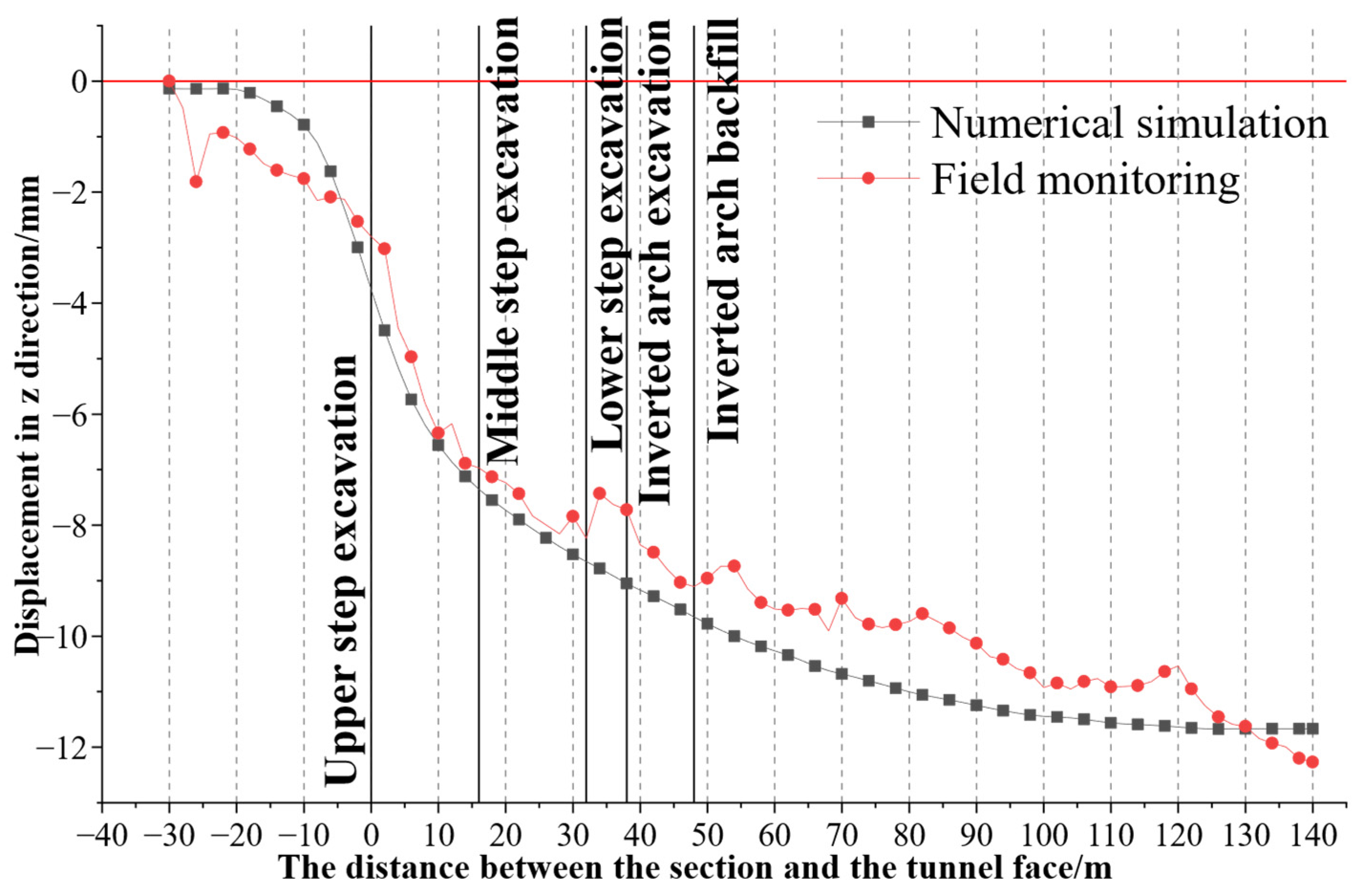
4.4. Rationality Verification of the Model
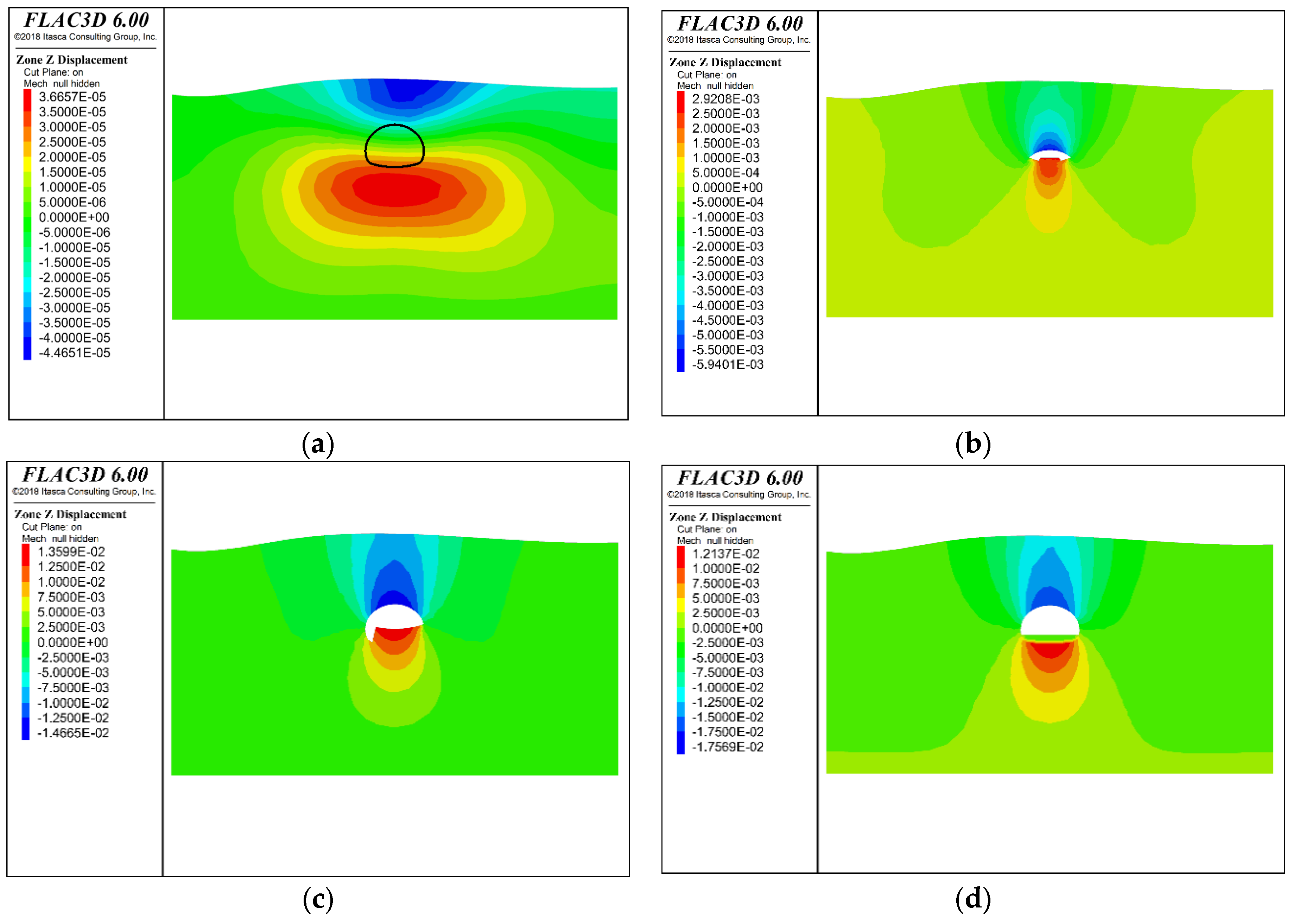
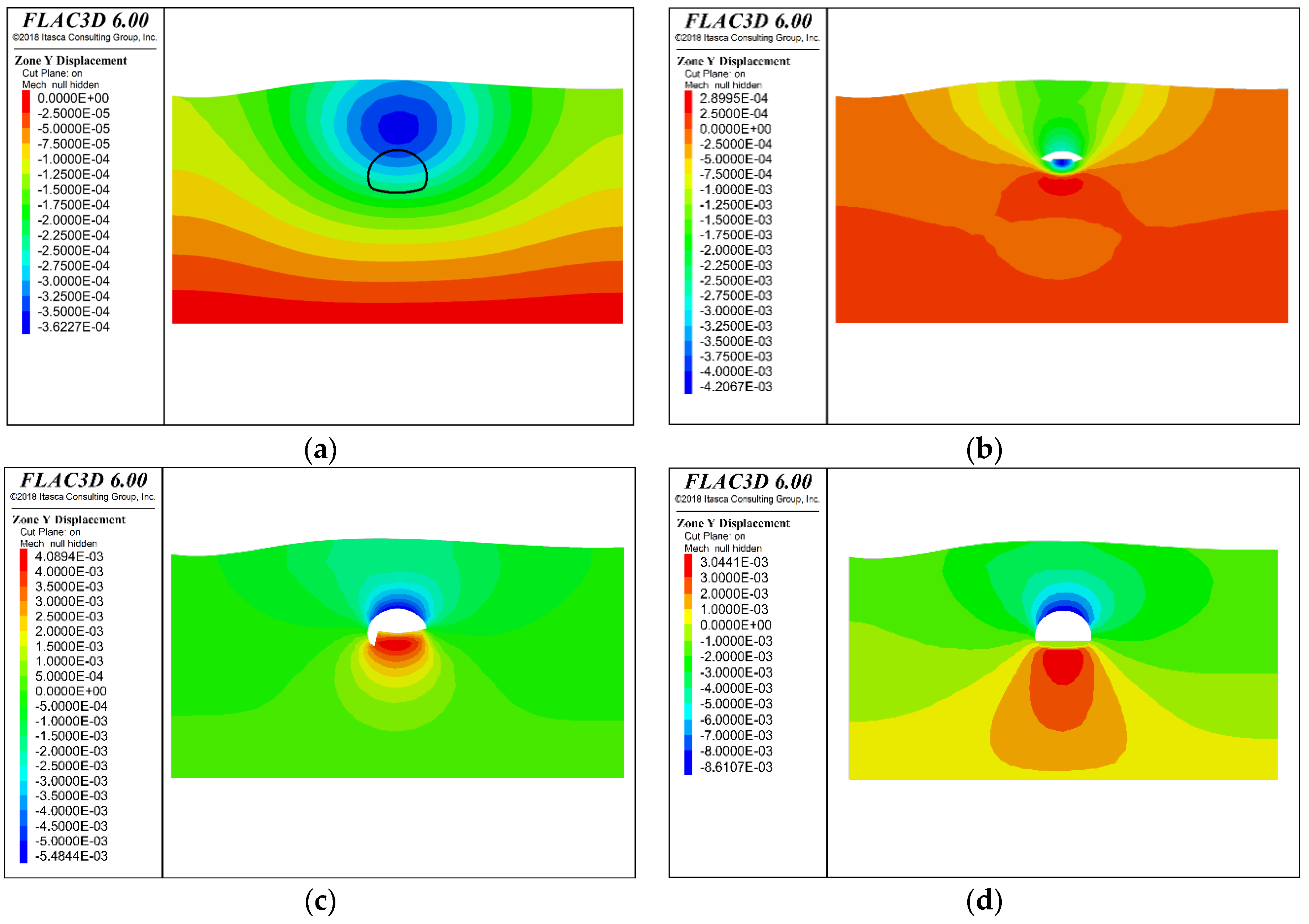
4.5. Analysis of the Surrounding Rock Displacement Cloud Diagram for the Tunnel Arch
4.6. Analysis of Full Displacement at Different Depths from the Vault
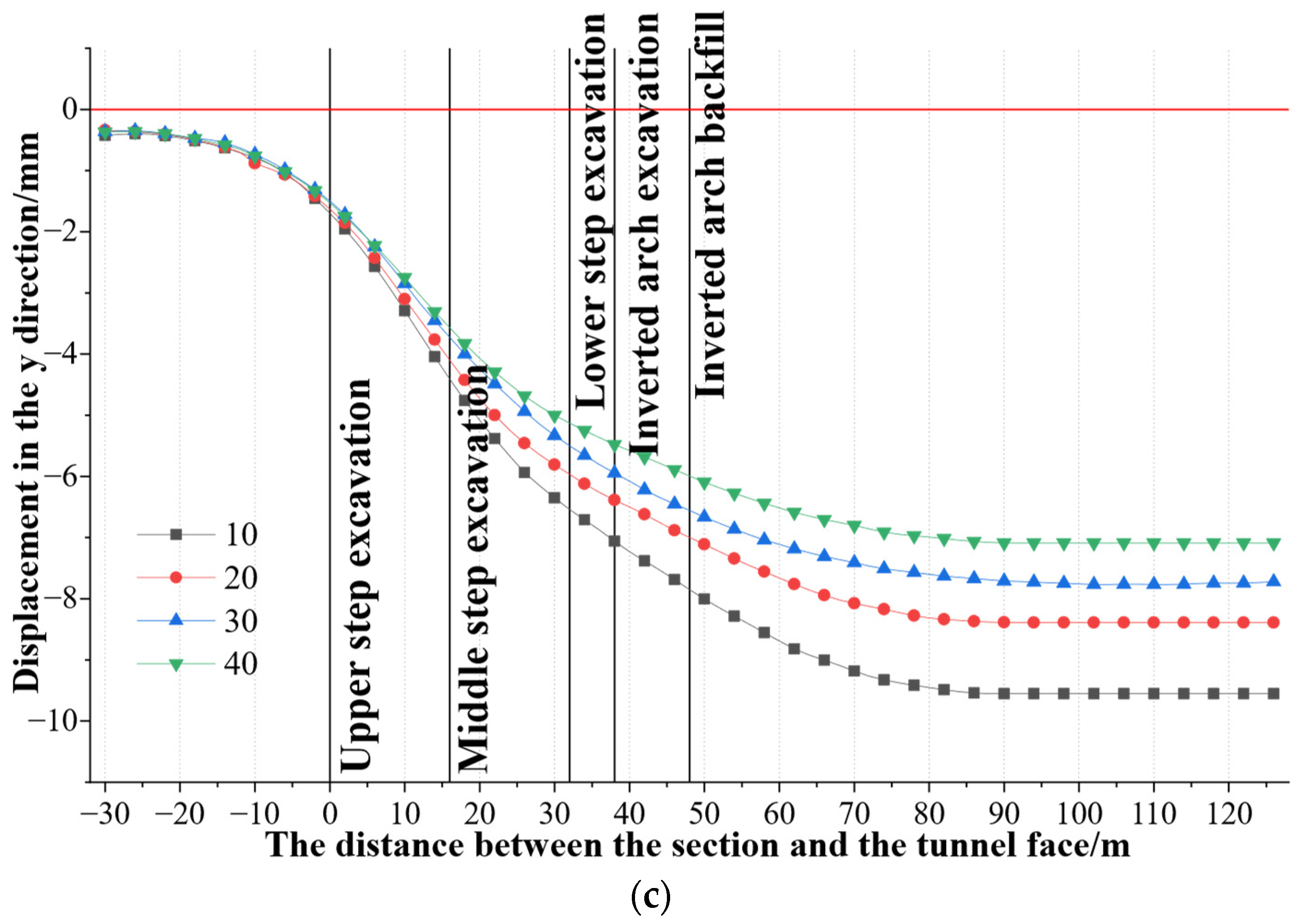
4.7. Full Displacement Analysis of the Tunnel under Different Burial Depths
5. Conclusions
- (1)
- According to the field monitoring data, the displacement of the tunnel changes most clearly in the range of −1~2.5 times the tunnel diameter. In addition, 40% to 60% of the total displacement is due to the tunnel excavation. The total change in surrounding rock displacement at each measurement point passes through four stages: slow pre-displacement growth, rapid increase, slow increase, and gradual stability.
- (2)
- The Gucheng tunnel has a large-span. The three-step excavation method is adopted for the tunnel, which leads to a sharp increase in the displacement release rate. This result includes the following main factors: The tunnel span increases rapidly due to the excavation of the upper and middle steps, and the overall settlement above the vault due to the excavation of lower steps and the inverted arch. The different excavation sequence for the left and right steps leads to the asymmetry in the displacement release on both sides of the tunnel. The displacement release rate caused by the excavation of the tunnel face can reach up to 65%.
- (3)
- The fitting equation of the vertical surrounding rock displacement data is determined by the Lee and Hoek longitudinal displacement curve equations, and this research indicates that the Hoek equation provides a better fit for the longitudinal deformation characteristics of the Gucheng tunnel. On the basis of these fitting equations, the final stable convergence value of the internal surrounding rock displacement can be predicted.
- (4)
- Based on measured data from the Gucheng tunnel, a numerical simulation model is established. The curve change law for vertical displacement and the actual monitoring results are basically the same; the area influenced by rapid growth of the tunnel-face spatial effect on the surrounding rock displacement is −1.0D~4D. In addition, the displacement release rate variation range increases when the measurement point lies closer to the vault, which in turn causes greater disturbance of the tunnel-face spatial effect on the surrounding rock. For a tunnel with a shallow burial depth, the tunnel-face spatial effect releases a large amount of vertical displacement and lateral displacement before excavation. The ahead displacement release rate exceeds 50%, and there is almost no interference to the longitudinal displacement.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chen, J.X.; Luo, Y.B. The stability of structure and its control technology for lager-span loess tunnel. Hazard Control Tunn. Undergr. Eng. 2019, 1, 93–101. (In Chinese) [Google Scholar]
- Luo, Y.B.; Chen, J.X.; Shi, Z.; Zhang, S.; Liu, W.; Li, Y. Mechanical and Deformation Characteristics and Optimization of Support Parameters for Superlarge-Span Tunnel: A Case Study from Laohushan Tunnel. Adv. Civ. Eng. 2020, 2020, 8882019. [Google Scholar] [CrossRef]
- Zhu, H.; Yang, L. Spatial effect analysis of excavation face of underground powerhouse test tunnel of Tianhuangping Pumped Storage Power Station. Geotech. Investig. Surv. 1994, 3, 1–6. (In Chinese) [Google Scholar]
- Lee, Y.N.; Yun, S.P.; Kim, D.Y.; Nam, H.K. Design and construction aspects of unlined oil storage caverns in rock. Tunn. Undergr. Space Technol. 1996, 11, 33–37. [Google Scholar] [CrossRef]
- Lee, Y.N.; Suh, Y.H.; Kim, D.Y.; Jue, K.S. Stress and deformation behaviour of oil storage caverns during excavation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1997, 34, 573. [Google Scholar] [CrossRef]
- Sato, T.; Kikuchi, T.; Sugihara, K. In-situ experiments on an excavation disturbed zone induced by mechanical excavation in Neogene sedimentary rock at Tono mine, central Japan. Eng. Geol. 2000, 56, 97–108. [Google Scholar] [CrossRef]
- Qiao, L.; Li, S.; Wang, Z.; Tian, H.; Bi, L. Geotechnical monitoring on the stability of a pilot underground crude-oil storage facility during the construction phase in China. Measurement 2016, 82, 421–431. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Z.; Xie, Y.; Liu, B. Study of site monitoring of vertical full displacement of vault surrounding rock of tunnels and its change law. Rock Soil Mech. 2013, 34, 1703–1708. (In Chinese) [Google Scholar]
- Zeng, H.; Wang, Y.; Li, J.; Yang, C.; Feng, G. On-site monitoring and analysis of single-hole four-lane highway tunnel. Railw. Eng. 2015, 4, 85–88. (In Chinese) [Google Scholar]
- Wu, A.; Wang, J.; Zhou, Z.; Huang, S.; Ding, X.; Dong, Z.; Zhang, Y. Engineering rock mechanics practices in the underground powerhouse at Jinping I hydropower station. J. Rock Mech. Geotech. Eng. 2016, 8, 640–650. [Google Scholar] [CrossRef][Green Version]
- Sun, Z.; Zhang, D.; Hou, Y.; Li, A. Whole-process deformation laws and determination of stability criterion of surrounding rock of tunnels based on statistics of field measured data. Chin. J. Geotech. Eng. 2021, 43, 1261–1270. (In Chinese) [Google Scholar]
- Wang, B.W. Impact Analysis of Mudstone Expansion on the Stability of Tunnel Lining. Master’s Thesis, Lanzhou Jiaotong University, Lanzhou, China, 2021. (In Chinese). [Google Scholar]
- Zhang, S.; Wang, Y.; Wang, S. Discussion on Construction Technology of Expansive Mudstone Tunnel in Expressway. In Proceedings of the 2016 International Conference on Education, Management, Computer and Society, Shenyang, China, 1–3 January 2016; Volume 37, pp. 363–366. [Google Scholar]
- Wang, Y.; Jiang, H.; Yan, X.; Liang, H.; Li, G.; Liu, X.; Peng, H.; Wan, S.; Bai, W. Support Measures to Prevent the Deformation and Failure of a Carbonaceous Mudstone Tunnel. Adv. Civ. Eng. 2021, 2021, 8833502. [Google Scholar] [CrossRef]
- Li, S.; Fu, J.; Liu, G.; Zhou, J. Research on intrusion and large arch bulge in lining structure for highway’s mudstone tunnel. Front. Phys. 2024, 12, 1345581. [Google Scholar] [CrossRef]
- Zacharoudiou, I.; Boek, E.S.; Crawshaw, J. The impact of drainage displacement patterns and Haines jumps on CO2 storage efficiency. Sci. Rep. 2018, 8, 15561. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Zhu, Z.H.; Wu, H.; Zhang, S.; Wang, J. Study of deformation characteristics of the high sidewall surrounding rock in super large underground caverns. Rock Soil Mech. 2019, 40, 4030–4040. [Google Scholar]
- Guo, R.; Fang, Y.; He, C. Study on the correlation between stress release and displacement release during tunnel excavation. J. Railw. Eng. Soc. 2010, 27, 46–50. (In Chinese) [Google Scholar]
- Su, Y.; He, M.; Gao, Q. Application of Rosenblueth method in evaluating stability reliability of anchor-shotcrete net support system for soft-fractrue surrounding rock. Chin. J. Geotech. Eng. 2004, 26, 378–382. (In Chinese) [Google Scholar]
- Sun, J.; Zhu, H. Mechanical Simulation and Analysis of Behaviour of Soft and Weak Rocks in the Construction of a Tunnel Opening. Rock Soil Mech. 1994, 15, 20–33. (In Chinese) [Google Scholar]
- Lee, Y.L. Prise en Compte des Non-Linéarités de Comportement des sols et des Roches dans la Modélisation du Creusement d’un Tunnel. (Nonlinear Characteristics of Soil and Rock Behavior in Tunnel Excavation Theory). Ph.D. Thesis, Ecole Nationale des Ponts et Chaussées, Marne-la-Vallée, France, 1994. (In French). [Google Scholar]
- Hoek, E.; Brown, E.T. Practical estimates of rock mass strength. Int. J. Rock Mech. Min. Sci. 1997, 34, 1165–1186. [Google Scholar] [CrossRef]
- Sun, S.F.; Yuan, Z.; Zhao, W.Q. Cause Analysis and Treatment Principles for Tunnel Lining Cracking in Sandy Mudstone with High Geostress. Mod. Tunn. Technol. 2013, 50, 170–175. (In Chinese) [Google Scholar]
- Qin, Z.G.; Chen, Y. Study on Engineering Geological Characteristics of Tertiary Strata of Zhongtiaoshan Tunnel on Menghua Railway. Tunn. Constr. 2014, 34, 1163–1167. (In Chinese) [Google Scholar]
- Peng, W.B. FLAC 3D Practical Tutorial; China Machine Press: Beijing, China, 2008. (In Chinese) [Google Scholar]
- JGJ 138-2016; Code for Design of Composite Structures. China Architecture & Building Press: Beijing, China, 2016. (In Chinese)










| Hole Position Number | No. 4 | No. 2 | No. 0 | No. 3 | |
|---|---|---|---|---|---|
| Depth of Measurement Point | |||||
| 10 (m) | 22.58% | 23.79% | 60.37% | 51.67% | |
| 14 (m) | 39.69% | / | 25.25% | 46.01% | |
| 18 (m) | 10.28% | 18.63% | 32.29% | 38.78% | |
| 20 (m) | 18.49% | 16.74% | 24.62% | 51.07% | |
| Hole Position Number | No. 4 (mm) | No. 2 (mm) | No. 0 (mm) | No. 3 (mm) | |
|---|---|---|---|---|---|
| Depth of Measurement Point | |||||
| 10 (m) | 11.9323 | 11.1051 | 5.3986 | 3.995 | |
| 14 (m) | 14.7126 | / | 11.5848 | 6.5775 | |
| 18 (m) | 22.4222 | 13.9131 | 13.4523 | 8.4912 | |
| 20 (m) | 19.8425 | 17.3692 | 12.2707 | 10.5543 | |
| Measurement Point Number | Upper Step Excavation and Support | Middle Step Excavation and Support | Lower Step Excavation and Support | Inverted Arch Excavation to the Final Stability of the Displacement |
|---|---|---|---|---|
| 4-10 | 15.06% | 16.73% | 15.93% | 29.70% |
| 4-14 | 19.33% | 3.36% | 14.35% | 23.26% |
| 4-18 | 18.39% | 6.30% | 10.59% | 54.44% |
| 4-20 | 20.63% | 6.50% | 10.95% | 43.44% |
| 2-10 | 9.42% | 4.14% | 14.58% | 48.06% |
| 2-18 | 18.50% | 13.43% | 22.46% | 26.97% |
| 2-20 | 24.19% | 3.20% | 15.10% | 40.77% |
| 0-10 | −0.15% | 1.63% | 15.94% | 22.21% |
| 0-14 | 6.15% | 0.48% | 13.43% | 54.69% |
| 0-18 | 7.37% | −0.19% | 14.12% | 46.41% |
| 0-20 | 32.14% | 3.84% | 8.61% | 30.79% |
| 3-10 | 6.87% | 5.01% | 6.00% | 30.46% |
| 3-14 | 5.79% | 11.55% | 9.23% | 27.43% |
| 3-18 | 2.66% | 5.37% | 34.38% | 18.81% |
| 3-20 | 3.29% | 5.63% | 18.45% | 21.55% |
| Function Types | Regression Analysis Method | Equation Form | Linear Transformation Rules | Displacement Convergence Value |
|---|---|---|---|---|
| logarithmic function | linear | u = a + blg(1 + t) | A = a; B = b; X = lg(1 + t); Y = u | (no convergence) |
| nonlinear | u = alg(1 + bt) | / | (no convergence) | |
| exponential function | linear | u = ae−(b/t) | A = lna; B = b; X = −1/t; Y = lnu | a |
| nonlinear | u = a(1 − e-bt) | / | a | |
| hyperbolic function | linear | u = a/(1 + b/t) | A = 1/a; B = b/a; X = 1/t; Y = 1/u | a |
| nonlinear | u = a[1 − 1/(1 + bt)2] | / | a |
| Measurement Point Number | Exponential Function | Hyperbolic Function | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Least-Square Method | L-M Arithmetic | Least-Square Method | L-M Arithmetic | |||||||||
| u = ae−(b/t) | u = a(1 − e−bt) | u = a/(1 + b/t) | u = a[1 − 1/(1 + bt)2] | |||||||||
| a | b | Rc | a | b | Rc | a | b | Rc | a | b | Rc | |
| 4-20 | 19.37 | 15.73 | 0.955 | 18.31 | 0.03 | 0.991 | 26.45 | 43.26 | 0.999 | 22.24 | 0.01 | 0.992 |
| 4-18 | 25.74 | 20.56 | 0.958 | 26.38 | 0.02 | 0.992 | 28.64 | 41.20 | 0.999 | 33.68 | 0.01 | 0.991 |
| 4-14 | 9.27 | 10.21 | 0.965 | 8.24 | 0.05 | 0.979 | 10.68 | 20.25 | 0.999 | 9.38 | 0.03 | 0.978 |
| 4-10 | 10.41 | 10.18 | 0.987 | 9.12 | 0.05 | 0.977 | 11.78 | 19.19 | 0.999 | 10.39 | 0.02 | 0.969 |
| 2-20 | 14.28 | 12.11 | 0.905 | 12.92 | 0.04 | 0.931 | 17.38 | 27.01 | 0.999 | 15.02 | 0.02 | 0.936 |
| 2-18 | 12.47 | 9.65 | 0.981 | 10.91 | 0.06 | 0.971 | 13.88 | 17.39 | 0.999 | 12.35 | 0.03 | 0.963 |
| 2-10 | 10.05 | 17.94 | 0.961 | 8.99 | 0.03 | 0.939 | 13.45 | 46.83 | 0.999 | 11.19 | 0.01 | 0.935 |
| 0-20 | 7.53 | 9.91 | 0.871 | 7.19 | 0.04 | 0.923 | 9.34 | 24.18 | 0.999 | 8.18 | 0.02 | 0.936 |
| 0-18 | 12.98 | 32.81 | 0.969 | 11.67 | 0.03 | 0.945 | 14.26 | 38.64 | 0.967 | 13.94 | 0.01 | 0.949 |
| 0-14 | 12.71 | 31.29 | 0.969 | 11.31 | 0.02 | 0.961 | 14.01 | 34.37 | 0.959 | 13.64 | 0.02 | 0.959 |
| 0-10 | 2.75 | 33.89 | 0.834 | 2.61 | 0.03 | 0.815 | 3.34 | 8.46 | 0.943 | 3.02 | 0.01 | 0.902 |
| 3-20 | 6.30 | 12.31 | 0.937 | 5.59 | 0.04 | 0.934 | 7.68 | 27.46 | 0.933 | 6.58 | 0.02 | 0.934 |
| 3-18 | 6.58 | 11.17 | 0.865 | 5.72 | 0.05 | 0.856 | 7.66 | 22.42 | 0.913 | 6.63 | 0.02 | 0.854 |
| 3-14 | 4.63 | 9.08 | 0.781 | 4.32 | 0.03 | 0.847 | 5.56 | 20.37 | 0.933 | 4.89 | 0.02 | 0.827 |
| 3-10 | 2.37 | 13.14 | 0.865 | 2.36 | 0.03 | 0.919 | 3.29 | 38.27 | 0.928 | 2.81 | 0.01 | 0.926 |
| The average value | 0.920 | 0.932 | 0.971 | 0.936 | ||||||||
| Measurement Point Number | (mm) | (mm) | (mm) |
|---|---|---|---|
| 4-20 | 3.84 | 26.45 | 30.29 |
| 4-18 | 2.51 | 28.64 | 31.15 |
| 4-14 | 6.34 | 10.68 | 17.02 |
| 4-10 | 2.85 | 11.78 | 14.63 |
| 2-20 | 3.78 | 17.38 | 21.16 |
| 2-18 | 2.84 | 13.88 | 16.72 |
| 2-10 | 2.84 | 13.45 | 16.29 |
| 0-20 | 4.45 | 9.34 | 13.79 |
| 0-18 | 5.07 | 14.26 | 19.33 |
| 0-14 | 3.26 | 14.01 | 17.27 |
| 0-10 | 3.45 | 4.34 | 6.79 |
| 3-20 | 4.56 | 7.68 | 12.24 |
| 3-18 | 2.26 | 7.66 | 9.92 |
| 3-14 | 1.90 | 5.56 | 7.46 |
| 3-10 | 1.67 | 3.29 | 4.96 |
| Measurement Point Number | Lee Equation | Hoek Equation | ||||
|---|---|---|---|---|---|---|
| 4-10 | 0.580 | 0.216 | 0.989 | 2.766 | −2.071 | 0.989 |
| 4-14 | 0.313 | 0.200 | 0.979 | 2.748 | −1.525 | 0.983 |
| 4-18 | 0.869 | 0.163 | 0.992 | 3.848 | −2.930 | 0.993 |
| 4-20 | 0.658 | 0.165 | 0.991 | 3.626 | −2.307 | 0.994 |
| 2-10 | 0.622 | 0.158 | 0.975 | 3.796 | −2.198 | 0.979 |
| 2-18 | 0.666 | 0.234 | 0.984 | 2.618 | −2.296 | 0.986 |
| 2-20 | 0.685 | 0.170 | 0.928 | 3.478 | −2.446 | 0.944 |
| 0-10 | 0.043 | 0.109 | 0.885 | 4.598 | −1.073 | 0.887 |
| 0-14 | 0.829 | 0.160 | 0.952 | 3.875 | −2.802 | 0.961 |
| 0-18 | 0.535 | 0.132 | 0.973 | 4.415 | −1.991 | 0.976 |
| 0-20 | 0.369 | 0.139 | 0.938 | 3.956 | −1.662 | 0.948 |
| 3-10 | 0.121 | 0.103 | 0.932 | 5.019 | −1.191 | 0.934 |
| 3-14 | 0.149 | 0.105 | 0.898 | 4.950 | −1.233 | 0.900 |
| 3-18 | 0.326 | 0.131 | 0.912 | 4.202 | −1.549 | 0.915 |
| 3-20 | 0.227 | 0.140 | 0.944 | 3.795 | −1.374 | 0.949 |
| The average value of | 0.951 | 0.955 | ||||
| Structure Types | Gravity () | Poisson Ratio | Internal Friction Angle () | ||
|---|---|---|---|---|---|
| Surrounding rock | 26.8 | 2.4 | 0.28 | 36.67 | 0.10 |
| Advanced support | 32.2 | 2.9 | 0.33 | 44.01 | 0.12 |
| Inverted arch | 25 | 35.5 | 0.20 | / | / |
| Feet-lock bolt | 78.5 | 210 | 0.30 | / | / |
| Steel-reinforced concrete composite structures | 25 | 31 | 0.20 | / | / |
| 23 | 29.7 | 0.20 | / | / | |
| 22 | 28.5 | 0.20 | / | / |
| Sense of Displacement | Vertical Displacement | Lateral Displacement | Longitudinal Displacement |
|---|---|---|---|
| Ahead displacement | 25.80% | 29.01% | 35.69% |
| Upper step excavation | 27.41% | 12.04% | 27.72% |
| Middle step excavation | 25.58% | 17.19% | 27.79% |
| Excavation of lower step inverted arch | 23.20% | 41.76% | 8.79% |
| Burial Depth of Tunnel Vault | Vertical Displacement | Lateral Displacement | Longitudinal Displacement |
|---|---|---|---|
| 10 m | 53.2% | 16.5% | 52.9% |
| 20 m | 33.3% | 14.3% | 28.1% |
| 30 m | 25.1% | 12.1% | 25.7% |
| 40 m | 19.3% | 11.5% | 23.8% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chi, D.; Luo, Y.; Chen, C.; Wang, S.; Wu, Y.; Hu, Y. Analysis of the Surrounding Rock Full-Displacement Variation in Large-Span Mudstone Highway Tunnels. Symmetry 2024, 16, 526. https://doi.org/10.3390/sym16050526
Chi D, Luo Y, Chen C, Wang S, Wu Y, Hu Y. Analysis of the Surrounding Rock Full-Displacement Variation in Large-Span Mudstone Highway Tunnels. Symmetry. 2024; 16(5):526. https://doi.org/10.3390/sym16050526
Chicago/Turabian StyleChi, Dechao, Yanbin Luo, Chengwei Chen, Shengqing Wang, Yunfei Wu, and Yuhang Hu. 2024. "Analysis of the Surrounding Rock Full-Displacement Variation in Large-Span Mudstone Highway Tunnels" Symmetry 16, no. 5: 526. https://doi.org/10.3390/sym16050526
APA StyleChi, D., Luo, Y., Chen, C., Wang, S., Wu, Y., & Hu, Y. (2024). Analysis of the Surrounding Rock Full-Displacement Variation in Large-Span Mudstone Highway Tunnels. Symmetry, 16(5), 526. https://doi.org/10.3390/sym16050526





