Finite Time Stability Analysis and Feedback Control for Takagi–Sugeno Fuzzy Time Delay Fractional-Order Systems

Abstract

This study treats the problem of Finite Time Stability Analysis (FTSA) and Finite Time Feedback Control (FTFC), using a Linear Matrix Inequalities Approach (LMIA). It specifically focuses on Takagi–Sugeno fuzzy Time Delay Fractional-Order Systems (TDFOS) that include nonlinear perturbations and interval Time Varying Delays (ITVDs). We consider the case of the Caputo Tempered Fractional Derivative (CTFD), which generalizes the Caputo Fractional Derivative (CFD). Two main results are presented: a two-step procedure is provided, followed by a more relaxed single-step procedure. Two examples are presented to show the reduction in conservatism achieved by the proposed methods. The first is a numerical example, while the second involves the FTFC of an inverted pendulum, which exhibits both symmetrical dynamics for small angular displacements and asymmetrical dynamics for larger deviations.

1. Introduction

Over the past few decades, a lot of work has been conducted to push the boundaries of advanced stability analysis for different types of symmetrical and asymmetrical systems, from simpler models to highly complex ones [1,2]. The main challenges in setting up the design conditions are often related to the specific class of stability analysis involved. In the literature, stability analysis typically falls into two categories: asymptotic stability analysis and Finite Time Stability Analysis (FTSA). It is important to note that FTSA is far more complex than asymptotic stability because it ensures the system’s states converge within a finite time, rather than over an infinite time horizon. On the other hand, the complexity of the class of system considered can make the design conditions much more difficult. Indeed, a system may be more complicated than another due to a variety of factors. In this study, we focus on three main factors: the nonlinear dynamic, the presence of a time delay, particularly an Interval Time Varying Delay (ITVD), and finally the fractional-order derivative particulary Caputo Tempered Fractional Derivative (CTFD), which is more general than the standard Caputo Fractional Derivative (CFD). The next sections provide the state-of-the-art of results related to these factors.

Since its creation by Takagi and Sugeno in 1985 [3], nonlinear systems can be effectively represented by a set of local linear models seamlessly integrated by the membership function, the Takagi–Sugeno fuzzy model. So, using the Linear Matrix Inequalities Approach (LMIA), a vast number of studies have been conducted to address stability analyses and the control of a broader range of systems described by the Takagi–Sugeno fuzzy model (e.g., [4,5]). It is widely recognized that time delay is a common phenomenon in various systems. In 2000, Takagi–Sugeno fuzzy models were expanded by Cao and Franck [6] to address nonlinear systems with time delays. There has been a surge in research activity on stability analyses and the control of delayed Takagi–Sugeno fuzzy models (e.g., [7,8,9]).

In recent years, the study of Fractional-Order Systems (FOS) has attracted increasing interest. Various types of fractional derivatives have appeared in the literature, such as the Caputo Fractional Derivative (CFD) [10,11] and the Caputo Tempered Fractional Derivative (CTFD) [12]. It is well known that the CTFD involves the exponential function, which has distinct properties and applications in fractional calculus. Stability results for FOS obtained using the CTFD are often superior compared to those obtained using the standard CFD [13]. Since their introduction, significant efforts have been made to provide advanced stability analysis methods for FOS, resulting in a variety of new techniques. For example, Chen et al., in [14], studied the stability of FOS with multiple orders. Chen et al., in [15], investigated the asymptotic stability of a class of neural networks’ FOS. Ben Makhlouf, in [16], presented the partial practical stability of FOS depending on a small parameter. Some other results have appeared regarding investigations of the analysis and control problems presented by Time Delay Fractional-Order Systems (TDFOS). The issue of sliding mode control for linear TDFOS is investigated in [17]. Using an integral inequality, He et al. [18] delved into the problem of asymptotic stability for various classes of differential TDFOS. Ben Makhlouf, in [19], studied the FTSA of nonlinear TDFOS using a fixed point approach. Naifar et al., in [19], presented the quasiuniform stability of FOS neural networks with mixed delay using the generalized Gronwall inequality. Thanh et al., in [20], studied the FTSA of FOS with ITVD. Phat et al., in [21], proved the FTSA of singular TDFOS. The problem of event-triggered control is treated in [18]. Based on LMIA and Mittag–Leffler function, sufficient conditions, which can be solved via the LMI Toolbox, were proposed in [20] to study the FTSA of a fractional-order linear system with ITVD. The CFD was considered in this work.

Motivated by the work presented in [20], we provide a more general version of the given LMIs conditions. In this concept, the proposed results allow for a lot more flexibility when it comes to resolving these LMIs, making solutions easier to find.

Inspired by the discussions above, this study explores the FTSA and the FTFC problems of Takagi–Sugeno TDFOS with nonlinear perturbations via LMIA. Cases of both CFD and CTFD are considered. The main highlights of this paper are as follows:

• Takagi–Sugeno fuzzy modeling is extensively employed for approximating nonlinear dynamics, including FOS. Recent papers have extended the modeling approach of FOS to include time-varying delay using the CFD. However, there are no studies dealing with the combination of CTFD and delayed Takagi–Sugeno fuzzy models in the literature. This work presents the first investigation of the class of delayed Takagi–Sugeno fuzzy models with a tempered derivative.
• This paper investigates the finite time stability problem of Takagi–Sugeno fuzzy TDFOS with CTFD. This problem is more challenging than the asymptotic stability problem for two reasons. Firstly, finite time stability is sensitive to initial conditions. Indeed, the stability is guaranteed for a restricted region of initial conditions, which requires stricter constraints compared to asymptotic stability. Secondly, the convergence of the system state is imposed onto to a ball centered at the origin within a finite time.
• The FTSA and FTFC results can be directly derived from the class of FOS with CFD as corollaries of the results obtained for the case of CTFD. This offers a unified framework that permits the seamless transition between CTFD and CFD.
• In [20], the LMI conditions depend on a specific positive scalar, which is the upper bound of the delay. The proposed results provide greater flexibility in resolving these LMIs. In fact, this upper bound can be any positive scalar.
• Two means of FTSA and FTFC are developed. In the first one, two stages are proposed in the analysis. LMIs are firstly solved and a minimizing problem, containing a Mittag–Leffler function, is then adressed. In the second way, the previous two stages are solved in only one stage, which allows us to reduce the conservatism.

The remainder of this paper is organized as follows: Section 2 covers the preliminaries, providing the necessary definitions for CTFD and finite time stability. In addition, the Takagi–Sugeno fuzzy TDFOS is presented. In Section 3, we present the FTSA of the considered model; this is followed by Section 4, where we address the FTFC. Section 5 illustrates our theoretical findings through two examples, and finally, Section 6 concludes the paper.

Notations: ${\left\{A\right\}}_{Sym}=A+A^T$.

2. Preliminaries

The purpose of this section is to provide some basic information about tempered fractional calculus.

Definition 1

([13]). Let $\delta >0,\tau \ge 0$ and $v\in L^1\left([c_1,c_2],\mathbb{R}\right)$. The tempered fractional integral of order δ of v is given by

$$\begin{array}{cc}\hfill I_{c_1^{+}}^{\delta ,\tau }v\left(\varkappa \right)& ={\displaystyle \frac{1}{\Gamma \left(\delta \right)}}{\int }_{c_1}^{\varkappa }{\left(\varkappa -w\right)}^{\delta -1}exp\left(-\tau \left(\varkappa -w\right)\right)v\left(w\right)dw,\hfill \end{array}$$

where $I_{c_1^{+}}^{\delta }$ is the Riemann–Liouville fractional integral of order δ.

Definition 2

([13]). Let $q\in \mathbb{N},q-1<\delta <q,\tau \ge 0,$ and $v\in A{C}^q[c_1,c_2]$. The CTFD of order δ of v is given by

$${}^{C}D_{c_1^{+}}^{\delta ,\tau }v\left(\varkappa \right)={\displaystyle \frac{exp(-\tau \varkappa )}{\Gamma (q-\delta )}}{\int }_{c_1}^{\varkappa }{\left(\varkappa -w\right)}^{q-\delta -1}{v}_{\tau }^{\left[q\right]}\left(w\right)dw,$$

(1)

where

$$v_{\tau }^{\left[q\right]}\left(\varkappa \right)={\left[{\displaystyle \frac{d}{d\varkappa }}\right]}^q\left(exp\left(\tau \varkappa \right)v\left(\varkappa \right)\right).$$

(2)

If $0<\delta <1$, then the Caputo tempered fractional derivative of order δ for $v\in AC[c_1,c_2]$ is given by:

$${}^{C}D_{c_1^{+}}^{\delta ,\tau }v\left(\varkappa \right)={\displaystyle \frac{exp(-\tau \varkappa )}{\Gamma (1-\delta )}}{\int }_{c_1}^{\varkappa }{\left(\varkappa -w\right)}^{-\delta }{\displaystyle \frac{d}{dw}}\left(exp\left(\tau w\right)v\left(w\right)\right)dw.$$

(3)

Definition 3

([11]). The Mittag–Leffler function is given by

$${\displaystyle E_{l_1,l_2}\left(s\right)=\sum _{\kappa =0}^{+\infty }\frac{s^{\kappa }}{\Gamma (\kappa l_1+l_2)},}$$

where $l_1>0$, $l_2>0$, $s\in \mathbb{C}$.

If $l_2=1$, we consider $E_{l_1}\left(s\right)=E_{l_1,1}\left(s\right)$.

Lemma 1

([13]). Let $P=P^T\in {\mathbb{R}}^{n\times n}$, $0<\delta <1$ and an absolutely continuous function $y:[c_1,c_2]⟶{\mathbb{R}}^n$; therefore,

$${}^{C}D_{c_1^{+}}^{\delta ,\tau }yTPy\left(\varkappa \right)\le 2y{\left(\varkappa \right)}^T{P}^C{D}_{c_1^{+}}^{\delta ,\tau }y\left(\varkappa \right).$$

(4)

Lemma 2

([13]). Consider the system

$${}^{C}D_{{{\varkappa }_0}^{+}}^{\delta ,\tau }v\left(\varkappa \right)=l_{1}v+l_2\left(\varkappa \right),\varkappa \ge {\varkappa }_0$$

(5)

where $0<\delta <1$ and $v\in {\mathbb{R}}^n$. The solution of the system is as follows: (2)

$$\begin{array}{ccc}\hfill v\left(\varkappa \right)& =& exp\left(-\tau (\varkappa -{\varkappa }_0)\right)E_{\delta }\left(l_1{(\varkappa -{\varkappa }_0)}^{\delta }\right)u\left({\varkappa }_0\right)\hfill \\ & +& {\int }_{{\varkappa }_0}^{\varkappa }{(\varkappa -w)}^{\delta -1}{E}_{\delta ,\delta }\left(l_1{(\varkappa -w)}^{\delta }\right)exp\left(-\tau (\varkappa -w)\right)l_2\left(w\right)dw.\hfill \end{array}$$

(6)

Lemma 3

([21]). Let $T\ge 0$, $m>0$$M_1\ge 1$, $M_2\ge 0$ and a function $H:[-m,T]⟶{\mathbb{R}}_{+}$ be non-decreasing. Then,

$$H\left(t\right)\le M_{1}H\left(0\right)+M_{2}H(t-m),\forall t\in [-m,T]$$

implies

$${\displaystyle H\left(t\right)\le M_{1}H\left(0\right)\sum _{\kappa =0}^{\left[\frac{T}{m}\right]+1}{M}_2^{\kappa }.}$$

In the following, let us consider the Takagi-=Sugeno TDFOS:

The model rule is as follows: $s(s\in \mathbb{S}=\{1,2,\cdots ,f\left\}\right)$. If $J_1\left(\zeta \right)$ is $Q_{s1}$ and $J_p\left(\zeta \right)$ is $Q_{sp}$, then

$$\left\{\begin{array}{cc}{}^{C}D_{0^{+}}^{\delta ,\tau }x\left(\zeta \right)\hfill & ={\phi }_{s}x\left(\zeta \right)+D_{s}x(\zeta -\iota \left(\zeta \right))+B_s\mu \left(\zeta \right)+f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right))),0\le \zeta \le T,\hfill \\ x\left(\zeta \right)\hfill & =\sigma \left(\zeta \right),\zeta \in [-{\iota }^{*},0],\hfill \end{array}\right.$$

(7)

with ${\phi }_s\in {\mathbb{R}}^{n\times n}$, $D_s\in {\mathbb{R}}^{n\times n}$, $B_s\in {\mathbb{R}}^{n\times m}$, $\sigma \in C\left([-{\iota }^{*},0],{\mathbb{R}}^n\right)$ and $0<{\iota }_{*}\le \iota \left(\zeta \right)\le {\iota }^{*}$, $\forall \zeta \ge 0$. $x\left(\zeta \right)$, $\mu \left(\zeta \right)$ and $f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))$ representing the state, the control input, and the nonlinear perturbations, respectively.

Assumption 1.

Assume that $\exists {\mathbf{𝘍}}_{1s}$ and ${\mathbf{𝘍}}_{2s}$, such that $f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))$, verifying the Lipschitz condition given below:

$$\begin{array}{c}\hfill f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))\le x^T\left(\zeta \right){\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}x\left(\zeta \right)+x^T(\zeta -\iota \left(\zeta \right)){\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}x(\zeta -\iota \left(\zeta \right)).\end{array}$$

(8)

Consider the following feedback controller:

The model rule is as follows: $s(s\in \mathbb{S}=\{1,2,\cdots ,f\left\}\right)$. If $J_1\left(\zeta \right)$ is $Q_{s1}$ and ⋯ and $J_p\left(\zeta \right)$ is $Q_{sp}$ then

$$\begin{array}{c}\hfill \mu \left(\zeta \right)={\Xi }_{s}x\left(\zeta \right),\end{array}$$

(9)

where ${\Xi }_s$ are feedback gains.

Using the fuzzy blending, (7) and (9) are expressed as follows:

$$\begin{array}{c}\hfill {\displaystyle {}^{C}D_{0^{+}}^{\delta ,\tau }x\left(\zeta \right)=\sum _{s=1}^f{\kappa }_s\left(J\left(\zeta \right)\right)({\phi }_{s}x\left(\zeta \right)+B_s\mu \left(\zeta \right)+D_{s}x(\zeta -\iota \left(\zeta \right))+f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))),}\end{array}$$

(10)

$${\displaystyle \mu \left(\zeta \right)=\sum _{t=1}^f{\kappa }_t\left(J\left(\zeta \right)\right){\Xi }_{t}x\left(\zeta \right),}$$

(11)

where

$${\kappa }_s\left(J\left(\zeta \right)\right)={\displaystyle \frac{{\prod }_{\varsigma =1}^p{Q}_{s\varsigma }\left(J_{\varsigma }\left(\zeta \right)\right)}{{\sum }_{\iota =1}^r{\prod }_{\varsigma =1}^p{Q}_{s\varsigma }\left(J_{\varsigma }\left(\zeta \right)\right)}}\mathrm{in}\mathrm{which}J\left(\zeta \right)=[J_1\left(\zeta \right),\dots ,J_p\left(\zeta \right)].$$

It is clear that

$$\begin{array}{c}\hfill {\kappa }_s\left(J\left(\zeta \right)\right)\ge 0,\sum _{s=1}^f{\kappa }_s\left(J\left(\zeta \right)\right)=1.\end{array}$$

(12)

In the rest, ${\kappa }_s$ is used to denote ${\kappa }_s\left(J\left(\zeta \right)\right)$. Combining (10) and (11), we get

$$\begin{array}{c}\hfill {\displaystyle {}^{C}D_{0^{+}}^{\delta ,\tau }x\left(\zeta \right)=\sum _{s=1}^f\sum _{t=1}^f{\kappa }_s{\kappa }_t(({\phi }_s+B_s{\Xi }_t)x\left(\zeta \right)+D_{s}x(\zeta -\iota \left(\zeta \right))+f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))).}\end{array}$$

(13)

Definition 4

([21]). The FOS (13) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$ if

$${\parallel \sigma \parallel }^2\le {\alpha }_1,$$

This implies the following:

$${\parallel x\left(\zeta \right)\parallel }^2\le {\alpha }_2,\forall \zeta \in [0,T],$$

with ${\displaystyle \parallel \sigma \parallel =\underset{\zeta \in [-{\iota }^{*},0]}{sup}\parallel \sigma \left(\zeta \right)\parallel }$.

Lemma 4

([22]). For the given matrices $\mathcal{S}$ and $\mathcal{T}$, we have

$$\begin{array}{c}\hfill 2\mathcal{S}\mathcal{T}\le {\displaystyle \frac{1}{\theta }}\mathcal{S}{\mathcal{S}}^T+\theta {\mathcal{T}}^T\mathcal{T},\end{array}$$

(14)

This is satisfied for any $\theta \in {\mathbb{R}}^{+}$.

For the sake of brevity, we use $x,x_{\iota },\mu$ and $f_s$ instead of $x\left(\zeta \right),x(\zeta -\iota (\zeta \left)\right),\mu \left(\zeta \right)$ and $f_s(\zeta ,x\left(\zeta \right),x(\zeta -\iota \left(\zeta \right)))$, respectively.

3. FTSA for Takagi–Sugeno TDFOS

In this section, we consider (13) with $\mu =0$:

$$\begin{array}{c}\hfill {\displaystyle {}^{C}D_{0^{+}}^{\delta ,\tau }x=\sum _{s=1}^f{\kappa }_s\left({\phi }_{s}x+D_s{x}_{\iota }+f_s\right).}\end{array}$$

(15)

Theorem 1.

For $\varsigma >0$, the FOS (15) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$ if there are $P\in {\mathbb{R}}^{n\times n}$ with $P=P^T>0$ and $\theta >0$, such that

$$\begin{array}{ccc}\hfill \left[\begin{array}{cc}{\left\{P{\phi }_s\right\}}_{Sym}+{\displaystyle \frac{1}{\theta }}PP+\theta {\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}-\varsigma P& P{D}_s\\ *& \theta {\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}-\varsigma P\end{array}\right]& <& 0\hfill \end{array}$$

(16)

$$\begin{array}{ccc}\hfill {\displaystyle \frac{{\lambda }_{max}\left(P\right)}{{\lambda }_{min}\left(P\right)}{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }{\alpha }_1}& <& {\alpha }_2,\hfill \end{array}$$

(17)

where

$$\begin{array}{c}\hfill {\displaystyle M_1=\underset{0\le l\le T}{sup}\left(exp\left(-\tau l\right)E_{\delta ,1}\left(\varsigma l^{\delta }\right)\right).}\end{array}$$

(18)

Proof. 

Let

$$\begin{array}{c}\hfill \vartheta \left(\zeta \right)=x^{T}Px.\end{array}$$

(19)

Using Lemma 1, we can obtain

$$\begin{array}{c}\hfill {}^{C}D_{0^{+}}^{\delta ,\tau }\vartheta \left(\zeta \right)\le 2x^{T}P{}^{C}D_{0^{+}}^{\delta ,\tau }x=2x^{T}P({\phi }_{s}x+D_s{x}_{\iota }+f).\end{array}$$

(20)

Based on Lemma 4, we have

$$\begin{array}{c}\hfill 2x^{T}Pf\le x^T\left({\displaystyle \frac{1}{\theta }}PP\right)x+\theta f^{T}f.\end{array}$$

(21)

Combining (20) and (21), we obtain

$${}^{C}D_{0^{+}}^{\delta ,\tau }\vartheta \left(\zeta \right)\le {\xi }^T\left[\begin{array}{cc}{\left\{P{\phi }_s\right\}}_{Sym}+{\displaystyle \frac{1}{\theta }}PP+\theta {\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}& P{D}_s\\ *& \theta {\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}\end{array}\right]\xi ,$$

based on inequality (8), where $\xi =\left[\begin{array}{c}x\\ x_{\iota }\end{array}\right]$.

The inequality (16) implies that

$${}^{C}D_{0^{+}}^{\delta ,\tau }\vartheta \left(\zeta \right)\le \varsigma \vartheta \left(\zeta \right)+\varsigma x{\left(\zeta -\iota \left(\zeta \right)\right)}^{T}Px\left(\zeta -\iota \left(\zeta \right)\right).$$

(22)

Let

$${}^{C}D_{0^{+}}^{\delta ,\tau }\vartheta \left(\zeta \right)-\varsigma \vartheta \left(\zeta \right).$$

Using (6) and (22), we can obtain

$$\begin{array}{ccc}\hfill \vartheta \left(\zeta \right)& =& {\displaystyle exp\left(-\tau \zeta \right)E_{\delta ,1}\left(\varsigma {\zeta }^{\delta }\right)\vartheta \left(0\right)+{\int }_0^{\zeta }{\left(\zeta -l\right)}^{\delta -1}exp\left(-\tau (\zeta -l)\right)E_{\delta ,\delta }\left(\varsigma {\left(\zeta -l\right)}^{\delta }\right)g\left(l\right)dl}\hfill \\ & \le & exp\left(-\tau \zeta \right)E_{\delta ,1}\left(\varsigma {\zeta }^{\delta }\right)\vartheta \left(0\right)\hfill \\ & +& {\displaystyle \varsigma {\int }_0^{\zeta }{\left(\zeta -l\right)}^{\delta -1}exp\left(-\tau (\zeta -l)\right)E_{\delta ,\delta }\left(\varsigma {\left(\zeta -l\right)}^{\delta }\right)x{\left(l-\iota \left(l\right)\right)}^{T}Px\left(l-\iota \left(l\right)\right)dl}\hfill \\ & \le & exp\left(-\tau \zeta \right)E_{\delta ,1}\left(\varsigma {\zeta }^{\delta }\right)\vartheta \left(0\right)\hfill \\ & +& {\displaystyle \varsigma \underset{0\le l\le \zeta }{sup}\left(x{\left(l-\iota \left(l\right)\right)}^{T}Px\left(l-\iota \left(l\right)\right)\right){\int }_0^{\zeta }{\left(\zeta -l\right)}^{\delta -1}{E}_{\delta ,\delta }\left(\varsigma {\left(\zeta -l\right)}^{\delta }\right)dl.}\hfill \end{array}$$

(23)

From Lemma 9 in [23], we can obtain the following:

$$\begin{array}{c}\hfill {\displaystyle {\int }_0^{\zeta }{\left(\zeta -l\right)}^{\delta -1}{E}_{\delta ,\delta }\left(\varsigma {\left(\zeta -l\right)}^{\delta }\right)dl={\zeta }^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma {\zeta }^{\delta }\right).}\end{array}$$

(24)

Therefore,

$$\begin{array}{ccc}\hfill \vartheta \left(\zeta \right)& \le & {\displaystyle exp\left(-\tau \zeta \right)E_{\delta ,1}\left(\varsigma {\zeta }^{\delta }\right)\vartheta \left(0\right)+\varsigma \underset{-{\iota }^{*}\le l\le \zeta -{\iota }_{*}}{sup}\vartheta \left(l\right){\zeta }^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma {\zeta }^{\delta }\right)}\hfill \\ & \le & {\displaystyle exp\left(-\tau \zeta \right)E_{\delta ,1}\left(\varsigma {\zeta }^{\delta }\right){\sigma }^T\left(0\right)P\sigma \left(0\right)+\varsigma \underset{-{\iota }^{*}\le l\le \zeta -{\iota }_{*}}{sup}\vartheta \left(l\right){\zeta }^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma {\zeta }^{\delta }\right).}\hfill \end{array}$$

(25)

For $0\le \nu \le \zeta \le T$, we can obtain

$${\displaystyle \vartheta \left(\nu \right)\le \underset{0\le l\le T}{sup}\left(exp\left(-\tau l\right)E_{\delta ,1}\left(\varsigma l^{\delta }\right)\right){\sigma }^T\left(0\right)P\sigma \left(0\right)+\varsigma \underset{-{\iota }^{*}\le l\le \zeta -{\iota }_{*}}{sup}\vartheta \left(l\right)T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right).}$$

(26)

Therefore,

$${\displaystyle \underset{-{\iota }^{*}\le l\le \zeta }{sup}\vartheta \left(l\right)\le \underset{0\le l\le T}{sup}\left(exp\left(-\tau l\right)E_{\delta ,1}\left(\varsigma l^{\delta }\right)\right)\underset{-{\iota }^{*}\le l\le 0}{sup}{\sigma }^T\left(l\right)P\sigma \left(l\right)+\varsigma \underset{-{\iota }^{*}\le l\le \zeta -{\iota }_{*}}{sup}\vartheta \left(l\right)T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right).}$$

(27)

Let ${\displaystyle H\left(\zeta \right)=\underset{-{\iota }^{*}\le l\le \zeta }{sup}\vartheta \left(l\right)}$, $M_2=\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right).$

Then,

$$H\left(\zeta \right)\le M_{1}H\left(0\right)+M_{2}H(\zeta -{\iota }_{*}),\forall \zeta \in [0,T].$$

It follows from Lemma 3 that

$${\displaystyle H\left(\zeta \right)\le M_{1}H\left(0\right)\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{M}_2^{\kappa }.}$$

Thus,

$${\displaystyle {\parallel x\left(\zeta \right)\parallel }^2\le \frac{{\lambda }_{max}\left(P\right)}{{\lambda }_{min}\left(P\right)}{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }\underset{-{\iota }^{*}\le l\le 0}{sup}{\parallel \sigma \left(l\right)\parallel }^2.}$$

Hence, if

$${\displaystyle \underset{-{\iota }^{*}\le l\le 0}{sup}{\parallel \sigma \left(l\right)\parallel }^2<{\alpha }_1,}$$

and

$${\displaystyle \frac{{\lambda }_{max}\left(P\right)}{{\lambda }_{min}\left(P\right)}{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }{\alpha }_1<{\alpha }_2,}$$

therefore,

$${\parallel x\left(\zeta \right)\parallel }^2\le {\alpha }_2.$$

Then, the FOS is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$. □

Remark 1.

Due to the existence of the term $PP$ in (16) and ${\lambda }_{max}\left(P\right),{\lambda }_{min}\left(P\right)$ in condition (17), the problem in Theorem 1 cannot be solved by the LMI Toolbox in one step. We propose, therefore, the next Theorem, which allows for the problem to be solved in two steps.

Theorem 2.

Thw following two steps should be confirmed:

Step 1: For a given $\varsigma >0$, there are $P\in {\mathbb{R}}^{n\times n}$ with $P=P^T>0$ and $\theta \in {\mathbb{R}}^{+}$, such that the following LMIs are satisfied:

$$\left[\begin{array}{ccc}{\left\{P{\phi }_s\right\}}_{Sym}+\theta {\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}-\varsigma P& P{D}_s& P\\ *& \theta {\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}-\varsigma P& 0\\ & *& -\theta I\end{array}\right]<0.$$

(28)

Step 2: For the given ${\alpha }_1>0$ and $T>0$, minimize ${\alpha }_2$ subject to (17);

then, the FOS (15) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$

Proof. 

(28) can be directly obtained by applying the Schur complement to (16) and solving (28) and (17) separately. □

Remark 2.

The two-step method, proposed in Theorem 2, is too conservate. In order to relax this result, we propose, in the next Theorem, conditions which can be solved by the LMI Toolbox in only one step.

Theorem 3.

For a given $\varsigma >0$, if the following one step is confirmed:

Minimize ${\alpha }_2$ subject to: $\exists \{P=P^T\in {\mathbb{R}}^{n\times n},{\epsilon }_1\in \mathbb{R},{\epsilon }_2\in \mathbb{R},\theta \in {\mathbb{R}}^{+}\}$ such that (28) and the following LMIs are satisfied:

$$\begin{array}{ccc}\hfill & & 0<{\epsilon }_{1}I<P<{\epsilon }_{2}I\hfill \end{array}$$

(29)

$$\begin{array}{ccc}\hfill & & {\displaystyle {\epsilon }_2{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }{\alpha }_1-{\epsilon }_1{\alpha }_2<0.}\hfill \end{array}$$

(30)

where $M_1$ is given in (18), then, the FOS (15) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$.

Proof. 

The one-step result is obtained by bounding P. □

When considering Takagi–Sugeno TDFOS with CFD and without perturbations,

$${\displaystyle {}^{C}D_{0^{+}}^{\delta ,\tau }x\left(\zeta \right)=\sum _{s=1}^f{\kappa }_s\left({\phi }_{s}x\left(\zeta \right)+D_{s}x(\zeta -\iota \left(\zeta \right))\right),0\le \zeta \le T,}$$

(31)

we derive the following two-step and one-step corollaries:

Corollary 1.

The following two steps should be confirmed:

Step 1: For a given $\varsigma >0$, there is $P\in {\mathbb{R}}^{n\times n}$ with $P=P^T>0$ such that the following LMIs are satisfied:

$$\left[\begin{array}{cc}{\left\{P{\phi }_s\right\}}_{Sym}-\varsigma P& P{D}_s\\ *& -\varsigma P\end{array}\right]<0.$$

(32)

Step 2: For a given ${\alpha }_1>0$ and $T>0$, minimize ${\alpha }_2$ subject to (17) for ${\displaystyle M_1=E_{\delta ,1}\left(\varsigma T^{\delta }\right)}$;

then, the FOS (31) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$.

Corollary 2.

For a given $\varsigma >0$, the following one step should be confirmed: Minimize ${\alpha }_2$ subject to: $\exists \{P=P^T\in {\mathbb{R}}^{n\times n},{\epsilon }_1\in \mathbb{R},{\epsilon }_2\in \mathbb{R}\}$ such that (29)–(32) and (30) for ${\displaystyle M_1=E_{\delta ,1}\left(\varsigma T^{\delta }\right)}$ are satisfied; then, the FOS (31) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$.

4. FTFC Takagi–Sugeno TDFOS

Now, we consider the system presented in (13).

Theorem 4.

For a given $\varsigma >0$, the FOS (13) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$ if there are $\beta \in {\mathbb{R}}^{+},$$X\in {\mathbb{R}}^{n\times n}$ with $X=X^T>0$ and $Y_t\in {\mathbb{R}}^{m\times n}$, such that

$$\begin{array}{ccc}\hfill & & {\mathsf{\Omega }}_{st}<0\mathit{for}t,s=1,\dots ,f\hfill \end{array}$$

(33)

$$\begin{array}{ccc}\hfill & & {\displaystyle \frac{{\lambda }_{max}\left(X\right)}{{\lambda }_{min}\left(X\right)}{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }{\alpha }_1<{\alpha }_2,}\hfill \end{array}$$

(34)

where

$$\begin{array}{c}\hfill {\mathsf{\Omega }}_{ts}=\left[\begin{array}{cccc}{\{{\phi }_{s}X+B_s{Y}_t\}}_{Sym}+\beta I-\varsigma X& D_{s}X& X{\mathbf{𝘍}}_{1s}^T& 0\\ *& -\varsigma X& 0& X{\mathbf{𝘍}}_{2s}^T\\ *& *& -\beta I& 0\\ & *& *& -\beta I\end{array}\right],\end{array}$$

(35)

in which $M_1$ is given by (18). If the previous conditions are satisfied, we can obtain

$$\begin{array}{c}\hfill {\Xi }_t=Y_t{X}^{-1}.\end{array}$$

(36)

Proof. 

Consider the Lyapunov function as presented in (19). Following a similar approach as in the proof of Theorem 1, we obtain:

$${}^{C}D_{0^{+}}^{\delta ,\tau }\vartheta \left(\zeta \right)\le {\xi }^T\left[\begin{array}{cc}P\{{\phi }_s+B_s{K}_t){\}}_{Sym}+{\displaystyle \frac{1}{\theta }}PP+\theta {\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}-\varsigma P& P{D}_s\\ *& \theta {\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}-\varsigma P\end{array}\right]\xi ;$$

then, the FTS of the closed-loop system (13) is ensured if

$$\begin{array}{ccc}\hfill \left[\begin{array}{cc}P\{{\phi }_s+B_s{K}_t){\}}_{Sym}+{\displaystyle \frac{1}{\theta }}PP+\theta {\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}-\varsigma P& P{D}_s\\ *& \theta {\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}-\varsigma P\end{array}\right]& <& 0,\hfill \end{array}$$

(37)

and (17) are satisfied.

By pre- and post-multiplying (37) by $diag(X,X)$ in which $X=P^{-1}$, we can obtain

$$\begin{array}{ccc}\hfill \left[\begin{array}{cc}{\{{\phi }_{s}X+B_s{Y}_t\}}_{Sym}+\beta I+{\displaystyle \frac{1}{\beta }}X{\mathbf{𝘍}}_{1s}^T{\mathbf{𝘍}}_{1s}X-\varsigma X& D_{s}X\\ *& {\displaystyle \frac{1}{\beta }}X{\mathbf{𝘍}}_{2s}^T{\mathbf{𝘍}}_{2s}X-\varsigma X\end{array}\right]& <& 0,\hfill \end{array}$$

(38)

where $\beta ={\displaystyle \frac{1}{\theta }}$.

After applying the Schur complement, (38) is equivalent to (33).

Since ${\lambda }_{min}\left(P\right)={\displaystyle \frac{1}{{\lambda }_{max}\left(X\right)}}$ and ${\lambda }_{max}\left(P\right)={\displaystyle \frac{1}{{\lambda }_{min}\left(X\right)}}$, then (34) and (17) are equivalent. □

Similarly to FTSA results, we can obtain the following FTFC results:

Theorem 5.

The following two steps should be confirmed:

Step 1: For a given $\varsigma >0$, there are $\beta \in {\mathbb{R}}^{+},$$X\in {\mathbb{R}}^{n\times n}$, with $X=X^T>0$ and $Y_t\in {\mathbb{R}}^{m\times n}$, such that LMIs (33) are satisfied.

Step 2: X is calculated in step 1. For a given ${\alpha }_1>0$ and $T>0$, minimize ${\alpha }_2$ subject to (34); then, the FOS (13) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$.

Proof. 

Due to the presence of an unknown matrix X in (33) and ${\lambda }_{max}\left(X\right)$, ${\lambda }_{min}\left(X\right)$ in (34), these conditions cannot be solved using existing solvers like the LMI Toolbox or YALMIP in MATLAB. Therefore, the problem is solved in two steps. In the first one, we compute X, which is then utilized in the second step. □

Theorem 6.

For a given $\varsigma >0$, the following step should be completed:

Minimize ${\alpha }_2$ subject to $\exists \{X=X^T\in {\mathbb{R}}^{n\times n},Y_s\in {\mathbb{R}}^{m\times n}{\epsilon }_1\in \mathbb{R},{\epsilon }_2\in \mathbb{R},\beta \in {\mathbb{R}}^{+}\}$ such that (33) and the following LMIs are satisfied:

$$\begin{array}{ccc}& & 0<{\epsilon }_{1}I<X<{\epsilon }_{2}I\hfill \end{array}$$

(39)

$$\begin{array}{ccc}& & {\displaystyle {\epsilon }_2{M}_1\sum _{\kappa =0}^{\left[\frac{T}{{\iota }_{*}}\right]+1}{\left[\varsigma T^{\delta }{E}_{\delta ,\delta +1}\left(\varsigma T^{\delta }\right)\right]}^{\kappa }{\alpha }_1-{\epsilon }_1{\alpha }_2<0,}\hfill \end{array}$$

(40)

where $M_1$ is given in (18) Then, the FOS (13) is FTS with respect to $\{{\alpha }_1,{\alpha }_2,T\}$, ${\alpha }_1<{\alpha }_2$.

Proof. 

By bounding X within ${\epsilon }_{1}I$ and ${\epsilon }_{2}I$, the problem can be solved in a single step. Indeed, ${\epsilon }_1<{\lambda }_{min}\left(X\right)$ and ${\epsilon }_2>{\lambda }_{max}\left(X\right)$. □

Remark 3.

All previous results can be applied to linear TDFOS when $s=1,{\phi }_s=\phi$, $B_s=B,D_s=D,f_s=f,{\mathbf{𝘍}}_{1s}={\mathbf{𝘍}}_1,{\mathbf{𝘍}}_{2s}={\mathbf{𝘍}}_2$.

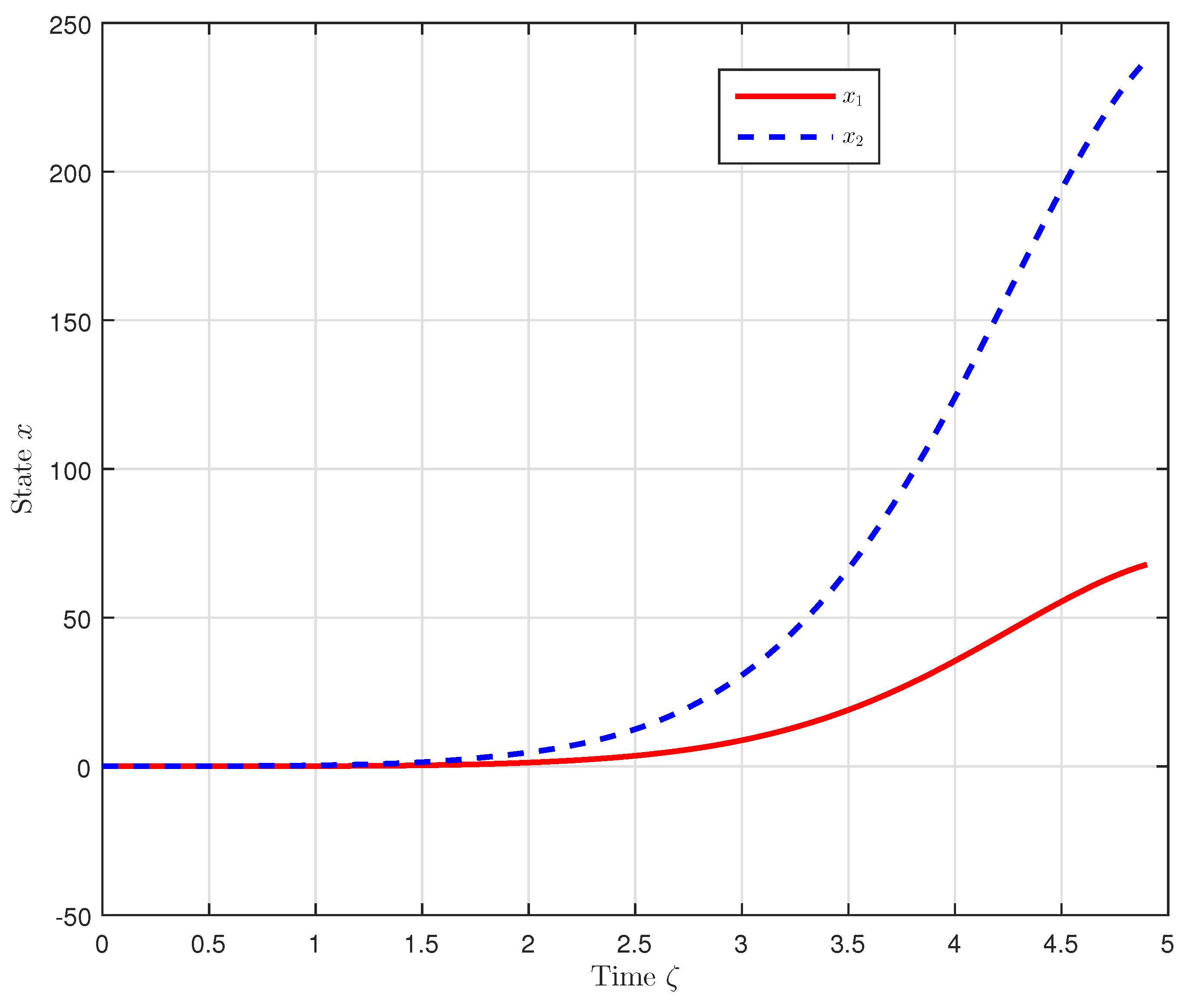
5. Illustrative Examples

5.1. Example 1

In order to demonstrate the advantage of our results compared to [20], we consider a linear FOS without control $\mu =0$, in which

$$\phi =\left[\begin{array}{cc}-31& 0\\ 0& -2.3\end{array}\right],D=\left[\begin{array}{cc}0.95& 0.85\\ 0& -1.9\end{array}\right],f=\left[\begin{array}{c}{\mathbf{𝘍}}_{1s}x\\ {\mathbf{𝘍}}_{2s}{x}_{\iota }\end{array}\right],B=\left[\begin{array}{c}0\\ 10\end{array}\right],\delta =0.7,T=2.8.$$

The ITVD is $\iota \left(\zeta \right)=2.3+0.3sin\left(\zeta \right)$. It is clear that ${\iota }_{*}=2$ and ${\iota }^{*}=2.6$.

Case 1: $\tau =0$ (The CFD case) and without perturbations ${\mathbf{𝘍}}_{1s}={\mathbf{𝘍}}_{1s}=\left[\begin{array}{cc}0& 0\end{array}\right]$.

The design procedure that provides the minimum of ${\alpha }_2$ involving LMIs conditions that can be obtained in one step is better that the two-step approach for two main reasons. The first one is the reduction in computational effort. Indeed, when the LMI problem and the minimization problem are solved simultaneously, the interaction between the two problems is handled automatically, avoiding the requirement of multiple recalculations. The second reason is the reduction in conservatism. In fact, when the two problems are solved together, the optimization problem can consider both simultaneously, leading to a possibly more integrated optimal solution.

Table 1. Comparison results of the minimum ${\alpha }_2$ for the CFD case without perturbations.

Methods	Minimum Allowed ${\mathit{\alpha }}_2$
Theorem of Thanh et al. [20]	${10}^{14}$
Corollary 1 for $\varsigma ={\iota }^{*}=2.6$	${10}^{14}$
Corollary 2 for $\varsigma ={\iota }^{*}=2.6$	${10}^{13}$
Corollary 1 for $\varsigma =0.9$	425
Corollary 2 for $\varsigma =0.9$	37

lists the comparison results regarding the minimum allowed ${\alpha }_2$, which was obtained in this paper using the theorem of Thanh et al. [20] and Corollaries 1 and 2. This demonstrates not only the advantage of the one-step result but also the importance of selecting a specific positive scalar $\varsigma$, which must not be equal to ${\iota }^{*}$.

Case 2: $\tau =1$ (The CTFD case) and with perturbations ${\mathbf{𝘍}}_1=\left[\begin{array}{cc}0.1& 0.5\end{array}\right]$, ${\mathbf{𝘍}}_2=\left[\begin{array}{cc}0.1& 0.2\end{array}\right]$.

According to (18), we get $M_1=1.19$ for $\zeta =0.605$.

Our objective is to calculate the minimum allowable ${\alpha }_2$, c and ${\alpha }_1=0.01$, such that the system is stable within a finite time.

Table 2. Comparison results of the minimum ${\alpha }_2$ for CTFD case.

Methods	Minimum Allowed ${\mathit{\alpha }}_2$
Theorem 2 for $\varsigma =0.9$	$29.4$
Theorem 3 for $\varsigma =0.9$	$2.8$

lists the comparison results regarding the minimum allowed ${\alpha }_2$ via Theorems 2 and 3. It can be seen in this table that the one-step process produces better results than the two-step process.

The time evolution of $x\left(\zeta \right)$ is shown in Figure 1 for the initial condition $\sigma \left(t\right)=\left[0.01-0.01\right].$

5.2. Example 2: Application to an Inverted Pendulum

The nonlinear dynamics of the inverted pendulum system are modeled using a two-rule Takagi–Sugeno fuzzy model [24]. The first rule describes the symmetrical dynamics, where the angular displacement is about 0, while the second rule captures the asymmetrical dynamics, with the angular displacement of about $\pm {\displaystyle \frac{\pi }{2}}$. The overall Takagi–Sugeno fuzzy model with perturbed delay and CTFD is as follows:

$$\begin{array}{c}\hfill {\displaystyle {}^{C}D_{0^{+}}^{\delta ,\tau }x=\sum _{s=1}^2{\kappa }_s\left(r{\phi }_{s}x+(1-r){\phi }_s{x}_{\iota }+B_s\mu +f_s\right),}\end{array}$$

(41)

where

$$\begin{array}{c}{\phi }_1=\left[\begin{array}{cc}0& 1\\ 17.249& 0\end{array}\right],{\phi }_2=\left[\begin{array}{cc}0& 1\\ 12.6305& 0\end{array}\right],B_1=\left[\begin{array}{c}0\\ -0.1765\end{array}\right],B_2=\left[\begin{array}{c}0\\ -0.0779\end{array}\right],\delta =0.7,\hfill \\ T=4.9,\tau =0.3,{\kappa }_1=(1-{\displaystyle \frac{1}{1+exp(-7(x_1-0.25\pi ))}})\times ({\displaystyle \frac{1}{1+exp(-7(x_1+0.25\pi ))}}),{\kappa }_2=1-{\kappa }_1.\hfill \end{array}$$

We chose $r=0.9,$ the ITVD and the initial conditions provided in Example 1, $f_1=f_2=\left[\begin{array}{c}\mathbf{𝘍}x\\ \mathbf{𝘍}{x}_{\iota }\end{array}\right]$ where $\mathbf{𝘍}=\left[\begin{array}{cc}0.1& 0\end{array}\right].$ Figure 2 shows the time evolution of $x\left(\zeta \right)$ without control. It demonstrates that the open-loop system is not finite-time-stable, as the states diverge over time.

Now, our objective is to design an FTFC. Therefore, by solving LMIs in Theorem 6, we can obtain: The minimum of ${\alpha }_2$ is $1.4$ and the gains are as follows:

$${\Xi }_1=\left[\begin{array}{cc}97.6276& 39.4687\end{array}\right],{\Xi }_2=\left[\begin{array}{cc}154.5451& 89.1207\end{array}\right].$$

Figure 3 shows the time evolution of $x\left(\zeta \right)$ with FTFC. It illustrates that the FTFC successfully prevents divergence. It is clear that $x_1$ and $x_2$ converge within $T=4.9$ s to a small ball.

6. Conclusions

This paper investigates the FTSA and FTFC challenges of using Takagi–Sugeno TDFOS with ITVD. By utilizing the Linear Matrix Inequalities Approach (LMIA), we establish conditions that account for nonlinear perturbations. Two methods are introduced: the first is presented in two steps, while the second is achieved in just one step. These conditions are solved using the LMI toolbox. The updated results are presented as straightforward corollaries for CFD and linear models. To highlight the effectiveness of these results, two illustrative examples are provided. Future research will explore the FTSA problem of singular Takagi–Sugeno TDFOS, which is significantly more challenging than that used for regular systems, as it affects both the regularity and the impulse-free issues. Additionally, improvements can be made by investigating challenges such as uncertainties and sensor faults. In future work, we will study the FTSA and FTFC of impulsive fractional-order time delay systems.