Bifurcations Associated with Three-Phase Polynomial Dynamical Systems and Complete Description of Symmetry Relations Using Discriminant Criterion
Abstract
:1. Introduction
2. A Family of Polynomial Dynamical Systems
2.1. Matrix Representation of Polynomial Dynamical Systems
2.2. Discriminant Criterion and Matrix Representation of 3D Polynomial Dynamical Systems
2.3. Symmetry Relations on the Sets of Coefficient Matrices and D-Vectors
3. Classification of Solutions to Autonomous Polynomial Equations
3.1. Representations of Autonomous and Integrable Polynomial Dynamical Systems
3.2. General Solutions to Autonomous Second-Order Polynomial Equations
- (a) ;In what follows, we will consider the equations with
- (b) D > 0; there are three solution familieswhere the − and + signs correspond, respectively, to and :Family U, with : solutions are not stable, since there is a “movable” singular point with ; next, they are (i) monotonically increasing because for C > 0; (ii) satisfy the condition ; and (iv) have two horizontal asymptotes .Family S, with : solutions are stable and .Family T, : and are time-independent solutions such that and the first corresponds to in . These stationary solutions and are ’nonisolated’: in every neighborhood, there is an infinite number of ‘regular’ solutions or .
- (c), : all the corresponding solutionsare not stable.
- (d), : the corresponding solutionsare not stable.
3.3. Equivalence Classes of D-Vectors and General Solutions to Autonomous Polynomial Equation Systems
3.4. Description of All Possible Solution Combinations in Terms of Discriminants
3.5. Analysis of Solutions to Cauchy Problems
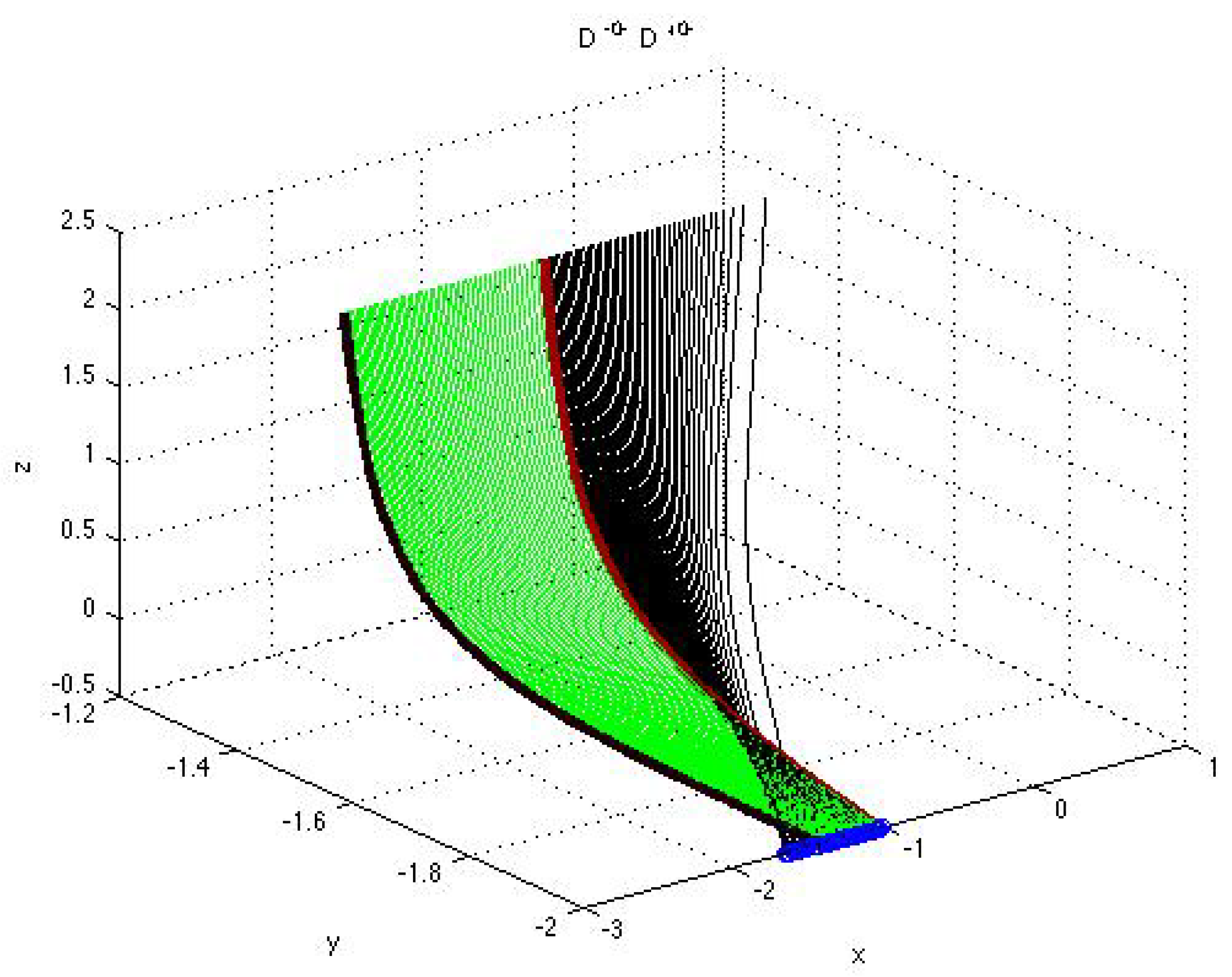
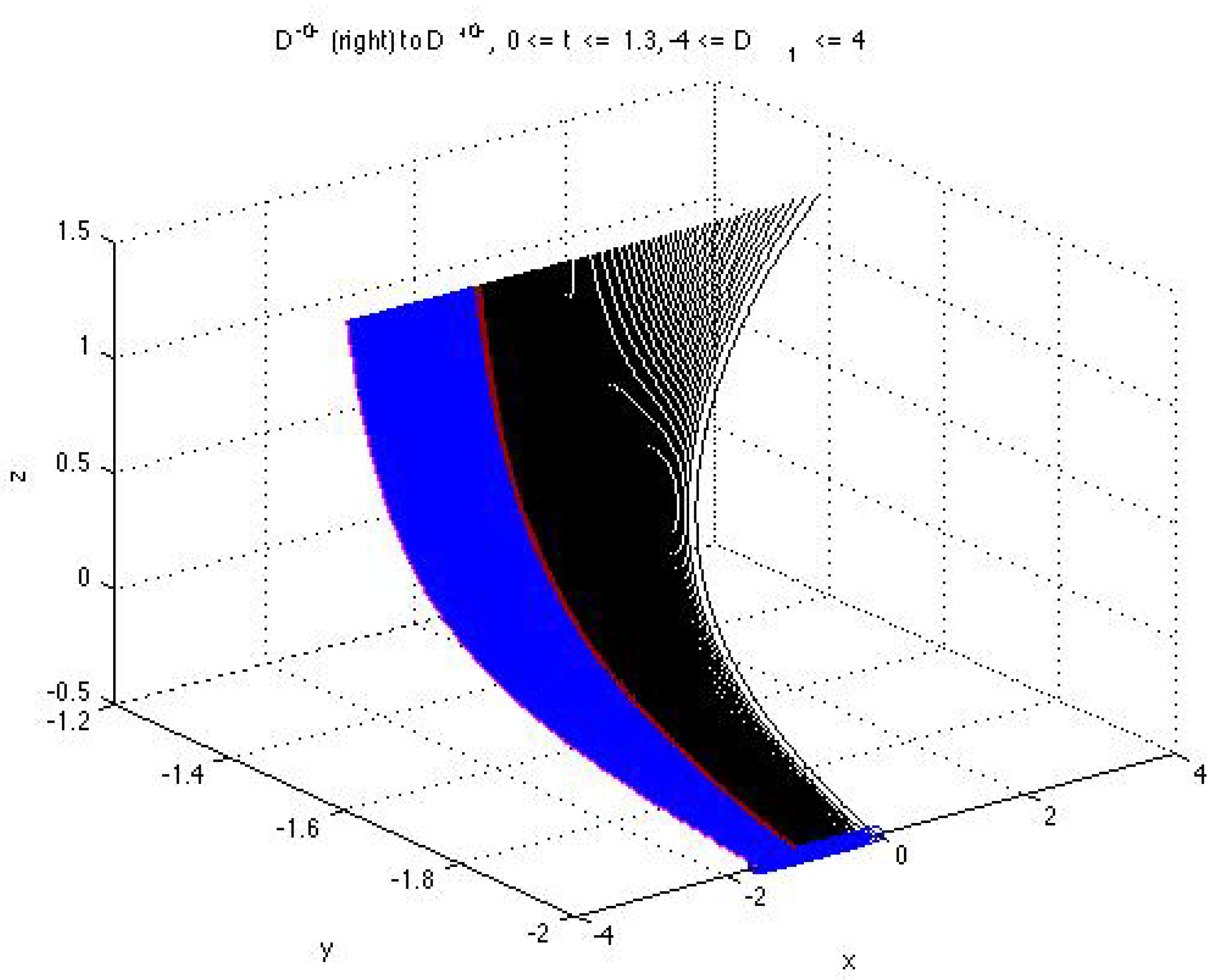
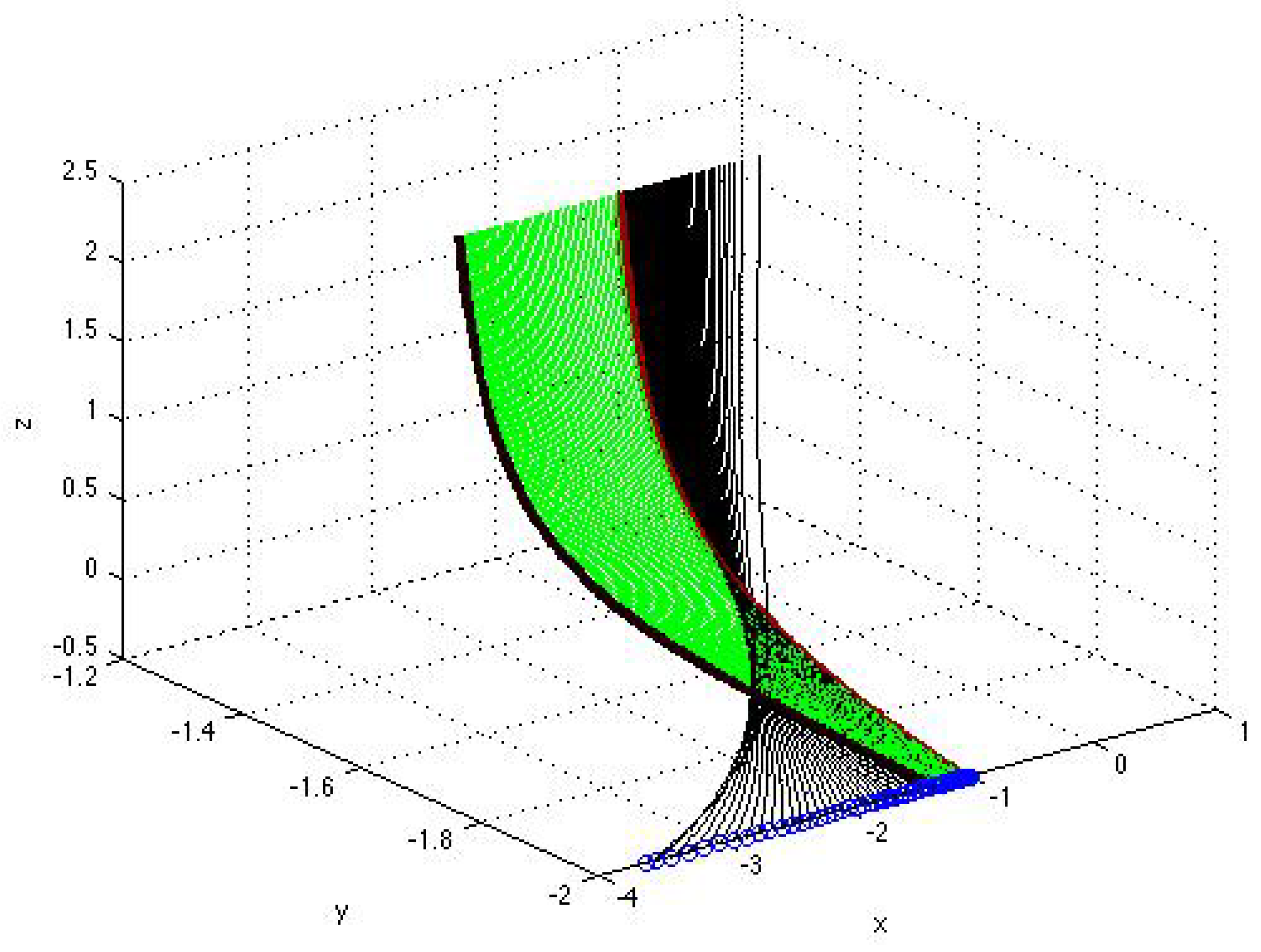
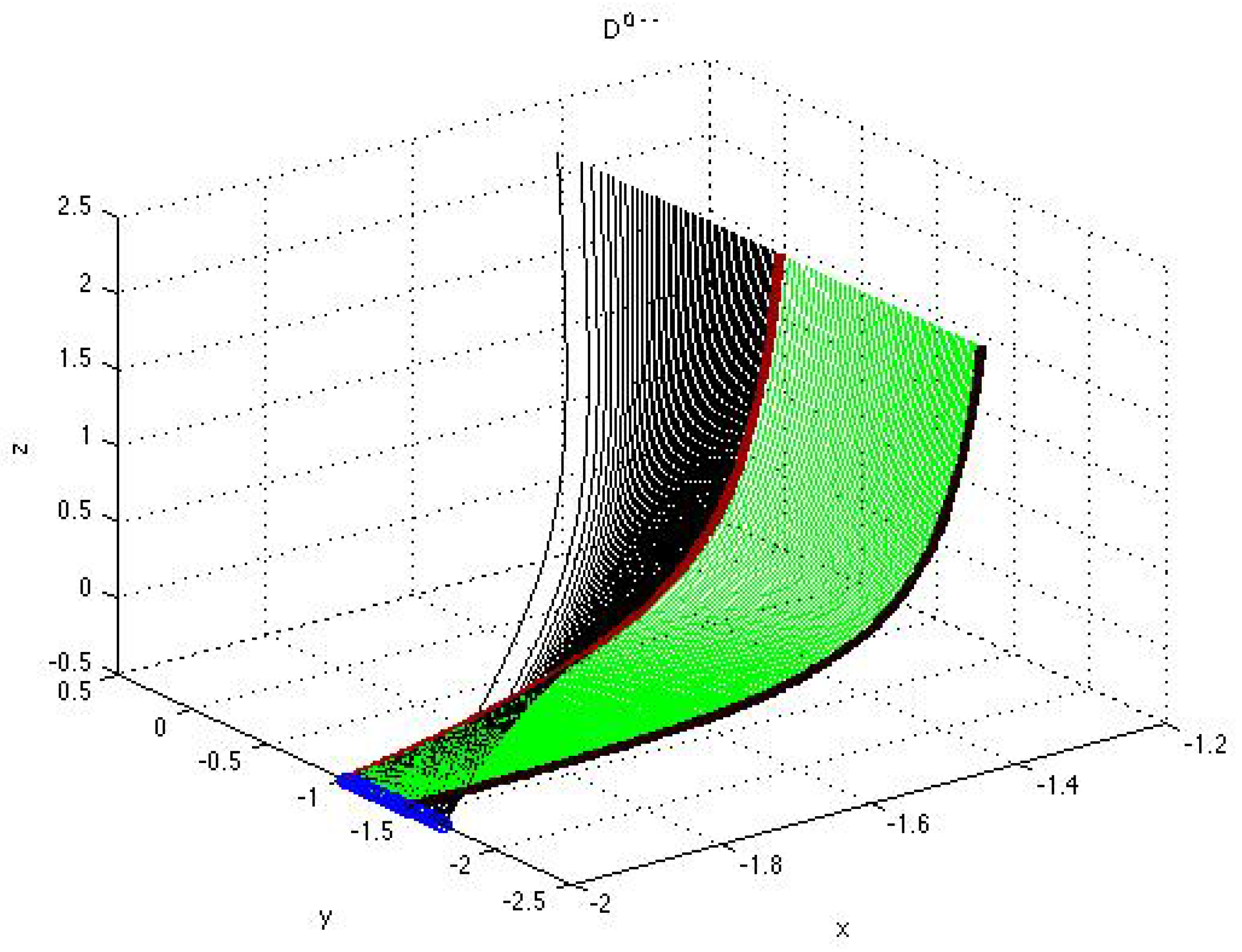
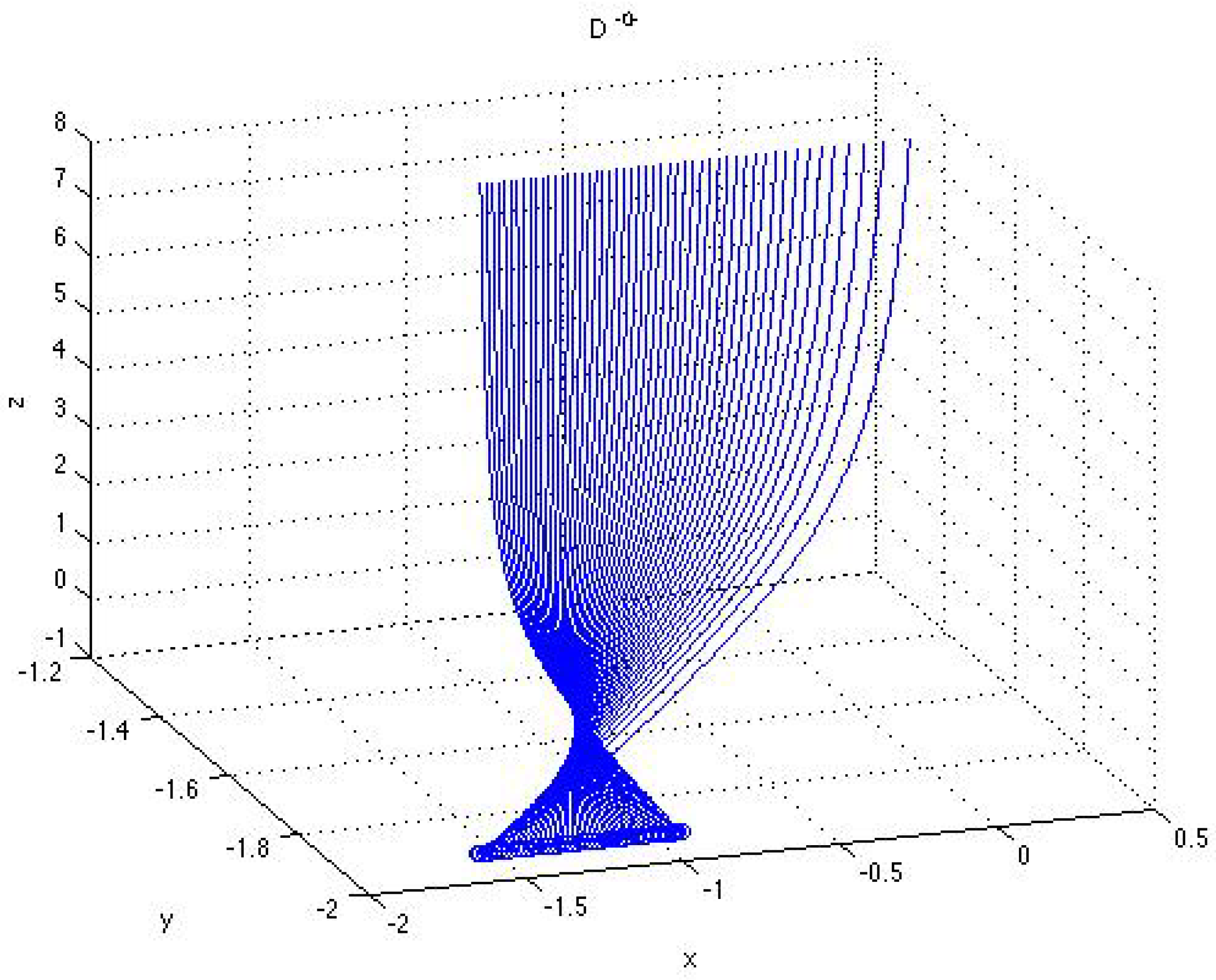
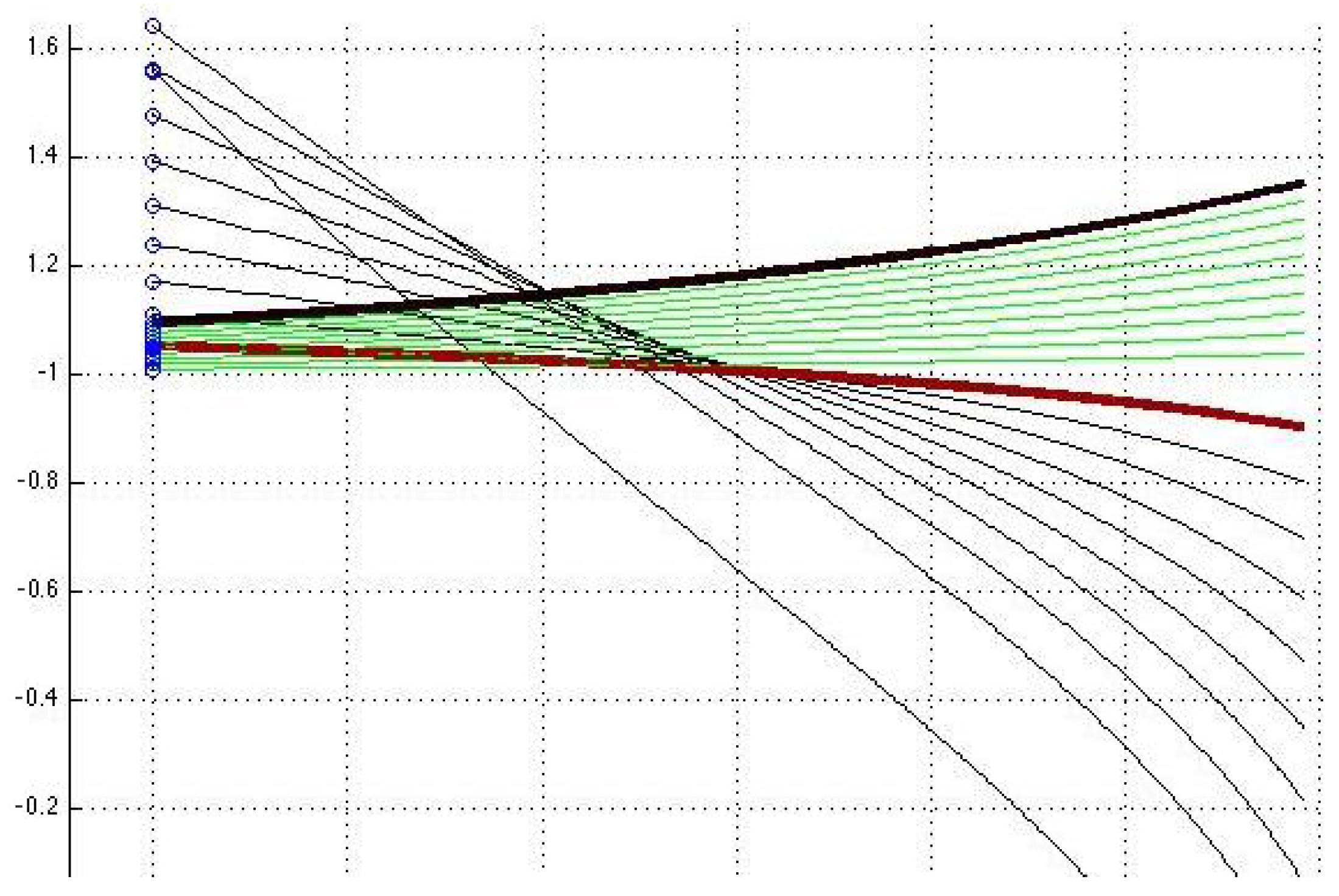
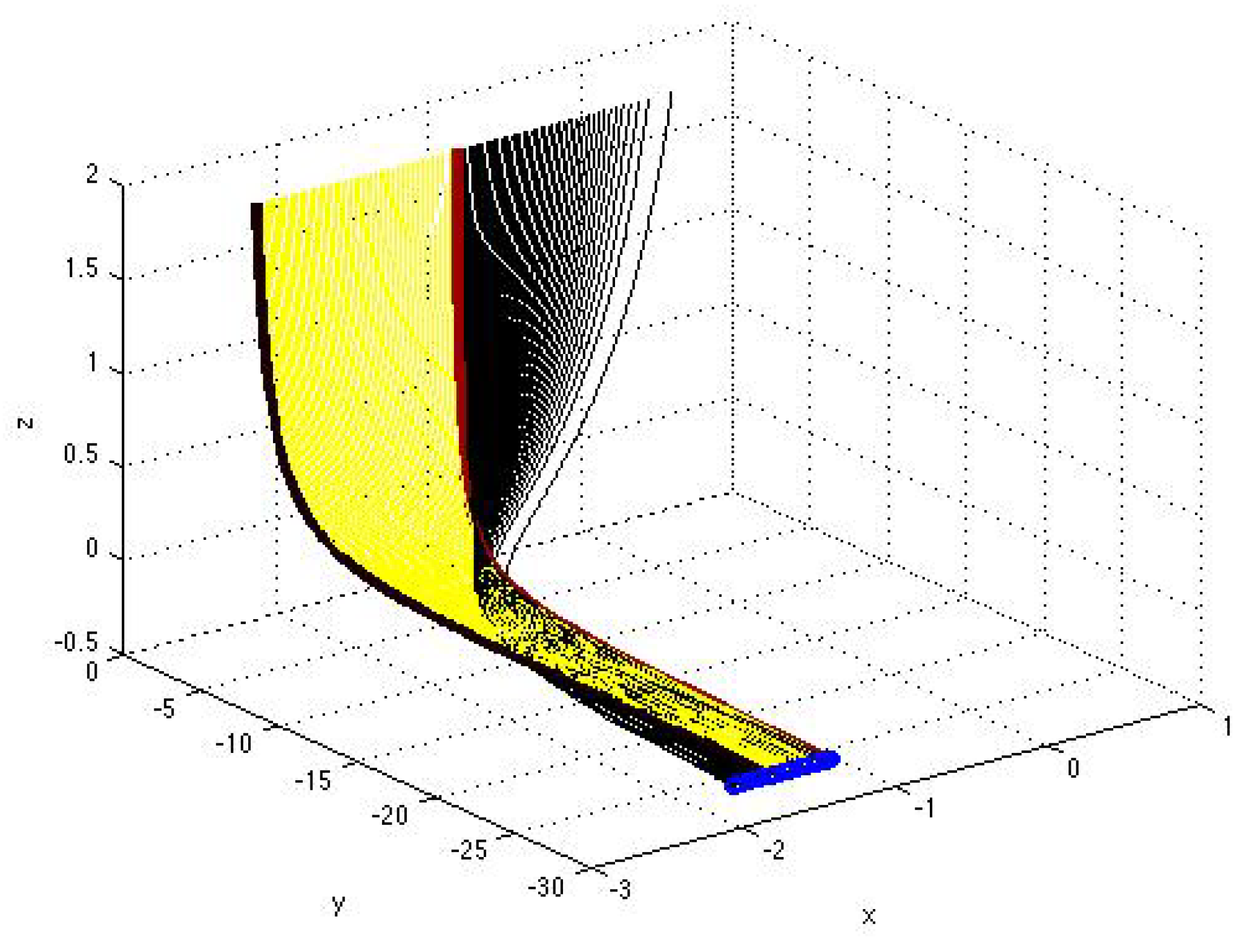
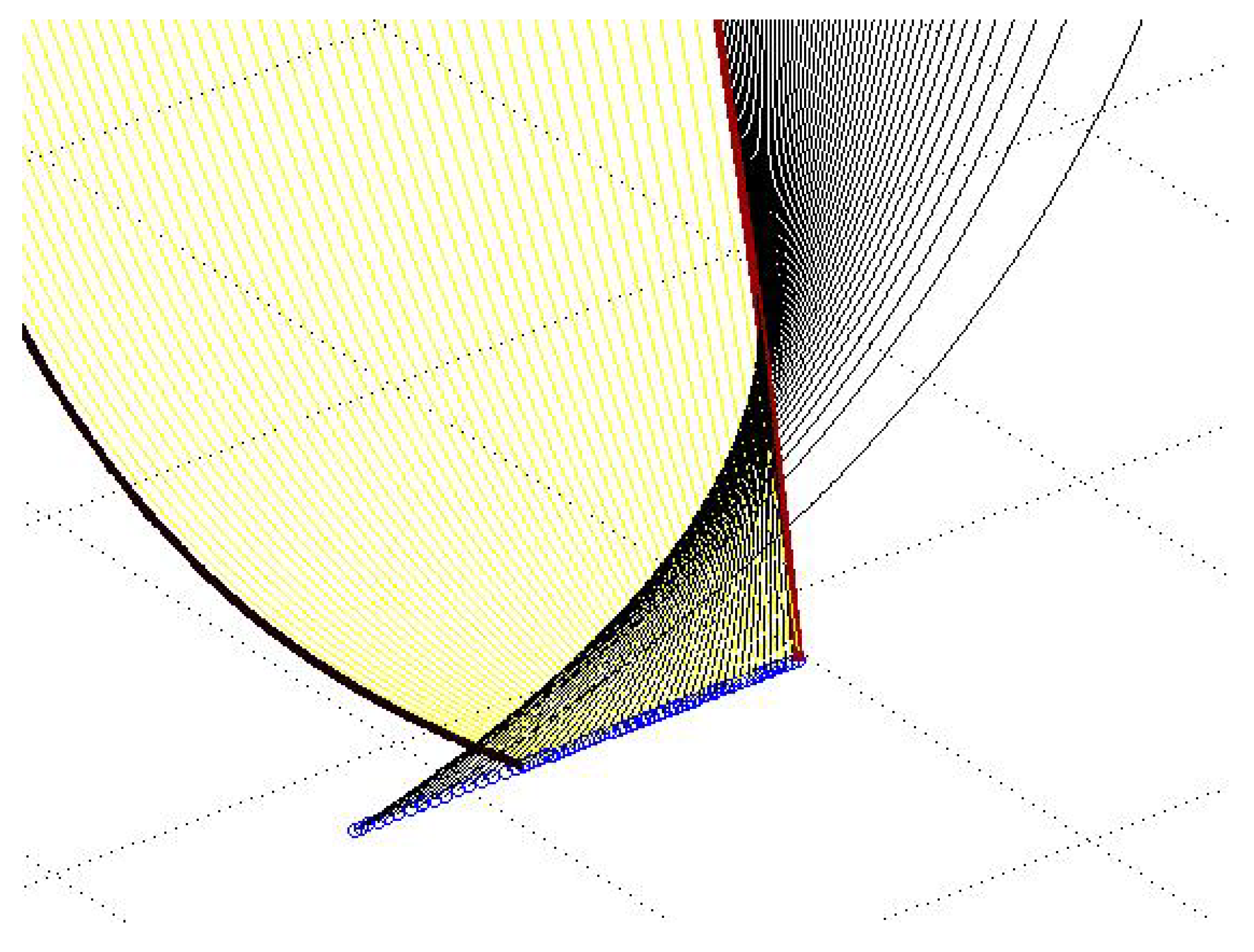
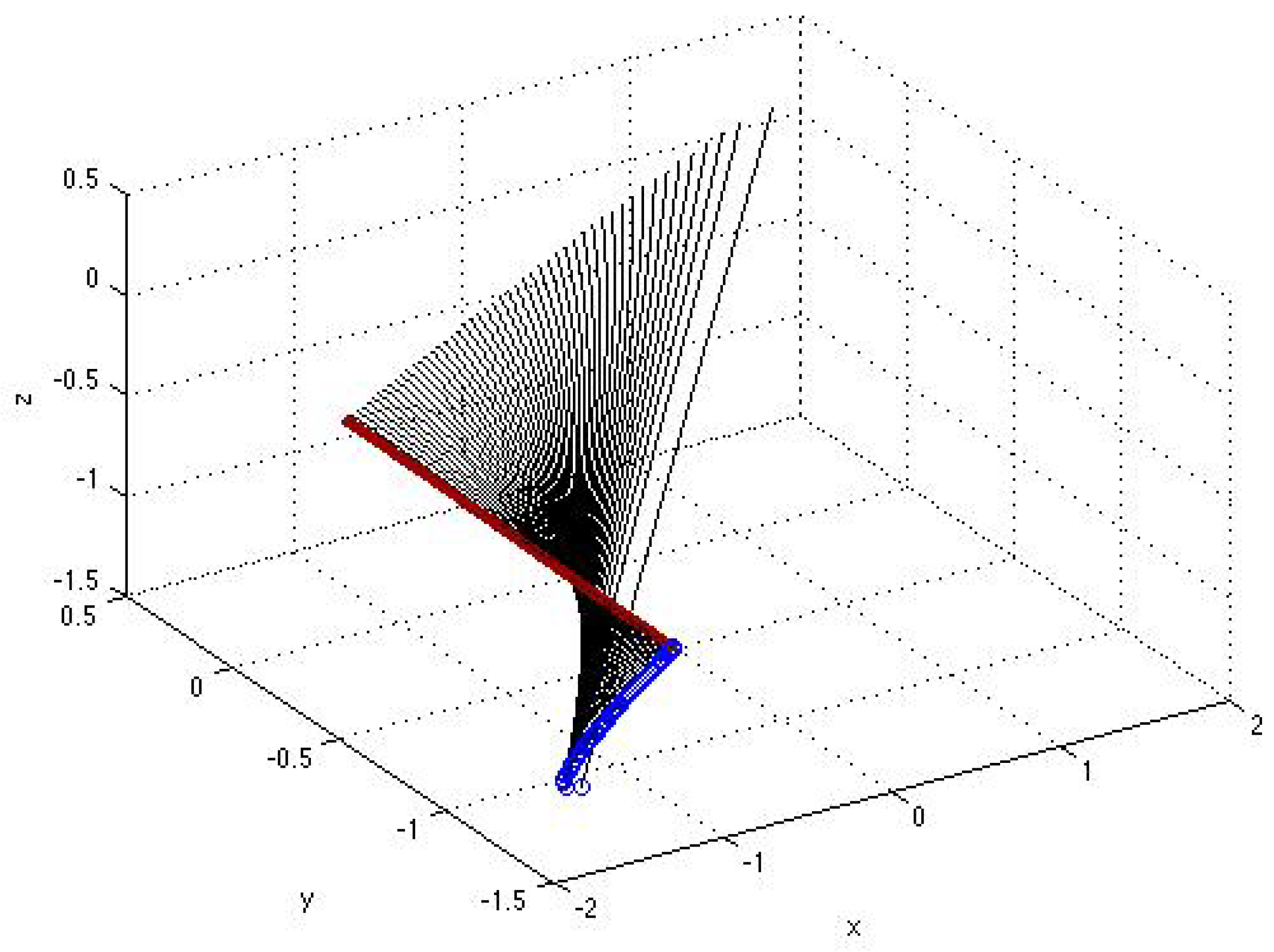
4. Analysis of Bifurcations
5. Conclusions
- -
- To develop the method of S- and D-vectors and the discriminant criterion to the polynomial DSs of higher dimensions and the order of the involved polynomials.
- -
- To clarify that the only type of bifurcations that may occur in quadratic polynomial DSs investigated in this paper is the discovered ‘twisted fold’.
- -
- To investigate the relations between the described symmetries of the D- and S-vectors and the possible symmetries of solutions to the polynomial DSs.
- -
- To find the symmetry-breaking bifurcations characteristic to the polynomial DSs under study.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Autonomous Polynomial Dynamical Systems Integrable in Elementary Functions
Appendix A.2. Examples with Bifurcations
References
- Gaiko, V.A. Global bifurcations and chaos in polynomial dynamical systems. In Proceedings of the 2003 International Conference Physics and Control. Proceedings, St. Petersburg, Russia, 20–22 August 2003; Volume 2, pp. 670–674. [Google Scholar] [CrossRef]
- Gaiko, V.A. Global Bifurcation Theory and Hilbert’s Sixteenth Problem. In Book Series: Mathematics and Its Applications; Kluwer: Boston, MA, USA, 2003; Volume 562. [Google Scholar]
- Luo, A. Polynomial Functional Dynamical Systems, E-Book; Springer International Publishing: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Bautin, N.N.; Leontovich, E.A. Methods and Examples of the Qualitative Analysis of Dynamical Systems in a Plane; Nauka: Moscow, Russia, 1990. [Google Scholar]
- Gaiko, V.A. On Global Bifurcation Theory of Polynomial Dynamical Systems and Its Applications. In Communications in Difference Equations; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar] [CrossRef]
- Shestopalov, Y.V.; Shakhverdiev, A.K. Qualitative theory of two-dimensional polynomial dynamical systems. Symmetry 2021, 13, 1884. [Google Scholar] [CrossRef]
- Buckley, I.; Leverett, M.C. Mechanism of Fluid Displacement in Sands. Trans. AIME 1942, 146, 107–119. [Google Scholar] [CrossRef]
- Suleimanov, B.A.; Guseynova, N.I.; Veliyev, E.F. Control of Displacement Front Uniformity by Fractal Dimensions. In Proceedings of the SPE-187784-MS, SPE Russian Petroleum Technology Conference, Moscow, Russia, 16–18 October 2017. [Google Scholar] [CrossRef]
- Suleimanov, B.A.; Veliyev, E.F.; Naghiyeva, N.V. Preformed particle gels for enhanced oil recovery. Int. J. Mod. Phys. B 2020, 34, 2050260. [Google Scholar] [CrossRef]
- Shakhverdiev, A.K. System optimization of non-stationary flooding for the purpose of increasing oil recovery. Pet. Eng. 2019, 44–49. [Google Scholar] [CrossRef]
- Shakhverdiev, A.K.; Arefiev, S.V.; Denisov, A.V.; Yunusov, R.R. Method for restoring the optimal mode of operation of the reservoir-well system, taking into account the instability of the displacement front. Oil Ind. 2020, 6, 52–57. [Google Scholar] [CrossRef]
- Shakhverdiev, A.K.; Arefiev, S.V. The concept of monitoring and optimization of oil reservoirs waterflooding under the conditions of displacement front instability. Oil Ind. 2021, 11, 104–109. [Google Scholar] [CrossRef]
- Shakhverdiev, A.K. Once again about oil recovery factor. Neft. Khozyaystvo 2014, 1, 44–48. [Google Scholar]
- Drozdov, A.N.; Gorelkina, E.I. Method of measuring the rates of water-gas mixtures injection wells during the exploitation of oil fields. Socar Proc. 2022, 1–8. [Google Scholar] [CrossRef]
- Suleimanov, B.A.; Ismailov, F.S.; Dyshin, O.A.; Veliyev, E.F. Selection methodology for screening evaluation of EOR methods. Pet. Sci. Technol. 2016, 34, 961–970. [Google Scholar] [CrossRef]
- Craig Forrest, F., Jr. The Reservoir Engineering Aspects of Waterflooding; Society of Petroleum Engineers of AIME: New York, NY, USA, 1971. [Google Scholar]
- Dake, L.P. The Practice of Reservoir Engineering; Shell Internationale Petroleum Maatschappij B.V.: The Hague, The Netherlands, 2001. [Google Scholar]
- Constantinescu, D. On the Bifurcations of a 3D Symmetric Dynamical System. Symmetry 2023, 15, 923. [Google Scholar] [CrossRef]
- Fenichel, N. Geometric Singular Perturbations Theory for Ordinary Differential Equations. J. Differ. Equ. 1979, 31, 53–98. [Google Scholar] [CrossRef]
- Bradley, W.T.; Cook, W.J. Two Proofs of the Existence and Uniqueness of the Partial Fraction Decomposition. Int. Math. Forum 2012, 7, 1517–1535. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shestopalov, Y.; Shakhverdiev, A.; Arefiev, S.V. Bifurcations Associated with Three-Phase Polynomial Dynamical Systems and Complete Description of Symmetry Relations Using Discriminant Criterion. Symmetry 2024, 16, 14. https://doi.org/10.3390/sym16010014
Shestopalov Y, Shakhverdiev A, Arefiev SV. Bifurcations Associated with Three-Phase Polynomial Dynamical Systems and Complete Description of Symmetry Relations Using Discriminant Criterion. Symmetry. 2024; 16(1):14. https://doi.org/10.3390/sym16010014
Chicago/Turabian StyleShestopalov, Yury, Azizaga Shakhverdiev, and Sergey V. Arefiev. 2024. "Bifurcations Associated with Three-Phase Polynomial Dynamical Systems and Complete Description of Symmetry Relations Using Discriminant Criterion" Symmetry 16, no. 1: 14. https://doi.org/10.3390/sym16010014
APA StyleShestopalov, Y., Shakhverdiev, A., & Arefiev, S. V. (2024). Bifurcations Associated with Three-Phase Polynomial Dynamical Systems and Complete Description of Symmetry Relations Using Discriminant Criterion. Symmetry, 16(1), 14. https://doi.org/10.3390/sym16010014








