Some Explicit Properties of Frobenius–Euler–Genocchi Polynomials with Applications in Computer Modeling
Abstract
1. Introduction
2. Preliminaries
3. The Frobenius–Euler–Genocchi Polynomials
4. Some Applications of Frobenius–Euler–Genocchi Polynomials of Order
5. Further Remarks
6. Computational Values and Graphical Representations of Frobenius–Euler–Genocchi Polynomials
7. Computational Values and Graphical Representations of Changhee–Frobenius–Euler–Genocchi Polynomials
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alatawi, M.S.; Khan, W.A. New type of degenerate Changhee-Genocchi polynomials. Axioms 2022, 11, 355. [Google Scholar] [CrossRef]
- Luo, Q.-M. On the Apostol Bernoulli polynomials. Cent. Eur. J. Math. 2004, 2, 509–515. [Google Scholar] [CrossRef]
- Belbachir, H.; Hadj-Brahim, S. Some explicit formulas of Euler-Genocchi polynomials. Integers 2019, 19, 1–14. [Google Scholar]
- Carlitz, L. Eulerian numbers and polynomials. Math. Mag. 1959, 32, 247–260. [Google Scholar] [CrossRef]
- Choi, J.; Kim, D.S.; Kim, T.; Kim, Y.H. A note on some identities of Frobenius-Euler numbers and polynomials. Int. J. Math. Math. Sci. 2012, 2012, 861797. [Google Scholar] [CrossRef]
- Goubi, M. On a generalized family of Euler-Genocchi polynomials. Integers 2021, 21, 1–13. [Google Scholar]
- Iqbal, A.; Khan, W.A.; Nadeem, M. New Type of Degenerate Changhee-Genocchi Polynomials of the Second Kind. In Soft Computing: Theories and Applications; Kumar, R., Verma, A.K., Sharma, T.K., Verma, O.P., Sharma, S., Eds.; Lecture Notes in Networks and Systems; Springer: Berlin/Heidelberg, Germany, 2023; Volume 627. [Google Scholar]
- Iqbal, A.; Khan, W.A. A New Family of Generalized Euler-Genocchi Polynomials Associated with Hermite Polynomials. In Soft Computing: Theories and Applications; Kumar, R., Verma, A.K., Sharma, T.K., Verma, O.P., Sharma, S., Eds.; Lecture Notes in Networks and Systems; Springer: Berlin/Heidelberg, Germany, 2023; Volume 627. [Google Scholar]
- Kim, T.; Kim, D.S.; Kim, H.K. On generalized degenerate Euler-Genocchi polynomials. Appl. Math. Sci. Eng. 2023, 31, 1. [Google Scholar] [CrossRef]
- Nadeem, M.; Khan, W.A. Symmetric Identities For Degenerate q-Poly-Genocchi Numbers And Polynomials. South East Asian J. Math. Math. Sci. 2023, 19, 17–28. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Pinter, A. Remarks on some relationships between the Bernoulli and Euler polynomials. Appl. Math. Lett. 2004, 17, 375–380. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, T.; Seo, J. A note on Changhee polynomials and numbers. Adv. Stud. Theor. Phys. 2013, 7, 993–1003. [Google Scholar] [CrossRef]
- Kwon, H.-I.; Kim, T.; Park, J.W. A note on degenerate Changhee-Genocchi polynomials and numbers. Glob. J. Pure Appl. Math. 2016, 12, 4057–4064. [Google Scholar]
- Kim, B.-M.; Jeong, J.; Rim, S.-H. Some explicit identities on Changhee-Genocchi polynomials and numbers. Adv. Differ. Equ. 2016, 202, 1–12. [Google Scholar] [CrossRef]
- Yasar, B.Y.; Ozarslan, M.A. Frobenius-Euler and Frobenius-Genocchi polynomials and their differential equations. New Trend Math. Sci. 2015, 3, 172–180. [Google Scholar]
- Kim, D.S.; Kim, T. Some new identities of Frobenius-Euler numbers and polynomials. J. Inequal. Appl. 2012, 2012, 307. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, T. Higher-order Frobenius-Euler and poly-Bernoulli mixed-type polynomials. Adv. Differ. Equ. 2013, 2013, 251. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Boutiche, M.A.; Rahmani, M. Some explicit formulas for the Frobenius- Euler polynomials of higher order. Appl. Math. Inf. Sci. 2017, 11, 621–626. [Google Scholar] [CrossRef]
- Kurt, B.; Simsek, Y. On the generalized Apostol-type Frobenius-Euler polynomials. Adv. Differ. Equ. 2013, 2013, 1. [Google Scholar] [CrossRef]
- Cakić, N.P.; Milovanović, G. On generalized Stirling number and polynomials. Math. Balk. 2004, 18, 241–248. [Google Scholar]
- Jamei, M.J.; Milovanović, G.; Dagli, M.C. A generalization of the array type polynomials. Math. Moravica 2022, 26, 37–46. [Google Scholar] [CrossRef]
- Luo, Q.M.; Srivastava, H.M. Some generalization of the Apostol-Genocchi polynomials and Stirling numbers of the second kind. Appl. Math. Comput. 2011, 217, 5702–5728. [Google Scholar] [CrossRef]
- Belbachir, H.; Hadj-Brahim, S.; Rachidi, M. On another approach for a family of Appell polynomials. Filomat 2018, 12, 4155–4164. [Google Scholar] [CrossRef]
- Borisov, B.S. The p-binomial transform Cauchy numbers and figurate numbers. Proc. Jangjeon Math. Soc. 2016, 19, 631–644. [Google Scholar]
- Wang, Y.; Dagli, M.C.; Liu, X.-M.; Qi, F. Explicit, determinantal, and recurrent formulas of generalized Eulerian polynomials. Axioms 2021, 10, 37. [Google Scholar] [CrossRef]
- Qi, F.; Guo, B.-N. Explicit formulas for special values of the Bell polynomials of the second kind and for the Euler numbers and polynomials. Mediterr. J. Math. 2017, 14, 140. [Google Scholar] [CrossRef]
- Kızılateş, C. Explicit, determinantal, recursive formulas, and generating functions of generalized Humbert-Hermite polynomials via generalized Fibonacci Polynomials. Math. Methods Appl Sci. 2023, 46, 9205–9216. [Google Scholar] [CrossRef]
- Dagli, M.C. Explicit, determinantal, recursive formulas and relations of the Peters polynomials and numbers. Math. Methods Appl. Sci. 2022, 45, 2582–2591. [Google Scholar] [CrossRef]
- Qi, F.; Dağlı, M.C.; Lim, D. Several explicit formulas for (degenerate) Narumi and Cauchy polynomials and numbers. Open Math. 2021, 19, 833–849. [Google Scholar] [CrossRef]
- Qi, F.; Zheng, M.-M. Explicit expressions for a family of the Bell polynomials and applications. Appl. Math. Comput. 2015, 258, 597–607. [Google Scholar] [CrossRef]
- Qi, F.; Niu, D.-W.; Lim, D.; Yao, Y.-H. Special values of the Bell polynomials of the second kind for some sequences and functions. J. Math. Anal. Appl. 2020, 491, 124382. [Google Scholar] [CrossRef]
- Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1974. [Google Scholar]
- Bourbaki, N. Functions of a Real Variable, Elementary Theory, Translated from the 1976 French original by Philip Spain. In Elements of Mathematics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]


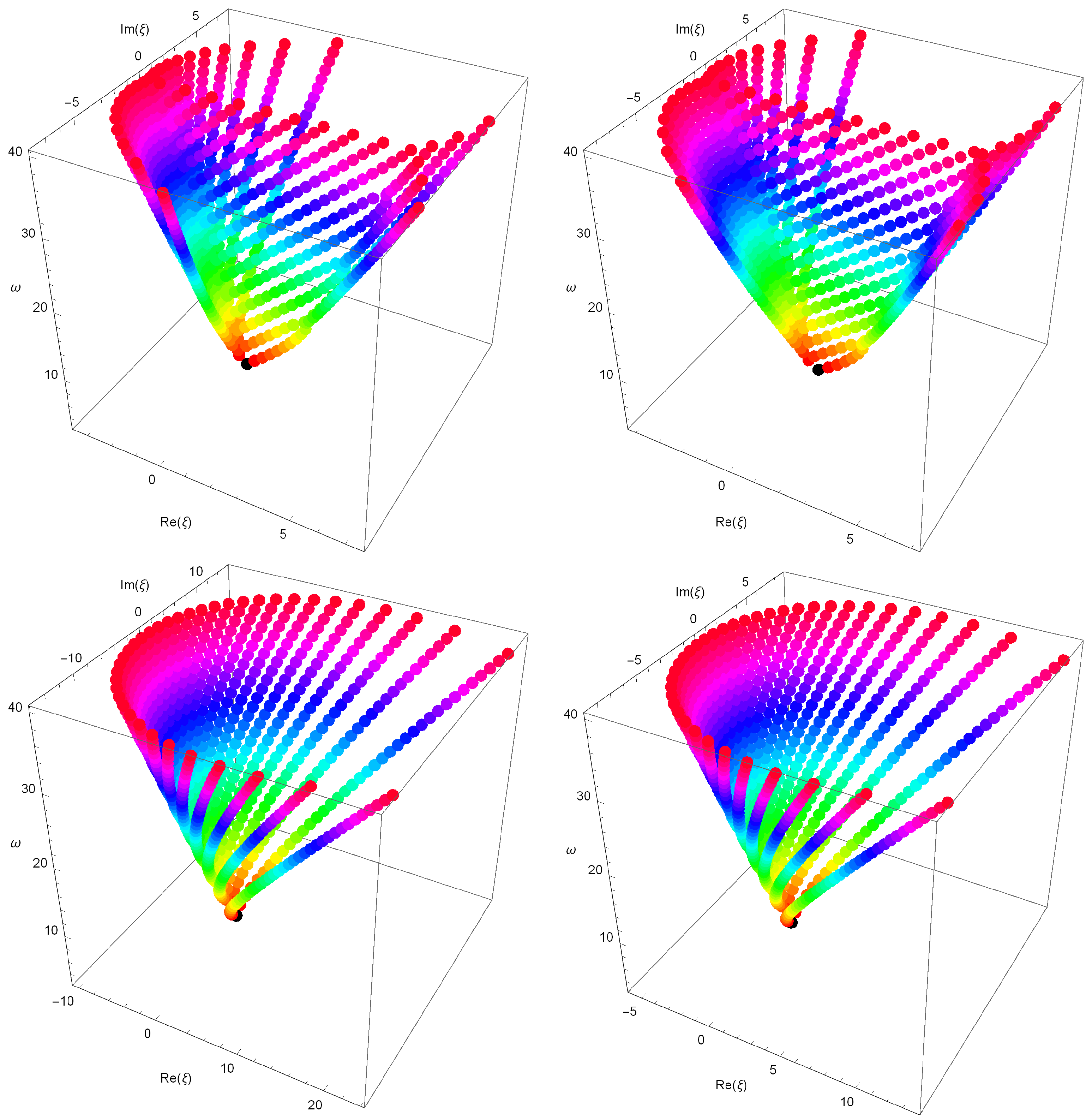

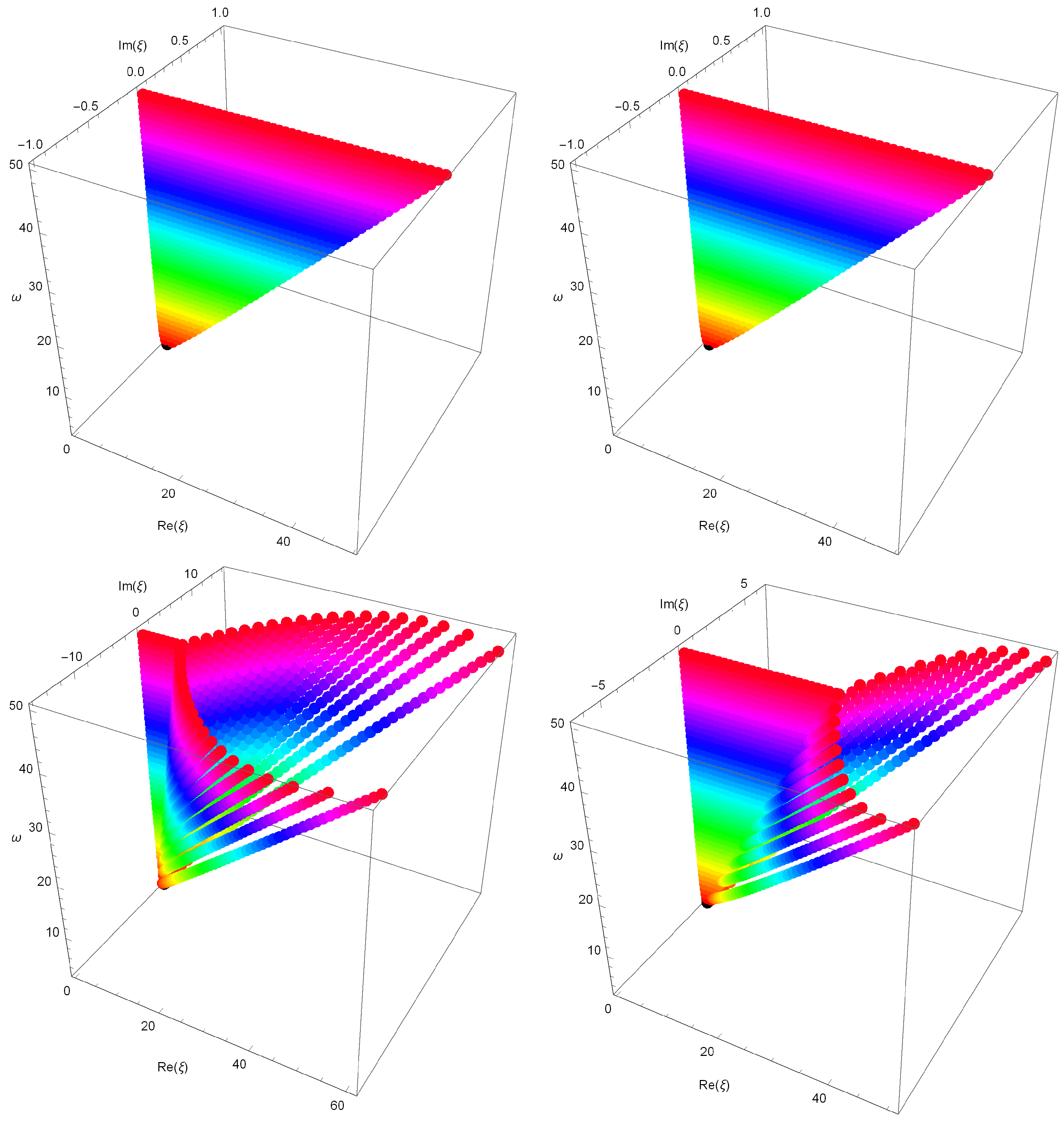
| Degree | |
|---|---|
| 3 | −0.50000 |
| 4 | −0.50000 − 0.86603i, −0.50000 + 0.86603 i |
| 5 | −1.0800, −0.2100 − 1.5819 i, −0.2100 + 1.5819 i |
| 6 | −1.1991 − 0.7701 i, −1.1991 + 0.7701 i, |
| 0.1991 − 2.2101 i, 0.1991 + 2.2101 i | |
| 7 | −1.6268, −1.1130 − 1.4787i, −1.1130 + 1.4787 i, |
| 0.6764 − 2.7763 i, 0.6764 + 2.7763 i | |
| 8 | −1.7906 − 0.7321 i, −1.7906 + 0.7321i, −0.9087 − 2.1385 i, |
| −0.9087 + 2.1385 i, 1.1993 − 3.2957i, 1.1993 + 3.2957 i | |
| 9 | −2.1611, −1.7990 − 1.4290 i, −1.7990 + 1.4290 i, −0.6261 − 2.7580 i, |
| −0.6261 + 2.7580 i, 1.7556 − 3.7778 i, 1.7556 + 3.7778 i | |
| 10 | −2.3477 − 0.7111 i, −2.3477 + 0.7111 i, −1.7030 − 2.0940 i, |
| −1.7030 + 2.0940 i, −0.2870 − 3.3436 i, −0.2870 + 3.3436 i, | |
| 2.3378 − 4.2296 i, 2.3378 + 4.2296 i | |
| 11 | −2.6889, −2.4100 − 1.3992 i, −2.4100 + 1.3992 i, |
| −1.5315 − 2.7306 i, −1.5315 + 2.7306 i, 0.0952 − 3.8998 i, | |
| 0.0952 + 3.8998 i, 2.9407 − 4.6560 i, 2.9407 + 4.6560 i |
| Degree | |
|---|---|
| 3 | 1.2500 |
| 4 | 0.97272, 2.5273 |
| 5 | 0.83715, 2.2045, 3.7084 |
| 6 | 0.75834, 2.0289, 3.3703, 4.8425 |
| 7 | 0.70700, 1.9225, 3.1696, 4.5035, 5.9475 |
| 8 | 0.67047, 1.8533, 3.0413, 4.2873, 5.6152, 7.0324 |
| 9 | 0.64266, 1.8052, 2.9562, 4.1418, 5.3905, 6.7113, 8.1024 |
| 10 | 0.62043, 1.7695, 2.8973, 4.0421, 5.2320, 6.4829, 7.7949, 9.1609 |
| 11 | 0.60202, 1.7414, 2.8545, 3.9723, 5.1194, 6.3151, 7.5666, 8.8684, 10.210 |
| 12 | 0.58638, 1.7185, 2.8218, 3.9220, 5.0389, 6.1915, 7.3925, 8.6427, 9.9333, 11.252 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alam, N.; Khan, W.A.; Kızılateş, C.; Obeidat, S.; Ryoo, C.S.; Diab, N.S. Some Explicit Properties of Frobenius–Euler–Genocchi Polynomials with Applications in Computer Modeling. Symmetry 2023, 15, 1358. https://doi.org/10.3390/sym15071358
Alam N, Khan WA, Kızılateş C, Obeidat S, Ryoo CS, Diab NS. Some Explicit Properties of Frobenius–Euler–Genocchi Polynomials with Applications in Computer Modeling. Symmetry. 2023; 15(7):1358. https://doi.org/10.3390/sym15071358
Chicago/Turabian StyleAlam, Noor, Waseem Ahmad Khan, Can Kızılateş, Sofian Obeidat, Cheon Seoung Ryoo, and Nabawia Shaban Diab. 2023. "Some Explicit Properties of Frobenius–Euler–Genocchi Polynomials with Applications in Computer Modeling" Symmetry 15, no. 7: 1358. https://doi.org/10.3390/sym15071358
APA StyleAlam, N., Khan, W. A., Kızılateş, C., Obeidat, S., Ryoo, C. S., & Diab, N. S. (2023). Some Explicit Properties of Frobenius–Euler–Genocchi Polynomials with Applications in Computer Modeling. Symmetry, 15(7), 1358. https://doi.org/10.3390/sym15071358







