Approximate Optimal Tracking Control for Partially Unknown Nonlinear Systems via an Adaptive Fixed-Time Observer
Abstract
1. Introduction
- An adaptive fixed-time disturbance observer (AFXDO) is put forward for the estimation of lumped disturbance;
- The controller is set up based on the proposed AFXDO;
- The weight law with a variable learning rate is proposed to avoid the oscillation phenomenon during the learning process.
2. Related Works
3. Problem Formulation and Preliminaries
3.1. Problem Formulation
- (1)
- The disturbance estimation error can converge to zero in a fixed time.
- (2)
- The trajectory tracking error and weight estimation error are uniformly ultimately bounded (UUB).
- (3)
- The designed control input approximates the optimal control policy.
3.2. Related Lemmas
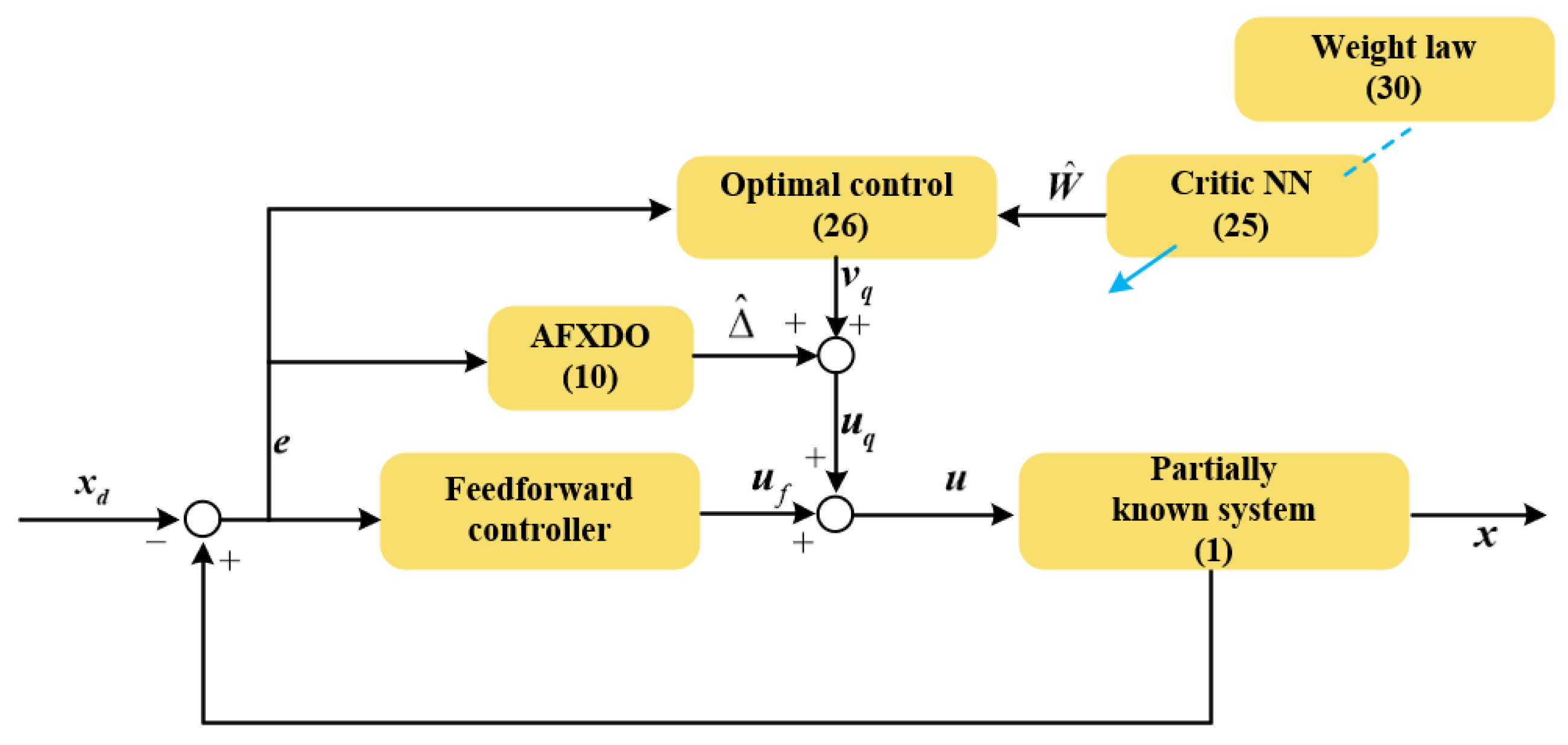
4. Main Results
4.1. Adaptive Fixed-Time Disturbance Observer
4.2. Optimal Tracking Control Scheme
4.3. Stability Analysis
- (1)
- The tracking error and weight error are UUB.
- (2)
- The approximate actual control input approximates the optimal control policy.
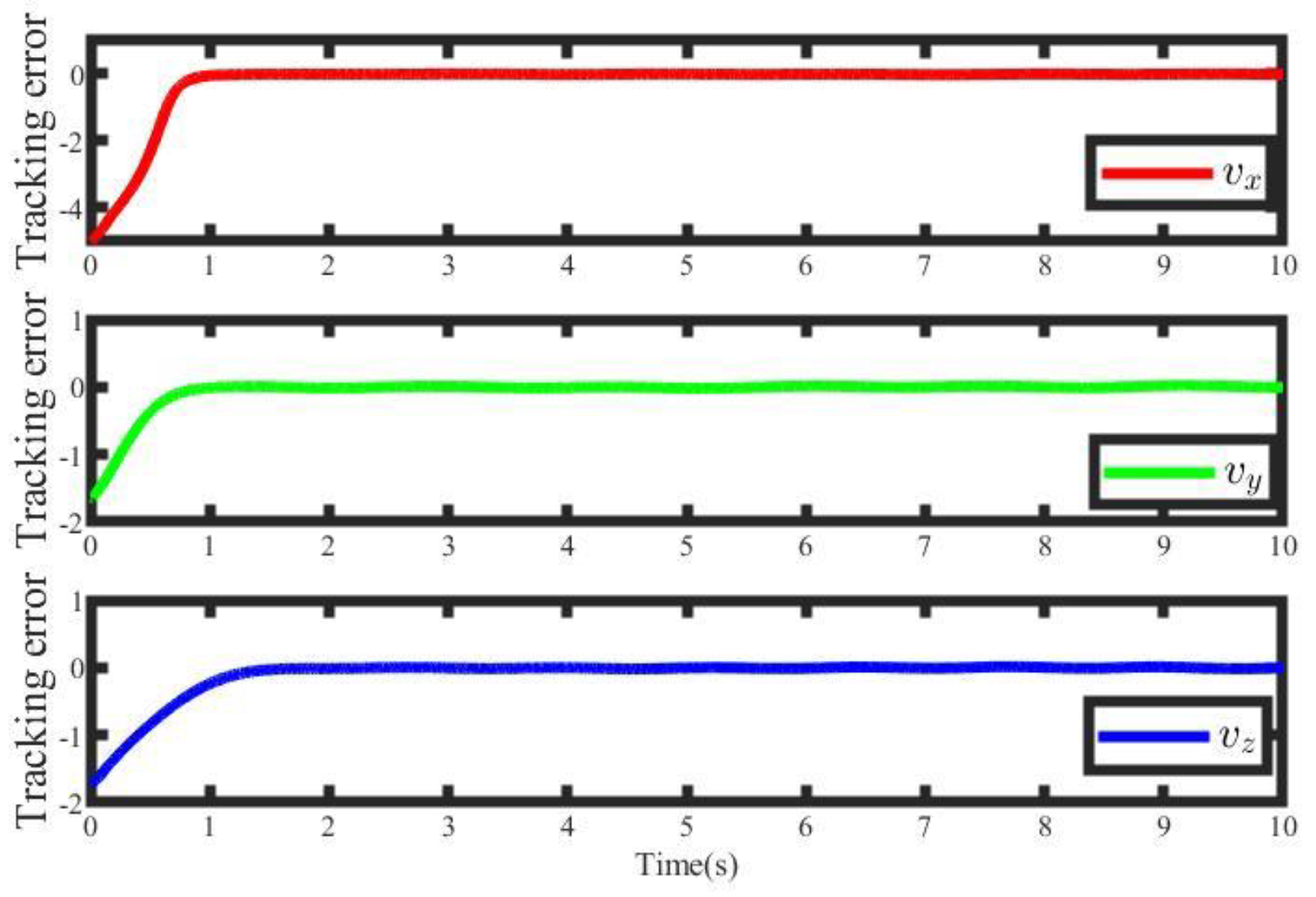
5. Simulation and Discussion
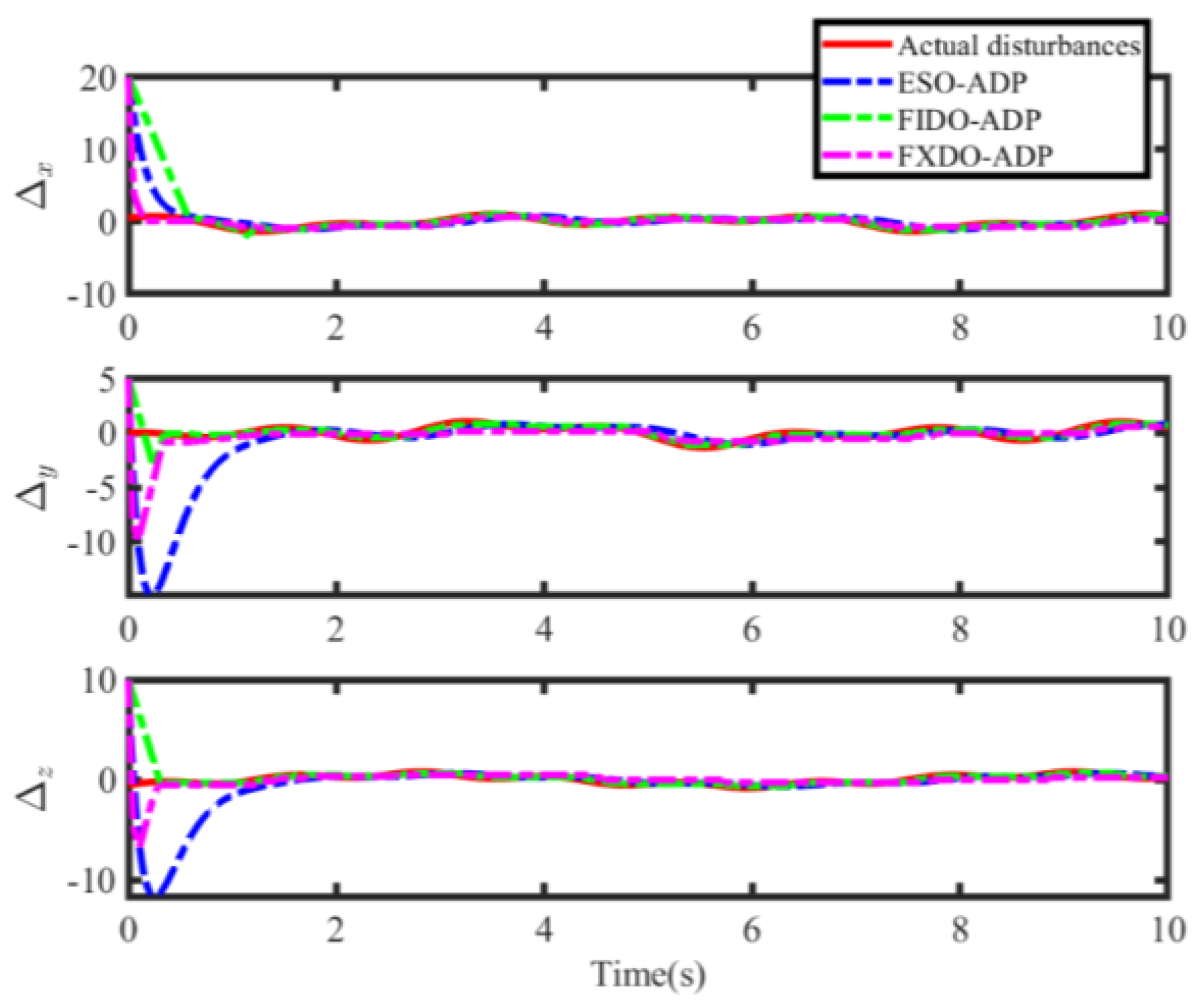
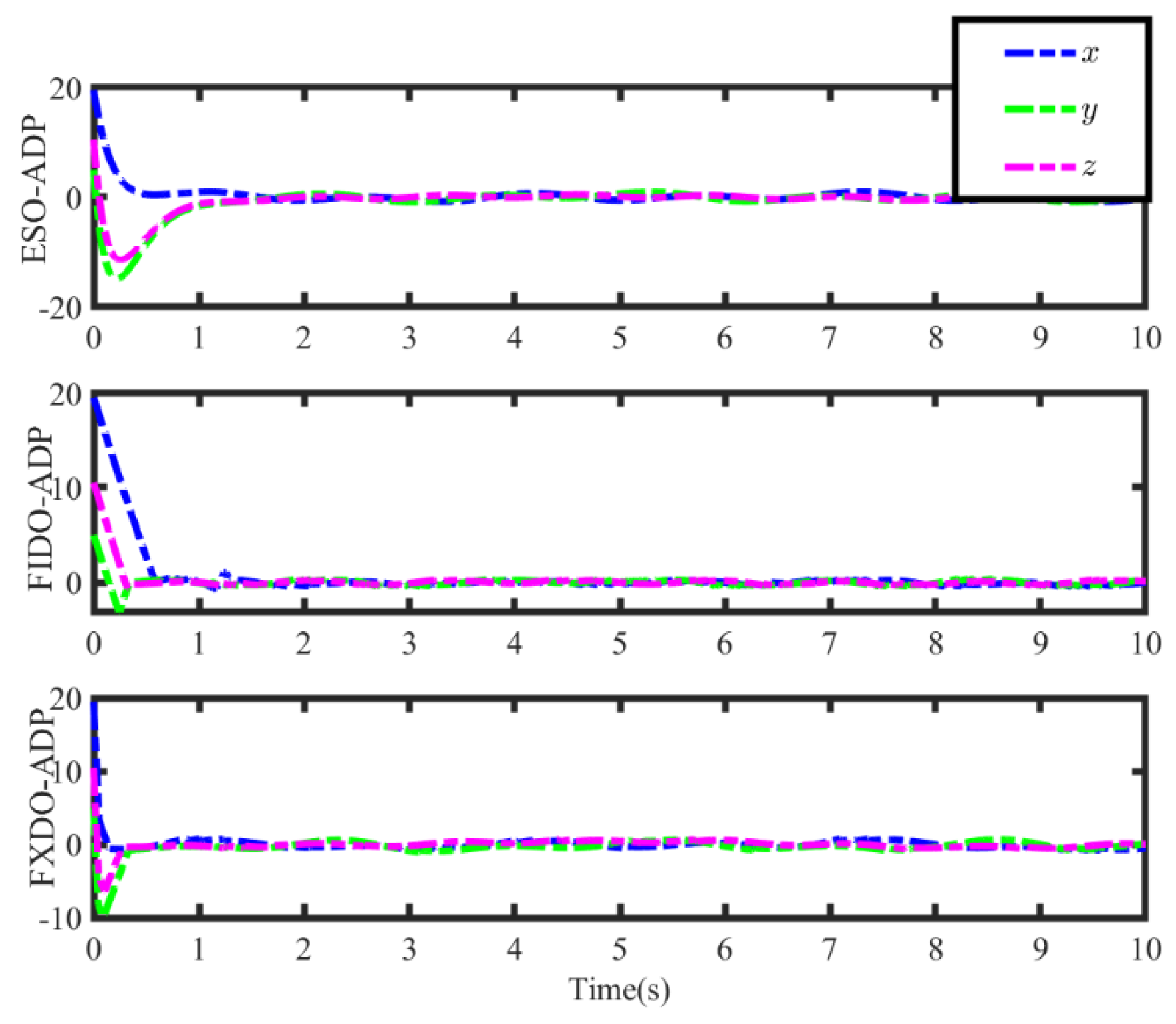
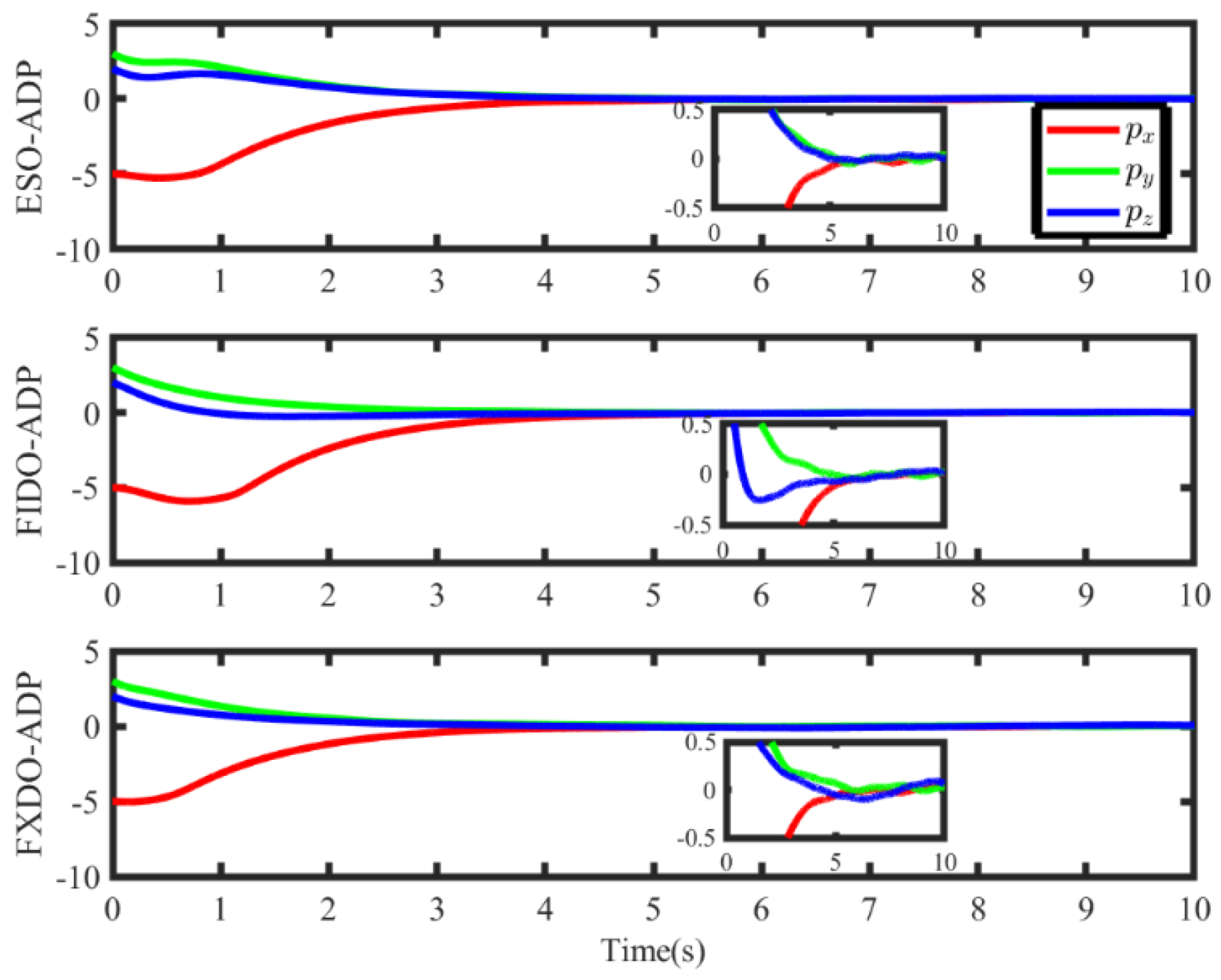
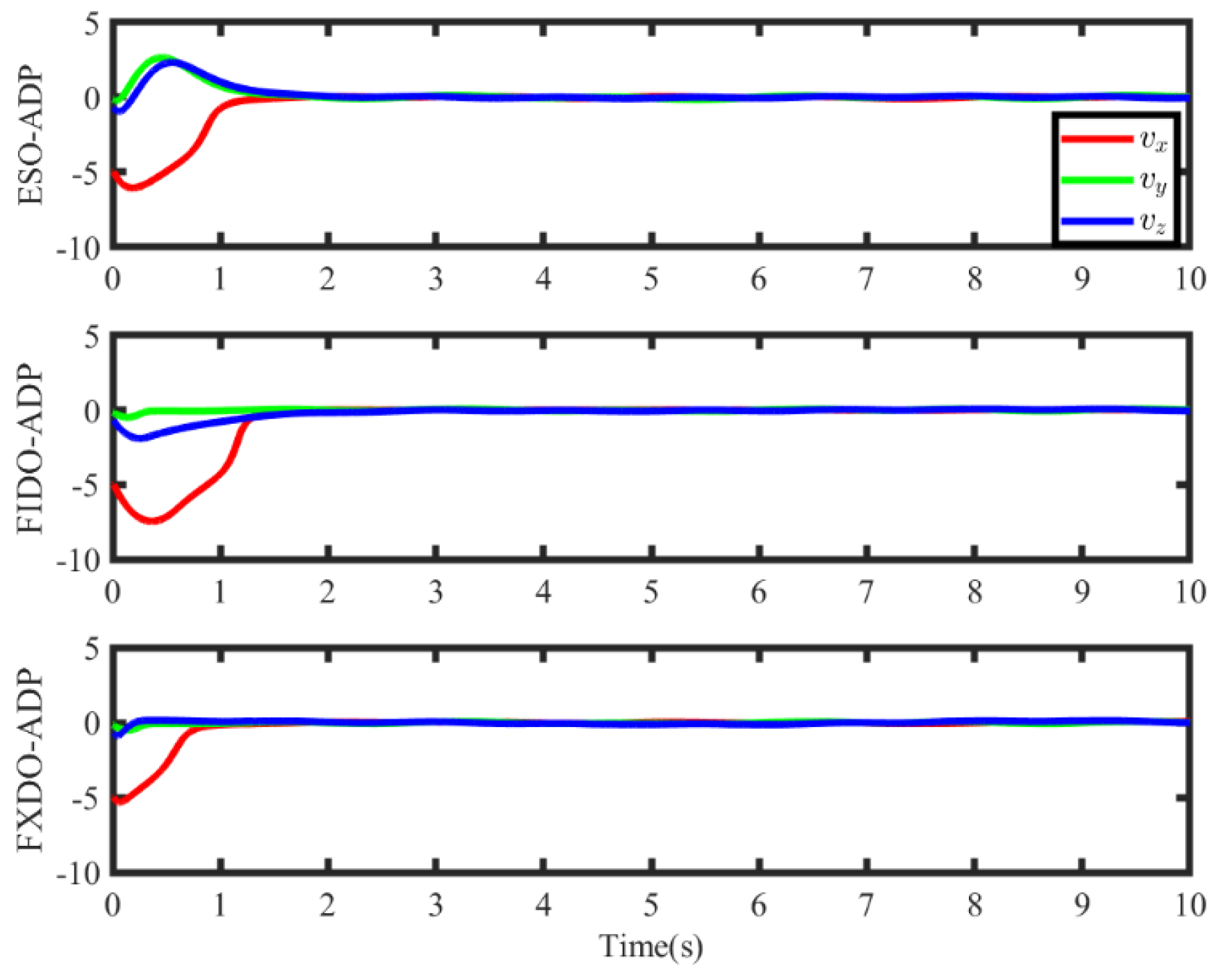
- Case 1: Effect of disturbance observer on closed-loop performance
- (1)
- The designed ADP controller with extended state observer (ESO-ADP): The observer in [4] is utilized for the estimation of disturbance with the ADP framework. The bandwidth is set as .
- (2)
- The designed ADP controller with finite-time DO (FIDO-ADP): The finite-time DO in [28] is employed with adaptive gains to eliminate the effect of disturbance in a finite time. The parameters of the observer are set as , , , and .
- (3)
- The designed ADP controller with the proposed fixed-time DO (FXDO-ADP): During the operation of this method, we select the following parameters in AFXDO to ensure stability: , , , , and .
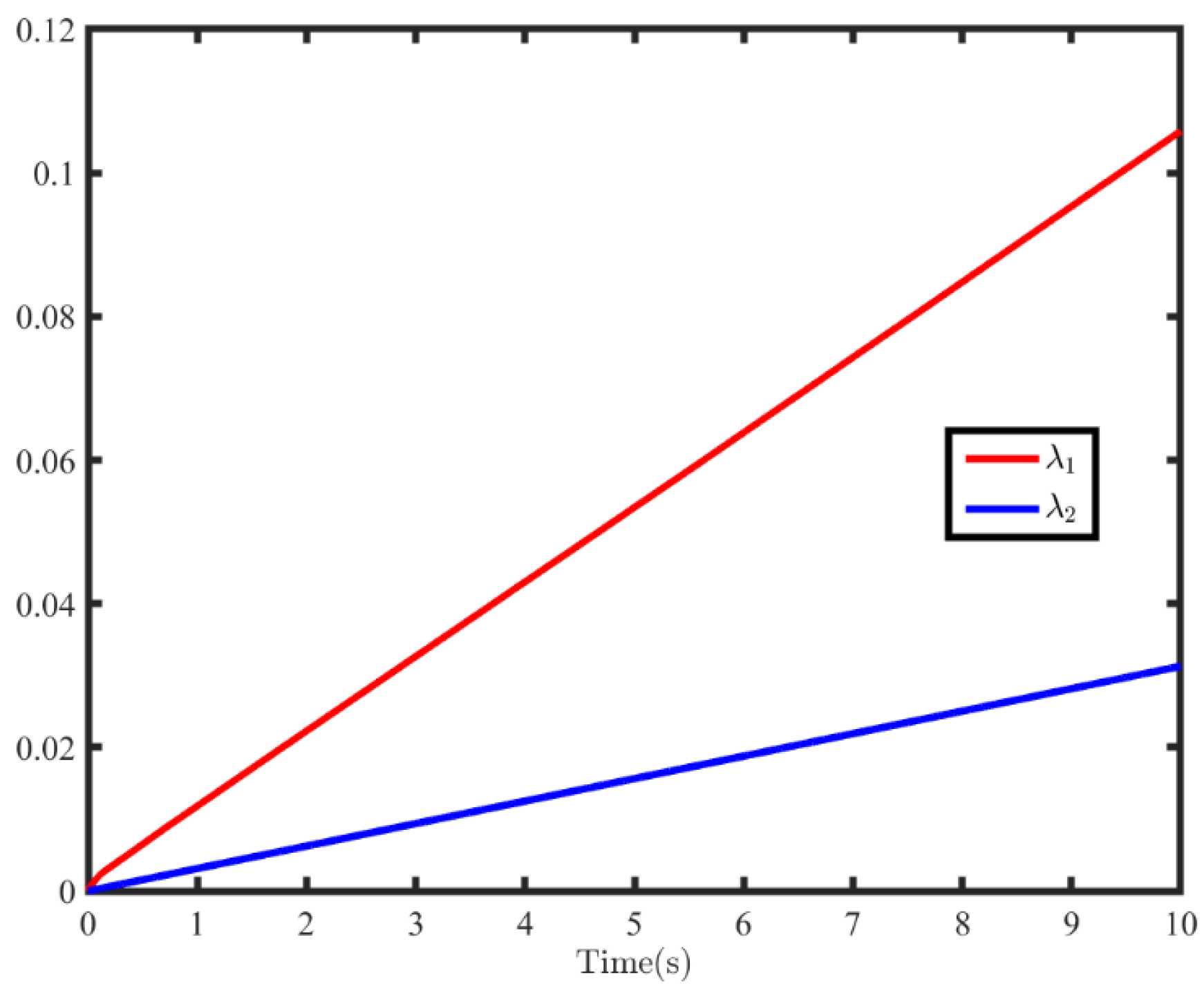
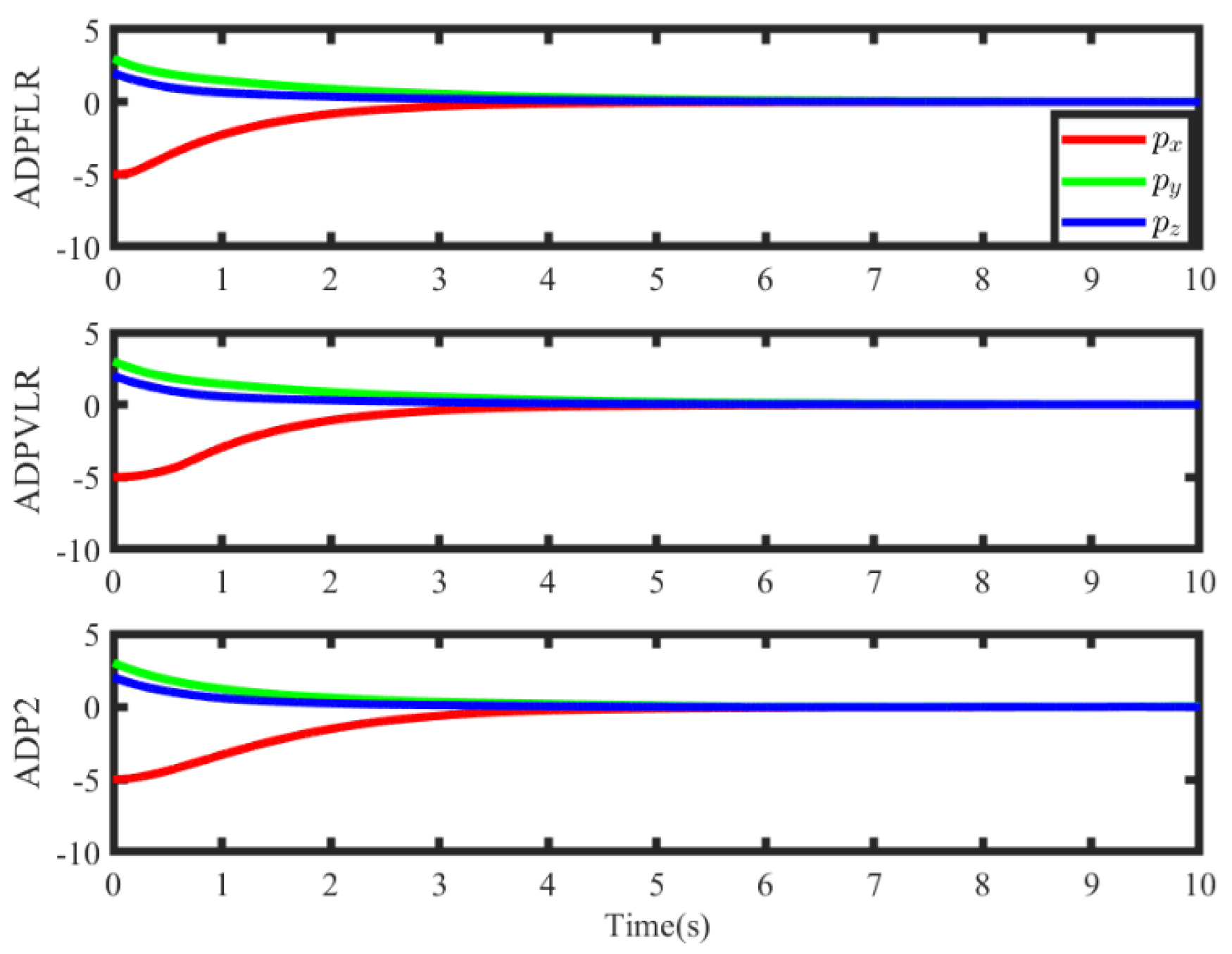
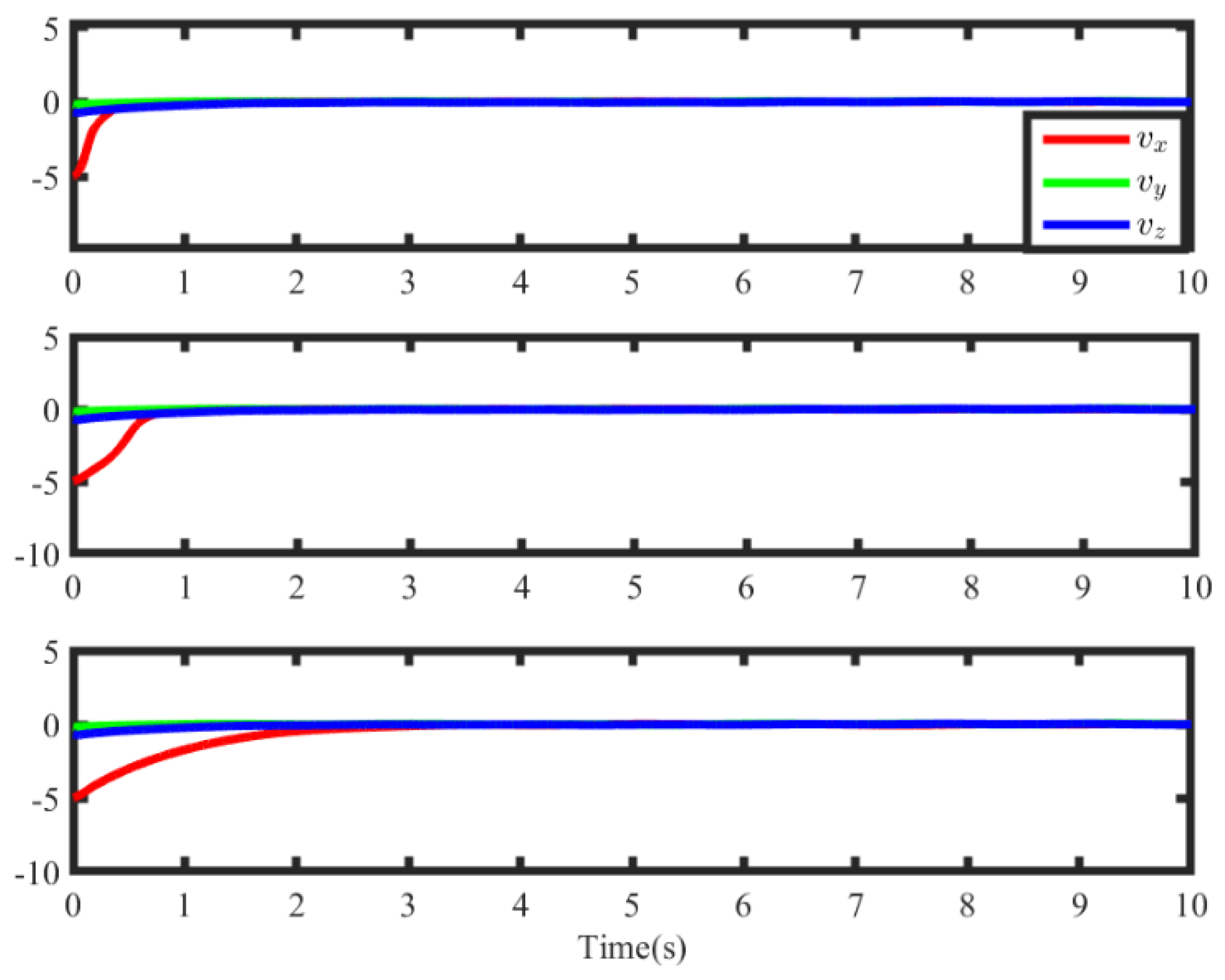
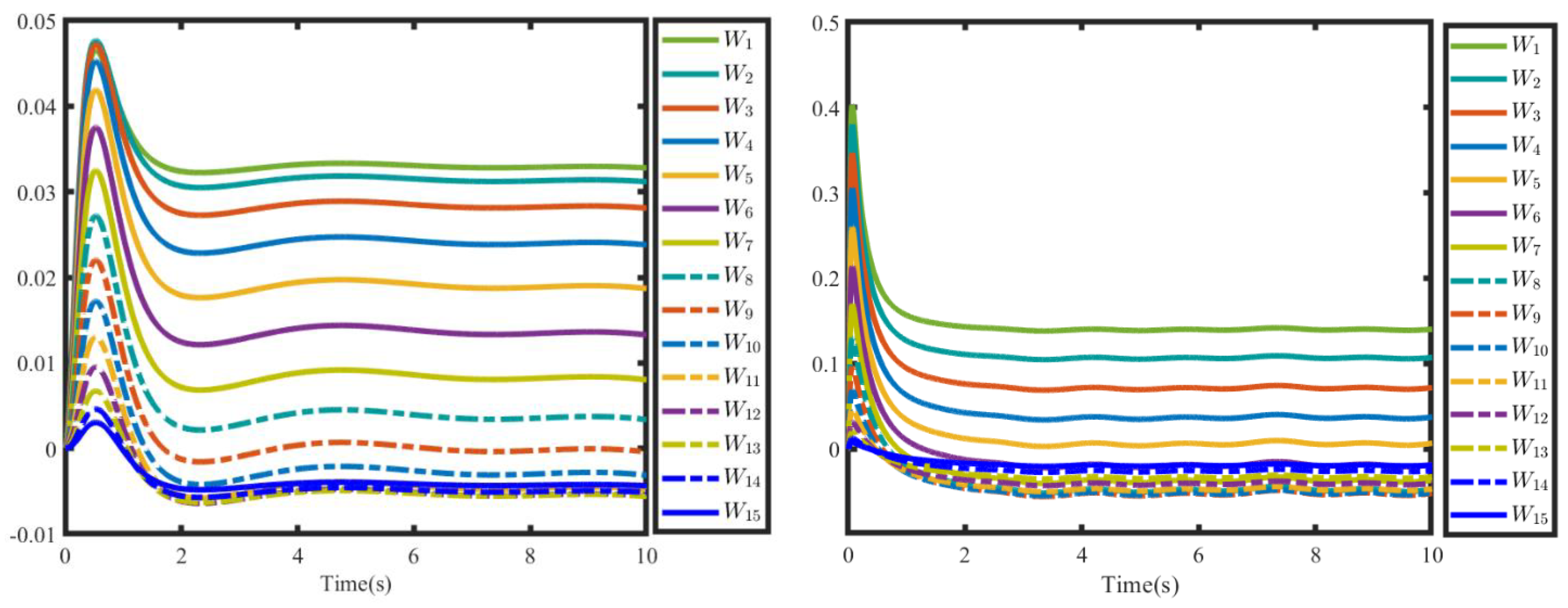
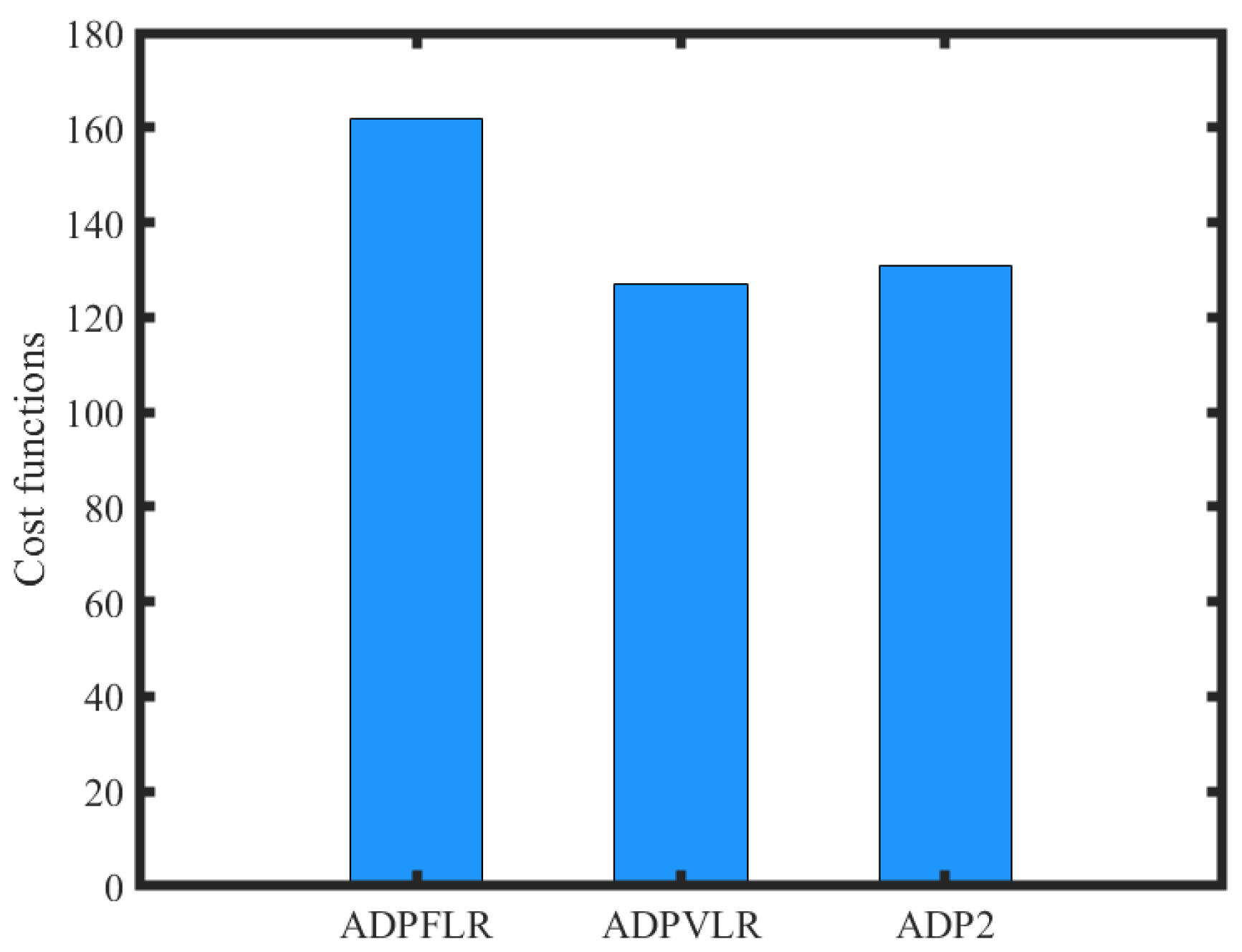
- Case 2: Effect of weight update on learning performance
- (1)
- The designed ADP controller with a fixed learning rate (ADPFLR) [50]: The fixed learning rate is set as to accelerate the convergence of tracking errors.
- (2)
- The designed ADP controller with a proposed variable learning rate (ADPVLR): The weight is updated by (30) rather than the fixed learning rate in ADPFLR. The parameters are selected as , .
- (3)
- The actor–critic ADP controller with the gradient method [35] (ADP2): The learning rate in actor and critic NNs are set as , .
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Castellini, A.; Marchesini, E.; Farinelli, A. Partially observable monte carlo planning with state variable constraints for mobile robot navigation. Eng. Appl. Artif. Intell. 2021, 104, 104382. [Google Scholar] [CrossRef]
- Dai, Y.; Ni, S.; Xu, D.; Zhang, L.; Yan, X.G. Disturbance-observer based prescribed-performance fuzzy sliding mode control for PMSM in electric vehicles. Eng. Appl. Artif. Intell. 2021, 104, 104361. [Google Scholar] [CrossRef]
- Park, B.S.; Yoo, S.J. Quantized-communication-based neural network control for formation tracking of networked multiple unmanned surface vehicles without velocity information. Eng. Appl. Artif. Intell. 2022, 114, 105160. [Google Scholar] [CrossRef]
- Shao, X.; Yue, X.; Li, J. Event-triggered robust control for quadrotors with preassigned time performance constraints. Appl. Math. Comput. 2021, 392, 125667. [Google Scholar] [CrossRef]
- Wang, Y.; Luo, G.; Wang, D. Observer-based fixed-time adaptive fuzzy control for SbW systems with prescribed performance. Eng. Appl. Artif. Intell. 2022, 114, 105026. [Google Scholar] [CrossRef]
- Wu, L.; Li, Z.; Liu, S.; Li, Z.; Sun, D. A novel multi-agent model-free adaptive control algorithm for a class of multivehicle systems with constraints. Symmetry 2023, 15, 168. [Google Scholar] [CrossRef]
- Wu, J.; Sun, W.; Su, S.F.; Wu, Y. Adaptive asymptotic tracking control for input-quantized nonlinear systems with multiple unknown control directions. IEEE Trans. Cybern. 2022, in press. [Google Scholar] [CrossRef]
- Qiyas, M.; Abdullah, S.; Khan, F.; Naeem, M. Banzhaf-Choquet-Copula-based aggregation operators for managing fractional orthotriple fuzzy information. Alex. Eng. J. 2022, 61, 4659–4677. [Google Scholar] [CrossRef]
- Khan, A.; Ashraf, S.; Abdullah, S.; Muhammad, A.; Thongchai, B. A novel decision aid approach based on spherical hesitant fuzzy Aczel-Alsina geometric aggregation information. AIMS Math. 2023, 8, 5148–5174. [Google Scholar] [CrossRef]
- Qiyas, M.; Naeem, M.; Abdullah, S.; Khan, F.; Khan, N.; Garg, H. Fractional orthotriple fuzzy rough Hamacher aggregation operators and-their application on service quality of wireless network selection. Alex. Eng. J. 2022, 61, 10433–10452. [Google Scholar] [CrossRef]
- Yahya, M.; Abdullah, S.; Almagrabi, A.O.; Botmart, T. Analysis of S-box based on image encryption application using complex fuzzy credibility Frank aggregation operators. IEEE Access 2022, 10, 88858–88871. [Google Scholar] [CrossRef]
- Mohammad, M.M.S.; Abdullah, S.; Al-Shomrani, M.M. Some linear Diophantine fuzzy similarity measures and their application in decision making problem. IEEE Access 2022, 10, 29859–29877. [Google Scholar] [CrossRef]
- Qiyas, M.; Madrar, T.; Khan, S.; Abdullah, S.; Botmart, T.; Jirawattanapaint, A. Decision support system based on fuzzy credibility Dombi aggregation operators and modified TOPSIS method. AIMS Math. 2022, 7, 19057–19082. [Google Scholar] [CrossRef]
- Ahmad, S.; Basharat, P.; Abdullah, S.; Botmart, T.; Jirawattanapanit, A. MABAC under non-linear diophantine fuzzy numbers: A new approach for emergency decision support systems. AIMS Math. 2022, 7, 17699–17736. [Google Scholar] [CrossRef]
- Midrar, T.; Khan, S.; Abdullah, S.; Botmart, T. Entropy based extended TOPOSIS method for MCDM problem with fuzzy credibility numbers. AIMS Math. 2022, 7, 17286–17312. [Google Scholar] [CrossRef]
- Ashraf, S.; Rehman, N.; Abdullah, S.; Batool, B.; Lin, M.; Aslam, M. Decision support model for the patient admission scheduling problem based on picture fuzzy aggregation information and TOPSIS methodology. Math. Biosci. Eng. 2022, 19, 3147–3176. [Google Scholar] [CrossRef]
- Batool, B.; Abdullah, S.; Ashraf, S.; Ahmad, M. Pythagorean probabilistic hesitant fuzzy aggregation operators and their application in decision-making. Kybernetes 2022, 51, 1626–1652. [Google Scholar] [CrossRef]
- Abdullah, S.; Al-Shomrani, M.M.; Liu, P.; Ahmad, S. A new approach to three-way decisions making based on fractional fuzzy decision-theoretical rough set. Int. J. Intell. Syst. 2022, 37, 2428–2457. [Google Scholar] [CrossRef]
- Ashraf, S.; Abdullah, S.; Chinram, R. Emergency decision support modeling under generalized spherical fuzzy Einstein aggregation information. J. Ambient Intell. Humaniz. Comput. 2022, 13, 2091–2117. [Google Scholar] [CrossRef]
- Shao, S.; Chen, M.; Zheng, S.; Lu, S.; Zhao, Q. Event-triggered fractional-order tracking control for an uncertain nonlinear system with output saturation and disturbances. IEEE Trans. Neural Netw. Learn. Syst. 2022, in press. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Y.; Tie, M. Hybrid adaptive learning neural network control for steer-by-wire systems via sigmoid tracking differentiator and disturbance observer. Eng. Appl. Artif. Intell. 2021, 104, 104393. [Google Scholar] [CrossRef]
- Zhang, J.; Zhao, W.; Shen, G.; Xia, Y. Disturbance observer-based adaptive finite-time attitude tracking control for rigid spacecraft. IEEE Trans. Syst. Man Cybern. Syst. 2020, 51, 6606–6613. [Google Scholar] [CrossRef]
- Nguyen, T.H.; Nguyen, T.T.; Nguyen, V.Q.; Le, K.M.; Tran, H.N.; Jeon, J.W. An adaptive sliding-mode controller with a modified reduced-order proportional integral observer for speed regulation of a permanent magnet synchronous motor. IEEE Trans. Ind. Electron. 2021, 69, 7181–7191. [Google Scholar] [CrossRef]
- Nguyen, N.P.; Oh, H.; Kim, Y.; Moon, J.; Yang, J.; Chen, W.H. Finite-time disturbance observer-based modified super-twisting algorithm for systems with mismatched disturbances: Application to fixed-wing UAVs under wind disturbances. Int. J. Robust Nonlin. Control 2021, 31, 7317–7343. [Google Scholar] [CrossRef]
- Mirzaei, M.J.; Mirzaei, M.; Aslmostafa, E.; Asadollahi, M. Robust observer-based stabilizer for perturbed nonlinear complex financial systems with market confidence and ethics risks by finite-time integral sliding mode control. Nonlinear Dyn. 2021, 105, 2283–2297. [Google Scholar] [CrossRef]
- Wang, X.; Zheng, W.X.; Wang, G. Distributed finite-time optimization of second-order multiagent systems with unknown velocities and disturbances. IEEE Trans. Neural Netw. Learn. Syst. 2022, in press. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, Y.; Zhao, Z.; Tang, X.; Yang, J.; Chen, I. Finite-time disturbance observer-based trajectory tracking control for flexible-joint robots. Nonlinear Dyn. 2021, 106, 459–471. [Google Scholar] [CrossRef]
- Huang, D.; Huang, T.; Qin, N.; Li, Y.; Yang, Y. Finite-time control for a UAV system based on finite-time disturbance observer. Aerosp. Sci. Technol. 2022, 129, 107825. [Google Scholar] [CrossRef]
- Polyakov, A. Nonlinear feedback design for fixed-time stabilization of linear control systems. IEEE Trans. Autom. Control. 2011, 57, 2106–2110. [Google Scholar] [CrossRef]
- Sun, L.; Sun, G.; Jiang, J. Disturbance observer-based saturated fixed-time pose tracking for feature points of two rigid bodies. Automatica 2022, 144, 110475. [Google Scholar] [CrossRef]
- Sun, J.; Yi, J.; Pu, Z.; Tan, X. Fixed-time sliding mode disturbance observer-based nonsmooth backstepping control for hypersonic vehicles. IEEE Trans. Syst. Man, Cybern. Syst. 2018, 50, 4377–4386. [Google Scholar] [CrossRef]
- Gao, J.; Fu, Z.; Zhang, S. Adaptive fixed-time attitude tracking control for rigid spacecraft with actuator faults. IEEE Trans. Ind. Electron. 2018, 66, 7141–7149. [Google Scholar] [CrossRef]
- Hu, G.; Guo, J.; Guo, Z.; Cieslak, J.; Henry, D. ADP-based intelligent tracking algorithm for reentry vehicles subjected to model and state uncertainties. IEEE Trans. Ind. Informat. 2022, in press. [Google Scholar] [CrossRef]
- Yang, Y.; Gao, W.; Modares, H.; Xu, C.Z. Robust actor-critic learning for continuous-time nonlinear systems with unmodeled dynamics. IEEE Trans. Fuzzy Syst. 2021, 30, 2101–2112. [Google Scholar] [CrossRef]
- El-Sousy, F.F.; Amin, M.M.; Al-Durra, A. Adaptive optimal tracking control via actor-critic-identifier based adaptive dynamic programming for permanent-magnet synchronous motor drive system. IEEE Trans. Ind. Appl. 2021, 57, 6577–6591. [Google Scholar] [CrossRef]
- Dierks, T.; Jagannathan, S. Optimal Control of Affine Nonlinear Continuous-Time Systems. In Proceedings of the 2010 American Control Conference, Baltimore, MD, USA, 30 June–2 July 2010; pp. 1568–1573. [Google Scholar]
- Xue, S.; Luo, B.; Liu, D. Event-triggered adaptive dynamic programming for zero-sum game of partially unknown continuous-time nonlinear systems. IEEE Trans. Syst. Man Cybern. Syst. 2018, 50, 3189–3199. [Google Scholar] [CrossRef]
- Wang, D.; Mu, C.; Liu, D.; Ma, H. On mixed data and event driven design for adaptive-critic-based nonlinear H∞ control. IEEE Trans. Neural Netw. Learn. Syst. 2017, 29, 993–1005. [Google Scholar] [CrossRef]
- Yang, H.; Hu, Q.; Dong, H.; Zhao, X. ADP-based spacecraft attitude control under actuator misalignment and pointing constraints. IEEE Trans. Ind. Electron. 2017, 69, 9342–9352. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, Z.T.; Tian, B.L.; Zong, Q. Event-based robust optimal consensus control for nonlinear multiagent system with local adaptive dynamic programming. IEEE Trans. Neural Netw. Learn. Syst. 2022, in press. [Google Scholar] [CrossRef]
- Song, R.; Lewis, F.L. Robust optimal control for a class of nonlinear systems with unknown disturbances based on disturbance observer and policy iteration. Neurocomputing 2020, 390, 185–195. [Google Scholar] [CrossRef]
- Pham, T.L.; Dao, P.N. Disturbance observer-based adaptive reinforcement learning for perturbed uncertain surface vessels. ISA Trans. 2022, 130, 277–292. [Google Scholar]
- Dong, H.; Yang, X. Learning-based online optimal sliding-mode control for space circumnavigation missions with input constraints and mismatched uncertainties. Neurocomputing 2022, 484, 13–25. [Google Scholar] [CrossRef]
- Zhang, H.; Park, J.H.; Yue, D.; Zhao, W. Nearly optimal integral sliding-mode consensus control for multiagent systems with disturbances. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 4741–4750. [Google Scholar] [CrossRef]
- Xia, R.; Wu, Q.; Shao, S. Disturbance observer-based optimal flight control of near space vehicle with external disturbance. Trans. Inst. Meas. Control. 2020, 42, 272–284. [Google Scholar] [CrossRef]
- Vamvoudakis, K.G.; Lewis, F.L. Online actor-critic algorithm to solve the continuous-time infinite horizon optimal control problem. Automatica 2010, 46, 878–888. [Google Scholar] [CrossRef]
- Shao, X.; Shi, Y.; Zhang, W. Input-and-measurement event-triggered output-feedback chattering reduction control for MEMS gyroscopes. IEEE Trans. Syst. Man Cybern. Syst. 2021, 52, 5579–5590. [Google Scholar] [CrossRef]
- Zuo, Z. Non-singular fixed-time terminal sliding mode control of non-linear systems. IET Control. Theory Appl. 2015, 9, 545–552. [Google Scholar] [CrossRef]
- Cruz-Zavala, E.; Moreno, J.A.; Fridman, L.M. Uniform robust exact differentiator. IEEE Trans. Autom. Control. 2011, 56, 2727–2733. [Google Scholar] [CrossRef]
- Na, J.; Lv, Y.; Zhang, K.; Zhao, J. Adaptive identifier-critic-based optimal tracking control for nonlinear systems with experimental validation. IEEE Trans. Syst. Man Cybern. Syst. 2020, 52, 459–472. [Google Scholar] [CrossRef]





| Literature | Controller Design | ||||
|---|---|---|---|---|---|
| Anti-Interference Manner | Assumptions | Convergence Results | Optimal Manner | Weight Update | |
| [22] | Integral DO | Known | Asymptotic convergence | Unconsidered | Unconsidered |
| [24,25,26,27,28] | Finite-time DO | Known | Finite-time convergence | Unconsidered | Unconsidered |
| [30,31,32] | Fixed-time DO | Known | Fixed-time convergence | Unconsidered | Unconsidered |
| [39] | Value function | Known | Unconsidered | Critic-only | Gradient Descent |
| [40] | Sliding mode control | Known | Unconsidered | Critic-only | Gradient Descent |
| [42,44] | DO | Known | Asymptotic convergence | Actor–critic | Gradient Descent |
| [43] | Finite-time DO | Known | Finite-time Convergence | Actor–critic | Gradient Descent |
| Our method | Fixed-time DO | Unknown | Fixed-time convergence | Critic-only | Improved gradient |
| Variable | Value |
|---|---|
| Step 1 | Initialize . |
| Step 2 | Compute the tracking errors, feedforward controller and lumped disturbance approximation (10). |
| Step 3 | Compute approximation of cost function (25) with critic NN. |
| Step 4 | Update the auxiliary variables and (29). |
| Step 5 | Update the weight of critic NN (30). |
| Step 6 | Calculate the optimal control (26) with the approximate weight of critic NN. |
| Step 7 | Update the controller according to lumped disturbance approximation and optimal control. |
| Step 8 | If , return to Step 2; else stop. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, Y.; Liu, Z. Approximate Optimal Tracking Control for Partially Unknown Nonlinear Systems via an Adaptive Fixed-Time Observer. Symmetry 2023, 15, 1136. https://doi.org/10.3390/sym15061136
Gao Y, Liu Z. Approximate Optimal Tracking Control for Partially Unknown Nonlinear Systems via an Adaptive Fixed-Time Observer. Symmetry. 2023; 15(6):1136. https://doi.org/10.3390/sym15061136
Chicago/Turabian StyleGao, Yanping, and Zuojun Liu. 2023. "Approximate Optimal Tracking Control for Partially Unknown Nonlinear Systems via an Adaptive Fixed-Time Observer" Symmetry 15, no. 6: 1136. https://doi.org/10.3390/sym15061136
APA StyleGao, Y., & Liu, Z. (2023). Approximate Optimal Tracking Control for Partially Unknown Nonlinear Systems via an Adaptive Fixed-Time Observer. Symmetry, 15(6), 1136. https://doi.org/10.3390/sym15061136





