Numerical Simulation Research Considering the Influence of Bridge Deck Cross Slope on Wind Performance of π-Type Composite Beam Cable-Stayed Bridge with a Symmetric Elevation Arrangement
Abstract
1. Introduction
2. Materials and Methods
2.1. Model Building
2.2. CFD Numerical Simulation
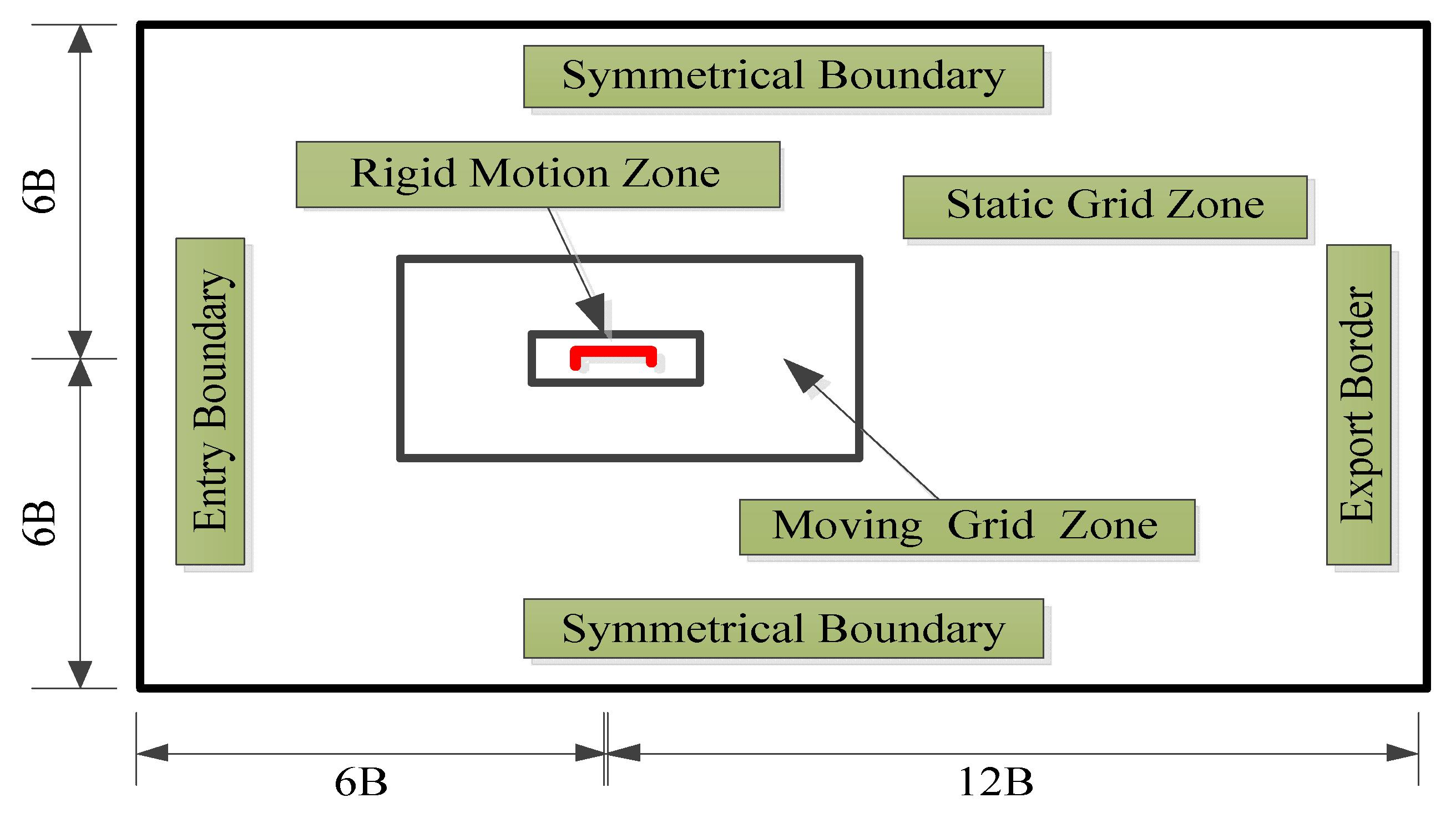
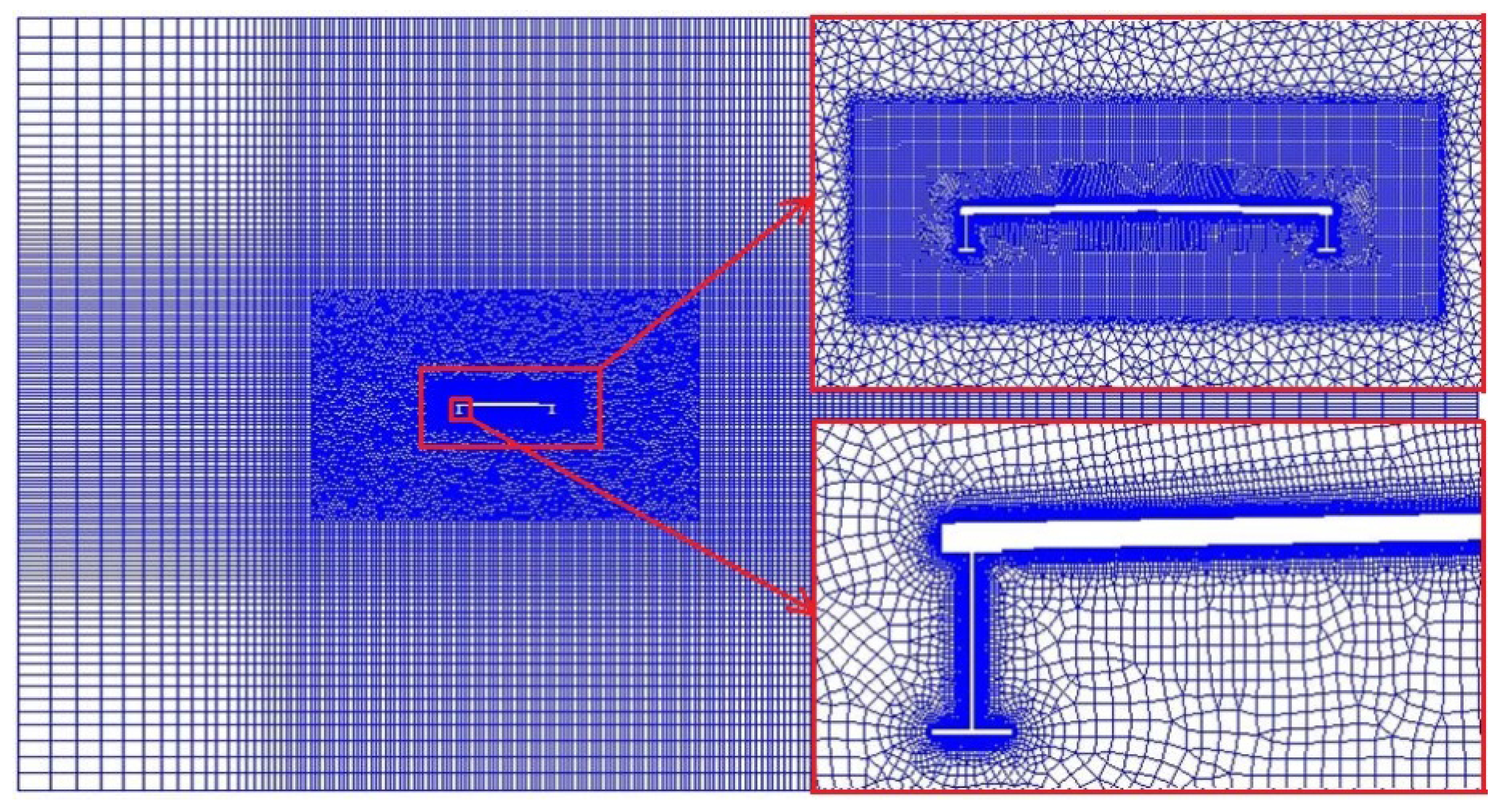
2.2.1. Computational Domain and Meshing
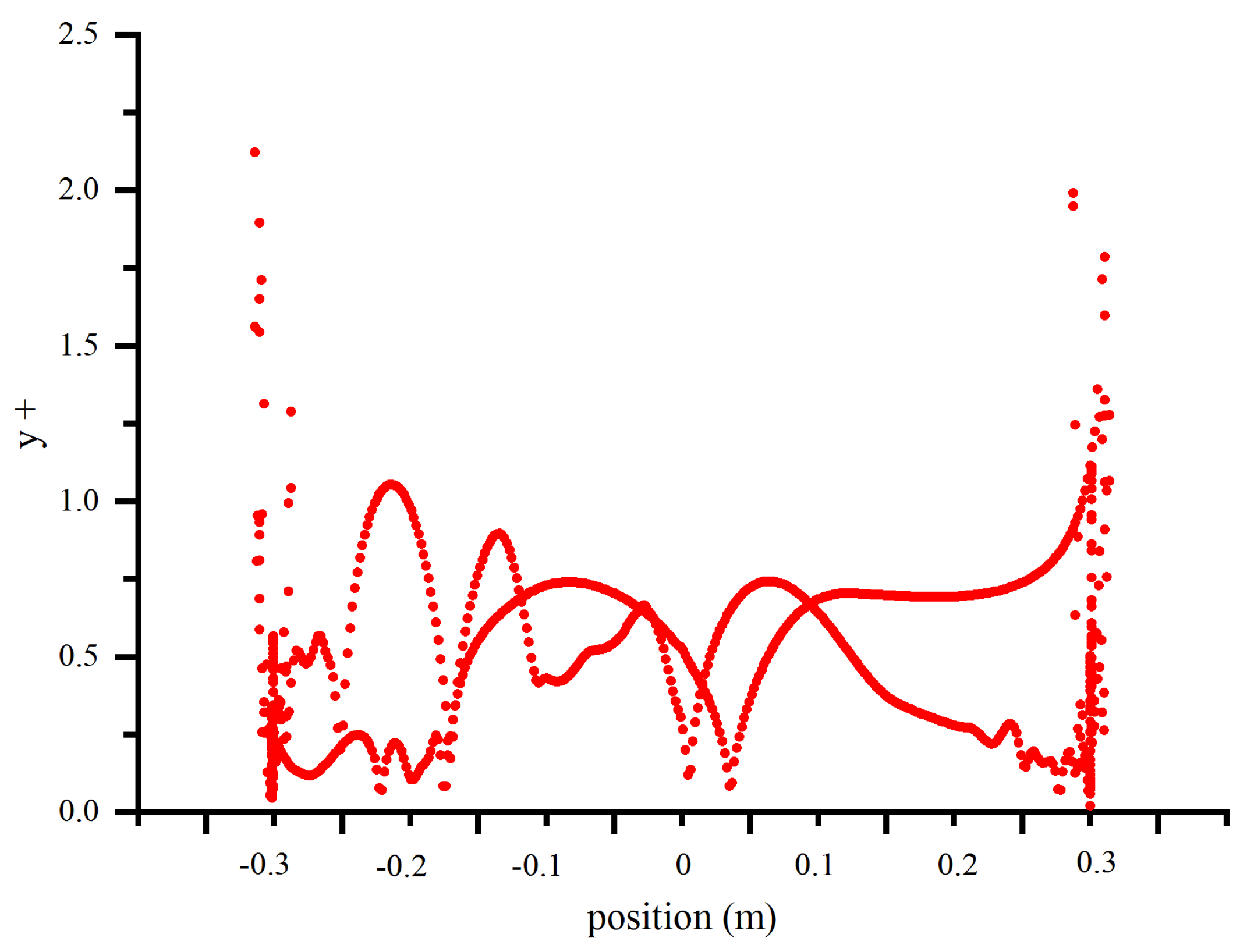
2.2.2. Numerical Simulation Verification
3. Results
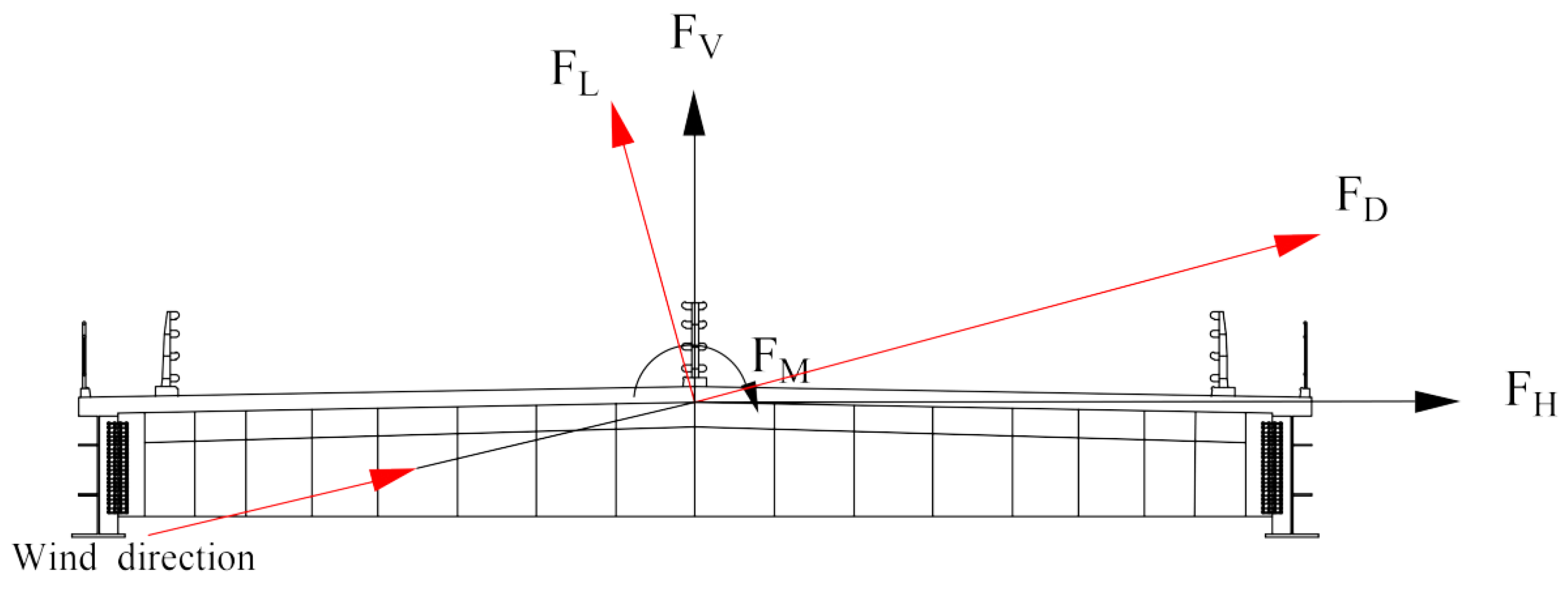
3.1. Static Wind Characteristics Results
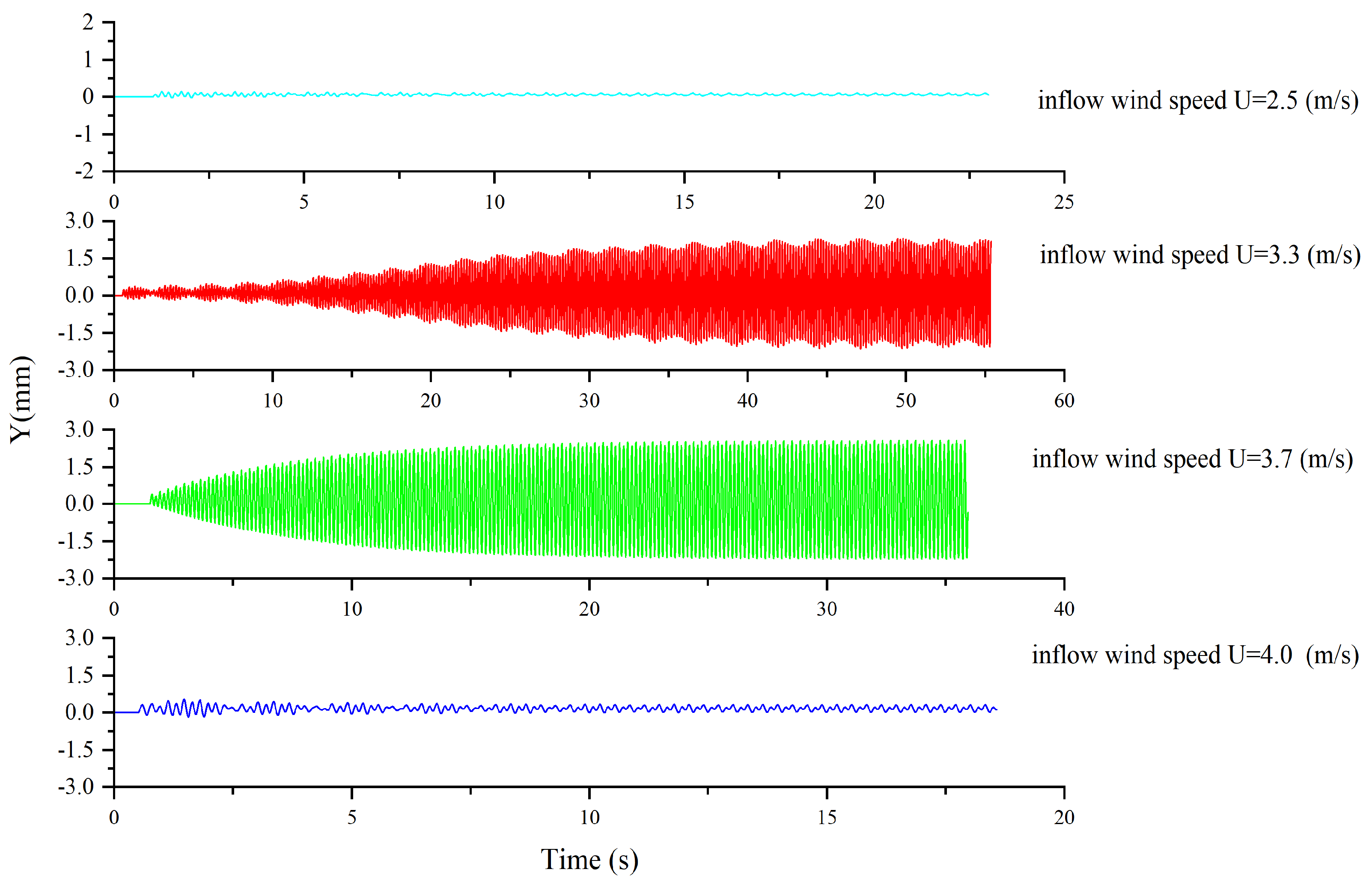
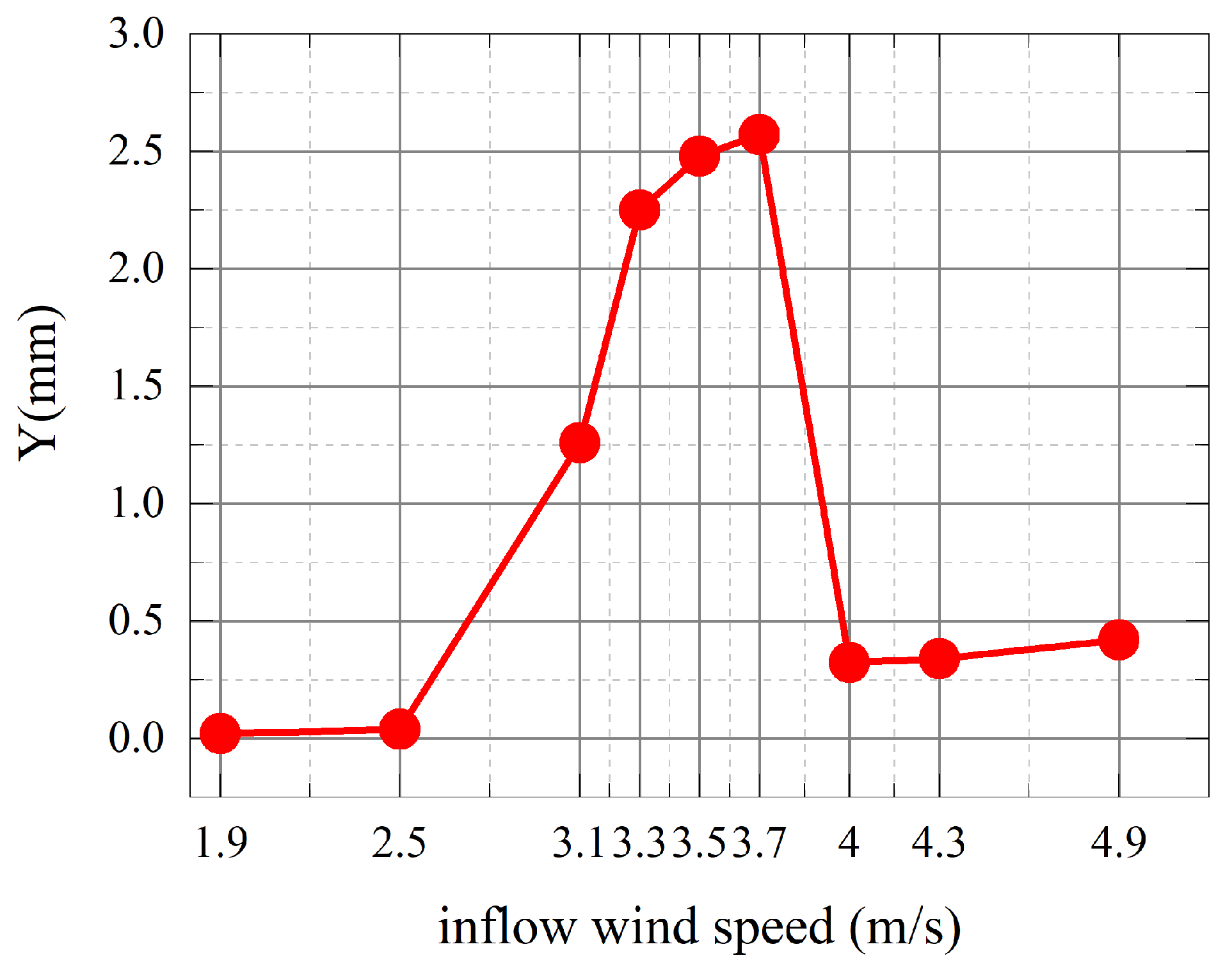
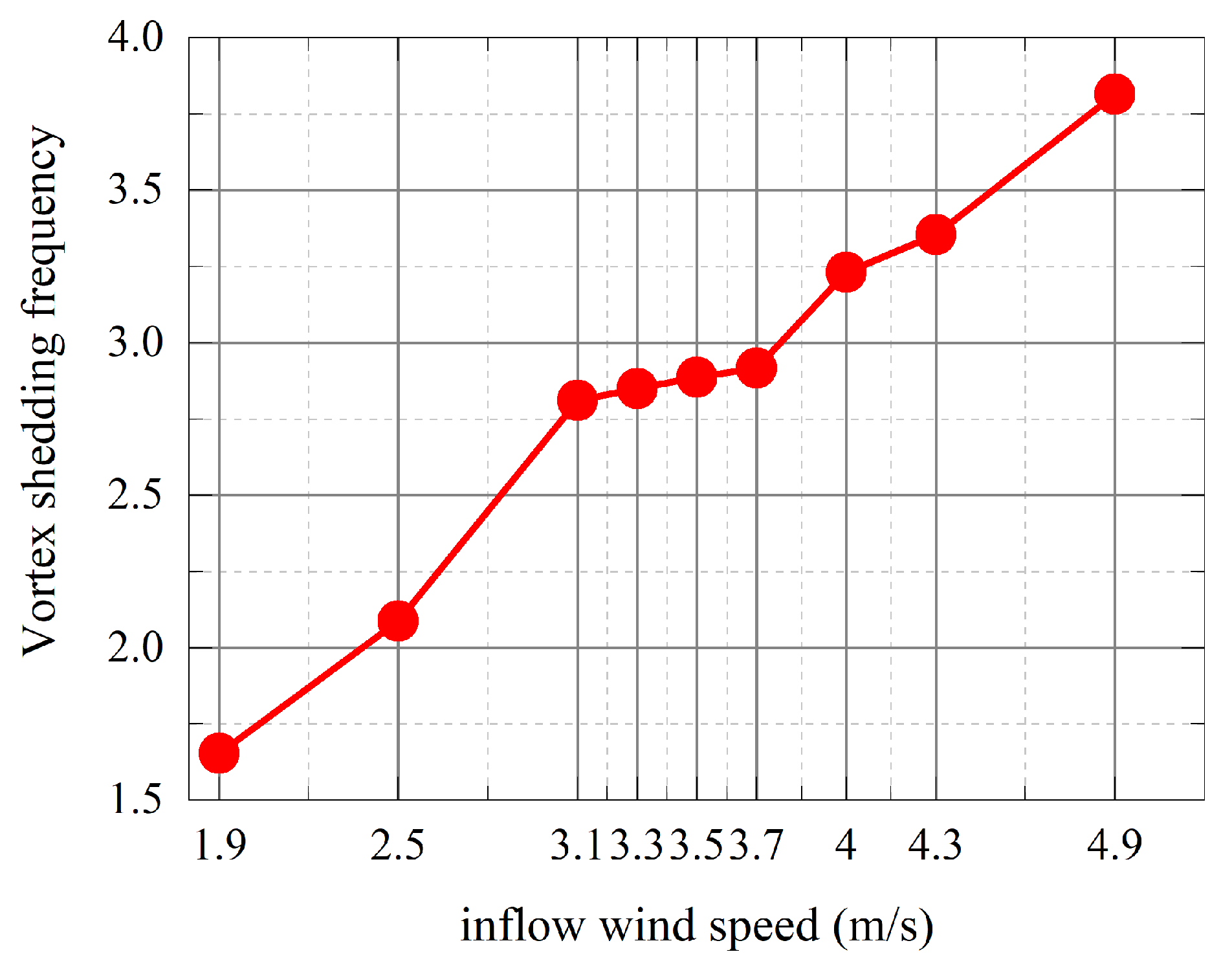
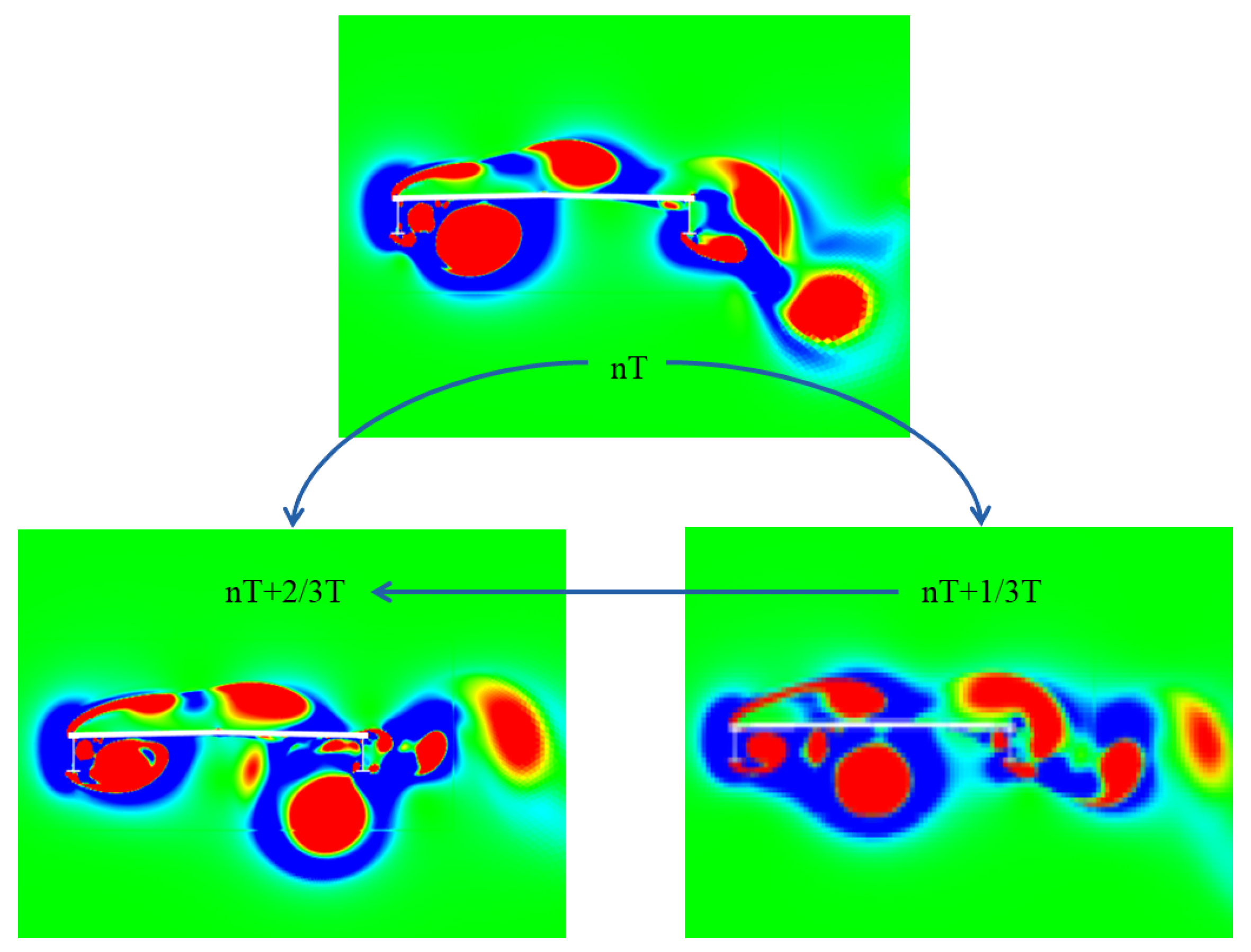
3.2. Vortex Vibration Performance Results
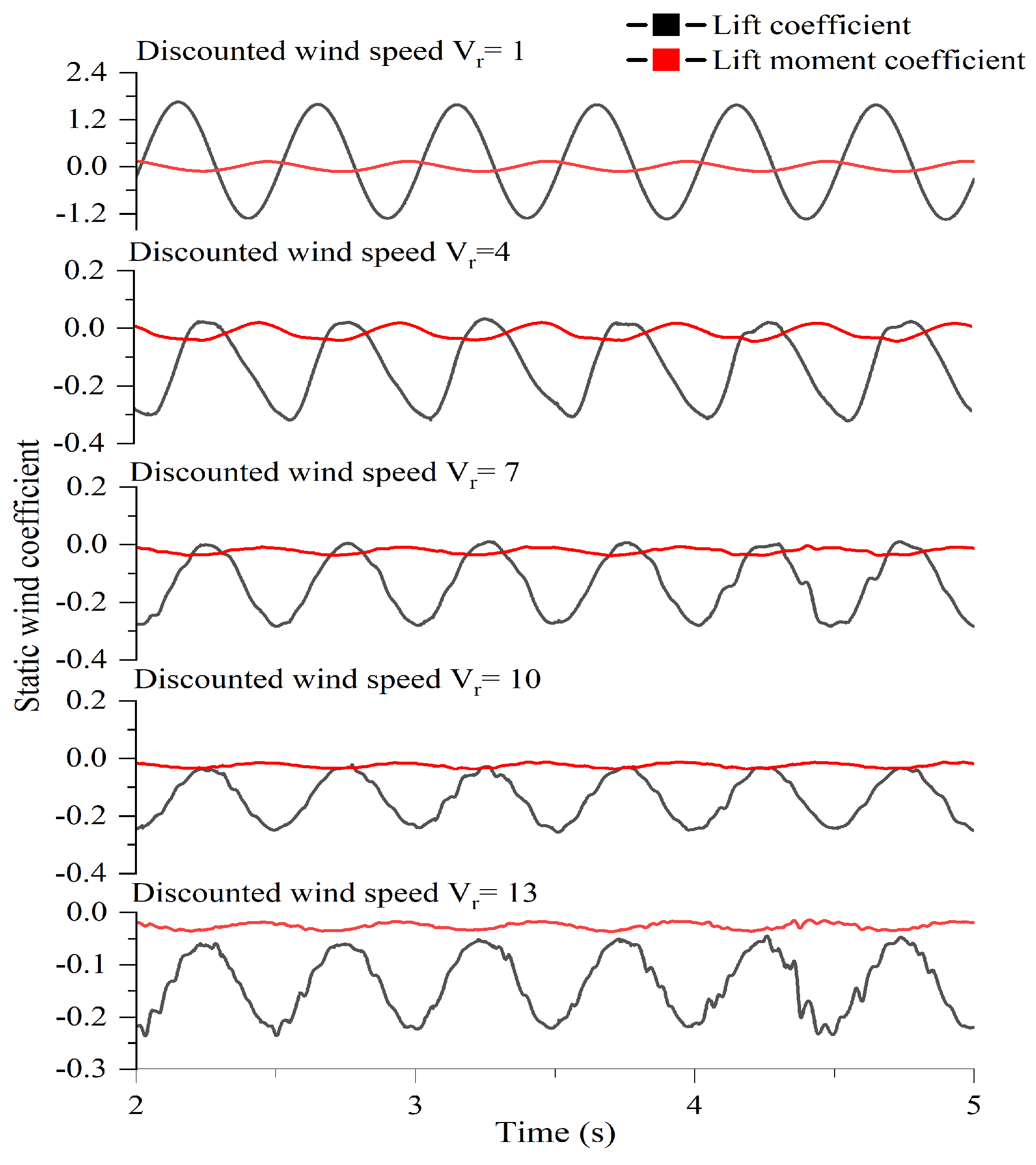
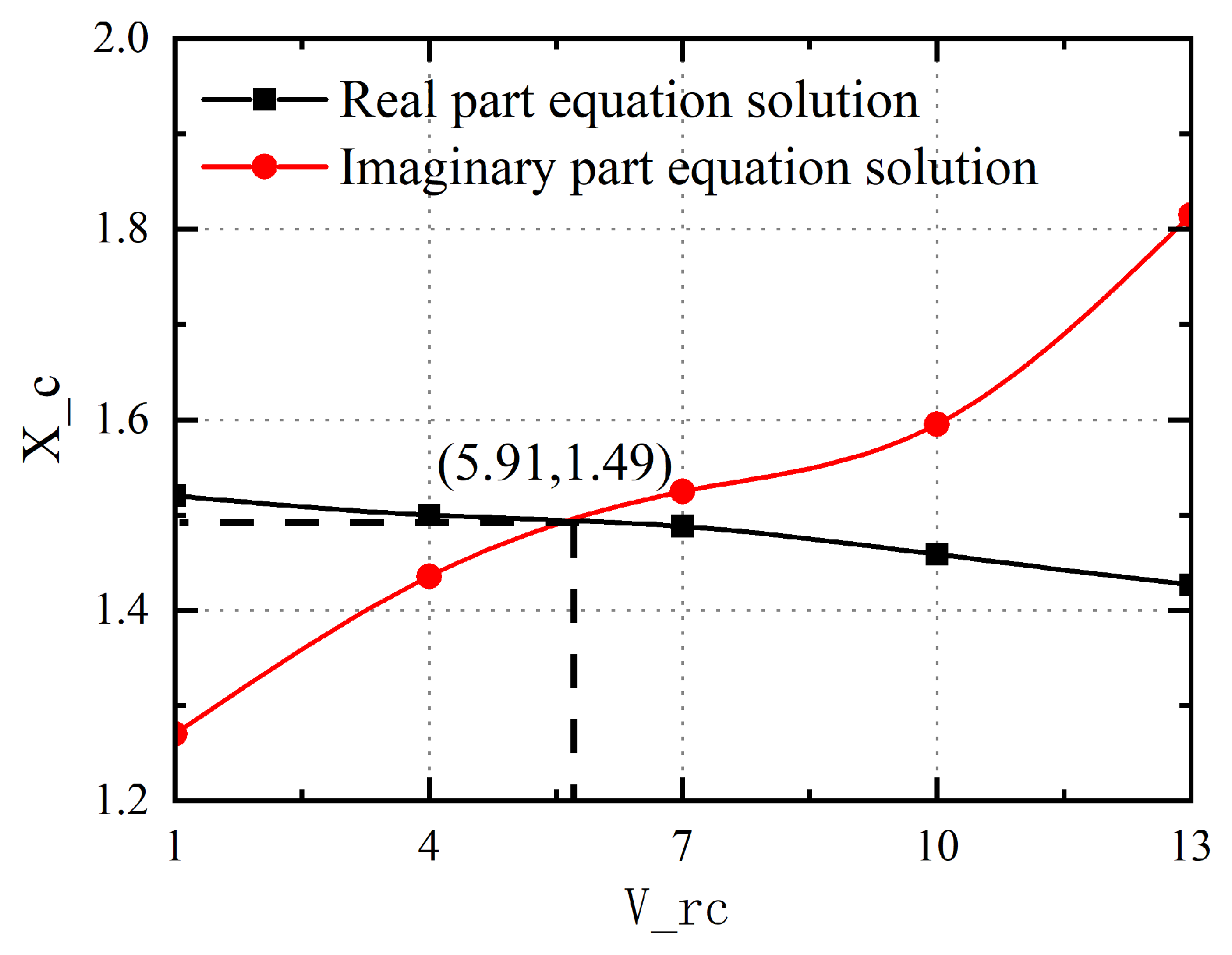
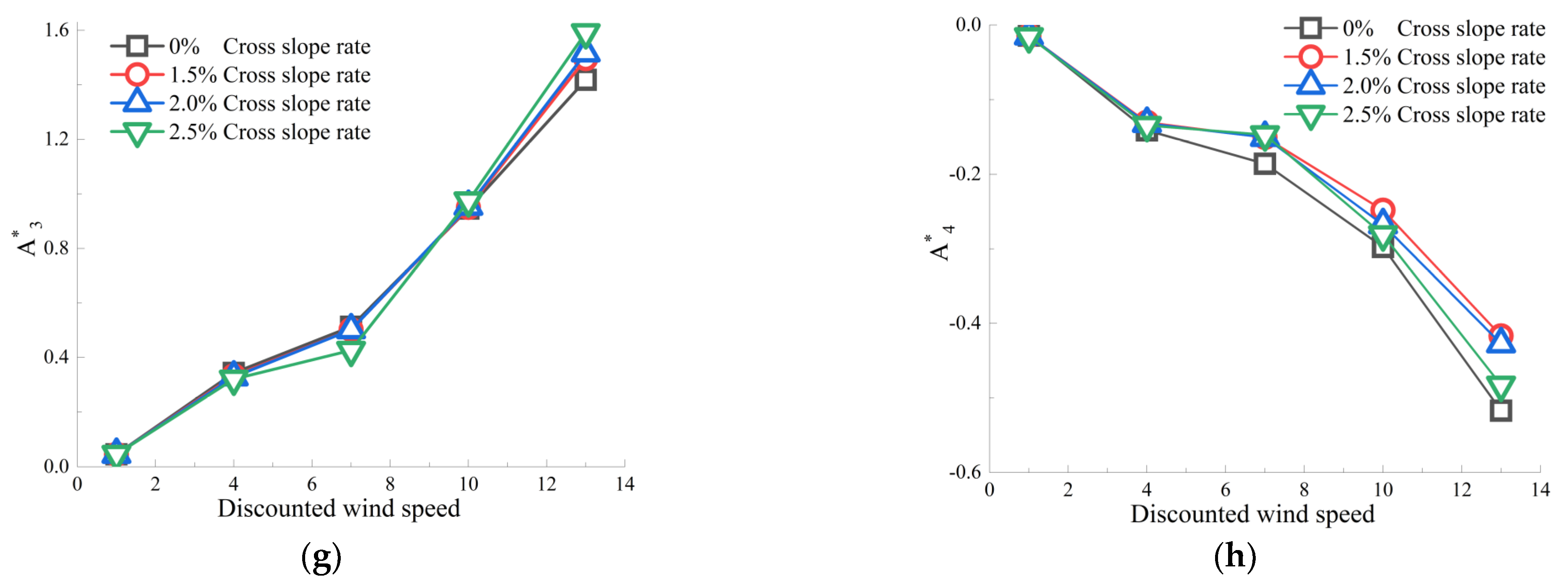
3.3. Flutter Performance Results
4. Discussion
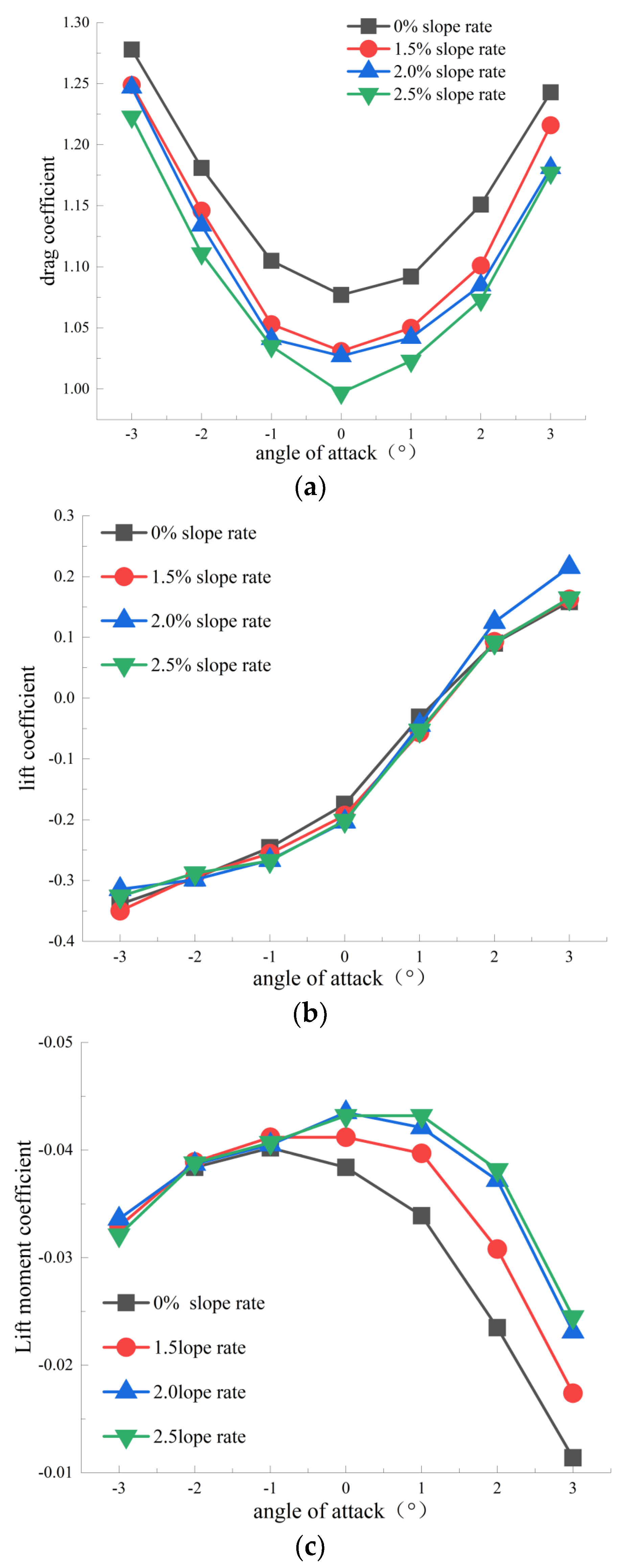
4.1. Effect on Static Wind Three-Component Force Coefficient
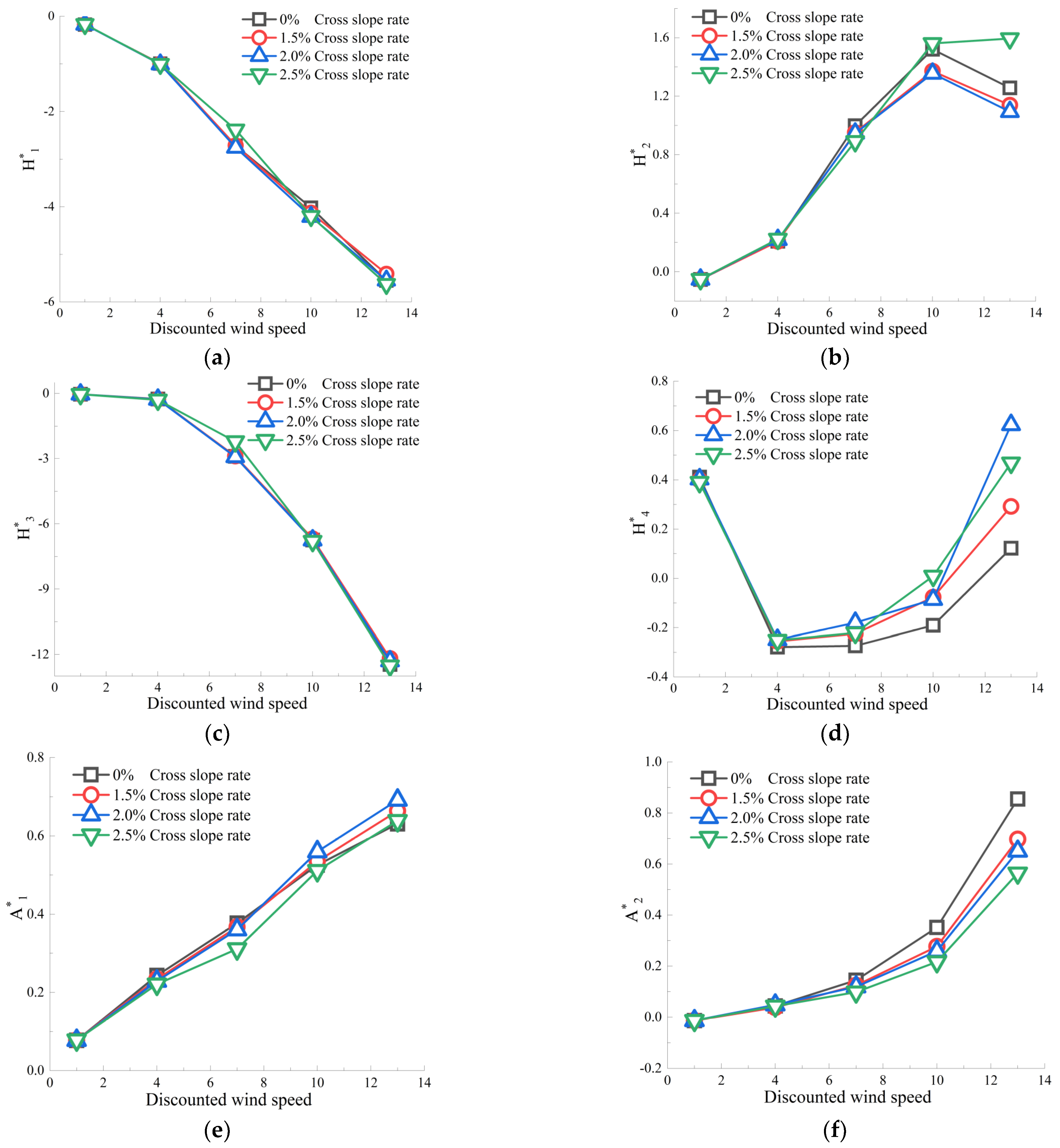
4.2. Effect on Flutter Stability
5. Conclusions
- (1)
- The static wind characteristics, vortex vibration performance, and flutter stability of the π-combined beam section of the Han River cable-stayed bridge were analyzed by numerical simulation, which provided a design reference for the preliminary wind resistance study. According to the analysis of the static wind characteristics of the Han River cable-stayed bridge, it is found that the change of the cross-slope of the beam section has a large influence on the resistance coefficient. The beam section with a 2.0% cross slope is relatively less affected by wind resistance, and the beam section without a cross slope can be used as the design benchmark to retain sufficient safety reserve space during design.
- (2)
- The vortex vibration was calculated for the π-shaped beam section of the Han River cable-stayed bridge by the loose-coupling method, so as to determine the wind speed “locking interval” as well as the maximum amplitude. It is found that the “locking phenomenon” occurred when the main beam vibration amplitude was larger in the wind speed range of 2.5–4.0 m/s. At the same time, the continuous vortex shedding up and down on the leeward side of the beam led to the vertical vortex-induced vibration of the π-shaped main beam section. It is necessary to further study the vibration suppression measures.
- (3)
- Eight aerodynamic derivatives of the Han River cable-stayed bridge were identified based on the time-domain method, and their flutter critical wind speeds were calculated. Within the discounted wind speed 13, the eight flutter derivatives are not very sensitive to the changes of the bridge deck cross slope ratios 0%, 1.5%, 2.0%, and 2.5%. However, compared with the case of no bridge deck cross slope, setting a certain bridge deck cross slope increases the flutter critical wind speed of the cable-stayed bridge. Due to the limited cases of bridge deck cross slope rates considered in the paper, the relationship between critical wind speed and bridge deck cross slope rates needs to be further investigated.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gao, G.; Zhu, L.; Han, W.; Li, J. Nonlinear post-flutter behavior and self-excited force model of a twin-side-girder bridge deck. J. Wind Eng. Ind. Aerodyn. 2018, 177, 227–241. [Google Scholar] [CrossRef]
- Vu, T.V.; Kim, Y.M.; Lee, H.E. Coupled flutter analysis of long-span bridges using full set of flutter derivatives. KSCE J. Civ. Eng. 2016, 20, 1501–1513. [Google Scholar] [CrossRef]
- Wu, B.; Liao, H.; Shen, H.; Wang, Q.; Mei, H.; Li, Z. Multimode coupled nonlinear flutter analysis for long-span bridges byconsidering dependence of flutter derivatives on vibration amplitude. Comput. Struct. 2022, 260, 106700. [Google Scholar] [CrossRef]
- Ruiheng, W. Study on the Stability of the Tower of a Single-Column Cable-Stayed Bridge with Variable Sections. Master’s Thesis, Chongqing University, Chongqing, China, 2019. [Google Scholar]
- Zhichao, Y.; Jianxin, Y.; Zhijun, N.; Ruiheng, W.; Liangliang, Z. Research on pylon galloping vibration of single-column bridge with variable cross-section of cable-stayed bridge. Highway 2020, 65, 207–211. [Google Scholar]
- Du, L.; Sun, X. Suppression of vortex-induced vibration using the rotary oscillation of a cylinder. Phys. Fluids 2015, 27, 023603. [Google Scholar] [CrossRef]
- Wang, W.; Wang, X.; Hua, X.; Song, G.; Chen, Z. Vibration control of vortex-induced vibrations of a bridge deck by a single-side pounding tuned mass damper. Eng. Struct. 2018, 173, 61–75. [Google Scholar] [CrossRef]
- Li, M.; Sun, Y.; Jing, H.; Li, M. Vortex-induced vibration optimization of a wide streamline box girder by wind tunnel test. KSCE J. Civ. Eng. 2018, 22, 5143–5153. [Google Scholar] [CrossRef]
- Kavrakov, I.; Argentini, T.; Omarini, S.; Rocchi, D.; Morgenthal, G. Determination of complex aerodynamic admittance of bridge decks under deterministic gusts using the Vortex Particle Method. J. Wind. Eng. Ind. Aerodyn. 2019, 193, 103971. [Google Scholar] [CrossRef]
- Lei, S.; Cui, W.; Patruno, L.; de Miranda, S.; Zhao, L.; Ge, Y. Improved state augmentation method for buffeting analysis of structures subjected to non-stationary wind. Probabilistic Eng. Mech. 2022, 69, 103309. [Google Scholar] [CrossRef]
- Ma, T.; Cui, W.; Zhao, L.; Yang, Y.; Ge, Y. Optimization of long-span suspension bridge erection procedure considering flutter risk in mixed extreme wind events. J. Wind Eng. Ind. Aerodyn. 2022, 222, 104889. [Google Scholar] [CrossRef]
- Haque, M.N.; Katsuchi, H.; Yamada, H.; Kim, H. Influence of geometric configuration on aerodynamics of streamlined bridge deck by unsteady RANS. Wind. Struct. Int. J. 2019, 28, 331–345. [Google Scholar]
- Haque, M.; Katsuchi, H.; Yamada, H.; Nishio, M. Investigation of edge fairing shaping effects on aerodynamic response of long-span bridge deck by unsteady RANS. Arch. Civ. Mech. Eng. 2016, 16, 888–900. [Google Scholar] [CrossRef]
- Guoqiang, Z. Influence of Railing Structure on Vortex-Induced Vibration of π-Shaped Bridge Section. Master’s Thesis, Chang’an University, Xi’An, China, 2015. [Google Scholar]
- Lee, H.; Moon, J.; Chun, N.; Lee, H.E. Effect of beam slope on the static aerodynamic response of edge-girder bridge-deck. Wind. Struct. 2017, 25, 157–176. [Google Scholar]
- Bruno, L.; Mancini, G. Importance of deck details in bridge aerodynamics. Struct. Eng. Int. 2002, 12, 289–294. [Google Scholar] [CrossRef]
- Wang, Q.; Liao, H.; Li, M.; Ma, C. Influence of aerodynamic configuration of a streamline box girder on bridge flutter and vortex-induced vibration. J. Mod. Transp. 2011, 19, 261–267. [Google Scholar] [CrossRef]
- 18. Shiling. Study on Shear Lag Effect of Main Girder of Cable-Stayed Bridge with Combined Beam. Master’s Thesis, Zhejiang University, Hangzhou, China, 2019.
- Thai, L.M.; Luat, D.T.; Van Ke, T.; Phung Van, M. Finite-Element Modeling for Static Bending Analysis of Rotating Two-Layer FGM Beams with Shear Connectors Resting on Imperfect Elastic Foundations. J. Aerosp. Eng. 2023, 36, 04023013. [Google Scholar] [CrossRef]
- Phung, M.V.; Nguyen, D.T.; Doan, L.T.; Nguyen, D.V.; Duong, T.V. Numerical investigation on static bending and free vibration responses of two-layer variable thickness plates with shear connectors. Iran. J. Sci. Technol. Trans. Mech. Eng. 2022, 46, 1047–1065. [Google Scholar] [CrossRef]
- Feng, W. Analysis of wind resistance performance of long-span continuous bridges based on CFD. Highw. Eng. 2018, 3. [Google Scholar]
- Mannini, C.; Šoda, A.; Schewe, G. Unsteady RANS modelling of flow past a rectangular cylinder: Investigation of Reynolds number effects. Comput. Fluids 2010, 39, 1609–1624. [Google Scholar] [CrossRef]
- Matsumoto, M.; Yagi, T.; Tamaki, H.; Tsubota, T. Vortex-induced vibration and its effect on torsional flutter instability in the case of B/D = 4 rectangular cylinder. J. Wind Eng. Ind. Aerodyn. 2008, 96, 971–983. [Google Scholar] [CrossRef]
- Shaobo, W.; Yuanjie, L.; Xingpei, L.; Minghao, Z.; Zhengwei, W. Coupled vibration analysis of elastic plate in viscous fluid. Chin. J. Mech. Eng. 2004, 40, 63–66. [Google Scholar]
- Zhixiong, W.; Zhitian, Z.; Kai, Q.; Changqing, W. Research on bending-torsional coupled vortex-induced resonance and aerodynamic vibration reduction measures of π-shaped open-section cable-stayed bridge. Vib. Shock. 2021, 40, 52–57, 94. [Google Scholar]
- Yihua, C. Forced Vibration Simulation Identification and Application of Wing Segment Flutter Derivatives. PhD Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2005. [Google Scholar]
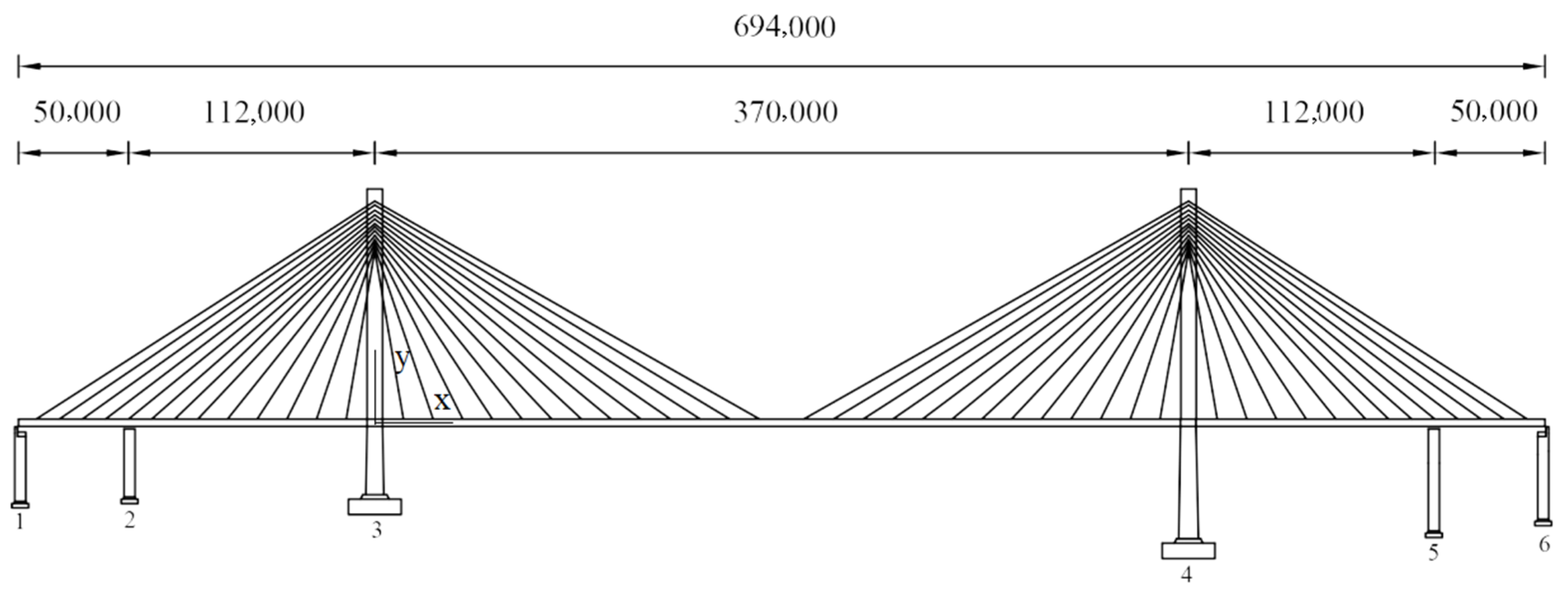
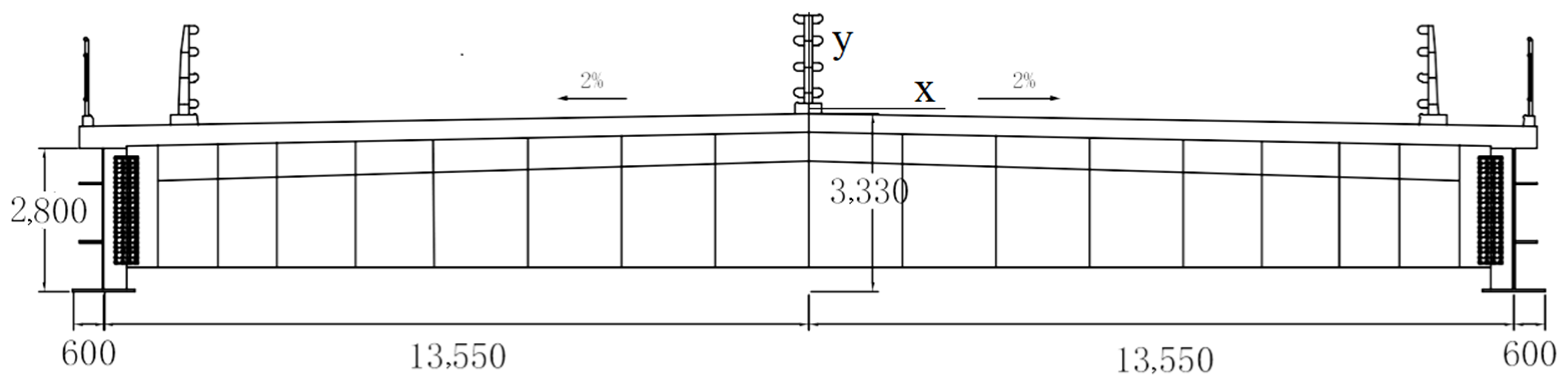
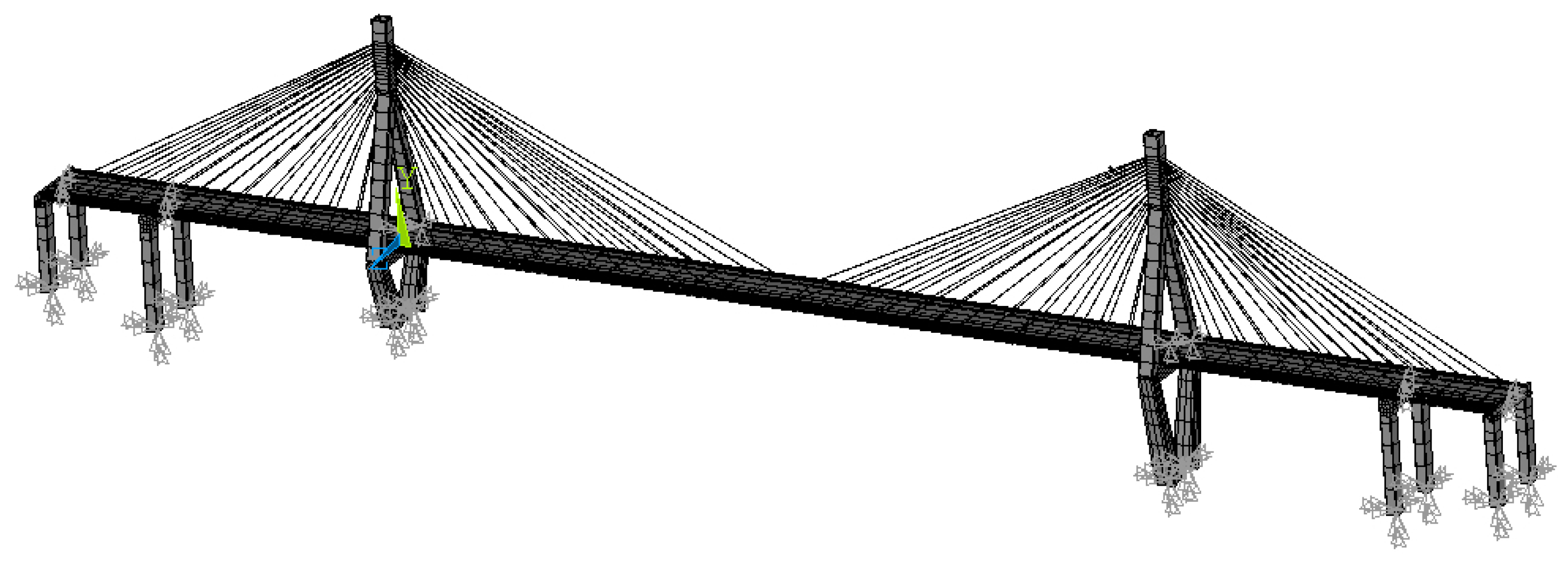
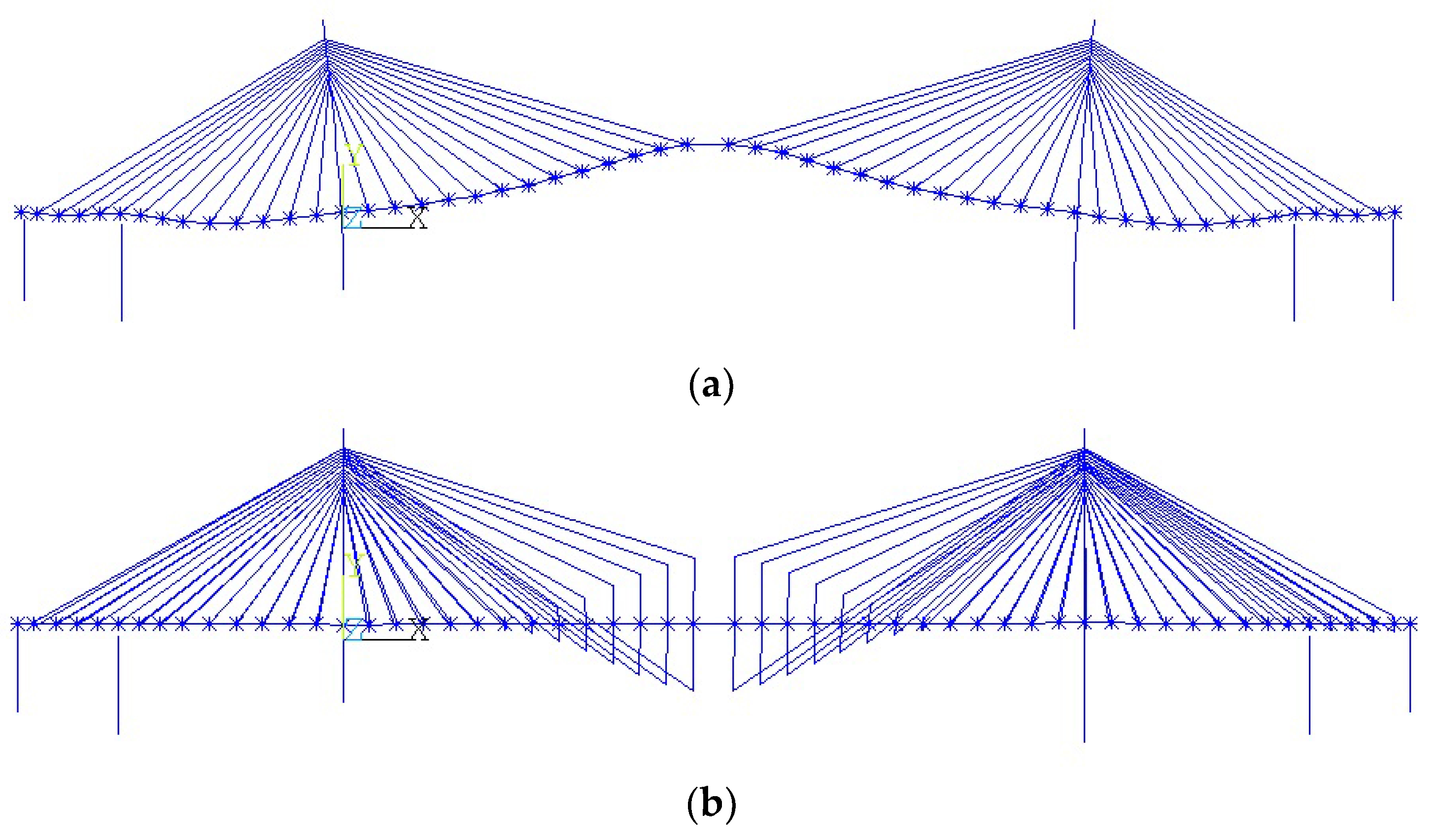






| Material Category | Material | Density (kg/m3) | Modulus of Elasticity (kN/m2) | Poisson’s Ratio |
|---|---|---|---|---|
| Steel for beam | Q370qENH | 7.85 × 103 | 2.06 × 1011 | 0.3 |
| Bridge deck concrete | C60 | 2.5 × 103 | 3.65 × 1010 | 0.2 |
| Bridge towers concrete | C50 | 2.5 × 103 | 3.45 × 1010 | 0.2 |
| Bridge pier concrete | C30 | 2.5 × 103 | 3.00 × 1010 | 0.2 |
| Cross-Sectional Category | Area (m2) | Ix (m4) | Iy (m4) |
|---|---|---|---|
| Beam section | 10.751 | 2.266 | 760.95 |
| Tower top section | 20.2853 | 125.929 | 90.7876 |
| Tower upper section | 18.2761 | 107.649 | 56.4391 |
| Tower cross section at cross beam | 32.9254 | 315.8245 | 203.532 |
| Tower bottom section | 23.3254 | 121.644 | 65.6024 |
| Order | Frequency/Hz | Vibration Type Features | Equivalent Mass kg/m or Equivalent Mass Moment of Inertia kg-m2/m |
|---|---|---|---|
| 2 | 0.388 | First order vertical bend | 42,863.87 |
| 5 | 0.591 | First order torsion | 8,132,574 |
| Boundary Conditions | Boundary Conditions for Different Wind Attack Angles α (°) | |
|---|---|---|
| α = 0 | α ≠ 0 | |
| Import boundaries | Speed portal | Velocity entry (vx = cosα, vy = sinα) |
| Upper boundary | Symmetrical boundaries | Velocity entry (vx = cosα, vy = sinα) |
| Lower boundary | Symmetrical boundaries | Velocity entry (vx = cosα, vy = sinα) |
| Export boundaries | Pressure outlet | Pressure outlet |
| Bridge cross-section | No sliding surface | No slippery wall surfaces |
| Parameter Name | Unit | Real Bridge Value | Scaling Ration | Model Values |
|---|---|---|---|---|
| Main beam height | m | 3.33 | 1/45 | 0.074 |
| Main beam width | m | 28.3 | 1/45 | 0.6283 |
| Equivalent mass | kg/m | 42,863.87 | 1/45 | 21.17 |
| Equivalent mass moment of inertia | kg-m2/m | 8,132,574 | 1/45 | 1.98 |
| Vertical bending fundamental frequency | Hz | 0.388 | 45/6 | 2.91 |
| Vertical bending damping ratio | 0.0079 | |||
| Wind speed ratio | 1/6 |
| Vertical Bending Frequency (Hz) | Torsion Frequency (Hz) | Vertical Bending Damping Ratio (%) | Torsional Damping Ratio (%) | Wind Speed Ratio |
|---|---|---|---|---|
| 2.91 | 4.43 | 0.005 | 0.005 | 6 |
| Slope of Bridge Deck Cross Slope (°) | Simulated Chattering Wind Speed (m/s) | Wind Speed Ratio | Wind Speed (m/s) at Solid Bridge Chatter Adjacent to the Street |
|---|---|---|---|
| 0 | 15.4 | 6 | 92.5 |
| 1.5 | 16.2 | 97.2 | |
| 2 | 16.1 | 96.6 | |
| 2.5 | 17.4 | 104.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Wang, X. Numerical Simulation Research Considering the Influence of Bridge Deck Cross Slope on Wind Performance of π-Type Composite Beam Cable-Stayed Bridge with a Symmetric Elevation Arrangement. Symmetry 2023, 15, 857. https://doi.org/10.3390/sym15040857
Zhang S, Wang X. Numerical Simulation Research Considering the Influence of Bridge Deck Cross Slope on Wind Performance of π-Type Composite Beam Cable-Stayed Bridge with a Symmetric Elevation Arrangement. Symmetry. 2023; 15(4):857. https://doi.org/10.3390/sym15040857
Chicago/Turabian StyleZhang, Shuaihui, and Xiangyang Wang. 2023. "Numerical Simulation Research Considering the Influence of Bridge Deck Cross Slope on Wind Performance of π-Type Composite Beam Cable-Stayed Bridge with a Symmetric Elevation Arrangement" Symmetry 15, no. 4: 857. https://doi.org/10.3390/sym15040857
APA StyleZhang, S., & Wang, X. (2023). Numerical Simulation Research Considering the Influence of Bridge Deck Cross Slope on Wind Performance of π-Type Composite Beam Cable-Stayed Bridge with a Symmetric Elevation Arrangement. Symmetry, 15(4), 857. https://doi.org/10.3390/sym15040857






