New Hermite–Hadamard Integral Inequalities for Geometrically Convex Functions via Generalized Weighted Fractional Operator

Abstract

The main purpose of this research is to concentrate on the development of new definitions for the weighted geometric fractional integrals of the left-hand side and right-hand side of the function ℵ with regard to an increasing function used as an integral kernel. Moreover, the newly developed class of left-hand side and right-hand side weighted geometric fractional integrals of a function ℵ, by applying an additional increasing function, identifies a variety of novel classes as special cases. This is a development of the previously established fractional integrals by making use of the class of geometrically convex functions. Geometrically convex functions in weighted fractional integrals of a function ℵ in the form of another rising function yield the Hermite–Hadamard inequality type. We also establish a novel midpoint identity and the associated inequalities for a class of weighted fractional integral functions known as geometrically convex with respect to an increasing function and symmetric with respect to the geometric mean of the endpoints of the interval. In order to demonstrate the validity of our research, we present examples. Moreover, fractional inequalities and their solutions are applied in many symmetrical domains.

1. Introduction and Preliminaries

The role of simple mathematical inequalities has been rediscovered over the last several decades as a result of their application to various branches of mathematics and applied research. In reality, the evolution of mathematical inequalities is extremely closely tied to the advancements in the theory of convex function. As is well known, the origins of the theory of convex functions may be traced back to the writings of several prominent mathematicians, including Jensen, Hardy, and Hadamard. In recent decades, researchers have been engaged in stimulating arguments over the convex function.

For simplicity, let us define the real function ℵ on a non-empty interval I of the real line $\mathbb{R}.$ If the inequality holds, then the function ℵ is said to be convex on I:

$$\aleph (\tau {\vartheta }_1+(1-\tau ){\vartheta }_2)\le \tau \aleph \left({\vartheta }_1\right)+(1-\tau )\aleph \left({\vartheta }_2\right),$$

(1)

holds for all ${\vartheta }_1,{\vartheta }_2\in I$ and $\tau \in [0,1]$.

On the subject of convex functions, the Hermite–Hadamard integral inequality (also known as the Hadamard-type integral inequality) is perhaps one of the most celebrated conclusions ever presented.

The following inequality is well known in the literature as the Hermite–Hadamard inequality.

Theorem 1.

Let $\aleph :I\subseteq \mathbb{R}\to \mathbb{R}$ be a convex function defined on the interval I of real numbers and ${\vartheta }_1,{\vartheta }_2\in I$ with ${\vartheta }_1<{\vartheta }_2.$ The following double inequality holds:

$$\aleph \left(\frac{{\vartheta }_1+{\vartheta }_2}{2}\right)\le \frac{1}{{\vartheta }_2-{\vartheta }_1}{\int }_{{\vartheta }_1}^{{\vartheta }_2}\aleph \left(x\right)dx\le \frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}.$$

(2)

The theory of convex sets and convex functions has received a lot of attention in recent years, and these concepts have been refined and extended in a range of fields. Thus, a wide range of Hermite–Hadamard-type inequalities and their applications have been discovered by a wide range of researchers across a wide range of fields, including mathematics, physics, biology, and chemistry. For important details about how the Hermite–Hadamard uniqueness theorem and basic convex function definitions have been improved, extended, and used, please see [1,2,3,4,5] and references therein.

Fractional calculus originated with a letter from L’Hospital to Leibniz inquiring about the notation that he was employing for the nth derivative of a linear function $\aleph \left(\tau \right)=\tau$, $\frac{D^n\tau }{D^{\tau }n}$. L’Hospital posed the question of what would happen if $n=\frac{1}{2}$. Leibniz said, “A seeming paradox from which practical ramifications will be deduced one day.” Mathematicians are increasingly turning to fractional calculus as a strong tool. According to Sarikaya and colleagues [6], the definitions of Riemannn–Liouville integrals were used to produce a novel version of the Hermite–Hadamard inequality. This finding piqued the interest of numerous researchers, who began investigating the subject further. For further information, as well as recent results and newly discovered properties pertaining to this operator, please see [7,8,9,10,11,12,13,14,15,16].

Definition 1

([17]). A function $\aleph :I\subseteq (0,\infty )\to \mathbb{R}$ is said to be geometrically convex (geometric arithmetically convex) if

$$\aleph \left({\vartheta }_1^{\tau }{\vartheta }_2^{1-\tau }\right)\le \tau \aleph \left({\vartheta }_1\right)+(1-\tau )\aleph \left({\vartheta }_2\right),$$

(3)

for all ${\vartheta }_1,{\vartheta }_2\in I$ and $\tau \in [0,1].$

Theorem 2.

A function $\aleph :I\subseteq (0,\infty )\to \mathbb{R}$ is geometrically convex on I and ${\vartheta }_1,{\vartheta }_2\in I$ with ${\vartheta }_1<{\vartheta }_2.$ If $\aleph \in L[{\vartheta }_1,{\vartheta }_2],$ then

$$\begin{array}{c}\hfill \aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\le \frac{1}{ln{\vartheta }_2-ln{\vartheta }_1}{\int }_{{\vartheta }_1}^{{\vartheta }_2}\frac{\aleph \left(\tau \right)}{\tau }d\tau \le \frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}.\end{array}$$

(4)

In [18], the authors introduced the notion of geometrically symmetric functions as follows.

Definition 2.

A function $\varpi :[{\vartheta }_1,{\vartheta }_2]\to \mathbb{R}$, $[{\vartheta }_1,{\vartheta }_2]\subseteq {\mathbb{R}}_{+}$, is said to be geometrically symmetric with respect to $\sqrt{{\vartheta }_1{\vartheta }_2},$ if

$$\varpi \left(\frac{{\vartheta }_1{\vartheta }_2}{\tau }\right)=\varpi \left(\tau \right),\tau \in [{\vartheta }_1,{\vartheta }_2].$$

(5)

The authors in [18] also proved the following Fejér-type integral inequalities, which provide a weighted generalization of (4).

Theorem 3.

A function $\aleph :I\subseteq (0,\infty )\to \mathbb{R}$ is geometrically convex on I and ${\vartheta }_1,{\vartheta }_2\in I$ with ${\vartheta }_1<{\vartheta }_2.$ If $\varpi :[{\vartheta }_1,{\vartheta }_2]\to (0,\infty )$ is continuous, positive, and geometrically symmetric to $\sqrt{{\vartheta }_1{\vartheta }_2}$, then

$$\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right){\int }_{{\vartheta }_1}^{{\vartheta }_2}\frac{\varpi \left(\tau \right)}{\tau }d\tau \le {\int }_{{\vartheta }_1}^{{\vartheta }_2}\frac{\aleph \left(\tau \right)\varpi \left(\tau \right)}{\tau }d\tau \le \frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}{\int }_{{\vartheta }_1}^{{\vartheta }_2}\frac{\varpi \left(\tau \right)}{\tau }d\tau .$$

(6)

In [19], İşcan, İ. proved that Hermite–Hadamard inequalities can be represented for geometrically convex functions in fractional integral form as follows:

Theorem 4.

A function $\aleph :I\subseteq (0,\infty )\to \mathbb{R}$ is geometrically convex on I and ${\vartheta }_1,{\vartheta }_2\in I$ with ${\vartheta }_1<{\vartheta }_2.$ If ℵ is a geometrically convex function on $[{\vartheta }_1,{\vartheta }_2]$, then the following inequalities for fractional integrals hold:

$$\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\le \frac{\Gamma (\alpha +1)}{2{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\alpha }}\left\{J_{{{\vartheta }_1}^{+}}^{\alpha }\aleph \left({\vartheta }_2\right)+J_{{{\vartheta }_2}^{-}}^{\alpha }\aleph \left({\vartheta }_1\right)\right\}\le \frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}.$$

(7)

In [20], Kunt, proved fractional Hermite–Hadamard–Fejér-type inequalities for geometrically convex functions:

Theorem 5.

A function $\aleph :I\subseteq (0,\infty )\to \mathbb{R}$ is geometrically convex on I and ${\vartheta }_1,{\vartheta }_2\in I$ with ${\vartheta }_1<{\vartheta }_2.$ If $w:[{\vartheta }_1,{\vartheta }_2]\to (0,\infty )$ is continuous, positive, and geometrically symmetric to $\sqrt{{\vartheta }_1{\vartheta }_2}$, then

$$\begin{array}{cc}\hfill \aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left\{J_{{{\vartheta }_1}^{+}}^{\alpha }\varpi \left({\vartheta }_2\right)+J_{{{\vartheta }_2}^{-}}^{\alpha }\varpi \left({\vartheta }_1\right)\right\}& \le \left\{J_{{{\vartheta }_1}^{+}}^{\alpha }\left(\aleph w\right)\left({\vartheta }_2\right)+J_{{{\vartheta }_2}^{-}}^{\alpha }\left(\aleph w\right)\left({\vartheta }_1\right)\right\}\hfill \\ \hfill & \le \frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}\left\{J_{{{\vartheta }_1}^{+}}^{\alpha }\varpi \left({\vartheta }_2\right)+J_{{{\vartheta }_2}^{-}}^{\alpha }\varpi \left({\vartheta }_1\right)\right\}.\hfill \end{array}$$

(8)

In this paper, we will prove the left-hand side and right-hand side weighted fractional and fractional Hermite-Hadamard-Fejér-type inequalities for geometrically convex functions. Moreover, we will obtain the identity for differentiable functions. Using this identity, we will establish some midpoint-type error estimations for geometrically convex functions with respect to an increasing function containing positive weighted geometrically symmetric function forms. Furthermore, we observe the validity of Theorems 6–9 in Figure 1, Figure 2, Figure 3 and Figure 4 respectively.

2. Weighted Fractional Integral

Definition 3.

Let $\aleph \in L[{\vartheta }_1,{\vartheta }_2]$, $[{\vartheta }_1,{\vartheta }_2]\subseteq \left(0,\infty \right).$ Let $\varpi :[{\vartheta }_1,{\vartheta }_2]\to \mathbb{R}$ be positive, integrable, and symmetric weighted functions. If ℘ is an increasing and positive function from $[{\vartheta }_1,{\vartheta }_2)$ onto itself such that its derivative ${\wp }^{\prime }$ is continuous on $({\vartheta }_1,{\vartheta }_2),$ then the left-hand side and right-hand side weighted fractional integrals of order $v>0$, respectively, are given as

$$\left({}_{\varpi }{\mathcal{I}}_{{\vartheta }_1^{+}}^{v,\wp }\aleph \right)\left(\tau \right)=\frac{{\varpi }^{-1}\left(\tau \right)}{\Gamma \left(v\right)}{\int }_{{\vartheta }_1}^{\tau }\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}{\left(ln\frac{\wp \left(\tau \right)}{\wp \left(\kappa \right)}\right)}^{v-1}\aleph \left(\kappa \right)\varpi \left(\kappa \right)d\kappa$$

(9)

and

$$\left({}_{\varpi }{\mathcal{I}}_{{\vartheta }_2^{-}}^{v,\wp }\aleph \right)\left(\tau \right)=\frac{{\varpi }^{-1}\left(\tau \right)}{\Gamma \left(v\right)}{\int }_{\tau }^{{\vartheta }_2}\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}{\left(ln\frac{\wp \left(\kappa \right)}{\wp \left(\tau \right)}\right)}^{v-1}\aleph \left(\kappa \right)\varpi \left(\kappa \right)d\kappa ,$$

(10)

for ${\varpi }^{-1}\left(\tau \right):=\frac{1}{\varpi \left(\tau \right)}.$

Remark 1. 

(i) Putting $\varpi \left(\tau \right)=1$, the operators (9) and (10) reduce to the fractional integrals of order $v>0$ with regard to the function $\wp \left(\tau \right)$ as follows:

$$\begin{array}{cc}\hfill & \left({\mathcal{I}}_{{\vartheta }_1^{+}}^{v,\wp }\aleph \right)\left(\tau \right)=\frac{1}{\Gamma \left(v\right)}{\int }_{{\vartheta }_1}^{\tau }\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}{\left(ln\frac{\wp \left(\tau \right)}{\wp \left(\kappa \right)}\right)}^{v-1}\aleph \left(\kappa \right)d\kappa \hfill \end{array}$$

and

$$\begin{array}{cc}\hfill & \left({\mathcal{I}}_{{\vartheta }_2^{-}}^{v,\wp }\aleph \right)\left(\tau \right)=\frac{1}{\Gamma \left(v\right)}{\int }_{\tau }^{{\vartheta }_2}\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}{\left(ln\frac{\wp \left(\kappa \right)}{\wp \left(\tau \right)}\right)}^{v-1}\aleph \left(\kappa \right)d\kappa .\hfill \end{array}$$

(ii) 

Putting $\wp \left(\tau \right)=\tau$, the operators (9) and (10) reduce to the weighted fractional integrals of order $v>0$ as follows:

$$\left({}_{\varpi }{\mathcal{I}}_{{\vartheta }_1^{+}}^v\aleph \right)\left(\tau \right)=\frac{{\varpi }^{-1}\left(\tau \right)}{\Gamma \left(v\right)}{\int }_{{\vartheta }_1}^{\tau }{\left(ln\frac{\tau }{\kappa }\right)}^{v-1}\frac{\aleph \left(\kappa \right)\varpi \left(\kappa \right)}{\kappa }d\kappa$$

and

$$\left({}_{\varpi }{\mathcal{I}}_{{\vartheta }_2^{-}}^v\aleph \right)\left(\tau \right)=\frac{{\varpi }^{-1}\left(\tau \right)}{\Gamma \left(v\right)}{\int }_{\tau }^{{\vartheta }_2}{\left(ln\frac{\kappa }{\tau }\right)}^{v-1}\frac{\aleph \left(\kappa \right)\varpi \left(\kappa \right)}{\kappa }d\kappa ,$$

for ${\varpi }^{-1}\left(\tau \right):=\frac{1}{\varpi \left(\tau \right)}.$

(iii) 

Putting $\varpi \left(\tau \right)=1$ and $\wp \left(\tau \right)=\tau$, the operators (9) and (10) reduce to Hadamard fractional integrals of order $v>0$, which is defined by [21]:

$$\left({\mathcal{I}}_{{\vartheta }_1^{+}}^v\aleph \right)\left(\tau \right)=\frac{1}{\Gamma \left(v\right)}{\int }_{{\vartheta }_1}^{\tau }{\left(ln\frac{\tau }{\kappa }\right)}^{v-1}\frac{\aleph \left(\kappa \right)}{\kappa }d\kappa$$

and

$$\left({\mathcal{I}}_{{\vartheta }_2^{-}}^v\aleph \right)\left(\tau \right)=\frac{1}{\Gamma \left(v\right)}{\int }_{\tau }^{{\vartheta }_2}{\left(ln\frac{\kappa }{\tau }\right)}^{v-1}\frac{\aleph \left(\kappa \right)}{\kappa }d\kappa .$$

This work shows how to assess a variety of Hermite–Hadamard–Fejér inequalities using weighted fractional operators with a positive geometrically symmetric weight function in the kernel.

3. Main Results

Throughout this paper, the functions ${\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right),{\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right):I\subset (0,\infty )\to \mathbb{R}$, being a differentiable function on $I^{\circ }$, the interior of I, are defined as:

$${\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)={\vartheta }_1^{\frac{\kappa }{2}}{\vartheta }_2^{\frac{2-\kappa }{2}}$$

and

$${\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)={\vartheta }_1^{\frac{2-\kappa }{2}}{\vartheta }_2^{\frac{\kappa }{2}}.$$

Lemma 1.

The following results hold.

(i)

Let $\varpi :[{\vartheta }_1,{\vartheta }_2]\to \mathbb{R}$ be an integrable function and geometrically symmetric weighted function with respect to $\sqrt{{\vartheta }_1{\vartheta }_2},$ with ${\vartheta }_1<{\vartheta }_2$, and then we have

$$\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)=\varpi \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right),$$

(11)

for every $\kappa \in [0,1].$

(ii)

For order $v>0$ and ℘ is an increasing and positive function from $[{\vartheta }_1,{\vartheta }_2)$ onto itself

$$\begin{array}{cc}\hfill & \left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)=\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ & =\frac{1}{2}\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\hfill \end{array}$$

(12)

Proof. 

Suppose that $\tau ={\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)={\vartheta }_1^{\frac{\kappa }{2}}{\vartheta }_2^{\frac{2-\kappa }{2}}$ with $\tau \in \left[{\vartheta }_1,{\vartheta }_2\right]$ and $\kappa \in [0,1],$ and then we have

$$\frac{{\vartheta }_1{\vartheta }_2}{\tau }={\vartheta }_1^{\frac{2-\kappa }{2}}{\vartheta }_2^{\frac{\kappa }{2}}={\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right).$$

Using the geometrically symmetric of $\varpi$ with respect to $\sqrt{{\vartheta }_1{\vartheta }_2}$, we have

$$\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)=\varpi \left(\tau \right)=\varpi \left(\frac{{\vartheta }_1{\vartheta }_2}{\tau }\right)=\varpi \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right).$$

Using the geometrically symmetric of $\varpi ,$ we have

$$\left(\varpi \circ \wp \right)\left(\kappa \right)=\varpi \left(\wp \left(\kappa \right)\right)=\varpi \left(\frac{{\vartheta }_1{\vartheta }_2}{\wp \left(\kappa \right)}\right),\forall \kappa \in \left[{\wp }^{-1}\left({\vartheta }_1\right),{\wp }^{-1}\left({\vartheta }_2\right)\right].$$

Hence, from the above and setting $\wp \left(\tau \right):=\frac{{\vartheta }_1{\vartheta }_2}{\wp \left(\kappa \right)}$, it follows that

$$\begin{array}{cc}\hfill & \left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\hfill \\ \hfill & =\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \hfill \\ \hfill & =\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^{v-1}\varpi \left(\frac{{\vartheta }_1{\vartheta }_2}{\wp \left(\kappa \right)}\right)\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}d\kappa \hfill \\ \hfill & =\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\kappa \right)\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}d\kappa \hfill \\ \hfill & =\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right),\hfill \end{array}$$

which brings the needed equality (12). □

4. Weighted Fractional Hermite–Hadamard-Type Inequalities for Geometrically Convex Functions

It is our goal in this section to develop some novel weighted fractional Hermite–Hadamard-type inequalities for geometrically convex functions with regard to an increasing function that has a positive weighted geometrically symmetric function form that are not previously known.

Theorem 6.

Let $\aleph :[{\vartheta }_1,{\vartheta }_2]\subset (0,\infty )\to \mathbb{R}$ be a geometrically convex function such that $\aleph \in L[{\vartheta }_1,{\vartheta }_2]$ with ${\vartheta }_1,{\vartheta }_2\in I$ and $v>0.$ Let $\varpi :[{\vartheta }_1,{\vartheta }_2]\to \mathbb{R}$ be a positive, integrable, and geometrically symmetric weight function with respect to $\sqrt{{\vartheta }_1{\vartheta }_2}$. If ℘ is an increasing and positive function from $[{\vartheta }_1,{\vartheta }_2)$ onto itself such that its derivative ${\wp }^{\prime }$ is continuous on $({\vartheta }_1,{\vartheta }_2)$, then the following inequalities for fractional integrals hold:

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\right.\\ \left.\times \left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\le \varpi \left({\vartheta }_2\right)\left({}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}{\mathcal{I}}_{\varpi \circ \wp }^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\\ +\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\\ \le \left[\frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}\right]\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right.\\ +\left.\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\end{array}$$

(13)

Proof. 

Using the geometric convexity of the function ℵ on $[{\vartheta }_1,{\vartheta }_2]$, we write

$$\aleph \left(\sqrt{{\pi }_1{\pi }_2}\right)\le \frac{\aleph \left({\pi }_1\right)+\aleph \left({\pi }_2\right)}{2},\mathrm{for}\mathrm{all}{\pi }_1,{\pi }_2\in \left[{\vartheta }_1,{\vartheta }_2\right].$$

Choosing ${\pi }_1={\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)$ and ${\pi }_2={\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)$, $\kappa \in [0,1],$ it follows that

$$2\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\le \aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)+\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right).$$

(14)

Multiplying both sides of (14) by ${\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)$ and integrating the resulting inequality with respect to $\kappa$ over $[0,1]$, we obtain

$$\begin{array}{c}2\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right){\int }_0^1{\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa \\ \le {\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa \\ +{\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa .\end{array}$$

(15)

From the left-hand side of the inequality in (15), we use (12) to obtain

$$\begin{array}{c}\frac{\Gamma \left(v\right)}{2{\left(ln\frac{{\vartheta }_2}{{\vartheta }_{{}_1}}\right)}^v}\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ =\frac{\Gamma \left(v\right)}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_{{}_1}}\right)}^v}\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\hfill \\ =\frac{1}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_{{}_1}}\right)}^v}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \hfill \\ ={\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(\frac{ln{\vartheta }_2-ln\wp \left(\tau \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}\frac{d\tau }{ln\frac{{\vartheta }_2}{{\vartheta }_1}}\hfill \\ ={\int }_0^1{\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa ,\left[\mathrm{where}\kappa :=\frac{ln{\vartheta }_2-ln\wp \left(\tau \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right].\hfill \end{array}$$

(16)

It follows that

$$\begin{array}{c}2\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right){\int }_0^1{\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa =\frac{\Gamma \left(v\right)}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\hfill \\ \times \left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\hfill \end{array}$$

(17)

It is possible to prove this by computing the weighted fractional operators,

$$\begin{array}{cc}\hfill & \varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ & =\varpi \left({\vartheta }_2\right)\frac{{\left(\varpi \circ \wp \right)}^{-1}{\wp }^{-1}\left({\vartheta }_2\right)}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\aleph \circ \wp \right)\left(\tau \right)\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \hfill \\ \hfill & +\varpi \left({\vartheta }_1\right)\frac{{\left(\varpi \circ \wp \right)}^{-1}{\wp }^{-1}\left({\vartheta }_1\right)}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\aleph \circ \wp \right)\left(\tau \right)\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \hfill \\ & =\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(\frac{ln{\vartheta }_2-ln\wp \left(\tau \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right)}^{v-1}\left(\aleph \circ \wp \right)\left(\tau \right)\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}\frac{d\tau }{ln\frac{{\vartheta }_2}{{\vartheta }_1}}\hfill \\ \hfill & +\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(\frac{ln\wp \left(\tau \right)-ln{\vartheta }_1}{ln{\vartheta }_2-ln{\vartheta }_1}\right)}^{v-1}\left(\aleph \circ \wp \right)\left(\tau \right)\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}\frac{d\tau }{ln\frac{{\vartheta }_2}{{\vartheta }_1}}.\hfill \end{array}$$

Setting ${\lambda }_1=\frac{ln{\vartheta }_2-ln\wp \left(\tau \right)}{ln{\vartheta }_2-ln{\vartheta }_1}$ and ${\lambda }_2=\frac{ln\wp \left(\tau \right)-ln{\vartheta }_1}{ln{\vartheta }_2-ln{\vartheta }_1}$, one can deduce that

$$\begin{array}{c}\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_0^1\lambda {{}_1}^{v-1}\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\lambda {}_1\\ \hfill +\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_0^1\lambda {{}_2}^{v-1}\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)d\lambda {}_2.\end{array}$$

By using the geometrically symmetric weighted function of Equation (11), we obtain the required calculation

$$\begin{array}{c}\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_0^1\lambda {{}_1}^{v-1}\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\lambda {}_1\\ \hfill +\frac{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}{\Gamma \left(v\right)}{\int }_0^1\lambda {{}_2}^{v-1}\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\lambda {}_2.\end{array}$$

As a consequence,

$$\begin{array}{c}{\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa +{\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa \\ =\frac{\Gamma \left(v\right)}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^v}\left[\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right.\\ \left.+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\end{array}$$

(18)

The following is the outcome of putting (17) and (18) into (15)

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\\ \le \varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\\ +\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right).\end{array}$$

(19)

Finally, it has been established that the left inequality of (13) has been demonstrated. We can show the second inequality of (13) using the geometrically convex function of ℵ, which yields

$$\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\le \frac{\kappa }{2}\aleph \left({\vartheta }_1\right)+\frac{2-\kappa }{2}\aleph \left({\vartheta }_2\right).$$

(20)

$$\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\le \frac{2-\kappa }{2}\aleph \left({\vartheta }_1\right)+\frac{\kappa }{2}\aleph \left({\vartheta }_2\right).$$

(21)

Adding (20) and (21), we have

$$\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)+\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\le \aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right).$$

(22)

Multiplying both sides of (22) by ${\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)$, we obtain the following by integrating the resulting inequality in terms of $\kappa$ on $[0,1]$.

$$\begin{array}{cc}\hfill {\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa & +{\int }_0^1{\kappa }^{v-1}\aleph \left({\Theta }_{{\vartheta }_2,{\vartheta }_1}\left(\kappa \right)\right)\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa \hfill \\ \hfill & \le \left[\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)\right]{\int }_0^1{\kappa }^{v-1}\varpi \left({\Theta }_{{\vartheta }_1,{\vartheta }_2}\left(\kappa \right)\right)d\kappa .\hfill \end{array}$$

(23)

Then, by using (18) in (23), we have

$$\begin{array}{c}\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\\ +\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\\ \le \left[\frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}\right]\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right.\\ +\left.\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\end{array}$$

(24)

This ends our proof. □

Remark 2. 

From Theorem 6, we can obtain some special cases as follows:

(i) 

If $\wp \left(\tau \right)=\tau$, then inequality (13) becomes

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}^v\varpi \right)\left({\vartheta }_2\right)+\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\varpi \right)\left({\vartheta }_1\right)\right]\\ \le \varpi \left({\vartheta }_2\right)\left({}_{\varpi }^v{\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}\aleph \right)\left({\vartheta }_2\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi }{\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\aleph \right)\left({\vartheta }_1\right)\\ \le \left[\frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}\right]\left[\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}^v\varpi \right)\left({\vartheta }_2\right)+\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\varpi \right)\left({\vartheta }_1\right)\right],\end{array}$$

(25)

where $\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}^v\varpi \right)\left({\vartheta }_2\right)$ and $\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\varpi \right)\left({\vartheta }_1\right)$ are defined in Remark 1 part(ii).

(ii) 

Assuming that $\wp \left(\tau \right)=\tau$ and $v=1,$ the inequality (13) simplifies to the inequality (6).

(iii) 

Assuming that $\wp \left(\tau \right)=\tau$ and $\varpi \left(\tau \right)=1,$ the inequality (13) simplifies to the inequality (7).

(iv) 

Assuming that $\wp \left(\tau \right)=\tau ,$$\varpi \left(\tau \right)=1$ and $v=1,$ the inequality (13) simplifies to the inequality (4).

Example 1. 

Let $\aleph \left(\tau \right)={\tau }^2$, $\tau \in \left[\frac{1}{2},2\right]$, and then $\varpi \left(\tau \right)={\left(ln\tau \right)}^2$ and $\wp \left(\tau \right)=2\sqrt{\tau }$; hence, ${\wp }^{\prime }\left(\tau \right)=\frac{1}{\sqrt{\tau }}$ and ${\wp }^{-1}\left(\tau \right)=\frac{{\tau }^2}{4}$.

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left(1\right)}^{{\wp }^{-1}\left(2\right)}{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }+{\int }_{{\wp }^{-1}\left(\frac{1}{2}\right)}^{{\wp }^{-1}\left(1\right)}{\left(ln\left(\sqrt{\tau }\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }\right]\\ =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{\frac{1}{4}}^1{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^2\frac{d\tau }{\tau }+{\int }_{\frac{1}{16}}^{\frac{1}{4}}{\left(ln\left(\sqrt{\tau }\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^2\frac{d\tau }{\tau }\right]\\ \hfill =\frac{2{\left(ln2\right)}^{v+2}}{v\left(v^2+3v+2\right)\Gamma \left(v\right)}+\frac{2^{-v-1}{\left(ln4\right)}^{v+2}}{v\left(v^2+3v+2\right)\Gamma \left(v\right)}=i_1\left(v\right).\end{array}$$

We also observe that

$$\begin{array}{c}\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left(1\right)}^{{\wp }^{-1}\left(2\right)}{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\aleph \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }+{\int }_{{\wp }^{-1}\left(\frac{1}{2}\right)}^{{\wp }^{-1}\left(1\right)}{\left(ln\left(4\sqrt{\tau }\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\aleph \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }\right]\\ =\frac{2}{\Gamma \left(v\right)}\left[{\int }_{\frac{1}{4}}^1{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^{2}d\tau +{\int }_{\frac{1}{16}}^{\frac{1}{4}}{\left(ln\left(4\sqrt{\tau }\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^{2}d\tau \right]\\ =\frac{1}{\Gamma \left(v\right)}\left[2^{-v-4}\left({(-1)}^{1-v}\left({(-ln\left(4\right))}^v\left(\left(v^2+v+vln\left(16\right)+4{ln}^2\left(2\right)\right)E_{1-v}(-ln\left(4\right))+4v+4+ln\left(256\right)\right)\right.\right.\right.\\ \left.-4{ln}^2\left(2\right)\Gamma \left(v\right)-(v+1+ln\left(16\right))\Gamma (v+1)\right)+16((v+1-2ln\left(4\right))\Gamma (v+1)-\Gamma (v+2,ln\left(4\right))\\ \hfill \left.\left.+ln\left(4\right)(2\Gamma (v+1,ln\left(4\right))+ln(4\left)\right(\Gamma \left(v\right)-\Gamma (v,ln(4\left)\right)\left)\right))\right)\right]=i_2\left(v\right),\end{array}$$

and

$$\begin{array}{c}\left[\frac{\aleph \left({\vartheta }_1\right)+\aleph \left({\vartheta }_2\right)}{2}\right]\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ =\frac{17}{8\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left(1\right)}^{{\wp }^{-1}\left(2\right)}{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }+{\int }_{{\wp }^{-1}\left(\frac{1}{2}\right)}^{{\wp }^{-1}\left(1\right)}{\left(ln\left(4\sqrt{\tau }\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }\right]\\ \hfill \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\frac{17{(ln\left(2\right))}^{v+2}}{2v\left(v^2+3v+2\right)\Gamma \left(v\right)}+\frac{17{\left(ln\left(4\right)\right)}^{v+2}}{2^{v+2}\left(2v^3+6v^2+4v\right)\Gamma \left(v\right)}=i_3\left(v\right).\end{array}$$

We observe the validity of Theorem 6.

Figure 1. Plot of the values of the functions obtained from the left-most, middle, and right-most terms in the inequality of Theorem 6.

Figure 1. Plot of the values of the functions obtained from the left-most, middle, and right-most terms in the inequality of Theorem 6.

Consider

$$\begin{array}{cc}\hfill & {\mathbb{D}}_1=\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa .\hfill \\ & {\mathbb{D}}_2=\frac{-1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa .\hfill \end{array}$$

Lemma 2. 

Let $0<{\vartheta }_1<{\vartheta }_2$ and let $\aleph :[{\vartheta }_1,{\vartheta }_2]\subseteq \left(0,\infty \right)\to \mathbb{R}$ be continuous with derivative ${\aleph }^{\prime }\in L[{\vartheta }_1,{\vartheta }_2]$. If $\varpi :[{\vartheta }_1,{\vartheta }_2]\to \mathbb{R}$ is a positive, integrable, and geometrically symmetric weight function with respect to $\sqrt{{\vartheta }_1{\vartheta }_2}$, and if ℘ is an increasing and positive function from $[{\vartheta }_1,{\vartheta }_2)$ onto itself such that its derivative ${\wp }^{\prime }\left(\tau \right)$ is continuous on $({\vartheta }_1,{\vartheta }_2)$, for $v>0,$ then

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ -\left[\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ :={\mathbb{D}}_1+{\mathbb{D}}_2.\hfill \end{array}$$

(26)

Proof. 

Let

$${\mathbb{D}}_1=\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa .$$

By integration by parts, applying Lemma 1 and Definition 3, we obtain

$$\begin{array}{cc}\hfill & ={\left.\frac{1}{\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left(\aleph \circ \wp \right)\left(\kappa \right)\right|}_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\hfill \\ \hfill & -\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\kappa \right)\left(\aleph \circ \wp \right)\left(\kappa \right)\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}d\kappa \hfill \\ & =\left(\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right)\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)-\varpi \left({\vartheta }_1\right)\hfill \\ \hfill & \times \frac{{\left(\varpi \circ \wp \right)}^{-1}{\wp }^{-1}\left({\vartheta }_1\right)}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left(\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\kappa \right)\left(\aleph \circ \wp \right)\left(\kappa \right)\frac{{\wp }^{\prime }\left(\kappa \right)}{{\wp }^{(}\kappa )}d\kappa \hfill \\ \hfill & =\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)-\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\hfill \\ \hfill & =\frac{1}{2}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ \hfill & -\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right).\hfill \end{array}$$

Similarly, we obtain

$$\begin{array}{cc}\hfill & {\mathbb{D}}_2=\frac{-1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \hfill \\ \hfill & ={\left.\frac{-1}{\Gamma \left(v\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(w\circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left(\aleph \circ \wp \right)\left(\kappa \right)\right|}_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\hfill \\ \hfill & -\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\kappa \right)\left(\aleph \circ \wp \right)\left(\kappa \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\kappa \hfill \\ & =\left(\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right)\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\hfill \\ & -\varpi \left({\vartheta }_1\right)\frac{{\left(w\circ \wp \right)}^{-1}{\wp }^{-1}\left({\vartheta }_2\right)}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\kappa \right)\left(\aleph \circ \wp \right)\left(\kappa \right)\frac{{\wp }^{\prime }\left(\kappa \right)}{\wp \left(\kappa \right)}d\kappa \hfill \\ \hfill & =\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)-\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\hfill \end{array}$$

$$\begin{array}{cc}\hfill & =\frac{1}{2}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right]\hfill \\ \hfill & -\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right).\hfill \end{array}$$

As a result, we can conclude,

$$\begin{array}{c}{\mathbb{D}}_1+{\mathbb{D}}_2:=\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right.\hfill \\ \left.+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right]\\ -\left[\varpi \left({\vartheta }_1\right)\left({}_{w\circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right.\\ \hfill \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\left.+\varpi \left({\vartheta }_2\right)\left({}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}{\mathcal{I}}_{\varpi \circ \wp }^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)\right],\end{array}$$

and we achieve the intended result. □

Remark 3.

If $\wp \left(\tau \right)=\tau ,$ then equality (26) becomes

$$\begin{array}{c}\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}^v\varpi \right)\left({\vartheta }_2\right)+\left({\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\varpi \right)\left({\vartheta }_1\right)\right]\hfill \\ -\left[\varpi \left({\vartheta }_2\right)\left({}_{\varpi }{\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{+}}^v\aleph \right)\left({\vartheta }_2\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi }{\mathcal{I}}_{{\sqrt{{\vartheta }_1{\vartheta }_2}}^{-}}^v\aleph \right)\left({\vartheta }_1\right)\right]\\ =\frac{1}{\Gamma \left(v\right)}{\int }_{{\vartheta }_1}^{\sqrt{{\vartheta }_1{\vartheta }_2}}\left[{\int }_{{\vartheta }_1}^{\kappa }{\left(ln\frac{\tau }{{\vartheta }_1}\right)}^{v-1}\varpi \left(\tau \right)\frac{d\tau }{\tau }\right]{\aleph }^{\prime }\left(\kappa \right)d\kappa \\ \hfill -\frac{1}{\Gamma \left(v\right)}{\int }_{\sqrt{{\vartheta }_1{\vartheta }_2}}^{{\vartheta }_2}\left[{\int }_{\kappa }^{{\vartheta }_2}{\left(ln\frac{{\vartheta }_2}{\tau }\right)}^{v-1}\varpi \left(\tau \right)\frac{d\tau }{\tau }\right]{\aleph }^{\prime }\left(\kappa \right)d\kappa .\end{array}$$

We will use the following notations for the rest of this section:

$$\begin{array}{cc}\hfill & {\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)=\aleph \left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right]\hfill \\ & -\left[\varpi \left({\vartheta }_2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_2\right)\right)+\varpi \left({\vartheta }_1\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left({\vartheta }_1\right)\right)\right].\hfill \end{array}$$

Theorem 7.

Consider a scenario in which all of the Lemma 2 criteria are satisfied and $|{\aleph }^{\prime }|$ is geometrically convex on $[{\vartheta }_1,{\vartheta }_2],$ and for $v>0$, we have

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)v\Gamma \left(v\right)}\left[{\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|+{\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right)\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|\right]\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)v\Gamma \left(v\right)}\left[{\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|+{\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right)\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|\right]\\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,{\vartheta }_2\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)v\Gamma \left(v\right)}\left\{\left({\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right)+{\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right)\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|\right.\\ \left.+\left({\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right)+{\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right)\right)\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|\right\},\end{array}$$

(27)

where ${\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right),$${\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right),$${\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right),$ and ${\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right)$ have the following meanings:

$$\begin{array}{cc}\hfill & {\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right)=-{\vartheta }_1\Gamma (v+1){(-1)}^{-v-2}\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}+v+1\right)-{\left(\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)}^{v+1}\hfill \\ & \times \left[\frac{1}{2}{\vartheta }_1{\vartheta }_2+{\vartheta }_1{E}_{-v}\left(\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}+v+1\right)\right],\hfill \\ \hfill & {\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right)={\vartheta }_1{\left(-1\right)}^{-v-2}\Gamma (v+2)-2^{-v-2}{\vartheta }_1{\left(ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{v+2}{E}_{-v-1}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right),\hfill \\ \hfill & {\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right)={\vartheta }_2\left(\Gamma (v+2)-{\left(ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{v+2}{E}_{-v-1}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)\right),\hfill \\ \hfill & {\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right)=-{\vartheta }_2\Gamma (v+1)\left(ln\frac{{\vartheta }_1}{{\vartheta }_2}+v+1\right)+{\left({\displaystyle \frac{1}{2}}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)}^{v+1}\left[\frac{1}{2}{\vartheta }_1{\vartheta }_2+{\vartheta }_2{E}_{-v}\left(\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\left(ln\frac{{\vartheta }_1}{{\vartheta }_2}+v+1\right)\right].\hfill \end{array}$$

Proof. 

We obtain the following result by applying Lemma 2 as well as the characteristics of the modulus and the geometrically convex function of $|{\aleph }^{\prime }|$

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|=\left|\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right.\hfill \\ \left.-\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right|\\ \le \frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|{\wp }^{\prime }\left(\kappa \right)d\kappa \\ \hfill +\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|{\wp }^{\prime }\left(\kappa \right)d\kappa .\end{array}$$

Since $\left|{\aleph }^{{}^{\prime }}\right|$ is a geometrically convex function on $\left[{\vartheta }_1,{\vartheta }_2\right],$ we have

$$\begin{array}{c}\left|({\aleph }^{\prime }\circ \wp )\left(\kappa \right)\right|\le \left|{\aleph }^{\prime }\left({\vartheta }_1^{\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}}{\vartheta }_2^{\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln{\vartheta }_2-ln{\vartheta }_1}}\right)\right|\hfill \\ \hfill \le \left(\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right)\left|{\aleph }^{{}^{\prime }}\left({\vartheta }_1\right)\right|+\left(\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln\left({\vartheta }_2\right)-ln{\vartheta }_1}\right)\left|{\aleph }^{{}^{\prime }}\left({\vartheta }_2\right)\right|.\end{array}$$

Consequently, we obtain

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|\hfill \\ \times \left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|\right]{\wp }^{\prime }\left(\kappa \right)d\kappa \\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|\\ \hfill \times \left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|\right]{\wp }^{\prime }\left(\kappa \right)d\kappa ,\end{array}$$

where

$${\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau =\frac{1}{v}{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^v$$

and

$${\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau =\frac{1}{v}{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^v.$$

Using the computations shown above, we can derive the following integral:

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)v\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^v\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|\right.\hfill \\ \left.+{\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)}^{v+1}\left|\left({\aleph }^{{}^{\prime }}\right)\left({\vartheta }_2\right)\right|\right]{\wp }^{\prime }\left(\kappa \right)d\kappa +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)v\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\hfill \\ \times \left[{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^{v+1}\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|+{\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right)}^v\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right)\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|\right]{\wp }^{\prime }\left(\kappa \right)d\kappa .\hfill \end{array}$$

(28)

We can derive the required result (27) by performing fundamental integral computations based on the inequality (28). □

Example 2.

Let $\aleph \left(\tau \right)={\tau }^2$, $\tau \in \left[\frac{1}{2},2\right]$, and then $\varpi \left(\tau \right)={\left(ln\tau \right)}^2$ and $\wp \left(\tau \right)=2\sqrt{\tau }$; hence, ${\wp }^{\prime }\left(\tau \right)=\frac{1}{\sqrt{\tau }}$ and ${\wp }^{-1}\left(\tau \right)=\frac{{\tau }^2}{4}$.

$$\begin{array}{c}\aleph \left(1\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\hfill \\ -\left[\varpi \left(2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\varpi \left(\frac{1}{2}\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\hfill \\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[\frac{1}{2},2\right],\infty }}{ln\left(4\right)\Gamma (v+1)}\left\{\left({\mathbb{W}}_1\left(\frac{1}{2},2\right)+{\mathbb{W}}_3\left(\frac{1}{2},2\right)\right)\left|{\aleph }^{\prime }\left(\frac{1}{2}\right)\right|+\left({\mathbb{W}}_2\left(\frac{1}{2},2\right)+{\mathbb{W}}_4\left(\frac{1}{2},2\right)\right)\left|{\aleph }^{\prime }\left(2\right)\right|\right\}.\hfill \end{array}$$

(29)

We also show that

$$\begin{array}{c}\aleph \left(1\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\hfill \\ =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left(1\right)}^{{\wp }^{-1}\left(2\right)}{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }+{\int }_{{\wp }^{-1}\left(\frac{1}{2}\right)}^{{\wp }^{-1}\left(1\right)}{\left(ln\left(\sqrt{\tau }\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }\right]\\ =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{\frac{1}{4}}^1{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^2\frac{d\tau }{\tau }+{\int }_{\frac{1}{16}}^{\frac{1}{4}}{\left(ln\left(\sqrt{\tau }\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^2\frac{d\tau }{\tau }\right]\\ \hfill =\frac{2{\left(ln2\right)}^{v+2}}{v\left(v^2+3v+2\right)\Gamma \left(v\right)}+\frac{2^{-v-1}{\left(ln4\right)}^{v+2}}{v\left(v^2+3v+2\right)\Gamma \left(v\right)},\end{array}$$

and

$$\begin{array}{cc}\hfill & \varpi \left(2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\varpi \left(\frac{1}{2}\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\hfill \\ & =\frac{1}{2\Gamma \left(v\right)}\left[{\int }_{{\wp }^{-1}\left(1\right)}^{{\wp }^{-1}\left(2\right)}{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\aleph \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }+{\int }_{{\wp }^{-1}\left(\frac{1}{2}\right)}^{{\wp }^{-1}\left(1\right)}{\left(ln\left(4\sqrt{\tau }\right)\right)}^{v-1}\varpi \left(2\sqrt{\tau }\right)\aleph \left(2\sqrt{\tau }\right)\frac{d\tau }{\tau }\right]\hfill \\ & =\frac{2}{\Gamma \left(v\right)}\left[{\int }_{\frac{1}{4}}^1{\left(ln\left(\frac{1}{\sqrt{\tau }}\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^{2}d\tau +{\int }_{\frac{1}{16}}^{\frac{1}{4}}{\left(ln\left(4\sqrt{\tau }\right)\right)}^{v-1}{\left(ln\left(2\sqrt{\tau }\right)\right)}^{2}d\tau \right]\hfill \\ & =\frac{1}{\Gamma \left(v\right)}\left[2^{-v-4}\left({(-1)}^{1-v}\left({(-ln\left(4\right))}^v\left(\left(v^2+v+vln\left(16\right)+4{ln}^2\left(2\right)\right)E_{1-v}(-ln\left(4\right))+4v+4+ln\left(256\right)\right)\right.\right.\right.\hfill \\ & \left.-4{ln}^2\left(2\right)\Gamma \left(v\right)-(v+1+ln\left(16\right))\Gamma (v+1)\right)+16((v+1-2ln\left(4\right))\Gamma (v+1)-\Gamma (v+2,ln\left(4\right))\hfill \\ & \left.\left.+ln\left(4\right)(2\Gamma (v+1,ln\left(4\right))+ln(4\left)\right(\Gamma \left(v\right)-\Gamma (v,ln(4\left)\right)\left)\right))\right)\right],\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill & {\mathbb{W}}_1\left(\frac{1}{2},2\right)=\frac{1}{2}\left(-2{(ln\left(2\right))}^{v+1}-{(-1)}^{-v}(v+1+ln\left(4\right))(\Gamma (v+1)-\Gamma (v+1,-ln\left(2\right)))\right),\hfill \\ & {\mathbb{W}}_2\left(\frac{1}{2},2\right)=\frac{1}{2}{(-1)}^{-v}\left(2{(-1)}^v{(ln\left(2\right))}^{v+1}+\Gamma (v+2)-(v+1)\Gamma (v+1,-ln\left(2\right))\right),\hfill \\ & {\mathbb{W}}_3\left(\frac{1}{2},2\right)=2^{-v-1}\left(2^{v+2}(v+1)(\Gamma (v+1)-\Gamma (v+1,ln\left(2\right)))-{ln}^{v+1}\left(4\right)\right),\hfill \\ & {\mathbb{W}}_4\left(\frac{1}{2},2\right)=-2(v+1-ln\left(4\right))\Gamma (v+1)+2\Gamma (v+2,ln\left(2\right))-4ln\left(2\right)\Gamma (v+1,ln\left(2\right)),\hfill \\ \hfill & {\aleph }^{{}^{\prime }}\left(\frac{1}{2}\right)=1,\hfill \\ \hfill & {\aleph }^{{}^{\prime }}\left(2\right)=4,\hfill \\ \hfill & \aleph \left(1\right)=1.\hfill \end{array}$$

We observe the validity of Theorem 7 by using all the above calculations in (29).

Figure 2. A graph of the error and error bound in the inequality of Theorem 7.

Figure 2. A graph of the error and error bound in the inequality of Theorem 7.

Theorem 8.

Consider a scenario in which all of the Lemma 2 criteria are fulfilled and $|{\aleph }^{\prime }{|}^q$ is geometrically convex on $[{\vartheta }_1,{\vartheta }_2]$ with $q\ge 1$, and the following inequality holds for $v>0$:

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}\left[{\left({\mathbb{K}}_1\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right]\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_1\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}\left[{\left({\mathbb{K}}_2\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right]\\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}\left\{{\left({\mathbb{K}}_1\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right.\\ +\left.{\left({\mathbb{K}}_2\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right\},\end{array}$$

(30)

where

$$\begin{array}{cc}\hfill & {\mathbb{K}}_1\left({\vartheta }_1,{\vartheta }_2\right)=={\vartheta }_1\left(-v{(-1)}^{-v}\Gamma \left(v\right)+\Gamma \left(1+v,\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right){\left(ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^v{\left(ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)}^{-v}\hfill \\ & {\mathbb{K}}_2\left({\vartheta }_1,{\vartheta }_2\right)={\vartheta }_2\left({(-1)}^{-v}\Gamma (1+v)-\Gamma \left(1+v,\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right),\hfill \end{array}$$

and ${\mathbb{W}}_1\left({\vartheta }_1,{\vartheta }_2\right),$${\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right),$${\mathbb{W}}_3\left({\vartheta }_1,{\vartheta }_2\right),$ and ${\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right)$ are defined in Theorem 7.

Proof. 

Using Lemma 2 as well as the properties of power mean inequality and the geometrically convex function of $|{\aleph }^{\prime }{|}^q$, we obtain

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|=\left|\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right.\hfill \\ \left.-\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right|\\ \le \frac{1}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{1-\frac{1}{q}}\\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{1}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{1-\frac{1}{q}}\\ \hfill \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}.\end{array}$$

Since ${\left|{\aleph }^{\prime }\right|}^q$ is a geometrically convex function on $\left[{\vartheta }_1,{\vartheta }_2\right]$, we have

$$\begin{array}{c}\left|({\aleph }^{\prime }\circ \wp )\left(\kappa \right)\right|\le {\left|{\aleph }^{\prime }\left({\vartheta }_1^{\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}}{\vartheta }_2^{\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln{\vartheta }_2-ln{\vartheta }_1}}\right)\right|}^q\hfill \\ \hfill \le \left(\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln\left({\vartheta }_2\right)-ln{\vartheta }_1}\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q.\end{array}$$

As a result, we have

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{1-\frac{1}{q}}\hfill \\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{1-\frac{1}{q}}\\ \hfill \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}},\end{array}$$

where it is obvious that

$$\begin{array}{c}{\mathbb{K}}_1\left({\vartheta }_1,{\vartheta }_2\right)={\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \hfill \\ \hfill ={\vartheta }_1\left(-v{(-1)}^{-v}\Gamma \left(v\right)+\Gamma \left(1+v,\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right){\left(ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^v{\left(ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)}^{-v}\end{array}$$

$$\begin{array}{c}{\mathbb{K}}_2\left({\vartheta }_1,{\vartheta }_2\right)={\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\wp }^{\prime }\left(\kappa \right)d\kappa \hfill \\ \hfill ={\vartheta }_2\left({(-1)}^{-v}\Gamma (1+v)-\Gamma \left(1+v,\frac{1}{2}ln\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right).\end{array}$$

By using calculations, we obtain the following inequality:

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\mathbb{K}}_1\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}\\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\mathbb{K}}_2\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{1-\frac{1}{q}}\\ \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}.\end{array}$$

(31)

We can obtain the necessary result (30) by performing basic integral calculations based on inequality (31). □

Example 3.

Let $\aleph \left(\tau \right)={\tau }^3$, $\tau \in \left[\frac{1}{2},2\right]$, then $\varpi \left(\tau \right)={\left(ln\tau \right)}^2$ and $\wp \left(\tau \right)=2\sqrt{\tau }$; hence ${\wp }^{\prime }\left(\tau \right)=\frac{1}{\sqrt{\tau }}$ and ${\wp }^{-1}\left(\tau \right)=\frac{{\tau }^2}{4}$.

$$\begin{array}{c}\aleph \left(1\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\hfill \\ -\left[\varpi \left(2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\varpi \left(\frac{1}{2}\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[\frac{1}{2},2\right],\infty }}{{\left(ln\left(4\right)\right)}^{\frac{1}{q}}{v}^{\frac{1}{q}}\Gamma \left(v\right)}\left\{{\left({\mathbb{K}}_1\left(\frac{1}{2},2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_1\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(\frac{1}{2}\right)\right|}^q+{\mathbb{W}}_2\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left(2\right)\right|}^q\right)}^{\frac{1}{q}}\right.\\ +\left.{\left({\mathbb{K}}_2\left(\frac{1}{2},2\right)\right)}^{1-\frac{1}{q}}{\left({\mathbb{W}}_3\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(\frac{1}{2}\right)\right|}^q+{\mathbb{W}}_4\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left(2\right)\right|}^q\right)}^{\frac{1}{q}}\right\}\\ =\frac{1}{32\Gamma \left(v\right)}\left[\frac{64{ln}^{v+2}\left(2\right)}{v\left(v^2+3v+2\right)}+\frac{2^{5-v}{ln}^{v+2}\left(4\right)}{v^3+3v^2+2v}-3^{-v-2}\left({(-1)}^{-v}\left({ln}^2\left(64\right)\Gamma \left(v\right)+4(v+1+ln\left(64\right))\Gamma (v+1)\right.\right.\right.\\ \left.-{ln}^2\left(64\right)\Gamma (v,-ln\left(8\right))-4\Gamma (v+2,-ln\left(8\right))-12ln\left(4\right)\Gamma (v+1,-ln\left(8\right))\right)+64(4(v+1-3ln\left(4\right))\\ \left.\left.\Gamma (v+1)-4\Gamma (v+2,ln(8\left)\right)+3ln\left(4\right)(ln(64)\Gamma (v)+4\Gamma (v+1,ln\left(8\right))-3ln(4)\Gamma (v,ln\left(8\right)\left)\right))\right)\right]\\ \le \frac{{ln}^2\left(2\right)}{\Gamma \left(v\right){\left(ln\left(4\right)\right)}^{\frac{1}{q}}}\left[{\left(\frac{{(-1)}^{-v}(\Gamma (v+1,-ln\left(2\right))-\Gamma (v+1))}{2v}\right)}^{\frac{q-1}{q}}\left(\frac{{(-1)}^{-v}{2}^{-2q-1}{3}^q(ln\left(4\right)}{v}\right.\right.\\ {\left.\frac{(\Gamma (v+1,-ln\left(2\right))-\Gamma (v+1)))+\left({16}^q-1\right)\Gamma (v+2)-\left({16}^q-1\right)\Gamma (v+2,-ln\left(2\right))}{v}\right)}^{\frac{1}{q}}\\ +{\left(\frac{2(\Gamma (v+1)-\Gamma (v+1,ln\left(2\right)\left)\right)}{v}\right)}^{\frac{q-1}{q}}\left(-\frac{2^{1-2q}{3}^q(v\Gamma \left(v\right)\left(v\left({16}^q-1\right)+{16}^q-{16}^{q}ln\left(4\right)-1\right)}{v}\right.\\ \hfill -\left.{\left.\frac{\left({16}^q-1\right)\Gamma (v+2,ln\left(2\right))+{16}^{q}ln\left(4\right)\Gamma (v+1,ln\left(2\right)))}{v}\right)}^{\frac{1}{q}}\right].\end{array}$$

We observe the validity of Theorem 8.

Figure 3. A graph of the error and error bound in the inequality of Theorem 8.

Figure 3. A graph of the error and error bound in the inequality of Theorem 8.

Theorem 9.

Consider a scenario in which all of the Lemma 2 criteria are met and $|{\aleph }^{\prime }{|}^q$ is geometrically convex on $[{\vartheta }_1,{\vartheta }_2]$ with $q>1$, and then we obtain the following inequality for $v>0$:

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}\left[{\left({\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_1\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{X}}_2\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right]\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}\left[{\left({\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_3\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{X}}_4\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right]\\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}\left\{{\left({\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_1\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{X}}_2\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right.\\ +\left.{\left({\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_3\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+{\mathbb{X}}_4\left({\vartheta }_1,{\vartheta }_2\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q\right)}^{\frac{1}{q}}\right\},\end{array}$$

(32)

where ${\mathbb{X}}_1\left({\vartheta }_1,{\vartheta }_2\right),$ ${\mathbb{X}}_2\left({\vartheta }_1,{\vartheta }_2\right),$ ${\mathbb{X}}_3\left({\vartheta }_1,{\vartheta }_2\right),$ ${\mathbb{X}}_4\left({\vartheta }_1,{\vartheta }_2\right),$ ${\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)$ and ${\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)$ are defined as follows:

$$\begin{array}{cc}\hfill & {\mathbb{X}}_1\left({\vartheta }_1,{\vartheta }_2\right)=\frac{2{\vartheta }_1\left(-1+ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)+\sqrt{{\vartheta }_1{\vartheta }_2}\left(2+ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}{2},\hfill \\ \hfill & {\mathbb{X}}_2\left({\vartheta }_1,{\vartheta }_2\right)=\frac{2{\vartheta }_1+\sqrt{{\vartheta }_1{\vartheta }_2}\left(-2+ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)}{2},\hfill \\ \hfill & {\mathbb{X}}_3\left({\vartheta }_1,{\vartheta }_2\right)=\frac{2{\vartheta }_2+\sqrt{{\vartheta }_1{\vartheta }_2}\left(-2+ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}{2},\hfill \\ \hfill & {\mathbb{X}}_4\left({\vartheta }_1,{\vartheta }_2\right)=\frac{2{\vartheta }_2\left(-1+ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)+\sqrt{{\vartheta }_1{\vartheta }_2}\left(2+ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)}{2},\hfill \\ & {\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)={\vartheta }_1{v}^{-p}\left[-{\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{vp-1}{E}_{-vp}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)+{\left(-1\right)}^{-vp-1}\Gamma \left(vp+1\right)\right],\hfill \\ & {\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)={\vartheta }_2{v}^{-p}\left[-{\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{vp-1}{E}_{-vp}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)+{\left(-1\right)}^{-vp-1}\Gamma \left(vp+1\right)\right].\hfill \end{array}$$

Proof. 

Using Lemma 2 as well as the properties of Hölder inequality and the geometrically convex function of $|{\aleph }^{\prime }{|}^q$, we obtain

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|=\left|\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right.\hfill \\ \left.-\frac{1}{\Gamma \left(v\right)}{\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right]\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right){\wp }^{\prime }\left(\kappa \right)d\kappa \right|\\ \le \frac{1}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{p}}\\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{1}{\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\left(\varpi \circ \wp \right)\left(\tau \right)\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{p}}\\ \hfill \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left|\left({\aleph }^{\prime }\circ \wp \right)\left(\kappa \right)\right|}^q{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}.\end{array}$$

Since ${\left|{\aleph }^{\prime }\right|}^q$ is a geometrically convex function on $\left[{\vartheta }_1,{\vartheta }_2\right]$, we have

$$\begin{array}{c}\left|({\aleph }^{\prime }\circ \wp )\left(\kappa \right)\right|\le {\left|{\aleph }^{\prime }\left({\vartheta }_1^{\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}}{\vartheta }_2^{\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln{\vartheta }_2-ln{\vartheta }_1}}\right)\right|}^q\hfill \\ \hfill \le \left(\frac{ln{\vartheta }_2-ln\wp \left(\kappa \right)}{ln{\vartheta }_2-ln{\vartheta }_1}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(\frac{ln\wp \left(\kappa \right)-ln{\vartheta }_1}{ln\left({\vartheta }_2\right)-ln{\vartheta }_1}\right){\left|{\aleph }^{\prime }\left({\vartheta }_2\right)\right|}^q.\end{array}$$

As a result, we obtain

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{p}}\hfill \\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right){\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|}^q\right]{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{p}}\\ \hfill \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right){\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|}^q\right]{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}.\end{array}$$

where it is obvious that

$$\begin{array}{c}{\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)={\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}{\left|{\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{\kappa }{\left(ln\frac{\wp \left(\tau \right)}{{\vartheta }_1}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \hfill \\ \hfill ={\vartheta }_1{v}^{-p}\left[-{\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{vp-1}{E}_{-vp}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_1}{{\vartheta }_2}\right)\right)+{\left(-1\right)}^{-vp-1}\Gamma \left(vp+1\right)\right],\end{array}$$

and

$$\begin{array}{c}{\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)={\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left|{\int }_{\kappa }^{{\wp }^{-1}\left({\vartheta }_2\right)}{\left(ln\frac{{\vartheta }_2}{\wp \left(\tau \right)}\right)}^{v-1}\frac{{\wp }^{\prime }\left(\tau \right)}{\wp \left(\tau \right)}d\tau \right|}^p{\wp }^{\prime }\left(\kappa \right)d\kappa \hfill \\ \hfill ={\vartheta }_2{v}^{-p}\left[-{\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)}^{vp-1}{E}_{-vp}\left(\frac{1}{2}ln\left(\frac{{\vartheta }_2}{{\vartheta }_1}\right)\right)+{\left(-1\right)}^{-vp-1}\Gamma \left(vp+1\right)\right].\end{array}$$

By using calculations, we obtain the following inequality:

$$\begin{array}{c}\left|{\Xi }^{v,\wp }({\vartheta }_1,{\vartheta }_2)\right|\le \frac{{\left|\right|\varpi \left|\right|}_{\left[{\vartheta }_1,\sqrt{{\vartheta }_1{\vartheta }_2}\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\mathbb{H}}_1^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}\hfill \\ \times {\left({\int }_{{\wp }^{-1}\left({\vartheta }_1\right)}^{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}\left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right){\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|}^q\right]{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}\\ +\frac{{\left|\right|\varpi \left|\right|}_{\left[\sqrt{{\vartheta }_1{\vartheta }_2},{\vartheta }_2\right],\infty }}{{\left(ln\frac{{\vartheta }_2}{{\vartheta }_1}\right)}^{\frac{1}{q}}\Gamma \left(v\right)}{\left({\mathbb{H}}_2^{v,p}\left({\vartheta }_1,{\vartheta }_2\right)\right)}^{\frac{1}{p}}\\ \hfill \times {\left({\int }_{{\wp }^{-1}\left(\sqrt{{\vartheta }_1{\vartheta }_2}\right)}^{{\wp }^{-1}\left({\vartheta }_2\right)}\left[\left(ln\frac{{\vartheta }_2}{\wp \left(\kappa \right)}\right){\left|{\aleph }^{\prime }\left({\vartheta }_1\right)\right|}^q+\left(ln\frac{\wp \left(\kappa \right)}{{\vartheta }_1}\right){\left|\aleph {}^{\prime }\left({\vartheta }_2\right)\right|}^q\right]{\wp }^{\prime }\left(\kappa \right)d\kappa \right)}^{\frac{1}{q}}.\end{array}$$

(33)

In order to achieve the required result (32), we must first perform some fundamental integral calculations on the basis of the inequality (33). □

Example 4.

Let $\aleph \left(\tau \right)={\tau }^2$, $\tau \in \left[\frac{1}{2},2\right]$, and then $\varpi \left(\tau \right)={\left(ln\tau \right)}^2$ and $\wp \left(\tau \right)=2\sqrt{\tau }$; hence, ${\wp }^{\prime }\left(\tau \right)=\frac{1}{\sqrt{\tau }}$ and ${\wp }^{-1}\left(\tau \right)=\frac{{\tau }^2}{4}$.

$$\begin{array}{c}\aleph \left(1\right)\left[\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\left({\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\varpi \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\\ -\left[\varpi \left(2\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{+}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(2\right)\right)+\varpi \left(\frac{1}{2}\right)\left({}_{\varpi \circ \wp }{\mathcal{I}}_{{\wp }^{-1}{\left(1\right)}^{-}}^{v,\wp }\left(\aleph \circ \wp \right)\right)\left({\wp }^{-1}\left(\frac{1}{2}\right)\right)\right]\\ \le \frac{{\left|\right|\varpi \left|\right|}_{\left[\frac{1}{2},2\right],\infty }}{{\left(ln\left(4\right)\right)}^{\frac{1}{q}}\Gamma \left(v\right)}\left\{{\left({\mathbb{H}}_1^{v,p}\left(\frac{1}{2},2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_1\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(\frac{1}{2}\right)\right|}^q+{\mathbb{X}}_2\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(2\right)\right|}^q\right)}^{\frac{1}{q}}\right.\\ +\left.{\left({\mathbb{H}}_2^{v,p}\left(\frac{1}{2},2\right)\right)}^{\frac{1}{p}}{\left({\mathbb{X}}_3\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(\frac{1}{2}\right)\right|}^q+{\mathbb{X}}_4\left(\frac{1}{2},2\right){\left|{\aleph }^{\prime }\left(2\right)\right|}^q\right)}^{\frac{1}{q}}\right\}.\end{array}$$

(34)

We observe the validity of Theorem 9.

Figure 4. A graph of the error and error bound in the inequality of Theorem 9.

Figure 4. A graph of the error and error bound in the inequality of Theorem 9.

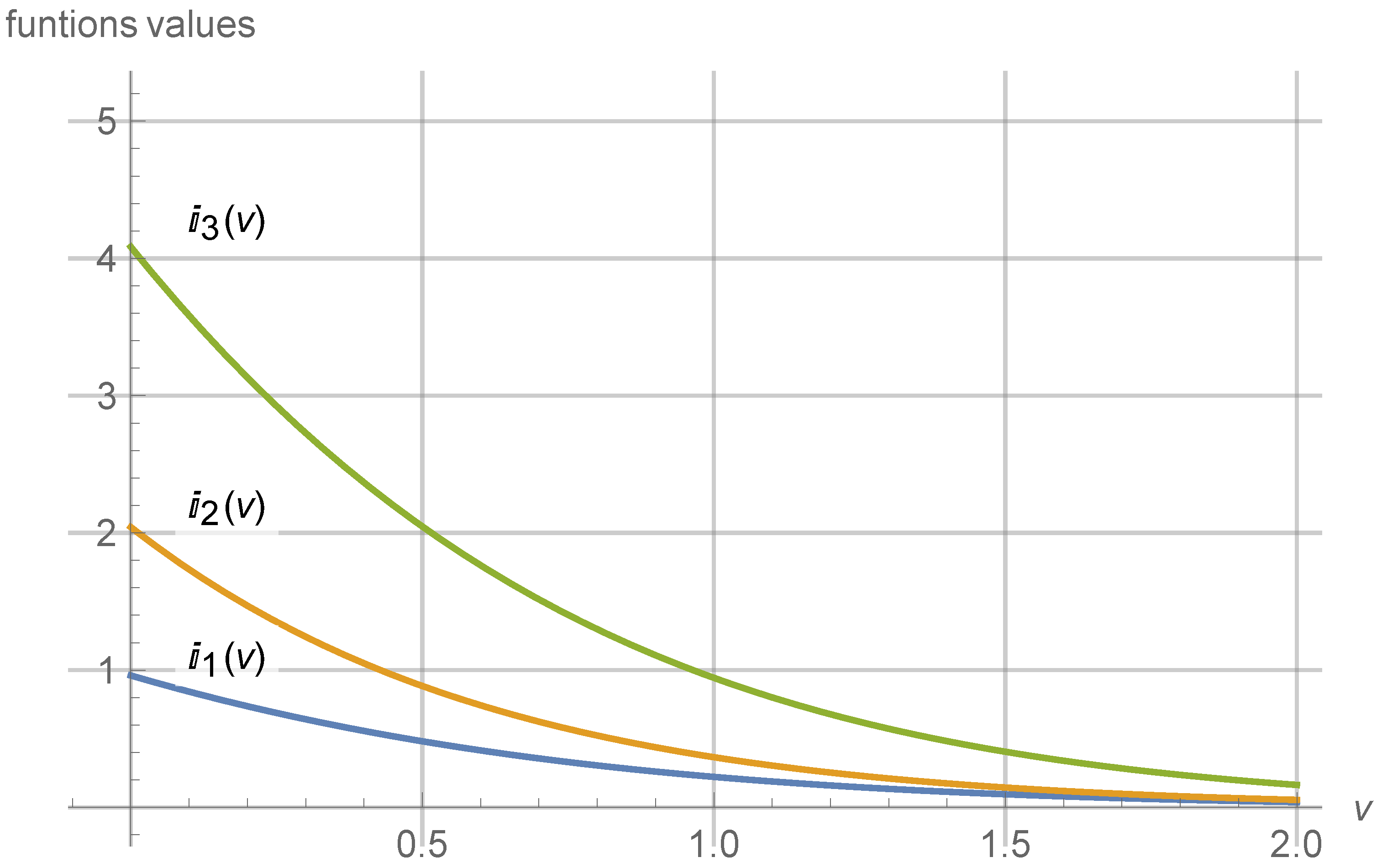
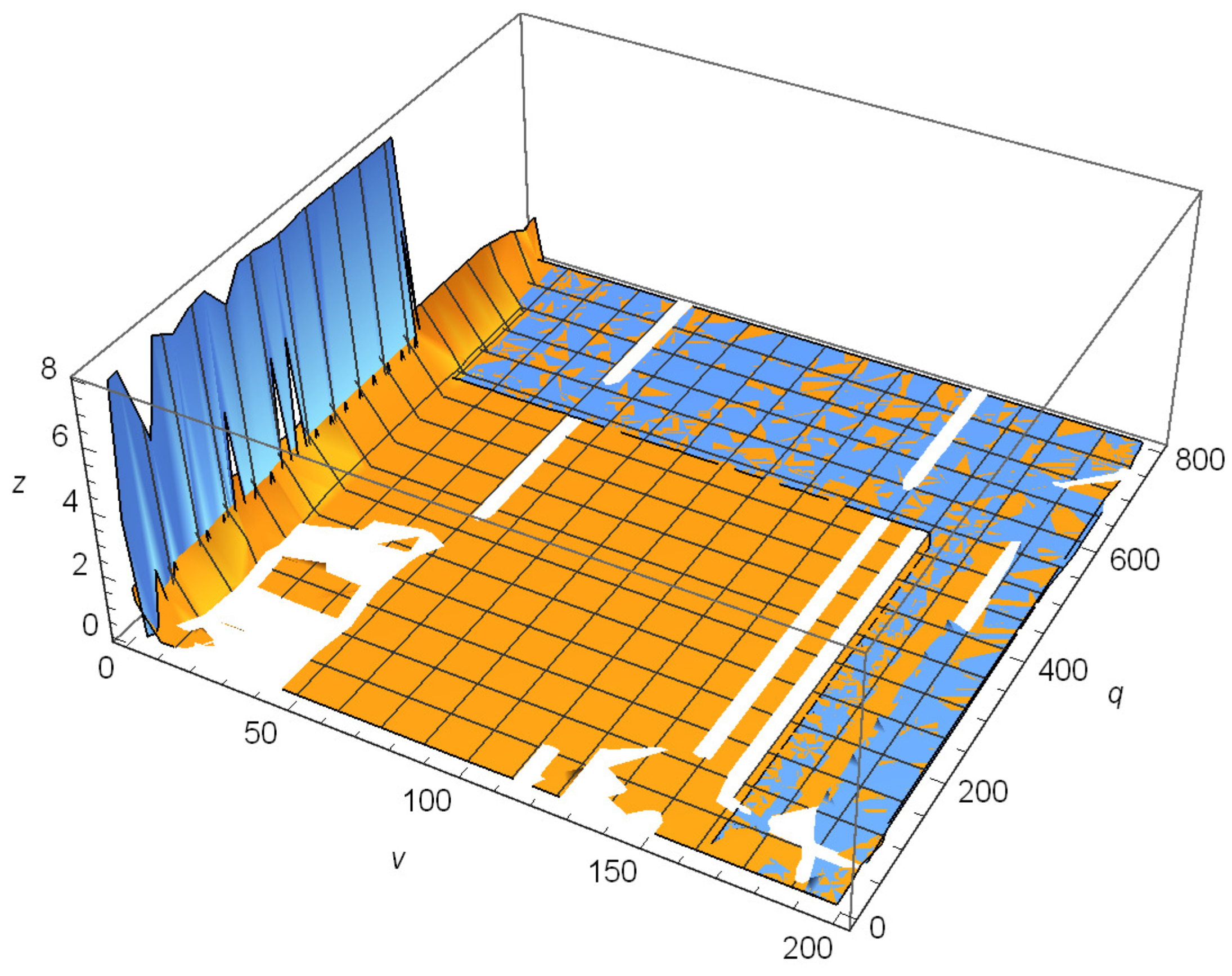
5. Conclusions

In this study, we show highly important and intriguing weighted-type inequalities for a very interesting generalized class of functions, namely geometrically convex functions, using a basic weighted fractional integral operator that depends on an expanding function. Our findings not only generalize a number of findings presented in [17,18,19], but they also enable the production of a number of new results by altering the rising function involved. As a result, both young researchers and those currently working in the field of fractional integral inequality can benefit from the findings, which will open up new areas of investigation in the mathematical sciences.