Additional Solar System Gravitational Anomalies
Abstract
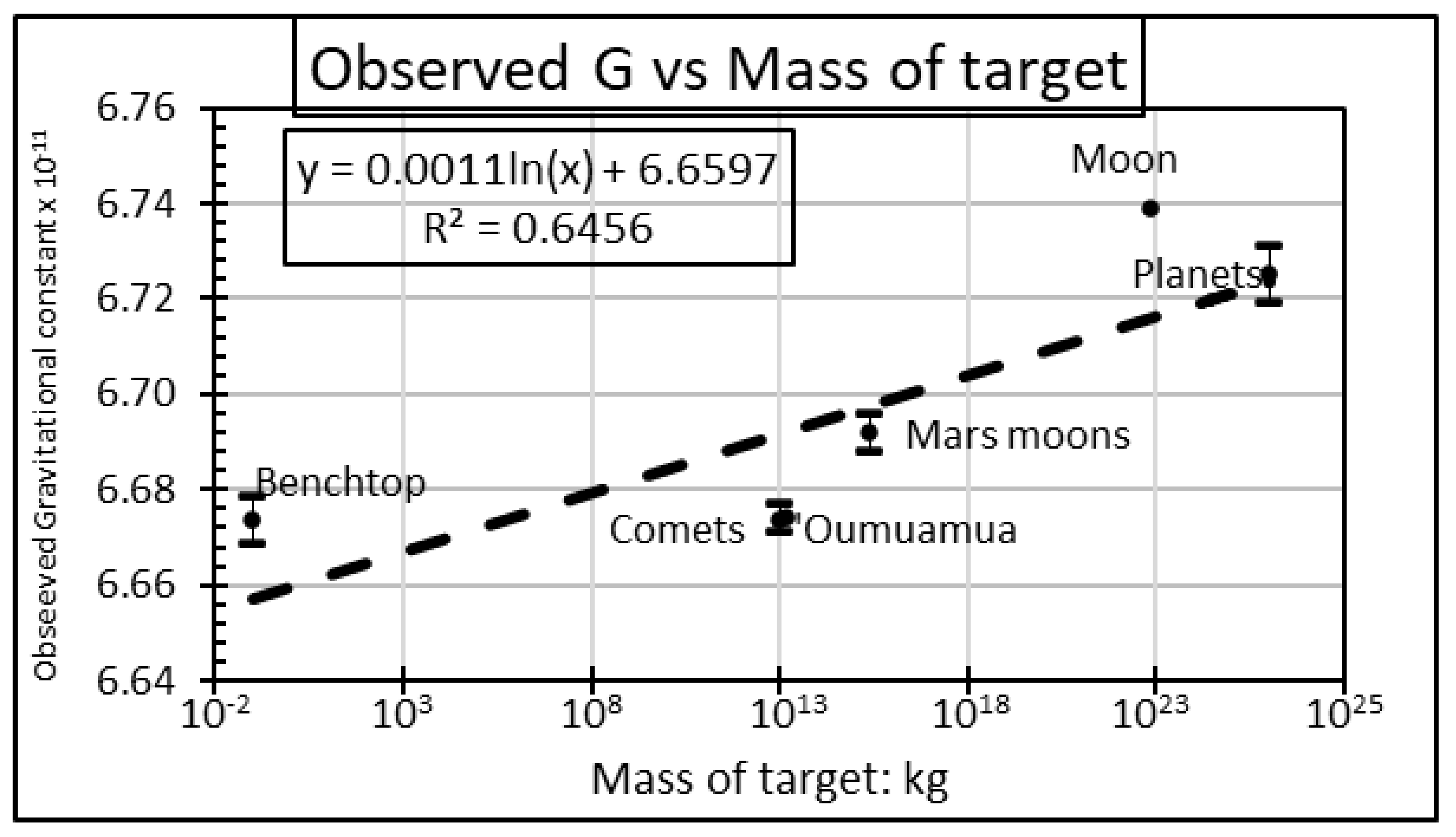
1. Introduction
- laboratory determinations of G by 13 teams using target masses up to 5 kg;
- orbital dynamics of the planets and of the moons of Earth and Mars, which assume Keplerian motion;
- non-gravitational acceleration (NGA) of ‘Oumuamua and 70 comets.
2. Solar System Gravitational Anomalies
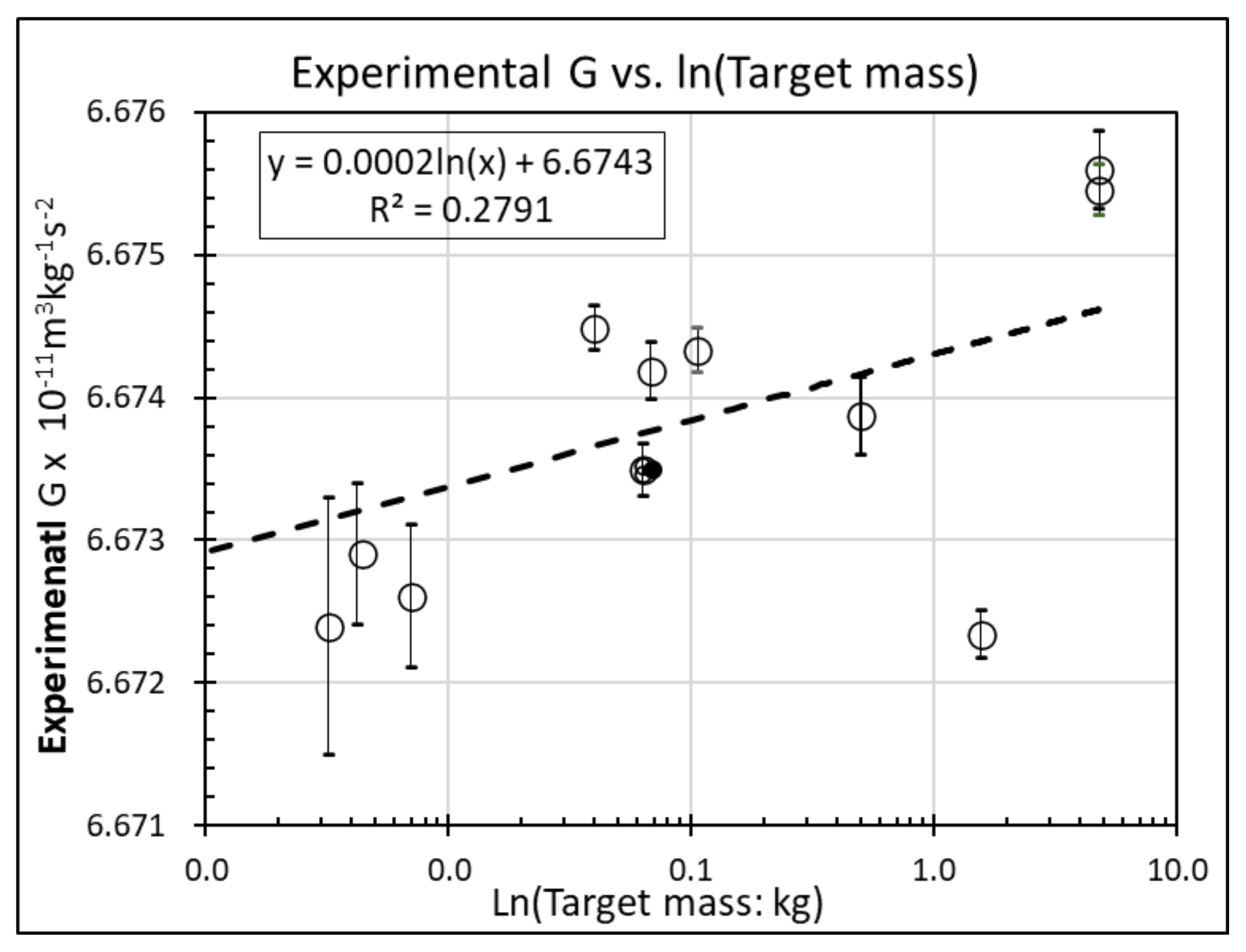
2.1. Experimental Determinations of G
| Panel A: Details of Published Studies | |||||||
| Reference | Apparatus | G (×10−11 m3⋅kg−1⋅s−2) | Attractor Mass (kg) | Target Mass (kg) | |||
| [20] | Torsion balance | 6.67387 ± 0.00027 | 54.00 | 0.5000 | |||
| [21] | Torsion pendulum, time of swing | 6.67400 ± 0.0007 | 20.98 | 0.0007 | |||
| [22] | Torsion pendulum, time of swing | 6.67239 ± 0.0009 | 12.50 | 0.0032 | |||
| [23] | Torsion balance | 6.6729 ± 0.0005 | 8.00 | 0.0044 | |||
| [24] | Torsion pendulum, time of swing | 6.674184 ± 0.000078 | 1.56 | 0.0680 | |||
| [25] | Angular acceleration feedback | 6.674484 ± 0.0000078 | 34.16 | 0.0400 | |||
| [26] | Torsion pendulum, time of swing | 6.67349 ± 0.000026 | 1.56 | 0.0630 | |||
| [26] | Torsion pendulum, time of swing | 6.6726 ± 0.0005 | 20.98 | 0.0070 | |||
| [27] | Torsion pendulum, time of swing | 6.67433 ± 0.00013 | 117.42 | 0.1060 | |||
| [28] | Laser interferometer | 6.67234 ± 0.00014 | 480.00 | 1.5600 | |||
| [29] | Torsion balance | 6.67545 ± 0.00018 | 44.00 | 4.8000 | |||
| [30] | Torsion balance | 6.67559 ± 0.00027 | 48.00 | 4.8000 | |||
| [31] | Torsion pendulum, time of swing | 6.67349 ± 0.00018 | 1.58 | 0.0700 | |||
| Panel B: Statistics for OLS Regressions Using Target Mass as the Independent Variable | |||||||
| Intercept | Slope | Adj R2 | |||||
| Value | Standard Error | p-Value | Value | Standard Error | p-Value | ||
| OLS Regression | 6.6743 | 0.00027 | <0.0001 | 0.000204 | 0.000137 | 0.016 | 0.279 |
| Bootstrapped standard errors (100,000 repetitions) | 6.6744 | 0.00031 | <0.0001 | 0.000202 | 0.000141 | 0.090 | 0.278 |
2.2. Planets’ Orbital Dynamics
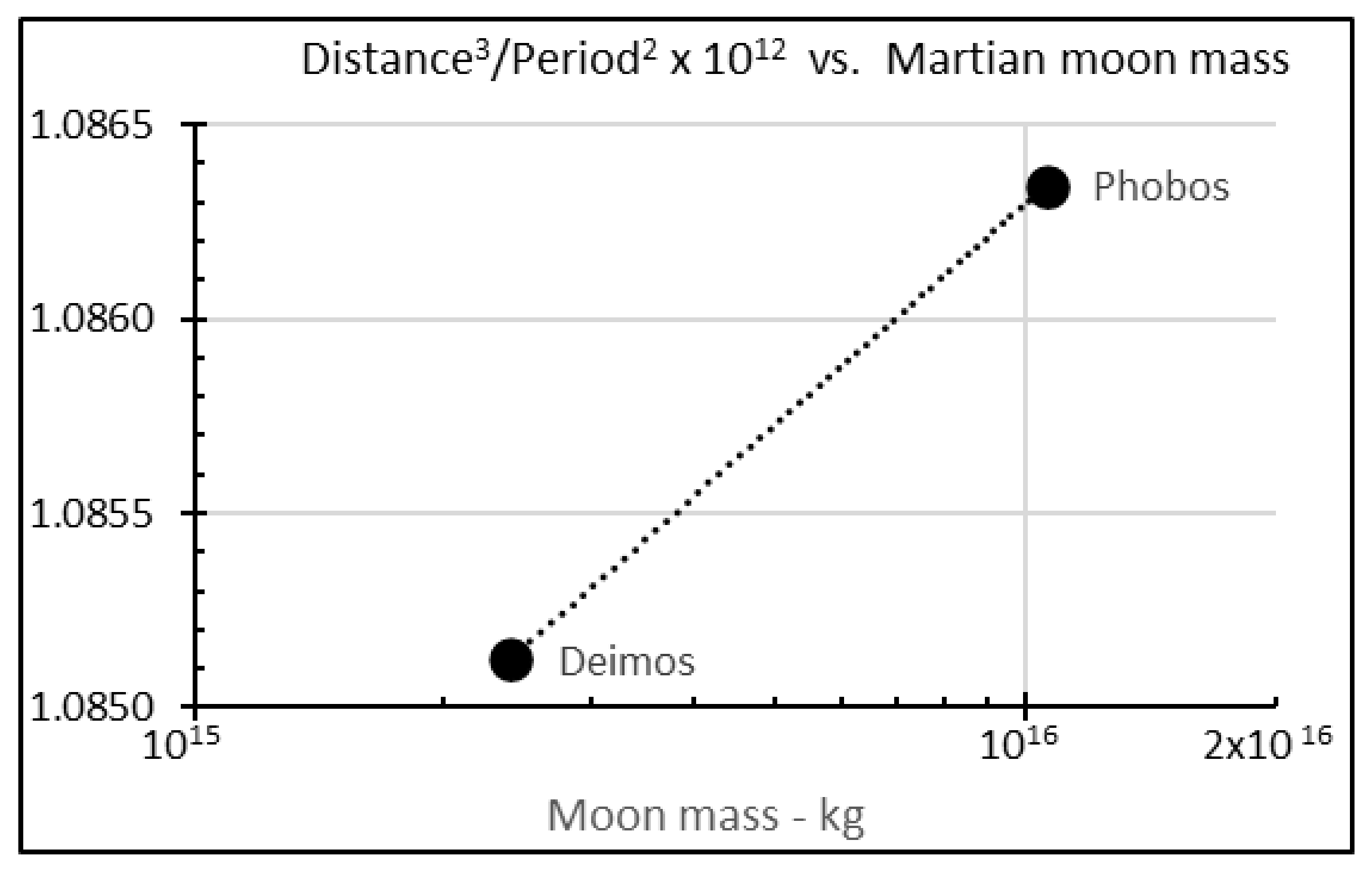
2.3. Moons of Earth and Mars
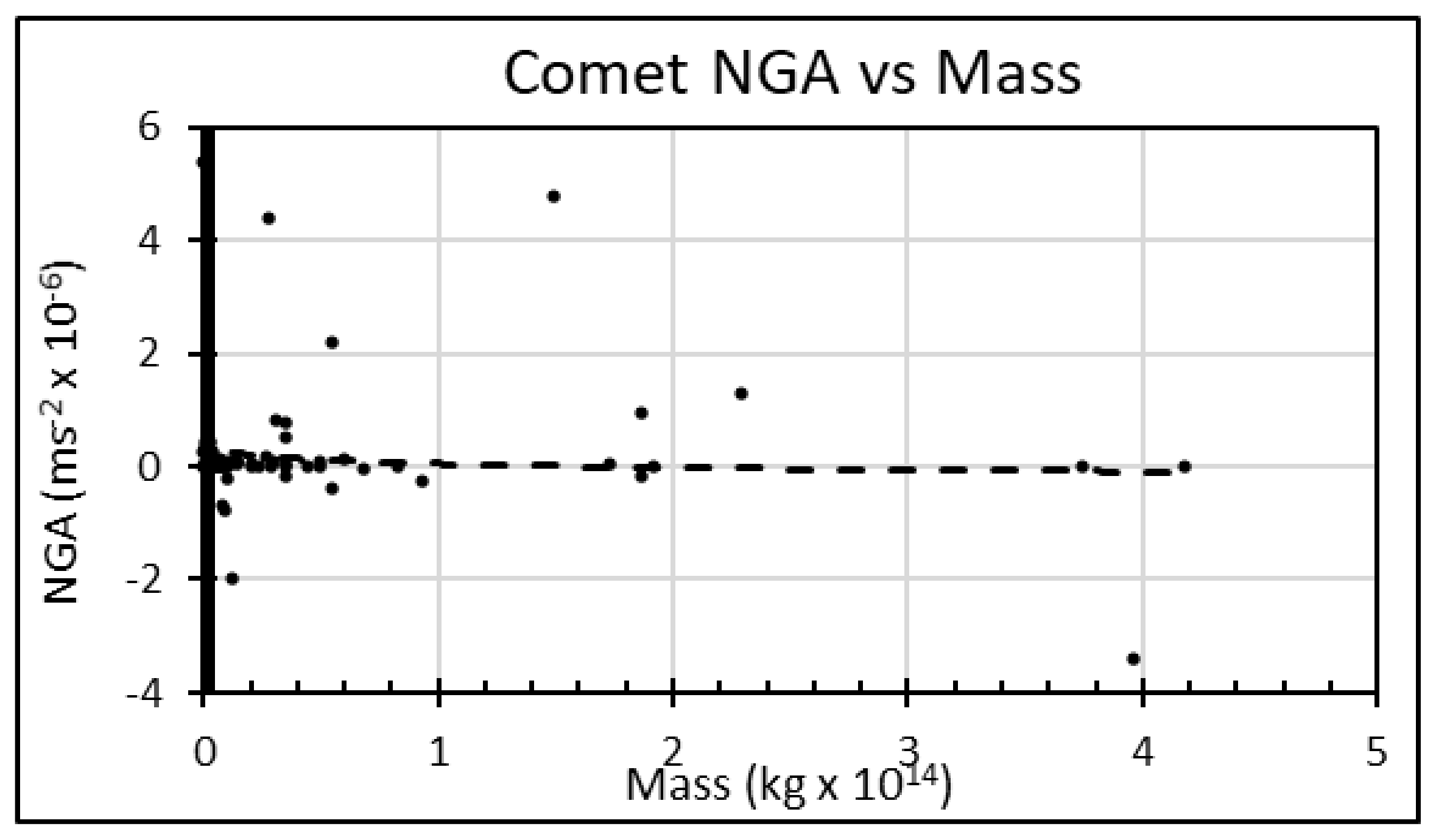
2.4. NGA of ‘Oumuamua and Comets
2.5. Other Possible Gravity Anomalies
3. Discussion
- Benchtop experiments = {6.674 + 0.000200 × ln(mtarget)} × 10−11
- Planets’ orbits
- Martian moons
- Earth’s Moon
- ‘Oumuamua
- Comets
- Official (IAU) value of G G = 6.6743 × 10−11 m3·kg−1·s−2 [5]
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Acedo, L. Anomalous post-Newtonian terms and the secular increase of the astronomical unit. Adv. Space Res. 2013, 52, 1297–1303. [Google Scholar] [CrossRef]
- Anderson, J.D.; Nieto, M.M. Astrometric solar-system anomalies. Proc. Int. Astron. Union 2009, 5, 189–197. [Google Scholar] [CrossRef]
- Debono, I.; Smoot, G.F. General relativity and cosmology: Unsolved questions and future directions. Universe 2016, 2, 23. [Google Scholar] [CrossRef]
- Iorio, L. Gravitational anomalies in the solar system? Int. J. Mod. Phys. D 2015, 24, 1530015. [Google Scholar] [CrossRef]
- International Astronomical Union. Astronomical Constants. 2018. Available online: http://asa.hmnao.com/static/files/2018/Astronomical_Constants_2018.pdf (accessed on 1 August 2021).
- Mohr, P.J.; Newell, D.B.; Taylor, B.N. CODATA recommended values of the fundamental physical constants: 2014. J. Phys. Chem. Ref. Data 2016, 45, 043102. [Google Scholar] [CrossRef]
- Trippe, S. The ‘missing mass problem’in astronomy and the need for a modified law of gravity. Z. Nat. A 2014, 69, 173–187. [Google Scholar]
- Micheli, M.; Farnocchia, D.; Meech, K.J.; Buie, M.W.; Hainaut, O.R.; Prialnik, D.; Schörghofer, N.; Weaver, H.A.; Chodas, P.W.; Kleyna, J.T.; et al. Non-gravitational acceleration in the trajectory of 1I/2017 U1 (‘Oumuamua). Nature 2018, 559, 223–226. [Google Scholar] [CrossRef]
- Ziaeepour, H. Making a quantum universe: Symmetry and gravity. Universe 2020, 6, 194. [Google Scholar] [CrossRef]
- Mannheim, P.D. Alternatives to dark matter and dark energy. Prog. Part. Nucl. Phys. 2006, 56, 340–445. [Google Scholar] [CrossRef]
- Luo, J.; Hu, Z.-K. Status of measurement of the Newtonian gravitational constant G. Class. Quantum Gravity 2000, 17, 2351. [Google Scholar] [CrossRef]
- Wu, J.; Li, Q.; Liu, J.; Xue, C.; Yang, S.; Shao, C.; Tu, L.; Hu, Z.; Luo, J. Progress in precise measurements of the gravitational constant. Ann. Physik 2019, 531, 1900013. [Google Scholar] [CrossRef]
- Rothleitner, C.; Schlamminger, S. Invited review article: Measurements of the Newtonian constant of gravitation, G. Rev. Sci. Instrum. 2017, 88, 111101. [Google Scholar] [CrossRef] [PubMed]
- Milyukov, V.; Fan, S.-H. The Newtonian gravitational constant: Modern status of measurement and the new CODATA value. Gravit. Cosmol. 2012, 18, 216–224. [Google Scholar] [CrossRef]
- Gillies, G.T. The Newtonian gravitational constant: Recent measurements and related studies. Rep. Prog. Phys. 1997, 60, 151. [Google Scholar] [CrossRef]
- Long, D.R. Why do we believe Newtonian gravitation at laboratory dimensions? Phys. Rev. D 1974, 9, 850. [Google Scholar] [CrossRef]
- Xue, C.; Liu, J.-P.; Li, Q.; Wu, J.-F.; Yang, S.-Q.; Liu, Q.; Shao, C.-G.; Tu, L.-C.; Hu, Z.-K.; Luo, J. Precision measurement of the Newtonian gravitational constant. Natl. Sci. Rev. 2020, 7, 1803–1817. [Google Scholar] [CrossRef]
- Gillies, G.T.; Unnikrishnan, C. The attracting masses in measurements of G: An overview of physical characteristics and performance. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2014, 372, 20140022. [Google Scholar] [CrossRef][Green Version]
- Nolting, F.; Schurr, J.; Schlamminger, S.; Kündig, W. A value for G from beam-balance experiments. Meas. Sci. Technol. 1999, 10, 487. [Google Scholar] [CrossRef]
- Armstrong, T.; Fitzgerald, M. New measurements of G using the measurement standards laboratory torsion balance. Phys. Rev. Lett. 2003, 91, 201101. [Google Scholar] [CrossRef]
- Bagley, C.H.; Luther, G.G. Preliminary results of a determination of the Newtonian constant of gravitation: A test of the Kuroda hypothesis. Phys. Rev. Lett. 1997, 78, 3047. [Google Scholar] [CrossRef]
- Gundlach, J.H.; Merkowitz, S.M. Measurement of Newton’s constant using a torsion balance with angular acceleration feedback. Phys. Rev. Lett. 2000, 85, 2869. [Google Scholar] [CrossRef]
- Hu, Z.-K.; Guo, J.-Q.; Luo, J. Correction of source mass effects in the HUST-99 measurement of G. Phys. Rev. D 2005, 71, 127505. [Google Scholar] [CrossRef]
- Karagioz, O.; Izmailov, V. Measurement of the gravitational constant with a torsion balance. Meas. Tech. 1996, 39, 979–987. [Google Scholar] [CrossRef]
- Lamporesi, G.; Bertoldi, A.; Cacciapuoti, L.; Prevedelli, M.; Tino, G.M. Determination of the Newtonian gravitational constant using atom interferometry. Phys. Rev. Lett. 2008, 100, 050801. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Xue, C.; Liu, J.-P.; Wu, J.-F.; Yang, S.-Q.; Shao, C.-G.; Quan, L.-D.; Tan, W.-H.; Tu, L.-C.; Liu, Q.; et al. Measurements of the gravitational constant using two independent methods. Nature 2018, 560, 582–588. [Google Scholar] [CrossRef]
- Luo, J.; Liu, Q.; Tu, L.-C.; Shao, C.-G.; Liu, L.-X.; Yang, S.-Q.; Li, Q.; Zhang, Y.-T. Determination of the Newtonian gravitational constant G with time-of-swing method. Phys. Rev. Lett. 2009, 102, 240801. [Google Scholar] [CrossRef]
- Luther, G.G.; Towler, W.R. Redetermination of the Newtonian gravitational constant G. Phys. Rev. Lett. 1982, 48, 121. [Google Scholar] [CrossRef]
- Newman, R.; Bantel, M.; Berg, E.; Cross, W. A measurement of G with a cryogenic torsion pendulum. Philos. Trans. R. Soc. A 2014, 372, 20140025. [Google Scholar] [CrossRef]
- Parks, H.V.; Faller, J.E. A simple pendulum laser interferometer for determining the gravitational constant. Philos. Trans. R. Soc. A 2014, 372, 20140024. [Google Scholar] [CrossRef] [PubMed]
- Prevedelli, M.; Cacciapuoti, L.; Rosi, G.; Sorrentino, F.; Tino, G. Measuring the Newtonian constant of gravitation G with an atomic interferometer. Philos. Trans. R. Soc. A 2014, 372, 20140030. [Google Scholar] [CrossRef]
- NASA. Planetary Fact. Sheet—Metric. 2019. Available online: https://nssdc.gsfc.nasa.gov/planetary/factsheet (accessed on 1 August 2021).
- Bannister, M.T.; Bhandare, A.; Dybczyński, P.A.; Fitzsimmons, A.; Guilbert-Lepoutre, A.; Jedicke, R.; Knight, M.M.; Meech, K.J.; McNeill, A.; Pfalzner, S.; et al. The natural history of ‘Oumuamua. Nat. Astron. 2019, 3, 594–602. [Google Scholar] [CrossRef]
- Coleman, L. The ‘Oumuamua encounter: How modern cosmology handled its first black swan. Symmetry 2021, 13, 510. [Google Scholar] [CrossRef]
- Sekanina, Z. Outgassing as trigger of 1I/Oumuamua’s nongravitational acceleration: Could this hypothesis work at all? arXiv 2019, arXiv:1905.00935. [Google Scholar]
- Katz, J. Evidence against non-gravitational acceleration of 1I/2017 U1 ‘Oumuamua. Astrophys. Space Sci. 2019, 364, 51. [Google Scholar] [CrossRef]
- Giorgini, J.D. DASTCOM5: JPL Small-Body Data Browser; ascl: 2009.2023; Astrophysics Source Code Library: Leicester, UK, 2020. [Google Scholar]
- Britt, D.; Consolmagno, G.; Merline, W.J. Small Body Density and Porosity: New Data, New Insights. In Proceedings of the 37th Annual Lunar and Planetary Science Conference, League City, TX, USA, 13–17 March 2006; p. 2214. [Google Scholar]
- Marsden, B.G.; Sekanina, Z.; Yeomans, D. Comets and nongravitational forces. V. Astron. J. 1973, 78, 211. [Google Scholar] [CrossRef]
- Acedo, L. The flyby anomaly: A multivariate analysis approach. Astrophys. Space Sci. 2017, 362, 42. [Google Scholar] [CrossRef]
- Anderson, J.D.; Campbell, J.K.; Ekelund, J.E.; Ellis, J.; Jordan, J.F. Anomalous orbital-energy changes observed during spacecraft flybys of Earth. Phys. Rev. Lett. 2008, 100, 091102. [Google Scholar] [CrossRef]
- Rivera, P.C. Gravitational weakening of seismic origin as a driving mechanism of some astronomical anomalies. Appl. Phys. Res. 2019, 11, 10–29. [Google Scholar] [CrossRef]
- Bialy, S.; Loeb, A. Could solar radiation pressure explain ‘Oumuamua’s peculiar acceleration? Astrophys. J. Lett. 2018, 868, L1. [Google Scholar] [CrossRef]
- Hashimoto, T.; Inoue, A.K.; Mawatari, K.; Tamura, Y.; Matsuo, H.; Farusawa, H.; Harikane, Y.; Shibuya, T.; Knudsen, K.K.; Kohno, K.; et al. Big Three Dragons: A z = 7.15 Lyman-break galaxy detected in [O iii] 88 μm,[C ii] 158 μm, and dust continuum with ALMA. Publ. Astron. Soc. Jpn. 2019, 71, 71. [Google Scholar] [CrossRef]
- Zhao, W.; Wright, B.S.; Li, B. Constraining the time variation of Newton’s constant G with gravitational-wave standard sirens and supernovae. J. Cosmol. Astropart. Phys. 2018, 2018, 052. [Google Scholar] [CrossRef]
- Anderson, J.D.; Schubert, G.; Trimble, V.; Feldman, M.R. Measurements of Newton’s gravitational constant and the length of day. EPL Europhys. Lett. 2015, 110, 10002. [Google Scholar] [CrossRef]
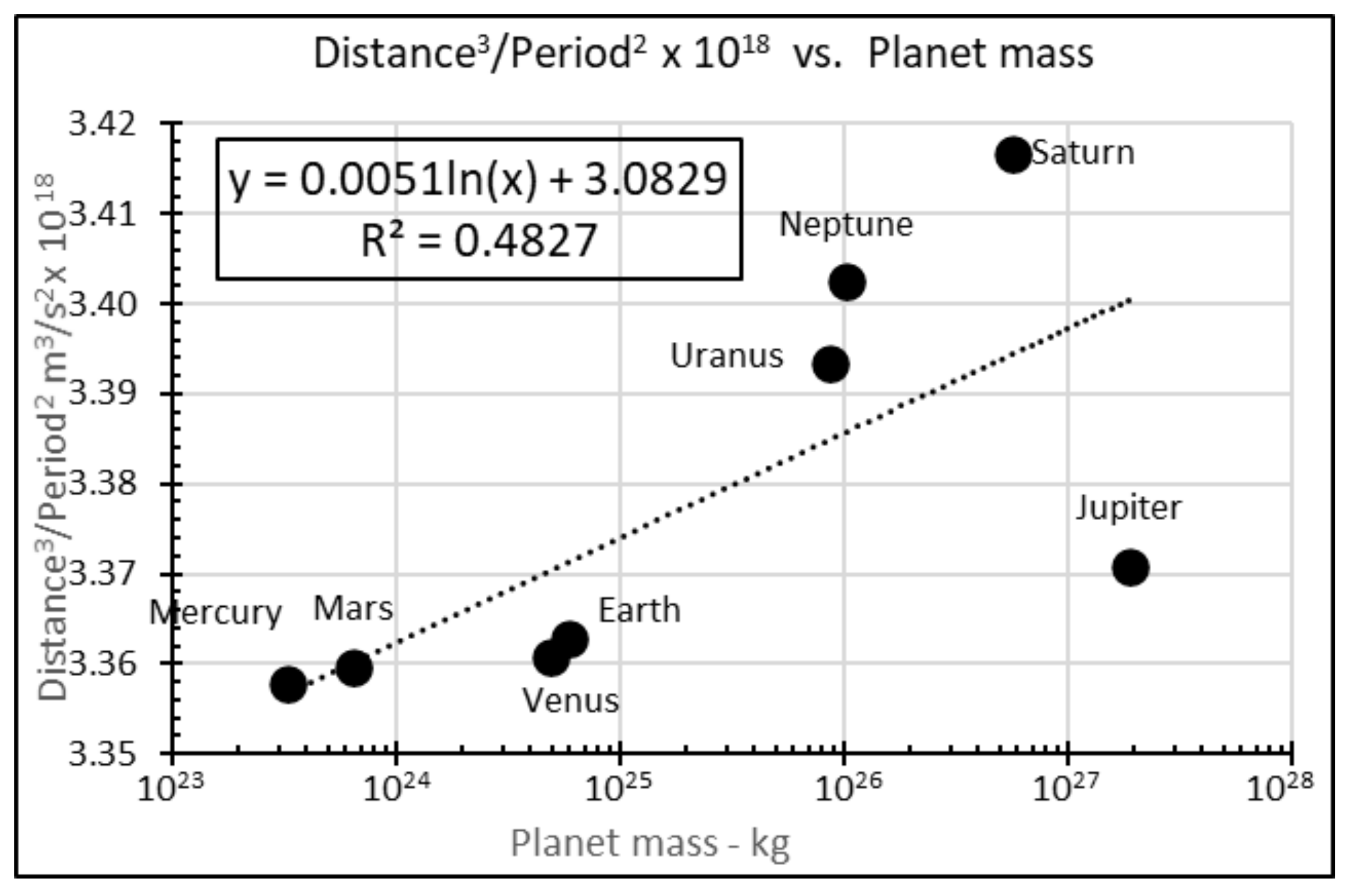
| Panel A: Planet Characteristics | ||||||||
| Mercury | Venus | Earth | Mars | Jupiter | Saturn | Uranus | Neptune | |
| Mass (kg × 1024) | 0.33 | 4.87 | 5.97 | 0.64 | 1.898 | 568 | 86.8 | 102 |
| Distance from Sun (m × 1010) | 5.79 | 10.82 | 14.96 | 22.79 | 77.86 | 143.35 | 287.25 | 449.51 |
| Orbital period (sec × 107) | 0.76 | 1.94 | 3.16 | 5.94 | 37.42 | 92.85 | 264.29 | 516.67 |
| Panel B: Calculated Parameters | ||||||||
| Distance3/Period2 × 1016 | 335.8 | 336.1 | 336.3 | 336.0 | 337.1 | 341.7 | 339.3 | 340.2 |
| Moon | Phobos | Deimos | |
|---|---|---|---|
| Mass (kg) | 7.35 × 1022 | 1.06 × 1016 | 2.40 × 1015 |
| Distance from Planet (m) | 3.84 × 108 | 9.38 × 106 | 2.35 × 107 |
| Orbital period (sec) | 2.36 × 106 | 2.76 × 104 | 1.09 × 05 |
| Panel B: Calculated Parameters | |||
| Distance3/Period2 × 1012 | 10.193 | 1.086 | 1.085 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Coleman, L. Additional Solar System Gravitational Anomalies. Symmetry 2021, 13, 1696. https://doi.org/10.3390/sym13091696
Coleman L. Additional Solar System Gravitational Anomalies. Symmetry. 2021; 13(9):1696. https://doi.org/10.3390/sym13091696
Chicago/Turabian StyleColeman, Les. 2021. "Additional Solar System Gravitational Anomalies" Symmetry 13, no. 9: 1696. https://doi.org/10.3390/sym13091696
APA StyleColeman, L. (2021). Additional Solar System Gravitational Anomalies. Symmetry, 13(9), 1696. https://doi.org/10.3390/sym13091696






