Dynamic Characteristics Analysis of a Circumferential Short Spring Dual Mass Flywheel with the Influence of Centrifugal Force and Friction
Abstract
1. Introduction
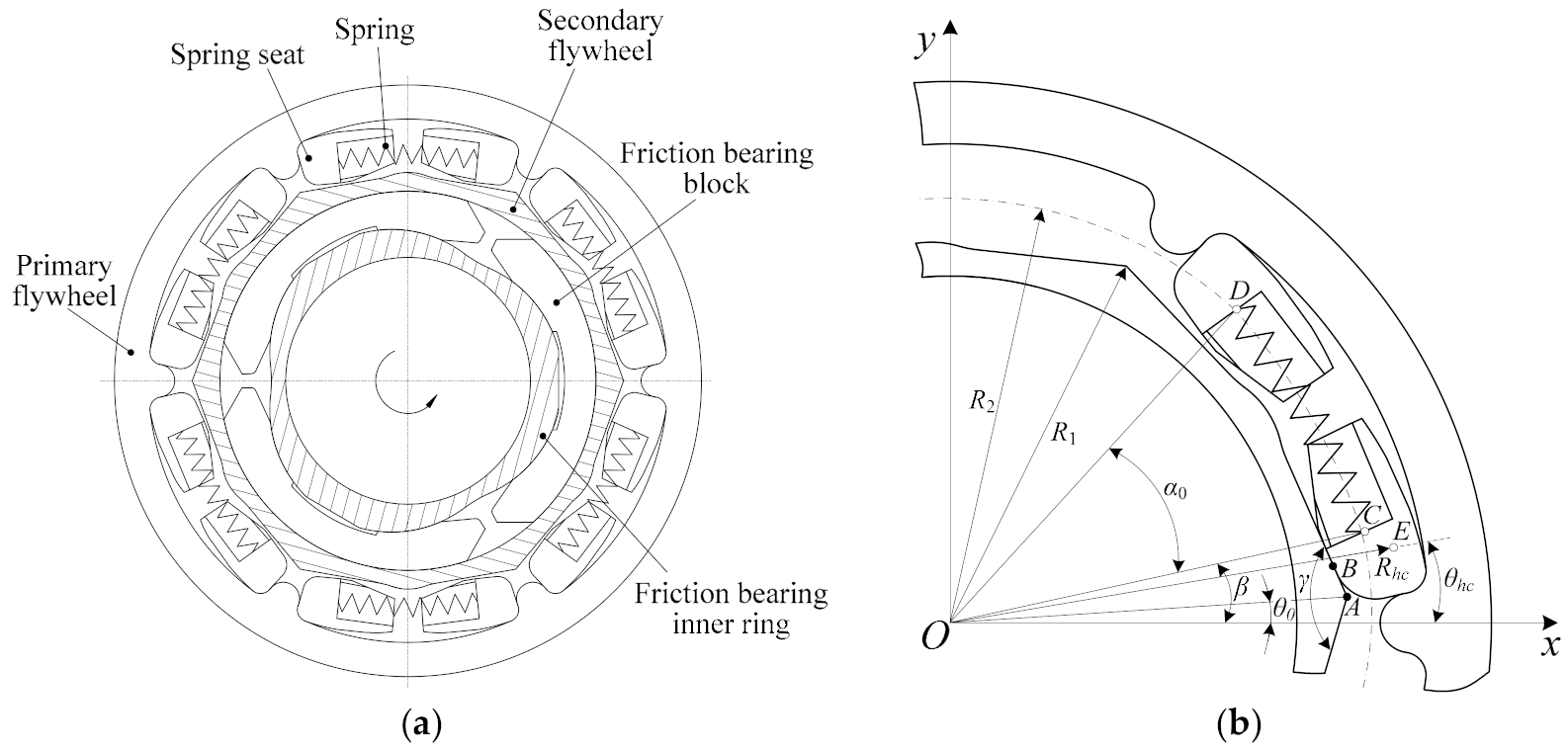
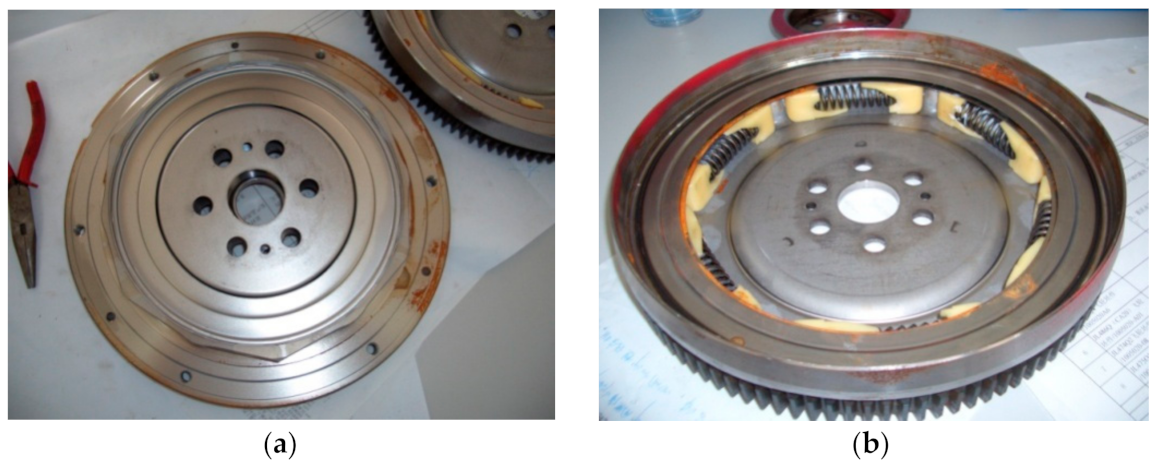
2. DMF Structure and Parameters
3. Mechanical Analysis Model
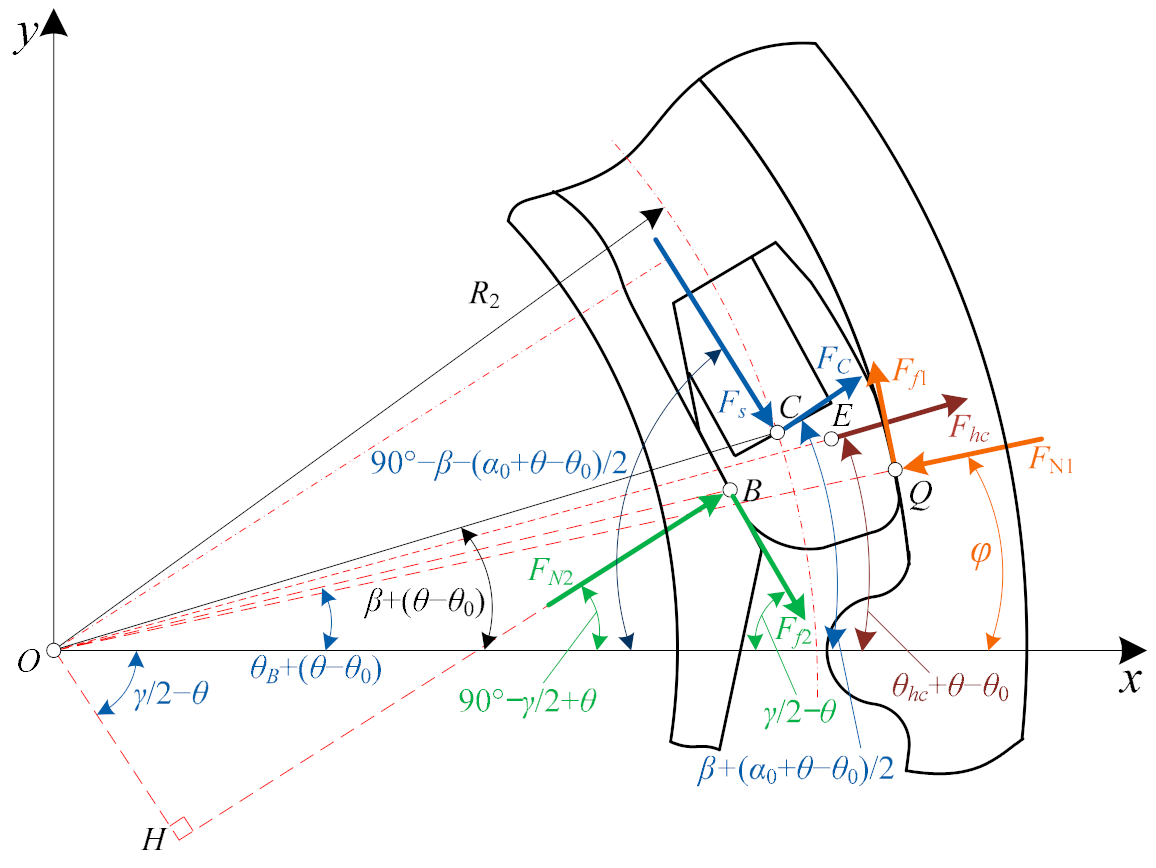
3.1. Determination of Equivalent Force Position between the Secondary Flywheel and Spring Seat
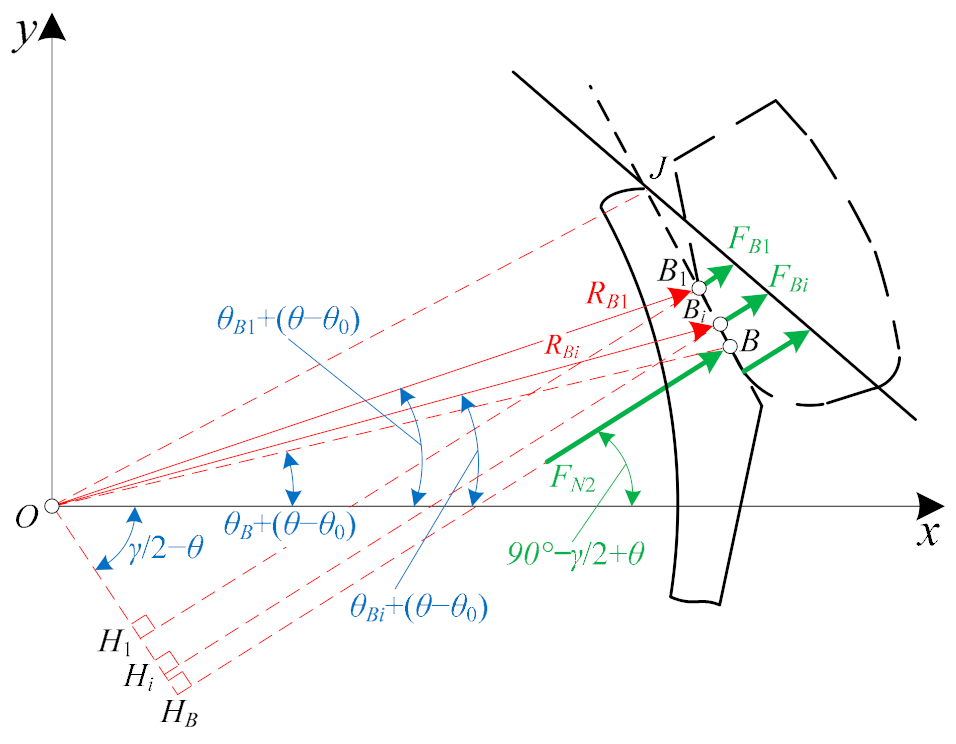
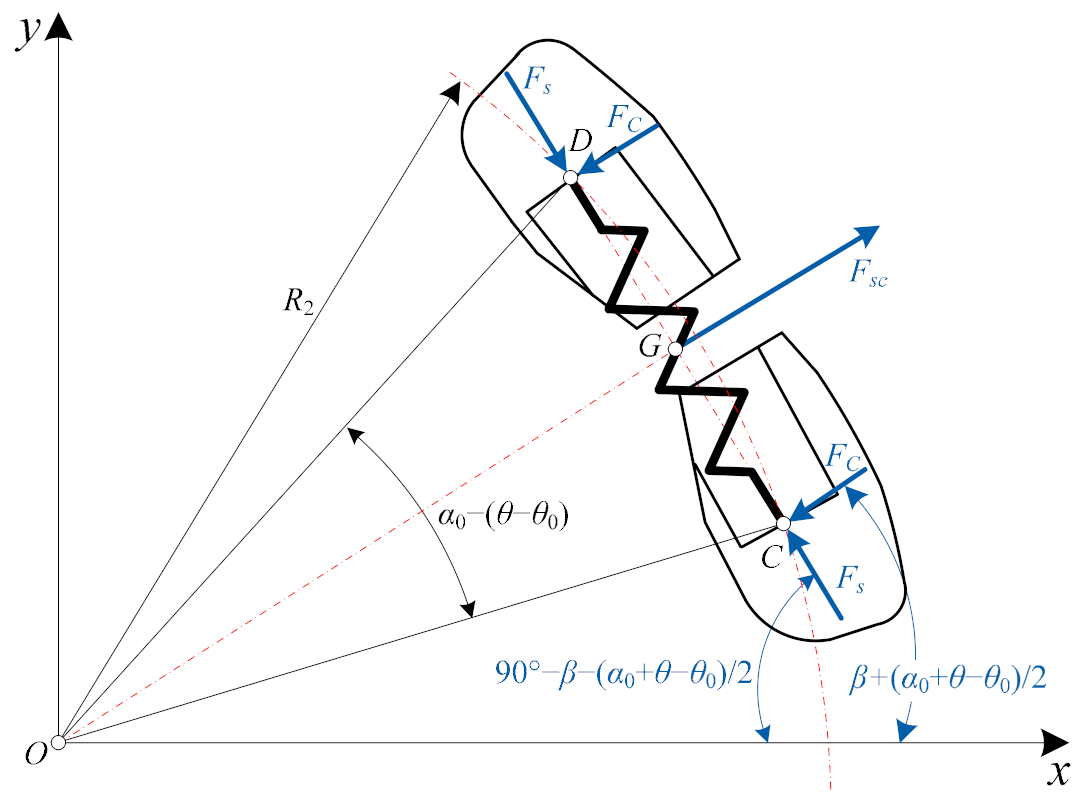
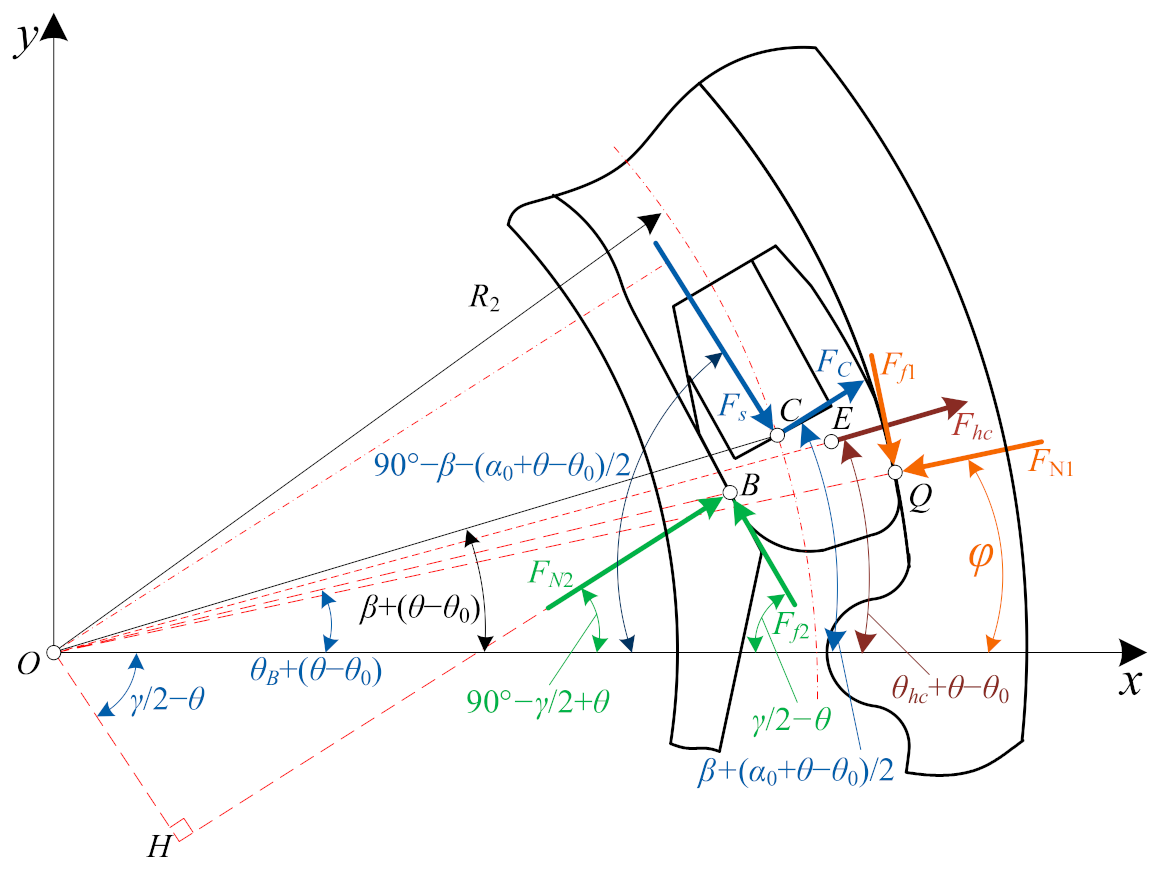
3.2. Force Analysis
3.2.1. When Relative Rotational Angle Increases
3.2.2. When Relative Rotational Angle Decreases
4. Results and Discussion
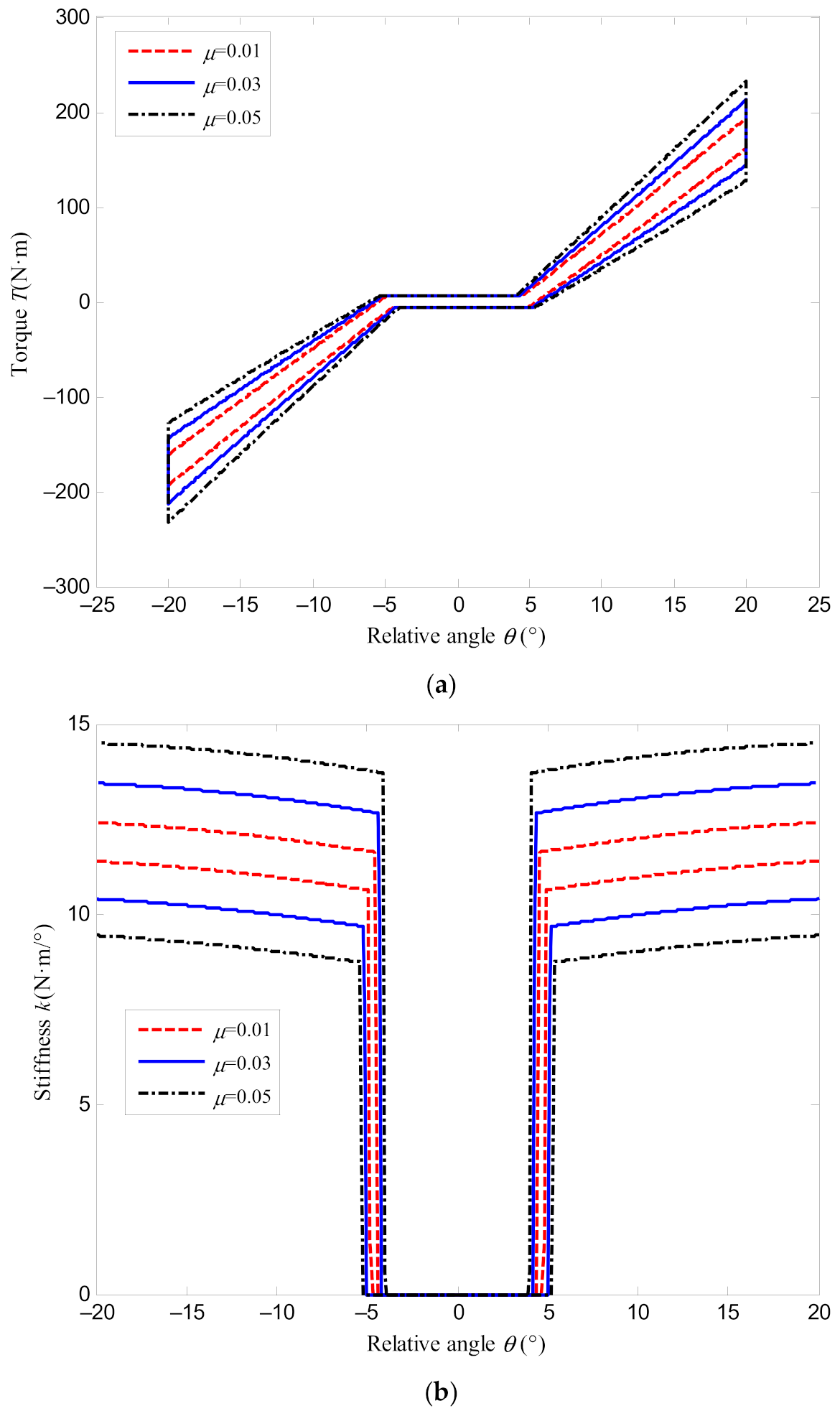
4.1. Torque and Stiffness Characteristics of the DMF
4.2. Influence of Parameters on Torque and Stiffness Characteristics
4.2.1. Effect of Speed
4.2.2. Effect of Friction Coefficient
4.2.3. Effect of Spring Mass
4.2.4. Effect of Spring Seat Mass
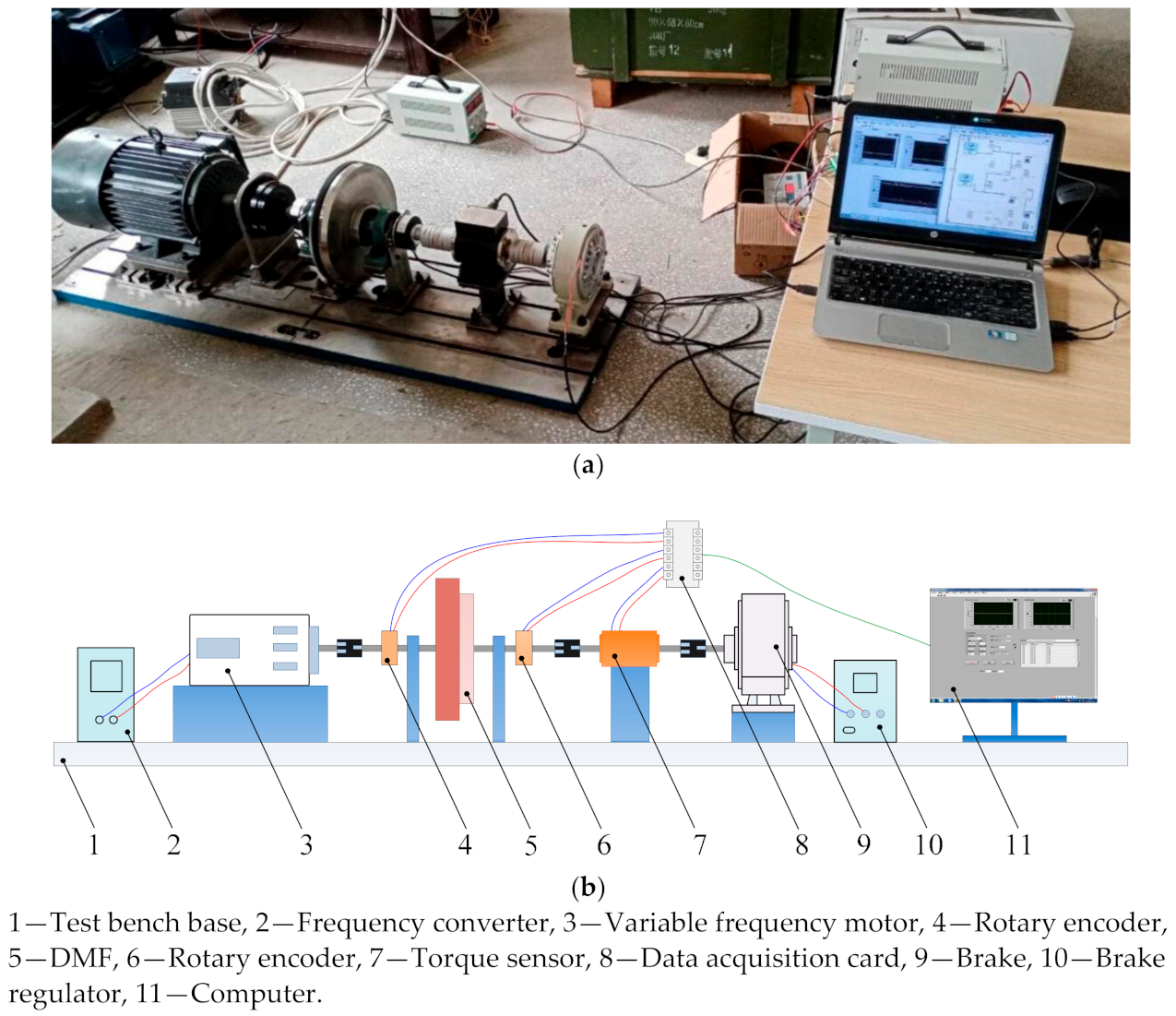
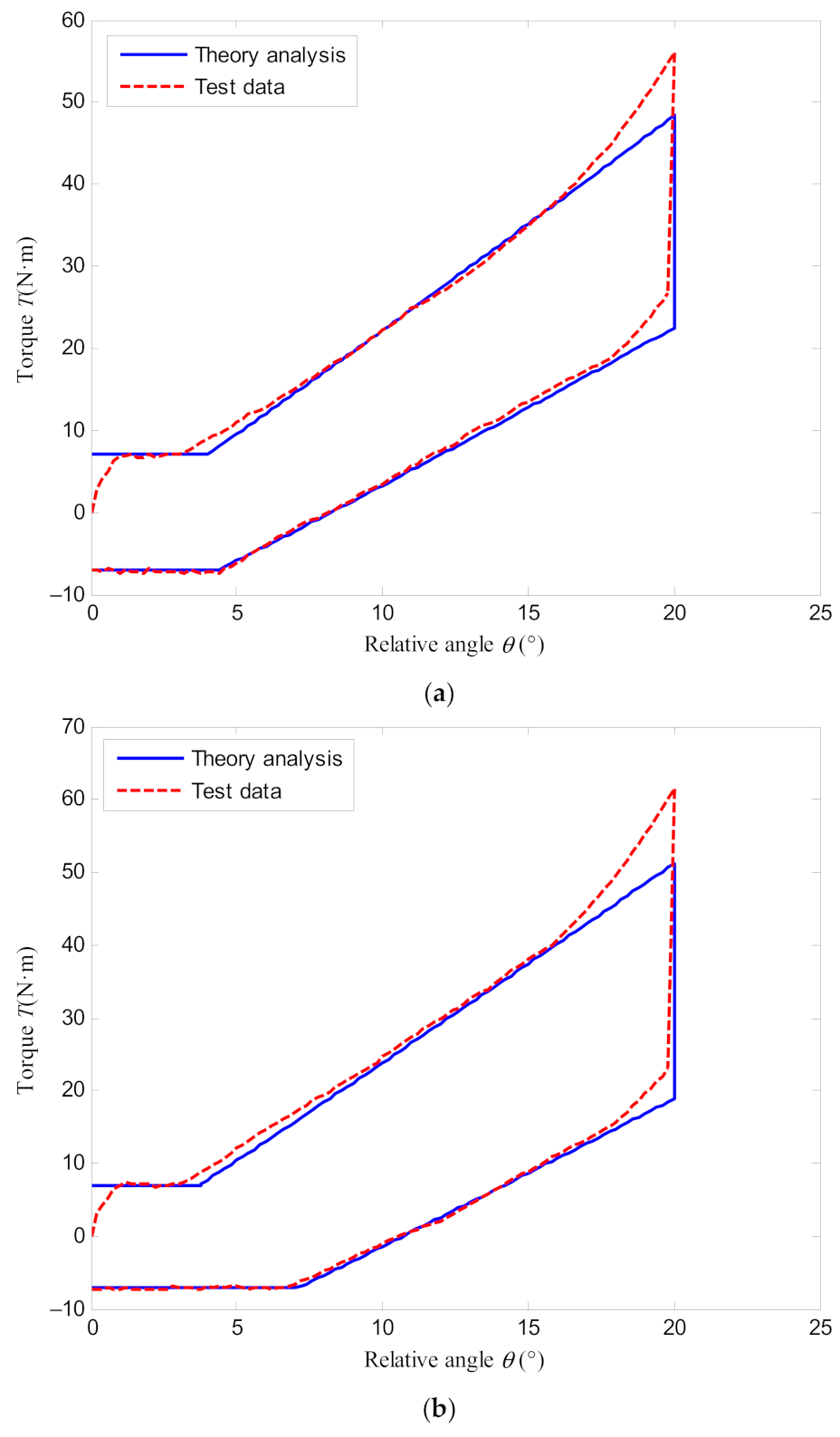
4.3. Dynamic Torque Characteristic Test of DMF
5. Conclusions
- The transmission torque of the DMF has hysteresis characteristic and the torque and stiffness when the relative rotational angle increases are obviously greater than when the relative rotational angle decreases. The greater the relative rotational angle, the larger the torque and stiffness. Besides, the torque transmitted and the stiffness of the DMF also have specific symmetrical characteristics.
- Speed has little influence on the DMF torque when the relative rotational angle increases, but it has a great influence on the torque when the relative rotational angle decreases. A larger rotational speed makes the torque difference between the increases and decreases of the relative rotational angle greater, which results in more obvious hysteresis characteristics of transmission torque. Regardless of whether the relative rotational angle increases or decreases, the higher the speed, the greater the overall stiffness of the DMF and the lag angle will be. However, for a higher speed, the stiffness will change slower with a relative rotational angle.
- When the relative rotational angle increases, a larger friction coefficient will make the torque and stiffness greater. When the relative rotational angle decreases, the torque and stiffness will turn small with the addition of the friction coefficient. Therefore, the larger the friction coefficient, the greater the difference of the torque and stiffness when the relative rotational angle increases and decreases.
- The mass of the damping spring has an effect on the torque characteristics of DMF at small relative rotational angles. The greater the spring mass, the less torque is transmitted. However, the torque under different spring masses becomes close with the increase of rotational angle. The spring mass has a certain influence on the stiffness of the DMF. If the spring mass is increased, the overall stiffness will increase, and the changing process of stiffness will become more gentle with relative rotational angle.
- When the relative rotational angle increases, the addition of spring seat mass makes the transmitted torque greater. While when the relative rotational angle decreases, the transmitted torque will turn smaller with a higher value of the spring seat mass. Spring seat mass almost does not affect the stiffness of the DMF. Regardless of whether the rotational angle increases or decreases, the stiffness characteristic curves are basically the same.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Szpica, D. Modelling of the operation of a Dual Mass Flywheel (DMF) for different engine-related distortions. Math. Comp. Model. Dyn. Syst. 2018, 24, 643–660. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, X.; Yan, Z.F.; Zeng, R. Matching model of dual mass flywheel and power transmission based on the structural sensitivity analysis method. Symmetry 2019, 11, 187. [Google Scholar] [CrossRef]
- More, S.; Medankar, P.; Nagargoje, M. Design and development of dual mass flywheel system. Int. J. Innov. Res. Sci. Eng. Technol. 2015, 4, 964–969. [Google Scholar] [CrossRef]
- Schaper, U.; Sawodny, O.; Mahl, T.; Blessing, U. Modeling and Torque Estimation of an Automotive Dual Mass Flywheel. In Proceedings of the 2009 American Control Conference, St. Louis, MO, USA, 10–12 June 2009; pp. 1207–1212. [Google Scholar] [CrossRef]
- Theodossiades, S.; Gnanakumar, M.; Rahnejat, H.; Kelly, P. Effect of a dual–Mass flywheel on the impact–induced noise in vehicular powertrain systems. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2006, 220, 747–761. [Google Scholar] [CrossRef]
- Lü, Z.H.; Chen, T. Design of the Elastic Characteristics of Torsional Damper with Double Mass Flywheel and Circumferential Spring. Automot. Eng. 2006, 28, 73–77. [Google Scholar]
- Li, Y.N.; Qian, X.J.; Sun, W. Simulation and Study on Dynamic Load Characteristics of Arc Spring. China Mech. Eng. 2012, 23, 155–160. [Google Scholar]
- Mahl, T.; Sawodny, O. Modelling of an automotive dual mass flywheel. In Proceedings of the IFAC Symposium on Mechatronic Systems, Cambridge, MA, USA, 13–15 September 2010; pp. 517–523. [Google Scholar] [CrossRef]
- Kim, T.H.; Song, H.L.; Hwang, S.H.; Kim, H.S. Analysis of Dual Mass Flywheel Using Discrete Arcspring Model. Key Eng. Mater. 2006, 326, 1607–1610. [Google Scholar] [CrossRef]
- Huang, Z.; Chen, D.M.; Wang, D.J.; Sun, W.; Guo, X.L. Design and Simulation Analysis on Arc Helix Spring of Dual Mass Flywheel. J. Acad. Armored Force Eng. 2009, 23, 31–35. [Google Scholar]
- Lü, Z.H.; Wu, Z.G.; Chen, T. The Design Principles and Performance Analysis of DMF-CSS Torsional Damper. Automot. Eng. 2003, 25, 493–497. [Google Scholar]
- He, L.P.; Xia, C.G.; Chen, S.D.; Guo, J.W.; Liu, Y. Parametric Investigation of Dual-Mass Flywheel Based on Driveline Start-Up Torsional Vibration Control. Shock. Vib. 2019, 2019, 3171698. [Google Scholar] [CrossRef]
- Tang, X.; Hu, X.; Yang, B.W.; Yu, H. Novel Torsional Vibration Modeling and Assessment of a Power-Split Hybrid Electric Vehicle Equipped With a Dual-Mass Flywheel. IEEE Trans. Veh. Technol. 2018, 67, 1990–2000. [Google Scholar] [CrossRef]
- Chen, L.; Shi, W.K.; Chen, Z.Y. Research on Damping Performance of Dual Mass Flywheel Based on Vehicle Transmission System Modeling and Multi-Condition Simulation. IEEE Access 2020, 8, 28064–28077. [Google Scholar] [CrossRef]
- Shi, W.K.; Long, Y.; Lu, Y.D. Study on multistage non-linear dual mass flywheel damper. J. Vib. Shock. 2009, 28, 92–96. [Google Scholar]
- Song, L.Q.; Zeng, L.P.; Zhang, S.P.; Zhou, J.D.; Niu, H.E. Design and analysis of a dual mass flywheel with continuously variable stiffness based on compensation principle. Mech. Mach. Theory. 2014, 79, 124–140. [Google Scholar] [CrossRef]
- Song, L.Q.; Zhou, J.D.; Wu, R.H.; Yin, Y.M.; Luo, S.M.; Tian, H.Y.; Fan, Z.Z. Research and Application on Design Theory of Friction Dual Mass Flywheel with Double-stage Piecewise Variable Stiffness. J. Mech. Eng. 2012, 48, 150–157. [Google Scholar] [CrossRef]
- Li, G.H.; Hu, J.J.; Qin, D.T. Study on natural torsional vibration characteristics of dual mass flywheel-radial spring type torsional vibration damper. China Mech. Eng. 2008, 19, 1800–1805. [Google Scholar]
- Zu, Q.H.; Chen, Z.Y.; Shi, W.K.; Mao, Y.; Chen, Z.Y. Torsional vibration semiactive control of drivetrain based on magnetorheological fluid dual mass flywheel. Math. Probl. Eng. 2015, 2015, 608737. [Google Scholar] [CrossRef]
- Kang, T.S.; Kauh, S.K.; Ha, K.P. Development of the displacement measuring system for a dual mass flywheel in a vehicle. Proc. Inst. Mech. Eng. Part. D J. Automob. Eng. 2009, 223, 1273–1281. [Google Scholar] [CrossRef]
- Sangue, S.; Lepoint, G.; Le Bournault, T. New approach to measure instantaneous angular behavior of a dual mass flywheel. In Proceedings of the Seoul 2000 World Automotive Congress, Seoul, Korea, 12–15 June 2000; pp. 12–15. [Google Scholar]
- Wu, F.; Mao, H. Dual Mass Flywheel Torsional Vibration Test-bed. J. Wuhan Univ. Technol. 2010, 32, 758–761. [Google Scholar]
- Tutuncu, N. Effect of anisotropy on stresses in rotating discs. Int. J. Mech. Sci. 1995, 37, 873–881. [Google Scholar] [CrossRef]
- Fairuz, Z.M.; Jahn, I.; Abdul-Rahman, R. The effect of convection area on the deformation of dry gas seal operating with supercritical CO2. Tribol. Int. 2019, 137, 349–365. [Google Scholar] [CrossRef]
- Yuan, F.; Zhu, X.C.; Du, Z.H. Experimental investigation for the effect of rotation on three-dimensional flow field in film-cooled turbine. Chin. J. Mech. Eng. 2007, 20, 10–15. [Google Scholar] [CrossRef]
- Huang, J.H.; Wei, J.H.; Qiu, M.X. Laminar flow in the gap between two rotating parallel frictional plates in hydro-viscous drive. Chin. J. Mech. Eng. 2012, 25, 144–152. [Google Scholar] [CrossRef]
- Xu, C.; Zhang, J.F.; Feng, P.F.; Yu, D.W.; Wu, Z.J. Characteristics of stiffness and contact stress distribution of a spindle-holder taper joint under clamping and centrifugal forces. Int. J. Mach. Tools Manuf. 2014, 82–83, 21–28. [Google Scholar] [CrossRef]
- Feng, P.F.; Yu, D.W.; Wu, Z.J.; Uhlmann, E. Jaw-chuck stiffness and its influence on dynamic clamping force during high-speed turning. Int. J. Mach. Tools Manuf. 2008, 48, 1268–1275. [Google Scholar] [CrossRef]
- Abadian, N.; Gheisari, R.; Keivani, M.; Kanani, A.; Mokhtari, J.; Rach, R.; Abadyan, M. Effect of the centrifugal force on the electromechanical instability of U-shaped and double-sided sensors made of cylindrical nanowires. J. Braz. Soc. Mech. Sci. Eng. 2016, 38, 2129–2148. [Google Scholar] [CrossRef]
- Hashimoto, H.; Wada, S.; Murayama, Y. An Influence of Centrifugal Force on the Performances of Thermo-hydrodynamic Turbulent Thrust Bearings. Bull. JSME 1984, 27, 1230–1236. [Google Scholar] [CrossRef][Green Version]
- Liu, C.Z.; Qin, D.T.; Liao, Y.H. Dynamic modeling and analysis of high-speed planetary gear including centrifugal force. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 3769–3778. [Google Scholar] [CrossRef]
- Belhocine, A.; Abdullah, O.I. A thermomechanical model for the analysis of disc brake using the finite element method in frictional contact. J. Therm. Stresses 2020, 43, 305–320. [Google Scholar] [CrossRef]
- Kosior, F.; Guyot, N.; Maurice, G. Analysis of frictional contact problem using boundary element method and domain decomposition method. Int. J. Numer. Meth. Engng. 2015, 46, 65–82. [Google Scholar] [CrossRef]
- Park, Y.H.; Kim, N.H.; Yim, H.J. Reliability-Based Design Sensitivity Analysis and Optimization for the Hyper-Elastic Structure Using the Meshfree Method. In Proceedings of the 2000 ASME Pressure Vessels and Piping Conference, Seattle, WA, USA, 23–27 July 2000; pp. 107–114. [Google Scholar]
- Almasi, A.; Kim, T.Y.; Laursen, T.A.; Song, J.H. A strong form meshfree collocation method for frictional contact on a rigid obstacle. Comput. Methods Appl. Mech. Engrg. 2019, 357, 112597. [Google Scholar] [CrossRef]
- Nguyen-Thanh, N.; Li, W.D.; Huang, J.Z.; Srikanth, N.; Zhou, K. An adaptive isogeometric analysis meshfree collocation method for elasticity and frictional contact problems. Int. J. Numer. Meth. Engng 2019, 120, 209–230. [Google Scholar] [CrossRef]
- Oliver, J.; Hartmann, S.; Cante, J.; Weyler, R.; Hernández, J. A contact domain method for large deformation frictional contact problems. Part 1: Theoretical basis. Comput. Methods Appl. Mech. Engrg. 2009, 198, 2591–2606. [Google Scholar] [CrossRef]
- Hartmann, S.; Oliver, J.; Weyler, R.; Cante, J.; Hernández, J. A contact domain method for large deformation frictional contact problems. Part 2: Numerical aspects. Comput. Methods Appl. Mech. Engrg. 2009, 198, 2607–2631. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, L.; Xu, Y.; Huang, J.; Song, L. Dynamic Characteristics Analysis of a Circumferential Short Spring Dual Mass Flywheel with the Influence of Centrifugal Force and Friction. Symmetry 2021, 13, 1501. https://doi.org/10.3390/sym13081501
Zeng L, Xu Y, Huang J, Song L. Dynamic Characteristics Analysis of a Circumferential Short Spring Dual Mass Flywheel with the Influence of Centrifugal Force and Friction. Symmetry. 2021; 13(8):1501. https://doi.org/10.3390/sym13081501
Chicago/Turabian StyleZeng, Liping, Yukun Xu, Jie Huang, and Liquan Song. 2021. "Dynamic Characteristics Analysis of a Circumferential Short Spring Dual Mass Flywheel with the Influence of Centrifugal Force and Friction" Symmetry 13, no. 8: 1501. https://doi.org/10.3390/sym13081501
APA StyleZeng, L., Xu, Y., Huang, J., & Song, L. (2021). Dynamic Characteristics Analysis of a Circumferential Short Spring Dual Mass Flywheel with the Influence of Centrifugal Force and Friction. Symmetry, 13(8), 1501. https://doi.org/10.3390/sym13081501




