Centralising Monoids with Low-Arity Witnesses on a Four-Element Set
Abstract
1. Introduction
2. Preliminaries
2.1. Notation and Basic Concepts
2.2. Fundamental Results on Clones, Centralisers and Centralising Monoids
- (a)
- M is a centralising monoid, i.e., there is such that .
- (b)
- , i.e., M is witnessed by .
- (I)
- and is a permutation of prime order or a retractive operation ;
- (II)
- is idempotent, i.e., ;
- (III)
- is a ternary (minority) Mal’cev operation arising as for from a Boolean group ;
- (IV)
- is a ternary majority operation;
- (V)
- is a proper semiprojection of arity n where .
- (a)
- F is a clone of idempotent operations, i.e., .
- (b)
- For every and all we have .
- (c)
- .
- (a)
- .
- (b)
- .
- (c)
- .
- (B)
- for all pairwise distinct there is such that ;
- (D)
- for all pairwise distinct there is such that .
- (B’)
- for all pairwise distinct there is such that ,
- (D’)
- for all pairwise distinct there is such that and ,
- (B”)
- for all pairwise distinct there is such that or or ,
- (D”)
- for all pairwise distinct there is satisfying one of the conditions or or ,
3. Monoids Witnessed by Unary Operations
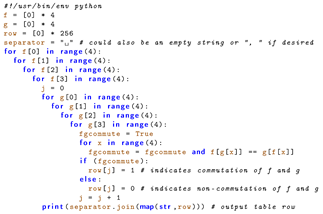
3.1. Computational Results for
3.2. Monoids Witnessed by Permutations
- (a)
- , and
- (b)
- for all the value is arbitrary, but for all .
3.3. Monoids Witnessed by Retractive Operations
3.4. Results
- and is a transposition of just two elements,
- is even and is a product of disjoint transpositions without fixed points,
- is any (non-identical) retraction,
- and is a transposition of just two elements,
- and has a single fixed point,
- and is a product of two disjoint transpositions (without fixed points),
- f is a retraction with for some ,
4. Monoids Witnessed by Binary Idempotent Operations
5. Monoids Witnessed by Mal’cev Operations of Boolean Groups
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1
Appendix A.2
Appendix B
Appendix C
References
- Cohn, P.M. Universal Algebra; Harper & Row Publishers: New York, NY, USA, 1965; pp. xv+333. [Google Scholar]
- Kuznecov, A.V. О средствах для oбнаружения невывoдимoсти или невыразимoсти [Means for detection of nondeducibility and inexpressibility]. In Лoгический вывoд [Logical Inference] (Moscow, 1974); Nauka: Moscow, Russia, 1979; pp. 5–33. (In Russian) [Google Scholar]
- Hermann, M. On Boolean primitive positive clones. Discret. Math. 2008, 308, 3151–3162. [Google Scholar] [CrossRef]
- Danil’čenko, A.F. О параметрическoй выразимoсти функций трехзначнoй лoгики [Parametric expressibility of functions of three-valued logic]. Algebra Log. 1977, 16, 397–416+493. (In Russian) [Google Scholar]
- Danil’čenko, A.F. Parametric expressibility of functions of three-valued logic. Algebra Log. 1977, 16, 266–280. [Google Scholar] [CrossRef]
- Danil’čenko, A.F. Параметрически замкнутые классы функций трехзначнoй лoгики [Parametrically closed classes of functions of three-valued logic]. Bul. Akad. Štiince RSS Moldoven. 1978, 2, 13–20+93. (In Russian) [Google Scholar]
- Danil’čenko, A.F. Некoтoрые свoйства решетки параметрически замкнутых классoв функций трехзначнoй лoгики [Some properties of the lattice of parametrically closed classes of functions of three-valued logic]. Mat. Issled. 1987, 98, 3–9+152. [Google Scholar]
- Danil’čenko, A.F. On parametrical expressibility of the functions of k-valued logic. In Finite Algebra and Multiple-Valued Logic (Szeged, 1979); North-Holland: Amsterdam, The Netherlands, 1981; Volume 28 of Colloquia Mathematica Societatis János Bolyai, pp. 147–159. [Google Scholar]
- Danil’čenko, A.F. Вoпрoсы параметрическoй выразимoсти функций трехзначнoй лoгики [Problems of Parametric Expressibility of Functions in Three-Valued Logic]. Ph.D. Thesis, Institut Matematiki s Vyčislitel’nym Centrom, Akademija Nauk Moldavskoj SSR. [Institute of Mathematics and Computing Centre of the Academy of Sciences of the Moldovian SSR], Kišinev, Moldova, 1979. (In Russian). [Google Scholar]
- Harnau, W. Die Definition von Vertauschbarkeitsmengen in der k-wertigen Logik und das Maximalitätsproblem [The definition of commutativity sets in k-valued logic and the maximality problem]. Z. Math. Logik Grundl. Math. 1974, 20, 339–352. [Google Scholar] [CrossRef]
- Harnau, W. Die vertauschbaren Funktionen der k-wertigen Logik und ein Basisproblem [The commuting functions of k-valued logic and a basis problem]. Z. Math. Logik Grundl. Math. 1974, 20, 453–463. [Google Scholar] [CrossRef]
- Harnau, W. Einige numerische Invarianten der teilweise geordneten Menge Φk von Vertauschbarkeitsmengen der k-wertigen Logik [Some numerical invariants of the partially ordered set Φk of commutativity sets in k-valued logic]. Elektron. Informationsverarbeit. Kybernetik 1974, 10, 543–551. [Google Scholar]
- Harnau, W. Über Kettenlängen der teilweise geordneten Menge Φk der Vertauschbarkeitsmengen der k-wertigen Logik [On lengths of chains of the partially ordered set Φk of commutativity sets in k-valued logic]. Math. Nachr. 1975, 68, 289–297. [Google Scholar] [CrossRef]
- Harnau, W. Die teilweise geordnete Menge Φk der Vertauschbarkeitsmengen der k-wertigen Logik [The partially ordered set Φk of commutativity sets in k-valued logic]. Z. Math. Logik Grundl. Math. 1976, 22, 19–28. [Google Scholar] [CrossRef]
- Harnau, W. Eine Verallgemeinerung der Vertauschbarkeit in der k-wertigen Logik [A generalisation of commutativity in k-valued logic]. Elektron. Informationsverarbeit. Kybernetik 1976, 12, 33–43. [Google Scholar]
- Jakubíková-Studenovská, D.; Šuličová, M. Centralizers of a monounary algebra. Asian Eur. J. Math. 2015, 8, 1550007. [Google Scholar] [CrossRef]
- Jakubíková-Studenovská, D.; Šuličová, M. Green’s relations in the commutative centralizers of monounary algebras. Demonstr. Math. 2015, 48, 536–545. [Google Scholar] [CrossRef][Green Version]
- Černegová, M.; Jakubíková-Studenovská, D. Reconstructability of a monounary algebra from its second centralizer. Comm. Algebra 2017, 45, 4656–4666. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. On endoprimal monoids in clone theory. In Proceedings of the 2009 IEEE 39th International Symposium on Multiple-Valued Logic—ISMVL 2009, Naha, Japan, 21–23 May 2009; IEEE Computer Soc.: Los Alamitos, CA, USA, 2009; pp. 167–172. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. Endoprimal monoids and witness lemma in clone theory. In Proceedings of the 2010 IEEE 40th International Symposium on Multiple-Valued Logic—ISMVL 2010, Barcelona, Spain, 26–28 May 2010; IEEE Computer Soc.: Los Alamitos, CA, USA, 2010; pp. 195–200. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. Maximal centralizing monoids and their relation to minimal clones. In Proceedings of the 2011 IEEE 41st International Symposium on Multiple-Valued Logic—ISMVL 2011, Tuusula, Finland, 23–25 May 2011; IEEE Computer Soc.: Los Alamitos, CA, USA, 2011; pp. 153–159. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. Some centralizing monoids on a three-element set. J. Mult. Valued Logic Soft Comput. 2012, 18, 211–221. [Google Scholar]
- Goldstern, M.; Machida, H.; Rosenberg, I.G. Some classes of centralizing monoids on a three-element set. In Proceedings of the 2015 IEEE 45th International Symposium on Multiple-Valued Logic—ISMVL 2015, Waterloo, ON, Canada, 18–20 May 2015; IEEE Computer Soc.: Los Alamitos, CA, USA, 2015; pp. 205–210. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. Centralizing monoids on a three-element set related to binary idempotent functions. In Proceedings of the 2016 IEEE 46th International Symposium on Multiple-Valued Logic—ISMVL 2016, Sapporo, Japan, 18–20 May 2016; IEEE Computer Soc.: Los Alamitos, CA, USA, 2016; pp. 84–89. [Google Scholar] [CrossRef]
- Machida, H.; Rosenberg, I.G. Centralizing monoids and the arity of witnesses. In Proceedings of the 2017 IEEE 47th International Symposium on Multiple-Valued Logic—ISMVL 2017, Novi Sad, Serbia, 22–24 May 2017; IEEE Computer Soc.: Los Alamitos, CA, USA, 2017; pp. 236–241. [Google Scholar] [CrossRef]
- Rosenberg, I.G. Minimal clones. I. The five types. In Lectures in Universal Algebra (Szeged, 1983); North-Holland: Amsterdam, The Netherlands, 1986; Volume 43 of Colloquia Mathematica Societatis János Bolyai, pp. 405–427. [Google Scholar] [CrossRef]
- Behrisch, M. All centralising monoids with majority witnesses on a four-element set. J. Mult. Valued Logic Soft Comput. in press.
- Behrisch, M.; Pöschel, R. Centralising groups of semiprojections and near unanimity operations. In Proceedings of the 2020 IEEE 50th International Symposium on Multiple-Valued Logic—ISMVL 2020, Miyazaki, Japan, 9–11 November 2020; IEEE Computer Soc.: Los Alamitos, CA, USA, 2020; pp. 291–296. [Google Scholar] [CrossRef]
- Behrisch, M. Centralising monoids with conservative majority operations as witnesses. In Proceedings of the 2021 IEEE 51st International Symposium on Multiple-Valued Logic—ISMVL 2021, Nur-Sultan, Kazakhstan, 25–27 May 2021; IEEE Computer Soc.: Los Alamitos, CA, USA, 2021; pp. 56–61. [Google Scholar] [CrossRef]
- Länger, H.; Pöschel, R. Relational systems with trivial endomorphisms and polymorphisms. J. Pure Appl. Algebra 1984, 32, 129–142. [Google Scholar] [CrossRef][Green Version]
- Bodnarčuk, V.G.; Kalužnin, L.A.; Kotov, V.N.; Romov, B.A. Теoрия Галуа для алгебр Пoста. I, II [Galois theory for Post algebras. I, II]. Kibernetika (Kiev) 1969, 5, 1–10, ibid. 1969, 5, 1–9. [Google Scholar]
- Geiger, D. Closed systems of functions and predicates. Pacific J. Math. 1968, 27, 95–100. Available online: http://projecteuclid.org/euclid.pjm/1102985564 (accessed on 21 July 2021). [CrossRef]
- Pöschel, R. A general Galois Theory for Operations and Relations and Concrete Characterization of Related Algebraic Structures; Report 1980; Akademie der Wissenschaften der DDR Institut für Mathematik: Berlin, Germany, 1980; Volume 1, p. 101. [Google Scholar]
- Pöschel, R. Concrete representation of algebraic structures and a general Galois theory. In Contributions to General Algebra (Proc. Klagenfurt Conf., Klagenfurt, 1978); Heyn: Klagenfurt, Austria, 1979; pp. 249–272. [Google Scholar]
- Ganter, B.; Wille, R. Formal Concept Analysis: Mathematical Foundations; Springer: Berlin/Heidelberg, Germany, 1999; pp. x+284, Translated from the 1996 German Original by Cornelia Franzke. [Google Scholar] [CrossRef]
- Ganter, B.; Obiedkov, S. Conceptual Exploration; Springer: Berlin/Heidelberg, Germany, 2016; pp. xvii+315. [Google Scholar] [CrossRef]
- Behrisch, M. All centralising monoids with majority witnesses on a four-element set. arXiv 2020, arXiv:2011.00338. [Google Scholar]
- Pöschel, R.; Kalužnin, L.A. Funktionen und Relationenalgebren. In Mathematische Monographien [Mathematical Monographs]; VEB Deutscher Verlag der Wissenschaften: Berlin, Germany, 1979; Volume 15, p. 259. [Google Scholar]
- Havlicek, H. Lineare Algebra für Technische Mathematiker [Linear Algebra for Technical Mathematicians]. In Berliner Studienreihe zur Mathematik [Berlin Study Series on Mathematics], 3rd ed.; Heldermann: Lemgo, Germany, 2012; Volume 16, pp. xii+424. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Behrisch, M.; Vargas-García, E. Centralising Monoids with Low-Arity Witnesses on a Four-Element Set. Symmetry 2021, 13, 1471. https://doi.org/10.3390/sym13081471
Behrisch M, Vargas-García E. Centralising Monoids with Low-Arity Witnesses on a Four-Element Set. Symmetry. 2021; 13(8):1471. https://doi.org/10.3390/sym13081471
Chicago/Turabian StyleBehrisch, Mike, and Edith Vargas-García. 2021. "Centralising Monoids with Low-Arity Witnesses on a Four-Element Set" Symmetry 13, no. 8: 1471. https://doi.org/10.3390/sym13081471
APA StyleBehrisch, M., & Vargas-García, E. (2021). Centralising Monoids with Low-Arity Witnesses on a Four-Element Set. Symmetry, 13(8), 1471. https://doi.org/10.3390/sym13081471





