The Skewed-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model
Abstract
:1. Introduction
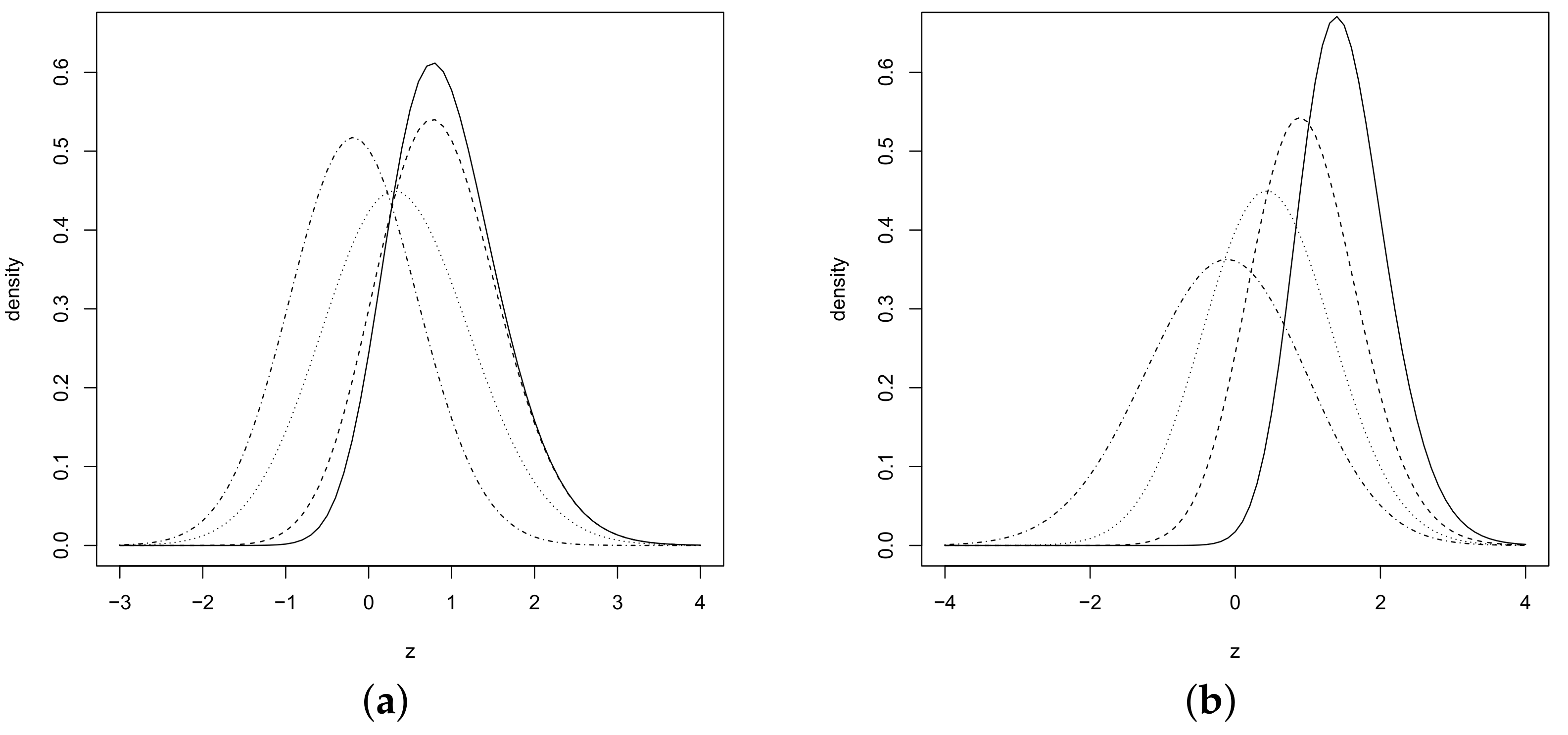
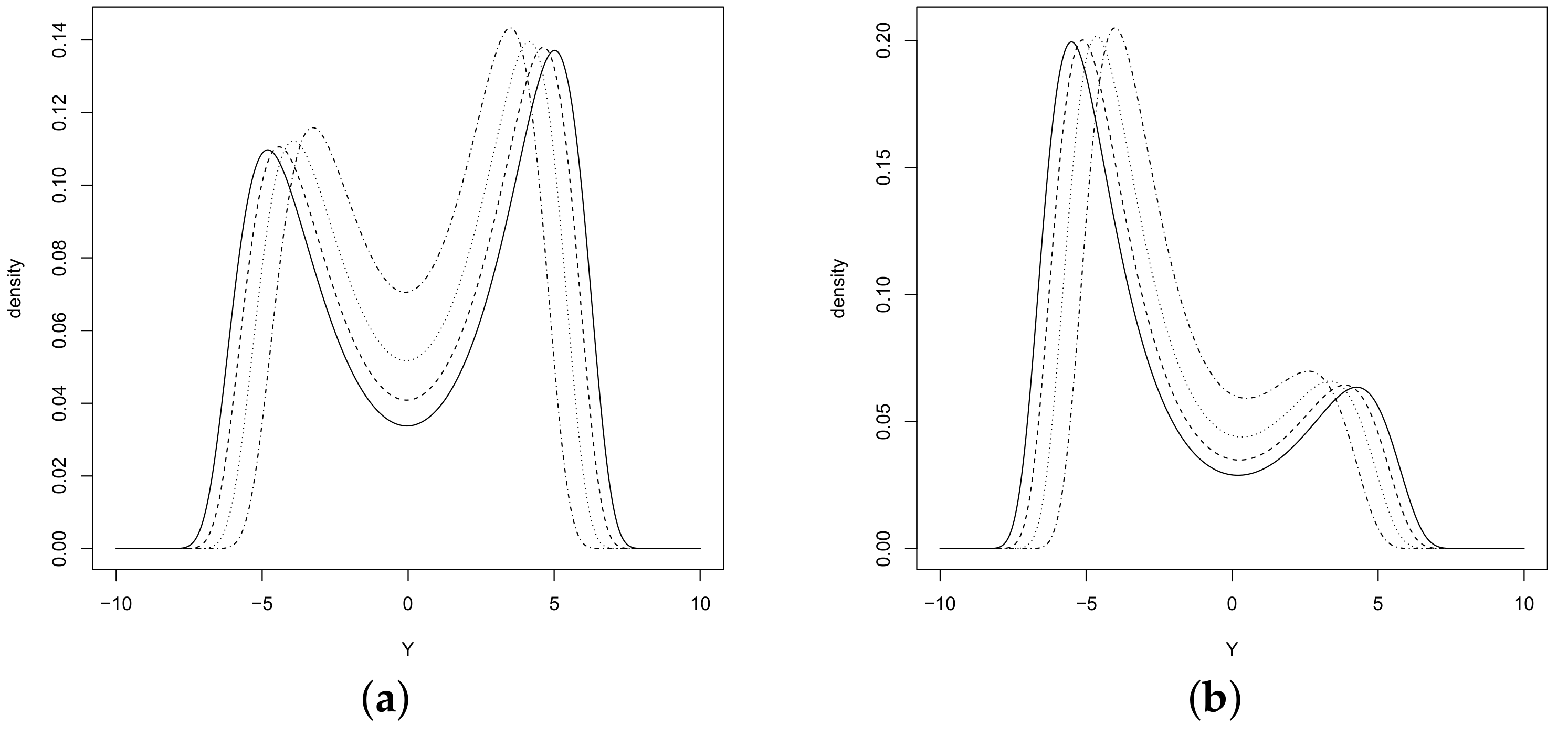
2. Skew-Elliptical Alpha-Power Model
2.1. Skew-Elliptical Sinh-Alpha-Power Model
- sinh-normal model, when and
- skew sinh-normal distribution, when
- sinh-normal power-normal distribution, when
- 1.
- and
- 2.
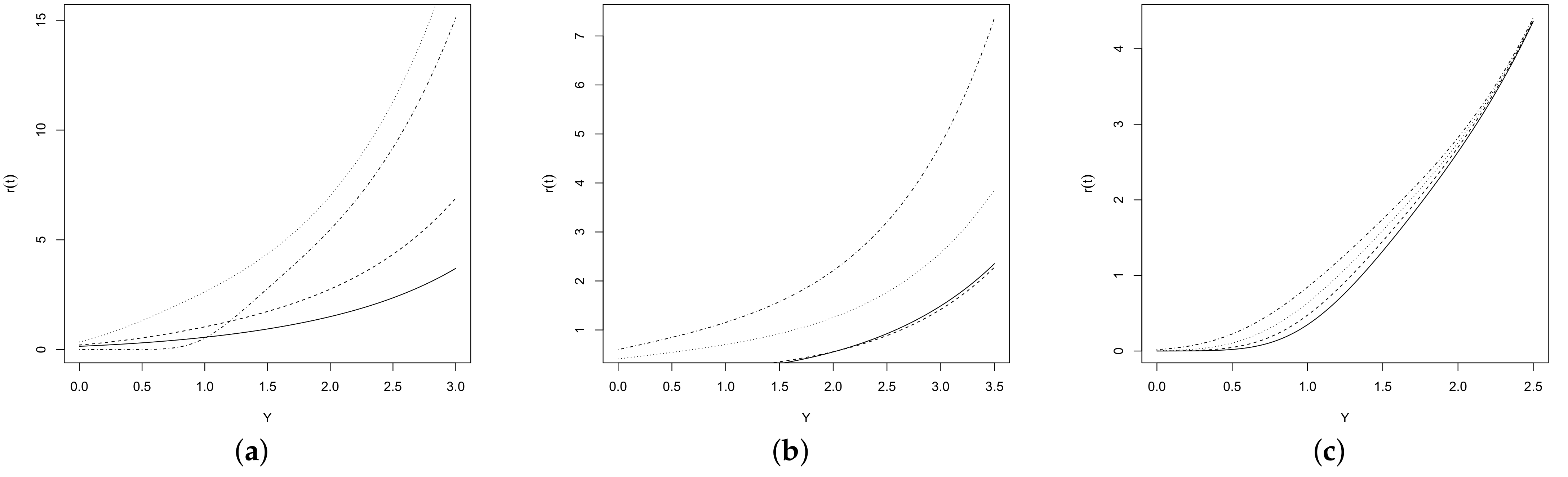
2.2. More Properties
- Let ; then, for constants andIn particular,
- Let ; then,
- Let ; then,
- Let ; then,
2.3. The Skew-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model
3. Inference
Observed Information Matrix
4. Simulation Study
5. Applications
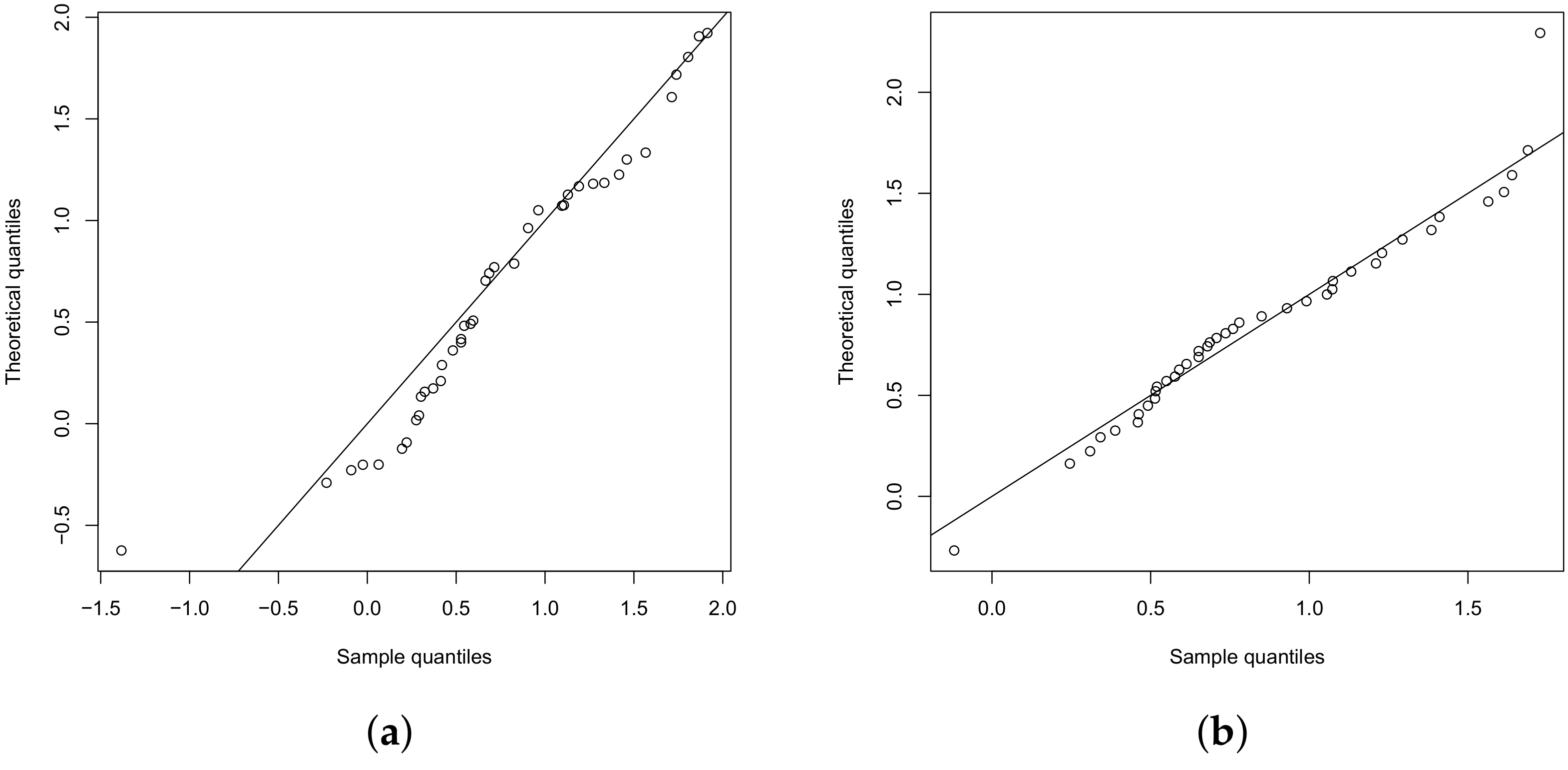
5.1. Application 1: Times to Failure
5.2. Application 2: Patients with Advanced Lung Cancer Status
6. Conclusions
- The introduction of two extra shape parameters makes the error distribution more flexible, allowing it to incorporate additional kurtosis and asymmetry.
- Maximum likelihood properties of large samples such as consistency and asymptotic normality were established.
- A simulation study was performed to evaluate of the ML estimations. As expected, the bias and standard deviation of our estimation decrease as the sample size n increases.
- In the applications, AIC criteria statistics are used. These criteria indicate that the model that best fit the data is SPESN model.
- A Bayesian approach will be worked on in a future work.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fang, K.T.; Zhang, Y.T. Generalized Multivariate Analysis; Sciences Press: Beijing, China; Springer: Berlin, Germany, 1990. [Google Scholar]
- Fang, K.T.; Kotz, S.; Ng, K.W. Symmetric Multivariate and Related Distribution; Chapman and Hall: London, UK, 1990. [Google Scholar]
- Gupta, A.K.; Varga, T. Elliptically Contoured Models in Statistics; Kluwer Academic Publishers: Boston, MA, USA, 1993. [Google Scholar]
- Kelker, D. Distribution Theory of Spherical Distributions and a Location Scale Parameter Generalization. Sankhya Ser. A 1970, 32, 419–430. [Google Scholar]
- Cambanis, S.; Huang, S.; Simons, G. On the Theory of Elliptically Contoured Distributions. J. Multivar. Anal. 1981, 11, 365–385. [Google Scholar] [CrossRef] [Green Version]
- Arellano-Valle, R. Distribuções Elipticas: Propriedades, Inferencia e Aplicações a Modelos de Regressão. Ph.D. Thesis, São Paulo University, São Paulo, Brazil, 1994, unpublished (In Portuguese). [Google Scholar]
- Lange, K.; Little, R.; Taylor, J. Robust statistical modelling using the t distribution. J. Am. Stat. Assoc. 1989, 84, 881–896. [Google Scholar]
- Arellano-Valle, R.; Bolfarine, H. On some characterizations of the Studen-t distribution. Stat. Probab. Lett. 1995, 25, 79–85. [Google Scholar] [CrossRef]
- Azzalini, A. A class of distributions which includes the normal ones. Scand. J. Stat. 1985, 12, 171–178. [Google Scholar]
- Owen, D.B. Tables for computing bi-variate normal probabilities. Ann. Math. Stat. 1956, 27, 1075–1090. [Google Scholar] [CrossRef]
- Durrans, S.R. Distributions of fractional order statistics in hydrology. Water Resour. Res. 1992, 28, 1649–1655. [Google Scholar] [CrossRef]
- Birnbaum, Z.W.; Saunders, S.C. A New Family of Life Distributions. J. Appl. Probab. 1969, 6, 319–327. [Google Scholar] [CrossRef]
- Birnbaum, Z.W.; Saunders, S.C. Estimation for a family of life distributions with applications to fatigue. J. Appl. Probab. 1969, 6, 328–347. [Google Scholar] [CrossRef]
- Díaz-García, J.A.; Leiva-Sánchez, V. A new family of life distributions based on the elliptically contoured distributions. J. Stat. Plan. Inference 2005, 128, 445–457. [Google Scholar] [CrossRef]
- Vilca-Labra, F.; Leiva-Sanchez, V. A new fatigue life model based on the family of skew-elliptical distributions. Commun. Stat. Theory Methods 2006, 35, 229–244. [Google Scholar] [CrossRef]
- Lachos, V.H.; Bolfarine, H.; Arellano-Valle, R.B.; Montenegro, L.C. Likelihood-based inference for multivariate skew-normal regression models. Commun. Stat. Theory Methods 2007, 36, 1769–1786. [Google Scholar] [CrossRef]
- Castillo, N.; Gomez, H.W.; Bolfarine, H. Epsilon Birnbaum-Saunders distribution family: Properties and inference. Stat. Pap. 2011, 52, 871–883. [Google Scholar] [CrossRef]
- Gómez, H.W.; Olivares, J.; Bolfarine, H. An extension of the generalized Birnbaum-Saunders distribution. Stat. Probab. Lett. 2009, 79, 331–338. [Google Scholar] [CrossRef]
- Martínez-Flórez, G.; Bolfarine, H.; Gómez, H.W. The Log-Linear Birnbaum-Saunders Power Model. Methodol. Comput. Appl. Probab. 2017, 19, 913–933. [Google Scholar] [CrossRef]
- Moreno-Arenas, G.; Martínez-Flórez, G.; Barrera-Causil, C. Proportional hazard Birnbaum-Saunders distribution with application to the survival data analysis. Rev. Colomb. Estad. 2016, 39, 129–147. [Google Scholar] [CrossRef]
- Rieck, J.R.; Nedelman, J.R. A log-linear model for the Birnbaum-Saunders distribution. Technometrics 1991, 33, 51–60. [Google Scholar]
- Galea, M.; Leiva, V.; Paula, G. Influence diagnostics in log-Birnbaum-Saunders regression models. J. Appl. Stat. 2004, 31, 1049–1064. [Google Scholar] [CrossRef]
- Barros, M.; Paula, G.A.; Leiva, V. A new class of survival regression models with heavy-tailed errors: Robustness and diagnostics. Lifetime Data Anal. 2008, 14, 316–332. [Google Scholar] [CrossRef] [PubMed]
- Leiva, V.; Vilca, F.; Balakrishnan, N.; Sanhueza, A. A Skewed Sinh-Normal Distribution and Its Properties and Application to Air Pollution. Commun. Stat. Theory Methods 2010, 39, 426–443. [Google Scholar] [CrossRef]
- Lemonte, A.J. A log-Birnbaum-Saunders regression model with asymmetric errors. J. Stat. Comput. Simul. 2012, 82, 1775–1787. [Google Scholar] [CrossRef] [Green Version]
- Santana, L.; Vilca, F.; Leiva, V. Influence analysis in skew-Birnbaum-Saunders regression models and applications. J. Appl. Stat. 2011, 38, 1633–1649. [Google Scholar] [CrossRef]
- Martínez-Flórez, G.; Bolfarine, H.; Gómez, Y.M.; Gómez, H.W. A unification of families of Birnbaum-Saunders distributions with applications. REVSTAT Stat. J. 2020, 18, 637–660. [Google Scholar]
- Chaibub Neto, E.; Branco, M. Bayesian Reference Analysis for Binomial Calibration Problem; IME-USP: São Paulo, Brazil, 2003. [Google Scholar]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series; Gordon and Breach Science Publishers: Amsterdam, The Netherlands, 1990; Volume 1–3. [Google Scholar]
- McCool, J.I. Confidence limits for Weibull regression with censored data. IEEE Trans. Reliab. 1980, 29, 145–150. [Google Scholar] [CrossRef]
- Chan, P.S.; Ng, H.K.T.; Balakrishnan, N.; Zhou, Q. Point and interval estimation for extreme-value regression model under Type-II censoring. Comput. Stat. Data Anal. 2008, 52, 4040–4058. [Google Scholar] [CrossRef]
- Akaike, H. A new look at statistical model identification. IEEE Trans. Autom. Control 1974, AU-19, 716–722. [Google Scholar] [CrossRef]
- Kalbfleisch, J.D.; Prentice, R.L. The Statistical Analysis of Faiture Time Data; Wiley: New York, NY, USA, 2002. [Google Scholar]




| True | n = 100 | n = 200 | n = 500 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Bias | se | MSE | Bias | se | MSE | Bias | se | MSE | ||||||
| −0.5 | −0.75 | 0.5 | −0.9 | 1.3 | 0.0921 | 0.5944 | 0.6525 | 0.0543 | 0.3668 | 0.4003 | 0.0352 | 0.2053 | 0.2334 | |
| −0.0766 | 0.7066 | 0.7719 | −0.0679 | 0.5287 | 0.5875 | −0.0434 | 0.2037 | 0.2270 | ||||||
| 0.1795 | 1.7074 | 1.8050 | 0.0959 | 0.8442 | 0.9009 | 0.0685 | 0.6270 | 0.6635 | ||||||
| −0.1949 | 1.3205 | 1.4016 | −0.0454 | 1.2749 | 1.3221 | −0.0257 | 0.3577 | 0.3902 | ||||||
| 0.5088 | 1.6844 | 1.7630 | 0.2800 | 1.0306 | 1.0900 | 0.0609 | 0.3562 | 0.3795 | ||||||
| −1 | −1 | 0.25 | −0.3 | 1.5 | −0.1087 | 0.6995 | 0.7749 | −0.0834 | 0.5313 | 0.5815 | −0.0649 | 0.3074 | 0.3320 | |
| 0.0980 | 0.7404 | 0.8209 | 0.0688 | 0.5500 | 0.5982 | 0.0505 | 0.3063 | 0.3290 | ||||||
| 0.1136 | 1.1513 | 1.2464 | 0.0766 | 1.0021 | 1.0450 | 0.0626 | 0.3697 | 0.3964 | ||||||
| −0.1217 | 1.5794 | 1.6623 | −0.0824 | 0.7816 | 0.8344 | −0.0437 | 0.4614 | 0.4854 | ||||||
| 0.4648 | 1.4808 | 1.5671 | 0.1977 | 0.5611 | 0.6067 | 0.0902 | 0.3467 | 0.3701 | ||||||
| 1 | 1.5 | 0.75 | 0.3 | 2 | 0.1089 | 0.6150 | 0.6655 | 0.0600 | 0.3760 | 0.4012 | 0.0529 | 0.2760 | 0.3021 | |
| −0.1019 | 0.5051 | 0.5774 | −0.0405 | 0.4160 | 0.4573 | −0.0257 | 0.2900 | 0.3186 | ||||||
| 0.1933 | 1.1773 | 1.2575 | 0.0659 | 1.0441 | 1.0983 | 0.0357 | 0.4767 | 0.5139 | ||||||
| 0.1595 | 1.6046 | 1.6647 | 0.0797 | 0.8889 | 0.9307 | 0.0448 | 0.5883 | 0.6182 | ||||||
| 0.3369 | 1.6177 | 1.7026 | 0.1618 | 1.2599 | 1.2910 | 0.0729 | 0.4479 | 0.4726 | ||||||
| Parameters | SHN | SESN | SPESN |
|---|---|---|---|
| 1.279 (0.143) | 2.011 (0.313) | 5.379 (0.189) | |
| 0.097 (0.170) | −0.961 (0.166) | −2.620 (0.060) | |
| 0.165 | 0.289 | ||
| −14.116 (1.571) | −13.870 (1.602) | −13.602 (1.579) | |
| 1.642 (0.618) | −0.932 (0.174) | ||
| 13.889 (4.991) | |||
| AIC | 129.235 | 125.360 | 122.917 |
| Parameters | SHN | SESN | SPESN |
|---|---|---|---|
| 2.412 (0.153) | 6.963 ( 2.185) | 43.489 (19.792) | |
| 1.524 (0.837) | −1.356 ( 0.713) | −5.678 (0.954) | |
| 0.041 ( 0.015) | 0.034 (0.007) | 0.033 (0.004) | |
| −0.129 ( 0.509) | 0.488 ( 0.305) | 0.5776 (0.194) | |
| 8.505 (5.523) | 9.793 (16.669) | ||
| 2.468 (0.306) | |||
| AIC | 322.208 | 193.285 | 154.786 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez-Flórez, G.; Bolfarine, H.; Gómez, Y.M. The Skewed-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model. Symmetry 2021, 13, 1297. https://doi.org/10.3390/sym13071297
Martínez-Flórez G, Bolfarine H, Gómez YM. The Skewed-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model. Symmetry. 2021; 13(7):1297. https://doi.org/10.3390/sym13071297
Chicago/Turabian StyleMartínez-Flórez, Guillermo, Heleno Bolfarine, and Yolanda M. Gómez. 2021. "The Skewed-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model" Symmetry 13, no. 7: 1297. https://doi.org/10.3390/sym13071297
APA StyleMartínez-Flórez, G., Bolfarine, H., & Gómez, Y. M. (2021). The Skewed-Elliptical Log-Linear Birnbaum–Saunders Alpha-Power Model. Symmetry, 13(7), 1297. https://doi.org/10.3390/sym13071297







