A Chebyshev Wavelet Collocation Method for Some Types of Differential Problems
Abstract
1. Introduction
2. Fundamental Definitions and Mathematical Concepts
2.1. Chebyshev and Shifted Chebyshev Polynomials
2.2. The Chebyshev Wavelets
2.3. Function Approximation
2.4. Estimation of the Truncated Series
2.5. Product Operation Matrix
2.6. Implementation of the Proposed Method
3. Examples of Application
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Huang, C.; Zhang, Z.; Song, Q. Spectral Methods for Substantial Fractional Differential Equations. J. Sci. Comput. 2018, 74, 1554–1574. [Google Scholar] [CrossRef]
- Ali, K.K.; Abd El Salam, M.A.; Mohamed, E.M.; Samet, B.; Kumar, S.; Osman, M.S. Numerical solution for generalized nonlinear fractional integro-differential equations with linear functional arguments using Chebyshev series. Adv. Differ. Equ. 2020, 2020, 494. [Google Scholar] [CrossRef]
- Abu Arqub, O. Application of residual power series method for the solution of time-fractional Schrödinger equations in one-dimensional space. Fundam. Inform. 2019, 166, 87–110. [Google Scholar] [CrossRef]
- Abu Arqub, O. Numerical Algorithm for the Solutions of Fractional Order Systems of Dirichlet Function Types with Comparative Analysis. Fundam. Informaticae 2019, 166, 111–137. [Google Scholar] [CrossRef]
- Kajani, M.T.; Vencheh, A.H.; Ghasemi, M. The Chebyshev wavelets operational matrix of integration and product operation matrix. Int. J. Comput. Math. 2009, 86, 1118–1125. [Google Scholar] [CrossRef]
- Ghasemi, M.; Babolian, E.; Kajani, M.T. Numerical solution of linear Fredholm integral equations using sine-cosine wavelets. Int. J. Comput. Math. 2007, 84, 979–987. [Google Scholar] [CrossRef]
- Amaratunga, K.; Williams, J.R.; Qian, S.; Weiss, J. Wavelet-Galerkin solutions for one-dimensional partial differential equations. Int. J. Numer. Methods Eng. 1994, 37, 2703–2716. [Google Scholar] [CrossRef]
- Daubechies, I. Ten Lectures on Wavelets; SIAM: Philadelphia, PA, USA, 1992. [Google Scholar]
- Majak, J.; Pohlak, M.; Eerme, M.; Lepikult, T. Weak formulation based Haar wavelet method for solving differential equations. Appl. Math. Comput. 2009, 211, 488–494. [Google Scholar] [CrossRef]
- Chun, Z.; Zheng, Z. Three-dimensional analysis of functionally graded plate based on the Haar wavelet method. Acta Mech. Solida Sin. 2007, 20, 95–102. [Google Scholar]
- Lam, H.F.; Ng, C.T. A probabilistic method for the detection of obstructed cracks of beam-type structures using spacial wavelet transform. Probabilistic Eng. Mech. 2008, 23, 237–245. [Google Scholar] [CrossRef]
- Noeiaghdam, S.; Sidorov, D.N.; Muftahov, I.R.; Zhukov, A.V. Control of Accuracy on Taylor-Collocation Method for Load Leveling Problem. Bull. Irkutsk. State Univ. Ser. Math. 2019, 30, 59–72. [Google Scholar] [CrossRef]
- Lepik, Ü.; Hein, H. Haar Wavelets; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Abd-Elhameed, W.M.; Doha, E.H.; Youssri, Y.H. New spectral second kind Chebyshev wavelets algorithm for solving linear and nonlinear second-order differential equations involving singular and Bratu type equations. In Abstract and Applied Analysis; Hindawi: London, UK, 2013; Volume 2013. [Google Scholar]
- Robertsson, J.O.A.; Blanch, J.O. Galerkin-Wavelet Modeling of Wave Propagation: Optimal Finite-Difference Stencil Design. Math. Comput. Model. 1994, 19, 31–38. [Google Scholar] [CrossRef]
- Heydari, M.H.; Hooshmandasl, M.R.; Maalek Ghaini, F.M. A new approach of the Chebyshev wavelets method for partial differential equations with boundary conditions of the telegraph type. Appl. Math. Model. 2014, 38, 1597–1606. [Google Scholar] [CrossRef]
- Kumar, V.; Mehra, M. Wavelet optimized finite difference method using interpolating wavelets for self-adjoint singularly perturbed problems. J. Comput. Appl. Math. 2009, 230, 803–812. [Google Scholar] [CrossRef]
- Turgut, A.K.; Triki, H.; Dhawan, S.; Moshokoa, S.K.; Ullah, M.Z.; Biswas, A. Computational Analysis of Shallow Water Waves with Korteweg-de Vries Equation. Sci. Iran. 2018, 25, 2582–2597. [Google Scholar]
- Turgut, A.K.; Dhawan, S. A practical and powerful approach to potential KdV and Benjamin equations. Beni-Suef Univ. J. Basic Appl. Sci. 2017, 6, 383–390. [Google Scholar]
- Turgut, A.K.; Dhawan, S.; Karakoc, S.B.G.; Raslan, K.R. Numerical Study of Rosenau-KdV Equation Using Finite Element Method Based on Collocation Approach. Math. Model. Anal. 2017, 22, 373–388. [Google Scholar]
- Machado, J.T.; Costa, A.C.; Quelhas, M.D. Wavelet analysis of human DNA. Genomics 2011, 98, 155–163. [Google Scholar] [CrossRef] [PubMed]
- Mao, Z.; Em Karniadakis, G. A Spectral Method (of Exponential Convergence) for Singular Solutions of the Diffusion Equation with General Two-Sided Fractional Derivative. SIAM J. Numer. Anal. 2018, 56, 24–49. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, R.; Osman, M.S.; Samet, B. A wavelet based numerical scheme for fractional order SEIR epidemic of measles by using Genocchi polynomials. Numer. Methods Partial. Equations 2021, 37, 1250–1268. [Google Scholar] [CrossRef]
- Yarmohammadi, M.; Javadi, S.; Babolian, E. Spectral iterative method and convergence analysis for solving nonlinear fractional differential equation. J. Comput. Phys. 2018, 359, 436–450. [Google Scholar] [CrossRef]
- Mason, J.C.; Handscomb, D.C. Chebyshev Polynomials; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Sweilam, N.H.; Nagy, A.M.; El-Sayed, A.A. Second kind shifted Chebyshev polynomials for solving space fractional order diffusion equation. Chaos Solitons Fractals 2015, 73, 141–147. [Google Scholar] [CrossRef]
- Piessens, R. Computing integral transforms and solving integral equations using Chebyshev polynomial approximations. J. Comput. Appl. Math. 2000, 121, 113–124. [Google Scholar] [CrossRef]
- Babolian, E.; Fattahzadeh, F. Numerical solution of differential equations by using Chebyshev wavelet operational matrix of integration. Appl. Math. Comput. 2007, 188, 417–426. [Google Scholar] [CrossRef]
- Zhou, F.; Xu, X. Numerical solution of the convection diffusion equations by the second kind Chebyshev wavelets. Appl. Math. Comput. 2014, 247, 353–367. [Google Scholar] [CrossRef]
- Mohammadi, F.; Hosseini, M.M. A comparative study of numerical methods for solving quadratic Riccati differential equations. J. Franklin Inst. 2011, 348, 156–164. [Google Scholar] [CrossRef]
- Yuanlu, L.I. Solving a nonlinear fractional differential equation using Chebyshev wavelets. Commun. Nonlinear Sci. Numer. 2010, 15, 2284–2292. [Google Scholar]
- Singh, I.; Arun, P.; Lima, F. Fourier analysis of nonlinear pendulum oscillations. Rev. Bras. Ensino Fis. 2018, 40, e1305. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Youssri, Y.H. Fifth-kind orthonormal Chebyshev polynomial solutions for fractional differential equations. Comput. Appl. Math. 2018, 37, 2897–2921. [Google Scholar] [CrossRef]
- Atta, A.G.; Moatimid, G.M.; Youssri, Y.H. Generalized Fibonaacci operational collocation approach for fractional initial value problem. Int. J. Appl. Comput. Math. 2018, 5, 9. [Google Scholar] [CrossRef]
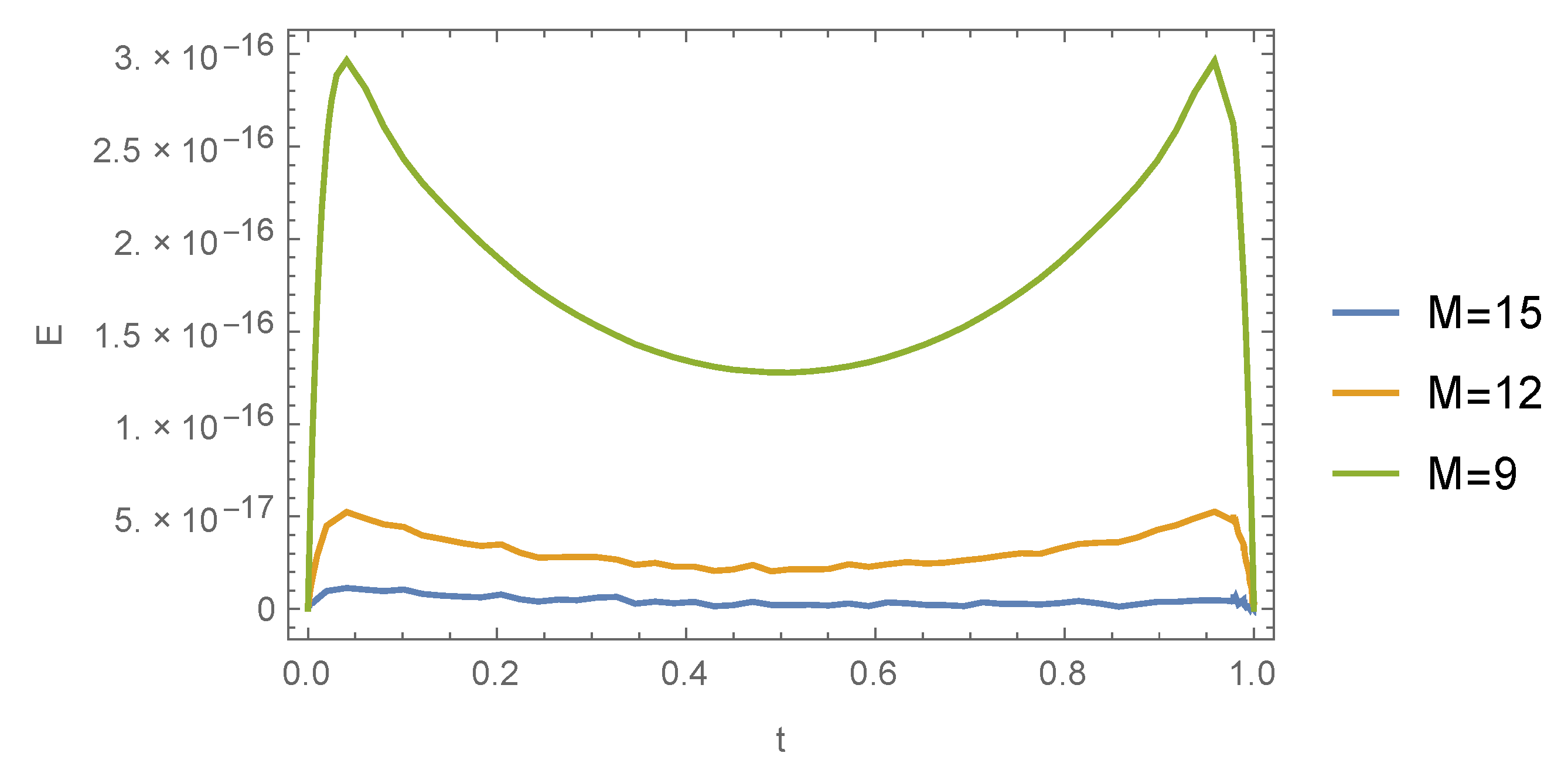
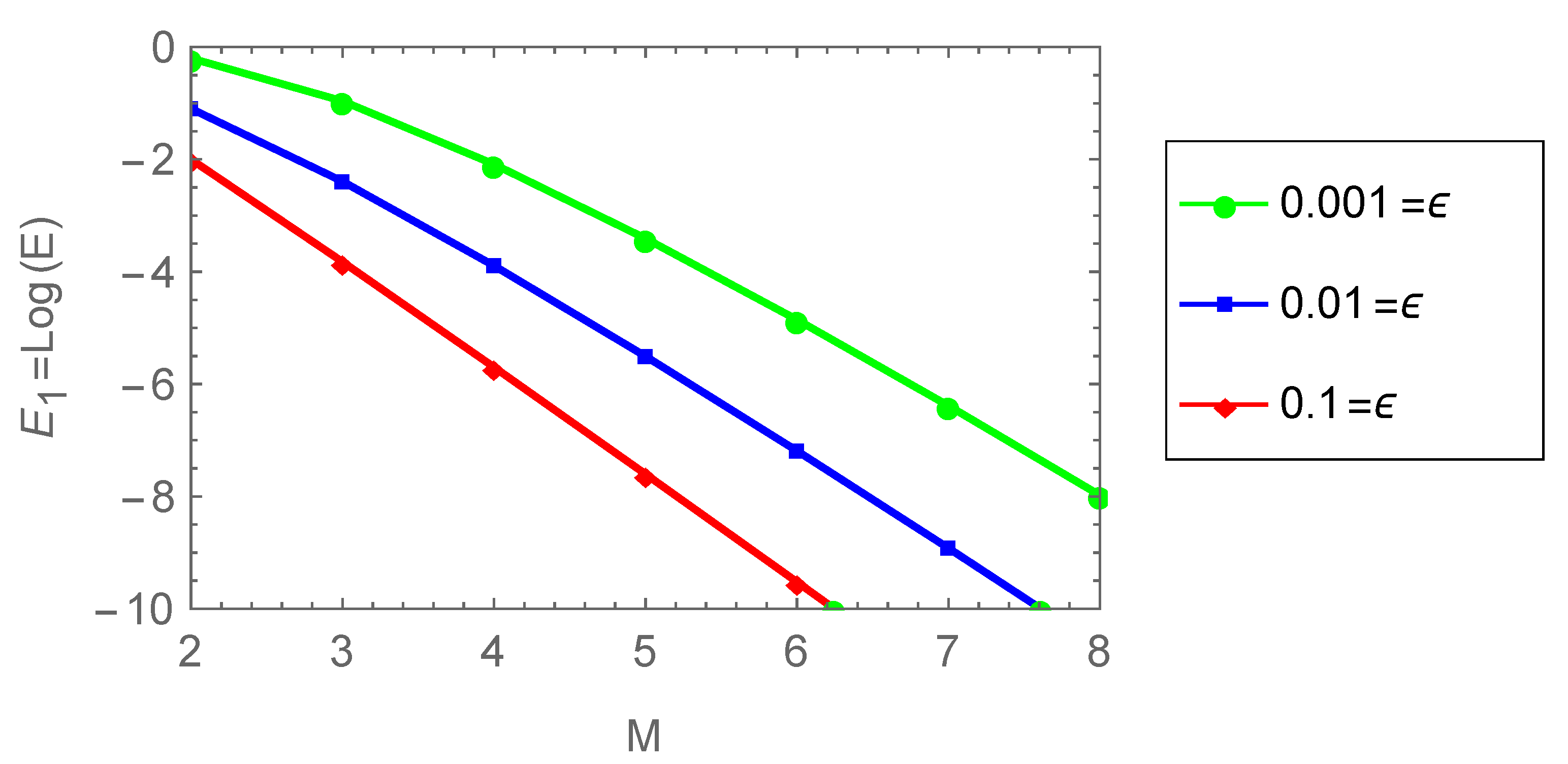
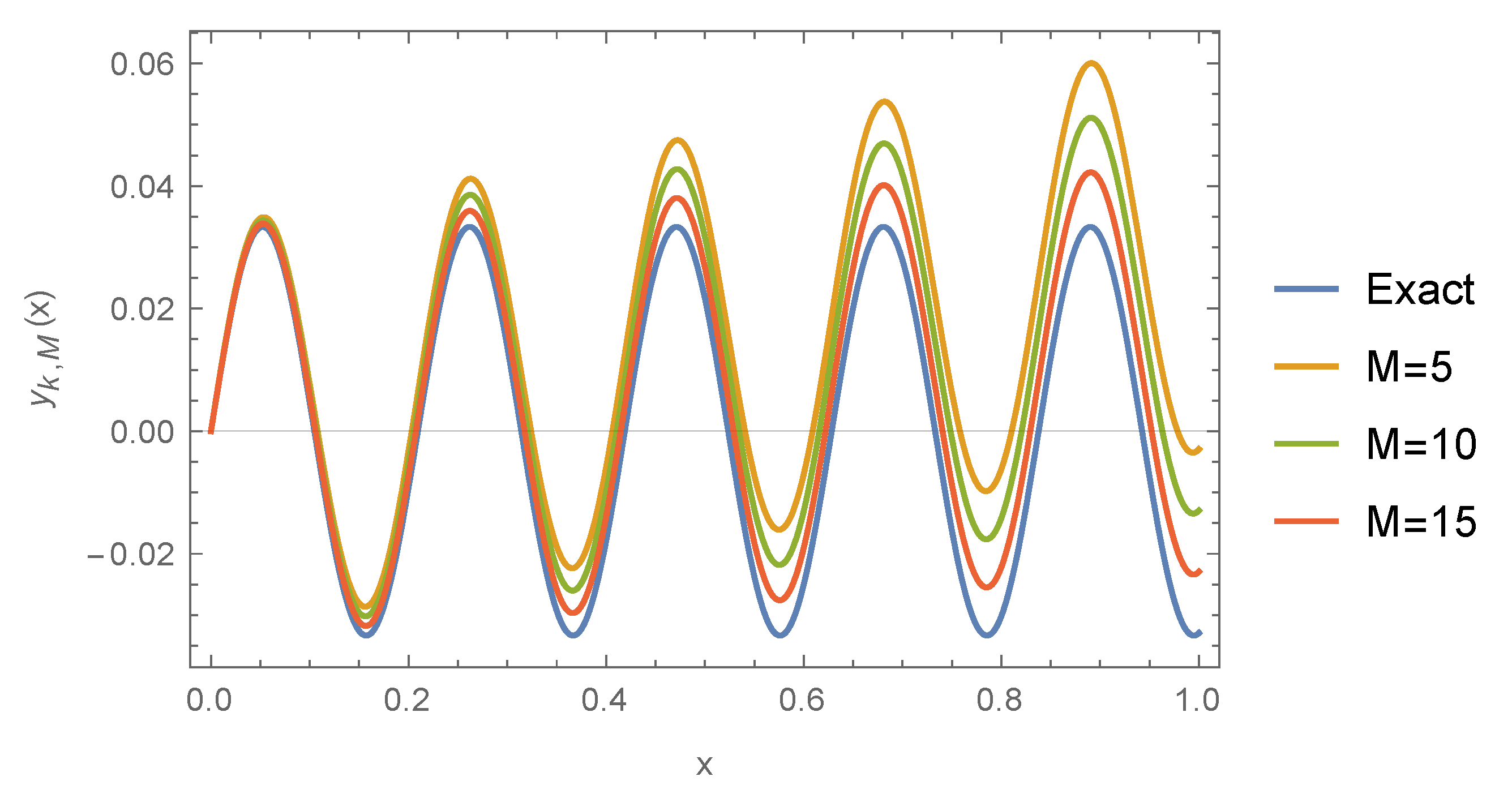
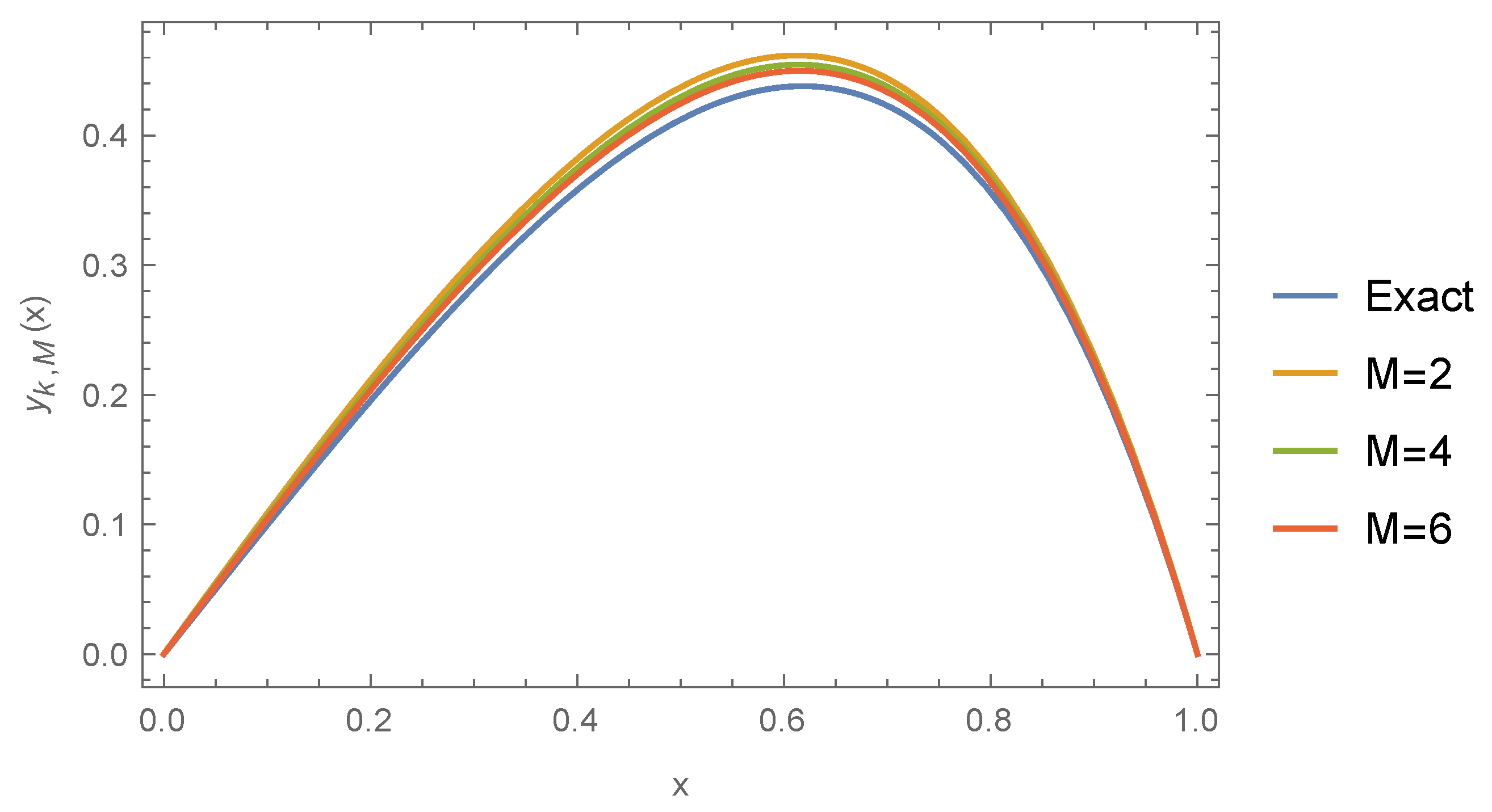
| 0 | 0 |
| k | M | |||
|---|---|---|---|---|
| 1 | 15 | |||
| 2 | 7 | |||
| 3 | 3 |
| M | 5 | 10 | 15 | 20 |
|---|---|---|---|---|
| E |
| M | 8 | 10 | 12 | 14 |
|---|---|---|---|---|
| E |
| s | RK6 | |||
|---|---|---|---|---|
| 0 | 1 | 1 | 1 | 1 |
| 1.0707773 | 1.0707685 | 1.0707724 | 1.070777 | |
| 1.10651554 | 1.1065354 | 1.1065142 | 1.1065155 | |
| 1.106542707 | 1.10654257 | 1.10654217 | 1.10654270 | |
| 1.07084925 | 1.0708984 | 1.0708487 | 1.0708492 | |
| 1 | 1 | 1 | 1 | 1 |
| t | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 2 | 1.95 | 1.9 | 1.85 | 1.8 | 1.75 | |
|---|---|---|---|---|---|---|
| E |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dhawan, S.; Machado, J.A.T.; Brzeziński, D.W.; Osman, M.S. A Chebyshev Wavelet Collocation Method for Some Types of Differential Problems. Symmetry 2021, 13, 536. https://doi.org/10.3390/sym13040536
Dhawan S, Machado JAT, Brzeziński DW, Osman MS. A Chebyshev Wavelet Collocation Method for Some Types of Differential Problems. Symmetry. 2021; 13(4):536. https://doi.org/10.3390/sym13040536
Chicago/Turabian StyleDhawan, Sharanjeet, José A. Tenreir Machado, Dariusz W. Brzeziński, and Mohamed S. Osman. 2021. "A Chebyshev Wavelet Collocation Method for Some Types of Differential Problems" Symmetry 13, no. 4: 536. https://doi.org/10.3390/sym13040536
APA StyleDhawan, S., Machado, J. A. T., Brzeziński, D. W., & Osman, M. S. (2021). A Chebyshev Wavelet Collocation Method for Some Types of Differential Problems. Symmetry, 13(4), 536. https://doi.org/10.3390/sym13040536








