The Statistical Damage Constitutive Model of the Mechanical Properties of Alkali-Resistant Glass Fiber Reinforced Concrete
Abstract
1. Introduction
2. Materials and Methods
2.1. Uniaxial Tensile Test Material
- (1)
- Alkali-resistant glass fiber
- (2)
- Concrete matrix materials
2.2. Mix Proportion Design of Concrete Samples
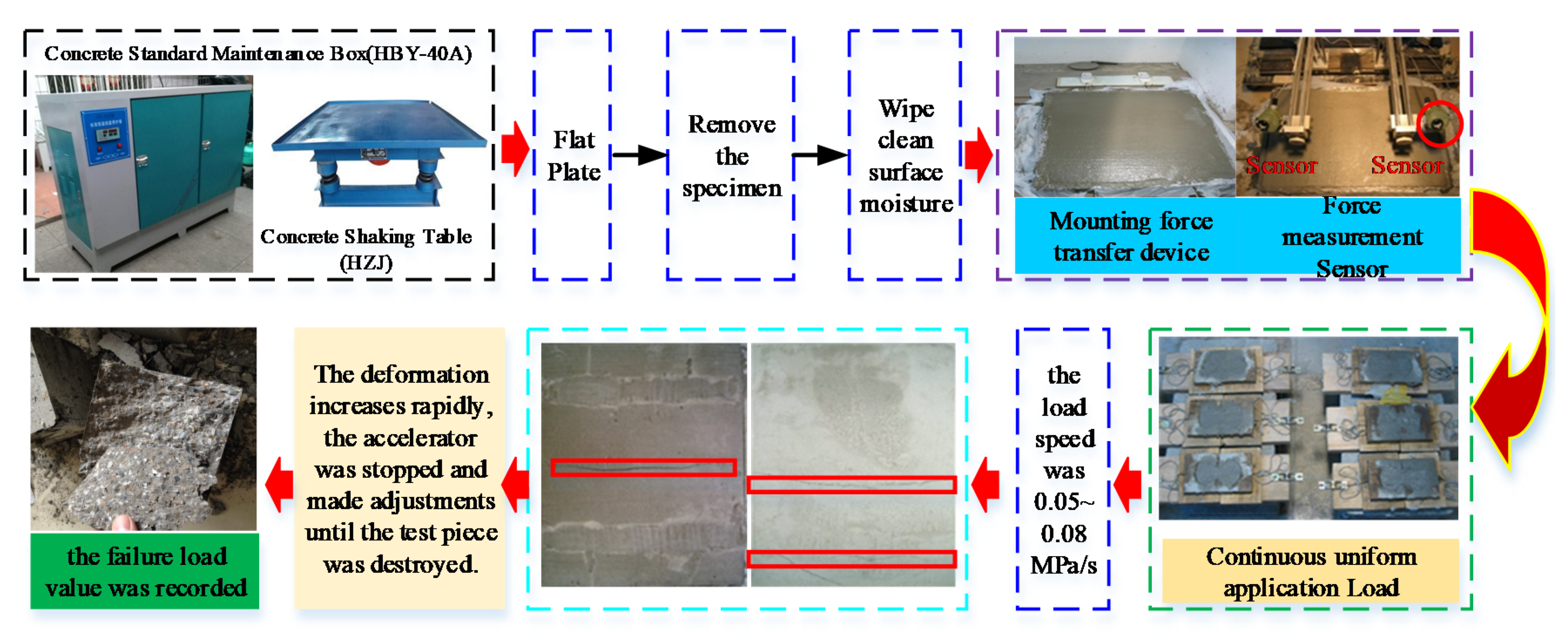
2.3. Preparation and Test Scheme for Concrete Samples
- (1)
- After the concrete specimens were cured, the surface moisture of the specimens was wiped off and a force transfer device was placed on both sides of the specimens.
- (2)
- The specimen force sensor was attached and the corresponding stress sensors were placed on both sides. The stress monitor was attached on the left and right sides of the specimens.
- (3)
- The test piece was continuously and uniformly loaded, and the load speed was 0.05–0.08 MPa/s. When the deformation of the test piece increased rapidly, the accelerator was stopped and adjustments were made until the test piece was destroyed. At this time, the failure load value was recorded.
3. Test Results and Analysis of the AR-GFRC
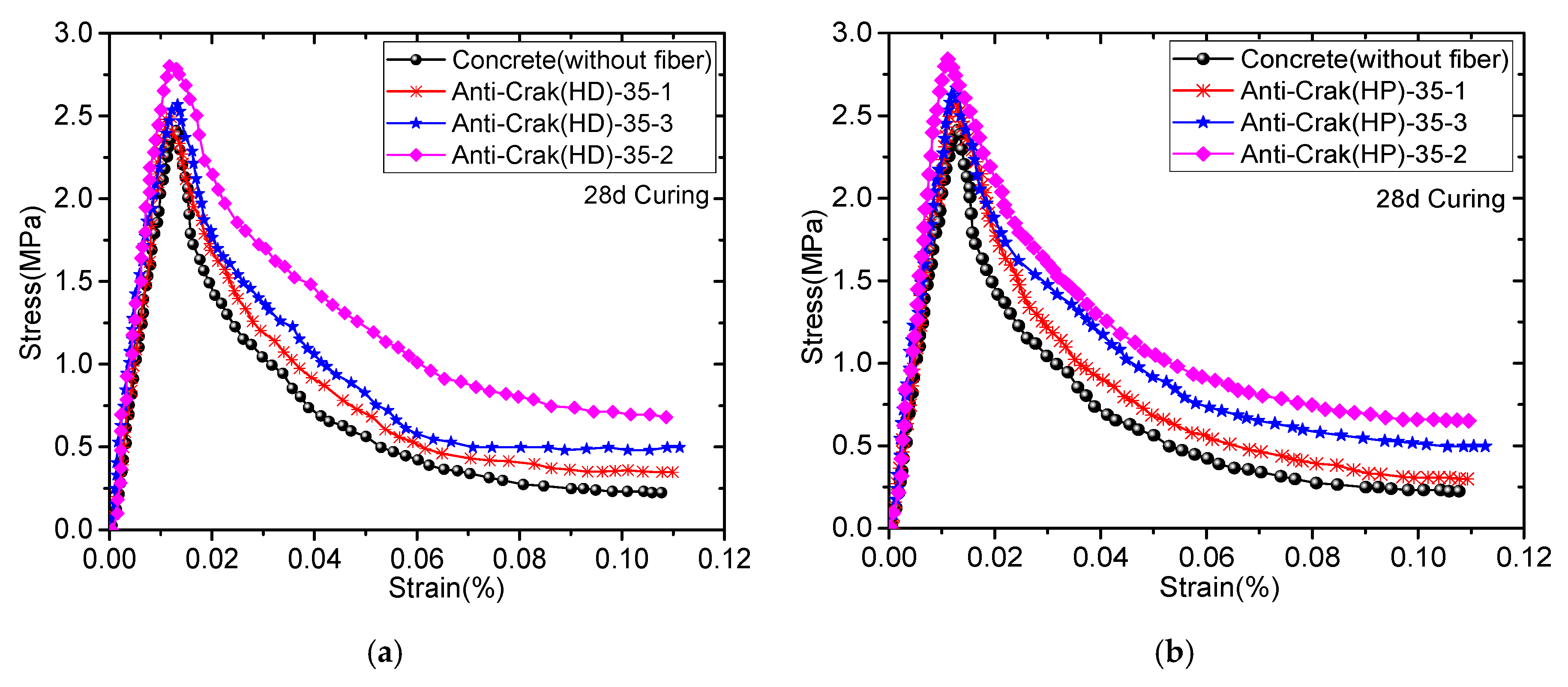
3.1. Effect of Fiber Content on the Tensile Strength of Concrete
3.2. Stress-Strain Curve of the Whole Process of the AR-GFRC
- (1)
- Elastic deformation to micro-fracture development stage (OA region)
- (2)
- Stress peak stage (AB region)
- (3)
- Progressive rupture stage (BC region)
- (4)
- Post-rupture stage (CD region)
4. Development of the Statistical Damage Constitutive Model for the AR-GFRC
4.1. Assumptions
- (1)
- Assuming that the AR-GFRC consists of a series of isotropic elastic micro-units, each of which is an ideal elastomer with the same stiffness prior to the destruction.
- (2)
- Assuming that the micro-unit strength follows the Weibull distribution.
- (3)
- Assuming that the concrete consists of an undamaged part and a damaged part and the stress-strain relationship of the undamaged part obeys Hooke’s law.
- (4)
- Assuming that the concrete damage only occurs under axial stress, damage occurs after a load has been applied, and immediately after damage, failure occurs.
4.2. Derivation of the Statistical Damage Constitutive Model
4.2.1. The Constitutive Equation of the Elastic Section of Uniaxial Tensile Concrete
4.2.2. The Constitutive Equation of the Inelastic Section in the Whole Process of Uniaxial Tensile Concrete
4.3. Parameter Determination of the Statistical Damage Constitutive Model
5. Verification of the Statistical Damage Constitutive Model of the AR-GFRC
5.1. Verification of Field Test
5.1.1. Project Overview and Field Test
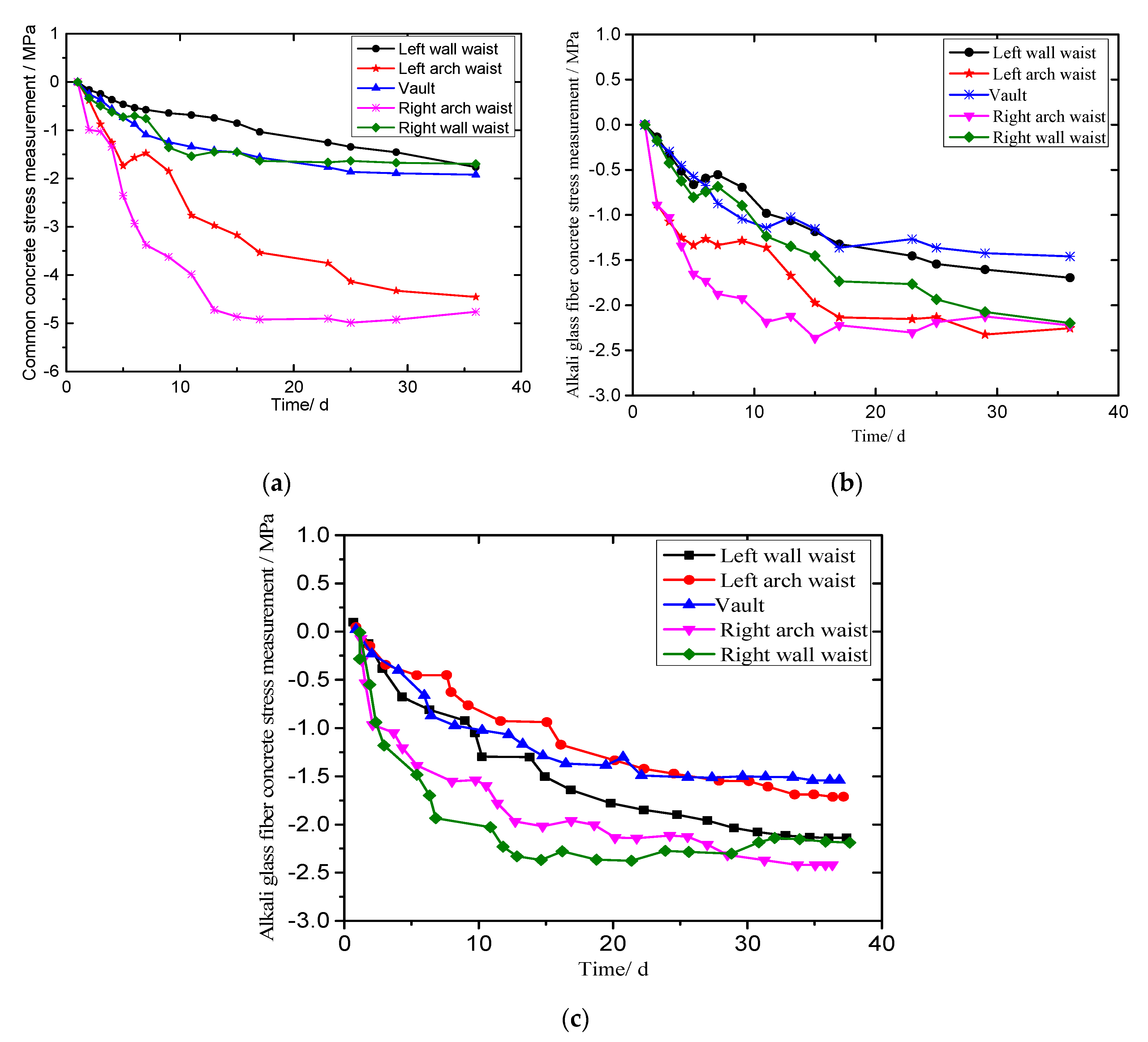
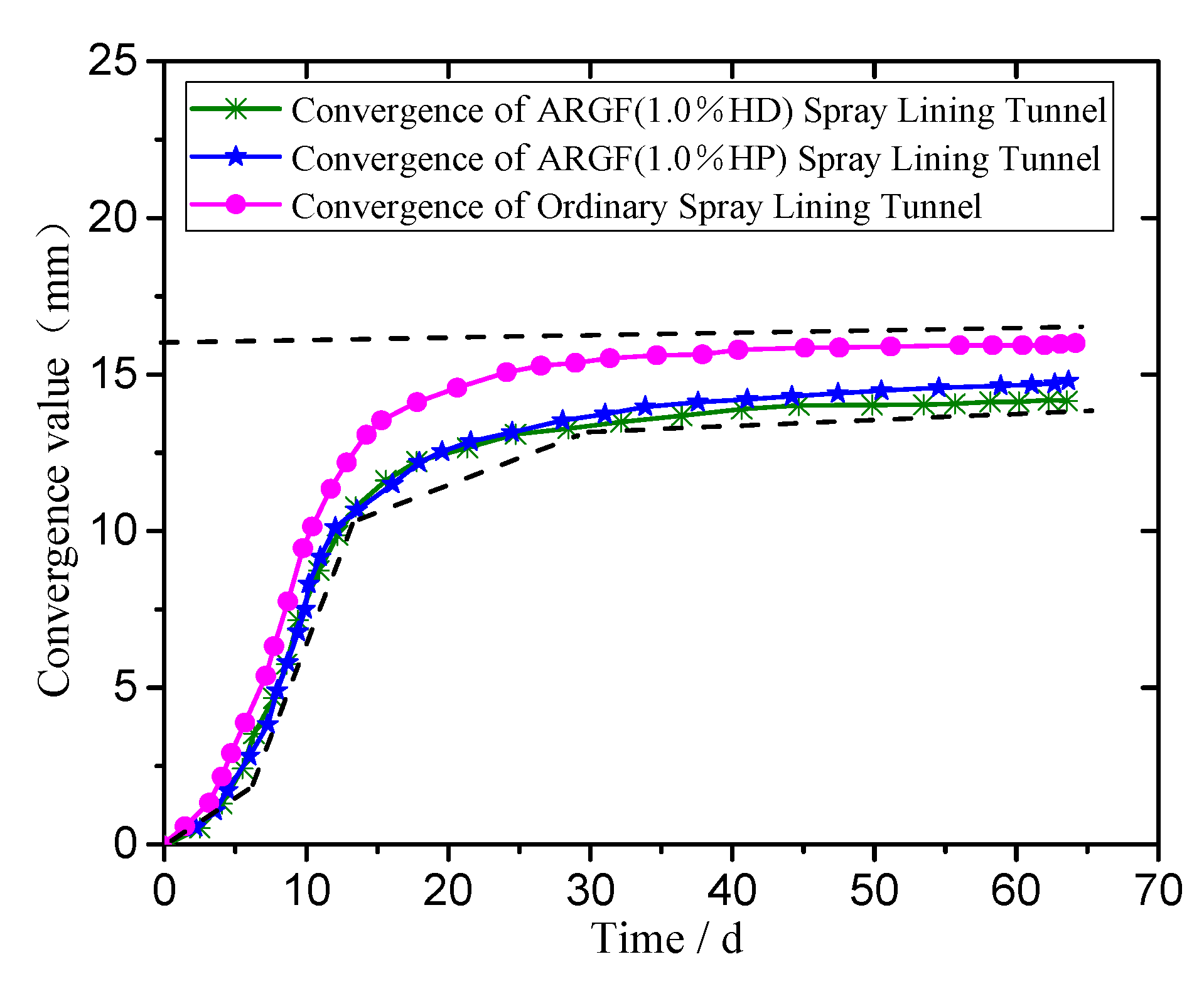
5.1.2. On-Site Deformation Monitoring and Result Analysis
- (1)
- Stress variation in concrete lining
- (2)
- Variation of the convergence value around the tunnel
5.2. Verification of the Theory and Laboratory Experiment
- (1)
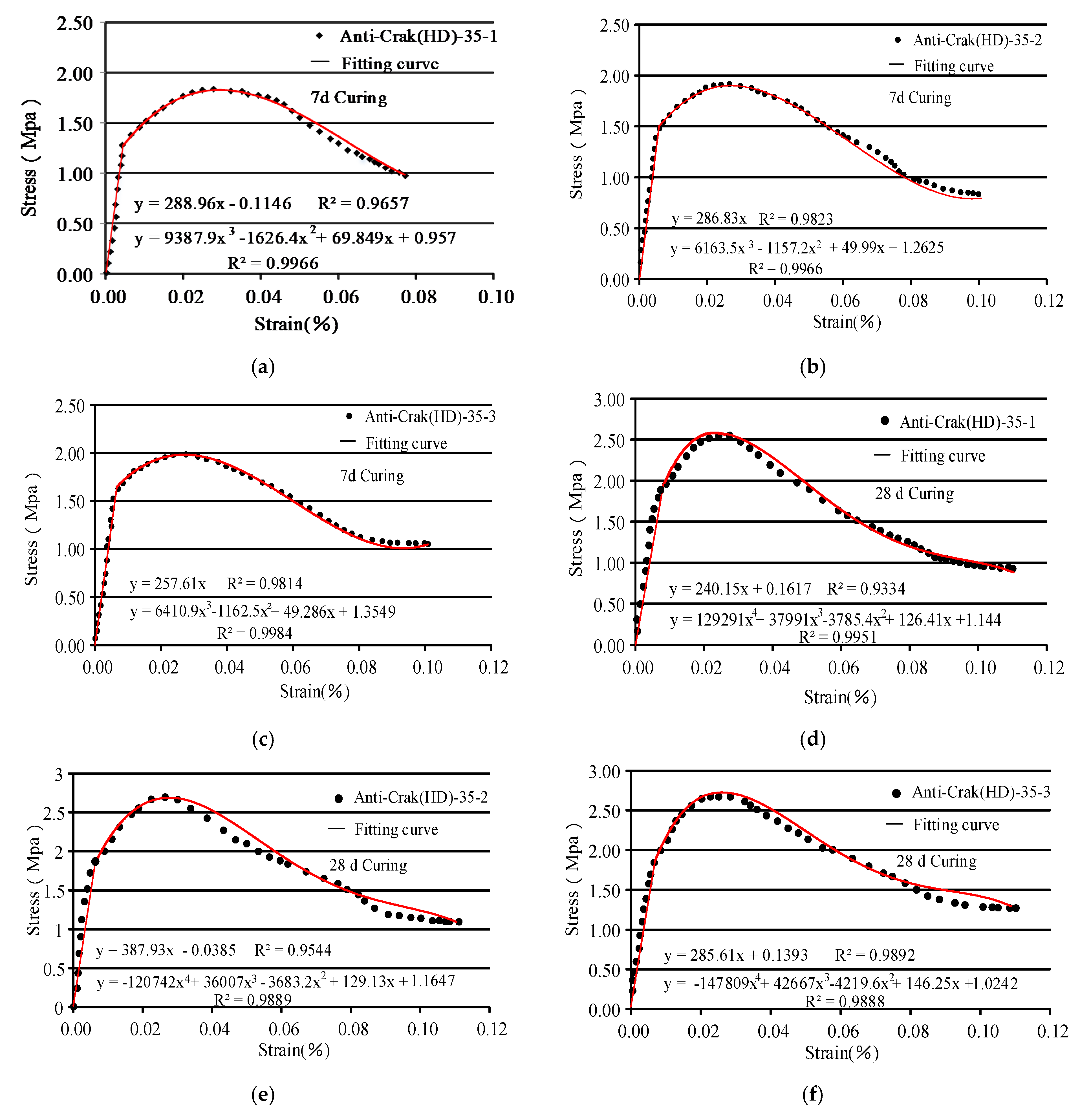
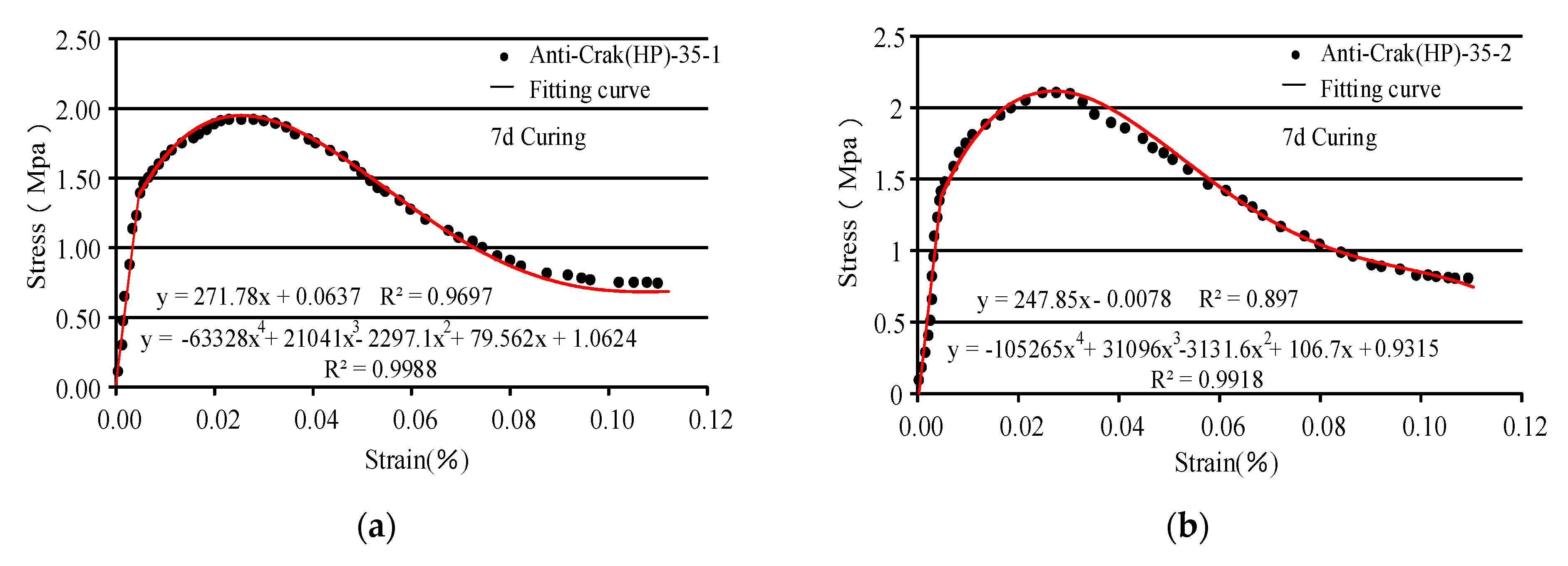
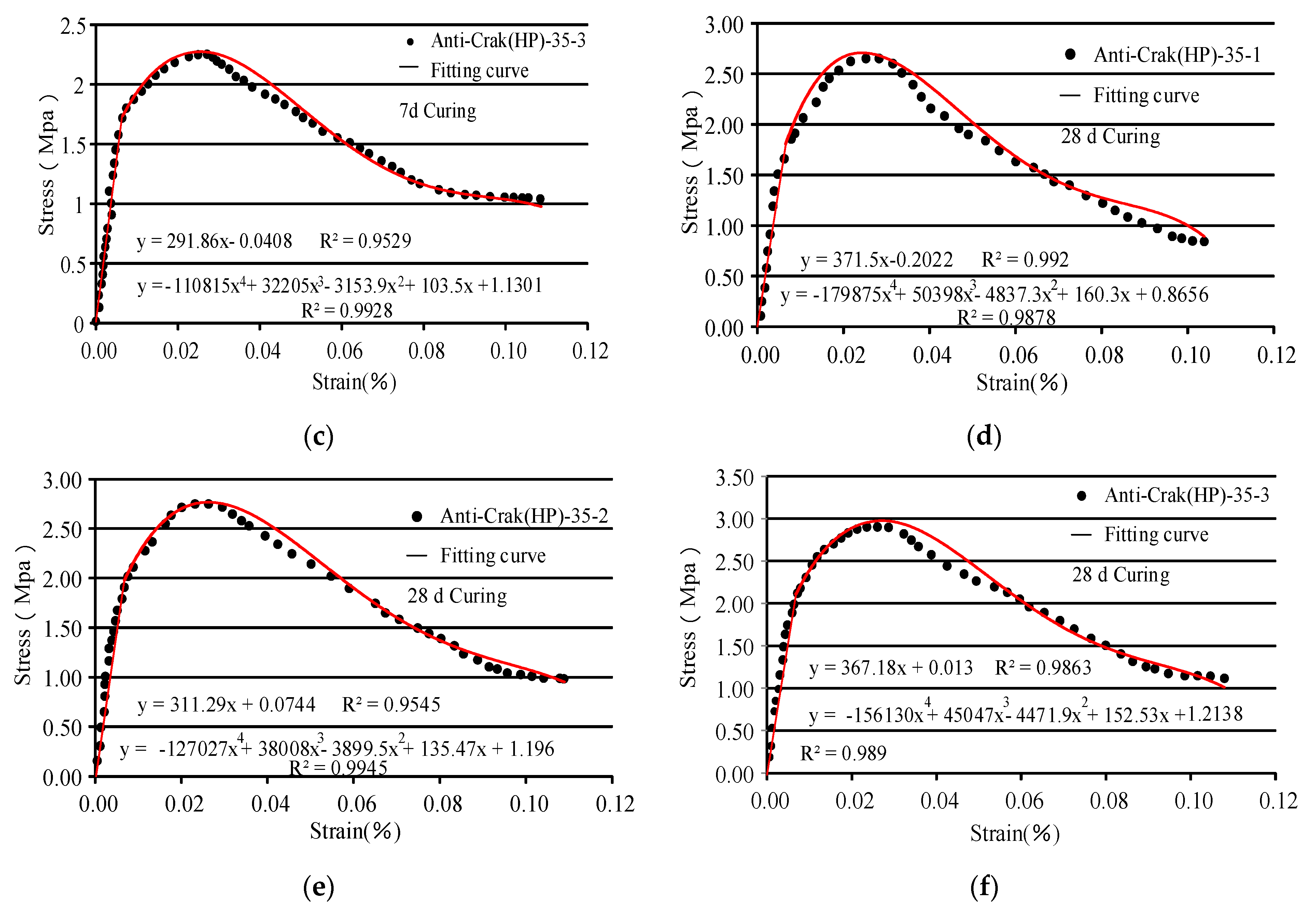
- The proposed constitutive model describes the post-peak damage evolution of the AR-GFRC and the trends and characteristics of the concrete strength in the stress state. The theoretical and experimental stress-strain curves are in good agreement for the different fiber contents. The early tensile strength of the concrete was improved by the addition of alkali-resistant glass fiber.
- (2)
- As shown in Figure 10a–f and Figure 11a–f, the proposed tensile statistical damage constitutive model of AR-GFRC can accurately describe the stress-strain evolution law of the different fiber contents of alkali-resistant glass fiber concrete in this concrete tensile test. The content of the alkali-resistant glass fiber doping range was considered 0 to 1.5%, the 7-day peak strength difference between HD and HP concrete under standard curing between the experimental results and the theoretical results is small, and the 28-d peak strength shows similar patterns of change. The theoretical equation curve is consistent with the indoor test data curve in the elastic stage, peak strength, and nonlinear change segment after its peak. Different types of fibers (HD and HP) have little effect on the theoretical and test curves. This shows that the proposed AR-GFRC tensile statistical damage model is also applicable to the description of the concrete stress-strain curve of HD and HP fibers.
6. Discussion
7. Conclusions
- (1)
- A new damage variable of the AR-GFRC was defined based on the results of the uniaxial tensile test and the stress-strain curve was redefined by taking into consideration the residual strength.
- (2)
- The meso-statistical damage theory, microcontinuum theory, and composite material theory were used to develop a statistical damage constitutive equation of the AR-GFRC based on a Weibull distribution. The calculation methods for determining the mechanical and statistical parameters of the concrete have been provided, and the research results can be used to provide reference data for practical concrete engineering.
- (3)
- The constitutive theoretical curve was verified in MATLAB based on the uniaxial tensile test data of the concrete. The theoretical and experimental stress-strain curves of the AR-GFRC were in good agreement.
- (4)
- The fiber contents (0.5%, 1%, and 1.5%) and the size and shape parameters in the constitutive equation were quantified, and it was determined that the proposed model was well suited to describe the microscopic damage evolution and failure mechanism of the concrete while considering the residual strength effects. The results provide a theoretical basis and have practical application value.
Author Contributions
Funding
Conflicts of Interest
References
- Fathi, H.; Lameie, T.; Maleki, M.; Yazdani, R. Simultaneous effects of fiber and glass on the mechanical properties of self-compacting concrete. Constr. Build. Mater. 2017, 133, 443–449. [Google Scholar] [CrossRef]
- Dimchev, M.; Caeti, R.; Gupta, N. Effect of carbon nanofibers on tensile and compressive characteristics of hollow particle filled composites. Mater. Des. 2010, 31, 1332–1337. [Google Scholar] [CrossRef]
- Takeda, K.; Nakashima, E. Nuclear Power and Environmental Concerns in the Aftermath of 3/11 Fukushima Daiichi Nuclear Power Plant Accident in Japan. In Globalization, Development and Security in Asia: Environment and Sustainable Development in Asia; World Scientific: Singapore, 2014. [Google Scholar]
- Keleştemur, O.; Arıcı, E.; Yıldız, S.; Gökçer, B. Performance evaluation of cement mortars containing marble dust and glass fiber exposed to high temperature by using Taguchi method. Constr. Build. Mater. 2014, 60, 17–24. [Google Scholar] [CrossRef]
- Tassew, S.T.; Lubell, A.S. Mechanical properties of glass fiber reinforced ceramic concrete. Constr. Build. Mater. 2014, 51, 215–224. [Google Scholar] [CrossRef]
- Kizilkanat, A.B.; Kabay, N.; Akyüncü, V.; Chowdhury, S.; Akça, A.H. Mechanical properties and fracture behavior of basalt and glass fiber reinforced concrete: An experimental study. Constr. Build. Mater. 2015, 100, 218–224. [Google Scholar] [CrossRef]
- Yuwaraj, M.G.; Santosh, B.D. Performance of Alkali-resistant Glass Fiber Reinforced Concrete. J. Reinf. Plast. Compos. 2006, 25, 617–630. [Google Scholar]
- Yildizel, S.A.; Ozturk, A.U. Micro Glass Fiber Reinforced Concrete. ICOCEE CESME 2018, 4, 24–27. [Google Scholar]
- Kwan, W.H.; Cheah, C.B.; Ramli, M.; Chang, K.Y. Alkali-resistant glass fiber reinforced high strength concrete in simulated aggressive environment. Materiales de Construcción 2018, 68, 1–14. [Google Scholar] [CrossRef]
- Chandramouli, K.; Rao, P.S.; Pannirselvam, N.; Sekhar, T.S.; Sravana, P. Chloride Penetration Resistance Studies on Concretes Modified with Alkali Resistant Glass Fibers. Am. J. Appl. Sci. 2010, 7, 371–375. [Google Scholar] [CrossRef][Green Version]
- Yurdakul, A.; Dölekçekiç, E.; Karasu, B.; Günkaya, G. Characterization of Commercially Available Alkali Resistant Glass Fiber for Concrete Reinforcement and Chemical Durability Comparison with SrO-Mn2O3-Fe2O3-MgO-ZrO2-SiO2(SMFMZS SYSTEM GLASSES). Anadolu Univ. J. Sci. Technol. A Appl. Sci. Eng. 2012, 13, 95–102. [Google Scholar]
- Qin, X.; Li, X.; Cai, X. The applicability of alkaline-resistant glass fiber in cement mortar of road pavement: Corrosion mechanism and performance analysis. Int. J. Pavement Res. Technol. 2017, 10, 536–544. [Google Scholar] [CrossRef]
- Kumar, J.D.C.; Abhilash GV, S.; Khan, P.K.; Manikantasai, G.; Tarakaram, V. Experimental Studies on Glass Fiber Concrete. Am. J. Eng. Res. 2016, 5, 100–104. [Google Scholar]
- Yıldızel, S.A. Effects of Barite Sand Addition on Glass Fiber Reinforced Concrete Mechanical Behavior. Int. J. Eng. Appl. Sci. 2017, 9, 100–105. [Google Scholar] [CrossRef][Green Version]
- Krishnan, K.A.; Anjana, R.; George, K.E. Effect of alkali-resistant glass fiber on polypropylene/polystyrene blends: Modeling and characterization. Polym. Compos. 2014, 37, 398–406. [Google Scholar] [CrossRef]
- Oh, H.S.; Moon, D.Y.; Kim, S.D. An Investigation on Durability of Mixture of Alkali-Resistant Glass and Epoxy for Civil Engineering Application. Procedia Eng. 2011, 14, 2223–2229. [Google Scholar] [CrossRef][Green Version]
- Dougill, J.W. On stable progressively fracturing solids. Zeitschrift für Angewandte Mathematik und Physik ZAMP 1976, 27, 432–437. [Google Scholar] [CrossRef]
- Lemaitre, J. Coupled elasto-plasticity and damage constitutive equations. Comput. Methods Appl. Mech. Eng. Fr. 1985, 51, 31–49. [Google Scholar] [CrossRef]
- Kandarpa, S.; Krikner, D.J.; Spencer, B.F. Stochastic damage model for brittle materials subjected to monotonic loading. J. Eng. Mech. ASCE 1996, 122, 788–795. [Google Scholar] [CrossRef]
- Krajcinovic, D.; Silva, M.A.G. Statistical aspects of the continuous damage theory. Int. J. Solids Struct. 1982, 18, 551–562. [Google Scholar] [CrossRef]
- Krajcinovic, D. Constitutive equations for damaging materials. J. Appl. Mech. 1983, 50, 355–360. [Google Scholar] [CrossRef]
- Breysse, D. Probabilistic formulation of damage-evolution law of cementious composites. J. Eng. Mech. 1990, 1169, 1489–1511. [Google Scholar] [CrossRef]
- Guo, Y.; Kuang, Y. An elastoplastic micro-mechanical damage model for quasi-brittle materials under uniaxial loading. Int. J. Damage Mech. 2019, 28, 1191–1202. [Google Scholar] [CrossRef]
- Bakhshi, M.; Mobasher, B. Simulated shrinkage cracking in the presence of Alkali Resistant Glass fibers. Spec. Publ. 2011, 280, 1–14. [Google Scholar]
- Eiras, J.N.; Kundu, T.; Bonilla, M. Nondestructive Monitoring of Ageing of Alkali Resistant Glass Fiber Reinforced Cement (GRC). J. Nondestruct. Eval. 2013, 32, 300–314. [Google Scholar] [CrossRef]
- Lemaitre, J. How to use damage mechanics. Nucl. Eng. Des. 1984, 80, 233–245. [Google Scholar] [CrossRef]
- Wengui, C.; Sheng, Z.; Minghua, Z. Study on a statistical damage constitutive model with conversion between softening and hardening properties of rock. Eng. Mech. 2006, 23, 110–115. [Google Scholar]
- Weiya, X.; Lide, W. Study on statistical damage constitutive model of rock. Chin. J. Rock Mech. Eng. 2002, 21, 787–791. [Google Scholar]
- Mehta, P.K.; Aitcin, P.C. Microstructural basis of selection of materials and mix proportions for high-strength concrete. Spec. Publ. 1990, 121, 265–286. [Google Scholar]
- Alireza, A.; Mehdi, G.; Akram, F. Glass fber-reinforced epoxy composite with surface modifed graphene oxide: Enhancement of interlaminar fracture toughness and thermo-mechanical performance. Polym. Bull. 2018. [Google Scholar] [CrossRef]
- Abeysinghe, C.M.; Thambiratnam, D.P.; Perera, N.J. Flexural performance of an innovative hybrid composite floor plate system comprising glass–fibre reinforced cement, polyurethane and steel laminate. Compos. Struct. 2013, 95, 179–190. [Google Scholar] [CrossRef][Green Version]
- Zhu, Z.; Zhang, C.; Meng, S.; Shi, Z.; Tao, S.; Zhu, D. A Statistical Damage Constitutive Model Based on the Weibull Distribution for Alkali-Resistant Glass Fiber Reinforced Concrete. Materials 2019, 12, 1908. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Zhu, Z.; Zhu, S.; He, Z.; Zhu, D.; Liu, J.; Meng, S. Nonlinear Creep Damage Constitutive Model of Concrete Based on Fractional Calculus Theory. Materials 2019, 12, 1505. [Google Scholar] [CrossRef] [PubMed]
- Yang, W.; Xue, M. Test method and equipment of stress-strain curves of concertein in direct tension. Ind. Constr. 2009, 39, 907–909. [Google Scholar]
- Chen, Y.; Du, C.; Zhou, W. Experimental study on the complete stress-deformation curve of full grade concrete under axial tension. J. Hydroelectr. Eng. 2010, 29, 76–81. [Google Scholar]
- Sun, F. Experimental Research for Complete Stress-Deformation Curve of Concrete in Uniaxial Tension. Master’s Thesis, Hohai University, Nanjing, China, 2007. [Google Scholar]
- Wu, N.; Zhang, C.; Maimaitiyusupu, S.; Zhu, Z. Investigation on Properties of Rock Joint in Compression Dynamic Test. KSCE J. Civ. Eng. 2019, 23, 3854–3863. [Google Scholar] [CrossRef]
- He, Z. The Research on Nonlinear Rheological Mechanics Models of Deep-Buried Tunnel Surrounding Rock and Its Application; HoHai University: Nanjing, China, 2018. [Google Scholar]
- Skrzypacz, P.; Nurakhmetov, D.; Wei, D. Generalized stiffness and effective mass coefficients for power-law Euler–Bernoulli beams. Acta Mech. Sin. 2020, 36, 160–175. [Google Scholar] [CrossRef]
- Eissa, F.H. Stress-Strength Reliability Model with the Exponentiated Weibull Distribution: Inferences and Applications. Int. J. Stat. Probab. 2018, 7. [Google Scholar] [CrossRef]
- Kasagani, H.; Rao, C.B.K. Effect of Short length Glass Fiber on dilated concrete in Compression and Tension. Proc. Inst. Civ. Eng. Struct. Build. 2018, 4, 1–12. [Google Scholar]
- Cao, R.L.; He, S.H.; Wei, J.; Wang, F. Study of modified statistical damage softening constitutive model for rock considering residual strength. Rock Soil Mech. 2013, 34, 1652–1661. [Google Scholar]
- Hilles, M.M.; Ziara, M.M. Mechanical behavior of high strength concrete reinforced with glass fiber. Eng. Sci. Technol. Int. J. 2019, 22, 920–928. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Nasiri, S.; Anders, D.; Weinberg, K. Prediction of dynamic properties of ultra-high performance concrete by an artificial intelligence approach. Adv. Eng. Softw. 2019, 127, 51–58. [Google Scholar] [CrossRef]
- Brandon, F.; Pedram, S. Contribution of Longitudinal GFRP Bars in Concrete Cylinders under Axial Compression. Can. J. Civ. Eng. 2018, 45, 458–468. [Google Scholar]
- Yu, W.; Xue, H.; Qian, M. Tensile and compressive properties of epoxy syntactic foams reinforced by short glass fiber. Indian J. Eng. Mater. Sci. 2017, 24, 283–289. [Google Scholar]
- Sun, W.; Zuo, Y.; Wang, S.; Wu, Z.; Liu, H.; Zheng, L.; Lou, Y. Pore structures of shale cores in different tectonic locations in the complex tectonic region: A case study of the Niutitang Formation in northern Guizhou, Southwest China. J. Nat. Gas Sci. Eng. 2020, 80, 103398. [Google Scholar] [CrossRef]
- Sun, W.; Zuo, Y.; Wu, Z.; Liu, H.; Zheng, L.; Wang, H.; Shui, Y.; Lou, Y.; Xi, S.; Li, T.; et al. Pore characteristics and evolution mechanism of shale in a complex tectonic area: Case study of the Lower Cambrian Niutitang Formation in Northern Guizhou, Southwest China. J. Pet. Sci. Eng. 2020, 193, 107373. [Google Scholar] [CrossRef]





| Type | Length/mm | Equivalent Diameter/um | Fracture Strength | Elongation at Break/% | Modulus/GPa | Melting Point/°C |
|---|---|---|---|---|---|---|
| Anti-Crak®HD | 6/12 | 14 | 1700 | 3.6 | 72 | 1580 |
| Anti-Crak®HP | 6/12 | 700 | 1700 | 3.6 | 72 | 1580 |
| Sieve Hole Size | 31.5 | 26.5 | 16.0 | 4.75 | 2.36 |
|---|---|---|---|---|---|
| Actual cumulative percentage of sieve remainder/% | 0 | 0~5 | 30~70 | 90~95 | 95~100 |
| Number | Name | Cement | Mineral Powder | Fly Ash | Sand | Stone | Water | Admixture | Fiber Contents (%) |
|---|---|---|---|---|---|---|---|---|---|
| C35 | Concrete (without fiber) | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 0 |
| HD-35-1 | Anti-Crak® (HD) Concrete | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 0.5 |
| HD-35-2 | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 1 | |
| HD-35-3 | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 1.5 | |
| HP-35-1 | Anti-Crak® (HP) Concrete | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 0.5 |
| HP-35-2 | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 1 | |
| HP-35-3 | 245 | 100 | 95 | 735 | 1040 | 175 | 10.5 | 1.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, X.; Zhang, C.; Zhou, X. The Statistical Damage Constitutive Model of the Mechanical Properties of Alkali-Resistant Glass Fiber Reinforced Concrete. Symmetry 2020, 12, 1139. https://doi.org/10.3390/sym12071139
Shi X, Zhang C, Zhou X. The Statistical Damage Constitutive Model of the Mechanical Properties of Alkali-Resistant Glass Fiber Reinforced Concrete. Symmetry. 2020; 12(7):1139. https://doi.org/10.3390/sym12071139
Chicago/Turabian StyleShi, Xianzeng, Cong Zhang, and Xingde Zhou. 2020. "The Statistical Damage Constitutive Model of the Mechanical Properties of Alkali-Resistant Glass Fiber Reinforced Concrete" Symmetry 12, no. 7: 1139. https://doi.org/10.3390/sym12071139
APA StyleShi, X., Zhang, C., & Zhou, X. (2020). The Statistical Damage Constitutive Model of the Mechanical Properties of Alkali-Resistant Glass Fiber Reinforced Concrete. Symmetry, 12(7), 1139. https://doi.org/10.3390/sym12071139





