Planetary Systems and the Hidden Symmetries of the Kepler Problem

Abstract

The question of whether the solar distances of the planetary system follow a regular sequence was raised by Kepler more than 400 years ago. He could not prove his expectation, inasmuch as the planetary orbits are not transformed into each other by the regular polyhedra. In 1989, Barut proposed another relation, which was inspired by the hidden symmetry of the Kepler problem. It was found to be approximately valid for our Solar System. Here, we investigate if exoplanet systems follow this rule. We find that the symmetry-governed sequence is valid in several systems. It is very unlikely that the observed regularity is by chance; therefore, our findings give support to Kepler’s guess, although with a different transformation rule.

1. Introduction

Kepler’s laws of planetary motion [1,2] played an essential role not only in the celestial mechanics, but also in the foundation of physics. Newton discovered the gravitational force based on these laws. However, there was still an expectation by Kepler which was not fulfilled: he was looking for the proportions of perfection in the sequence of planets [2,3]. He expected to find Divine Harmony in the distances of the planets. In particular, he thought that the orbits of the six planets (known at his time) are related to each other by inserting the five regular polyhedra between them. In other words, he was looking for a transformation, which determines the outer planetary orbitals from that of the first one. He could not prove this hypothesis.

Newton’s gravitational force explains all the laws of planetary motion, of course. However, the question of where should the planets move around the Sun is not answered by the Newtonian mechanics. It is determined by the complicated initial conditions; therefore, their distances could be quite different as well.

Empirical rules, such as the Bode formula [4,5], revealed some regularity later on, but neither their theoretical background nor their practical application in associating the sequence number to the planets was completely convincing (see below for more details).

In the late 20th century, Barut proposed a new relation [6], which was inspired by the hidden symmetries of the Kepler problem. He realized that a space and time dilatation, which corresponds to the O(4,2) dynamical group, transforms the planetary orbits into each other. He found that the distances of the planets in our Solar System follow this rule to a good approximation. Nevertheless, his proposal is not very widely accepted.

With the advent of the discovery of the exoplanets, we are in the situation where the problem can be investigated in several systems. By doing so, the present work is meant to be a contribution to this long-standing discussion.

In what follows, we first recall some transformation rules between the planetary orbits, with special attention on Barut’s proposal. Although formally this latter approach is similar to the Bode-type rules, it has two very interesting new aspects: (i) It is not empirical, rather it is inspired by the hidden symmetries of the Kepler problem. (ii) The key concept is that of the dynamical algebra, which was originally introduced for quantum systems, but here it is applied in celestial mechanics. Then, we review very briefly the hierarchy of symmetries of the Kepler problem. Afterwards (in Section 3), we investigate some exoplanet systems in order to test if their observed characteristics follow the symmetry-inspired regularity. Finally, some discussion follows.

2. Methods

2.1. Transformations of Planetary Orbits

The best known rule of this kind is the Bode formula [4,5], which gives the semi-major axis of the planetary orbits:

$$R=0.4+0.3\times 2^n$$

(1)

in astronomical units (1 A.U. is the semi-major axis of Earth’s orbit). The sequence numbers (n) for the planets

Me, Mercury; V, Venus; E, Earth; Ma, Mars; A, Asteroids; J, Jupiter; S, Saturn; U, Uranus; N, Neptune; P, Pluto, are as follows:

• $-\infty$:Me, 0:V, 1:E, 2:Ma, 3:A, 4:J, 5:S, 6:U, 7:P.

It does not account for the orbit of Neptune. As it is seen, the numbers are not regular. (Although Pluto is not considered today as a planet [7], we mention it here due to the fact that the historical applications of the transformations rules included it.)

Another rule was derived [8] from the equation of motion

$$\frac{m{v}^2}{R}=\frac{GMm}{R^2},v_n^2{R}_n=GM$$

(2)

by requiring a quantization (of the angular momentum per unit mass)

$$v_n{R}_n=n\sigma .$$

(3)

This approach has some similar features to the Bode rule: a good fit can be obtained, but the n values are somewhat arbitrary, and there is no reason for the quantization.

Later, it was realized that the Bode rule is related to the rotational and scale invariance [9], and it was shown that one can obtain this kind of rule from a disk model [10]. Ragnarsson invented a new law [11], in which Jupiter plays a central role, in particular the logarithmic distances of the planets show a symmetry around this planet. Further empirical rules were also applied (e.g., [12]). The significance of this kind of rule was investigated statistically in [13].

Here, we concentrate on the proposal of Barut [6], which was inspired by the hidden symmetries of the Kepler problem. He found that the logarithms of the planetary velocities, periods, and distances follow straight lines as a function of the natural sequence number of the planets:

• 1:Me, 2:V, 3:E, 4:Ma, 5:A, 6:J, 7:S, 8:U, 9:N, 10:P.

He also realized that the simple time and space dilatation

$$t\to e^{3\lambda n}t,x\to e^{2\lambda n}x$$

(4)

which gives the equations

$$\begin{array}{c}log{v}_n=log{v}_0-\lambda n,\\ log{R}_n=log{R}_0+2\lambda n,\\ log{T}_n=log{T}_0+3\lambda n,\end{array}$$

(5)

connect the orbitals to each other. Here, $\lambda$ is a constant, but with this single parameter we can predict any planetary orbital, if we know one of them.

The dilatation (4) is a transformation, which is suggested by the O(4,2) dynamical group of the Kepler problem [6].

Therefore, Barut’s proposal gives the transformation required by Kepler’s guess: it determines the planetary orbitals from the first one (in a simple sequence), and it has a theoretical connection to the hidden symmetry of the Kepler problem.

2.2. Symmetries of the Kepler Problem

The Kepler problem is of profound importance in the quantum mechanics, too, known as the hydrogen atom. The revealing of its symmetries had contributions both from the classical and from the quantum mechanics. Here, we consider the bound state problem, i.e., the energy is negative and the orbit is elliptical.

Geometrical symmetry: The obvious symmetry of the Kepler problem is that of the rotation in the three-dimensional space: O(3), which leaves invariant the $x_1^2+x_2^2+x_3^2$ quadratic form of the coordinates $x_i$. As a consequence, the angular momentum is conserved. This symmetry is called geometrical symmetry because it transforms the geometrical variables, i.e., space coordinates, into each other (and does not mix them with others, e.g., with the momenta). It leaves invariant not only the total Hamiltonian, but also its kinetic and potential parts [14].

Dynamical symmetry: In addition to the angular momentum, the Kepler problem has another conserved vector, too, called the Laplace or Runge–Lenz vector. The six constants of motion generate the O(4) group, which leaves invariant the $x_1^2+x_2^2+x_3^2+x_4^2$ quadratic form [14]. In contrast with the O(3) symmetry, however, not all the O(4) transformations leave the kinetic and potential energy invariant, separately; only the total energy is conserved. The O(4) symmetry is specific for the $1/r$ potential; it is called dynamical symmetry.

The hidden O(4) symmetry was found by Fock [15] and Bargman [16], and Györgyi could construct the coordinate space of the O(4) transformations [17,18]. He gave a detailed four-dimensional description of the problem, and his equations describe an inertia motion on a four-dimensional sphere. There, the orbits are perfect, i.e., they are circles. Furthermore, the problem is reduced to a kinematical one. The gravitational force of the three-dimensional treatment disappears; instead, we see an inertia motion in curved space (in particular, on a sphere) in the four-dimensional space.

Another four-dimensional treatment was presented by Wulfman [19] in the language of the more recent (geometrized) Hamiltonian mechanics.

The elements of the symmetry group connect the orbitals of the same energy.

Dynamical group: The dynamical group connects the orbitals of different energy, too. It is generated by all the constants of motion, including the ones with explicit time dependence [20]. (Note that the total time derivative is obtained as the sum of the partial derivative and the Poisson bracket; therefore, even quantities with nonzero partial derivative may have zero total derivative.) The symmetry group (as a subgroup of the dynamical group) is generated by the constants of motion without explicit time dependence.

The dynamical group of the Kepler problem is O(4,2) [6,14], which leaves invariant the $x_1^2+x_2^2+x_3^2+x_4^2-x_5^2-x_6^2$ quadratic form.

This group found its first physical application as a global or external space-time transformation. In particular, the Maxwell equations are invariant with respect to the conformal group, which is a particular nonlinear realization of O(4,2). It builds up as follows. To the six generators of the O(3,1) Lorentz group, one adds four generators of transformation that result in the Poincare group or inhomogeneous Lorentz group IO(3,1). The inclusion of the inversion, a single discrete operation, requires the addition of five new generators. Four are in the form of a 4-vector, and the fifth one is a scalar, the dilatation.

O(4,2) appears also as the dynamical group of a rest frame system having internal degrees of freedom. From the quantum mechanical viewpoint, the problem of finding the dynamical algebra of the Kepler problem (H atom) appears in the following way. The states are labeled by the quantum numbers $|nlm\rangle$. We must find an algebra that contains the O(4) symmetry algebra as a subalgebra and includes operators that ladder n and l [14]. The incorporation of states with different energy requires O(4,1), and O(4,2) is necessary to include continuum states and transition operators.

The external and internal O(4,2) groups were combined in [21]. The authors described the system of the two bodies in a six-dimensional space. The equations of motion are rotational invariant in the six-dimensional space, i.e., they are conformally invariant in the four dimensional Minkowsky space. The total algebra is the direct sum of the conformal algebra in the space of external position coordinates and the dynamical algebra in the space of the internal position coordinates. This surprising and interesting interconnection between the two roles of the O(4,2) led Barut to apply the space and time dilations of Equation (4), which take the planetary orbitals into each other, as described by Equation (5).

For a recent review on the symmetries of the H-atom (Kepler problem), we refer to the work of Maclay [22].

3. Results

The tests were carried out on selected exoplanet systems taken from the NASA Exoplanet Archive [23]. The following selection criteria were applied: the observed datasets contain the data of the semi-major axis of the orbit, the orbital period, and the eccentricity for each planet in the systems. It was also preferable to know the masses of the planets besides that of the star. Systems with 5–7 planets are represented in the selection. The range of semi-major axes is obviously much narrower than that of the Solar System but the selection contains systems of different sizes.

The data are summarized in

Table 1. Planetary data.

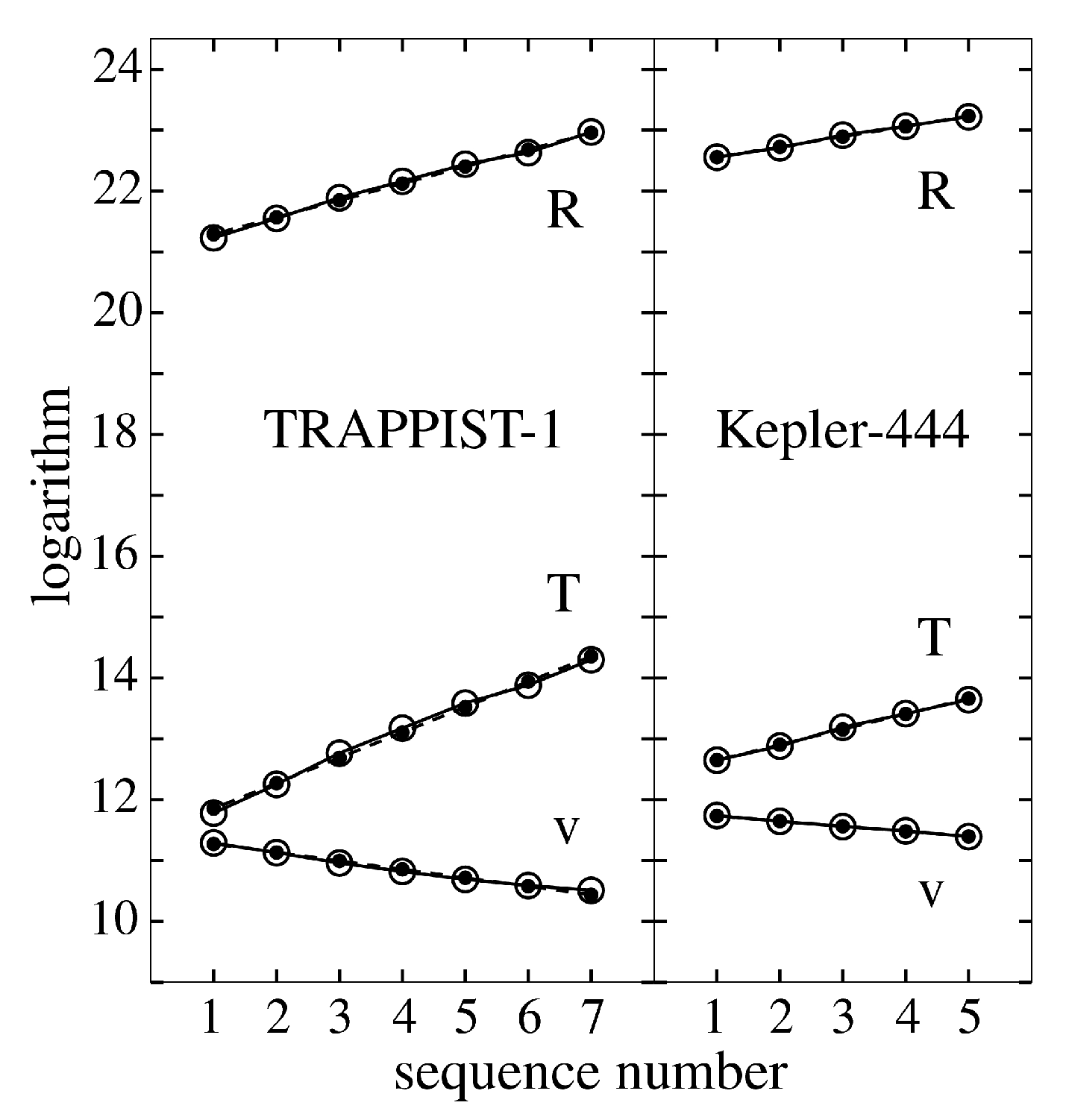
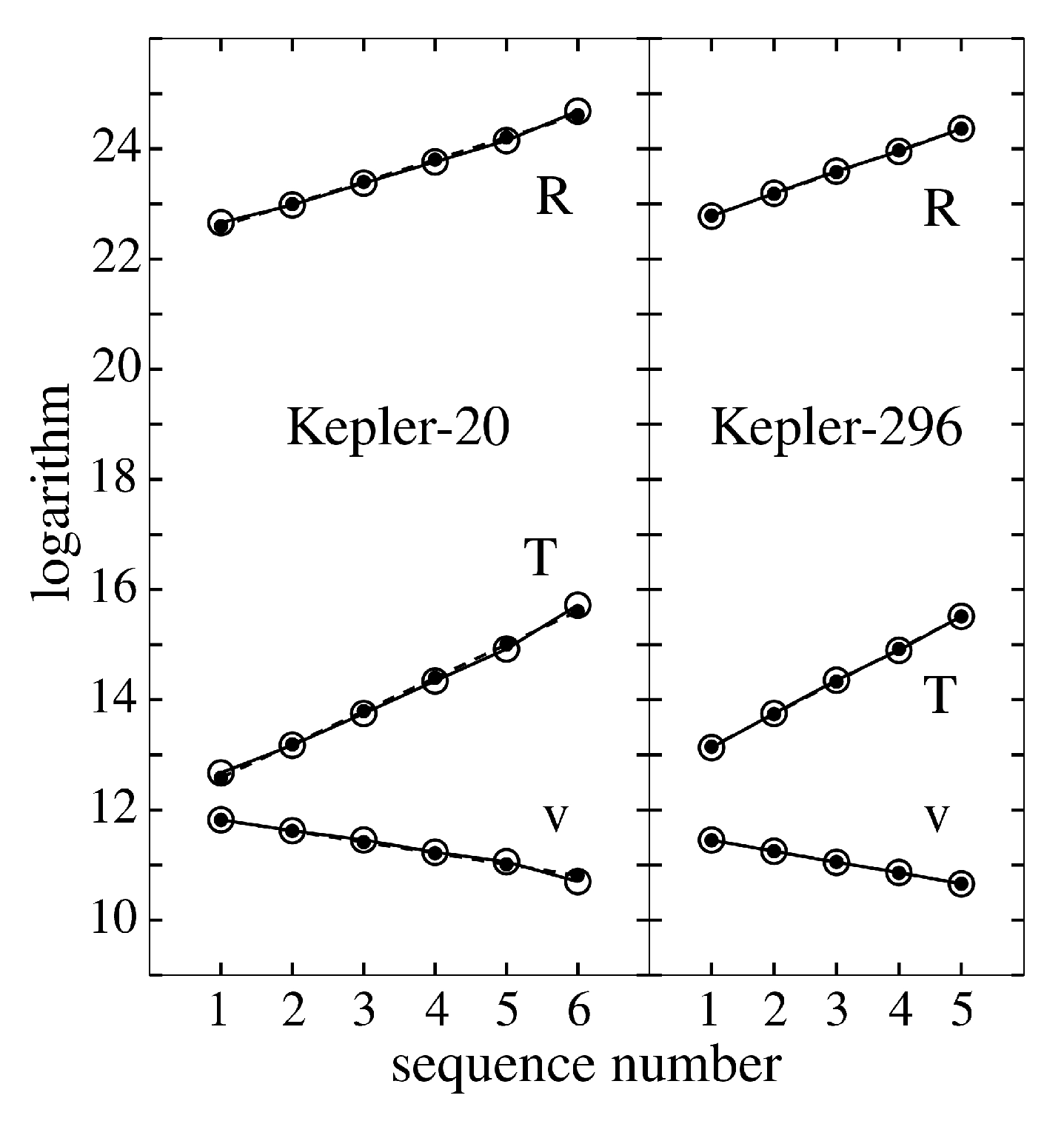
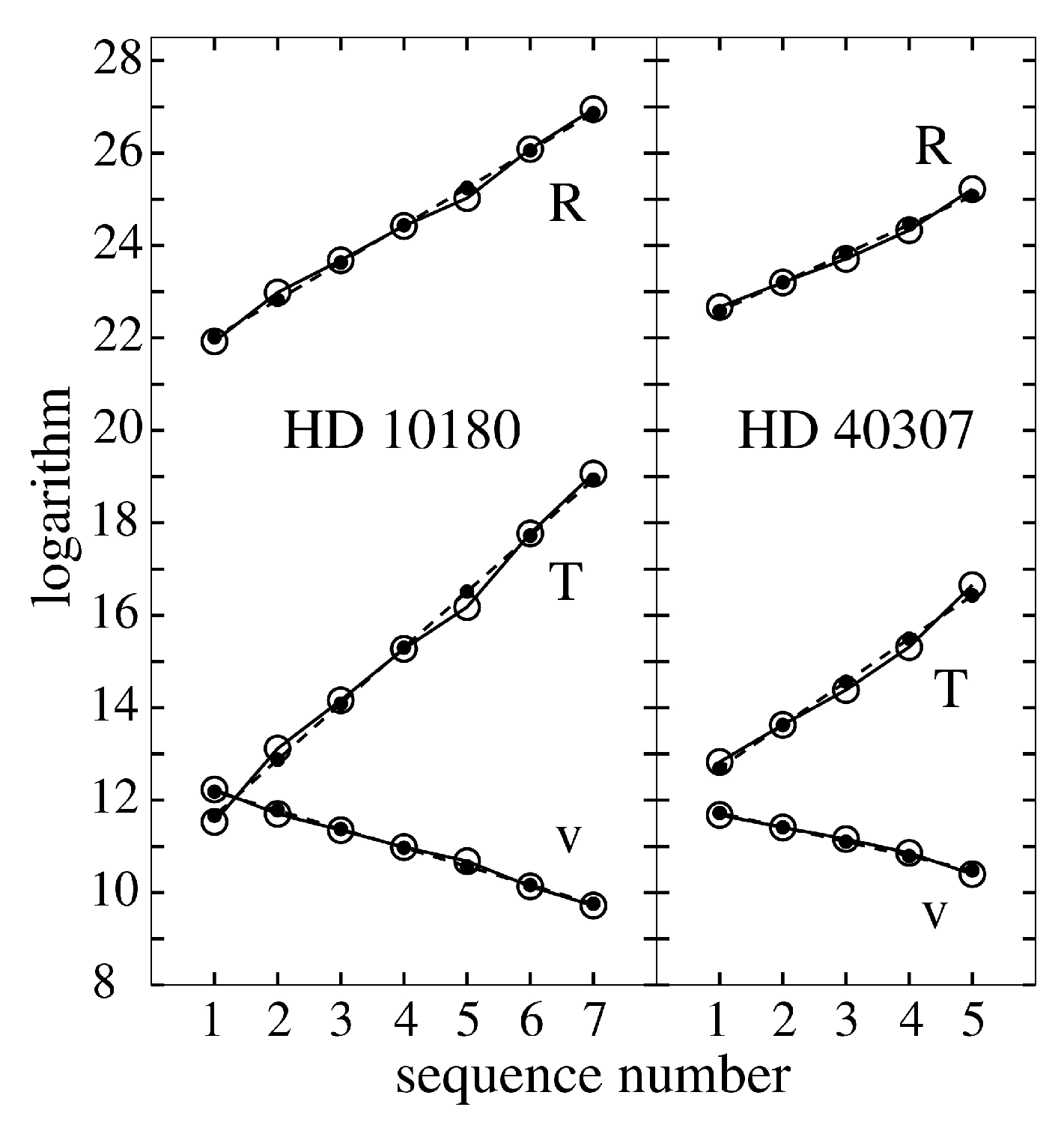
Planets	T (Day)	Smax (AU)	ecc.
TRAPPIST-1b	1.51087	0.0111	0.081
TRAPPIST-1c	2.42182	0.0152	0.083
TRAPPIST-1d	4.04961	0.0214	0.070
TRAPPIST-1e	6.09962	0.0282	0.085
TRAPPIST-1f	9.20669	0.0371	0.063
TRAPPIST-1g	12.35294	0.0451	0.061
TRAPPIST-1h	18.76700	0.0630	0.000
HD 10,180 b	1.17766	0.0222	0.001
HD 10,180 c	5.75969	0.0641	0.073
HD 10,180 d	16.35700	0.1286	0.131
HD 10,180 e	49.74800	0.2699	0.051
HD 10,180 f	122.74400	0.4929	0.119
HD 10,180 g	604.67000	1.4270	0.263
HD 10,180 h	2205.00000	3.3810	0.095
HD 40,307 b	4.31230	0.0468	0.200
HD 40,307 c	9.61840	0.0799	0.060
HD 40,307 d	20.43200	0.1321	0.070
HD 40,307 f	51.76000	0.2470	0.020
HD 40,307 g	197.80000	0.6000	0.290
Kepler-20 b	3.69612	0.0463	0.030
Kepler-20 e	6.09852	0.0639	0.280
Kepler-20 c	10.85409	0.0949	0.160
Kepler-20 f	19.57758	0.1396	0.320
Kepler-20 g	34.94000	0.2055	0.150
Kepler-20 d	77.61130	0.3506	0.600
Kepler-296 c	5.84164	0.0521	0.330
Kepler-296 b	10.86438	0.0790	0.330
Kepler-296 d	19.85029	0.1180	0.330
Kepler-296 e	34.14211	0.1690	0.330
Kepler-296 f	63.33627	0.2550	0.330
Kepler-444 b	3.60011	0.0418	0.160
Kepler-444 c	4.54588	0.0488	0.310
Kepler-444 d	6.18939	0.0600	0.180
Kepler-444 e	7.74349	0.0696	0.100
Kepler-444 f	9.74049	0.0811	0.290

, where the planets are ordered according to their central distances. The observed data of orbital periods (T) and central distances (smax: semi-major axes, i.e., R in Equation (5)) were converted to SI units (seconds and meters) and the mean orbital speeds were computed by using the formula [24]:

$$v_0=\frac{2\pi R}{T}[1-\frac{1}{4}{e}^2-\frac{3}{64}{e}^4-\frac{5}{256}{e}^6-\frac{175}{\mathrm{16,384}}{e}^8].$$

(6)

The parameters were obtained from a least square fit to the observed data. Each system has specific values of $\lambda$ and ($R_0,T_0,v_0$), but $\lambda$ was determined from a simultaneous fit to ($R,T,v$). The logarithm of the observed and calculated values of R, T, and v are shown in Figure 1, Figure 2 and Figure 3.

4. Conclusions

As illustrated by the figures, the observed data of the planetary motion seem to follow a symmetry-inspired regularity. In particular, they are in line with the transformation rules described by Equations (4) and (5), obtained from the dynamical group of the Kepler problem [6]. It is hard to believe that this coincidence is completely by chance. Therefore, our finding seems to support the historical conjecture by Kepler on the regular sequence of the planetary distances (although with a different transformation rule). Obviously, much work remains to be done before a really conclusive answer can be obtained. We leave the systematic study for later investigations; nevertheless, it is worth mentioning that preliminary studies indicate similar regularity in other systems, too. The purpose of the present short paper is to draw attention to the conjecture by Barut and illustrate that the exoplanet systems provide us with a very interesting possibility to test it.

In light of the present findings, several interesting questions can be raised. We mention here a few of them. The symmetry-inspired rules describe, rather than explain, the regularity of the orbits (similarly to many other symmetry-based models and theories in different branches of physics.) How is the observed sequence related to the dynamics of the formation of the planetary systems?

In the case irregularities occur, do they indicate unobserved planets? Could they be fingerprints of unusual mechanisms in the formation of the system?

From the viewpoint of the symmetry considerations, our study gives an interesting example for the export of an originally quantum mechanical concept, i.e., dynamical algebra, to the classical mechanics.