Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source
Abstract
1. Introduction
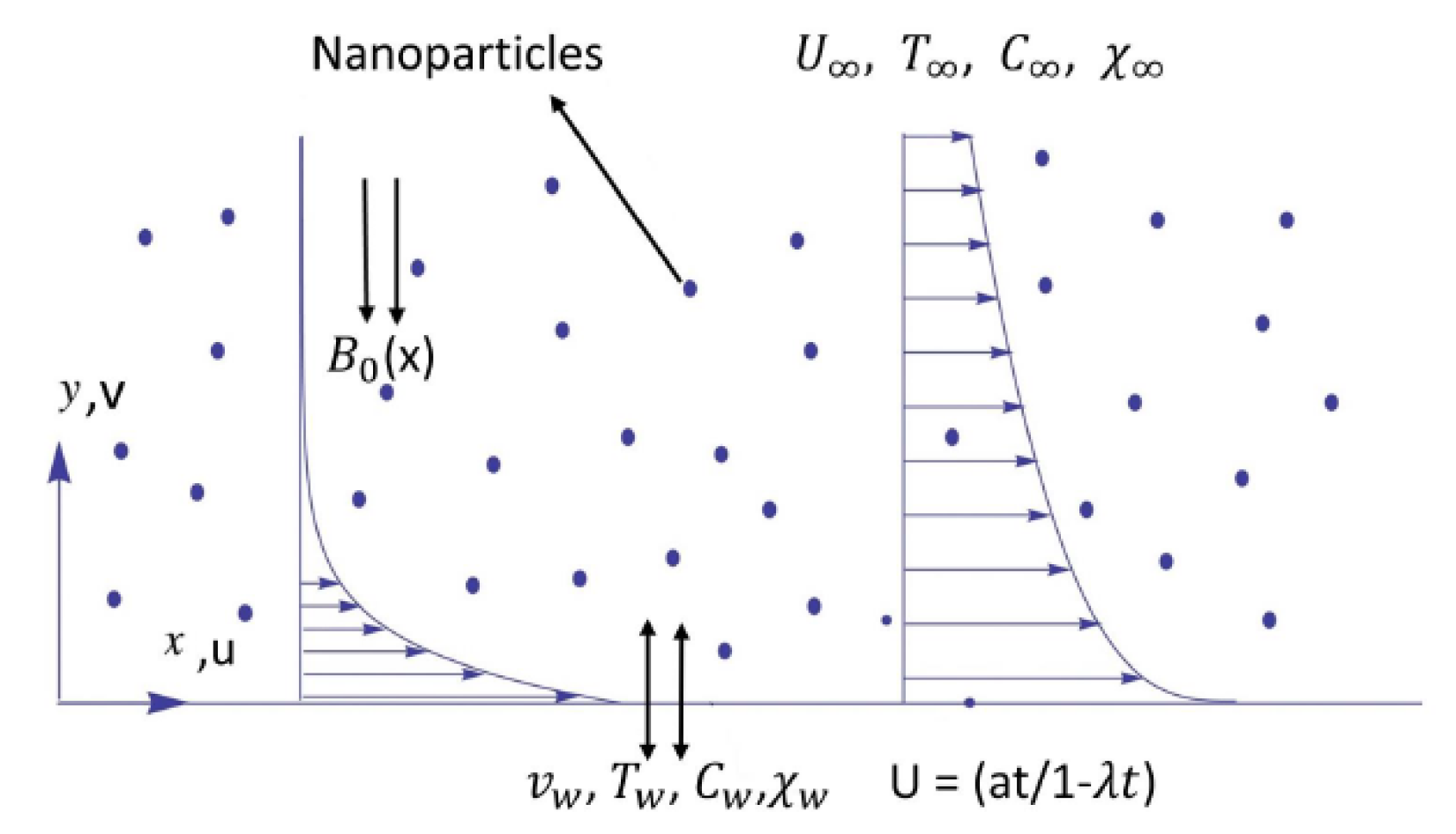
2. Mathematical Formulation
3. Implementation of Method
4. Results and Discussion
5. Conclusions
- The fluid velocity, temperature solutal and nano-particle profile are seen to increase with an increment in unsteadiness parameter.
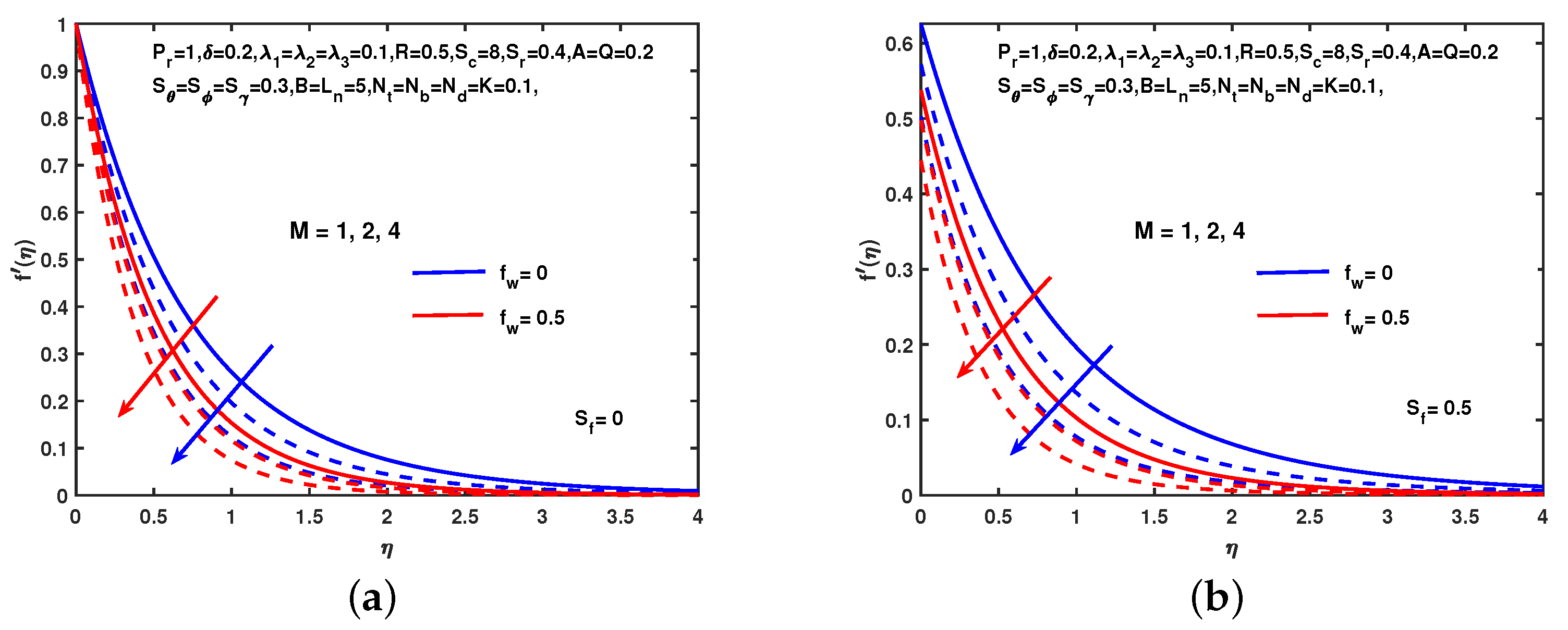
- The fluid velocity and micro-rotation declines while temperature shows opposite behavior with the enhancement in magnetic parameter, suction, hydro-dynamic, and thermal slips.
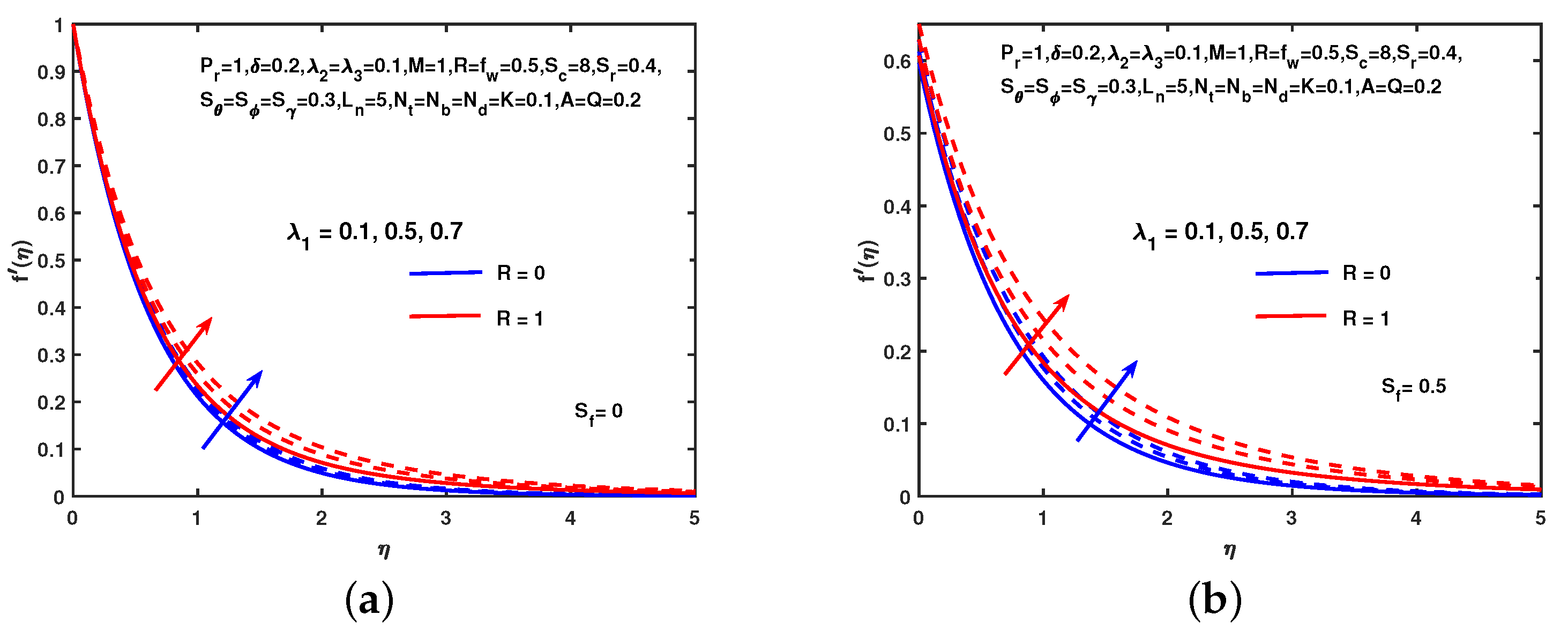
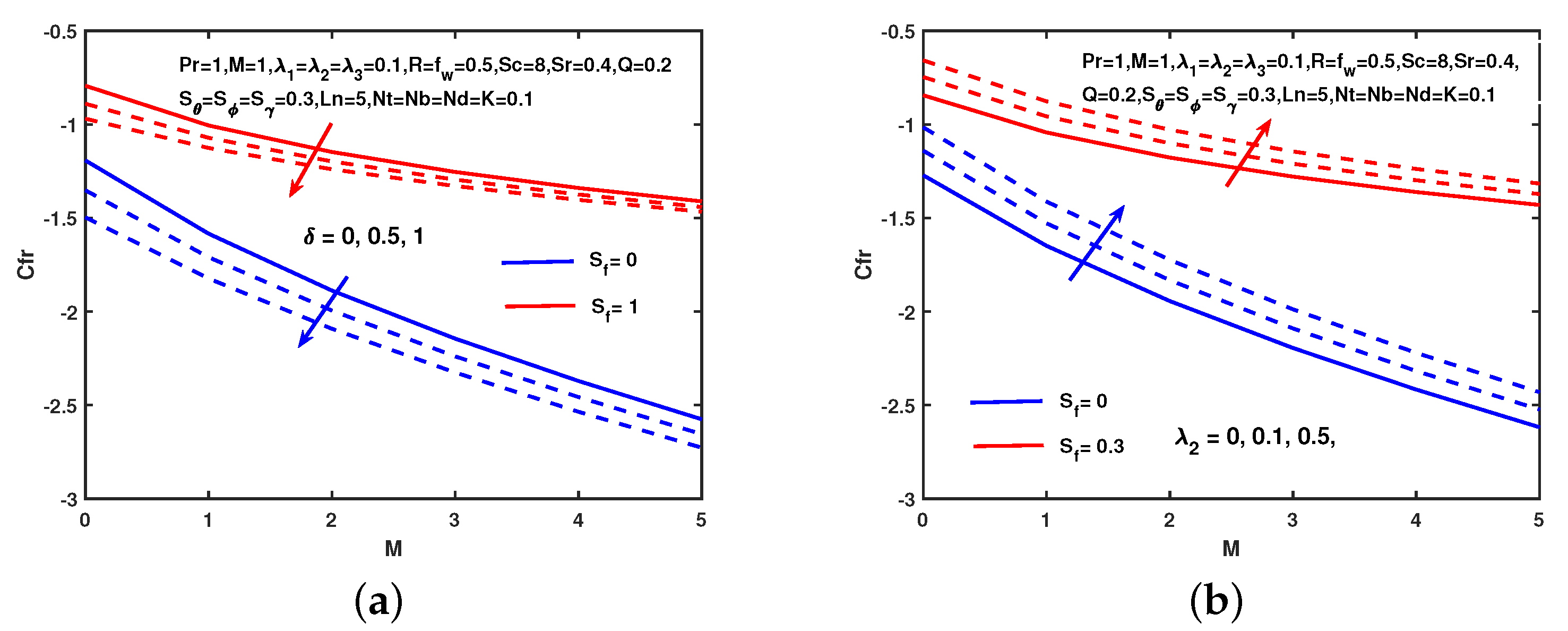
- The skin-friction coefficient decline with the increment of slip parameters, magnetic and unsteadiness parameter but shows the opposite effect for increasing values of hydrodynamic slip and thermal buoyancy.
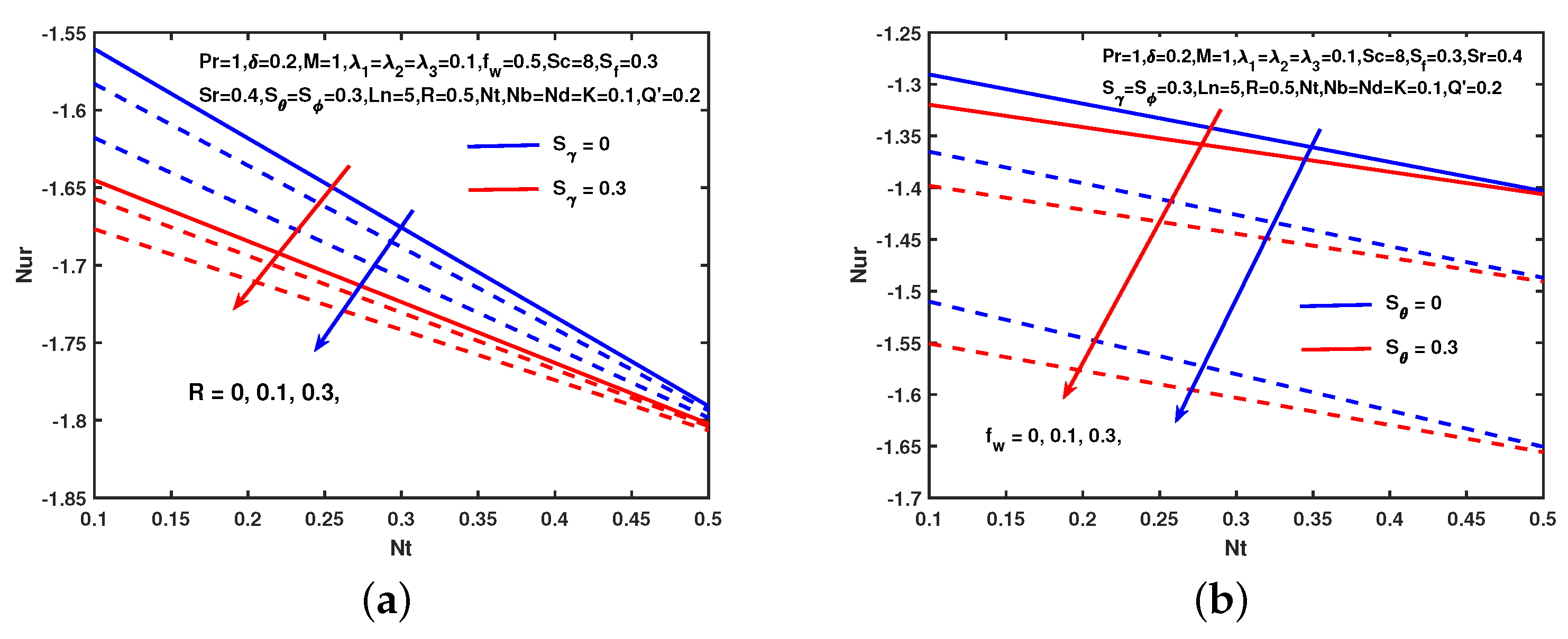
- The reduced Nusselt number decreases with the enhancement in suction, radiation, thermophoresis parameter, thermal, and solutal slips.
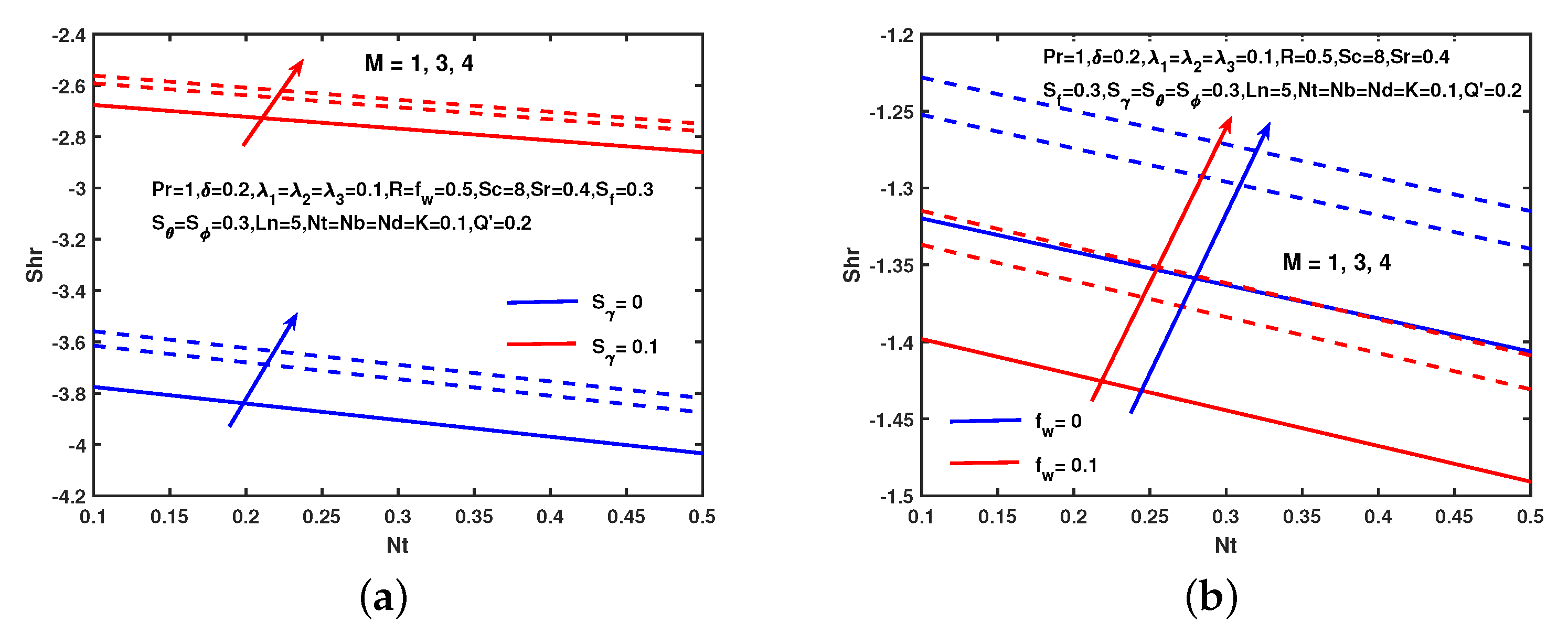
- The Sherwood number increases with an increase in magnetic parameter, suction parameter, and hydro-dynamic slip.
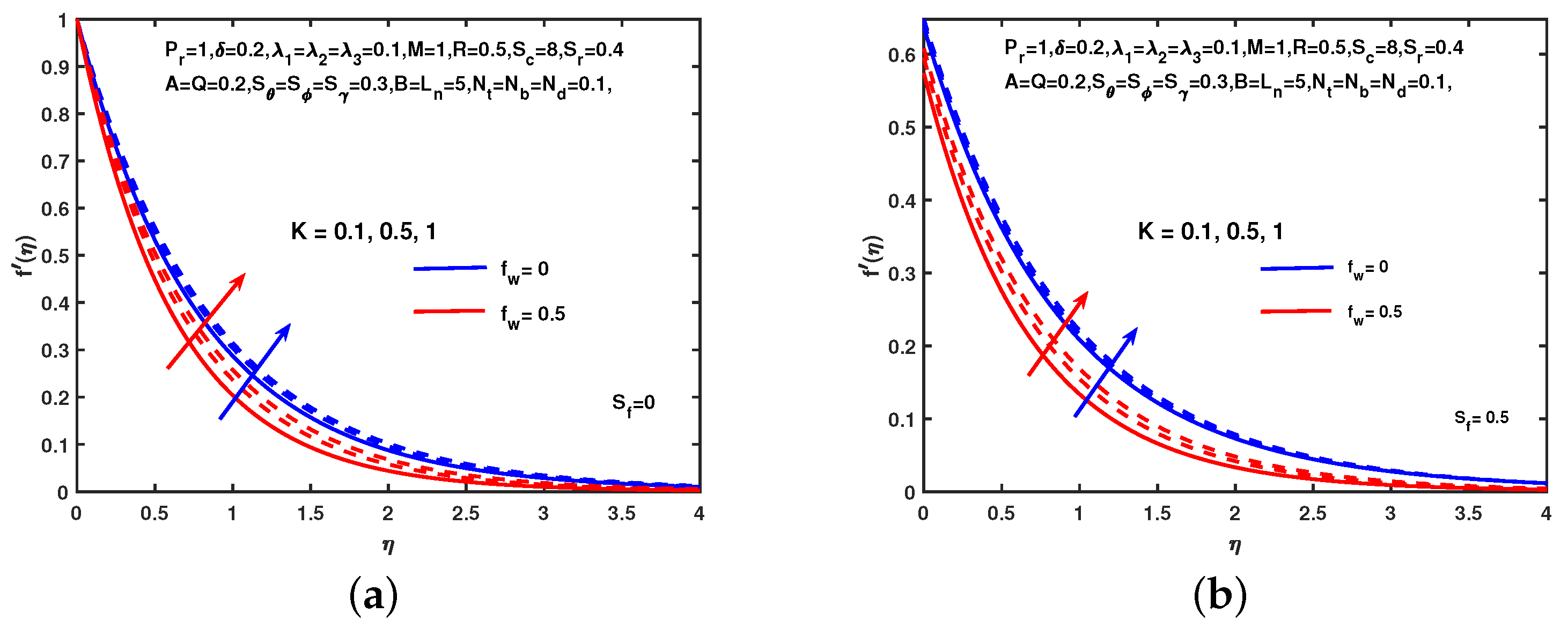
- The fluid velocity and micro-rotation increase with the increment in K, , and .
- Temperature and solutal concentration increase with the increment in thermophoresis parameter, Schmidt number, Brownian motion parameter, Soret parameter, and thermal slip while the nano-particle concentration declines as the values of thermophoresis parameter, lewis number, and nano-particle slip increase.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| M | Magnetic parameter |
| Material parameter | |
| Radiation parameter | |
| Unsteadiness parameter | |
| Buoyancy parameter | |
| Brownian motion parameter | |
| Thermo-phoresis parameter | |
| Prandtl number | |
| Lewis number | |
| R | Thermal radiation parameter |
| Dufour parameter | |
| Soret parameter | |
| Schmidt number | |
| Q | Chemical reaction |
| Suction/Injection parameter | |
| N | Microrotation vector |
| Electrical conductivity | |
| Thermal diffusivity | |
| Spin gradient viscosity | |
| g | Gravity |
| Mean absorption coefficient | |
| Stefan-Boltzmann constant | |
| T | Temperature |
| Sheet temperature | |
| Ambient temperature | |
| Reference temperature | |
| Solutal concentration | |
| Ambient solutal concentration | |
| Velocity of sheet | |
| Reference solutal concentration | |
| Nanoparticle volume fraction | |
| Ambient nanoparticle concentration | |
| Reference nanoparticle concentration | |
| Thermal diffusivity | |
| Molecular diffusivity | |
| Brownian diffusivity | |
| Soret diffusivity | |
| Dufour diffusivity | |
| Dynamic viscosity | |
| k | Vortex viscosity |
| Fluid density | |
| Velocity components | |
| Cartesian coordinates | |
| Reduced skin friction co-efficient | |
| Local Nusselt number | |
| Reduced Sherwood number |
References
- Özerinç, S.; Kakaç, S.; Yazıcıoğlu, A.G. Enhanced thermal conductivity of nanofluids: A state-of-the-art review. Microfluid. Nanofluidics 2010, 8, 145–170. [Google Scholar] [CrossRef]
- Godson, L.; Raja, B.; Lal, D.M.; Wongwises, S. Enhancement of heat transfer using nanofluids—an overview. Renew. Sustain. Energy Rev. 2010, 14, 629–641. [Google Scholar] [CrossRef]
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Technical Report; Argonne National Laboratory: Argonne, IL, USA, 1995. [Google Scholar]
- Babu, D.V. Unsteady MHD Free Convection flow in a Casson Nano Fluid Through Porous Medium with Suction and Heat Source. Int. J. Eng. Sci. Math. 2019, 8, 62–75. [Google Scholar]
- Ali, B.; Nie, Y.; Khan, S.A.; Sadiq, M.T.; Tariq, M. Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction. Processes 2019, 7, 628. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Khan, U.; Ahmed, N.; Rashidi, M.M. A study of heat and mass transfer on magnetohydrodynamic (MHD) flow of nanoparticles. Propuls. Power Res. 2018, 7, 72–77. [Google Scholar] [CrossRef]
- Akbari, O.A.; Toghraie, D.; Karimipour, A.; Marzban, A.; Ahmadi, G.R. The effect of velocity and dimension of solid nanoparticles on heat transfer in non-Newtonian nanofluid. Phys. Low-Dimensional Syst. Nanostruct. 2017, 86, 68–75. [Google Scholar] [CrossRef]
- Hayat, T.; Ahmad, S.; Khan, M.I.; Alsaedi, A. Simulation of ferromagnetic nanomaterial flow of Maxwell fluid. Results Phys. 2018, 8, 34–40. [Google Scholar] [CrossRef]
- Kamal, F.; Zaimi, K.; Ishak, A.; Pop, I. Stability analysis of MHD stagnation-point flow towards a permeable stretching/shrinking sheet in a nanofluid with chemical reactions effect. Sains Malays. 2019, 48, 243–250. [Google Scholar] [CrossRef]
- VMSS Kiran Kumar, R.; Vindo Kumar, G.; Verma, S. Unsteady Magnetohydrodynamic Stagnation Point Flow of a Nanofluid over a Slendering Stretching Sheet Using Buongiorno’s Model. Int. J. Res. Ind. Eng. 2018, 7, 84–105. [Google Scholar]
- Mabood, F.; Shateyi, S. Multiple Slip Effects on MHD Unsteady Flow Heat and Mass Transfer Impinging on Permeable Stretching Sheet with Radiation. Model. Simul. Eng. 2019, 2019, 11. [Google Scholar] [CrossRef]
- Das, U.J.; Dorjee, S. Magnetohydrodynamic Boundary Layer Flow with Soret/Dufour effects in presence of Heat source and Chemical Reaction. Int. J. Appl. Eng. Res. 2019, 14, 485–490. [Google Scholar]
- Jena, S.; Dash, G.; Mishra, S. Chemical reaction effect on MHD viscoelastic fluid flow over a vertical stretching sheet with heat source/sink. Ain Shams Eng. J. 2016. [Google Scholar] [CrossRef]
- Malarselvi, A.; Bhuvaneswari, M.; Sivasankaran, S.; Ganga, B.; Hakeem, A.A. Impacts of chemical reaction on MHD double diffusive flow with suction/blowing and slip. J. Phys. Conf. Ser. Iop Publ. 2018, 1139, 012089. [Google Scholar] [CrossRef]
- Nandeppanavar, M.M.; Kemparaju, M.; Shakunthala, S. MHD stagnation point slip flow due to a non-linearly moving surface with effect of non-uniform heat source. Nonlinear Eng. 2019, 8, 270–282. [Google Scholar] [CrossRef]
- Baag, S.; Mishra, S.; Hoque, M.M.; Anika, N.N. Magnetohydrodynamic boundary layer flow over an exponentially stretching sheet past a porous medium with uniform heat source. J. Nanofluids 2018, 7, 570–576. [Google Scholar] [CrossRef]
- Imtiaz, M.; Hayat, T.; Alsaedi, A.; Hobiny, A. Homogeneous-heterogeneous reactions in MHD flow due to an unsteady curved stretching surface. J. Mol. Liq. 2016, 221, 245–253. [Google Scholar] [CrossRef]
- Kempannagari, A.K.; Reddy, J.V.R.; Sugunamma, V.; Sandeep, N. Impact of frictional heating on MHD radiative ferrofluid past a convective shrinking surface. Defect and diffusion forum. Trans. Tech. Publ. 2017, 378, 157–174. [Google Scholar]
- Pordanjani, A.H.; Aghakhani, S.; Karimipour, A.; Afrand, M.; Goodarzi, M. Investigation of free convection heat transfer and entropy generation of nanofluid flow inside a cavity affected by magnetic field and thermal radiation. J. Therm. Anal. Calorim. 2019, 137, 997–1019. [Google Scholar] [CrossRef]
- Malvandi, A.; Safaei, M.; Kaffash, M.; Ganji, D. MHD mixed convection in a vertical annulus filled with Al2O3–water nanofluid considering nanoparticle migration. J. Magn. Magn. Mater. 2015, 382, 296–306. [Google Scholar] [CrossRef]
- Karimipour, A.; D’Orazio, A.; Shadloo, M.S. The effects of different nano particles of Al2O3 and Ag on the MHD nano fluid flow and heat transfer in a microchannel including slip velocity and temperature jump. Phys. Low-Dimensional Syst. Nanostruct. 2017, 86, 146–153. [Google Scholar] [CrossRef]
- Abdollahzadeh Jamalabadi, M.Y.; Ghasemi, M.; Alamian, R.; Wongwises, S.; Afrand, M.; Shadloo, M.S. Modeling of Subcooled Flow Boiling with Nanoparticles under the Influence of a Magnetic Field. Symmetry 2019, 11, 1275. [Google Scholar] [CrossRef]
- Mishra, S.; Hoque, M.M.; Mohanty, B.; Anika, N. Heat transfer effect on MHD flow of a micropolar fluid through porous medium with uniform heat source and radiation. Nonlinear Eng. 2019, 8, 65–73. [Google Scholar] [CrossRef]
- Hydromagnetic Stagnation Point Flow of Micropolar Fluids due to a Porous Stretching Surface with Radiation and Viscous Dissipation Effects. Sci. Int. (Lahore) 2015, 27, 3965–3971.
- Subba, R.G.R.; Jawad, C.A.; Rashad, A. Mixed convection from a vertical surface embedded in a porous medium saturated with a non-Newtonian nanofluid. J. Appl. Fluid Mech. 2013, 6, 301–309. [Google Scholar]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S. Finite Element Analysis of Thermo-Diffusion and Multi-Slip Effects on MHD Unsteady Flow of Casson Nano-Fluid over a Shrinking/Stretching Sheet with Radiation and Heat Source. Appl. Sci. 2019, 9, 5217. [Google Scholar] [CrossRef]
- Khan, S.A.; Nie, Y.; Ali, B. Multiple Slip Effects on Magnetohydrodynamic Axisymmetric Buoyant Nanofluid Flow above a Stretching Sheet with Radiation and Chemical Reaction. Symmetry 2019, 11, 1171. [Google Scholar] [CrossRef]
- Khan, I.; Alqahtani, A.M. MHD Nanofluids in a Permeable Channel with Porosity. Symmetry 2019, 11, 378. [Google Scholar] [CrossRef]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S. Finite Element Simulation of Multi-Slip Effects on Unsteady MHD Bioconvective Micropolar nanofluid Flow Over a Sheet with Solutal and Thermal Convective Boundary Conditions. Coatings 2019, 9, 842. [Google Scholar] [CrossRef]
- Hayat, T.; Rashid, M.; Alsaedi, A. MHD convective flow of magnetite-Fe3O4 nanoparticles by curved stretching sheet. Results Phys. 2017, 7, 3107–3115. [Google Scholar] [CrossRef]
- Malarselvi, A.; Bhuvaneswari, M.; Sivasankaran, S.; Ganga, B.; Hakeem, A.A. Effect of Slip and Convective Heating on Unsteady MHD Chemically Reacting Flow Over a Porous Surface with Suction. In Applied Mathematics and Scientific Computing; Springer: Berlin/Heidelberg, Germany, 2019; pp. 357–365. [Google Scholar]
- Mozaffari, S.; Tchoukov, P.; Atias, J.; Czarnecki, J.; Nazemifard, N. Effect of asphaltene aggregation on rheological properties of diluted athabasca bitumen. Energy Fuels 2015, 29, 5595–5599. [Google Scholar] [CrossRef]
- Darjani, S.; Koplik, J.; Pauchard, V. Extracting the equation of state of lattice gases from random sequential adsorption simulations by means of the Gibbs adsorption isotherm. Phys. Rev. 2017, 96, 052803. [Google Scholar] [CrossRef] [PubMed]
- Mozaffari, S.; Li, W.; Dixit, M.; Seifert, S.; Lee, B.; Kovarik, L.; Mpourmpakis, G.; Karim, A.M. The role of nanoparticle size and ligand coverage in size focusing of colloidal metal nanoparticles. Nanoscale Adv. 2019, 1, 4052–4066. [Google Scholar] [CrossRef]
- Li, W.; Ivanov, S.; Mozaffari, S.; Shanaiah, N.; Karim, A.M. Palladium Acetate Trimer: Understanding Its Ligand-Induced Dissociation Thermochemistry Using Isothermal Titration Calorimetry, X-ray Absorption Fine Structure, and 31P Nuclear Magnetic Resonance. Organometallics 2018, 38, 451–460. [Google Scholar] [CrossRef]
- Mozaffari, A.; Sharifi-Mood, N.; Koplik, J.; Maldarelli, C. Self-diffusiophoretic colloidal propulsion near a solid boundary. Phys. Fluids 2016, 28, 053107. [Google Scholar] [CrossRef]
- Mozaffari, S.; Tchoukov, P.; Mozaffari, A.; Atias, J.; Czarnecki, J.; Nazemifard, N. Capillary driven flow in nanochannels–Application to heavy oil rheology studies. Colloids Surfaces Physicochem. Eng. Asp. 2017, 513, 178–187. [Google Scholar] [CrossRef]
- Liu, F.; Darjani, S.; Akhmetkhanova, N.; Maldarelli, C.; Banerjee, S.; Pauchard, V. Mixture effect on the dilatation rheology of asphaltenes-laden interfaces. Langmuir 2017, 33, 1927–1942. [Google Scholar] [CrossRef]
- Lok, Y.; Amin, N.; Pop, I. Unsteady mixed convection flow of a micropolar fluid near the stagnation point on a vertical surface. Int. J. Therm. Sci. 2006, 45, 1149–1157. [Google Scholar] [CrossRef]
- Sharma, P.; Sinha, S.; Yadav, R.; Filippov, A.N. MHD mixed convective stagnation point flow along a vertical stretching sheet with heat source/sink. Int. J. Heat Mass Transf. 2018, 117, 780–786. [Google Scholar] [CrossRef]
- Ellahi, R.; Zeeshan, A.; Shehzad, N.; Alamri, S.Z. Structural impact of Kerosene-Al2O3 nanoliquid on MHD Poiseuille flow with variable thermal conductivity: Application of cooling process. J. Mol. Liq. 2018, 264, 607–615. [Google Scholar] [CrossRef]
- Dhanai, R.; Rana, P.; Kumar, L. Critical values in slip flow and heat transfer analysis of non-Newtonian nanofluid utilizing heat source/sink and variable magnetic field: Multiple solutions. J. Taiwan Inst. Chem. Eng. 2016, 58, 155–164. [Google Scholar] [CrossRef]
- Ashraf, M.; Bashir, S. Numerical simulation of MHD stagnation point flow and heat transfer of a micropolar fluid towards a heated shrinking sheet. Int. J. Numer. Methods Fluids 2012, 69, 384–398. [Google Scholar] [CrossRef]
- Abdul Latiff, N.A.; Uddin, M.J.; Bég, O.A.; Ismail, A.I. Unsteady forced bioconvection slip flow of a micropolar nanofluid from a stretching/shrinking sheet. Proc. Inst. Mech. Eng. Part J. Nanomater. Nanoeng. Nanosyst. 2016, 230, 177–187. [Google Scholar] [CrossRef]
- Pal, D. Combined effects of non-uniform heat source/sink and thermal radiation on heat transfer over an unsteady stretching permeable surface. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1890–1904. [Google Scholar] [CrossRef]
- Haile, E.; Shankar, B. Heat and mass transfer in the boundary layer of unsteady viscous nanofluid along a vertical stretching sheet. J. Comput. Eng. 2014, 2014. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Boundary layer flow and heat transfer over an unsteady stretching vertical surface. Meccanica 2009, 44, 369–375. [Google Scholar] [CrossRef]
- Gireesha, B.; Ramesh, G.; Bagewadi, C. Heat transfer in MHD flow of a dusty fluid over a stretching sheet with viscous dissipation. J. Appl. Sci. Res. 2012, 3, 2392–2401. [Google Scholar]
- Jalil, M.; Asghar, S.; Yasmeen, S. An exact solution of MHD boundary layer flow of dusty fluid over a stretching surface. Math. Probl. Eng. 2017, 2017. [Google Scholar] [CrossRef]
- Eldabe, N.T.; Ouaf, M.E. Chebyshev finite difference method for heat and mass transfer in a hydromagnetic flow of a micropolar fluid past a stretching surface with Ohmic heating and viscous dissipation. Appl. Math. Comput. 2006, 177, 561–571. [Google Scholar] [CrossRef]
- Hsiao, K.L. Micropolar nanofluid flow with MHD and viscous dissipation effects towards a stretching sheet with multimedia feature. Int. J. Heat Mass Transf. 2017, 112, 983–990. [Google Scholar] [CrossRef]
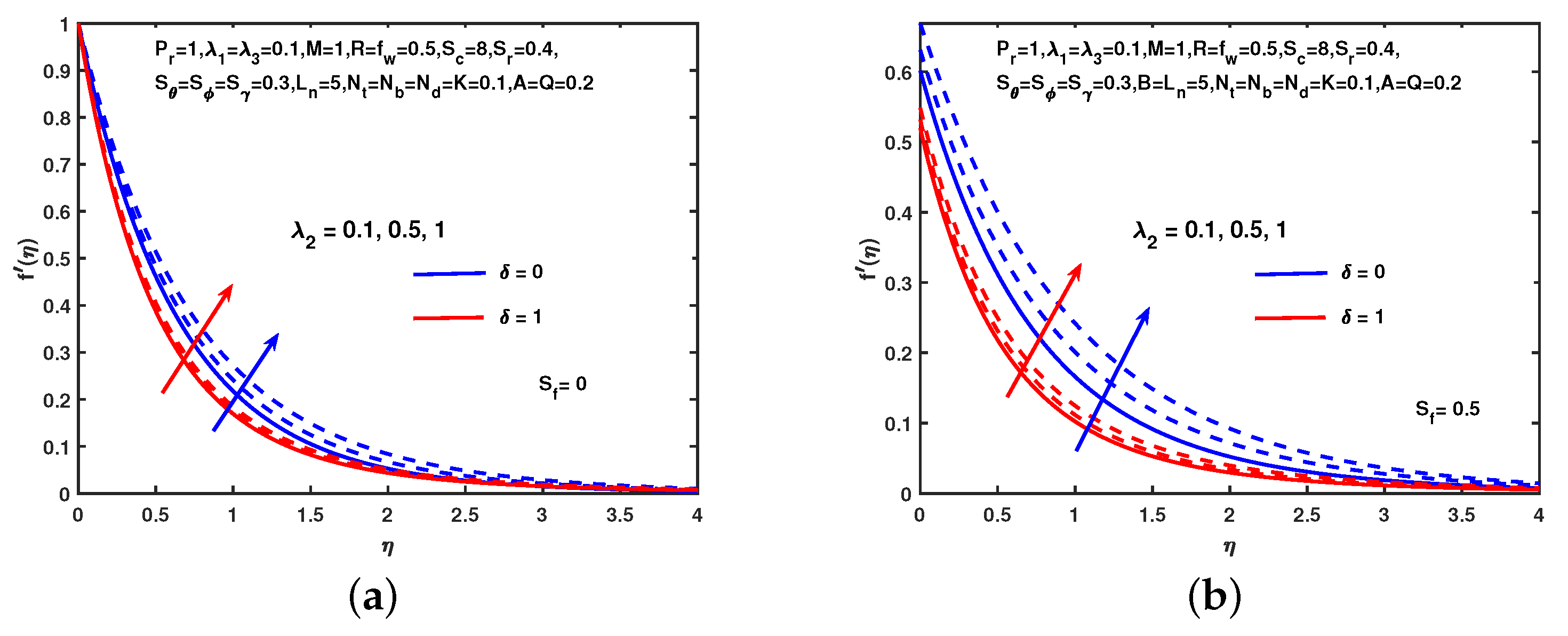
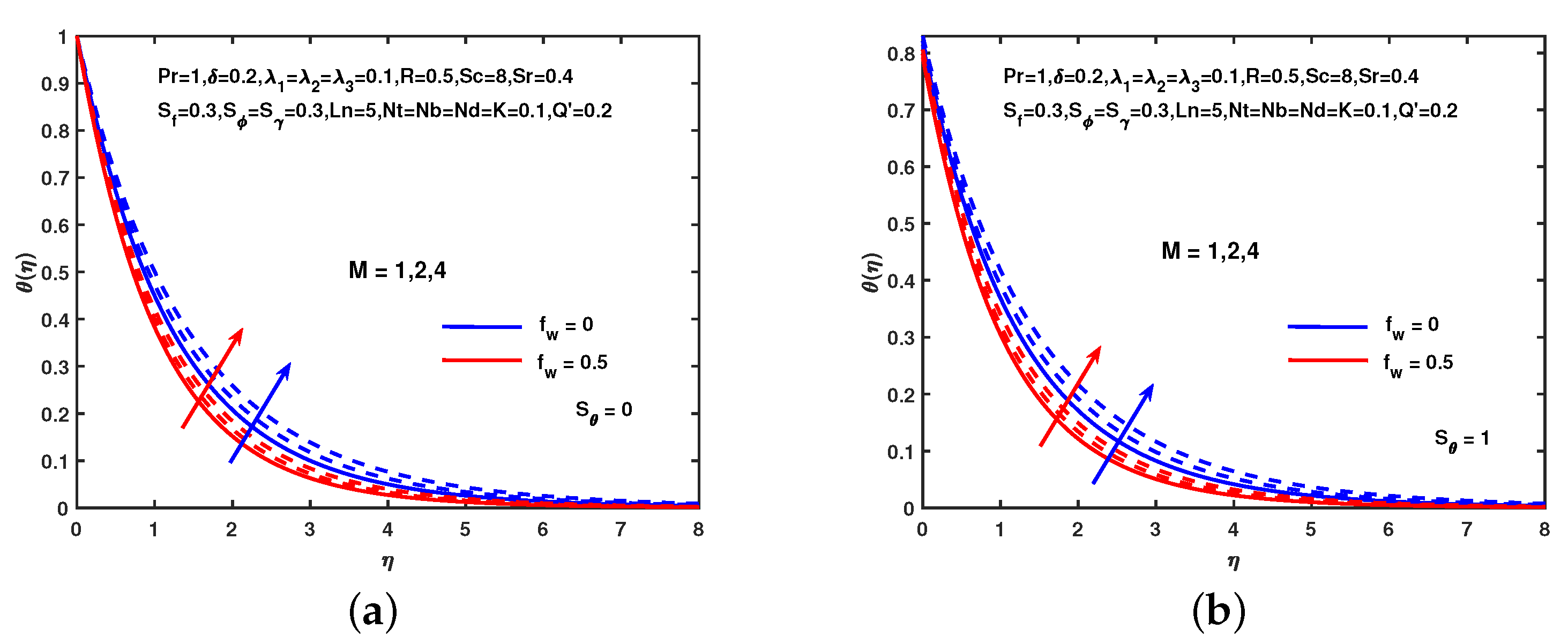
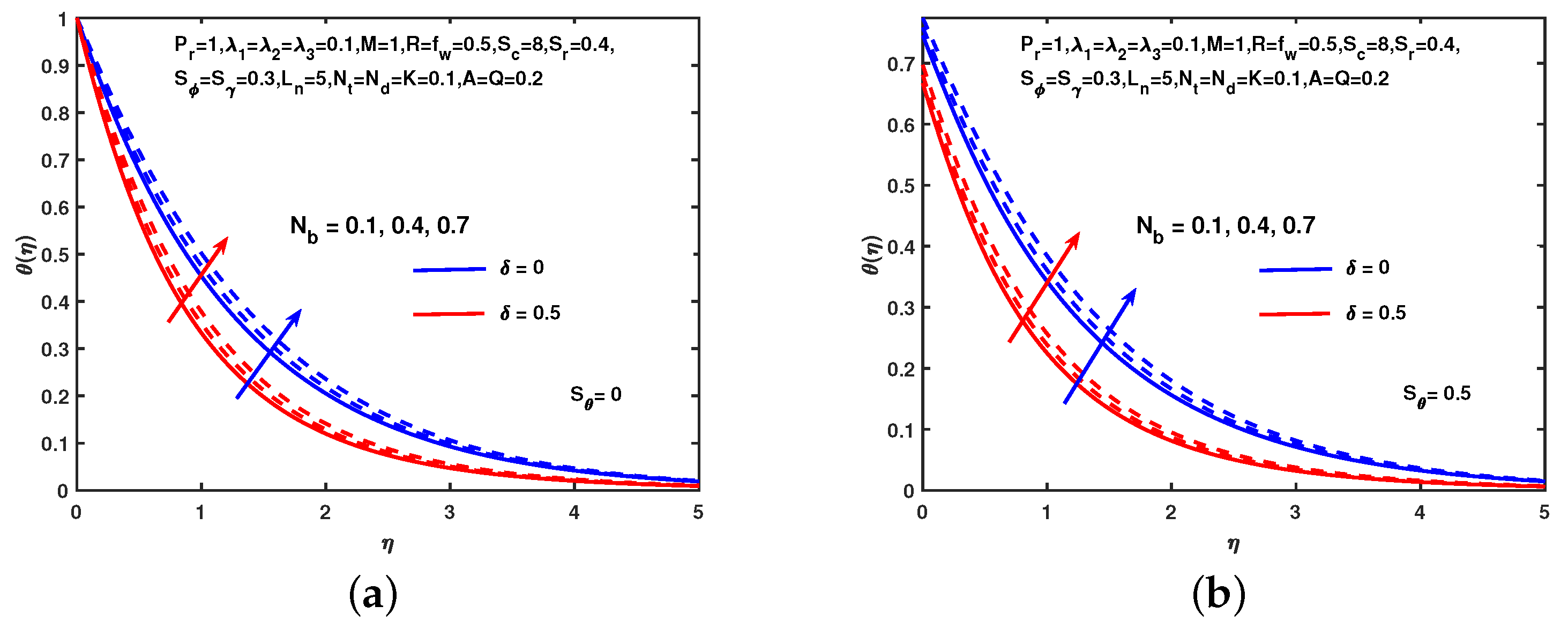



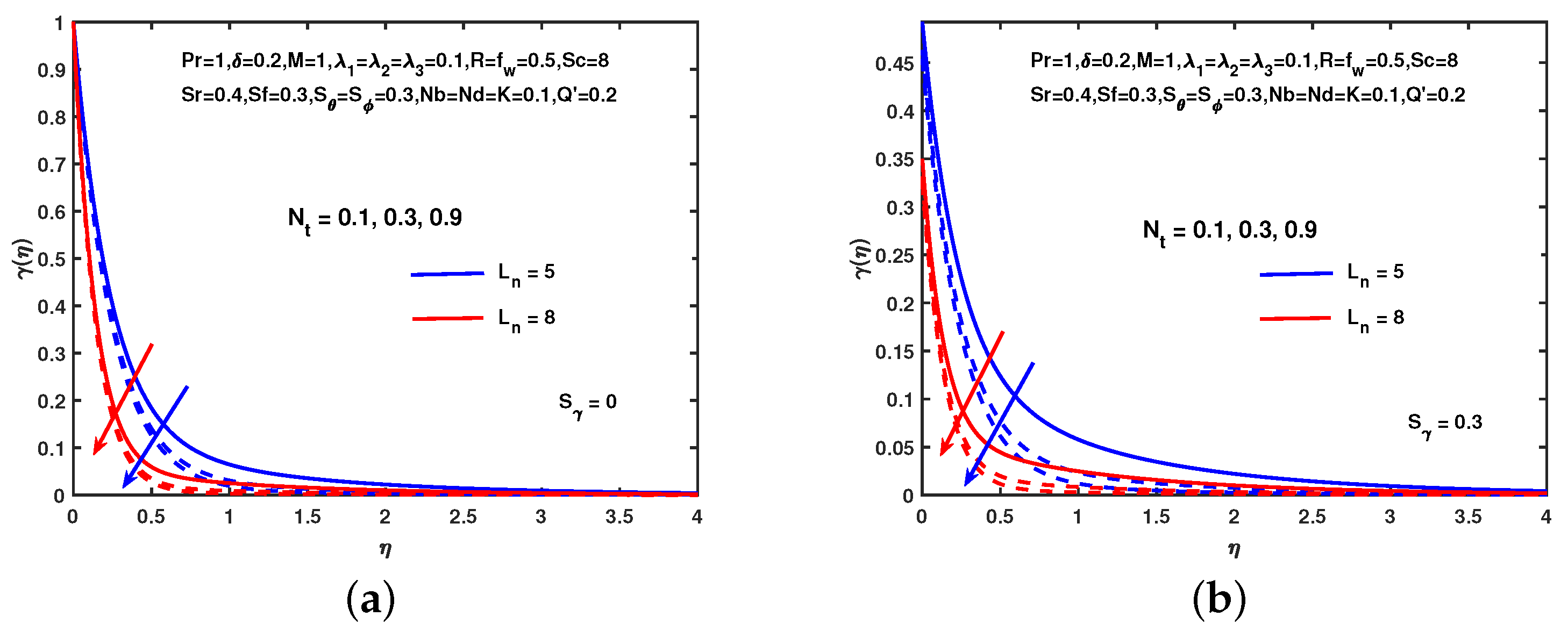
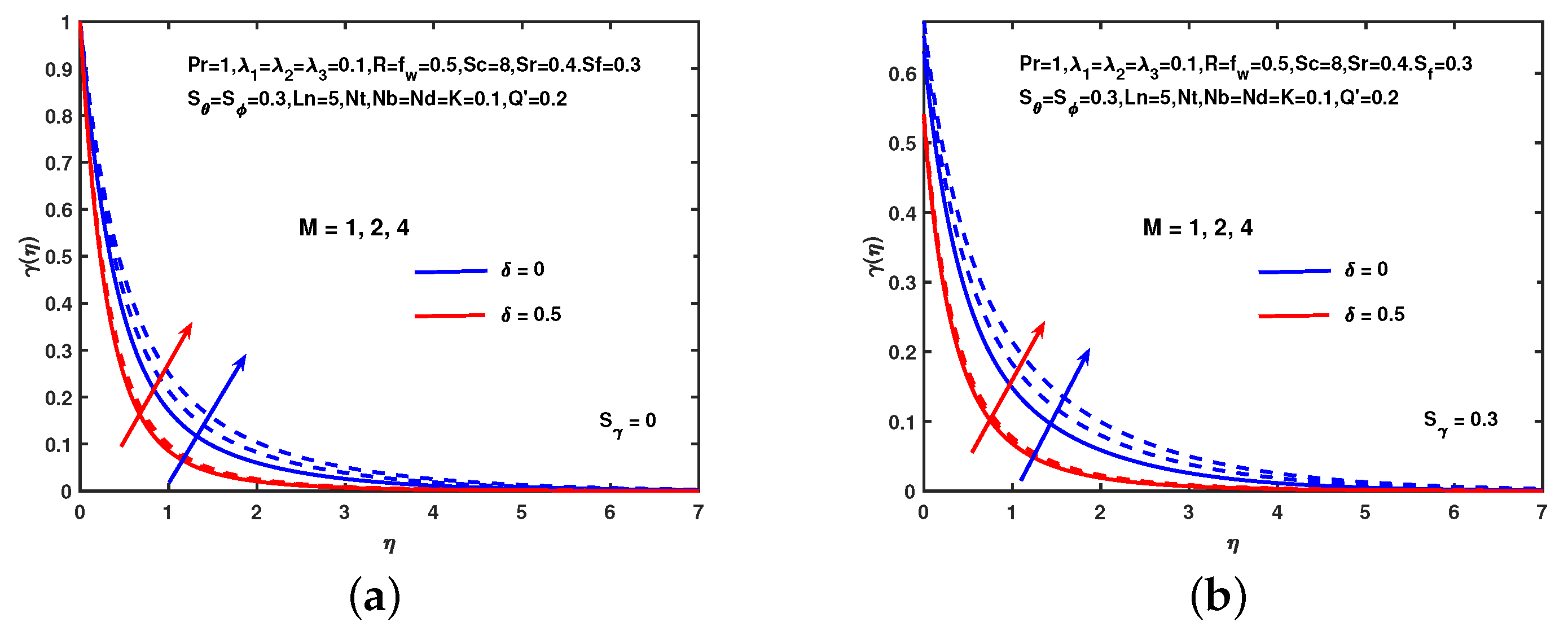
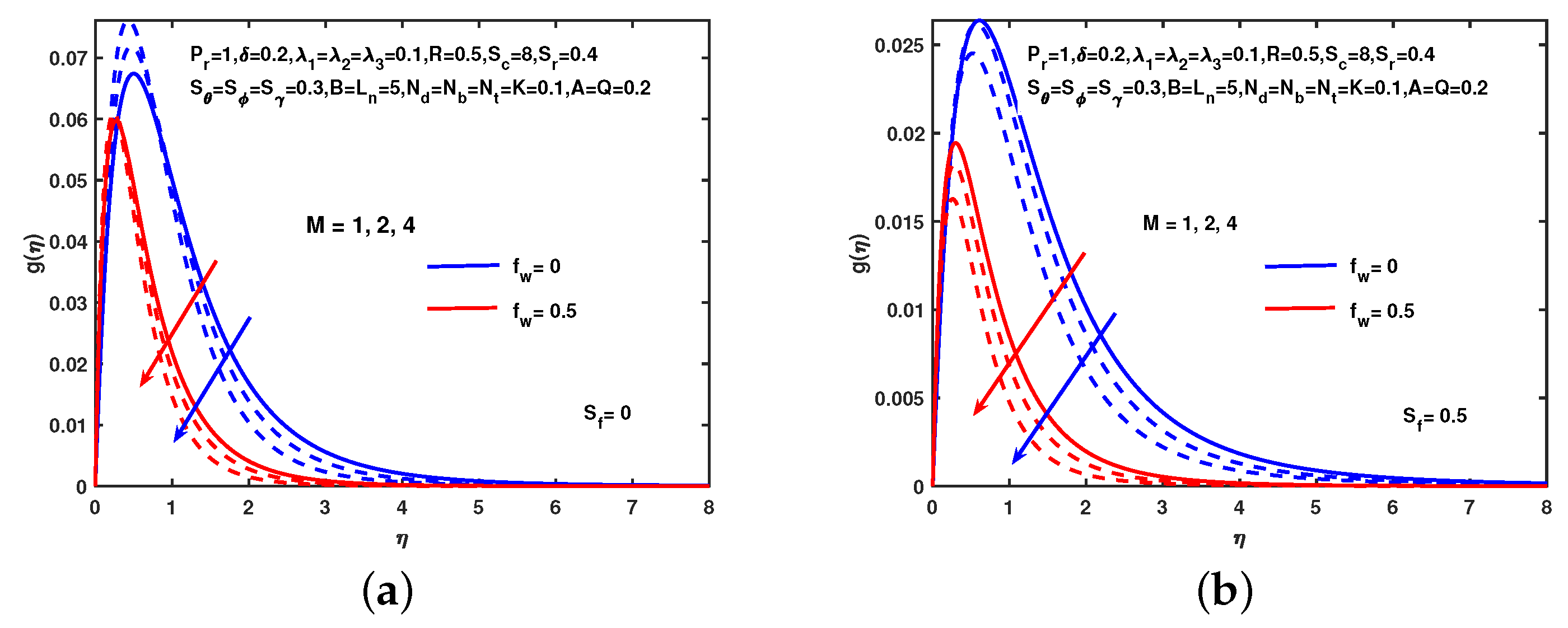
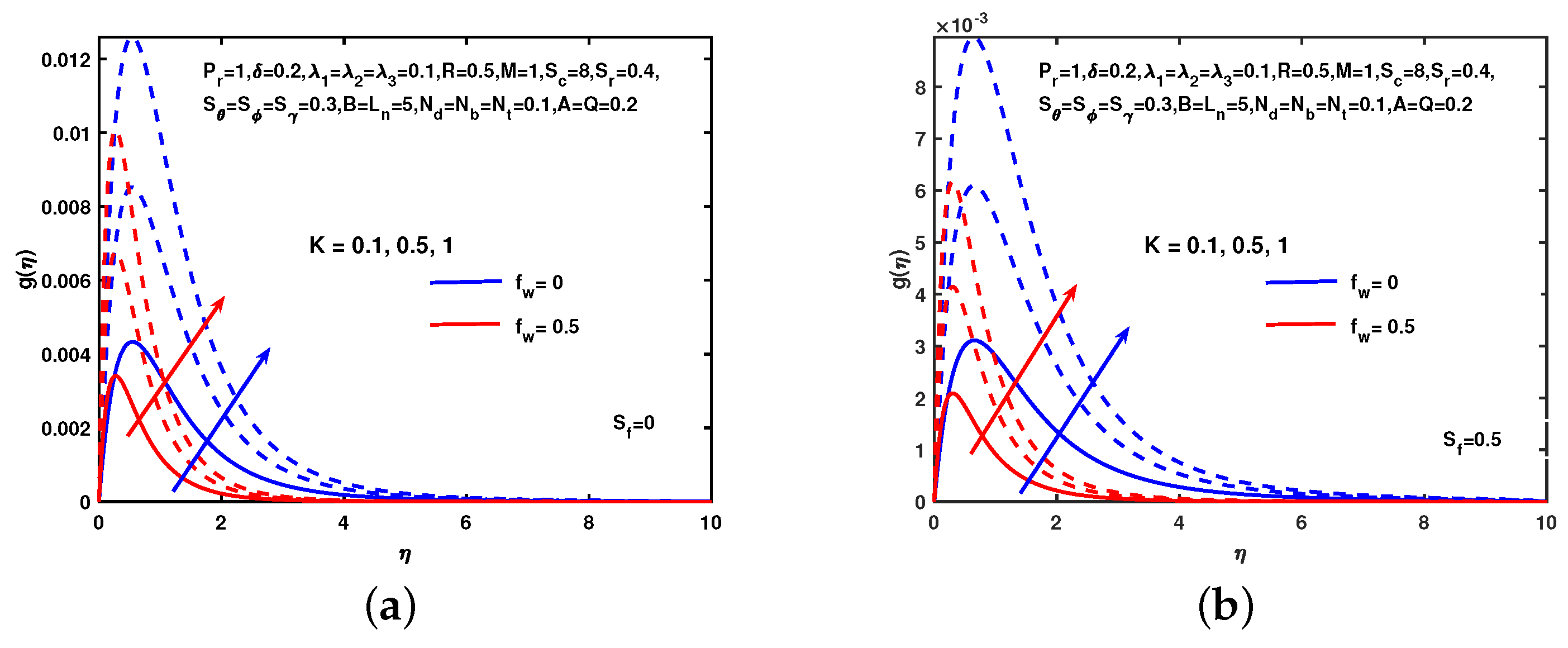
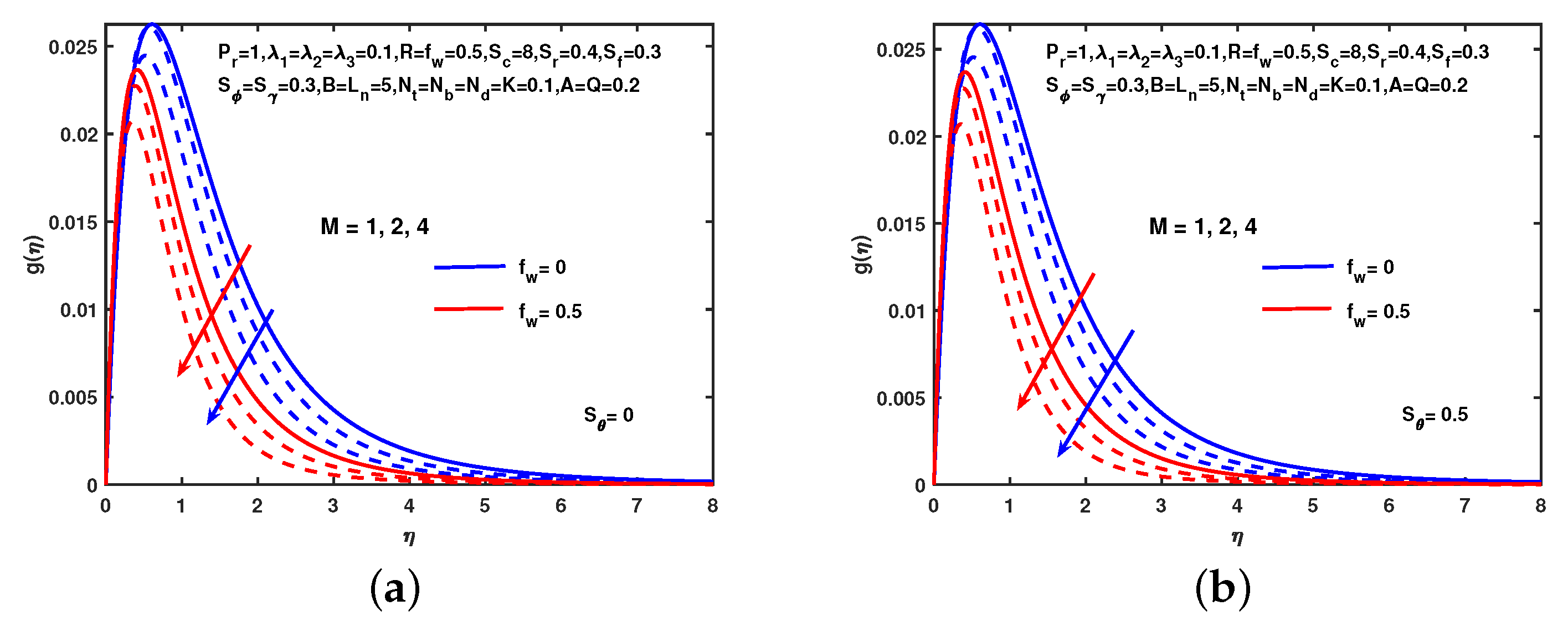
| Liaqat et al. | Fazle et al. | Ishak et al. | Dulal et al. | Haile et al. | Ishak et al. [47] | Our Results | Error in % | |
|---|---|---|---|---|---|---|---|---|
| [29] | [11] | [47] | [45] | [46] | (a) | (b) | ||
| 0.72 | 0.8086 | 0.8088 | - | - | - | 0.8086313498 | 0.8086339299 | 0.0004 |
| 1.00 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0004 | 1.000000000 | 1.0000080213 | 0.0008 |
| 3.00 | 1.9236 | 1.9237 | 1.9237 | 1.9236 | 1.9234 | 1.923682594 | 1.9236777221 | 0.0004 |
| 10.0 | 3.7206 | 3.7207 | 3.7207 | 3.7207 | 3.7205 | 3.720673901 | 3.7206681683 | 0.0002 |
| 100 | 12.2946 | - | 12.2941 | 12.2962 | 12.2962 | 12.294083260 | 12.294051659 | 0.0002 |
| M | Gireesha et al. [48] | Mudassar et al. [49] | Bagh et al. [5] | Our Results | Error in % |
|---|---|---|---|---|---|
| (a) | (b) | ||||
| 0.0 | 1.000 | 1.000000 | 1.0000080 | 1.0000130 | 0.00130 |
| 0.2 | 1.095 | 1.095445 | 1.0954458 | 1.0954463 | 0.00013 |
| 0.5 | 1.224 | 1.224745 | 1.2247446 | 1.2247454 | 0.00003 |
| 1.0 | 1.414 | 1.414214 | 1.4142132 | 1.4142180 | 0.00002 |
| 1.2 | 1.483 | 1.483240 | 1.4832393 | 1.4832402 | 0.00001 |
| 1.5 | 1.581 | 1.581139 | 1.5811384 | 1.5811396 | 0.00003 |
| 2.0 | 1.732 | 1.732051 | 1.7320504 | 1.7320516 | 0.00003 |
| M | K | [50] | [51] | Our Results | [50] | [51] | Our Results |
|---|---|---|---|---|---|---|---|
| 0.0 | 0.2 | 0.9098 | 0.90976 | 0.909798 | 0.0950 | 0.09500 | 0.094895 |
| 0.5 | 1.1148 | 1011437 | 1.114378 | 0.1051 | 0.10509 | 0.105088 | |
| 1.0 | 1.2871 | 1.28711 | 1.287148 | 0.1121 | 0.11212 | 0.112048 | |
| 0.0 | 1.4142 | 1.41423 | 1.414228 | 0.0000 | 0.00000 | 0.000000 | |
| 0.5 | 1.1408 | 1.14073 | 1.140772 | 0.2112 | 0.21116 | 0.211165 | |
| 2.0 | 0.7697 | 0.76958 | 0.769755 | 0.3586 | 0.35855 | 0.358646 |
| M | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.5 | 0.945865 | 0.183972 | 0.708908 | 1.705238 | |||||
| 1.0 | 0.1 | 0.1 | 0.1 | 0.2 | 5.0 | 1.033896 | 0.190324 | 0.694612 | 1.691997 |
| 3.0 | 1.271767 | 0.199565 | 0.659158 | 1.655360 | |||||
| 0.5 | 0.1 | 0.945865 | 0.183972 | 0.708908 | 1.705238 | ||||
| 0.5 | 0.1 | 0.1 | 0.2 | 5.0 | 0.848947 | 0.174640 | 0.725500 | 1.719654 | |
| 1.0 | 0.737892 | 0.165374 | 0.742820 | 1.734753 | |||||
| 0.5 | 0.1 | 0.1 | 0.945865 | 0.183972 | 0.708908 | 1.705238 | |||
| 0.5 | 0.1 | 0.2 | 5.0 | 0.908433 | 0.180855 | 0.715054 | 1.710706 | ||
| 1.0 | 0.863521 | 0.177375 | 0.722139 | 1.717023 | |||||
| 0.5 | 0.1 | 0.1 | 0.1 | 0.2 | 5.0 | 0.945865 | 0.183972 | 0.708908 | 1.705238 |
| 0.5 | 0.913618 | 0.182366 | 0.713483 | 1.709592 | |||||
| 1.0 | 0.874410 | 0.180529 | 0.718900 | 1.714746 | |||||
| 0.5 | 0.1 | 0.1 | 0.1 | 0.2 | 5.0 | 0.945865 | 0.183972 | 0.708908 | 1.705238 |
| 0.8 | 1.030296 | 0.145774 | 0.886881 | 1.852248 | |||||
| 1.0 | 1.054940 | 0.137761 | 0.931947 | 1.889387 | |||||
| 0.5 | 0.1 | 0.1 | 0.1 | 0.2 | 5.0 | 0.945865 | 0.183972 | 0.708908 | 1.705238 |
| 10.0 | 0.950153 | 0.184260 | 0.710358 | 2.175390 | |||||
| 13.0 | 0.951190 | 0.184309 | 0.711291 | 2.338004 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdal, S.; Ali, B.; Younas, S.; Ali, L.; Mariam, A. Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source. Symmetry 2020, 12, 49. https://doi.org/10.3390/sym12010049
Abdal S, Ali B, Younas S, Ali L, Mariam A. Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source. Symmetry. 2020; 12(1):49. https://doi.org/10.3390/sym12010049
Chicago/Turabian StyleAbdal, Sohaib, Bagh Ali, Saba Younas, Liaqat Ali, and Amna Mariam. 2020. "Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source" Symmetry 12, no. 1: 49. https://doi.org/10.3390/sym12010049
APA StyleAbdal, S., Ali, B., Younas, S., Ali, L., & Mariam, A. (2020). Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source. Symmetry, 12(1), 49. https://doi.org/10.3390/sym12010049







