First Integral Technique for Finding Exact Solutions of Higher Dimensional Mathematical Physics Models
Abstract
1. Introduction
2. Preliminaries
Conformable Derivative
3. Feng’s First Integral Method (FIM)
4. Exact Solutions of Conformable mRLW Equation, (1+2) Dimensional Conformable pKP Equation and (1+2) Dimensional Conformable DLW System
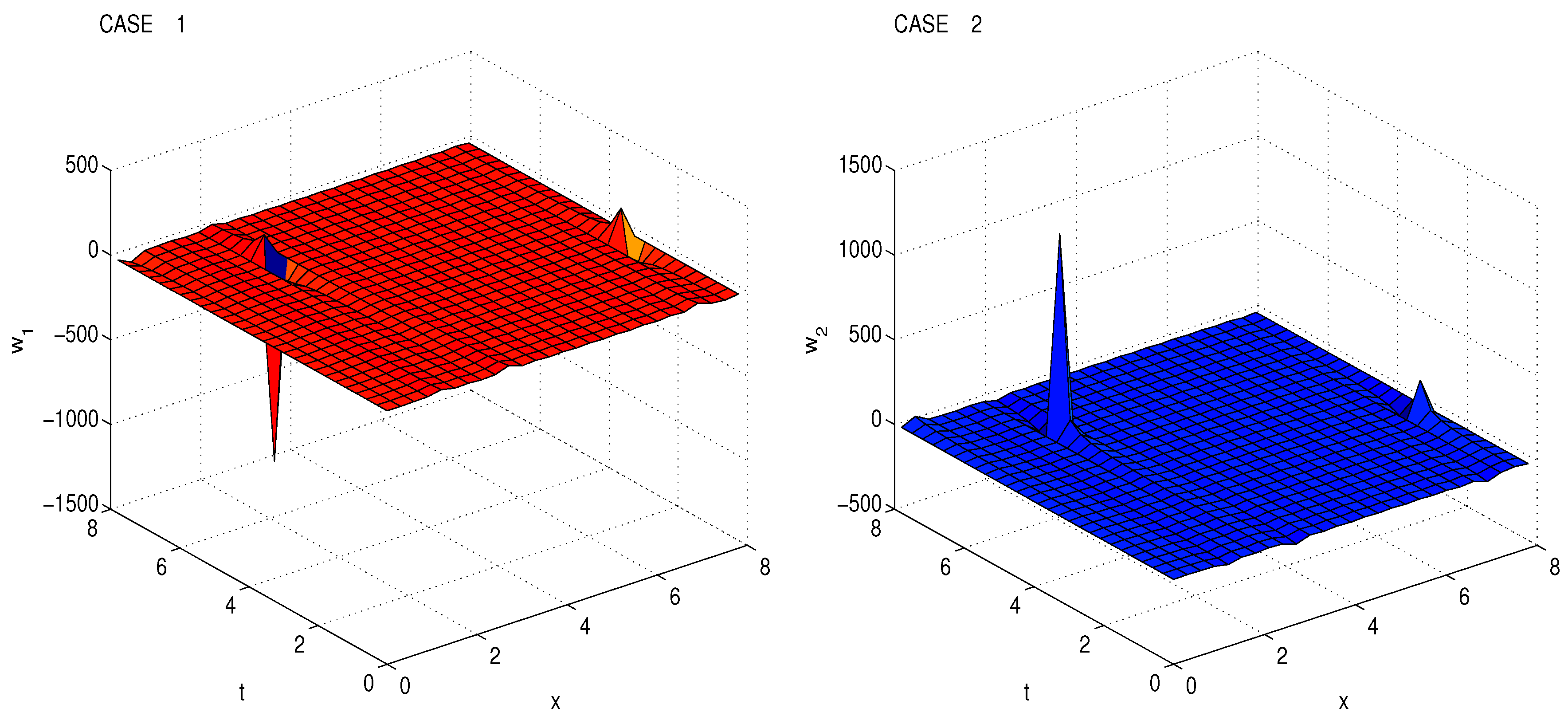
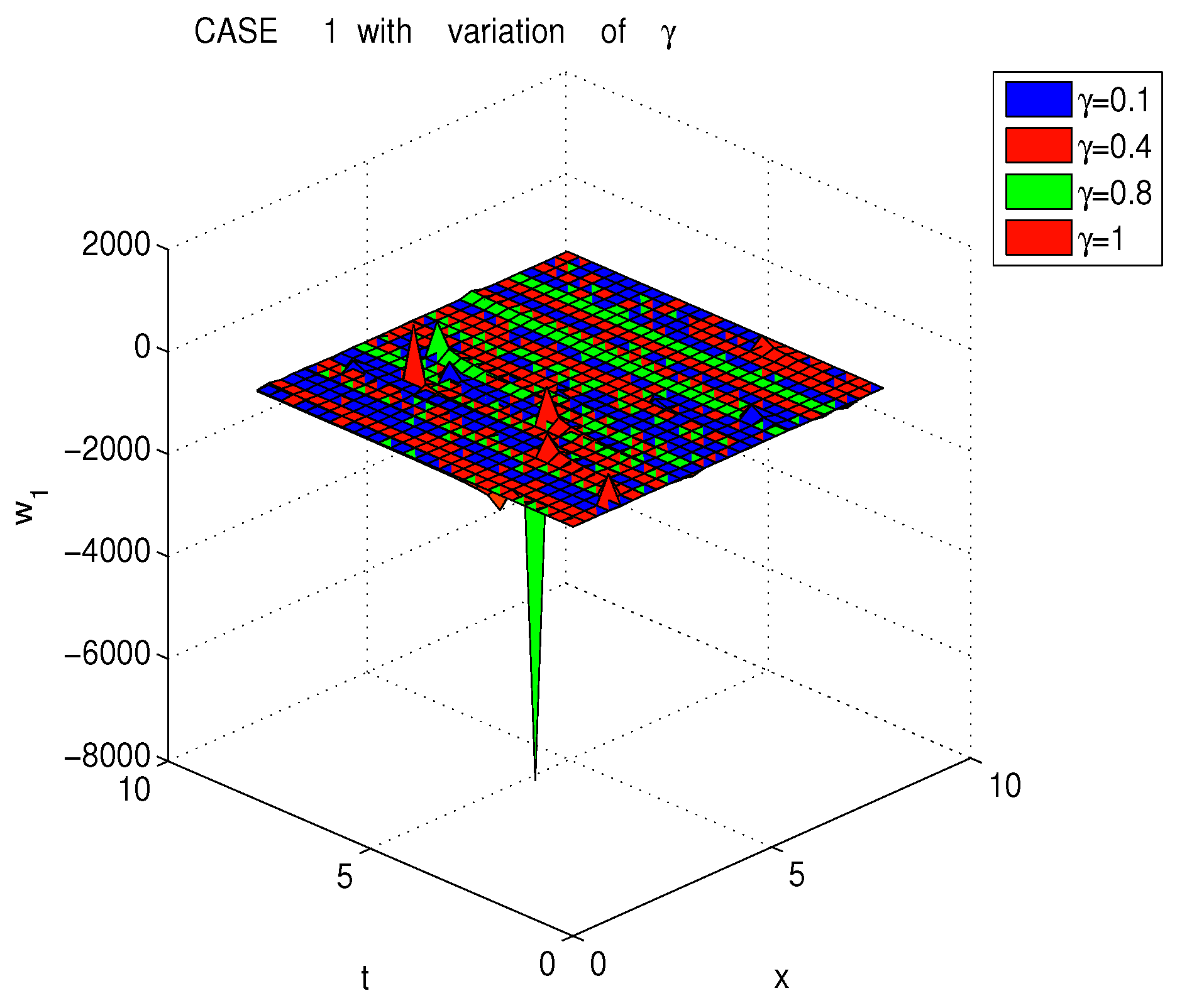
4.1. Exact Solutions of Conformable Space-Time mRLW Equation
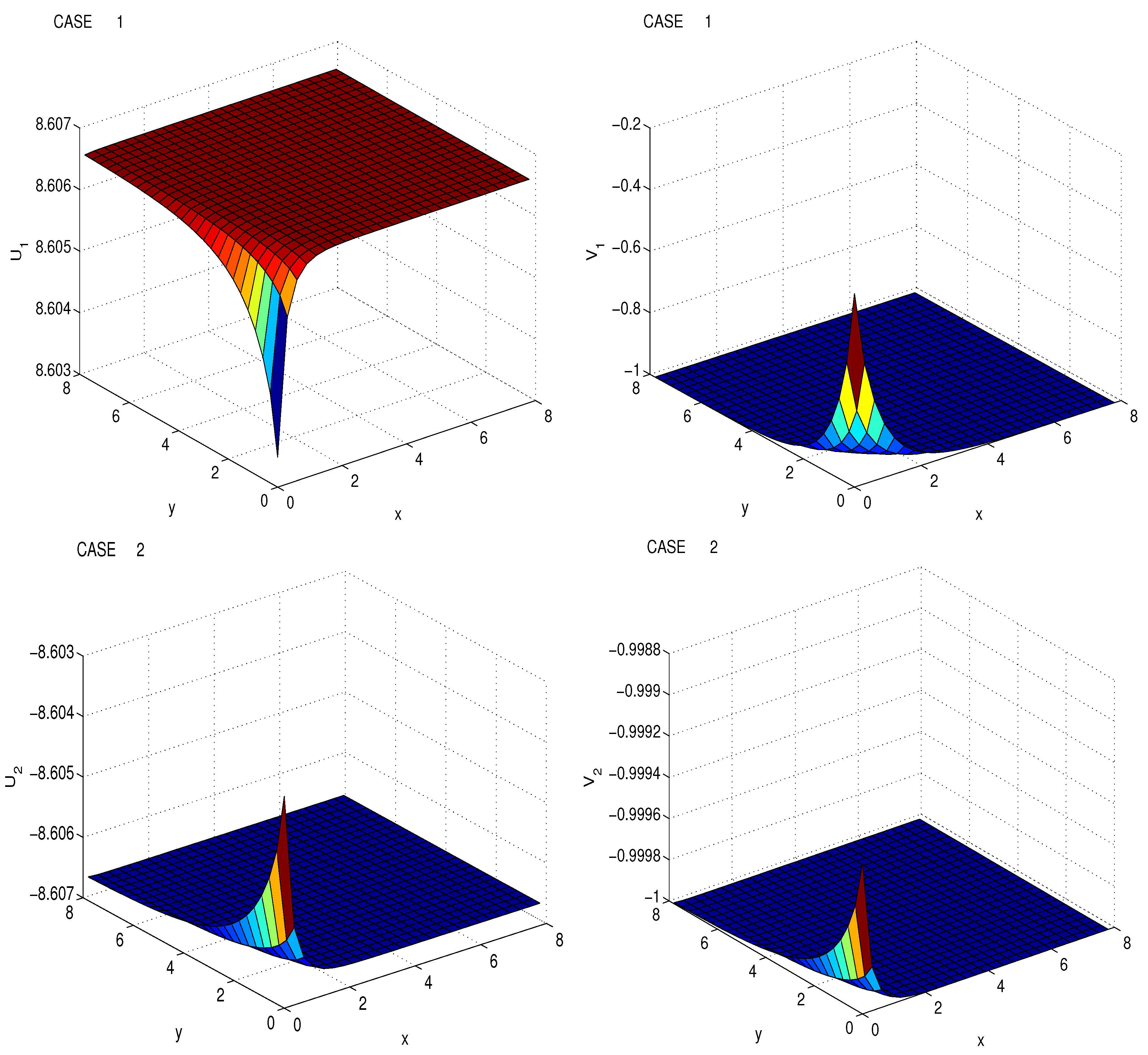
4.2. Exact Solutions of Conformable Space-Time pKP Equation
4.3. Exact Solutions of Conformable Space-Time DLW System
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Kilbas, A.A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations. North-Holland Mathematics Studies; Elsevier Science: Amsterdam, The Netherlands, 2004. [Google Scholar]
- Mille, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications (Vol. 198); Elsevier: San Diego, CA, USA, 1999. [Google Scholar]
- Elizarraraz, D.; Verde-Star, L. Fractional divided differences and the solutions of differential equations of fractional order. Adv. Appl. Math. 2000, 24, 260–283. [Google Scholar] [CrossRef][Green Version]
- Levi, L.; Payroutet, F. A time-fractional step method for conservation lawrelated obstacle problems. Adv. Appl. Math. 2001, 27, 768–789. [Google Scholar] [CrossRef]
- Momani, S.; Odibat, Z. Homotopy perturbation method for nonlinear partial differential equations of fractional order. Phys. Lett. A 2007, 365, 345–350. [Google Scholar] [CrossRef]
- Dehghan, M.; Manafian, J.; Saadatmandi, A. Solving nonlinear fractional partial differential equations using the homotopy analysis method. Numer. Methods Partial Differ. Equ. 2010, 26, 448–479. [Google Scholar] [CrossRef]
- Xia, T.C.; Wang, L. New explicit and exact solutions for the Nizhnik-Novikov-Vesselov equation. Appl. Math. E-Notes 2001, 1, 139–142. [Google Scholar]
- Elwakil, A.S.; Abdou, M.A. New exact travelling wave solutions using modified extended tanh-function method. Chaos, Solitons Fractals 2007, 31, 840–852. [Google Scholar] [CrossRef]
- Fan, E. Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A 2000, 277, 212–218. [Google Scholar] [CrossRef]
- Aksoy, E.; Kaplan, M.; Bekir, A. Exponential rational function method for space-time fractional differential equations. Waves Random Complex Media 2016, 26, 142–151. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, H.Q. Fractional sub-equation method and its applications to nonlinear fractional PDEs. Phys. Lett. A 2011, 375, 1069–1073. [Google Scholar] [CrossRef]
- Feng, Z. On explicit exact solutions to the compound Burgers-KdV equation. Phys. Lett. A 2002, 293, 57–66. [Google Scholar] [CrossRef]
- Javeed, S.; Saif, S.; Waheed, A.; Baleanu, D. Exact solutions of fractional mBBM equation and coupled system of fractional Boussinesq-Burgers. Results Phys. 2018, 9, 1275–1281. [Google Scholar] [CrossRef]
- Javeed, S.; Saif, S.; Baleanu, D. New exact solutions of fractional Cahn–Allen equation and fractional DSW system. Adv. Differ. Equ. 2018, 1, 459. [Google Scholar] [CrossRef]
- Abdel-Salam, E.A.; Gumma, E.A. Analytical solution of nonlinear space–time fractional differential equations using the improved fractional Riccati expansion method. Ain Shams Eng. J. 2015, 6, 613–620. [Google Scholar] [CrossRef]
- Dehghan, M.; Salehi, R. The solitary wave solution of the two-dimensional regularized long-wave equation in fluids and plasma. Comput. Phys. Commun. 2011, 2, 305–320. [Google Scholar] [CrossRef]
- Aguero, M.; Najera, M.; Carrillo, M. Non classic solitonic structures in DNA’s vibrational dynamics. Int. J. Mod. Phys. B 2008, 22, 2571–2582. [Google Scholar] [CrossRef]
- Eslami, M. Solutions for space time fractional (2+1) dimensional dispersive long wave equations. Iran. J. Sci. Technol. Trans. A Sci. 2017, 41, 1027–1032. [Google Scholar] [CrossRef]
- Neirameh, A. New soliton solutions to the fractional perturbed nonlinear Schrodinger equation with power law nonlinearity. SeMA J. 2016, 73, 309–323. [Google Scholar] [CrossRef]
- Khalil, R.; Al-Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D.; Alsaedi, A. New properties of conformable derivative. Open Math. 2015, 13, 889–898. [Google Scholar] [CrossRef]
- Abdeljawad, T. On conformable fractional calculus. J. Comput. App. Math. 2015, 279, 57–66. [Google Scholar] [CrossRef]
- Benkhettoua, N.; Hassania, S.; Torres, D.F.M. A conformable fractional calculus on arbitrary time scales. J. King Saud Univ. Sci. 2016, 28, 93–98. [Google Scholar] [CrossRef]
- Tasbozan, O.; Cenesiz, Y.; Kurt, A. New solutions for conformable fractional Boussinesq and combined Kdv-mKdv equations using the Jacobi elliptic function expansion method. Eur. Phys. J. Plus 2016, 131, 244. [Google Scholar] [CrossRef]
- Hammad, M.A.; Khalil, R. Conformable fractional heat equation. Int. J. Pure Appl. Math. 2014, 94, 215–221. [Google Scholar]
- Lou, S.Y. Painleve test for the integrable dispersive long wave equation in two space dimensions. Phys. Lett. A 1993, 176, 96–100. [Google Scholar] [CrossRef]
- Paguin, G.; Winternitz, P. Group theoretical analysis of dispersive long wave equations in two space dimensions. Phys. D Nonlinear Phenom. 1990, 46, 122–138. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Javeed, S.; Riaz, S.; Saleem Alimgeer, K.; Atif, M.; Hanif, A.; Baleanu, D. First Integral Technique for Finding Exact Solutions of Higher Dimensional Mathematical Physics Models. Symmetry 2019, 11, 783. https://doi.org/10.3390/sym11060783
Javeed S, Riaz S, Saleem Alimgeer K, Atif M, Hanif A, Baleanu D. First Integral Technique for Finding Exact Solutions of Higher Dimensional Mathematical Physics Models. Symmetry. 2019; 11(6):783. https://doi.org/10.3390/sym11060783
Chicago/Turabian StyleJaveed, Shumaila, Sidra Riaz, Khurram Saleem Alimgeer, M. Atif, Atif Hanif, and Dumitru Baleanu. 2019. "First Integral Technique for Finding Exact Solutions of Higher Dimensional Mathematical Physics Models" Symmetry 11, no. 6: 783. https://doi.org/10.3390/sym11060783
APA StyleJaveed, S., Riaz, S., Saleem Alimgeer, K., Atif, M., Hanif, A., & Baleanu, D. (2019). First Integral Technique for Finding Exact Solutions of Higher Dimensional Mathematical Physics Models. Symmetry, 11(6), 783. https://doi.org/10.3390/sym11060783





