Beta Exponentiated Modified Weibull Distribution: Properties and Application
Abstract
1. Introduction
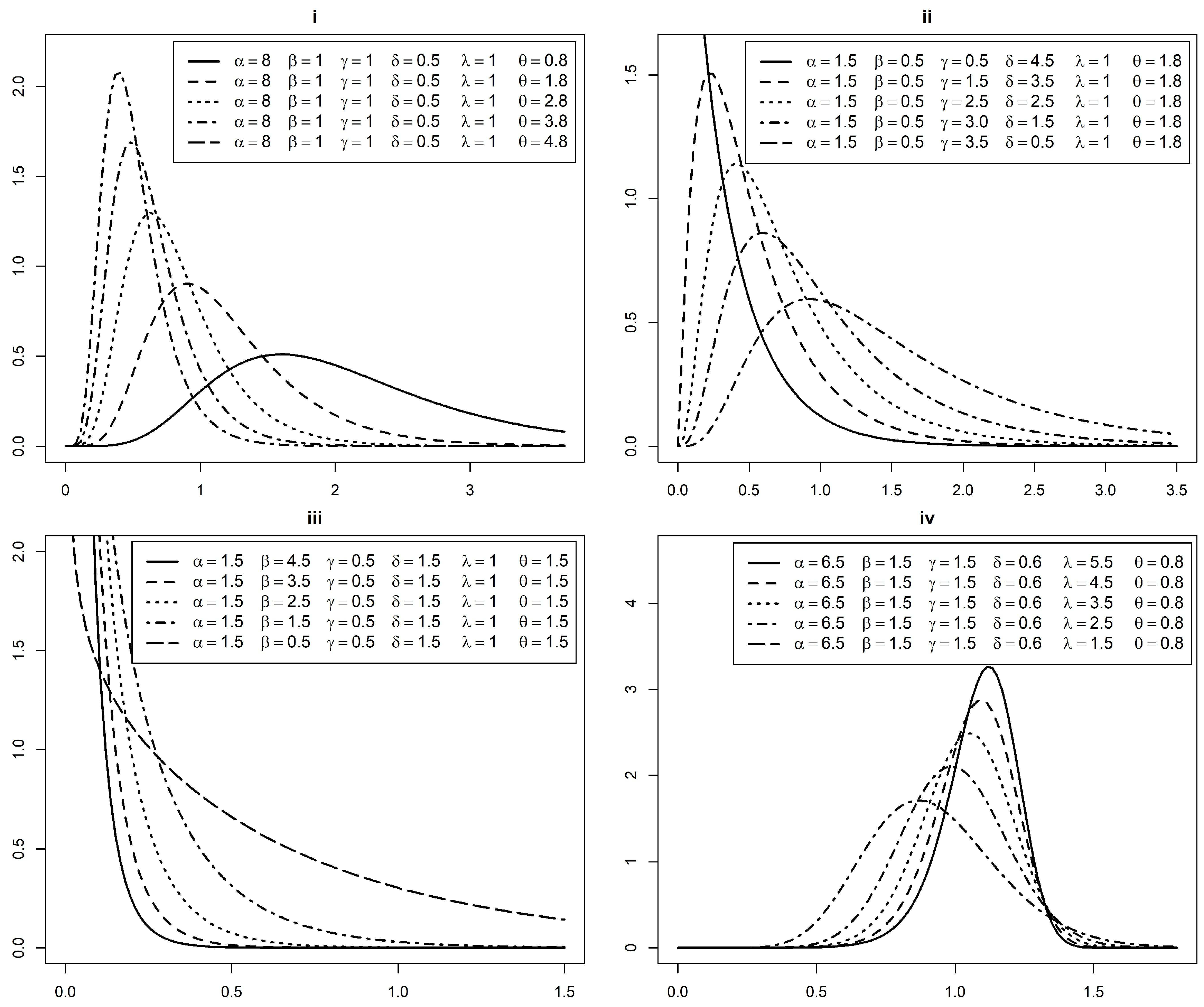
2. Beta Exponentiated Modified Weibull Distribution
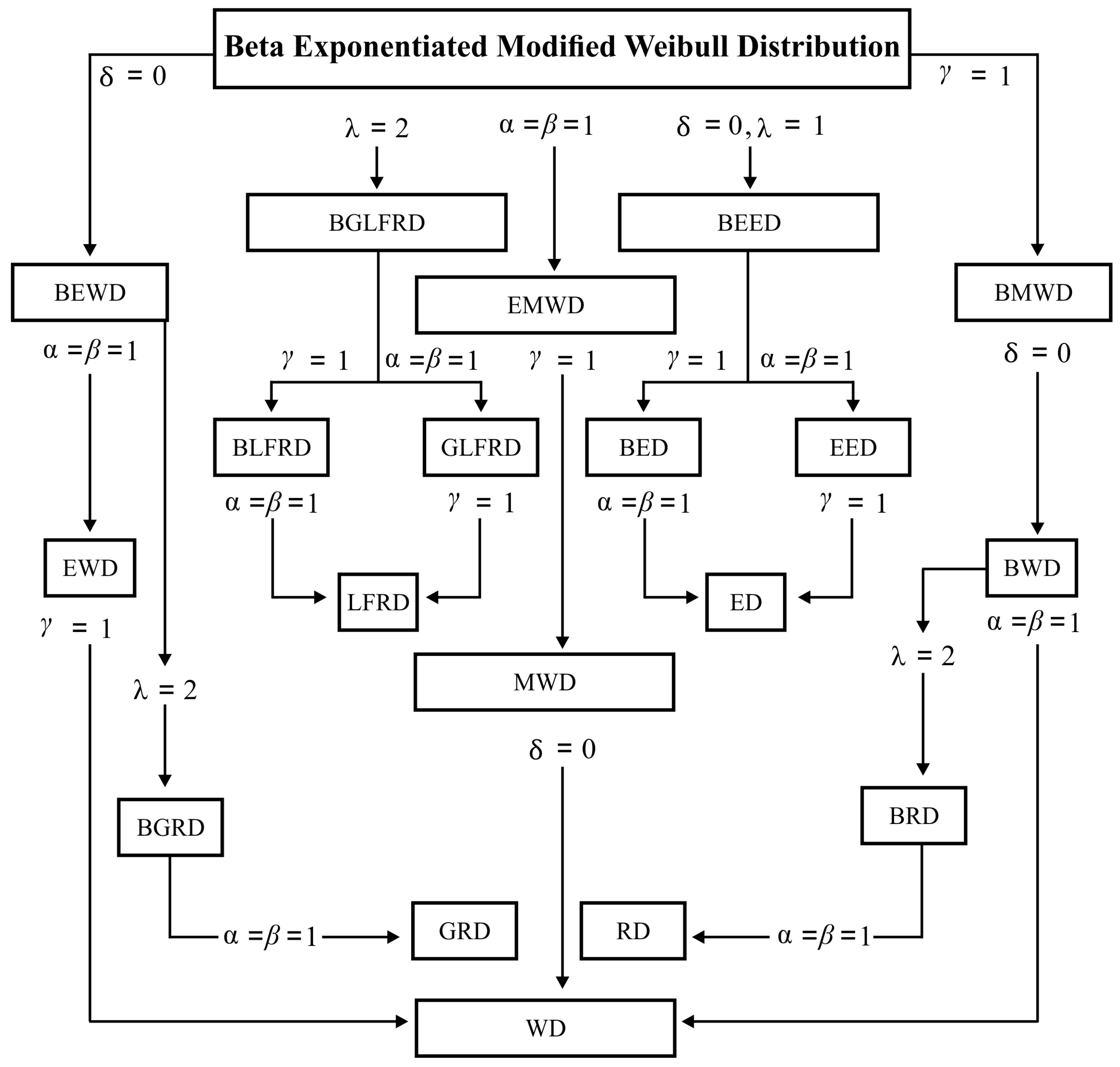
2.1. Special Cases of the BEMW Distribution
2.1.1. Beta Exponentiated Weibull (BEW) Distribution
2.1.2. Beta Generalized Rayleigh (BGR) Distribution
2.1.3. Beta Modified Weibull (BMW) Distribution
2.1.4. Beta Rayleigh (BR) Distribution
2.1.5. Beta Exponentiated Exponential (BEE) Distribution
2.1.6. Beta Generalized Linear Failure Rate (BGLFR) Distribution
2.1.7. Exponentiated Modified Weibull (EMW) Distribution
3. Expansion of the Probability Distribution and Density Function
4. Statistical Properties
Moments
5. Order Statistics of the Beta Exponentiated Modified Weibull Distribution
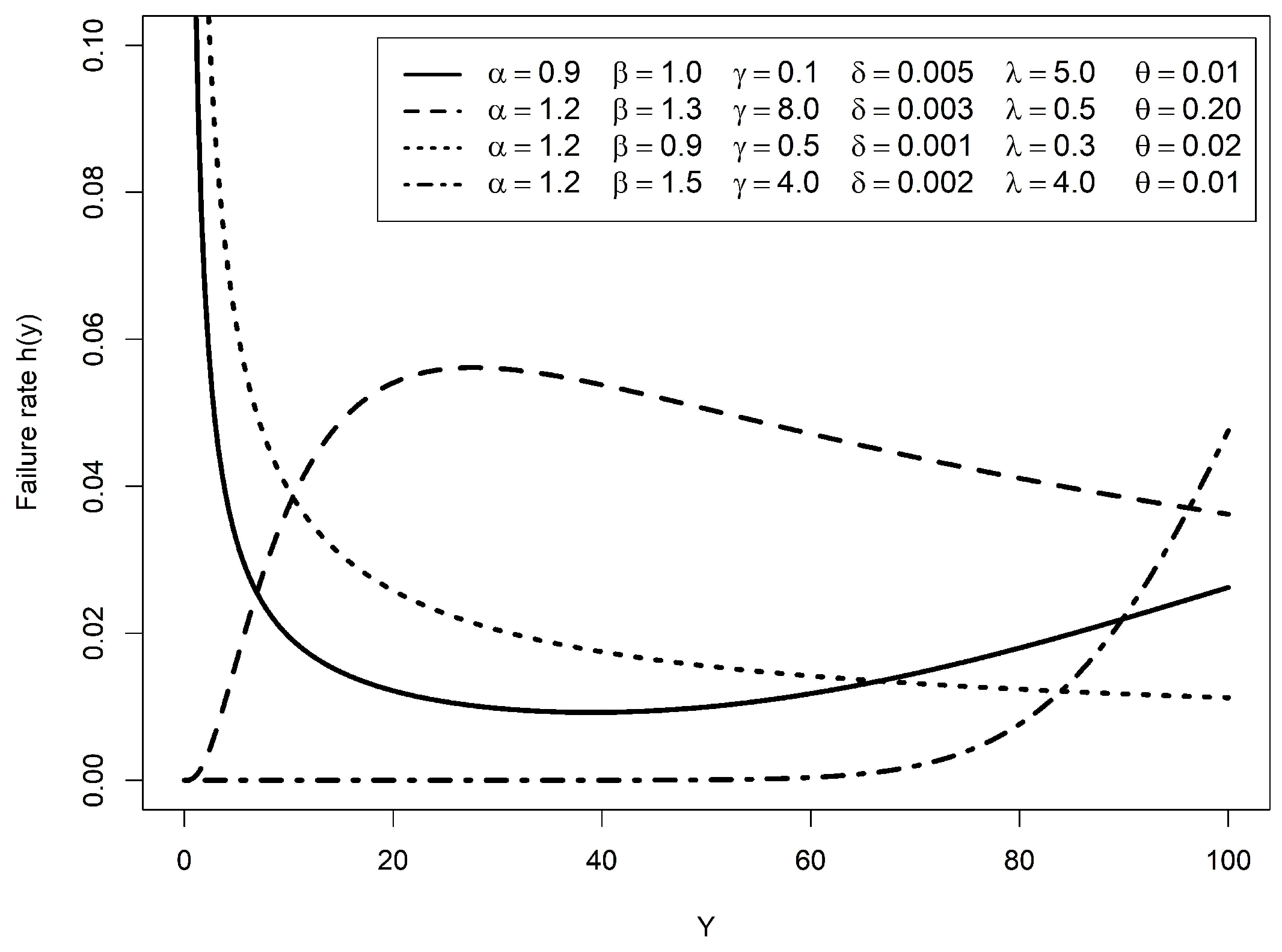
6. Reliability Analysis
6.1. Survival Function
6.2. Hazard Function
7. Parameter Estimation
7.1. Maximum Likelihood Estimation
7.2. Least Squares Estimation
8. Application
9. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Weibull, W.T. A statistical distribution function of wide applicability. J. Appl. Mech. 1951, 103, 293–297. [Google Scholar]
- Murthy, D.P.; Xie, M.; Jiang, R. Weibull Models; John Wiley and Sons: New York, NY, USA, 2004. [Google Scholar]
- Cordeiro, G.M.; Lemonte, A.J. On the Marshall-Olkin extended Weibull distribution. Stat. Pap. 2013, 54, 333–353. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Ortega, E.M.M.; Nadarajah, S. The Kumaraswamy Weibull distribution with application to failure data. J. Frankl. Inst. 2010, 347, 1399–1429. [Google Scholar] [CrossRef]
- Aryal, G.R.; Chris, P. Transmuted Weibull Distribution: A Generalization of the Weibull Probability Distribution. Eur. J. Pure Appl. Math. 2011, 4, 89–102. [Google Scholar]
- Pal, M.; Ali, M.M.; Woo, J. Exponentiated weibull distribution. Statistica 2006, 66, 139–147. [Google Scholar]
- Silva, G.O.; Ortega, E.M.M.; Cordeiro, G.M. The beta modified Weibull distribution. Lifetime Data Anal. 2010, 16, 409–430. [Google Scholar] [CrossRef]
- Lee, C.; Famoye, F.; Olumolade, O. Beta-Weibull Distribution: Some Properties and Applications to Censored Data. J. Mod. Appl. Stat. Methods 2017, 6, 173–186. [Google Scholar] [CrossRef]
- Saboor, A.; Pogany, T.K. Marshall-Olkin gamma-Weibull distribution with applications. Commun. Stat. Theory Methods 2016, 45, 1559–1563. [Google Scholar] [CrossRef]
- Pogány, T.K.; Saboor, A.; Provost, S. The Marshall–Olkin exponential Weibull distribution. Hacett. J. Math. Stat. 2015, 44, 1579–1594. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Ortega, E.M.; Lemonte, A.J. The exponential–Weibull lifetime distribution. J. Stat. Comput. Simul. 2014, 84, 2592–2606. [Google Scholar] [CrossRef]
- Leipnik, R.O.Y.B.; Pearce, C.E.M. Independent non-identical five-parameter gamma-Weibull variates and their sums. ANZIAM J. 2004, 46, 265–271. [Google Scholar] [CrossRef][Green Version]
- Mudholkar, G.S.; Srivastava, D.K. Exponentiated Weibull family for analyzing bathtub failure-rate data. IEEE Trans. Reliab. 1993, 42, 299–302. [Google Scholar] [CrossRef]
- Mudholkar, G.S.; Srivastava, D.K.; Freimer, M. The exponentiated Weibull family: A reanalysis of the bus-motor-failure data. Technometrics 1995, 37, 436–445. [Google Scholar] [CrossRef]
- Lai, C.D.; Xie, M.; Murthy, D.N.P. A modified Weibull distribution. IEEE Trans. Reliab. 2003, 52, 33–37. [Google Scholar] [CrossRef]
- Elbatal, I. Exponentiated modified Weibull distribution. Econ. Qual. Control 2011, 26, 189–200. [Google Scholar] [CrossRef]
- Famoye, F.; Lee, C.; O lumolade, O. The beta-Weibull distribution. J. Stat. Theory Appl. 2005, 4, 121–136. [Google Scholar]
- Singla, N.; Jain, K.; Kumar Sharma, S. The Beta Generalized Weibull distribution: Properties and applications. Reliab. Eng. Syst. Saf. 2012, 102, 5–15. [Google Scholar] [CrossRef]
- Pal, M.; Tiensuwan, M. The Beta Transmuted Weibull Distribution. Austrian J. Stat. 2015, 43, 133. [Google Scholar] [CrossRef]
- Eugene, N.; Lee, C.; Famoye, F. Beta-normal distribution and its applications. Commun. Stat. Theory Methods 2002, 31, 497–512. [Google Scholar] [CrossRef]
- Jones, M.C. Family of distributions arising from distribution of order statistics. Test 2004, 13, 1–43. [Google Scholar] [CrossRef]
- Paranaíba, P.F.; Ortega, E.M.; Cordeiro, G.M.; Pescim, R.R. The beta Burr XII distribution with application to lifetime data. Comput. Stat. Data Anal. 2011, 55, 1118–1136. [Google Scholar] [CrossRef]
- Nadarajah, S.; Kotz, S. The beta exponential distribution. Reliab. Eng. Syst. Saf. 2006, 91, 689–697. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Lemonte, A.J. The beta Laplace distribution. Stat. Probab. Lett. 2011, 81, 973–982. [Google Scholar] [CrossRef]
- Akinsete, A.; Famoye, F.; Lee, C. The beta-Pareto distribution. Statistics 2008, 42, 547–563. [Google Scholar] [CrossRef]
- Nadarajah, S.; Kotz, S. The beta Gumbel distribution. Math. Probl. Eng. 2004, 4, 323–332. [Google Scholar] [CrossRef]
- Castellares, F.; Montenegro, L.C.; Cordeiro, G.M. The beta log-normal distribution. J. Stat. Comput. Simul. 2013, 83, 203–228. [Google Scholar] [CrossRef]
- Alshawarbeh, E.; Lee, C.; Famoye, F. The beta-Cauchy distribution. J. Probab. Stat. Sci. 2012, 10, 41–57. [Google Scholar]
- Condino, F.; Domma, F.; Lee, C. The beta-Dagum distribution. In Proceedings of the 45th Scientific Meeting of the Italian Statistical Society, Padua, Italy, 29 June–1 July 2010. [Google Scholar]
- Cordeiro, G.M.; Cristino, C.T.; Hashimoto, E.M.; Ortega, E.M. The beta generalized Rayleigh distribution with applications to lifetime data. Stat. Pap. 2013, 54, 133–161. [Google Scholar] [CrossRef]
- Kundu, D.; Raqab, M.Z. Generalized Rayleigh distribution: Different methods of estimations. Comput. Stat. Data Anal. 2005, 49, 187–200. [Google Scholar] [CrossRef]
- Hughes, S.A.; Borgman, L.E. Beta-Rayleigh distribution for shallow water wave heights. J. Hydrodyn. 1987, 17–31. [Google Scholar]
- Barreto-Souza, W.; Santos, A.H.; Cordeiro, G.M. The beta generalized exponential distribution. J. Stat. Comput. Simul. 2010, 80, 159–172. [Google Scholar] [CrossRef]
- Gupta, R.D.; Kundu, D. Exponentiated exponential family: An alternative to gamma and Weibull distributions. Biom. J. 2001, 43, 117–130. [Google Scholar] [CrossRef]
- Jafari, A.A.; Mahmoudi, E. Beta-linear failure rate distribution and its applications. arXiv 2012, arXiv:1212.5615. [Google Scholar]
- Sarhan, A.M.; Kundu, D. Generalized linear failure rate distribution. Commun. Stat. Theory Methods 2009, 38, 642–660. [Google Scholar] [CrossRef]
- Sarhan, A.M.; Zaindin, M. Modified Weibull distribution. APPS Appl. Sci. 2009, 11, 123–136. [Google Scholar]
- Cordeiro, G.M.; Nadarajah, S. Closed-form expressions for moments of a class of beta generalized distributions. Braz. J. Probab. Stat. 2011, 25, 14–33. [Google Scholar] [CrossRef]
- Xu, K.; Xie, M.; Tang, L.C.; Ho, S.L. Application of neural networks in forecasting engine systems reliability. Appl. Soft Comput. 2003, 2, 255–268. [Google Scholar] [CrossRef]
- Danielsson, J. Financial Risk Forecasting: The Theory and Practice of Forecasting Market Risk with Implementation in R and Matlab; John Wiley and Sons: New York, NY, USA, 2011. [Google Scholar]
| Length | Average | Minimum | Maximum | Median | Standard Deviation | ||
|---|---|---|---|---|---|---|---|
| 40 | 6.2525 | 1.6 | 9.0 | 5.075 | 6.50 | 7.825 | 1.95553 |
| Model | AIC | BIC | AICc | ||||||
|---|---|---|---|---|---|---|---|---|---|
| BEMWD | 2.6629 | 0.9664 | 2.9256 | 0.2769 | 1.6231 | 0.3785 | 24.99 | 35.13 | 27.54 |
| (0.5594) | (2.0912) | (0.5068) | (7.7832) | (37.7734) | (23.1998) | ||||
| EMWD | - | - | 26.9086 | 7.6903 | 15.1406 | 0.01399 | 28.69 | 35.44 | 29.83 |
| (0.0916) | (15.0308) | (0.5086) | (742.7817) | ||||||
| MWD | - | - | - | 0.1895 | 0.2810 | 4.0529 | 34.34 | 39.41 | 35.01 |
| (7.3718) | (3.3962) | (2775.03) | |||||||
| ED | - | - | - | - | - | 0.1817 | 30.56 | 32.25 | 30.66 |
| (8.0248) |
| Model | AIC | BIC | AICc | ||||||
|---|---|---|---|---|---|---|---|---|---|
| BEMWD | 1.4268 | 1.3079 | 1.500 | 0.9999 | 1.9999 | 1.8999 | 18.34 | 28.47 | 20.89 |
| (3.7841) | (0.3475) | (0.0003) | (0.0019) | (0.0119) | (0.0115) | ||||
| EMWD | - | - | 1.5129 | 0.9689 | 1.8378 | 1.7407 | 34.34 | 41.10 | 35.48 |
| (0.0006) | (0.0483) | (0.1911) | (0.2035) | ||||||
| MWD | - | - | - | 0.9893 | 1.9366 | 1.8392 | 32.34 | 37.41 | 33.01 |
| (0.0179) | (0.0920) | (0.0925) | |||||||
| ED | - | - | - | - | - | 0.1353 | 24.56 | 26.25 | 24.66 |
| (9.8036) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shahzad, M.N.; Ullah, E.; Hussanan, A. Beta Exponentiated Modified Weibull Distribution: Properties and Application. Symmetry 2019, 11, 781. https://doi.org/10.3390/sym11060781
Shahzad MN, Ullah E, Hussanan A. Beta Exponentiated Modified Weibull Distribution: Properties and Application. Symmetry. 2019; 11(6):781. https://doi.org/10.3390/sym11060781
Chicago/Turabian StyleShahzad, Mirza Naveed, Ehsan Ullah, and Abid Hussanan. 2019. "Beta Exponentiated Modified Weibull Distribution: Properties and Application" Symmetry 11, no. 6: 781. https://doi.org/10.3390/sym11060781
APA StyleShahzad, M. N., Ullah, E., & Hussanan, A. (2019). Beta Exponentiated Modified Weibull Distribution: Properties and Application. Symmetry, 11(6), 781. https://doi.org/10.3390/sym11060781





