An Extension of the CODAS Approach Using Interval-Valued Intuitionistic Fuzzy Set for Sustainable Material Selection in Construction Projects with Incomplete Weight Information
Abstract
:1. Introduction
2. Literature survey
2.1. Material Selection in Construction Industry and Sustainability
2.2. MSP and Various MCDMs
3. Preliminaries
- Ifthen
- If, then
- If, then;
- If, then
- (1)
- 1 − β1 = β1c = ([r1,s1], [p1,q1])
- (2)
- β1 ∩ β2 = ([min(p1,p2), min(q1,q2)], [max(r1,r2), max(s1,s2)])
- (3)
- β1 ∪ β2 = ([max(p1,p2), max(q1,q2)], [min(r1,r2), min(s1,s2)])
- (4)
- β1 + β2 = ([p1 + p2 − p1p2, q1 + q2 − q1q2], [r1r2, s1s2])
- (5)
- β1 · α2 = ([p1p2, q1q2], [r1 + r2 − r1r2, s1 + s2 − s1s2])
- (6)
- λβ = ([1 − (1 − p)λ,1 − (1 − q)λ], [rλ, sλ])
- (7)
- βλ = ([pλ, qλ], [1 − (1 − r)λ,1 − (1 − s)λ])
4. Proposed CODAS Method Using IVIFNs
- (1)
- A weak ranking: ;
- (2)
- A strict ranking: ;
- (3)
- A ranking of differences: ;
- (4)
- A ranking with multiples: ;
- (5)
- An interval form: ;
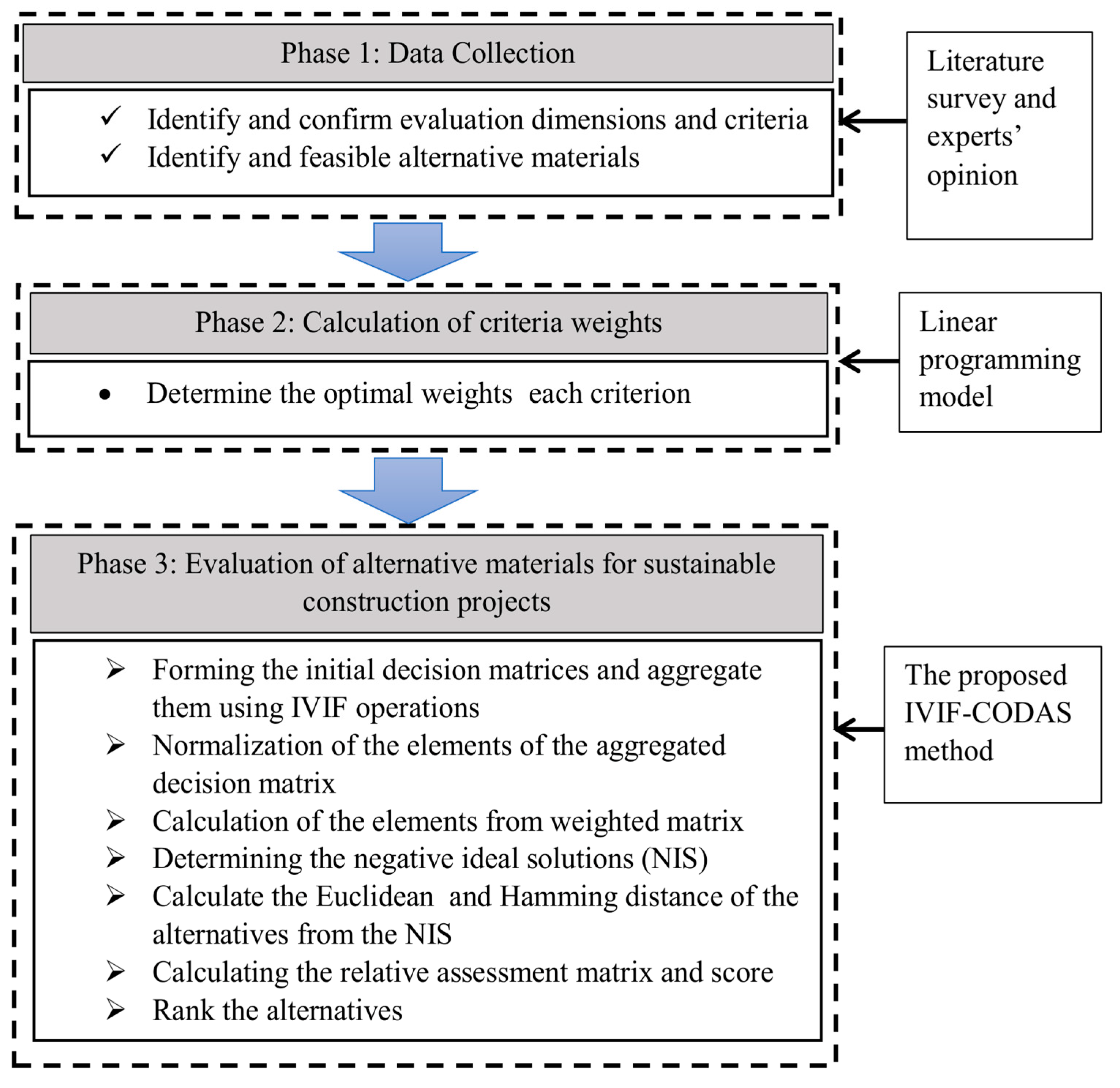
5. Application of the IVIF--CODAS in MSPs
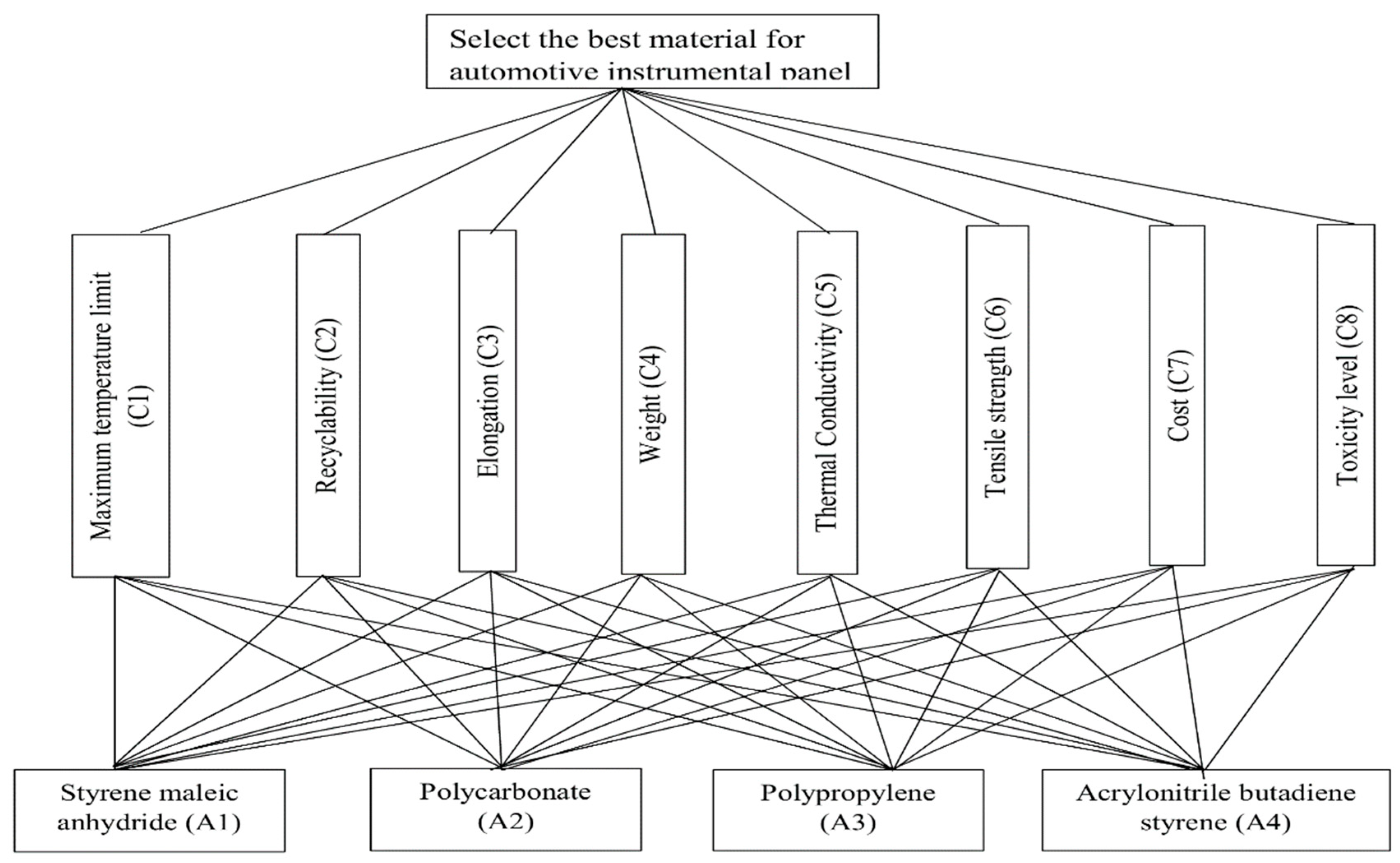
5.1. Illustrative Example
- The collective decision matrix is shown in Table 3 which is computed by aggregating the opinions of the decision makers (DM1, DM2, …, DM5).
- The consensus making model [39] is used to determine the weights of the evaluation criteria. In our study, the criteria weights are unknown while the weights of experts () are known in advance. Hence, applying model () and assuming that the criteria weights are partially known as follows: . To compute the criteria priorities, Equations (16)–(18) and model (M − 1) are used to develop the linear programming model given below:
- The final criteria weights () are obtained by solving the above model and they are represented by the following weight vector .
- Weighted normalized decision matrix, shown in Table 4, is computed by finding the normalized decision matrix and then combining the criteria weights obtained in the previous step with the normalized decision matrix.
- Interval-valued intuitionistic fuzzy negative ideal solution for each of the criteria is shown in Table 5.
- Hamming distance and Euclidean distance of the alternatives from the Interval-valued intuitionistic fuzzy negative ideal solution is shown in Table 6.
- Relative assessment matrix, assessment scores, and rank of the alternatives are given in Table 7.
- Table 8 shows the ranking orders by the proposed method along with four other existing methods for result comparisons.
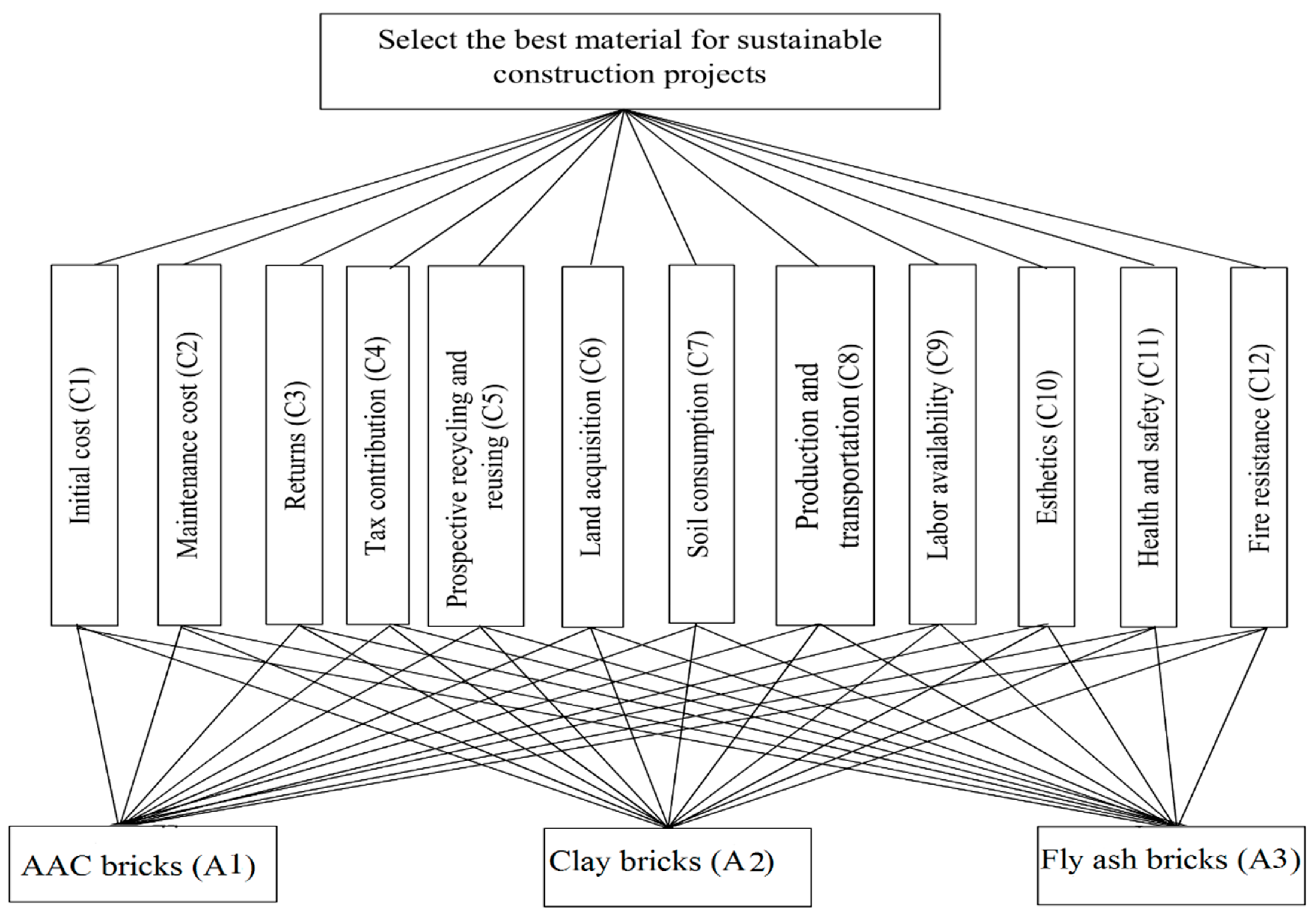
5.2. A Real Case of MSP in Sustainable Construction Projects
6. Result Discussion
6.1. Comparisons
- (1)
- Crisp ratings is used to evaluate the classical CODAS method but this ratings often fail in real life scenario as real life problems are much uncertain. For example, the construction company may consider some criteria as highly important and to signify the importance, the corresponding rating scale need to be more flexible. This “highly important” term can be preferably expressed using as an IVIF number rather than a single crisp number . However, in this paper, we use IVIF numbers to assess the alternative bricks and criteria importance since DMs can flexibly express their opinions using IVIF numbers.
- (2)
- Compared with fuzzy CODAS, the proposed IVIF-CODAS has an advantage. Grattan-Guinness [52] argued that it is a difficult task for decision makers to represent linguistic ratings in the form of a single membership degree in classical fuzzy set theory. In response, Atanassov [53] introduced IFS as an extension of fuzzy sets. In IFS, hesitation margin is introduced as a new concept and the sum of membership and non-membership degree may be less than one. However, both in fuzzy set and IFS, the membership values are exact and crisp in nature. To present the membership and non-membership values are in intervals, Atanassov and Gargov [8] extended IFS in IVIFS. Thus, in group decision making problems the extended IVIF-CODAS method offers a better treatment in handling uncertainty in the decision making process.
- (3)
- As it is difficult to show the applicability and trustworthiness of a newly proposed method, hence it is necessary to assess it in solving several MCDM problems. Wang et al. [54] asserted a comparison which is only way to apprehend the validity of newly proposed MCDM model (here, IVIF-CODAS). To justify any proposed approach, one has to compare it with several related approaches for the same problem. Accordingly, we have presented two illustrative examples and found encouraging results that show the similarity of IVIF-CODAS to other methods. One can consider this to be one of the advantages of the novel approach that is reckoned to be applicable irrespective of its case studies.
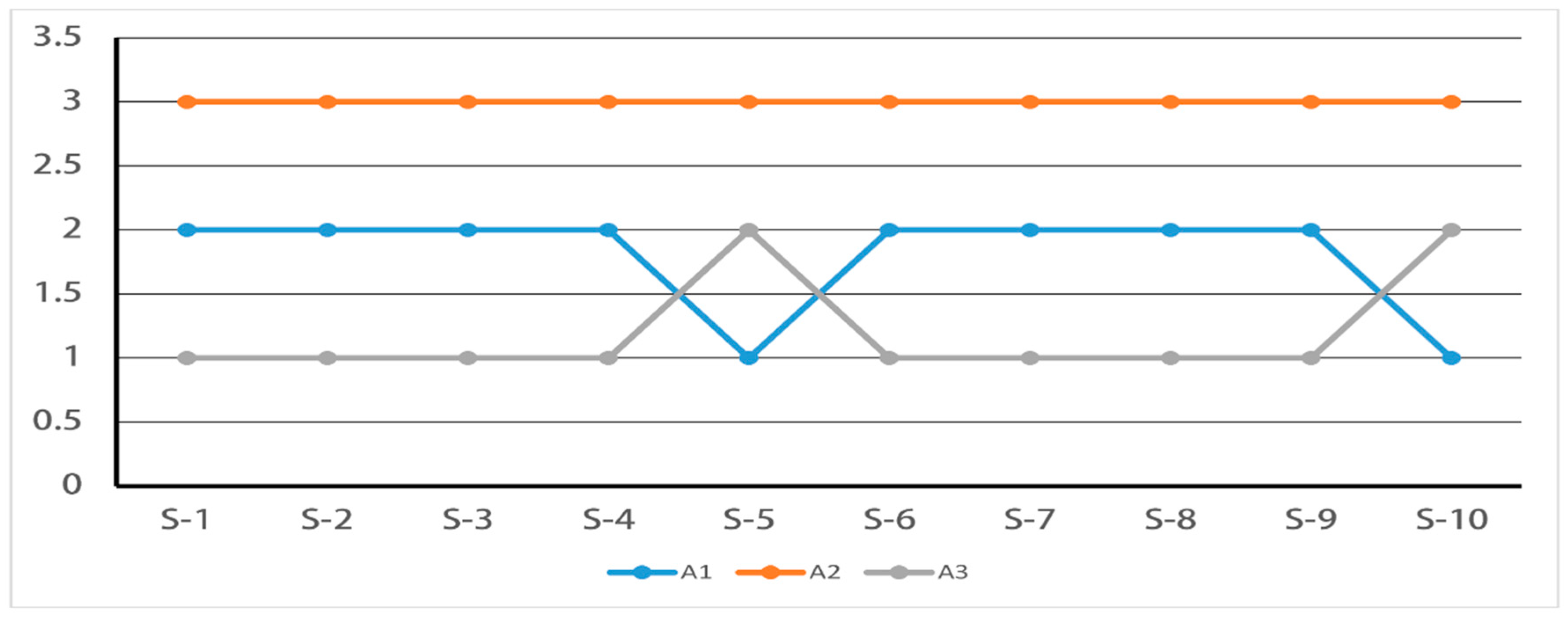
6.2. Sensitivity Analysis
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Jahan, A.; Edwards, K.L. A State-of-the-Art Survey on the Influence of Normalization Techniques in Ranking: Improving the Materials Selection Process in Engineering Design. Mater. Des. 2015, 65, 335–342. [Google Scholar] [CrossRef]
- Yazdani, M.; Payam, A.F. A Comparative Study on Material Selection of Microelectromechanical Systems Electrostatic Actuators Using Ashby, VIKOR and TOPSIS. Mater. Des. 2015, 65, 328–334. [Google Scholar] [CrossRef]
- Petković, D.; Madić, M.; Radovanović, M.; Gečevska, V. Application Of The Performance Selection Index Method For Solving Machining MCDM Problems. FU Mech. Eng. 2017, 15, 15–97. [Google Scholar] [CrossRef]
- Liao, T.W. Two Interval Type 2 Fuzzy TOPSIS Material Selection Methods. Mater. Des. 2015, 88, 1088–1099. [Google Scholar] [CrossRef]
- Liu, H.-C.; Liu, L.; Wu, J. Material Selection Using an Interval 2-Tuple Linguistic VIKOR Method Considering Subjective and Objective Weights. Mater. Des. 1980–2015 2013, 52, 158–167. [Google Scholar] [CrossRef]
- Mousavi, S.M.; Vahdani, B.; Tavakkoli-Moghaddam, R.; Tajik, N. Soft Computing Based on a Fuzzy Grey Group Compromise Solution Approach with an Application to the Selection Problem of Material Handling Equipment. Int. J. Comput. Integr. Manuf. 2014, 27, 547–569. [Google Scholar] [CrossRef]
- Xue, Y.-X.; You, J.-X.; Lai, X.-D.; Liu, H.-C. An Interval-Valued Intuitionistic Fuzzy MABAC Approach for Material Selection with Incomplete Weight Information. Appl. Soft Comput. 2016, 38, 703–713. [Google Scholar] [CrossRef]
- Atanassov, K.; Gargov, G. Interval Valued Intuitionistic Fuzzy Sets. Fuzzy Sets Syst. 1989, 31, 343–349. [Google Scholar] [CrossRef]
- Cheng, S.-H. Autocratic Multiattribute Group Decision Making for Hotel Location Selection Based on Interval-Valued Intuitionistic Fuzzy Sets. Inf. Sci. 2018, 427, 77–87. [Google Scholar] [CrossRef]
- Wen, M.; Zhao, H.; Xu, Z.; Lei, Q. Definite Integrals for Aggregating Continuous Interval-Valued Intuitionistic Fuzzy Information. Appl. Soft Comput. 2018, 70, 875–895. [Google Scholar] [CrossRef]
- Rashid, T.; Faizi, S.; Zafar, S. Distance Based Entropy Measure of Interval-Valued Intuitionistic Fuzzy Sets and Its Application in Multicriteria Decision Making. Adv. Fuzzy Syst. 2018, 2018, 1–10. [Google Scholar] [CrossRef]
- Wang, C.-Y.; Chen, S.-M. Multiple Attribute Decision Making Based on Interval-Valued Intuitionistic Fuzzy Sets, Linear Programming Methodology, and the Extended TOPSIS Method. Inf. Sci. 2017, 397–398, 155–167. [Google Scholar] [CrossRef]
- Gupta, P.; Mehlawat, M.K.; Grover, N.; Pedrycz, W. Multi-Attribute Group Decision Making Based on Extended TOPSIS Method under Interval-Valued Intuitionistic Fuzzy Environment. Appl. Soft Comput. 2018, 69, 554–567. [Google Scholar] [CrossRef]
- Das, S.; Kar, S.; Pal, T. Group Decision Making Using Interval-Valued Intuitionistic Fuzzy Soft Matrix and Confident Weight of Experts. J. Artif. Intell. Soft Comput. Res. 2014, 4, 57–77. [Google Scholar] [CrossRef]
- Das, S.; Kar, M.B.; Pal, T.; Kar, S. Multiple Attribute Group Decision Making Using Interval-Valued Intuitionistic Fuzzy Soft Matrix. In Proceedings of the 2014 IEEE International Conference on Fuzzy Systems (FUZZ-IEEE), Beijing, China, 7–11 July 2014; pp. 2222–2229. [Google Scholar] [CrossRef]
- Stanujkić, D.; Karabašević, D. An extension of the WASPAS method for decision-making problems with intuitionistic fuzzy numbers: A case of website evaluation. Oper. Res. Eng. Sci. Theor. Appl. 2018, 1, 29–39. [Google Scholar] [CrossRef]
- Gharzeldeen, M.N.; Beheiry, S.M. Investigating the Use of Green Design Parameters in UAE Construction Projects. Int. J. Sustain. Eng. 2015, 8, 93–101. [Google Scholar] [CrossRef]
- Popovic, M.; Kuzmanovic, M.; Savic, G. A comparative empirical study of Analytic Hierarchy Process and Conjoint analysis: Literature review. Decis. Mak. Appl. Manag. Eng. 2018, 1, 1–153. [Google Scholar] [CrossRef]
- Medineckiene, M.; Zavadskas, E.K.; Björk, F.; Turskis, Z. Multi-Criteria Decision-Making System for Sustainable Building Assessment/Certification. Arch. Civ. Mech. Eng. 2015, 15, 11–18. [Google Scholar] [CrossRef]
- Kibert, C.J. Sustainable Construction: Proceedings of the First International Conference of CIB TG 16, November 6-9, 1994, Tampa, Florida, USA; University of Florida Center: Gainesville, FL, USA, 1994. [Google Scholar]
- Radhi, H. On the Effect of Global Warming and the UAE Built Environment. In Global Warming; InTech: Vienna, Austria, 2010. [Google Scholar]
- Elchalakani, M.; Elgaali, E. Sustainable Concrete Made of Construction and Demolition Wastes Using Recycled Wastewater in the UAE. J. Adv. Concr. Technol. 2012, 10, 110–125. [Google Scholar] [CrossRef]
- Al-Hajj, A.; Hamani, K. Material Waste in the UAE Construction Industry: Main Causes and Minimization Practices. Archit. Eng. Des. Manag. 2011, 7, 221–235. [Google Scholar] [CrossRef]
- Ljungberg, L.Y. Materials Selection and Design for Development of Sustainable Products. Mater. Des. 2007, 28, 466–479. [Google Scholar] [CrossRef]
- Florez, L.; Castro-Lacouture, D. Optimization Model for Sustainable Materials Selection Using Objective and Subjective Factors. Mater. Des. 2013, 46, 310–321. [Google Scholar] [CrossRef]
- Bahraminasab, M.; Jahan, A. Material Selection for Femoral Component of Total Knee Replacement Using Comprehensive VIKOR. Mater. Des. 2011, 32, 4471–4477. [Google Scholar] [CrossRef]
- Jahan, A.; Mustapha, F.; Sapuan, S.M.; Ismail, M.Y.; Bahraminasab, M. A Framework for Weighting of Criteria in Ranking Stage of Material Selection Process. Int. J. Adv. Manuf. Technol. 2012, 58, 411–420. [Google Scholar] [CrossRef]
- Chauhan, A.; Vaish, R. Magnetic Material Selection Using Multiple Attribute Decision Making Approach. Mater. Des. 1980–2015 2012, 36, 1–5. [Google Scholar] [CrossRef]
- Karande, P.; Chakraborty, S. Application of Multi-Objective Optimization on the Basis of Ratio Analysis (MOORA) Method for Materials Selection. Mater. Des. 2012, 37, 317–324. [Google Scholar] [CrossRef]
- Maity, S.R.; Chatterjee, P.; Chakraborty, S. Cutting Tool Material Selection Using Grey Complex Proportional Assessment Method. Mater. Des. 1980–2015 2012, 36, 372–378. [Google Scholar] [CrossRef]
- Anojkumar, L.; Ilangkumaran, M.; Vignesh, M. A Decision Making Methodology for Material Selection in Sugar Industry Using Hybrid MCDM Techniques. Int. J. Mater. Prod. Technol. 2015, 51, 102–126. [Google Scholar] [CrossRef]
- Zavadskas, E.K.; Bausys, R.; Juodagalviene, B.; Garnyte-Sapranaviciene, I. Model for Residential House Element and Material Selection by Neutrosophic MULTIMOORA Method. Eng. Appl. Artif. Intell. 2017, 64, 315–324. [Google Scholar] [CrossRef]
- Tian, G.; Zhang, H.; Feng, Y.; Wang, D.; Peng, Y.; Jia, H. Green Decoration Materials Selection under Interior Environment Characteristics: A Grey-Correlation Based Hybrid MCDM Method. Renew. Sustain. Energy Rev. 2018, 81, 682–692. [Google Scholar] [CrossRef]
- Xu, Z.S. Methods for Aggregating Interval-Valued Intuitionistic Fuzzy Information and Their Application to Decision Making. Control Decis. 2007, 22, 215–219. [Google Scholar] [CrossRef]
- Xu, Z.-S.; Jian, C. Approach to Group Decision Making Based on Interval-Valued Intuitionistic Judgment Matrices. Syst. Eng.-Theory Pract. 2007, 27, 126–133. [Google Scholar] [CrossRef]
- Xu, Z.S.; Chen, J. An Overview of Distance and Similarity Measures of Intuitionistic Fuzzy Sets. Int. J. Uncertain. Fuzziness Knowl.-Based Syst. 2008, 16, 529–555. [Google Scholar] [CrossRef]
- Xu, Z. A Deviation-Based Approach to Intuitionistic Fuzzy Multiple Attribute Group Decision Making. Group Decis. Negot. 2010, 19, 57–76. [Google Scholar] [CrossRef]
- Park, J.H.; Lim, K.M.; Park, J.S.; Kwun, Y.C. Distances between Interval-Valued Intuitionistic Fuzzy Sets. J. Phys. Conf. Ser. 2008, 96, 012089. [Google Scholar] [CrossRef]
- Zhang, X.; Xu, Z. Soft Computing Based on Maximizing Consensus and Fuzzy TOPSIS Approach to Interval-Valued Intuitionistic Fuzzy Group Decision Making. Appl. Soft Comput. 2015, 26, 42–56. [Google Scholar] [CrossRef]
- Hashemi, H.; Bazargan, J.; Mousavi, S.M.; Vahdani, B. An Extended Compromise Ratio Model with an Application to Reservoir Flood Control Operation under an Interval-Valued Intuitionistic Fuzzy Environment. Appl. Math. Model. 2014, 38, 3495–3511. [Google Scholar] [CrossRef]
- Chen, T.-Y. Interval-Valued Intuitionistic Fuzzy QUALIFLEX Method with a Likelihood-Based Comparison Approach for Multiple Criteria Decision Analysis. Inf. Sci. 2014, 261, 149–169. [Google Scholar] [CrossRef]
- Pamučar, D.; Lukovac, V.; Božanić, D.; Komazec, N. Multi-criteria FUCOM-MAIRCA model for the evaluation of level crossings: Case study in the Republic of Serbia. Oper. Res. Eng. Sci. Theor. Appl. 2018, 1, 108–129. [Google Scholar] [CrossRef]
- Hassanpour, M. Evaluation of Iranian Wood and Cellulose Industries. Decis. Mak. Appl. Manag. Eng. 2019, 2, 13–34. [Google Scholar] [CrossRef]
- Dhanjode, C.S.; Ralegaonkar, R.V.; Dakwale, V.A. Design and Development of Sustainable Construction Strategy for Residential Buildings: A Case Study for Composite Climate. Int. J. Sustain. Constr. Eng. Technol. 2013, 4, 12–20. [Google Scholar]
- Mahendran, K.; Sivaram, T.; Shahulhameed, M.; Logaraja, R. A Comparative Study on Various Building Blocks as an Alternative to Conventional Bricks. In Proceedings of the International Conference on Emering Trends in Engineering and Management Research, Nashik, Maharastra, 23 March 2016; pp. 1097–1109. [Google Scholar]
- Benton, R. Reduce, Reuse, Recycle … and Refuse. J. Macromarket. 2015, 35, 111–122. [Google Scholar] [CrossRef]
- Hamid, Z.A.; Anuar Mohamad Kamar, K. Aspects of Off-site Manufacturing Application towards Sustainable Construction in Malaysia. Constr. Innov. 2012, 12, 4–10. [Google Scholar] [CrossRef]
- Pamucar, D.; Bozanic, D.; Lukovac, V.; Komazec, N. Normalized weighted geometric bonferroni mean operator of interval rough numbers—Application in interval rough DEMATEL-COPRAS. FU Mech. Eng. 2018, 16, 171–191. [Google Scholar] [CrossRef]
- Ashtiani, B.; Haghighirad, F.; Makui, A.; Montazer, G.A. Extension of Fuzzy TOPSIS Method Based on Interval-Valued Fuzzy Sets. Appl. Soft Comput. 2009, 9, 457–461. [Google Scholar] [CrossRef]
- Vahdani, B.; Hadipour, H.; Sadaghiani, J.S.; Amiri, M. Extension of VIKOR Method Based on Interval-Valued Fuzzy Sets. Int. J. Adv. Manuf. Technol. 2010, 47, 1231–1239. [Google Scholar] [CrossRef]
- Pamučar, D.; Petrović, I.; Ćirović, G. Modification of the Best–Worst and MABAC Methods: A Novel Approach Based on Interval-Valued Fuzzy-Rough Numbers. Expert Syst. Appl. 2018, 91, 89–106. [Google Scholar] [CrossRef]
- Grattan-Guinness, I. Fuzzy Membership Mapped onto Intervals and Many-Valued Quantities. Z. Für Math. Log. Grundlagen Math. 1976, 22, 149–160. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic Fuzzy Sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Wang, P.; Li, Y.; Wang, Y.-H.; Zhu, Z.-Q. A New Method Based on TOPSIS and Response Surface Method for MCDM Problems with Interval Numbers. Math. Probl. Eng. 2015, 2015, 1–11. [Google Scholar] [CrossRef]
- Debnath, A.; Roy, J.; Kar, S.; Zavadskas, E.; Antucheviciene, J. A Hybrid MCDM Approach for Strategic Project Portfolio Selection of Agro By-Products. Sustainability 2017, 9, 1302. [Google Scholar] [CrossRef]
- Chatterjee, P.; Mondal, S.; Boral, S.; Banerjee, A.; Chakraborty, S. A novel hybrid method for non-traditional machining process selection using factor relationship and Multi-Attributive Border Approximation Method. FU Mech. Eng. 2017, 15, 15–439. [Google Scholar] [CrossRef]
| Linguistic Terms | Corresponding IVIFNs |
|---|---|
| Very High (VH) | ([0.9,1], [0,0]) |
| High (H) | ([0.8,0.8], [0.1,0.1]) |
| Medium High (MH) | ([0.6,0.7], [0.2,0.3]) |
| Medium (M) | ([0.5,0.5], [0.4,0.5]) |
| Medium Low (ML) | ([0.3,0.4], [0.5,0.6]) |
| Low (L) | ([0.2,0.2], [0.7,0.7]) |
| Very Low (VL) | ([0,0.1], [0.8,0.9]) |
| Max | Max | Max | Max | Max | Max | Min | Min | ||
|---|---|---|---|---|---|---|---|---|---|
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | ||
| DM1 | A1 | MH | H | MH | MH | H | MH | MH | MH |
| A2 | M | MH | H | H | H | MH | H | MH | |
| A3 | VH | H | VH | H | H | VH | H | VH | |
| A4 | H | VH | H | H | VH | H | VH | H | |
| DM2 | A1 | MH | H | MH | MH | MH | H | MH | MH |
| A2 | MH | M | MH | M | MH | M | M | M | |
| A3 | H | VH | H | VH | H | VH | H | H | |
| A4 | H | H | VH | H | VH | H | VH | VH | |
| DM3 | A1 | MH | H | MH | H | MH | MH | H | MH |
| A2 | MH | MH | H | H | H | H | MH | H | |
| A3 | VH | VH | H | H | VH | VH | H | H | |
| A4 | H | VH | H | H | H | VH | H | H | |
| DM4 | A1 | H | MH | H | MH | MH | H | MH | H |
| A2 | MH | MH | M | MH | M | MH | M | MH | |
| A3 | H | VH | H | VH | H | H | VH | H | |
| A4 | H | H | VH | H | H | H | VH | VH | |
| DM5 | A1 | MH | H | MH | H | MH | H | MH | MH |
| A2 | H | MH | H | MH | H | MH | M | M | |
| A3 | VH | H | VH | H | VH | H | VH | H | |
| A4 | H | VH | H | H | H | VH | H | M |
| C1 | C2 | C3 | C4 | |
| A1 | ([0.636, 0.719], [0.181, 0.264]) | ([0.755, 0.779], [0.121, 0.144]) | ([0.636, 0.719], [0.181, 0.264]) | ([0.645, 0.724], [0.176, 0.255]) |
| A2 | ([0.668, 0.716], [0.204, 0.284]) | ([0.687, 0.753], [0.169, 0.247]) | ([0.775, 0.787], [0.136, 0.171]) | ([0.782, 0.800], [0.124, 0.157]) |
| A3 | ([0.931, 0.956], [0.021, 0.021]) | ([0.943, 0.978], [0.010, 0.010]) | ([0.915, 0.925], [0.036, 0.036]) | ([0.926, 0.946], [0.026, 0.026]) |
| A4 | ([0.925, 0.925 ], [0.036, 0.036]) | ([0.947, 0.967], [0.016, 0.016]) | ([0.941, 0.956], [0.021, 0.021]) | ([0.925, 0.925], [0.036, 0.036]) |
| C5 | C6 | C7 | C8 | |
| A1 | ([0.654, 0.729], [0.171, 0.245]) | ([0.713, 0.758], [0.141, 0.186]) | ([0.618, 0.709], [0.191, 0.282]) | ([0.636, 0.719], [0.181, 0.264]) |
| A2 | ([0.775, 0.787], [0.136, 0.171]) | ([0.727, 0.774], [0.149, 0.208]) | ([0.718, 0.741], [0.180, 0.237]) | ([0.727, 0.774], [0.149, 0.208]) |
| A3 | ([0.921, 0.935], [0.031, 0.031]) | ([0.954, 1.000], [0.000, 0.000]) | ([0.904, 0.904], [0.046, 0.046]) | ([0.915, 0.925], [0.036, 0.036]) |
| A4 | ([0.964, 1.000], [0.000, 0.000]) | ([0.925, 0.925], [0.036, 0.036]) | ([0.964, 1.000], [0.000, 0.000]) | ([0.941, 0.956], [0.021, 0.021]) |
| C1 | C2 | C3 | C4 | |
| A1 | ([0.042,0.049], [0.028,0.035]) | ([0.056,0.056], [0.014, 0.021]) | ([0.090,0.105], [0.030, 0.045]) | ([0.042,0.049], [0.014, 0.021]) |
| A2 | ([0.035,0.035], [0.028, 0.035]) | ([0.042,0.049], [0.014, 0.021]) | ([0.120,0.120], [0.030, 0.045]) | ([0.056,0.056], [0.014, 0.021]) |
| A3 | ([0.063,0.070], [0.028, 0.035]) | ([0.056,0.056], [0.014, 0.021]) | ([0.135,0.150], [0.030, 0.045]) | ([0.056,0.056], [0.014, 0.021]) |
| A4 | ([0.056,0.056], [0.028, 0.035]) | ([0.063,0.070], [0.014, 0.021]) | ([0.120,0.120], [0.030, 0.045]) | ([0.056,0.056], [0.014, 0.021]) |
| C5 | C6 | C7 | C8 | |
| A1 | ([0.144,0.144], [0.018, 0.018]) | ([0.087,0.102], [0.029, 0.044]) | ([0.102,0.119], [0.034, 0.051]) | ([0.087,0.102], [0.029, 0.044]) |
| A2 | ([0.144,0.144], [0.018, 0.018]) | ([0.087,0.102], [0.029, 0.044]) | ([0.102,0.119], [0.017, 0.017]) | ([0.087,0.102], [0.029, 0.044]) |
| A3 | ([0.144,0.144], [0.018, 0.018]) | ([0.087,0.102], [0.000, 0.000]) | ([0.102,0.119], [0.017, 0.017]) | ([0.087,0.102], [0.000, 0.000]) |
| A4 | ([0.162,0.180], [0.018, 0.018]) | ([0.087,0.102], [0.015, 0.015]) | ([0.102,0.119], [0.000, 0.000]) | ([0.087,0.102], [0.015, 0.015]) |
| Criteria | IVIF Negative Ideal Solutions |
|---|---|
| C1 | ([0.1407 0.1703], [0.7286 0.7692)] |
| C2 | ([0.1520 0.1791], [0.7167 0.7572)] |
| C3 | ([0.4668 0.5526], [0.2885 0.3857)] |
| C4 | ([0.1427 0.1722], [0.7191 0.7598)] |
| C5 | ([0.5886 0.7290], [0.1710 0.2450)] |
| C6 | ([0.5158 0.5787], [0.2650 0.3472)] |
| C7 | ([0.5401 0.6705], [0.2120 0.3007)] |
| C8 | ([0.4668 0.5526], [0.2885 0.3857)] |
| A1 | A2 | A3 | A4 | |
|---|---|---|---|---|
| ED | 0.0182 | 0.1899 | 0.767 | 0.8317 |
| HD | 0.0117 | 0.1264 | 0.5361 | 0.5824 |
| Relative Assessment Matrix | Appraisal Scores | Ranking | ||||
|---|---|---|---|---|---|---|
| A1 | A2 | A3 | A4 | |||
| A1 | 0 | −0.2864 | −1.2731 | −1.3842 | −2.9437 | 4 |
| A2 | 0.2864 | 0 | −0.9867 | −1.0977 | −1.798 | 3 |
| A3 | 1.2731 | 0.9867 | 0 | −0.1111 | 2.1487 | 2 |
| A4 | 1.3842 | 1.0977 | 0.1111 | 0 | 2.593 | 1 |
| MCDM Methods | Ranking Order |
|---|---|
| Classical CODAS | A4 > A3 > A2 >A1 |
| Fuzzy CODAS | A4 > A3 > A2 >A1 |
| IVIF-VIKOR | A3 > A4 > A2 >A1 |
| IVIF-TOPSIS | A4 > A3 > A2 >A1 |
| The proposed IVIF-CODAS | A4 > A3 > A2 >A1 |
| Decision Makers | Expertise |
|---|---|
| DM1 | Head of establishing standards and techniques with 21 years of work experience |
| DM2 | Health, Safety and Environment (HSE) management employee and the head of operations evaluation with 20 years of work experience |
| DM3 | Expert supervisor of construction project implementation with 21 years of work experience |
| DM4 | Project manager with 17 years of work experience |
| DM5 | Financial manager with 18 years of work experience |
| Dimension | Criteria | Description |
|---|---|---|
| Economical | Initial cost (C1) | Cost considered for purchasing/manufacturing of materials |
| Maintenance cost (C2) | Cost considered for maintaining in its lifetime | |
| Disposal cost (C3) | Cost considered for material disposal | |
| Tax contribution (C4) | Tax regarding the materials | |
| Environmental | Raw material extraction (C5) | It is necessary to manufacture the final material |
| Land acquisition (C6) | Land required for the material construction | |
| Soil consumption (C7) | It is required by the material at the time of manufacturing and operation | |
| Production and transportation (C8) | Comfortable transportation and production is important | |
| Social | Fire resistance (C9) | Necessary arrangements to resist fire |
| Esthetics (C10) | Looking of the material | |
| Use of local material (C11) | To develop society, more use of local material is needed | |
| Labor availability (C12) | Quality labor is vital for production |
| Dimension | Criteria | Local Weights | Global Weights | Rank |
|---|---|---|---|---|
| EC | - | 0.4268 | - | - |
| C1 | 0.3487 | 0.1488 | 2 | |
| C2 | 0.1857 | 0.0793 | 6 | |
| C3 | 0.4031 | 0.1720 | 1 | |
| C4 | 0.0625 | 0.0267 | 11 | |
| EN | - | 0.3568 | - | - |
| C5 | 0.3838 | 0.1369 | 3 | |
| C6 | 0.3396 | 0.1212 | 4 | |
| C7 | 0.0143 | 0.0051 | 12 | |
| C8 | 0.2623 | 0.0936 | 5 | |
| SO | - | 0.2164 | - | - |
| C9 | 0.2387 | 0.0517 | 9 | |
| C10 | 0.2180 | 0.0472 | 10 | |
| C11 | 0.3000 | 0.0649 | 7 | |
| C12 | 0.2434 | 0.0527 | 8 |
| C1 | C2 | C3 | C4 | C5 | C6 | C7 | C8 | C9 | C10 | C11 | C12 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| DM1 | A1 | MH | MH | MH | MH | MH | MH | M | MH | MH | H | H | H |
| A2 | H | H | H | MH | H | H | VH | MH | M | M | MH | M | |
| A3 | H | MH | H | MH | H | H | H | H | MH | H | H | H | |
| DM2 | A1 | VH | MH | MH | VH | MH | MH | M | MH | VH | H | H | H |
| A2 | H | VH | H | MH | H | VH | VH | MH | M | M | VH | VH | |
| A3 | H | MH | VH | MH | VH | H | VH | VH | MH | VH | H | H | |
| DM3 | A1 | MH | MH | MH | MH | MH | MH | M | MH | MH | MH | H | H |
| A2 | H | MH | H | MH | MH | H | MH | MH | M | M | MH | M | |
| A3 | H | MH | MH | MH | H | H | H | H | MH | H | H | MH | |
| DM4 | A1 | M | MH | MH | MH | MH | M | M | M | MH | H | H | H |
| A2 | H | M | H | M | M | H | M | MH | M | M | MH | M | |
| A3 | H | MH | M | MH | H | H | H | H | M | H | M | H | |
| DM5 | A1 | VH | MH | MH | VH | MH | MH | M | MH | VH | H | H | H |
| A2 | H | VH | H | MH | H | VH | VH | MH | M | M | VH | VH | |
| A3 | H | MH | VH | MH | VH | H | VH | VH | MH | VH | H | H |
| C1 | C2 | C3 | C4 | |
| A1 | ([0.673, 0.754], [0.174, 0.264]) | ([0.600, 0.700], [0.200, 0.144]) | ([0.600, 0.700], [0.200, 0.264]) | ([0.691, 0.793], [0.125, 0.255]) |
| A2 | ([0.800, 0.800], [0.100, 0.343]) | ([0.733, 0.785], [0.145, 0.356]) | ([0.800, 0.800], [0.100, 0.249]) | ([0.584, 0.666], [0.245, 0.288]) |
| A3 | ([0.800, 0.800], [0.100, 0.046]) | ([0.600, 0.700], [0.200, 0.046]) | ([0.733, 0.785], [0.145, 0.056]) | ([0.600, 0.700], [0.200, 0.056]) |
| C5 | C6 | C7 | C8 | |
| A1 | ([0.600, 0.700], [0.200, 0.245]) | ([0.584, 0.666], [0.245, 0.186]) | ([0.500, 0.500], [0.400, 0.282]) | ([0.584, 0.666], [0.245, 0.264]) |
| A2 | ([0.704, 0.726], [0.180, 0.249]) | ([0.834, 0.865], [0.061, 0.340]) | ([0.760, 0.839], [0.117, 0.383]) | ([0.600, 0.700], [0.200, 0.373]) |
| A3 | ([0.834, 0.865], [0.061, 0.076]) | ([0.800, 0.800], [0.100, 0.036]) | ([0.834, 0.865], [0.061, 0.066]) | ([0.834, 0.865], [0.061, 0.071]) |
| C9 | C10 | C11 | C12 | |
| A1 | ([0.691, 0.793], [0.125, 0.264]) | ([0.755, 0.779], [0.111, 0.264]) | ([0.800, 0.800], [0.100, 0.264]) | ([0.800, 0.800], [0.100, 0.264]) |
| A2 | ([0.500, 0.500], [0.400, 0.373]) | ([0.500, 0.500], [0.400, 0.373]) | ([0.691, 0.793], [0.125, 0.373]) | ([0.614, 0.637], [0.264, 0.373]) |
| A3 | ([0.584, 0.666], [0.245, 0.071]) | ([0.854, 0.904], [0.051, 0.071]) | ([0.746, 0.746], [0.170, 0.071]) | ([0.755, 0.779], [0.111, 0.071]) |
| C1 | C2 | C3 | C4 | |
| A1 | ([0.149, 0.180], [0.718, 0.763]) | ([0.133, 0.167], [0.727, 0.724]) | ([0.448, 0.557], [0.294, 0.376]) | ([0.129, 0.159], [0.702, 0.760]) |
| A2 | ([0.149, 0.180], [0.693, 0.788]) | ([0.133, 0.167], [0.708, 0.793]) | ([0.448, 0.557], [0.205, 0.363]) | ([0.129, 0.159], [0.743, 0.771]) |
| A3 | ([0.149, 0.180], [0.693, 0.693]) | ([0.133, 0.167], [0.727, 0.693]) | ([0.448, 0.557], [0.245, 0.200]) | ([0.129, 0.159], [0.727, 0.696]) |
| C5 | C6 | C7 | C8 | |
| A1 | ([0.540, 0.700], [0.200, 0.245]) | ([0.422, 0.508], [0.348, 0.329]) | ([0.437, 0.473], [0.416, 0.301]) | ([0.429, 0.512], [0.344, 0.477]) |
| A2 | ([0.540, 0.700], [0.180, 0.249]) | ([0.422, 0.508], [0.189, 0.456]) | ([0.437, 0.473], [0.140, 0.399]) | ([0.440, 0.538], [0.344, 0.477]) |
| A3 | ([0.540, 0.700], [0.061, 0.076]) | ([0.422, 0.508], [0.222, 0.206]) | ([0.437, 0.473], [0.085, 0.090]) | ([0.612, 0.665], [0.344, 0.477]) |
| C9 | C10 | C11 | C12 | |
| A1 | ([0.166, 0.217], [0.773, 0.786]) | ([0.181, 0.213], [0.773, 0.786]) | ([0.554, 0.598], [0.295, 0.502]) | ([0.192, 0.218], [0.722, 0.786]) |
| A2 | ([0.120, 0.137], [0.773, 0.786]) | ([0.120, 0.137], [0.773, 0.786]) | ([0.479, 0.593], [0.295, 0.502]) | ([0.147, 0.174], [0.722, 0.786]) |
| A3 | ([0.140, 0.182], [0.773, 0.786]) | ([0.205, 0.247], [0.773, 0.786]) | ([0.517, 0.558], [0.295, 0.502]) | ([0.181, 0.213], [0.722, 0.786]) |
| NIS | IVIF Negative Ideal Solutions |
|---|---|
| C1 | ([0.149, 0.180], [0.718, 0.788]) |
| C2 | ([0.133, 0.167], [0.727, 0.793]) |
| C3 | ([0.448, 0.557], [0.294, 0.376]) |
| C4 | ([0.129, 0.159], [0.743, 0.771]) |
| C5 | ([0.540, 0.700], [0.200, 0.249]) |
| C6 | ([0.422, 0.508], [0.348, 0.456]) |
| C7 | ([0.437, 0.473], [0.416, 0.399]) |
| C8 | ([0.429, 0.512], [0.344, 0.477]) |
| C9 | ([0.120, 0.137], [0.773, 0.786]) |
| C10 | ([0.120, 0.137], [0.773, 0.786]) |
| C11 | ([0.479, 0.558], [0.295, 0.502]) |
| C12 | ([0.147, 0.174], [0.722, 0.786]) |
| A1 | A2 | A3 | |
|---|---|---|---|
| ED | 0.3519 | 0.3258 | 0.9647 |
| HD | 0.2107 | 0.1682 | 0.6426 |
| Relative Assessment Matrix | Appraisal Scores | Ranking | |||
|---|---|---|---|---|---|
| A1 | A2 | A3 | |||
| A1 | 0.000 | 0.069 | −1.045 | −0.976 | 2 |
| A2 | −0.069 | 0.000 | −1.113 | −1.182 | 3 |
| A3 | 1.045 | 1.113 | 0.000 | 2.158 | 1 |
| MCDM Methods | Ranking Order |
|---|---|
| Classical CODAS | A3 > A2 > A1 |
| Fuzzy CODAS | A3 > A1 > A2 |
| IVIF-VIKOR | A3 > A2 > A1 |
| IVIF-TOPSIS | A3 > A1 > A2 |
| The proposed IVIF-CODAS | A3 > A1 > A2 |
| Alternatives | Scenario 1 | Scenario 2 | Scenario 3 | Scenario 4 | Scenario 5 | Scenario 6 | Scenario 7 | Scenario 8 | Scenario 9 | Scenario 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| A1 | 2 | 2 | 2 | 2 | 1 | 2 | 2 | 2 | 2 | 1 |
| >A2 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 |
| >A3 | 1 | 1 | 1 | 1 | 2 | 1 | 1 | 1 | 1 | 2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roy, J.; Das, S.; Kar, S.; Pamučar, D. An Extension of the CODAS Approach Using Interval-Valued Intuitionistic Fuzzy Set for Sustainable Material Selection in Construction Projects with Incomplete Weight Information. Symmetry 2019, 11, 393. https://doi.org/10.3390/sym11030393
Roy J, Das S, Kar S, Pamučar D. An Extension of the CODAS Approach Using Interval-Valued Intuitionistic Fuzzy Set for Sustainable Material Selection in Construction Projects with Incomplete Weight Information. Symmetry. 2019; 11(3):393. https://doi.org/10.3390/sym11030393
Chicago/Turabian StyleRoy, Jagannath, Sujit Das, Samarjit Kar, and Dragan Pamučar. 2019. "An Extension of the CODAS Approach Using Interval-Valued Intuitionistic Fuzzy Set for Sustainable Material Selection in Construction Projects with Incomplete Weight Information" Symmetry 11, no. 3: 393. https://doi.org/10.3390/sym11030393
APA StyleRoy, J., Das, S., Kar, S., & Pamučar, D. (2019). An Extension of the CODAS Approach Using Interval-Valued Intuitionistic Fuzzy Set for Sustainable Material Selection in Construction Projects with Incomplete Weight Information. Symmetry, 11(3), 393. https://doi.org/10.3390/sym11030393






