On the Symmetry of the Bone Structure Density over the Nasopalatine Foramen via Accurate Fractal Dimension Analysis
Abstract
:1. Introduction
- Body: it is most of the bone, pyramidal, is part of orbit, nasal cavity, infratemporal fossa, and the middle third of the face. It presents, in its anterior region, both the anterior nasal spine and the nasal notch.
- Frontal apophysis, which is articulated with nasal, frontal, ethmoid, and lacrimal bones.
- Zygomatic apophysis, that is articulated medially with the maxillary process of zygomatic bone.
- Palatine process, extending medially by forming the greatest part of hard palate, articulating in the middle line with the contralateral maxilla one, and later with palatal bone, and
- Alveolar process, which supports the upper teeth. The convex region that covers canine by vestibular is the canine eminence. There is a concavity mesial to this, i.e., the incisive fossa. Also, the canine fossa is a concavity which is distal to the canine one. The most posterior region of the alveolar process is the tuberosity of the maxilla [2].
- Anterior zone, which covers from the intermaxillary suture to the canine eminence.
- Middle zone: canine eminence and zygomatic-alveolar or infratemporal crest, and
- Posterior zone: distal to zygomatic-alveolar crest.
2. Materials and Methods
3. Results and Discussion
3.1. Description of the Sample
- Age: it was found a mean age equal to years with a standard deviation of years.
- DCV: a mean of and a standard deviation equal to were found.
- DCP: with a mean of and a standard deviation equal to .
- DVD: a mean of and a standard deviation of were obtained.
- DVI: with a mean of and a standard deviation equal to .
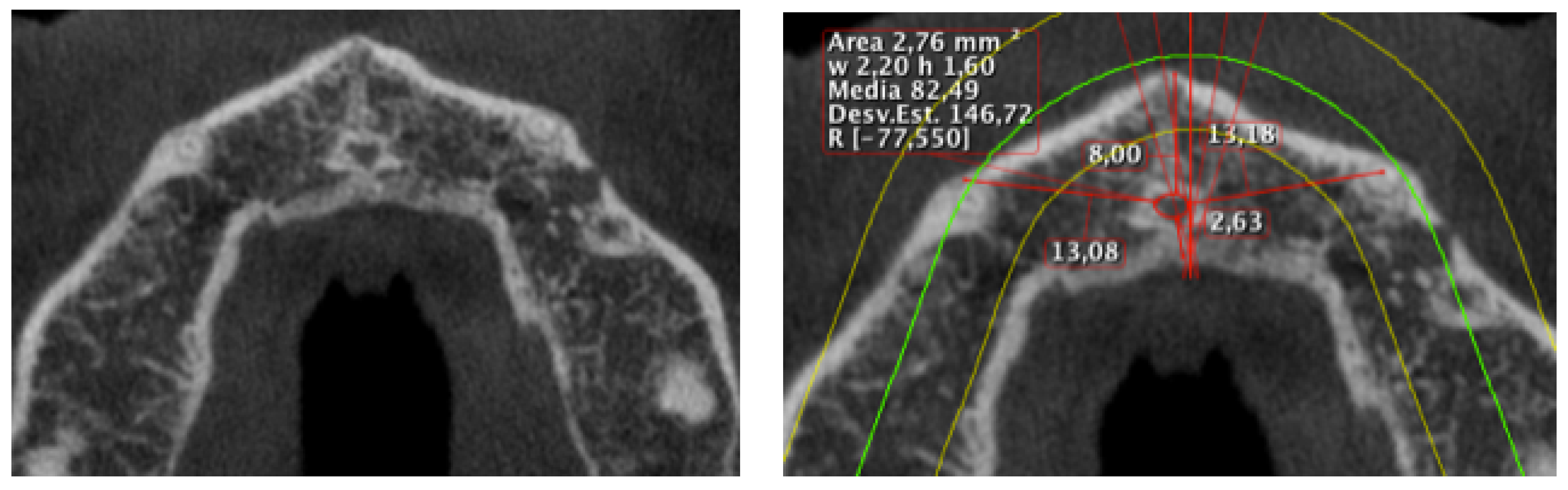
- Area: a mean of and a standard deviation equal to were found.
- W: a mean equal to and a standard deviation of were found.
- H: with a mean equal to and a standard deviation of .
- Mean: a mean equal to and a standard deviation of was obtained.
- DIM: a mean fractal dimension of and a standard deviation equal to were found.
3.2. Sample Description by Sex
3.2.1. Female Population
- Age: a mean age of years with a standard deviation equal to years was found.
- DCV: a mean equal to and a standard deviation of were obtained.
- DCP: with a mean equal to and a standard deviation equal to .
- DVD: a mean of and a standard deviation equal to were found.
- DVI: it was found a mean equal to with a standard deviation of .
- Area: with a mean equal to and a standard deviation equal to .
- W: a mean equal to and a standard deviation of were found.
- H: a mean of and a standard deviation equal to were found.
- Mean: a mean of and a standard deviation of were obtained.
- DIM: a mean fractal dimension of and a standard deviation equal to were found.
3.2.2. Male Population
- Age: it was found a mean age equal to years with a standard deviation of years.
- DCV: a mean of and a standard deviation equal to were found.
- DCP: with a mean of and a standard deviation equal to .
- DVD: a mean of and a standard deviation of were obtained.
- DVI: with a mean equal to and a standard deviation of .
- Area: a mean of and a standard deviation equal to were found.
- W: a mean equal to and a standard deviation of were found.
- H: with a mean of and a standard deviation equal to .
- Mean: a mean equal to and a standard deviation of were obtained.
- DIM: a mean fractal dimension equal to and a standard deviation of were found.
3.3. Some Comparisons by Sex
- Age: no significative differences were found. In fact, a p-value equal to was obtained by the Mann–Whitney test.
- DCV: in this case, significative differences were found by a p-value of 0.01* (* means that significative differences were found at a confidence level of 95%.) in the Mann–Whitney test.
- DCP: no significative differences were found by a Mann–Whitney p-value equal to .
- DVD: a p-value of was provided by the Mann–Whitney test. As such, no significative differences were found.
- DVI: the Mann–Whitney test provided a p-value equal to . Thus, no significative differences were found.
- Area: no differences were observed. In fact, the Mann–Whitney test provided a p-value equal to .
- W: a p-value of was found in the Mann–Whitney test. As such, no significative differences were found.
- H: There were no significative differences. In fact, the Mann–Whitney test provided a p-value of .
- Mean: the Mann–Whitney test threw a p-value equal to , so no significative differences were found.
- DIM: no significative differences were found. In fact, a p-value of was provided by the Mann–Whitney test.
3.4. A First Step towards Symmetry
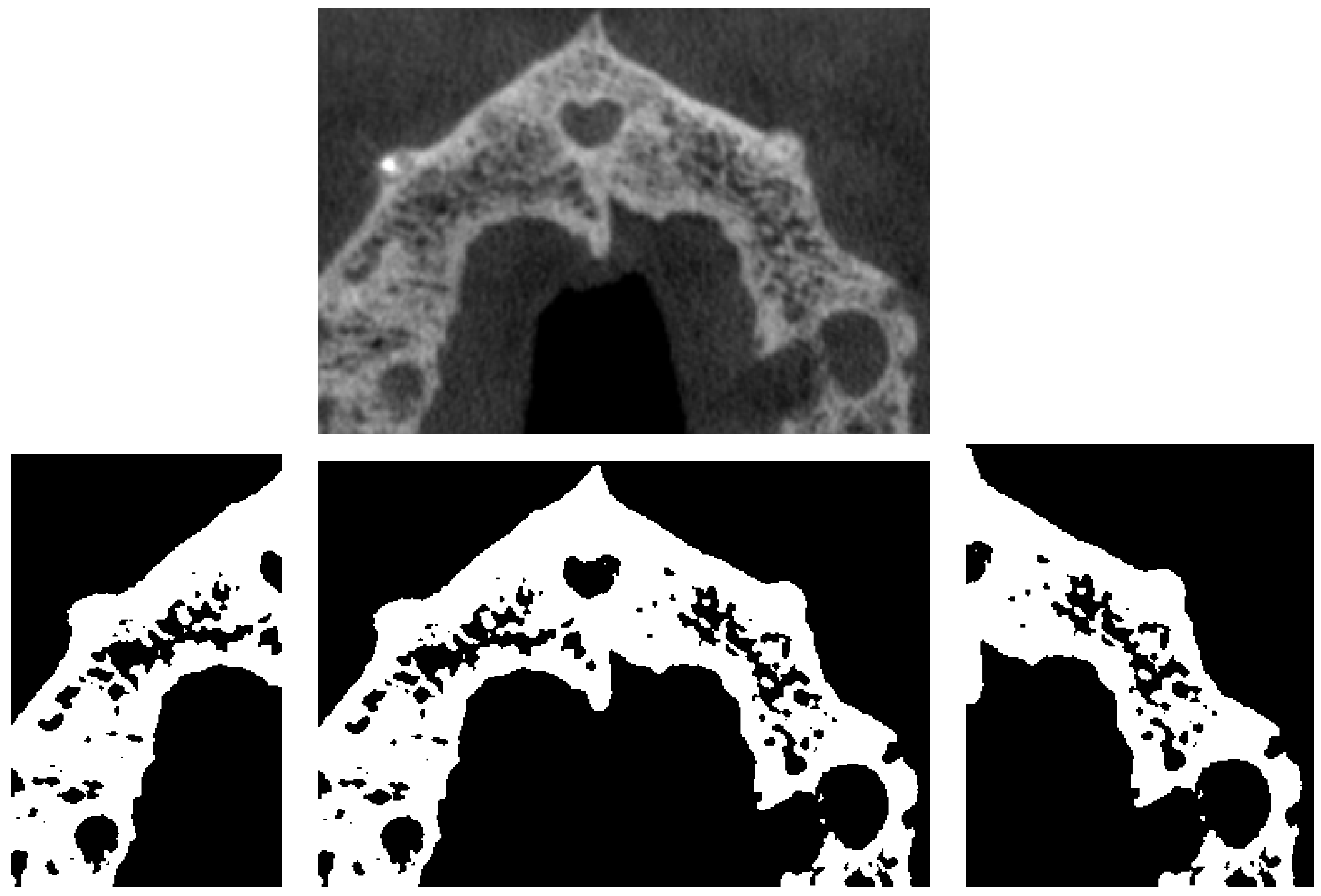
3.5. Fractal Dimension Analysis
3.6. Fractal Dimension Analysis for Group One Patients
3.7. Fractal Dimension Analysis for Patients in Group Two
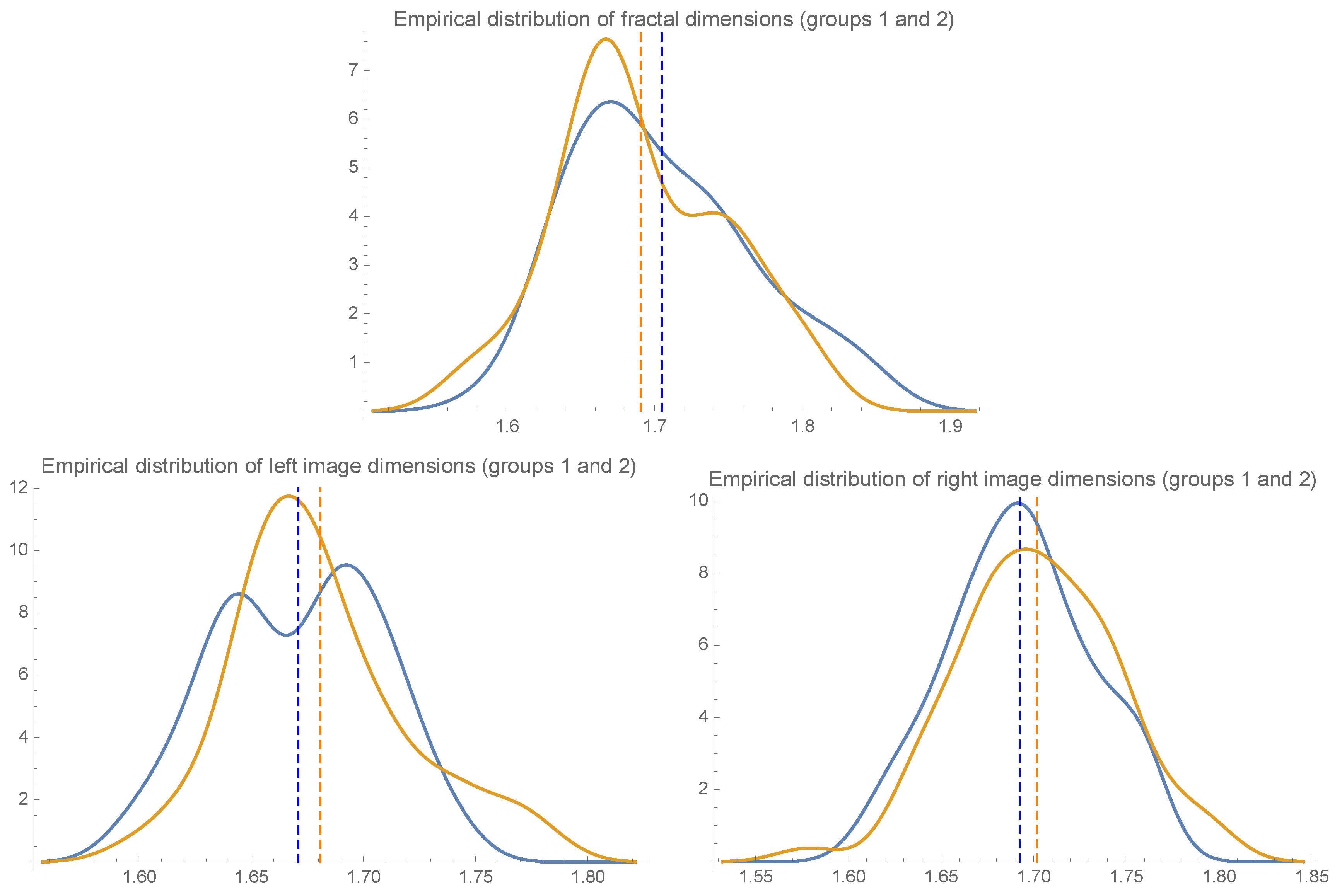
3.8. Analysis of Fractal Dimension by Groups
Fractal Dimension Comparison between Groups One and Two
3.9. A Note on the Multiple Comparisons Problem
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
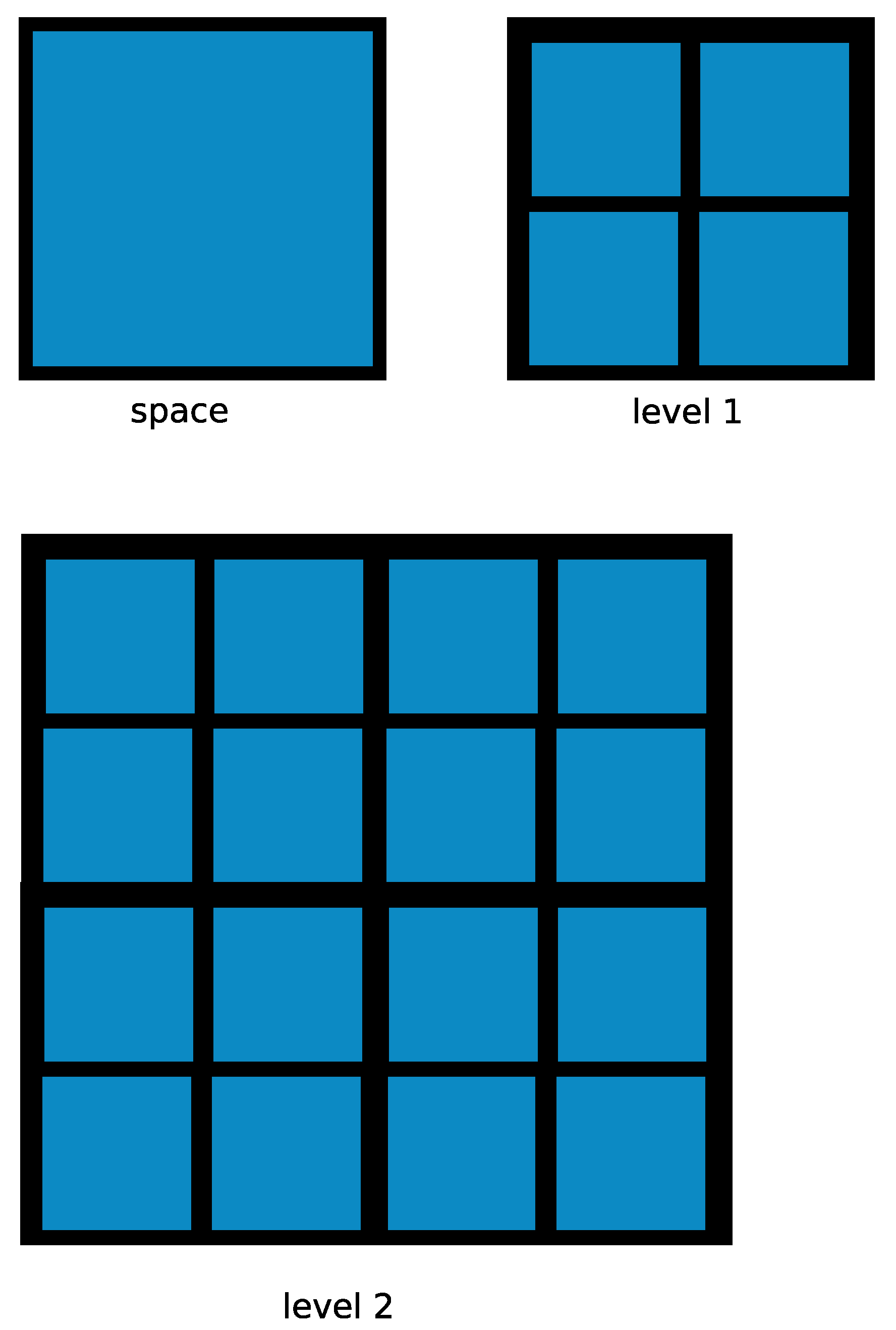
Appendix A. Mathematical Foundations on Fractal Dimension Calculations
- (i)
- for each , there exists such that .
- (ii)
- for all .
References
- Gómez de Ferraris, M.E.; Campos Muñoz, A. Histología, Embriología e Ingeniería Tisular Bucodental; Panamericana: Madrid, Spain, 2009; ISBN 978-607-7743-01-9. [Google Scholar]
- Velayos Santana, J.L. Anatomía de la Cabeza para Odontólogos; Panamericana: Madrid, Spain, 2007; ISBN 978-84-9835-068-5. [Google Scholar]
- López Muñiz, A.; Hernández González, L.C.; del Valle Soto, M.; Suárez Garnacho, S.; Carbajo Pérez, E.; Junceda Moreno, J. Anatomía Topográfica Humana; Ediciones de la Universidad de Oviedo: Oviedo, Spain, 2008; ISBN 978-84-7468-911-2. [Google Scholar]
- Nart Molina, J.; Marcuschamer Gittler, E.; Rumeu Milá, J.; Santos Alemany, A.; Griffin, T.J. Preservación del reborde alveolar. Por qué y cuándo. Periodoncia y Osteointegración 2007, 17, 229–237. [Google Scholar]
- Chimenos Kustner, E.; Ribera Uribe, M.; López López, J. Gerodontología; Sociedad Española de Gerodontología: Santiago de Compostela, Spain, 2012; ISBN 978-84-695-3382-6. [Google Scholar]
- Misch, C.E. Contemporary Implant Dentistry; Mosby Elsevier: St. Louis, MI, USA, 2008; ISBN 978-0-323-04373-1. [Google Scholar]
- Shah, N.; Bansal, N.; Logani, A. Recent advances in imaging technologies in dentistry. World J. Radiol. 2014, 6, 794–807. [Google Scholar] [CrossRef] [PubMed]
- Bornstein, M.M.; Balsiger, R.; Sendi, P.; von Arx, T. Morphology of the nasopalatine canal and dental implant surgery: A radiographic analysis of 100 consecutive patients using limited cone beam computed tomography. Clin. Oral Implants Res. 2011, 22, 295–301. [Google Scholar] [CrossRef] [PubMed]
- Liang, X.; Jacobs, R.; Martens, W.; Hu, Y.Q.; Adriaensens, P.; Quirynen, M.; Lambrichts, I. Macro- and micro-anatomical, histological and computed tomography scan characterization of the nasopalatine canal. J. Clin. Periodontol. 2009, 36, 598–603. [Google Scholar] [CrossRef] [PubMed]
- Hakbilen, S.; Magat, G. Evaluation of anatomical and morphological characteristics of the nasopalatine canal in a Turkish population by cone beam computed tomography. Folia Morphol. 2018, 77, 527–535. [Google Scholar] [CrossRef] [PubMed]
- Ruttimann, U.E.; Webber, R.L.; Hazelrig, J.B. Fractal dimension from radiographs of peridental alveolar bone. Oral Surg. Oral Med. Oral Pathol. 1992, 74, 98–110. [Google Scholar] [CrossRef]
- Pérez-García, V.M.; Fitzpatrick, S.; Pérez-Romasanta, L.A.; Pesic, M.; Schucht, P.; Arana, E.; Sánchez-Gómez, P. Applied mathematics and nonlinear sciences in the war on cancer. Appl. Math. Nonlinear Sci. 2016, 1, 423–436. [Google Scholar] [CrossRef]
- Rojas, C.; Belmonte-Beitia, J. Optimal control problems for differential equations applied to tumor growth: State of the art. Appl. Math. Nonlinear Sci. 2018, 3, 375–402. [Google Scholar] [CrossRef]
- Fernández-Martínez, M.; Gómez García, F.J.; Sánchez Guerrero, Y.; López Jornet, P. An intelligent system to study the fractal dimension of trabecular bones. J. Intell. Fuzzy Syst. 2018, 35, 4533–4540. [Google Scholar] [CrossRef]
- Fernández-Martínez, M. A survey on fractal dimension for fractal structures. Appl. Math. Nonlinear Sci. 2016, 1, 437–472. [Google Scholar] [CrossRef]
| Whole Sample (n = 130) | Female Group (n = 68) | Male Group (n = 62) | ||||
|---|---|---|---|---|---|---|
| Attribute | Mean | Std. Dev. | Mean | Std. Dev. | Mean | Std. Dev. |
| Age | 53.67 | 8.20 | 54.62 | 8.99 | 53.03 | 7.05 |
| DCV | 7.54 | 1.53 | 7.29 | 1.63 | 8.75 | 1.35 |
| DCP | 3.99 | 1.65 | 4.05 | 1.69 | 4.13 | 1.26 |
| DVD | 12.95 | 1.75 | 12.82 | 1.62 | 13.71 | 1.96 |
| DVI | 12.98 | 1.34 | 12.38 | 1.62 | 13.13 | 1.70 |
| Area | 5.63 | 2.18 | 5.33 | 1.98 | 5.18 | 2.60 |
| W | 2.96 | 0.71 | 3.03 | 0.72 | 2.64 | 0.73 |
| H | 2.23 | 0.59 | 2.26 | 0.44 | 2.01 | 0.66 |
| Mean | 158.66 | 120.83 | 142.65 | 107.52 | 160.16 | 98.83 |
| DIM | 1.69 | 0.09 | 1.68 | 0.13 | 1.69 | 0.10 |
| Dim. of Binary Images | Dim. of Left Images | Dim. of Right Images | |||||
|---|---|---|---|---|---|---|---|
| n | Mean | Std. Dev. | Mean | Std. Dev. | Mean | Std. Dev. | |
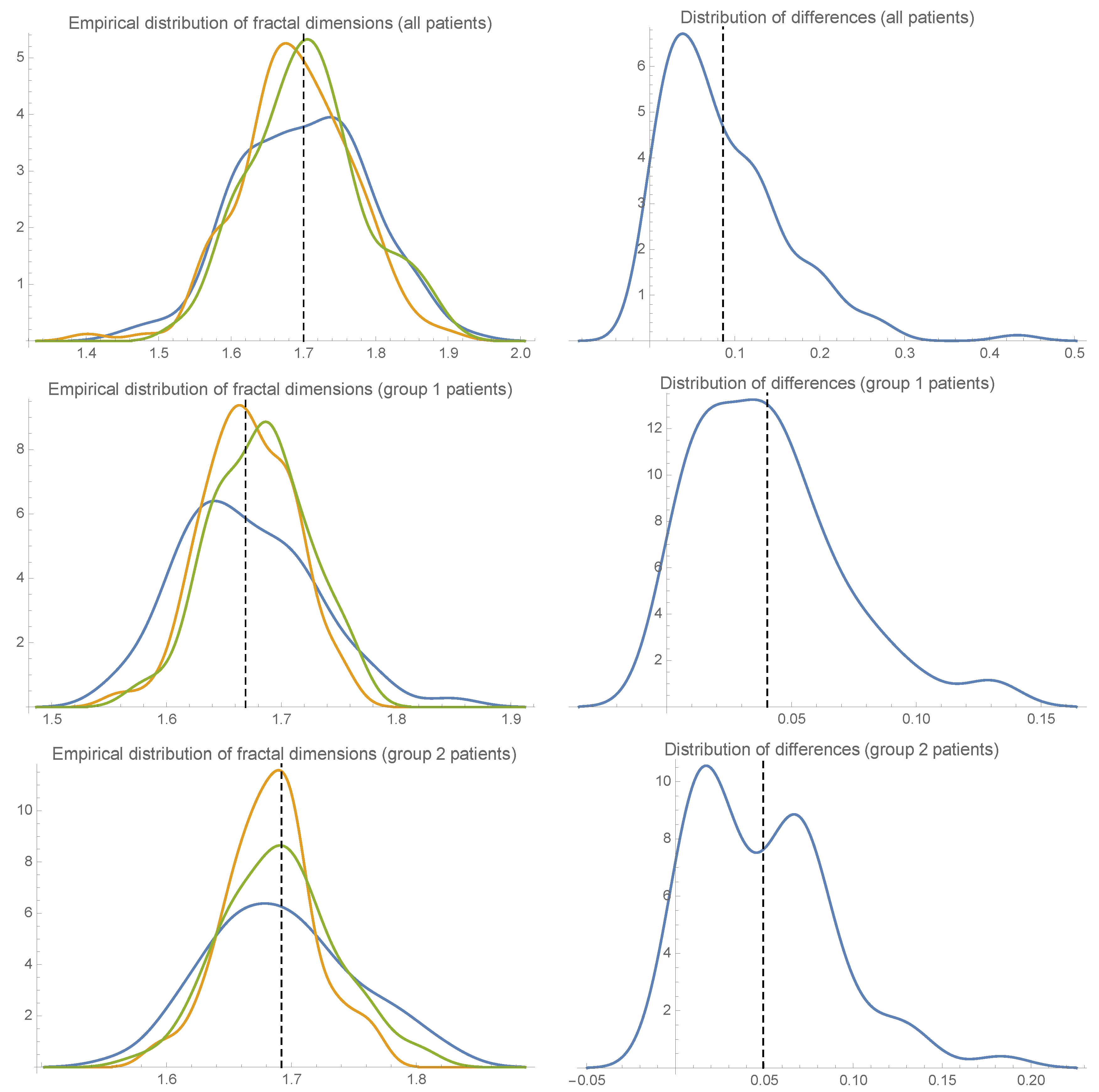
| Whole sample | 130 | 1.70 | 0.09 | 1.68 | 0.08 | 1.72 | 0.08 |
| Group One | 65 | 1.67 | 0.06 | 1.66 | 0.04 | 1.68 | 0.04 |
| Group Two | 65 | 1.70 | 0.06 | 1.68 | 0.04 | 1.70 | 0.04 |
| Differences (in abs.) among Left and Right DIMs | |||
|---|---|---|---|
| Mean | Std. Dev. | Mann–Whitney (p-Value) | |
| Whole sample | 0.09 | 0.07 | 0.21 |
| Group One | 0.04 | 0.03 | 0.19 |
| Group Two | 0.05 | 0.04 | 0.38 |
| Group One vs. Two Comparison | |
|---|---|
| CBCT images | M-W p-Value |
| Whole images | 0.28 |
| Left images | 0.29 |
| Right images | 0.19 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bornstein, M.M.; Fernández-Martínez, M.; Guirao, J.L.G.; Gómez-García, F.J.; Guerrero-Sánchez, Y.; López-Jornet, P. On the Symmetry of the Bone Structure Density over the Nasopalatine Foramen via Accurate Fractal Dimension Analysis. Symmetry 2019, 11, 202. https://doi.org/10.3390/sym11020202
Bornstein MM, Fernández-Martínez M, Guirao JLG, Gómez-García FJ, Guerrero-Sánchez Y, López-Jornet P. On the Symmetry of the Bone Structure Density over the Nasopalatine Foramen via Accurate Fractal Dimension Analysis. Symmetry. 2019; 11(2):202. https://doi.org/10.3390/sym11020202
Chicago/Turabian StyleBornstein, Michael M., Manuel Fernández-Martínez, Juan L. G. Guirao, Francisco J. Gómez-García, Yolanda Guerrero-Sánchez, and Pía López-Jornet. 2019. "On the Symmetry of the Bone Structure Density over the Nasopalatine Foramen via Accurate Fractal Dimension Analysis" Symmetry 11, no. 2: 202. https://doi.org/10.3390/sym11020202
APA StyleBornstein, M. M., Fernández-Martínez, M., Guirao, J. L. G., Gómez-García, F. J., Guerrero-Sánchez, Y., & López-Jornet, P. (2019). On the Symmetry of the Bone Structure Density over the Nasopalatine Foramen via Accurate Fractal Dimension Analysis. Symmetry, 11(2), 202. https://doi.org/10.3390/sym11020202










