A Physiologically Based Pharmacokinetic Model Relates the Subcutaneous Bioavailability of Monoclonal Antibodies to the Saturation of FcRn-Mediated Recycling in Injection-Site-Draining Lymph Nodes
Highlights
- Subcutaneous dosing of monoclonal antibodies (mAbs) leads to high local drug concentration at the injection site, in the dosing site draining lymph nodes, and in antigen-presenting cells (APC), resident in peripheral lymph nodes.
- The elevated local IgG concentration is predicted to result in a transient saturation of the FcRn recycling pathway in APCs and consequently to an increased degradation of macropinocytosed IgG, which is predominantly composed of the dosed mAb.
- Elevated degradation of macropinocytosed IgG during the first pass of the subcutaneously dosed mAb was predicted to manifest in an about 70% bioavailability of the drug.
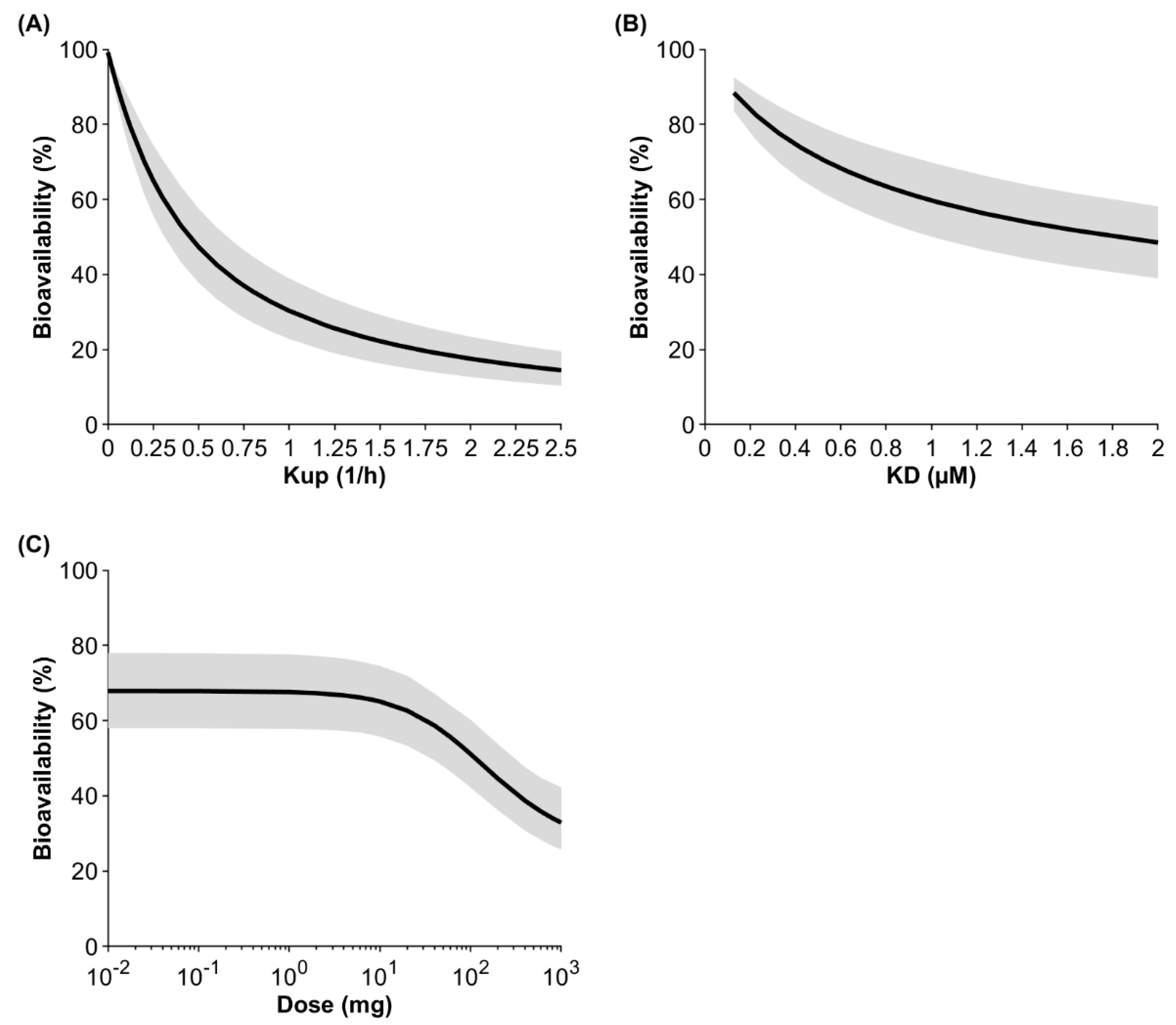
- The bioavailability of mAbs can mechanistically be predicted from in vitro FcRn binding data and local concentration in the draining lymph node of the subcutaneous dosing site.
- The model can be used to understand the role of non-specific uptake of IgG in the dosing site draining lymph nodes and its impact on the bioavailability of monoclonal antibodies.
Abstract
1. Introduction
2. Material and Methods
2.1. Model Structure
2.2. Model Parameterization
| Parameter | Arm | Abdomen | Back | Thigh | References |
|---|---|---|---|---|---|
| SC site volume (L/kg) | 0.00077 | 0.00099 | 0.00115 | 0.00099 | Calculated based on [22] |
| Vascular volume (% of the SC site) | 5 | 5 | 5 | 5 | [15] |
| Endosomal volume (% of the SC site) | 0.06 | 0.06 | 0.06 | 0.06 | [20,21] |
| Interstitial volume (% of the SC site) | 59.5 | 59.5 | 59.5 | 59.5 | [15] |
| Blood flow (% of the cardiac output) | 0.088 | 0.035 | 0.105 | 0.073 | [23] |
| Afferent lymph flow (% of the total lymph flow) | 0.191 | 0.205 | 0.262 | 0.272 | [22] |
| Efferent lymph flow (% of the afferent lymph flow) | 93.0 | 93.0 | 93.0 | 93.0 | [26] |
| Lymph capillary volume (L/kg) | 0.000352 | 0.000392 | 0.000415 | 0.000387 | [22] |
| Peripheral lymph node volume (L/kg) | 0.000116 | 8.38 × 10−5 | 9.52 × 10−5 | 8.38 × 10−5 | [25] |
2.3. Bioavailability Calculation
2.4. Model Validation
2.5. Sensitivity Analyses
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Viola, M.; Sequeira, J.; Seiça, R.; Veiga, F.; Serra, J.; Santos, A.C.; Ribeiro, A.J. Subcutaneous delivery of monoclonal antibodies: How do we get there? J. Control. Release 2018, 286, 301–314. [Google Scholar] [CrossRef]
- Zheng, Y.; Tesar, D.B.; Benincosa, L.; Birnböck, H.; Boswell, C.A.; Bumbaca, D.; Cowan, K.J.; Danilenko, D.M.; Daugherty, A.L.; Fielder, P.J. Minipig as a potential translatable model for monoclonal antibody pharmacokinetics after intravenous and subcutaneous administration. mAbs 2012, 4, 243–255. [Google Scholar] [CrossRef]
- Kraft, T.E.; Richter, W.F.; Emrich, T.; Knaupp, A.; Schuster, M.; Wolfert, A.; Kettenberger, H. Heparin chromatography as an in vitro predictor for antibody clearance rate through pinocytosis. mAbs 2020, 12, 1683432. [Google Scholar] [CrossRef]
- Grinshpun, B.; Thorsteinson, N.; Pereira, J.N.; Rippmann, F.; Nannemann, D.; Sood, V.D.; Fomekong Nanfack, Y. Identifying biophysical assays and in silico properties that enrich for slow clearance in clinical-stage therapeutic antibodies. mAbs 2021, 13, 1932230. [Google Scholar] [CrossRef] [PubMed]
- Sánchez-Félix, M.; Burke, M.; Chen, H.H.; Patterson, C.; Mittal, S. Predicting bioavailability of monoclonal antibodies after subcutaneous administration: Open innovation challenge. Adv. Drug Deliv. Rev. 2020, 167, 66–77. [Google Scholar] [CrossRef]
- Richter, W.F.; Christianson, G.J.; Frances, N.; Grimm, H.P.; Proetzel, G.; Roopenian, D.C. Hematopoietic cells as site of first-pass catabolism after subcutaneous dosing and contributors to systemic clearance of a monoclonal antibody in mice. mAbs 2018, 10, 803–813. [Google Scholar] [CrossRef]
- Challa, D.K.; Wang, X.; Montoyo, H.P.; Velmurugan, R.; Ober, R.J.; Ward, E.S. Neonatal Fc receptor expression in macrophages is indispensable for IgG homeostasis. mAbs 2019, 11, 848–860. [Google Scholar] [CrossRef] [PubMed]
- Fathallah, A.M.; Bankert, R.B.; Balu-Iyer, S.V. Immunogenicity of subcutaneously administered therapeutic proteins—A mechanistic perspective. AAPS J. 2013, 15, 897–900. [Google Scholar] [CrossRef] [PubMed]
- Steinman, R.M.; Brodie, S.E.; Cohn, Z.A. Membrane flow during pinocytosis. A stereologic analysis. J. Cell Biol. 1976, 68, 665–687. [Google Scholar] [CrossRef]
- Sallusto, F.; Cella, M.; Danieli, C.; Lanzavecchia, A. Dendritic cells use macropinocytosis and the mannose receptor to concentrate macromolecules in the major histocompatibility complex class II compartment: Downregulation by cytokines and bacterial products. J. Exp. Med. 1995, 182, 389–400. [Google Scholar] [CrossRef]
- Blank, F.; Rothen-Rutishauser, B.; Gehr, P. Dendritic cells and macrophages form a transepithelial network against foreign particulate antigens. Am. J. Respir. Cell Mol. Biol. 2007, 36, 669–677. [Google Scholar] [CrossRef]
- Varkhede, N.; Forrest, M.L. Understanding the monoclonal antibody disposition after subcutaneous administration using a minimal physiologically based pharmacokinetic model. J. Pharm. Pharm. Sci. 2018, 21, 130s. [Google Scholar] [CrossRef] [PubMed]
- Hu, S.; D’Argenio, D.Z. Predicting monoclonal antibody pharmacokinetics following subcutaneous administration via whole-body physiologically-based modeling. J. Pharmacokinet. Pharmacodyn. 2020, 47, 385–409. [Google Scholar] [CrossRef] [PubMed]
- Zheng, F.; Hou, P.; Corpstein, C.D.; Park, K.; Li, T. Multiscale pharmacokinetic modeling of systemic exposure of subcutaneously injected biotherapeutics. J. Control. Release 2021, 337, 407–416. [Google Scholar] [CrossRef] [PubMed]
- Gill, K.L.; Gardner, I.; Li, L.; Jamei, M. A bottom-up whole-body physiologically based pharmacokinetic model to mechanistically predict tissue distribution and the rate of subcutaneous absorption of therapeutic proteins. AAPS J. 2016, 18, 156–170. [Google Scholar] [CrossRef] [PubMed]
- Supersaxo, A.; Hein, W.R.; Steffen, H. Effect of molecular weight on the lymphatic absorption of water-soluble compounds following subcutaneous administration. Pharm. Res. 1990, 7, 167–169. [Google Scholar] [CrossRef]
- Zou, P.; Wang, F.; Wang, J.; Lu, Y.; Tran, D.; Seo, S.K. Impact of injection sites on clinical pharmacokinetics of subcutaneously administered peptides and proteins. J. Control. Release 2021, 336, 310–321. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Gardner, I.; Dostalek, M.; Jamei, M. Simulation of monoclonal antibody pharmacokinetics in humans using a minimal physiologically based model. AAPS J. 2014, 16, 1097–1109. [Google Scholar] [CrossRef] [PubMed]
- Rippe, B.; Haraldsson, B. Transport of macromolecules across microvascular walls: The two-pore theory. Physiol. Rev. 1994, 74, 163–219. [Google Scholar] [CrossRef] [PubMed]
- Crapo, J.D.; Barry, B.E.; Gehr, P.; Bachofen, M.; Weibel, E.R. Cell number and cell characteristics of the normal human lung. Am. Rev. Respir. Dis. 1982, 126, 332–337. [Google Scholar]
- Herring, N.; Paterson, D.J. Levick’s Introduction to Cardiovascular Physiology; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Nathanson, S.D.; Nelson, L.; Karvelis, K.C. Rates of flow of technetium 99m-labeled human serum albumin from peripheral injection sites to sentinel lymph nodes. Ann. Surg. Oncol. 1996, 3, 329–335. [Google Scholar] [CrossRef] [PubMed]
- Patel, N.; Clarke, J.F.; Salem, F.; Abdulla, T.; Martins, F.; Arora, S.; Tsakalozou, E.; Hodgkinson, A.; Arjmandi-Tash, O.; Cristea, S. Multi-phase multi-layer mechanistic dermal absorption (MPML MechDermA) model to predict local and systemic exposure of drug products applied on skin. CPT Pharmacomet. Syst. Pharmacol. 2022, 11, 1060–1084. [Google Scholar] [CrossRef]
- Valentin, J. Guide for the Practical Application of the ICRP Human Respiratory Tract Model: ICRP Supporting Guidance 3Approved by ICRP Committee 2 in October 2000. Ann. ICRP 2002, 32, 13. [Google Scholar] [CrossRef]
- University of Arkansas for Medical Science. Lymphatic Tables. 2023. Available online: https://medicine.uams.edu/neurobiology/education/medical-school-courses/human-structure-module/anatomy-tables/lymphatic-tables/. (accessed on 22 June 2023).
- Jafarnejad, M.; Woodruff, M.C.; Zawieja, D.C.; Carroll, M.C.; Moore, J., Jr. Modeling lymph flow and fluid exchange with blood vessels in lymph nodes. Lymphat. Res. Biol. 2015, 13, 234–247. [Google Scholar] [CrossRef] [PubMed]
- Savage, D.F.; Egea, P.F.; Robles-Colmenares, Y.; O’Connell, J.D., III; Stroud, R.M. Architecture and selectivity in aquaporins: 2.5 Å X-ray structure of aquaporin Z. PLoS Biol. 2003, 1, e72. [Google Scholar] [CrossRef] [PubMed]
- Mellor, R.; Stanton, A.; Azarbod, P.; Sherman, M.; Levick, J.; Mortimer, P. Enhanced cutaneous lymphatic network in the forearms of women with postmastectomy oedema. J. Vasc. Res. 2000, 37, 501–512. [Google Scholar] [CrossRef]
- Ying, M.; Pang, B. Three-dimensional ultrasound measurement of cervical lymph node volume. Br. J. Radiol. 2009, 82, 617–625. [Google Scholar] [CrossRef]
- Zuther, J.; Norton, S. Lymphedema Management; Thieme: Stuttgart, Germany, 2013; Volume 3. [Google Scholar]
- Camara, A.; Lavanant, A.C.; Abe, J.; Desforges, H.L.; Alexandre, Y.O.; Girardi, E.; Igamberdieva, Z.; Asano, K.; Tanaka, M.; Hehlgans, T. CD169+ macrophages in lymph node and spleen critically depend on dual RANK and LTbetaR signaling. Proc. Natl. Acad. Sci. USA 2022, 119, e2108540119. [Google Scholar] [CrossRef] [PubMed]
- Komohara, Y.; Ohnishi, K.; Takeya, M. Possible functions of CD 169-positive sinus macrophages in lymph nodes in anti-tumor immune responses. Cancer Sci. 2017, 108, 290–295. [Google Scholar] [CrossRef]
- Mellman, I.S.; Steinman, R.M.; Unkeless, J.C.; Cohn, Z.A. Selective iodination and polypeptide composition of pinocytic vesicles. J. Cell Biol. 1980, 86, 712–722. [Google Scholar] [CrossRef] [PubMed]
- Walk, E.L.; McLaughlin, S.; Coad, J.; Weed, S.A. Use of high frequency ultrasound to monitor cervical lymph node alterations in mice. PLoS ONE 2014, 9, e100185. [Google Scholar] [CrossRef] [PubMed]
- Smith, G.; Griffiths, B.; Mollison, D.; Mollison, P. Uptake of IgG after intramuscular and subcutaneous injection. Lancet 1972, 299, 1208–1212. [Google Scholar] [CrossRef]
- Li, Z.; Yu, X.; Li, Y.; Verma, A.; Chang, H.P.; Shah, D.K. A two-pore physiologically based pharmacokinetic model to predict subcutaneously administered different-size antibody/antibody fragments. AAPS J. 2021, 23, 62. [Google Scholar] [CrossRef] [PubMed]
- Hu, S.; Datta-Mannan, A.; D’Argenio, D.Z. Physiologically based modeling to predict monoclonal antibody pharmacokinetics in humans from in vitro physiochemical properties. mAbs 2022, 14, 2056944. [Google Scholar] [CrossRef] [PubMed]
- Fan, Y.-Y.; Farrokhi, V.; Caiazzo, T.; Wang, M.; O’Hara, D.M.; Neubert, H. Human FcRn tissue expression profile and half-life in PBMCs. Biomolecules 2019, 9, 373. [Google Scholar] [CrossRef] [PubMed]
- Zou, P. Predicting human bioavailability of subcutaneously administered fusion proteins and monoclonal antibodies using human intravenous clearance or antibody isoelectric point. AAPS J. 2023, 25, 31. [Google Scholar] [CrossRef] [PubMed]
- Schoch, A.; Kettenberger, H.; Mundigl, O.; Winter, G.; Engert, J.; Heinrich, J.; Emrich, T. Charge-mediated influence of the antibody variable domain on FcRn-dependent pharmacokinetics. Proc. Natl. Acad. Sci. USA 2015, 112, 5997–6002. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Shah, D.K. Physiologically based pharmacokinetic modeling to characterize the effect of molecular charge on whole-body disposition of monoclonal antibodies. AAPS J. 2023, 25, 48–64. [Google Scholar] [CrossRef]
- Mellman, I.S.; Plutner, H.; Steinman, R.M.; Unkeless, J.C.; Cohn, Z.A. Internalization and degradation of macrophage Fc receptors during receptor-mediated phagocytosis. J. Cell Biol. 1983, 96, 887–895. [Google Scholar] [CrossRef]



| Cmax Ratio | tmax Ratio | AUC Ratio | ||||
|---|---|---|---|---|---|---|
| Observed | Predicted | Observed | Predicted | Observed | Predicted | |
| Arm/Abdomen | 0.92 | 0.96 | 1.26 | 0.98 | 0.98 | 0.95 |
| Thigh/Abdomen | 1.14 | 1.13 | 0.97 | 0.77 | 1.13 | 1.06 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stader, F.; Liu, C.; Derbalah, A.; Momiji, H.; Pan, X.; Gardner, I.; Jamei, M.; Sepp, A. A Physiologically Based Pharmacokinetic Model Relates the Subcutaneous Bioavailability of Monoclonal Antibodies to the Saturation of FcRn-Mediated Recycling in Injection-Site-Draining Lymph Nodes. Antibodies 2024, 13, 70. https://doi.org/10.3390/antib13030070
Stader F, Liu C, Derbalah A, Momiji H, Pan X, Gardner I, Jamei M, Sepp A. A Physiologically Based Pharmacokinetic Model Relates the Subcutaneous Bioavailability of Monoclonal Antibodies to the Saturation of FcRn-Mediated Recycling in Injection-Site-Draining Lymph Nodes. Antibodies. 2024; 13(3):70. https://doi.org/10.3390/antib13030070
Chicago/Turabian StyleStader, Felix, Cong Liu, Abdallah Derbalah, Hiroshi Momiji, Xian Pan, Iain Gardner, Masoud Jamei, and Armin Sepp. 2024. "A Physiologically Based Pharmacokinetic Model Relates the Subcutaneous Bioavailability of Monoclonal Antibodies to the Saturation of FcRn-Mediated Recycling in Injection-Site-Draining Lymph Nodes" Antibodies 13, no. 3: 70. https://doi.org/10.3390/antib13030070
APA StyleStader, F., Liu, C., Derbalah, A., Momiji, H., Pan, X., Gardner, I., Jamei, M., & Sepp, A. (2024). A Physiologically Based Pharmacokinetic Model Relates the Subcutaneous Bioavailability of Monoclonal Antibodies to the Saturation of FcRn-Mediated Recycling in Injection-Site-Draining Lymph Nodes. Antibodies, 13(3), 70. https://doi.org/10.3390/antib13030070






