Agreement of Four Equations for Computing Dewfall in Northern Germany
Abstract
:1. Introduction
2. Materials and Methods
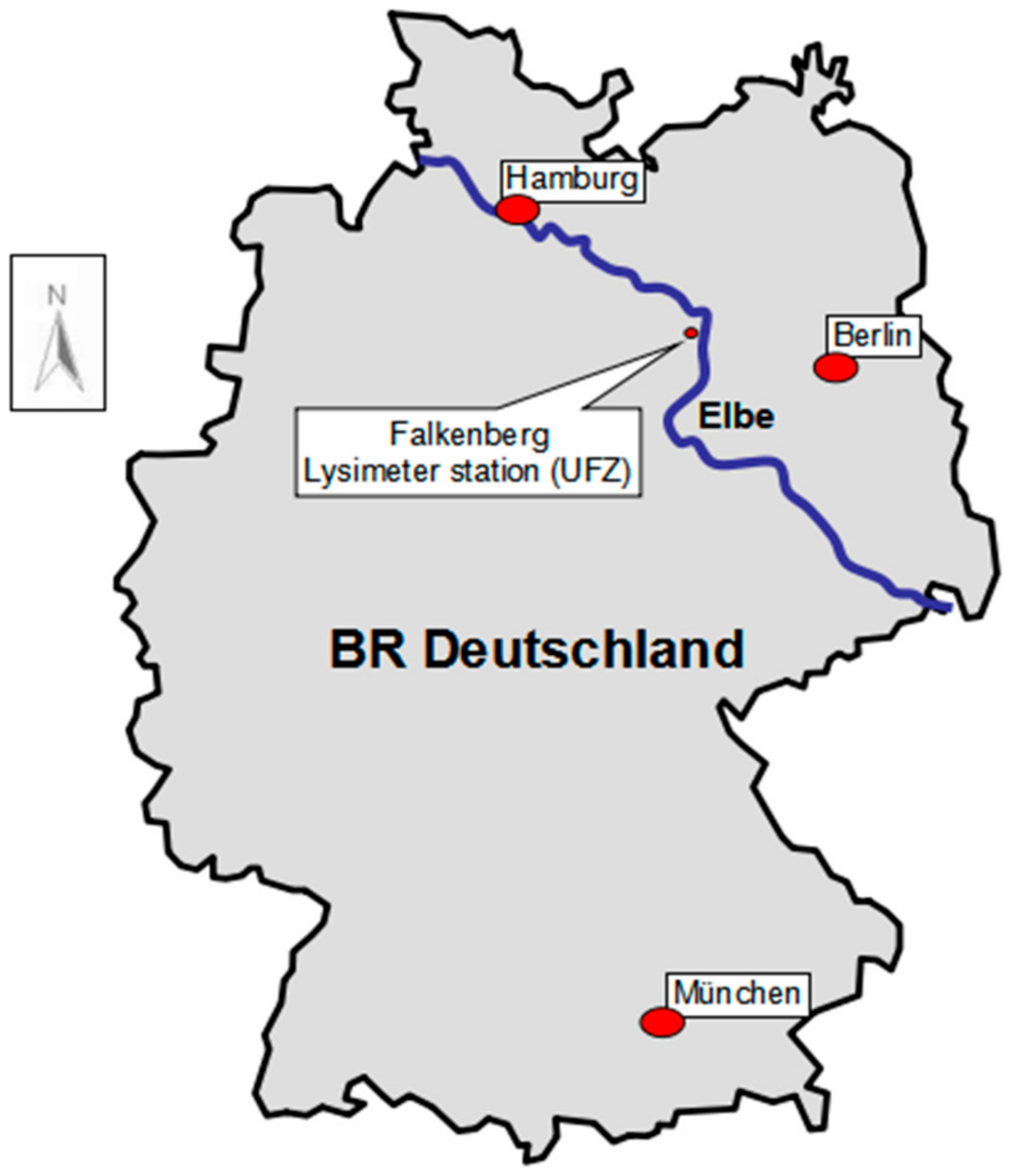
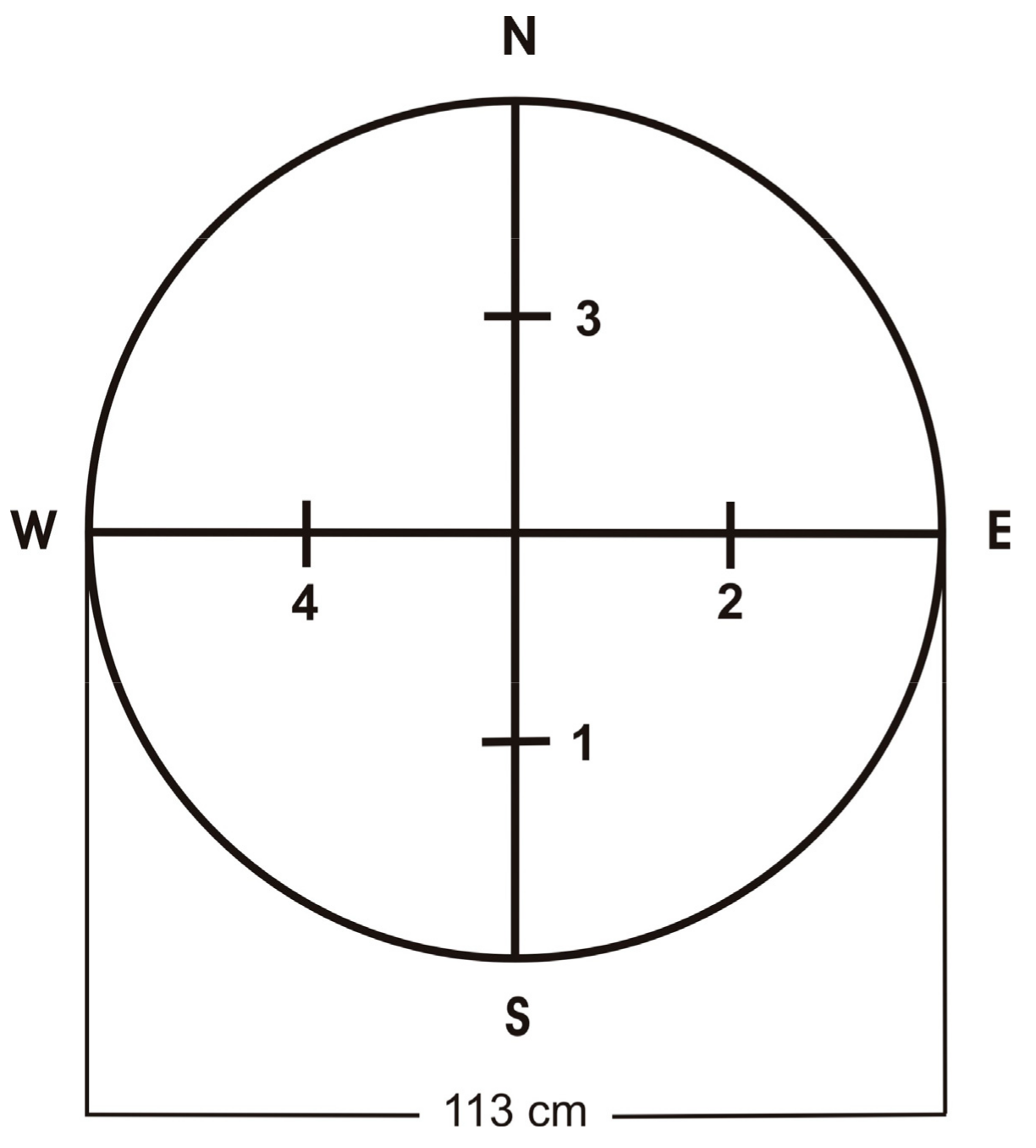
2.1. Data Collection
2.2. Equations
3. Results
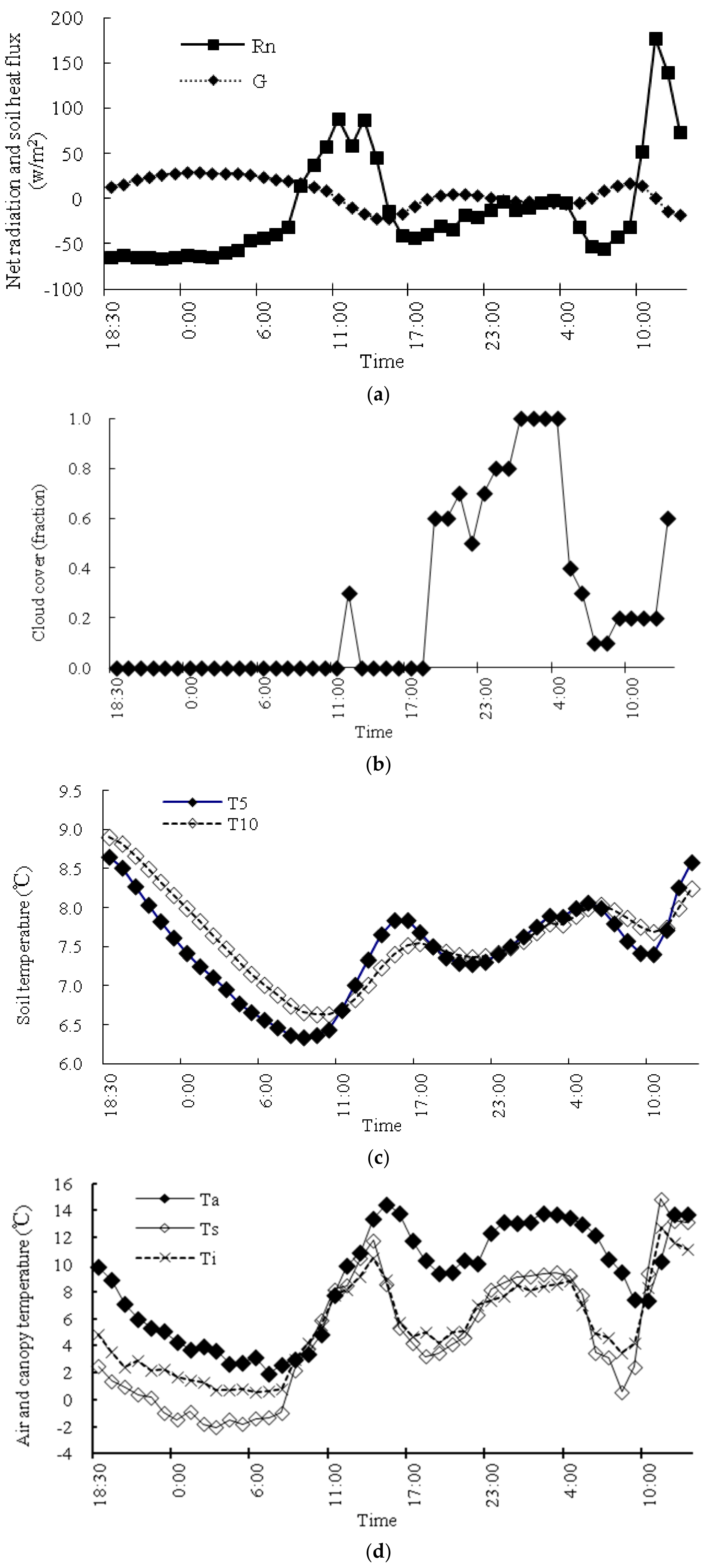
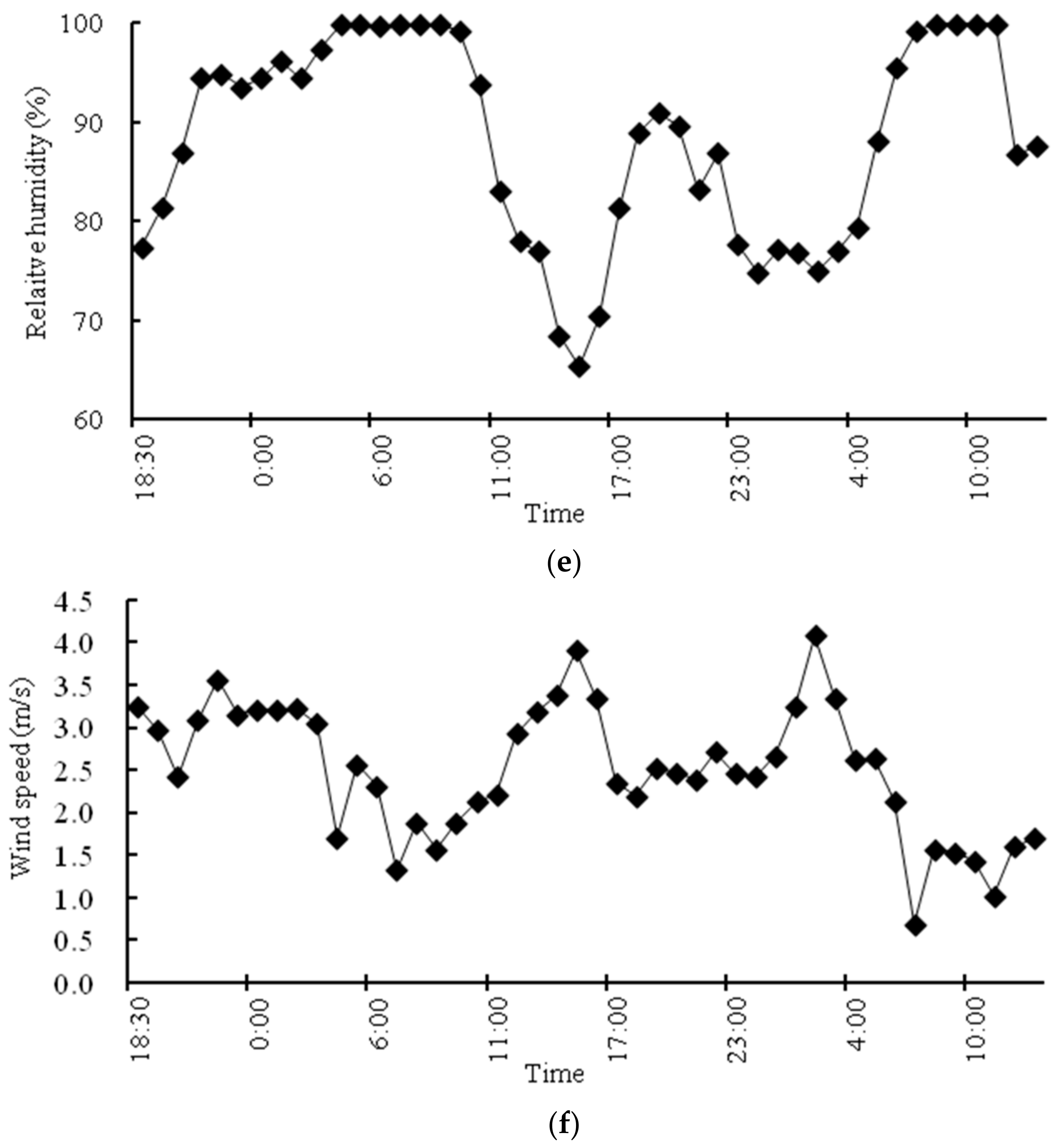
3.1. Meteorological Data
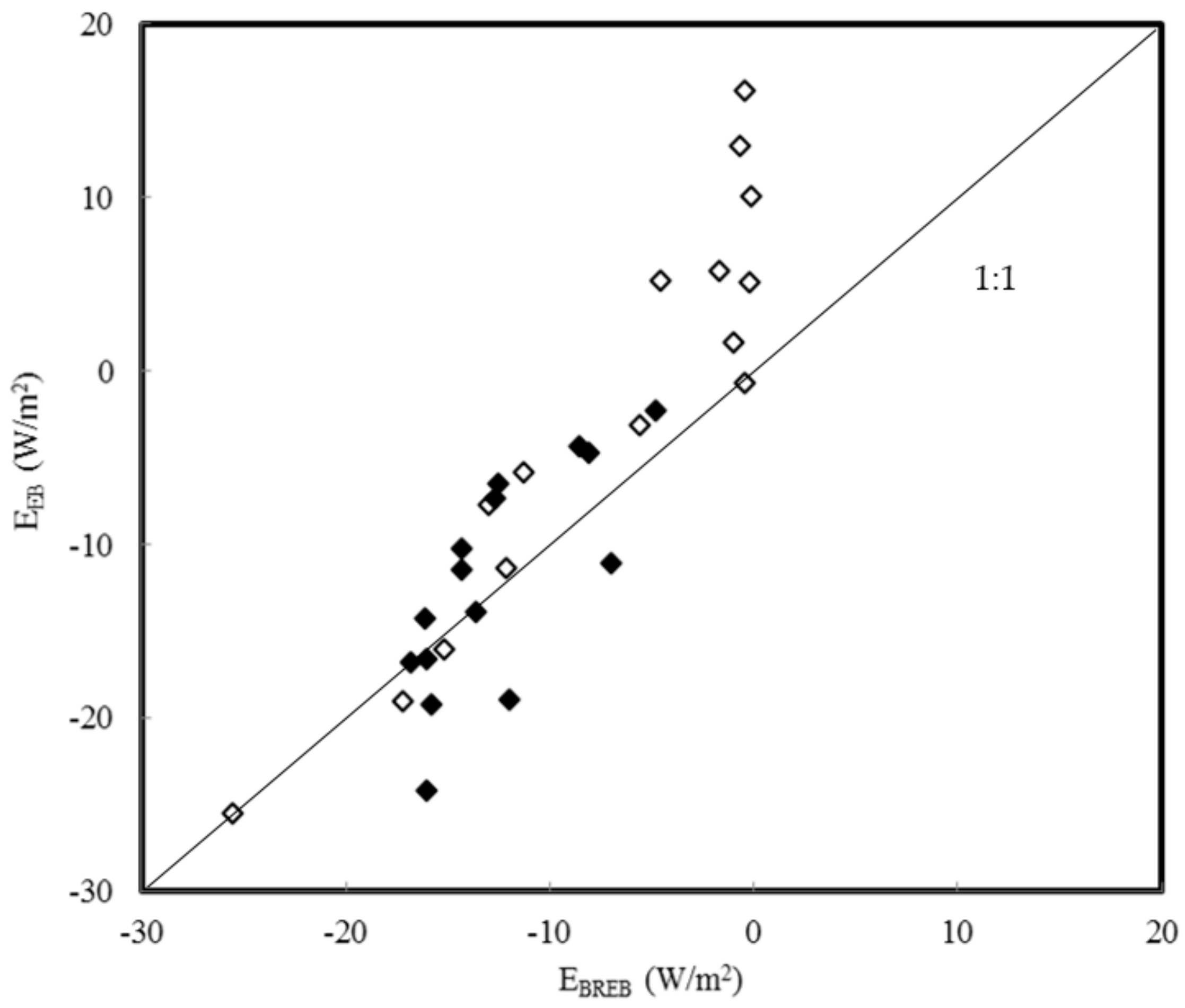
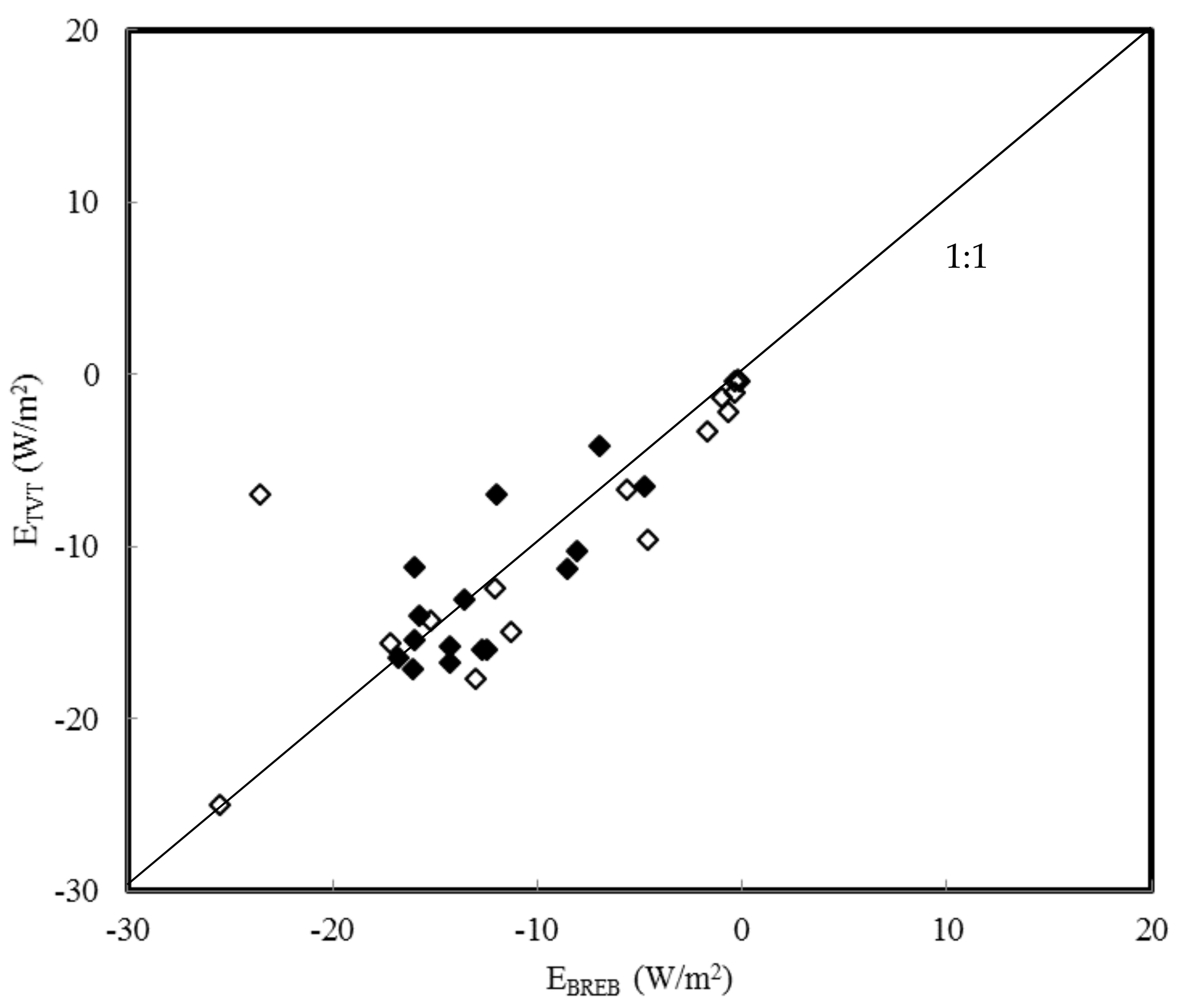
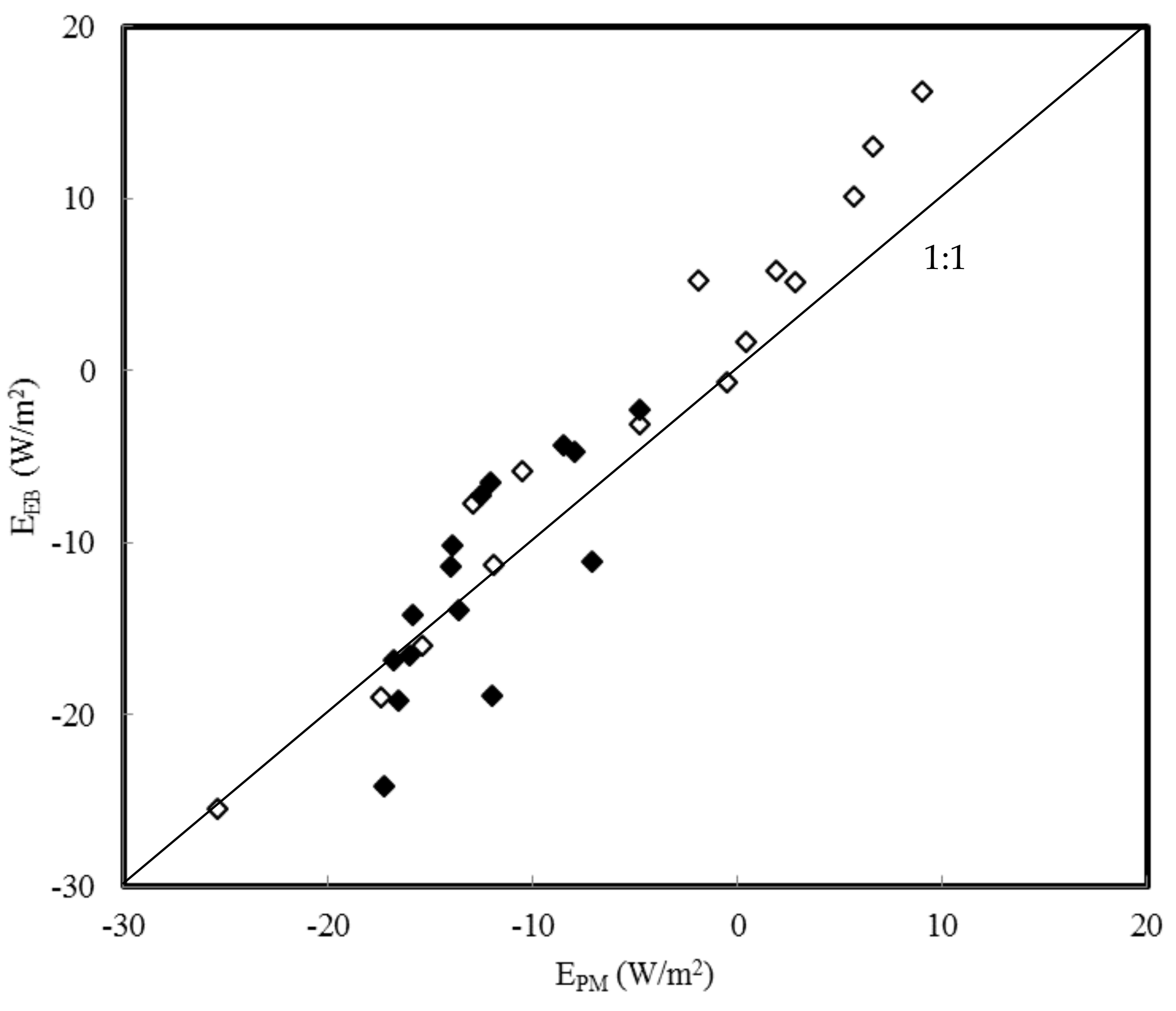
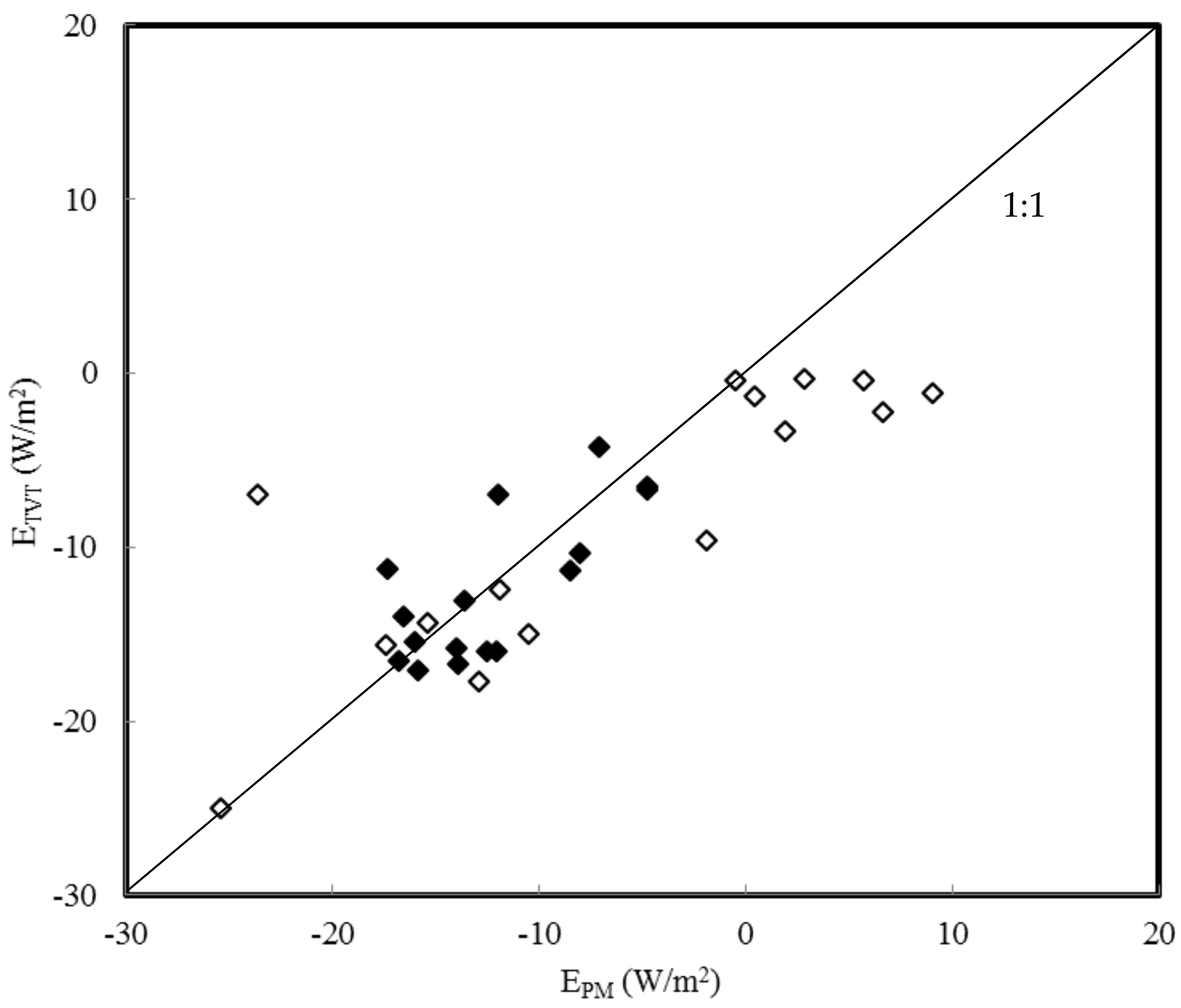
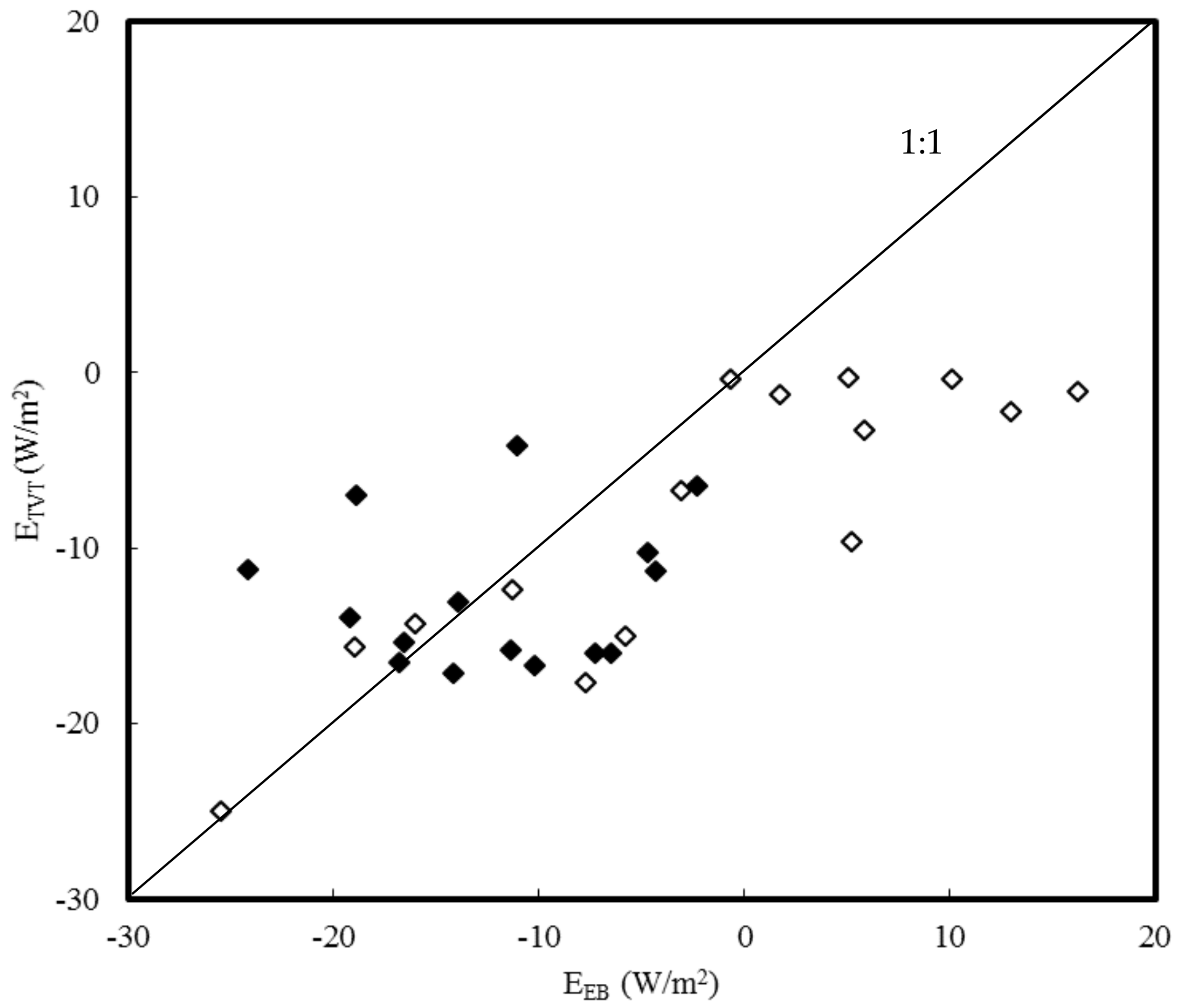
3.2. Performance of the Equations
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
Appendix A.1. Energy Balance Equation (EB)
Appendix A.2. Equation for Turbulent Vapour Transport (TVT)
Appendix A.3. Penman-Monteith Equation (PM)
Appendix A.4. Bowen Ratio-Energy Balance Equation (BREB)
References
- Garratt, J.R.; Segal, M. On the contribution of atmospheric moisture to dew formation. Bound.-Layer Meteorol. 1988, 45, 209–236. [Google Scholar] [CrossRef]
- Wallin, J.R. Agrometeorological aspects of dew. Agric. Meteorol. 1967, 4, 85–102. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Berkowicz, S.M. Dew deposition and drying in a desert system: A simple simulation model. J. Arid Environ. 1999, 42, 211–222. [Google Scholar] [CrossRef]
- Li, X.Y. Effect of gravel and sand mulches on dew deposition in the semiarid region of China. J. Hydrol. 2002, 260, 151–160. [Google Scholar] [CrossRef]
- Danin, A.; Bar-Or, Y.; Dor, I.; Yisraeli, T. The role of cyanobacteria in stabilization of sand dunes in southern Israel. Ecol. Mediterr. 1989, 15, 55–64. [Google Scholar]
- Lange, O.L.; Kidron, G.; Büdel, B.; Meyer, A.; Kilian, E.; Abeliovich, A. Taxonomic composition and photosynthetic characteristics of the “biological soil crusts” covering sand dunes in the western Negev Desert. Funct. Ecol. 1992, 6, 519–527. [Google Scholar] [CrossRef]
- Baier, W. Studies on dew formation under semi-arid conditions. Agric. Metorol. 1966, 3, 103–112. [Google Scholar] [CrossRef]
- Tuller, S.E.; Chilton, R. The role of dew in the seasonal moisture balance of a summer-dry climate. Agric. Meteorol. 1973, 11, 135–142. [Google Scholar] [CrossRef]
- Stewart, J.B. Evaporation from the wet canopy of a pine forest. Water Resour. Res. 1977, 13, 915–921. [Google Scholar] [CrossRef]
- Barradas, V.L.; Glez-Medellin, M.G. Dew and its effect on two heliophile understory species of a tropical dry deciduous forest in Mexico. Int. J. Biometeorol. 1999, 43, 1–7. [Google Scholar] [CrossRef]
- Stone, E.C. Dew as an ecological factor. II. The effect of artificial dew on the survival of Pinus ponderosa and associated species. Ecology 1957, 38, 414–422. [Google Scholar] [CrossRef]
- Fritschen, L.J.; Doraiswamy, P. Dew: An addition to the hydrologic balance of Douglas fir. Water Resour. Res. 1973, 9, 891–894. [Google Scholar] [CrossRef]
- Meissner, R.; Seeger, J.; Rupp, H.; Seyfarth, M.; Borg, H. Measurement of dew, fog, and rime with a high-precision gravitation lysimeter. J. Plant Nutr. Soil Sci. 2007, 170, 335–344. [Google Scholar] [CrossRef]
- Neumann, J. Estimating the amount of dewfall. Meteorol. Atmos. Phys. 1956, 9, 197–203. [Google Scholar] [CrossRef]
- Long, I.F. Some observations on dew. Meteorol. Mag. 1958, 87, 161–168. [Google Scholar]
- Monteith, J.L. Dew: Facts and fallacies. In The Water Relations of Plants; Rutter, A.J., Whitehead, F.H., Eds.; Wiley: New York, NY, USA, 1963; pp. 37–56. [Google Scholar]
- Pedro, M.J.; Gillespie, T.J. Estimating dew duration. I. Utilizing micrometeorological data. Agric. Meteorol. 1982, 25, 283–296. [Google Scholar] [CrossRef]
- Pedro, M.J.; Gillespie, T.J. Estimating dew duration. II. Utilizing standard weather station data. Agric. Meteorol. 1982, 25, 297–310. [Google Scholar] [CrossRef]
- Severini, M.; Moriconi, M.L.; Tonna, G.; Olivieri, B. Dewfall and evapotranspiration determination during day and nighttime on an irrigated lawn. J. Appl. Meteorol. 1984, 23, 1241–1246. [Google Scholar] [CrossRef]
- Janssen, L.H.J.M.; Römer, F.G. The frequency and duration of dew occurrence over a year. Tellus B 1991, 43, 408–419. [Google Scholar] [CrossRef]
- Madeira, A.C.; Gillespie, T.J.; Duke, C.L. Effect of wetness on turfgrass canopy reflectance. Agric. For. Meteorol. 2001, 107, 117–130. [Google Scholar] [CrossRef]
- Sentelhas, P.C.; Gillespie, T.J. Estimating hourly net radiation for leaf wetness duration using the Penman-Monteith equation. Theor. Appl. Climatol. 2008, 91, 205–215. [Google Scholar] [CrossRef]
- Sudmeyer, R.A.; Nulsen, R.A.; Scott, W.D. Measured dewfall and potential condensation on grazed pasture in the Collie River basin, southwestern Australia. J. Hydrol. 1994, 154, 255–269. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; van Boxel, J.H.; Nieveen, J. Nighttime exchange processes near the soil surface of a maize canopy. Agric. For. Meteorol. 1996, 82, 155–169. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Berkowicz, S.M. Dew measurements along a longitudinal sand dune transect, Negev Desert, Israel. Int. J. Biometeorol. 2000, 43, 184–190. [Google Scholar] [CrossRef] [PubMed]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Kruit, R.J.W.; Berkowicz, S.M. Contribution of dew to the water budget of a grassland area in the Netherlands. Water Resour. Res. 2006, 42, 446–455. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Berkowicz, S.M. Passive dew collection in a grassland area, The Netherlands. Atmos. Res. 2008, 87, 377–385. [Google Scholar] [CrossRef]
- Luo, W.; Goudriaan, J. Dew formation on rice under varying durations of nocturnal radiative loss. Agric. For. Meteorol. 2000, 104, 303–313. [Google Scholar] [CrossRef]
- Bowen, I.S. The ratio of heat losses by conduction and by evaporation from any water surface. Phys. Rev. 1926, 27, 779–787. [Google Scholar] [CrossRef]
- Atzema, A.J.; Jacobs, A.F.G.; Wartena, L. Moisture distribution within a maize crop due to dew. Neth. J. Agric. Sci. 1990, 38, 117–129. [Google Scholar]
- Jacobs, A.F.G.; van Pul, W.A.J.; van Dijken, A. Similarity moisture dew profiles within a corn canopy. J. Appl. Meteorol. 1990, 29, 1300–1306. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; van Pul, A.; El-Kilani, R.M.M. Dew formation and the drying process within a maize canopy. Bound.-Layer Meteorol. 1994, 69, 367–378. [Google Scholar] [CrossRef]
- Wolf, A.; Nick, S.; Kanat, A.; Johnson, D.A.; Laca, M. Effects of different eddy covariance correction schemes on energy balance closure and comparisons with the modified Bowen ratio system. Agric. For. Meteorol. 2008, 148, 942–952. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Berkowicz, S.M. A simple model for potential dewfall in an arid region. Atmos. Res. 2002, 64, 85–295. [Google Scholar] [CrossRef]
- Campbell, G.S.; Norman, J.M. An Introduction to Environmental Biophysics, 2nd ed; Springer: New York, NY, USA, 1998. [Google Scholar]
- Xiao, H. Factors Affecting Dewfall, Its Measurement with Lysimeters, and Its Estimation with Micrometeorological equations. Ph.D. Thesis, Faculty of Natural Sciences III, Martin-Luther-University Halle-Wittenberg, Halle, Germany, 2010. [Google Scholar]
- Tennekes, H. Outline of a second-order theory of turbulent pipe flow. AIAA J. 1968, 6, 1735–1740. [Google Scholar] [CrossRef]
- Businger, J.A.; Wyngaard, J.C.; Izuml, Y.; Bradley, E.F. Flux-profile relationships in the atmospheric surface layer. J. Atmos. Sci. 1971, 28, 181–189. [Google Scholar] [CrossRef]
- Telford, J.W. A theoretical value for von Karman’s constant. Pure Appl. Geophys. 1982, 120, 648–661. [Google Scholar] [CrossRef]
- Bergmann, J.C. A physical interpretation of von Karman’s constant based on asymptotic considerations—A new value. J. Atmos. Sci. 1998, 55, 3403–3407. [Google Scholar] [CrossRef]
- Monteith, J.L.; Unsworth, M.H. Principles of Environmental Physics, 2nd ed.; Arnold: London, UK, 1990. [Google Scholar]
- Shaw, R.H.; Pereira, A.R. Aerodynamic roughness of a plant canopy: A numerical experiment. Agric. Meteorol. 1982, 26, 51–65. [Google Scholar] [CrossRef]
- Biftu, G.F.; Gan, T.Y. Assessment of evapotranspiration models applied to a watershed of Canadian Prairies with mixed land-uses. Hydrol. Process. 2000, 14, 1305–1325. [Google Scholar] [CrossRef]
- Maki, T. Interrelationships between zero-plane displacement, aerodynamic roughness length and plant canopy height. J. Agric. Meteorol. 1975, 31, 7–15. [Google Scholar] [CrossRef]
- Inclán, M.G.; Forkel, R. Comparison of energy fluxes calculated with the Penman–Monteith equation and the vegetation models SiB and Cupid. J. Hydrol. 1995, 166, 193–211. [Google Scholar] [CrossRef]
- Madeira, A.C.; Kim, K.S.; Taylor, S.E.; Gleason, M.L. A simple cloud-based energy balance model to estimate dew. Agric. For. Meteorol. 2002, 111, 55–63. [Google Scholar] [CrossRef]
- Holtslag, A.A.M.; de Bruin, H.A.R. Applied modeling of the nighttime surface energy balance over land. J. Appl. Meteorol. 1988, 27, 689–704. [Google Scholar] [CrossRef]
- Brutsaert, W. Evaporation into the Atmosphere: Theory, History, and Applications; Reidel: Dordrecht, The Netherlands, 1982. [Google Scholar]
- Penman, H.L. Natural evaporation from open water, bare soil and grass. Proc. R. Soc. Lond. A 1948, 193, 120–145. [Google Scholar] [CrossRef]
- Monteith, J.L. Principles of Environmental Physics; Arnold: London, UK, 1973. [Google Scholar]
- Buck, A.L. New equations for computing vapor pressure and enhancement factor. J. Appl. Meteorol. 1981, 20, 1527–1532. [Google Scholar] [CrossRef]

| Time | EEB | ETVT | EPM | EBREB | g | gΨ | EEB | ETVT | EPM | EBREB |
|---|---|---|---|---|---|---|---|---|---|---|
| W/m2 | W/m2 | W/m2 | W/m2 | mol/m2/s | mol/m2/s | W/m2 | W/m2 | W/m2 | W/m2 | |
| Data for 18:30 on 19 November to 8:00 on 20 November 2009 | ||||||||||
| 18:30 | 47.2 | −42.4 | 2.4 | −15.8 | 0.467 | 0.234 | −19.2 | −14.0 | −16.6 | −15.8 |
| 19:00 | 45.5 | −46.7 | −2.1 | −16.0 | 0.429 | 0.203 | −16.6 | −15.4 | −16.0 | −16.0 |
| 20:00 | 17.7 | −34.1 | −10.0 | −16.0 | 0.351 | 0.154 | −24.2 | −11.2 | −17.3 | −16.0 |
| 21:00 | 32.2 | −50.1 | −13.0 | −16.8 | 0.450 | 0.238 | −16.8 | −16.5 | −16.8 | −16.8 |
| 22:00 | 37.8 | −51.8 | −12.0 | −16.1 | 0.519 | 0.306 | −14.2 | −17.1 | −15.9 | −16.1 |
| 23:00 | 43.9 | −50.5 | −9.6 | −14.3 | 0.461 | 0.240 | −10.2 | −16.7 | −13.9 | −14.3 |
| 0:00 | 46.2 | −48.5 | −8.3 | −12.5 | 0.470 | 0.252 | −6.5 | −16.0 | −12.1 | −12.5 |
| 1:00 | 28.0 | −39.8 | −11.2 | −13.6 | 0.469 | 0.268 | −13.9 | −13.1 | −13.6 | −13.6 |
| 2:00 | 41.5 | −47.8 | −10.4 | −14.3 | 0.472 | 0.253 | −11.4 | −15.8 | −14.0 | −14.3 |
| 3:00 | 42.3 | −48.4 | −10.8 | −12.7 | 0.446 | 0.232 | −7.3 | −16.0 | −12.5 | −12.7 |
| 4:00 | 1.5 | −21.1 | −11.9 | −12.0 | 0.251 | 0.099 | −18.9 | −7.0 | −12.0 | −12.0 |
| 5:00 | 29.1 | −34.3 | −8.4 | −8.5 | 0.378 | 0.190 | −4.3 | −11.3 | −8.5 | −8.5 |
| 6:00 | 25.1 | −31.1 | −7.9 | −8.1 | 0.339 | 0.159 | −4.7 | −10.3 | −8.0 | −8.1 |
| 7:00 | 1.4 | −12.6 | −7.0 | −7.0 | 0.196 | 0.070 | −11.1 | −4.2 | −7.1 | −7.0 |
| 8:00 | 16.7 | −19.8 | −4.8 | −4.8 | 0.278 | 0.124 | −2.3 | −6.5 | −4.8 | −4.8 |
| Data for 18:00 on 20 November to 8:00 on 21 November 2009 | ||||||||||
| 18:00 | 24.3 | −47.3 | −11.0 | −17.2 | 0.315 | 0.123 | −19.0 | −15.6 | −17.4 | −17.2 |
| 19:00 | 35.9 | −45.6 | −4.7 | −11.3 | 0.363 | 0.166 | −5.8 | −15.0 | −10.5 | −11.3 |
| 20:00 | 25.6 | −37.6 | −5.6 | −12.1 | 0.355 | 0.166 | −11.3 | −12.4 | −11.9 | −12.1 |
| 21:00 | 43.2 | −29.1 | 8.4 | −4.6 | 0.343 | 0.154 | 5.2 | −9.6 | −1.9 | −4.6 |
| 22:00 | 25.7 | −20.4 | 4.0 | −5.6 | 0.388 | 0.214 | −3.1 | −6.7 | −4.8 | −5.6 |
| 23:00 | 29.7 | −3.9 | 15.0 | −1.0 | 0.348 | 0.176 | 1.7 | −1.3 | 0.4 | −1.0 |
| 0:00 | 39.9 | −1.1 | 22.4 | −0.1 | 0.343 | 0.168 | 10.1 | −0.4 | 5.7 | −0.1 |
| 1:00 | 28.7 | −1.1 | 16.0 | −0.4 | 0.378 | 0.204 | −0.7 | −0.4 | −0.5 | −0.4 |
| 2:00 | 41.2 | −0.9 | 23.3 | −0.2 | 0.458 | 0.276 | 5.1 | −0.3 | 2.8 | −0.2 |
| 3:00 | 67.1 | −3.4 | 37.6 | −0.4 | 0.578 | 0.382 | 16.2 | −1.1 | 9.0 | −0.4 |
| 3:30 | 53.1 | −6.6 | 28.1 | −0.7 | 0.473 | 0.285 | 13.0 | −2.2 | 6.6 | −0.7 |
| 4:00 | 36.7 | −10.1 | 16.9 | −1.7 | 0.372 | 0.196 | 5.8 | −3.3 | 1.9 | −1.7 |
| 5:00 | 22.2 | −43.5 | −6.7 | −15.2 | 0.373 | 0.183 | −16.0 | −14.3 | −15.4 | −15.2 |
| 6:00 | 25.7 | −75.8 | −22.7 | −25.5 | 0.305 | 0.109 | −25.5 | −25.0 | −25.4 | −25.5 |
| 7:00 | 25.4 | −21.1 | −23.4 | −23.5 | 0.099 | 0.016 | −39.4 | −7.0 | −23.6 | −23.5 |
| 8:00 | 31.2 | −53.7 | −12.9 | −13.0 | 0.227 | 0.064 | −7.7 | −17.7 | −12.9 | −13.0 |
| ΔT (°C) | u (m/s) | ||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| 1 | 55.2 | 32.8 | 20.6 | 13.8 | 9.7 |
| 2 | 64.9 | 44.3 | 31.0 | 22.3 | 16.5 |
| 3 | 69.7 | 50.9 | 37.6 | 28.3 | 21.7 |
| 4 | 72.7 | 55.3 | 42.4 | 32.9 | 25.9 |
| 5 | 75.0 | 58.6 | 46.1 | 36.6 | 29.4 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, H.; Meissner, R.; Borg, H.; Wang, R.; Cao, Q. Agreement of Four Equations for Computing Dewfall in Northern Germany. Water 2017, 9, 607. https://doi.org/10.3390/w9080607
Xiao H, Meissner R, Borg H, Wang R, Cao Q. Agreement of Four Equations for Computing Dewfall in Northern Germany. Water. 2017; 9(8):607. https://doi.org/10.3390/w9080607
Chicago/Turabian StyleXiao, Huijie, Ralph Meissner, Heinz Borg, Ruoshui Wang, and Qiqi Cao. 2017. "Agreement of Four Equations for Computing Dewfall in Northern Germany" Water 9, no. 8: 607. https://doi.org/10.3390/w9080607
APA StyleXiao, H., Meissner, R., Borg, H., Wang, R., & Cao, Q. (2017). Agreement of Four Equations for Computing Dewfall in Northern Germany. Water, 9(8), 607. https://doi.org/10.3390/w9080607




