Water Use and Yield of Soybean under Various Irrigation Regimes and Severe Water Stress. Application of AquaCrop and SIMDualKc Models

Abstract

Data relative to two soybean seasons, several irrigation scheduling treatments, including moderate and severe deficit irrigation, and rain-fed cropping were used to parameterize and assess the performance of models AquaCrop and SIMDualKc, the latter combined with the Stewart’s yield model. SIMDualKc applies the FAO56 dual crop coefficient approach for computing and partitioning evapotranspiration (ET) into actual crop transpiration (T_{c act}) and soil evaporation (E_s), while AquaCrop uses an approach that depends on the canopy cover curve. The calibration-validations of models were performed by comparing observed and predicted soil water content (SWC) and grain yield. SIMDualKc showed good accuracy for SWC estimations, with normalized root mean square error (NRMSE) ≤ 7.6%. AquaCrop was less accurate, with NRMSE ≤ 9.2%. Differences between models regarding the water balance terms were notable, and the ET partition revealed a trend for under-estimation of T_{c act} by AquaCrop, mainly under severe water stress. Yield predictions with SIMDualKc-Stewart models produced NRMSE < 15% while predictions with AquaCrop resulted in NRMSE ≤ 23% due to under-estimation of T_{c act}, particularly for water stressed treatments. Results show the appropriateness of SIMDualKc to support irrigation scheduling and assessing impacts on yield when combined with Stewart’s model.

1. Introduction

Uruguay is characterized by a warm temperate and humid climate, where summer crops are commonly rain-fed. Due to rainfall uncertainty, supplemental irrigation is often required for achieving high yields [1]. Thus, adequate irrigation scheduling has to be considered for soybean production. Predicting soybean yield response to water is required for assessing irrigation management strategies to be adopted by farmers. Attention should be paid to the crop stages where water stress is most critical, with several studies having identified the period from flowering to grain filling as the most sensitive to water stress [2,3,4].

Crop growth and yield models are often used. The CROPGRO-Soybean model is probably the most used to simulate soybean growth and yield. It is one of the Decision Support System for Agrotechnology Transfer-Cropping System Models (DSSAT-CSM) whose features are discussed in detail by Jones et al. [5]. Because DSSAT-CSM are oriented to represent the growth and yield processes considering a variety of constraints and stresses, they are rarely used for assessing water use or for developing irrigation scheduling scenarios. However, several applications of CROPGRO-Soybean are reported [6,7,8]. The RZWQM-CROPGRO hybrid model for soybean production [6] combines the more precise approach to water and solutes dynamics of RZWQM with the accurate prediction of yield of CROPGRO-Soybean, thus, resulting in a more useful model for practical applications related to water. Another modeling approach consists of the model SoySim [9] that has been tested on several locations and different crop varieties, growth constraints, and cropping practices. Moreover, it has been compared with other models: CROPGRO-Soybean [5], Sinclair-Soybean [10], and WOFOST [11]. A recent application of SoySim to yield prediction in Brazil was reported by Cera et al. [12]. Crop growth and yield models are quite complex, require a large number of parameters, and their parameterization is generally difficult and demanding in terms of agronomic data acquisition. Therefore, these models are generally more adequate for research purposes or for yield prediction than for operational use as a support to irrigation management by the farmers, and they may be less accurate in simulating soil water dynamics and water use; however, these models, like SOYGRO, may be useful to define irrigation schedules [13].

The Food and Agriculture Organization (FAO) AquaCrop model [14], a hybrid semi-empiric and deterministic model, is aimed at both crop yield and water use simulation and has become quite popular recently, likely because it is less demanding in terms of parameterization than the models referred to above [15,16]. However, it is much more complex in parameterization than simplified approaches combining a soil water balance model and a water-yield model such as the SIMDualKc water balance model in combination with the Stewart’s water-yield model [17,18], as reported by Paredes et al. [19,20] and by Pereira et al. [21].

Applications of the Stewart’s model are often reported in literature aiming at simplifying the assessment of irrigation scheduling impacts on yields [22,23,24,25] as it has fewer parameterization requirements than the above referred crop growth and yield models. The Stewart’s model linearly relates the relative yield loss (1 − Y_a/Y_m) to the relative evapotranspiration (ET) deficit (1 − ET_{c act}/ET_c) through the water-yield response factor K_y, where the actual and potential yields (Y_a and Y_m) are produced when ET are, respectively, the actual and potential crop ET (ET_{c act} and ET_c). A modified version of the Stewart’s model, where (1 − ET_{c act}/ET_c) is replaced by the relative transpiration deficit (1 − T_{c act}/T_c), was successful reported for cereals [19,21] and grain legumes [20,26], with actual and potential transpiration (T_{c act} and T_c) computed with the water balance SIMDualKc model, which partitions daily ET in its components T_{c act} and soil evaporation E_s.

Considering that SIMDualKc has already been calibrated and successfully used in various applications worldwide, and that AquaCrop acceptably predicted soybean yields in southern Brazil [27], the objectives of the present study consisted of: (1) parameterizing and testing the AquaCrop model for different water management treatments; (2) calibration and validation of the SIMDualKc model for the same treatments; (3) analyzing soybean water balance terms and evapotranspiration partition with both the AquaCrop and the SIMDualKc models; (4) assessing the accuracy of the AquaCrop model and the Stewart’s water-yield model combined with SIMDualKc to predict soybean yields under various water stress conditions; and (5) assessing the strengths and weaknesses of both modelling approaches for supporting irrigation management.

2. Material and Methods

2.1. Site Characterization and Description of the Experiments

Field experiments were developed during the soybean cropping seasons of 2009–2010 to 2012–2013 in an Experimental Station at Paysandú, western Uruguay (32°22′ S, 58°4′ W, and 50 m elevation). Data for 2009–2010 and 2010–2011 were incomplete, lacking adequate soil water observations that could be used for models testing or validation; nevertheless, data were appropriate for soybean yield assessments. The average annual temperature during the period 1993–2014 was 18.3 °C and the average annual precipitation was 1327 mm, but with large inter-annual variability due to impacts of the El Niño Southern Oscillation [28] and the Pacific Decadal Oscillation [29]. Local climate is warm temperate, with humid and hot summers: Cfa according to the Köppen-Geiger classification [30]. Weather daily data including maximum and minimum air temperature (°C), solar radiation (MJ·m⁻²·d⁻¹), air relative humidity (%), wind speed (m·s⁻¹), and precipitation (mm) were collected by an automatic meteorological station (Vantage Pro 2TM, Davis Instruments, Hayward, CA, USA) located near the experimental fields. These data were used to compute daily reference evapotranspiration (ET_o) with the FAO Penman Monteith (FAO-PM) equation [31]. The variability of daily rainfall and ET_o during the soybean crop seasons is given in Figure A1.

The soil in the experimental fields is a Eutric Cambisol, loamy in the top layer and clay loamy underneath. The total available water (TAW), which represents the difference between the water storage in the root zone at field capacity (33 kPa) and permanent wilting point (1500 kPa), is 176 mm and 144 mm for soils 1 and 2, respectively. The respective main soil hydraulic properties are presented in

Table A1. Main soil hydraulic properties of the experimental site, Paysandú.

Layer Depth (m)	Soil 1				Soil 2
	θsat (cm3·cm−3)	θFC (cm3·cm−3)	θWP (cm3·cm−3)	Ksat (cm·day−1)	θsat (cm3·cm−3)	θFC (cm3·cm−3)	θWP (cm3·cm−3)	Ksat (cm·day−1)
0–0.20	0.52	0.36	0.16	57.4	0.46	0.30	0.14	40.5
0.20–0.60	0.52	0.45	0.29	64.7	0.50	0.40	0.26	50.2
0.60–1.00	0.54	0.37	0.19	65.4	0.47	0.32	0.18	51.5

Notes: θ_sat, θ_FC,, and θ_WP are, respectively, the soil water content at saturation, field capacity, and wilting point; K_sat is the saturated hydraulic conductivity.

. The soil water content (SWC) was measured with a calibrated neutron probe (503DR HYDROPROBE, InstroTek Inc., Martinez, CA, USA). Measurements were performed every 0.10 m until a maximum depth of 1.00 m. Soil sampling was used for the upper 0.10 m layer. Plots were cropped with the soybean variety “Don Mario 5.1i RR” (maturity group V) that is of indeterminate growth and has high yield potential. Each plot was 5 m × 2 m, with five rows spaced 0.4 m. The plant density was 30 plants m⁻². Cropping practices were those recommended locally by the extension services. The irrigation system consisted of pressure compensating in-line drippers spaced 0.20 m and discharging 1.5 L·h⁻¹. Irrigations were scheduled by performing a simple daily soil water balance applied to a depth of 1.0 m using the computed ET_o and the measured SWC data. The irrigation trigger was a depletion of 60% of TAW during periods when water stress was induced, and a depletion of 40% of TAW otherwise. Irrigation depths were set to refill SWC up to 90% of θ_FC in the periods when water stress was not allowed and up to 60% of θ_FC otherwise.

The following treatments were adopted:

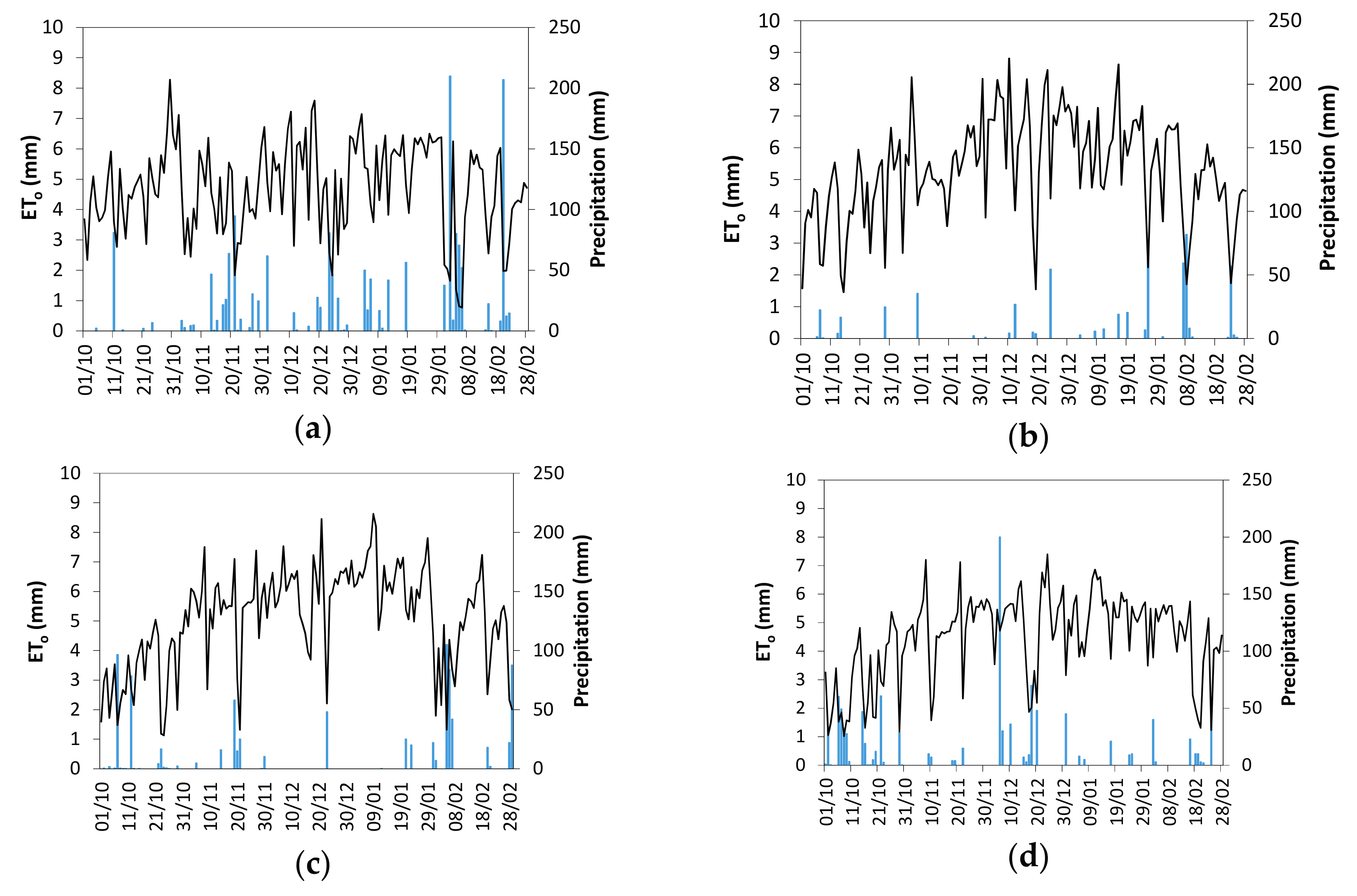
(a)

FI, full irrigation, aimed at fully satisfying crop water requirements, thus to avoid water stress in all crop growth stages;

(b)

DI_GFill, deficit irrigation during the flowering to grain filling periods;

(c)

DI_Veg, deficit irrigation during the vegetative period;

(d)

DI_Veg-GFill, deficit irrigation during the vegetative to the grain filling periods;

(e)

Rain-fed.

Water deficits were induced by withholding irrigation or precipitation using rain shelters to allow for water deficits to be induced at desired timings in the crop season. Three replications of the referred five irrigation treatments were adopted. Completely random blocks were used. To assure good crop establishment, no stress was allowed during emergence. The irrigation depths applied during both crop seasons and all irrigation treatments are presented in

Table A2. Crop growth stages dates and cumulated growing degree days (CGDD) for experimental seasons of 2011–2012 and 2012–2013.

		Crop Growth Stages
Year		Initial	Crop Development	Mid-Season	Late-Season
2011–2012	Dates	11 November to 29 November	30 November to 20 December	21 December to 4 March	5 March to 9 April
	CGDD (°C) *	336	654	2015	2640
2012–2013	Dates	3 December to 17 December	18 December to 17 January	18 January to 24 March	24 March to 25 March
	CGDD (°C) *	363	759	1894	2235

Note: * values obtained using a base temperature of 5 °C and a cut-off temperature of 30 °C.

.

The dates of each crop growth stage as defined in FAO56 [31] and the respective cumulated growing degree days (CGDD, °C) are presented in

Table A3. Net irrigation depths (mm) of all irrigation treatments in soybean seasons of 2011–2012 and 2012–2013.

	Irrigation Depths					Irrigation Depths
Dates	FI	DIGFill	DIVeg	DIVeg-GFill	Dates	FI	DIGFill	DIVeg	DIVeg-GFill
16 November 2011	36	36	36	36	5 December 2012	18	18	18	18
5 December 2011	36	36			29 December 2012	54	54	54	54
10 December 2011	36				4 January 2013	36	36	36	54
14 December 2011	36				9 January 2013	36	36		36
19 December 2011	36	36			14 January 2013	36	36
1 January 2012	48	54			21 January 2013	36	36		36
4 January 2012	36				28 January 2013	36	36
9 January 2012	18	54		54	11 February 2013	36		54	54
20 January 2012			54		16 February 2013	54		54
30 January 2012	36		36		15 March 2013		54		54
15 February 2012	36		36
Total	354	216	162	90	Total	342	306	216	306

. Measurements of the photosynthetically active radiation (PAR) were performed in the treatment FI using a ceptometer (Decagon AccuPar LP 80). Following Farahani et al. [32], these measurements were converted into canopy cover (CC) and fraction of ground cover (f_c) for use with AquaCrop and SIMDualKc, respectively. The crop height (h, m) and rooting depths (Z_r, m) were randomly measured, and the maximum root depth observed was 1 m. The final above ground biomass and soybean grain yield were obtained from harvesting all experimental plots, thus, three samples per irrigation treatment were used; samples were oven dried to a constant weight at 65 ± 5 °C.

2.2. Modelling

Two modelling approaches were used: (a) the SIMDualKc [33] soil water balance model that uses the FAO56 dual crop coefficient approach for partitioning crop ET and was combined with the modified Stewart’s global water-yield model [17] for yield predictions; and (b) the crop growth and yield model AquaCrop, that partitions ET based upon the canopy cover (CC).

As revised previously [34,35], the FAO56 dual crop coefficient approach (dual-K_c, [31,36]) accurately models and partitions ET as described in several studies (e.g., [37,38]) and when compared with the dual-source Shuttleworth-Wallace model [39]. The SIMDualKc model has been positively tested for actual transpiration using sap-flow measurements [40,41] and for soil evaporation using micro-lysimeters [42,43] including soybeans [26]. The SIMDualKc model computes crop evapotranspiration (ET_c) under standard/potential, non-limiting conditions as

$${\mathrm{ET}}_{\mathrm{c}}=({\mathrm{K}}_{\mathrm{cb}}+{\mathrm{K}}_{\mathrm{e}}){\mathrm{ET}}_{\mathrm{o}}$$

(1)

where ET_o (mm) is the reference evapotranspiration, K_cb (dimensionless) is the potential basal crop coefficient that describes transpiration (T_c), and K_e (dimensionless) is the soil water evaporation coefficient that describes soil evaporation (E_s). The model provides for separately computing potential transpiration T_c = K_cb ET_o (mm) and soil evaporation E_s = K_e ET_o (mm). The actual crop ET (ET_{c act}, mm) is computed by the model as a function of the available soil water in the root zone (ASW): when soil water extraction is smaller than the depletion fraction for no stress (p) then ET_{c act} = ET_c, otherwise ET_{c act} < ET_c and decreases with decreasing ASW. The ET_{c act} and the T_{c act} are, therefore, defined as

$${\mathrm{ET}}_{\mathrm{c}\mathrm{act}}=({\mathrm{K}}_{\mathrm{s}} {\mathrm{K}}_{\mathrm{cb}}+{\mathrm{K}}_{\mathrm{e}}){\mathrm{ET}}_{\mathrm{o}}$$

(2)

$${\mathrm{T}}_{\mathrm{c}\mathrm{act}}={\mathrm{K}}_{\mathrm{s}} {\mathrm{K}}_{\mathrm{cb}} {\mathrm{ET}}_{\mathrm{o}}$$

(3)

where K_s (dimensionless) is the water stress coefficient (0–1). K_s is computed through a soil water balance applied to the entire root zone (SWB). Soil evaporation is given as

$${\mathrm{E}}_{\mathrm{s}}={\mathrm{K}}_{\mathrm{e}} {\mathrm{ET}}_{\mathrm{o}}$$

(4)

with K_e depending on the fraction of ground cover by vegetation (f_c) and the SWC in the soil layer with depth Z_e of 10–15 cm. K_e is computed daily through an SWB of the evaporation layer, which is characterized by the readily and total evaporable water (REW, TEW, mm); REW and TEW may be computed from the soil textural and water holding characteristics of the top-layer [31,36]. K_e is adjusted for mulches and for the fraction of soil wetted by irrigation and exposed to radiation.

The SWB of the root zone is performed by computing the soil water depletion D_r,i at the end of every day i:

$${\mathrm{D}}_{\mathrm{r},\mathrm{i}}={\mathrm{D}}_{\mathrm{r},\mathrm{i}-1}-{(\mathrm{P}-\mathrm{RO})}_{\mathrm{i}}-{\mathrm{I}}_{\mathrm{i}}-{\mathrm{CR}}_{\mathrm{i}}+{\mathrm{ET}}_{\mathrm{c}\mathrm{act},\mathrm{i}}+{\mathrm{DP}}_{\mathrm{i}}$$

(5)

where the depletion D_r,i−1 of the precedent day is i − 1 and the precipitation P, runoff RO, net irrigation depth I, capillary rise CR, deep percolation DP, and crop ET_{c act} are in mm and refer to day i. CR was not considered because the water table was deep. RO was computed using the curve number (CN) approach [44]. DP was computed with a parametric equation [45] requiring two parameters, a_D characterizing storage and b_D referring to the velocity of vertical drainage, both estimated from soil physical characteristics [45].

The SIMDualKc model calibration consists of searching the model crop parameters—basal crop coefficients K_cb and depletion fraction for no stress p, soil evaporation parameters Z_e, TEW and REW, runoff curve number CN, and DP parameters a_D and b_D—that minimize the deviations between the simulated and observed SWC values. The calibration is performed through an iterative procedure of searching the best parameter values within a reasonable range until SWC errors stabilize, as discussed by Pereira et al. [21]. This procedure is first applied to the crop parameters and, after, to the remaining parameters and, finally, to all parameters together. Validation consists of testing the model using the calibrated set of parameters with one or more sets of independent field data collected in the same or different years. However, if validation is performed in a soil with different characteristics, then parameters Z_e, TEW, REW, a_D, and b_D have to be adjusted as described by Giménez et al. [46] for maize in Paysandú. Model calibration was performed using SWC values observed in the FI treatment in 2011–2012. The validation used all other datasets of 2011–2012 and 2012–2013.

As stated above, the SIMDualKc model was combined with a modified version of the water-yield model proposed by Stewart et al. [17] to assess the impacts of water deficits on yields. The version used in the present study assumes a linear variation of the relative yield loss with the relative crop transpiration deficit [19]:

$$1-\frac{{\mathrm{Y}}_{\mathrm{a}}}{{\mathrm{Y}}_{\mathrm{m}}}={\mathrm{K}}_{\mathrm{y}}\left(1-\frac{{\mathrm{T}}_{\mathrm{c}\mathrm{act}}}{{\mathrm{T}}_{\mathrm{c}}}\right)$$

(6)

where Y_a and Y_m are the actual and maximum yields (kg·ha⁻¹) corresponding, respectively, to the seasonal T_{c act} and T_c (mm), and K_y is the water-yield response factor. The Y_a values consist of observed dry grain. Values for Y_m were obtained from maximum yields observed, further using the Wageningen method [18] and checking results against maximal yields achieved by best farmers. The resulting Y_m are 6.15 and 5.22 t·ha⁻¹, respectively, for 2011–2012 and 2012–2013. The value K_y = 1.25 was adopted from solving Equation (6) relative to K_y using all experimental data available. After knowing K_y and Y_m, yield predictions were performed by solving Equation (6) in relation to Y_a for all T_{c act} results of SIMDualKc.

The AquaCrop model is a crop growth and yield model used for a variety of field crops, including soybean, mainly aiming at yield prediction. The model is described by Raes et al. [14] and Vanuytrecht et al. [47], and its open source is described by Foster et al. [48], as well as in various papers quoted there. T_c is computed as

T_c = CC*K_cTr,x ET_o

(7)

where CC* is the crop canopy cover (%) adjusted for micro-advective effects, and K_cTr,x is the maximum standard crop transpiration coefficient (dimensionless) that corresponds to the K_{cb mid} parameter in FAO56 [31]. T_{c act} is obtained by adjusting T_c using the water stress coefficient K_s (0–1), as

T_{c act} = K_s T_c

(8)

K_s in AquaCrop is, however, more complex than in FAO56 because it describes the effects of the soil water stress on various processes and the depletion fractions p are inputs of the model that, contrary to SIMDualKc, do not require calibration [14].

Soil evaporation is also obtained from CC* as

E_s = K_r (1 − CC*) K_ex ET_o

(9)

where K_ex is the maximum soil evaporation coefficient (non-dimensional) and K_r is the evaporation reduction coefficient (0–1), with K_r < 1 when insufficient water is available in the top soil to respond to the evaporative demand of the atmosphere [14]. The product K_r (1 − CC*) K_ex corresponds to K_e as defined in FAO56 as described above. The canopy cover (CC) is similar to f_c in FAO56 but while SIMDualKc uses observed f_c for adjusting K_e, in AquaCrop the CC observations are used to parameterize a CC* curve which is performed in three phases and focuses on four parameters that describe the curve: canopy cover at 90% emergence (cc_o), maximum canopy cover (CC_x), canopy growth coefficient (CGC), and canopy decline coefficient (CDC) [14].

The above ground dry biomass (B, t·ha⁻¹) is estimated by the model using the water transpired by the crop throughout the season and the normalized biomass water productivity (BWP*, g·m⁻²). BWP* represents B produced per unit of area considering the cumulative transpiration and ET_o [14]. The crop yield (Y, t·ha⁻¹) is computed from B as

Y = f_HIHI_o B

(10)

where HI_o is the reference harvest index, describing the harvestable proportion of B, and f_HI is an adjustment factor integrating five water stress factors [14].

The model parameterization was initialized using the parameter values proposed by Raes et al. [14]. The parameterizations of the CC curves were first performed using a trial and error procedure. Once these curves were properly parameterized, the trial and error procedure was applied to search the K_cTr,x value that leads to a better fit of SWC. In this search, the CN and REW values found for SIMDualKc were used. Growth and yield parameters of AquaCrop were obtained using the above-ground biomass observations. The parameters retained after parameterization using FI data of 2011–2012 were used for model testing using all data sets.

“Goodness-of-fit” indicators were used to assess the accuracy of model simulations at calibration and validation of SIMDualKc and parameterization and testing of AquaCrop. Indicators, following Legates and McCabe Jr. [49], Moriasi et al. [50], and described by Pereira et al. [21], were computed from the pairs of observed and predicted values, respectively, O_i and P_i (i = 1, 2, ..., n) with means $\overline{\mathrm{O}}$ and $\overline{\mathrm{P}}$. The regression coefficient b₀ of a regression forced to the origin relating O_i and P_i was used to verify the similarity between the simulated and observed values. The determination coefficient R² of the ordinary least squares regression of the same variables was used to assess the dispersion of pairs of O_i and P_i values along the regression line, with large R² indicating that a large fraction of the variance of observations was explained by the model. The root mean square error (RMSE) and the normalized root mean square error relative to the mean of observations (NRMSE) were adopted to assess modelling errors. In addition, the Nash and Sutcliff [51] modelling efficiency (EF) was adopted to express the relative magnitude of the mean square error (MSE = RMSE²) in relation to the variance of the observed data [49].

3. Results and Discussion

3.1. Soil Water Simulation and Models Calibration and Parameterization

Simulations with both models are presented in Figure 1: Figure 1a,b refer to DI_Veg-GFill in 2011–2012, when a severe water deficit was applied from the vegetative growth to grain filling, a sensitive period to water stress; Figure 1c,d refer to FI in 2012–2013, where water stress was avoided; and Figure 1e,f are relative to rain-fed cropping in 2012–2013, where only a limited stress occurred during pod formation. Water stress for the rain-fed crop is smaller than for that of the DI_Veg-GFill treatment because, contrarily to the latter, rainfall was not avoided during any period. Results show that both models behaved well and in a similar way, which is due to their careful calibration/parameterization.

The “goodness-of-fit” indicators relative to all SWC simulations with SIMDualKc and AquaCrop (

Table 1. “Goodness-of-fit” indicators of the simulation of SWC with SIMDualKc and AquaCrop.

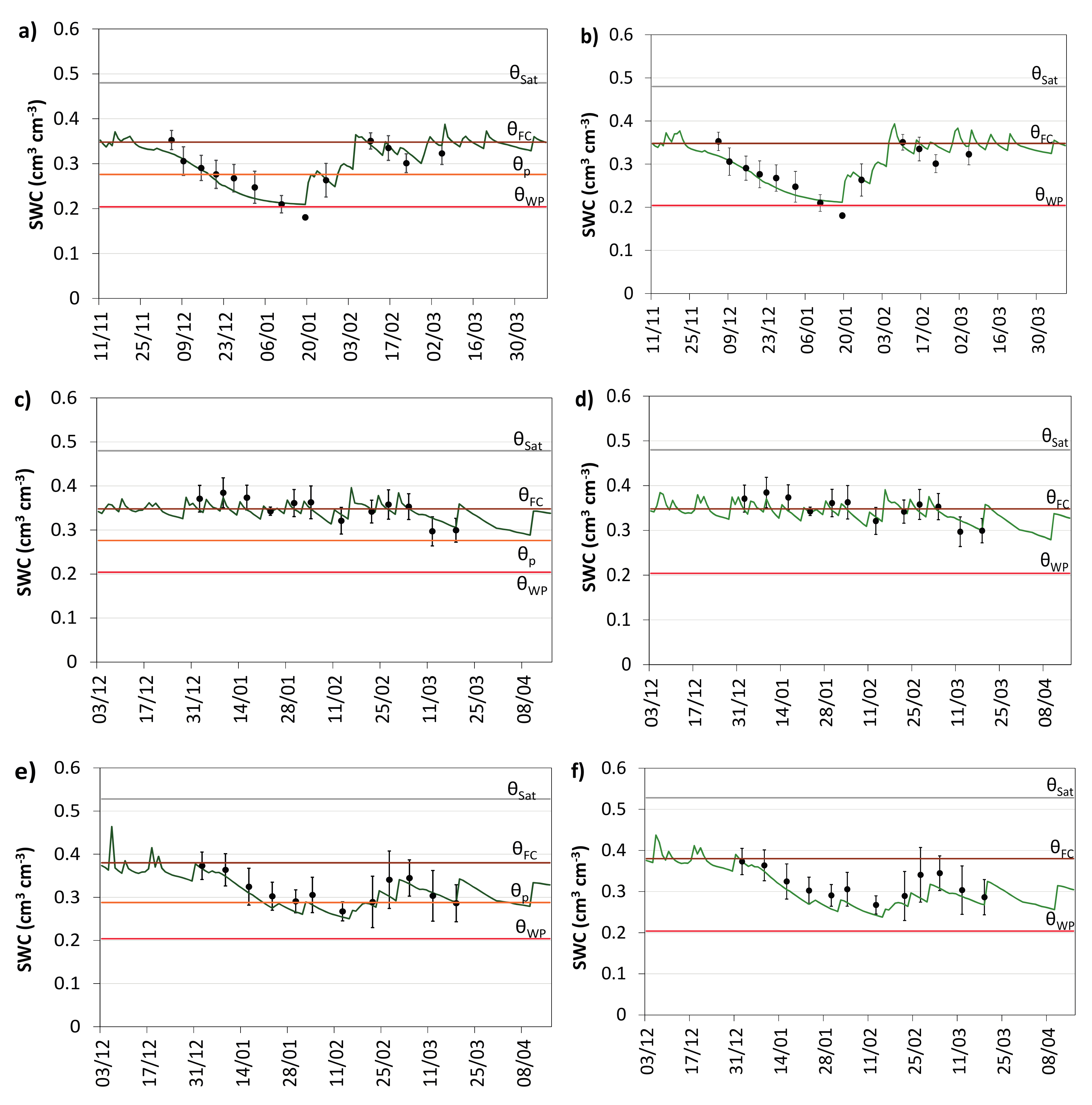
Model	Crop Season	Irrigation Strategy	b0	R2	RMSE (cm3·cm−3)	NRMSE (%)	EF
SIMDualKc	2011–2012	FI	0.99	0.65	0.019	5.6	0.63
		DIGFill	0.98	0.73	0.025	7.6	0.71
		DIVeg	0.99	0.86	0.019	6.6	0.86
		DIVeg-GFill	0.97	0.84	0.017	5.9	0.79
		Rain-fed	0.98	0.83	0.019	6.5	0.82
	2012–2013	FI	0.98	0.74	0.017	4.8	0.61
		DIGFill	0.98	0.94	0.014	4.2	0.91
		DIVeg	0.99	0.79	0.017	5.1	0.69
		DIVeg-GFill	1.01	0.82	0.015	4.8	0.80
		Rain-fed	0.95	0.86	0.019	6.0	0.64
AquaCrop	2011–2012	FI	0.99	0.61	0.020	6.1	0.57
		DIGFill	1.03	0.72	0.028	8.5	0.64
		DIVeg	1.00	0.93	0.010	3.4	0.93
		DIVeg-GFill	1.00	0.83	0.021	7.3	0.83
		Rain-fed	0.99	0.88	0.016	5.3	0.88
	2012–2013	FI	0.97	0.76	0.018	5.0	0.58
		DIGFill	0.95	0.92	0.021	6.6	0.79
		DIVeg	1.00	0.76	0.023	7.0	0.41
		DIVeg-GFill	1.06	0.87	0.022	7.3	0.54
		Rain-fed	0.92	0.86	0.029	9.2	0.16

Notes: b₀ and R² are the coefficients of regression and determination, respectively; RMSE is the root mean square error; NRMSE is the normalized root mean square error; EF is the model efficiency; FI is full irrigation; DI_GFill is deficit irrigation during the flowering to grain filling periods; DI_Veg is deficit irrigation during the vegetative period; DI_Veg-GFill is deficit irrigation during the vegetative to the grain filling periods.

) show a better model performance when SIMDualKc is used. Regression coefficients (b₀) ranged from 0.95 to 1.01 and R² varied from 0.65 to 0.94 for SIMDualKc applications indicating that the predicted and observed values were statistically similar and a large fraction of the total variance of the observed SWC values was explained by the model. Wider but acceptable values were obtained for AquaCrop, with b₀ ranging from 0.92 to 1.06 and R² varying from 0.61 to 0.92. The estimation errors were small for SIMDualKc (RMSE < 0.025 cm³·cm⁻³ and NRMSE < 7.6%) and slightly larger for AquaCrop (RMSE < 0.029 cm³·cm⁻³ and NRMSE < 9.2%). Model efficiency was high for SIMDualKc, with EF ranging from 0.61 to 0.91, indicating that simulation errors MSE were much smaller than the variance of SWC observations. In contrast, EF values obtained for AquaCrop showed a wider range of variation, 0.16 to 0.93, indicating that MSE varied widely relative to the variance of observations. Overall, results indicate that though both models are appropriate for simulating daily SWC, SIMDualKc performed better.

The SIMDualKc calibrated parameters—K_cb, p, TEW, REW, Z_e, CN, a_D, and b_D—are presented in

Table 2. SIMDualKc calibrated parameters and AquaCrop conservative and calibrated parameters.

Model	Parameters		Values
SIMDualKc	Crop	Kcb ini	0.15
		Kcb mid	1.10
		Kcb end	0.35
		p ini, p dev, p mid, and p end	0.50
	Soil evaporation	REW (mm)	10
		TEW (mm)	23
		Ze (m)	0.10
	Deep percolation	aD	370/360 *
		bD	−0.017
	Runoff	CN	80
AquaCrop	Conservative crop	Base temperature(°C)	5
		Cut-off temperature (°C)	30
		Canopy cover at 90% emergence (cco, %)	1.5
		Soil water depletion threshold for canopy expansion (Upper and lower thresholds)	0.15 0.65
		Shape factor for water stress coefficient for canopy expansion	3.0
		Soil water depletion threshold for stomatal control	0.50
		Shape factor for water stress coefficient for stomatal control	3.0
	Calibrated crop	Crop coefficient for transpiration (KcTr,x)	1.10
		Adjusted biomass (water) productivity (BWP *, g·m−2)	14
		Maximum canopy cover (CCx, %)	100
		Canopy growth coefficient (CGC, % GDD−1)	0.744
		Canopy decline coefficient (CDC, % GDD−1)	0.440

Notes: K_{cb ini}, K_{cb mid} and K_{cb end} are respectively the basal crop coefficients for the initial, mid and end-season stages; p _ini, p _dev, p _{mid, and} p _end are the depletion fractions for no stress for the initial, crop development, mid and end-seasons stages; REW and TEW are the readily and total evaporable water; Z_e is the depth of the soil evaporation layer; CN is the curve number; a_D and b_D are the parameters of the deep percolation equation [46]. * different values were obtained due to the spatial heterogeneity of the soil among experimental plots.

. CN, Z_e, REW, TEW, a_D, and b_D are the same as those previously obtained by Giménez et al. [46] for the same experimental area because they essentially depend upon the soil characteristics rather than the crop. The K_cb and p values are equal to those proposed by Allen et al. [31], K_{cb ini} = 0.15, K_{cb mid} = 1.10 and K_{cb end} = 0.33. Slightly lower K_{cb mid} values were obtained by Odhiambo and Irmak [52] and Wei et al. [26]. K_{cb ini} and K_{cb end} reported by those authors are about the same as for the current study. Calibrated K_{cb mid} values are also coherent to the single crop coefficients K_{c mid} reported by Karam et al. [3], Tabrizi et al. [53] and Payero and Irmak [54]. Thus, results relative to potential K_cb and p values confirm those proposed in FAO56 [31].

Relative to AquaCrop, the “goodness-of-fit” of CC curves for FI in both seasons have shown a slight under-estimation trend, with b₀ = 0.93, but other goodness-of-fit indicators were generally high, with an R² of 0.99 and RMSE of 6.8% and 6.4% for 2011–2012 and 2012–2013 seasons, respectively. These values are within the range of other AquaCrop applications to soybean [15,16,55]; thus, one may consider the parameterization of the CC curves in the current study adequate.

The conservative and non-conservative parameters used in AquaCrop are also presented in Table 2. The value for K_cTr,x = 1.10 equals the K_{cb mid} calibrated with SIMDualKc (Table 2). Similar values were reported by Abi Saab et al. [15] and Paredes et al. [16]. BWP* = 14 g·m⁻² equals the one reported by Abi Saab et al. [15] and Khoshravesh et al. [55]; a higher value was reported by Paredes et al. [16]. For no-stress conditions, HI_o observed in both seasons averaged 0.38. That HI_o value equals that reported by Paredes et al. [16]; slightly smaller values were reported by Andrade [2] and larger values by Abi Saab et al. [15] and Khoshravesh et al. [55]. Differences in BWP* and HI_o values may relate to soybean varieties. Results analyzed show that parameters in Table 2 are appropriate for use in Uruguay.

3.2. Water Balance and Water Use Components

The actual ET_c computed with SIMDualKc and AquaCrop were quite similar (

Table 3. Simulated soil evaporation (E_s), actual transpiration (T_{c act}), and the ratio E_s/ET_{c act} for the various crop growth stages and all different irrigation treatments of soybean when using the SIMDualKc (SIM) and AquaCrop (Aqua) models in the 2011–2012 and 2012–2013 seasons.

Year/Strategy		Crop Stage
		Initial		Crop Development		Mid-Season		Late Season		Full Season
		SIM	Aqua	SIM	Aqua	SIM	Aqua	SIM	Aqua	SIM	Aqua
2011–2012/FI	Es (mm)	77	68	28	26	4	9	3	11	112	114
	Tc act (mm)	16	6	71	64	436	419	83	85	606	574
	Es/ETc act (%)	83	92	28	29	1	2	3	11	16	17
DIGFill	Es (mm)	75	70	22	27	3	9	2	11	102	117
	Tc act (mm)	16	6	71	64	407	416	83	85	577	571
	Es/ETc act (%)	82	92	24	30	1	2	2	11	15	17
DIVeg	Es (mm)	77	68	3	8	2	59	3	38	85	173
	Tc act (mm)	16	6	68	58	283	167	83	44	450	275
	Es/ETc act (%)	83	92	4	12	1	26	3	46	16	39
DIVeg-GFill	Es (mm)	75	70	3	8	2	34	2	25	82	137
	Tc act (mm)	16	6	68	61	262	235	83	68	429	370
	Es/ETc act (%)	82	92	4	12	1	13	2	27	16	27
Rain-fed	Es (mm)	70	70	3	8	2	36	3	22	78	136
	Tc act (mm)	16	6	68	61	290	250	83	67	457	384
	Es/ETc act (%)	81	92	4	12	1	13	3	25	15	26
2012–2013/FI	Es (mm)	64	62	51	49	3	1	1	3	119	115
	Tc act (mm)	11	2	95	96	291	295	50	61	447	454
	Es/ETc act (%)	85	97	35	34	1	0	2	5	21	20
DIGFill	Es (mm)	64	62	47	49	3	1	1	3	115	115
	Tc act (mm)	12	2	95	95	277	270	50	57	434	424
	Es/ETc act (%)	84	97	33	34	1	0	2	5	21	21
DIVeg	Es (mm)	63	63	39	48	2	8	1	5	105	124
	Tc act (mm)	12	2	95	95	250	248	50	58	407	403
	Es/ETc act (%)	84	97	29	34	1	3	2	8	21	24
DIVeg-GFill	Es (mm)	62	62	43	50	3	1	1	3	109	116
	Tc act (mm)	12	2	95	95	280	293	50	61	437	451
	Es/ETc act (%)	84	97	31	34	1	0	2	5	20	20
Rain-fed	Es (mm)	64	62	34	43	2	11	1	5	101	121
	Tc act (mm)	12	2	95	95	245	233	48	53	400	383
	Es/ETc act (%)	84	97	26	31	1	5	2	9	20	24

), which agree with the results of SWC simulation discussed above. However, its partition on T_{c act} and E_s produced different values, with AquaCrop generally giving a smaller T_{c act} and a larger E_s. Comparing Equations (3)–(7) and Equations (4)–(9), it is apparent that differences mainly stem from procedures used to compute the actual K_cb and K_e. In fact, the daily K_cb and K_{cb act} curves obtained with SIMDualKc and AquaCrop are quite different (Figure 2), particularly under severe water stress (Figure 2a,b). Differences largely stem from the form of the potential K_cb curve, with SIMDualKc using the typical linear variation of K_cb for the four crop growth stages adopted in FAO56 [31], i.e., a K_cb curve defined with only three values—K_{cb ini}, K_{cb mid}, and K_{cb end}—(Figure 2a,c,e), while a curvilinear variation of K_cb dictated by the parameterized CC curve is adopted in AquaCrop (Figure 2b,d,f). Without a very severe stress, the variation of K_cb are somewhat similar for both models (Figure 2c,d, and Figure 2e,f) but when a severe water stress occurs, e.g., DI_Veg-GFill in 2011–2012 (Figure 2b), the K_cb curve of AquaCrop is far from representing the potential K_cb defined in FAO56 [31,35] because this model does not use K_{cb mid} but just the maximum K_cTr,x. When water stress occurs but it does not affects crop development noticeably, as is the case of the rain-fed treatment, both Figure 2e,f show a similar behavior of K_{cb act} until the end of February, but not afterwards, likely due to the model approach used to compute the stress coefficient K_s in AquaCrop which includes various stresses in addition to soil water deficits.

The daily variation of the K_e in all examples of Figure 2 shows a similar behavior during the initial and early vegetative crop stages, though AquaCrop shows a tendency to estimate a larger K_e. In contrast, K_e tends to be larger afterwards when water stress occurs particularly during mid-season. Differences in K_e computed by both models increase when water deficits are larger (Figure 2a,b). Differences between models are due to the fact that K_e in SIMDualKc varies with the observed f_c and the daily computed depletion of the soil evaporation layer [33], while K_e in AquaCrop depends upon the fitted CC curve. Therefore, K_e tends to be higher with AquaCrop when water deficits occur. Under no stress conditions, differences are negligible (Figure 2c,d) as observed by Paredes et al. [16].

Analyzing the ET estimates and partition into E_s and T_{c act} during the initial period (Table 3) it was observed that E_s simulated by SIMDualKc represented 81% to 85% of ET_{c act} while AquaCrop simulated a larger E_s corresponding to 92% to 97% of ET_{c act}, thus resulting in a small T_{c act} during this period.

Throughout the crop development stage, E_s progressively decreased, as shown in Figure 2, due to the progressive decrease of the soil surface fraction exposed to solar radiation. During this period, E_s/ET_{c act} falls, in average, to 29% and 33% when computed with SIMDualKc and AquaCrop, respectively. During the mid-season, the soil is nearly fully shadowed by the crop and the energy available for evaporation drops to minimum values. Thus, E_s/ET_{c act} falls to 2% and 6% on average when computed with SIMDualKc and AquaCrop, respectively (Table 3). However, estimated E_s/ET_{c act} using AquaCrop had a very wide range, from 1% to 26%, likely due to the heavy dependency of E_s on CC (Equation (7)), i.e., whenever the model simulated high impacts of water stress and reduced CC, as for DI_Veg-GFill and the rain-fed treatments during 2011–2012, higher E_s/ET_{c act} values were estimated. Thus, differences between models relative to T_{c act} amounted to up to 40% when water stress occurred, with higher T_{c act} values being estimated by SIMDualKc (Table 3). During the late season, despite lower coverage of the soil due to leaf senescence, because watering events were small and infrequent, E_s/ET_{c act} increased slightly with SIMDualKc but to a higher value averaging 15% when using AquaCrop. Farahani et al. [32] also reported high E_s/ET_{c act} ratios with AquaCrop under water stress. Consequently, it could be concluded that AquaCrop tends to underestimate T_{c act} throughout the crop season, mainly under water deficit conditions.

Differences relative to the non-consumptive use terms, runoff, and deep percolation are notable, particularly for the 2012–2013 season (

Table 4. Water balance components computed with the SIMDualKc and AquaCrop models for all irrigation treatments and both crop seasons of 2011–2012 and 2012–2013.

Treatment	Model	P (mm)	I (mm)	ΔSWC (mm)	DP (mm)	RO (mm)	ETc act (mm)	Tc act (mm)	Es (mm)	Es/ETc act (%)
2011–2012
FI	SIMDualKc	821	354	16	266	207	718	606	112	16
	AquaCrop	821	354	23	245	266	688	574	114	17
DIGFill	SIMDualKc	676	288	37	190	132	679	577	102	15
	AquaCrop	676	288	−8	109	159	688	571	117	17
DIVeg	SIMDualKc	773	162	15	211	204	535	450	85	16
	AquaCrop	773	162	19	248	259	448	275	173	39
DIVeg-GFill	SIMDualKc	628	90	22	100	129	511	429	82	16
	AquaCrop	628	90	6	80	138	507	370	137	27
Rain-fed	SIMDualKc	821	0	17	98	205	535	457	78	15
	AquaCrop	821	0	5	89	217	520	384	136	26
2012–2013
FI	SIMDualKc	786	342	10	408	164	566	447	119	21
	AquaCrop	786	342	20	306	273	569	454	115	20
DIGFill	SIMDualKc	666	306	39	304	158	549	434	115	21
	AquaCrop	666	306	56	216	274	539	424	115	21
DIVeg	SIMDualKc	746	216	45	330	164	512	407	105	21
	AquaCrop	746	216	−2	159	274	527	403	124	24
DIVeg-GFill	SIMDualKc	668	306	39	318	149	546	437	109	20
	AquaCrop	668	306	30	180	257	567	451	116	20
Rain-fed	SIMDualKc	786	0	61	183	164	501	400	101	20
	AquaCrop	786	0	75	139	219	504	383	121	24

Notes: P is precipitation, I is net irrigation depths, ΔSWC is variation in stored soil water, DP is deep percolation, RO is runoff, ET_{c act} is actual crop evapotranspiration, T_{c act} is the actual crop transpiration, E_s is the soil evaporation.

). RO and DP, whose sum equals the difference between the water input and ET_{c act}, differ between models, with differences stemming from computational approaches as also observed by Pereira et al. [21]. The CN value used for RO computations was the same with both models but related computational processes are different [14,33], thus RO values are also different.

DP values computed with SIMDualKc were generally higher, up to 171 mm, than those estimated with AquaCrop (Table 4) due to differences in the computation of DP: SIMDualKc uses a parametric function (Liu et al. 2006) whose parameters a_D and b_D are calibrated, as per this application. In contrast, in AquaCrop, DP is estimated using a quasi-deterministic redistribution and drainage module based on the hydraulic characteristics of the soil [14] but does not use calibrated parameters. Possible deficiencies in that DP module were referred by Pereira et al. [21] and Iqbal et al. [56], as well as Farahani et al. [32] who compared computed with field observed DP. As analyzed by several authors (e.g., [57]), AquaCrop had not been tested for severe water stress conditions yet. Results herein relative to the soil water balance components and the insufficiencies in partition of ET_{c act} support the need for improving that model.

3.3. Yield Predictions

The available data on water use and transpiration, biomass, and yield covering four seasons, 2009–2010 to 2012–2013, were used to test the biomass and yield predictions by AquaCrop and the Stewart’s model combined with SIMDualKc (Stew-SIM). Yields of all treatments were significantly different as per an application of ANOVA (data not shown).

Yield predictions often show better results with the Stew-SIM combined approach relative to AquaCrop (

Table 5. Deviations between predicted and observed soybean final yield (kg·ha⁻¹) when using the SIMDualKc-Stewart’s approach and the AquaCrop model for all observed data.

Year	Irrig. Strategy	Observed *	SIMDualKc-Stewart			AquaCrop
			Predicted	Deviation		Predicted	Deviation
		(kg·ha−1)	(kg ha−1)	(kg·ha−1)	(%)	(kg·ha−1)	(kg·ha−1)	(%)
2009–2010	FI	4225 (±215)	4281	−56	−1	5179	−954	−23
	DIGFill	2107 (±748)	3490	−1383	−66	4270	−2163	−103
	Rain-fed	4209 (±91)	4278	−68	−2	5182	−973	−23
2010–2011	FI	6293 (±209)	6038	255	4	5089	1204	19
	DIVeg	4856 (±1324)	4830	26	1	4407	449	9
	DIVeg-GFill	4592 (±584)	4394	199	4	3626	966	21
	Rain-fed	4377 (±502)	3804	573	13	3684	693	16
2011–2012	FI	5368 (±133)	5456	−88	−2	5425	−57	−1
	DIGFill	4071 (±294)	5114	−1043	−26	5367	−1296	−32
	DIVeg	4597 (±178)	3620	977	21	2725	1872	41
	DIVeg-GFill	3491 (±228)	3370	121	3	3662	−171	−5
	Rain-fed	4493 (±105)	3705	788	18	3764	729	16
2012–2013	FI	5402 (±591)	5446	−44	−1	5287	115	2
	DIGFill	4605 (±556)	5227	−622	−14	4930	−325	−7
	DIVeg	4045 (±66)	4797	−752	−19	4768	−723	−18
	DIVeg-GFill	4069 (±87)	5276	−1206	−30	5269	−1200	−29
	Rain-fed	4721 (±495)	4683	38	1	4547	174	4

Note: * dried at 65 ± 5 °C; the standard deviation is presented between brackets.

). The Stew-SIM approach shows a tendency for slightly over-predicting yields (b₀ = 1.04), with relative deviations between predicted and simulated yields ranging from 1% to 66% (Table 5). AquaCrop results do not show any tendency for under- or over-estimation (b₀ = 0.99) but deviations vary in a wider range, from 1% to 103%. Deviations between observed and simulated yields using the Stew-SIM approach are in the range of those reported by Ma et al. [6] using the CROPGRO-Soybean and the hybrid RZWQM-CROPGRO-Soybean model, and by Banterng et al. [58] when using the CROPGRO-Soybean model.

The “goodness-of-fit” indicators relative to all yield predictions with AquaCrop were poor, with RMSE = 1.01 t ha⁻¹, NRMSE = 22.8%, and EF = −0.41. The negative EF indicates that the MSE is larger than the variance of observations, thus, modelling predictions are poor and there is no effective advantage in using this model. Nevertheless, results in the current study relative to AquaCrop applications are in the range of those reported by Mercau et al. [7] using CROPGRO-Soybean and Cera et al. [12] using SoySim. However, better results using AquaCrop for soybean were reported by Abi Saab et al. [15], Paredes et al. [16], and Battisti et al. [27] whose studies only considered small water stress levels. The above referred results are likely due to the previously discussed poor estimation of actual transpiration when water stress occurs. Katerji et al. [57] and Pereira et al. [21] also reported that AquaCrop biomass and yield predictions were poor under severe water stress because they were hampered by poor estimations of T_{c act}. Good predictions were, however, obtained with AquaCrop for vining pea [59], which was cultivated without water stress, thus confirming that the use of AquaCrop predictions is only recommended when severe water stress is not considered.

The “goodness-of-fit” indicators relative to yield predictions with the Stew-SIM combined approach were RMSE = 0.65 t·ha⁻¹, NRMSE = 14.5%, and EF = 0.43, which are much better than the indicators relative to the AquaCrop predictions. These RMSE and NRMSE values are in the range of those reported for other model applications, e.g., with the CROPGRO-Soybean model [8]. However, much lower NRMSE were reported when using DSSAT CSM CROPGRO-Soybean [5] and with the hybrid RZWQM-CROPGRO model for soybean [6]. Lower RMSE values were also reported by Setiyono et al. [9] when using the SoySim model in a comparative study using the models CROPGRO-Soybean, Sinclair-Soybean, and WOFOST. Results for these models [9] resulted in a much higher RMSE than the one obtained with the combined Stew-SIM approach. Therefore, the latter is adequate to predict yields aimed at assessing impacts of alternative irrigation scheduling strategies even when a severe stress is considered.

4. Conclusions

Experimental results relative to various deficit irrigation scheduling treatments confirm that the crop growth stage from flowering to grain filling is the most sensitive to water stress. However, the highest impacts of water stress were observed when deficits were imposed from the vegetative to the grain filling period.

Both SIMDualKc and AquaCrop models were successfully calibrated and validated for soybean using SWC data relative to all treatments and two soybean seasons. The accuracy for simulating the SWC dynamics along the crop seasons was better for SIMDualKc and lower for AquaCrop mainly for the treatments subjected to severe water stress. The water balance terms resulting from the application of both models were quite different, mainly due to different procedures for computing the daily actual basal crop coefficient and the evaporation coefficient, resulting in different values of T_{c act} and E_s. Computations of potential and actual K_cb in SIMDualKc follow the well-established FAO56 dual-K_c methodology while maximum and actual K_cb values in AquaCrop depend heavily on the fitted CC curve which only works well for non-stressed crops. Relative to E_s, there are large computational differences, also due to the strong dependency of K_e on the fitted CC curve in AquaCrop, while K_e in SIMDualKc is obtained after calibration of the parameters characterizing the evaporative top soil layer and considering the observed f_c fraction.

Differences between models are quite evident in terms of non-consumptive water use, RO and DP. Differences in RO, computed with the same CN, resulted from differences in the algorithms used for the calculations by the models. Relative to DP, the computation modules are very different: in AquaCrop a quasi-deterministic module is used but without calibration; on the contrary, a parametric function is used in SIMDualKc but after calibration of its parameters.

It can be concluded that the calibrated parameters of both SIMDualKc and AquaCrop may be further used for soybean in this region and that SIMDualKc performed more accurately in computing the soil water balance, mainly in estimating T_{c act}, thus proving to be more appropriate to support advising farmers on supplemental irrigation scheduling.

Both the AquaCrop model and the SIMDualKc-Stewart’s combined approach may be used for yield predictions. However, AquaCrop responded poorly when severe water stress was imposed, which relates to the above referred poor estimation of T_{c act} under those conditions. Thus, whenever the model fitting of CC is worse, the model poorly estimates T_{c act} and, as a consequence, biomass and yield are under-estimated. Results herein clearly identified the main weaknesses of AquaCrop, thus, the need for its further improvement for high water deficit situations. Contrarily, yield predictions with the Stew-SIM approach were good because T_{c act} was predicted accurately and the empirical yield response factor K_y was calibrated. Thus, that simple approach can be further used when devising supplemental irrigation strategies for soybean in Uruguay.

Based on the current study, the next step is to design supplemental irrigation strategies to cope with climate variability in line with previous studies [13,60] and to consider water productivity and economic farmers’ returns. Further research should also assess the usability of weather forecasts for supporting real time irrigation scheduling.