Simulating Spawning and Juvenile Rainbow Trout (Oncorhynchus mykiss) Habitat in Colorado River Based on High-Flow Effects
Abstract
:1. Introduction
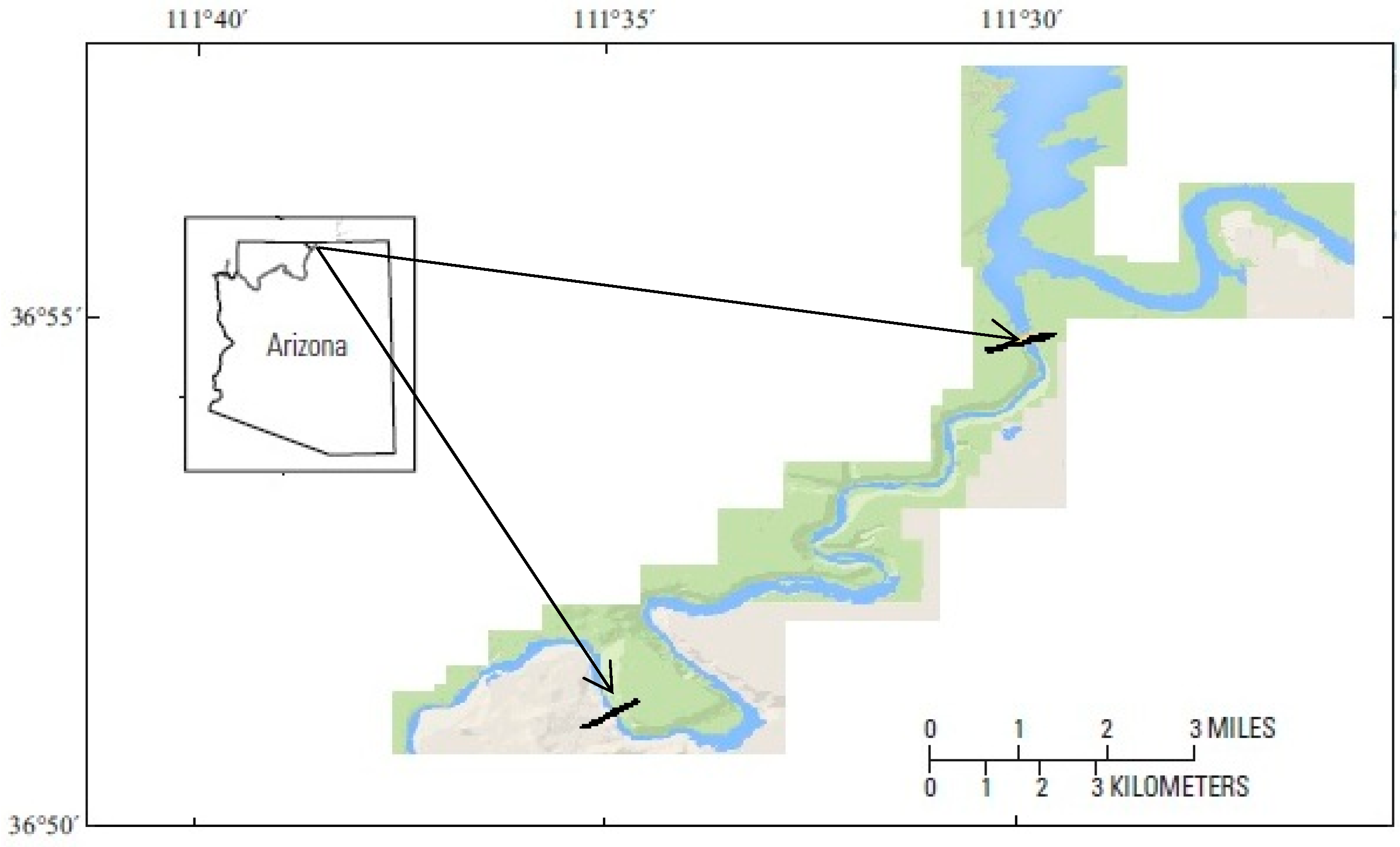
2. Study Areas and Mathematical
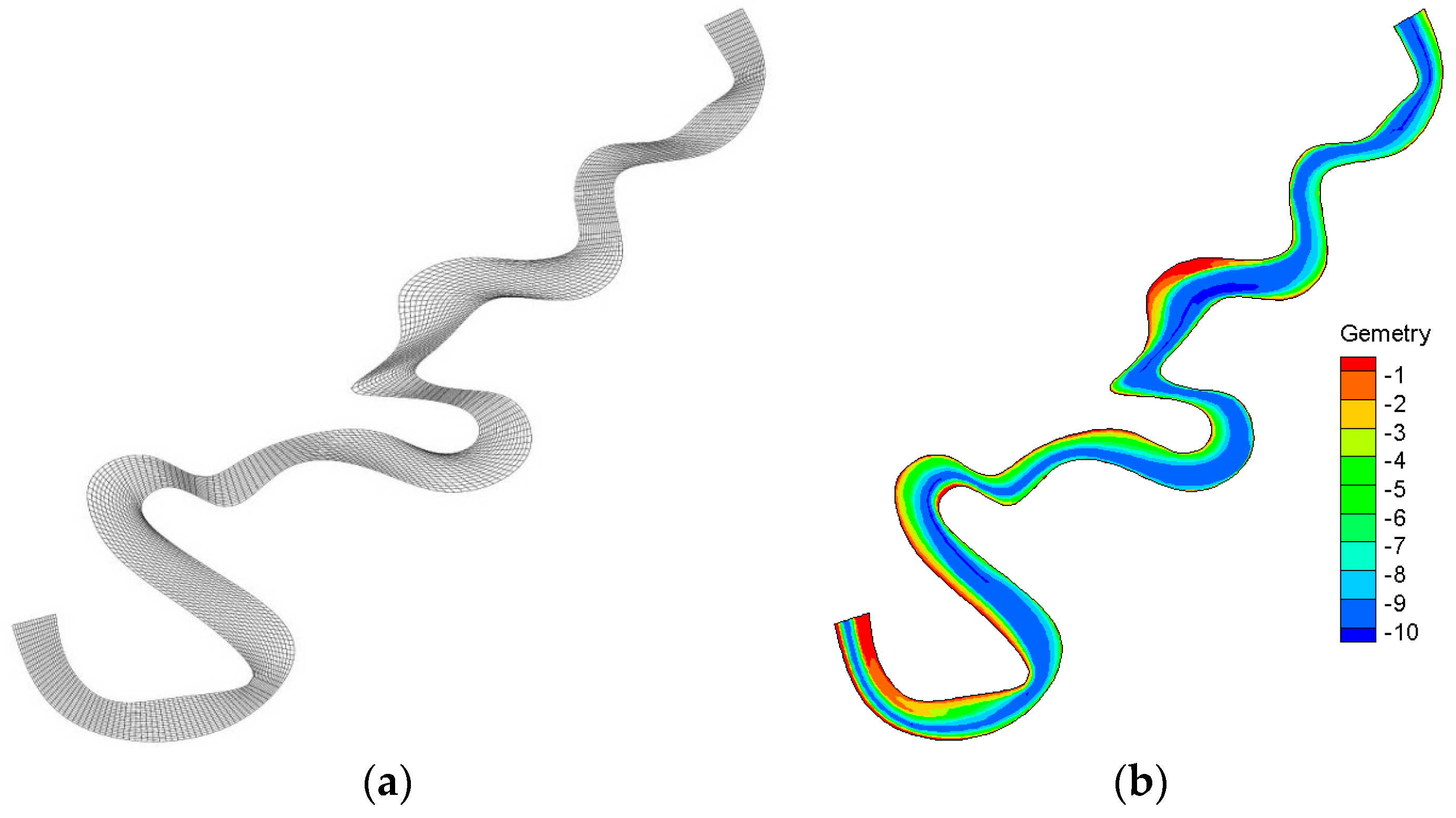
2.1. Study Areas and Mathematical Description of CFD Simulations
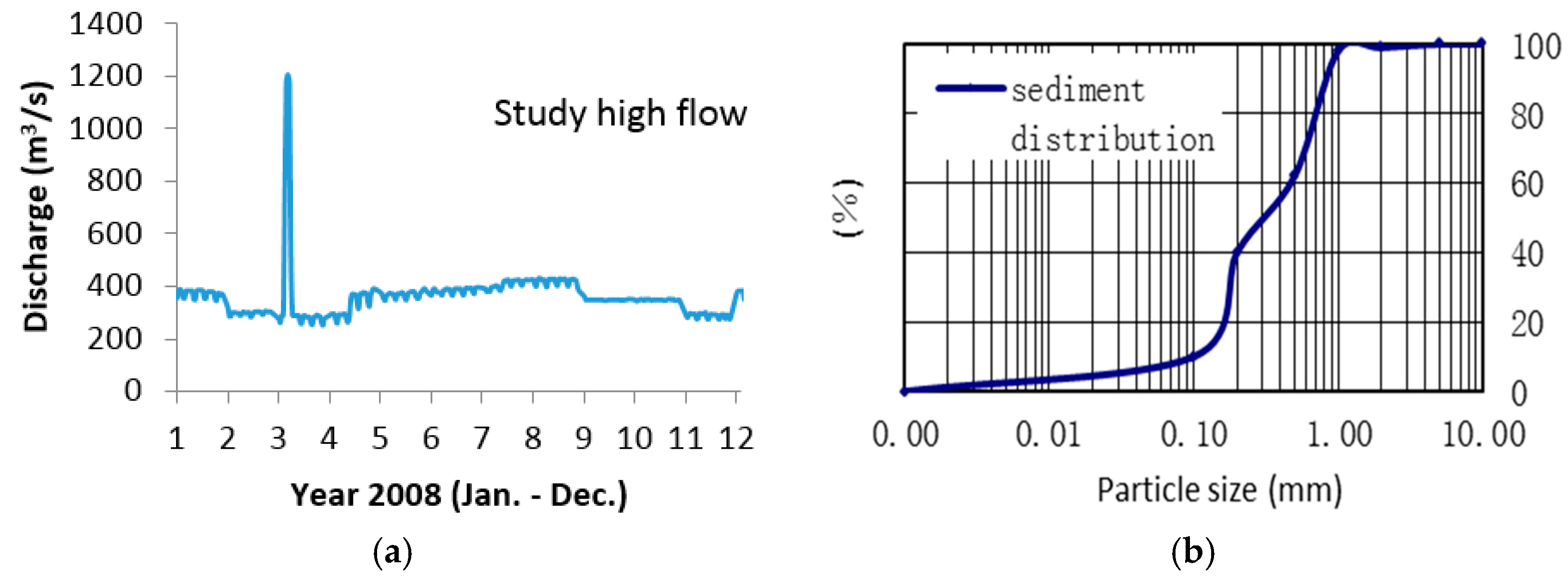
2.2. Sediment Transport
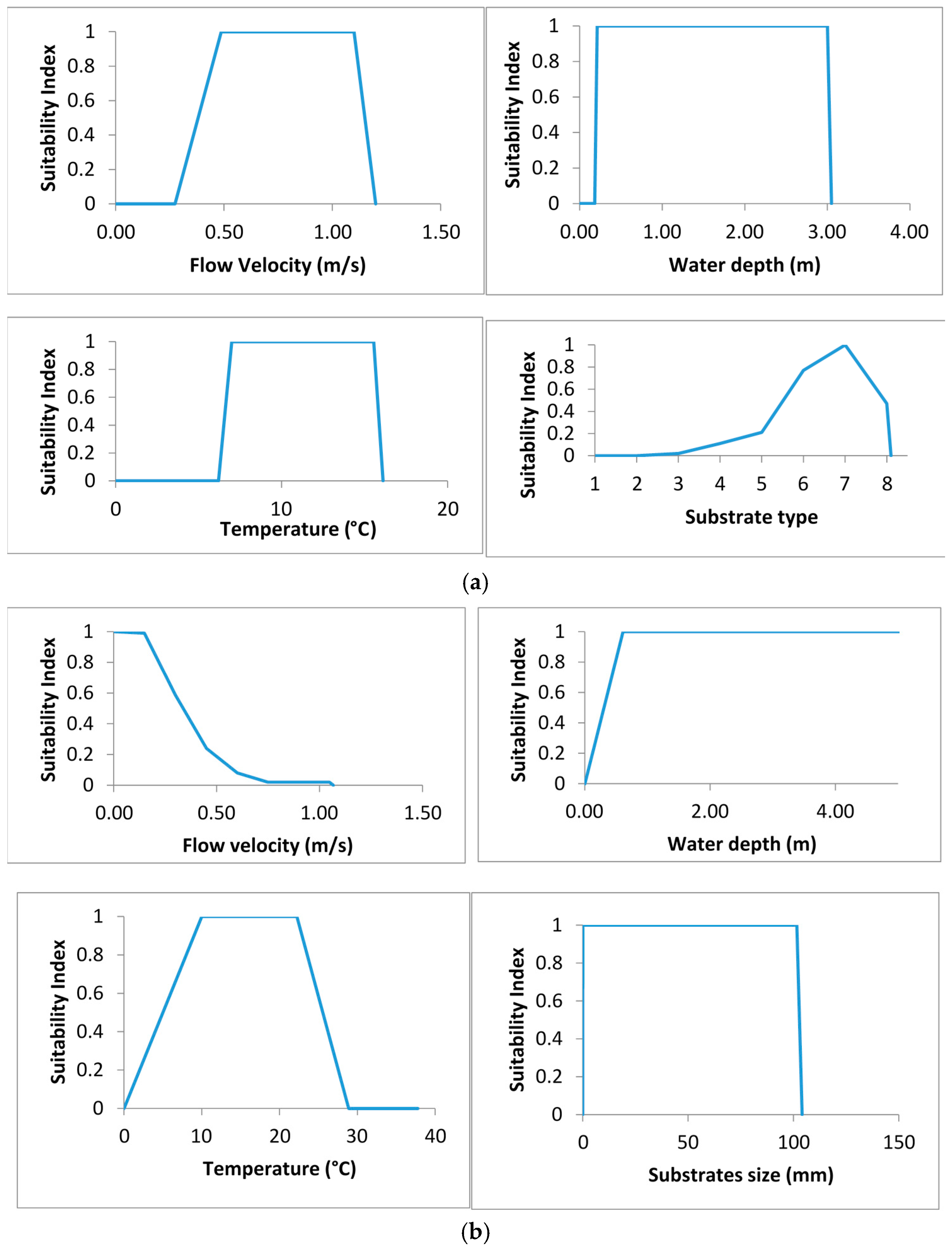
2.3. Habitat Construction
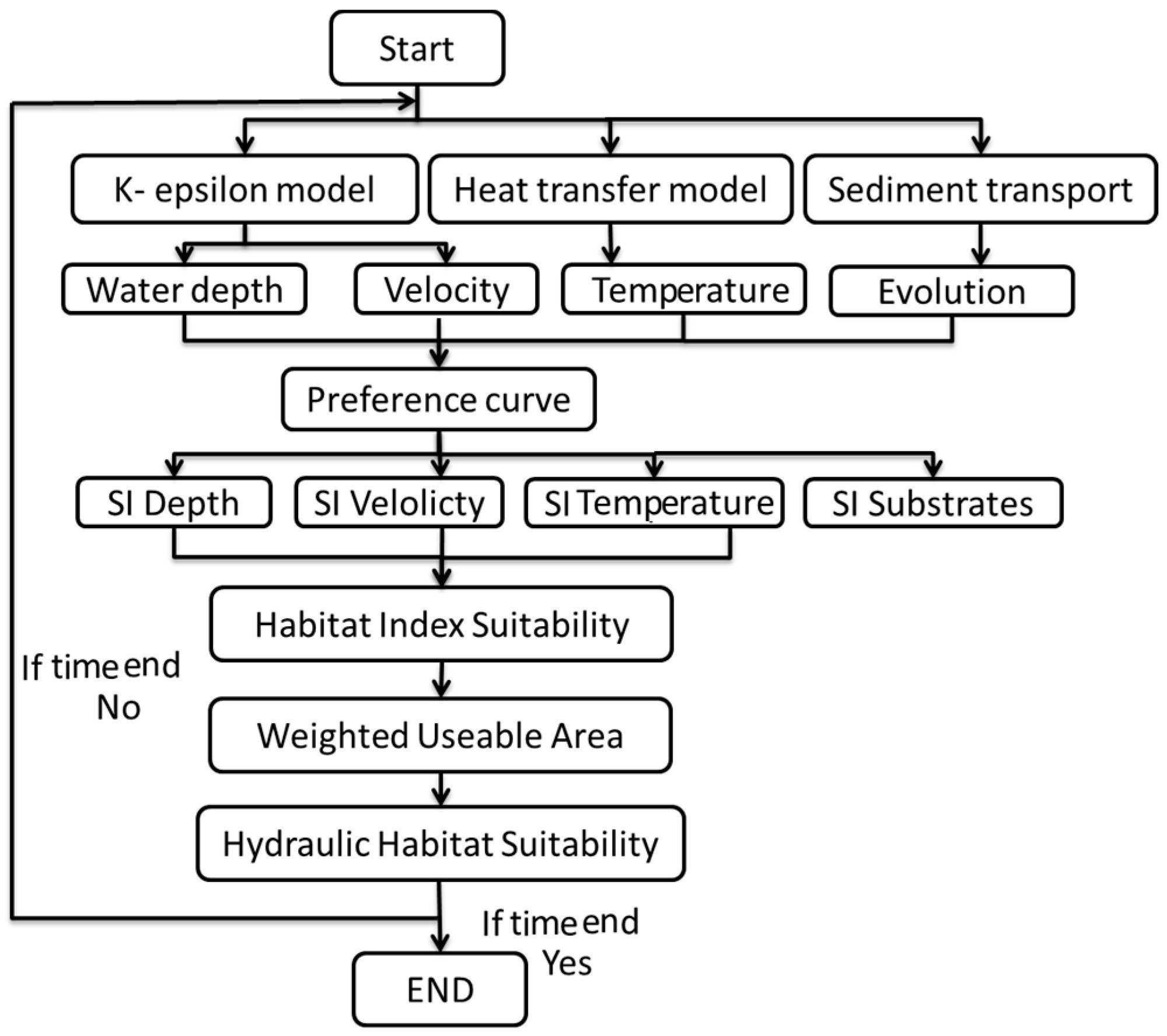
2.4. WUA and OSI Construction Procedure
3. Numerical Model Setup and Implementation
4. Results and Discussion
4.1. High Flow Effects on Velocity and Temperature
4.2. Hydrodynamic Simulation Results
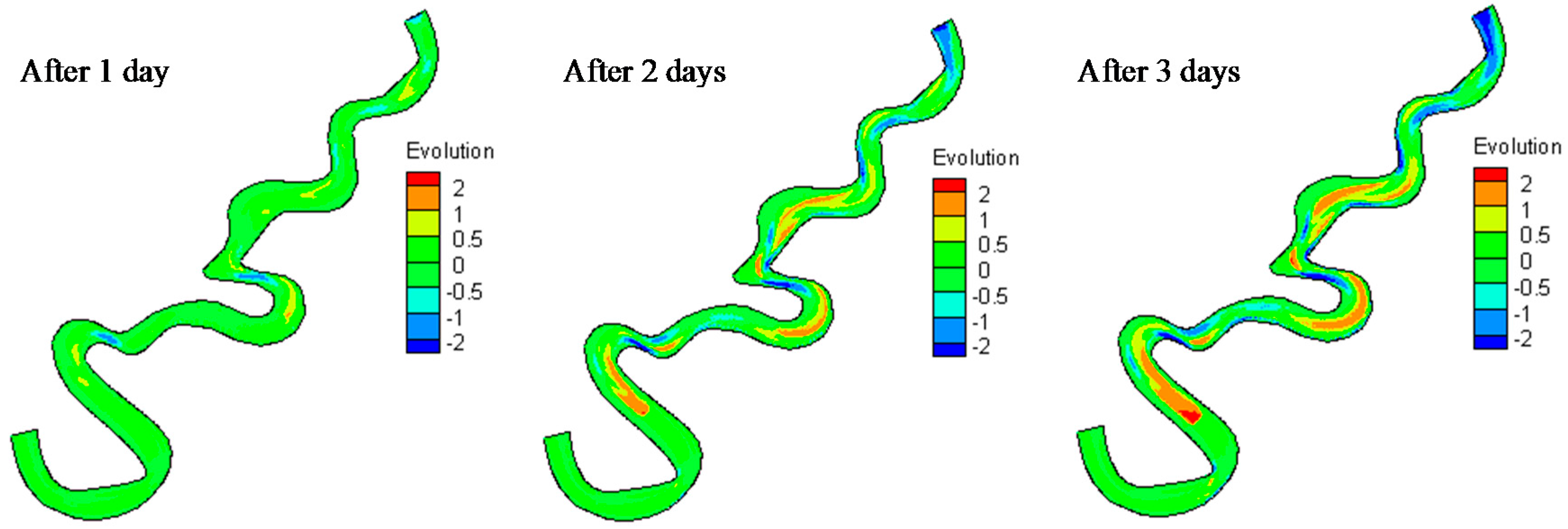
4.2.1. High Flow Effects on River Bed Elevation and Water Depth
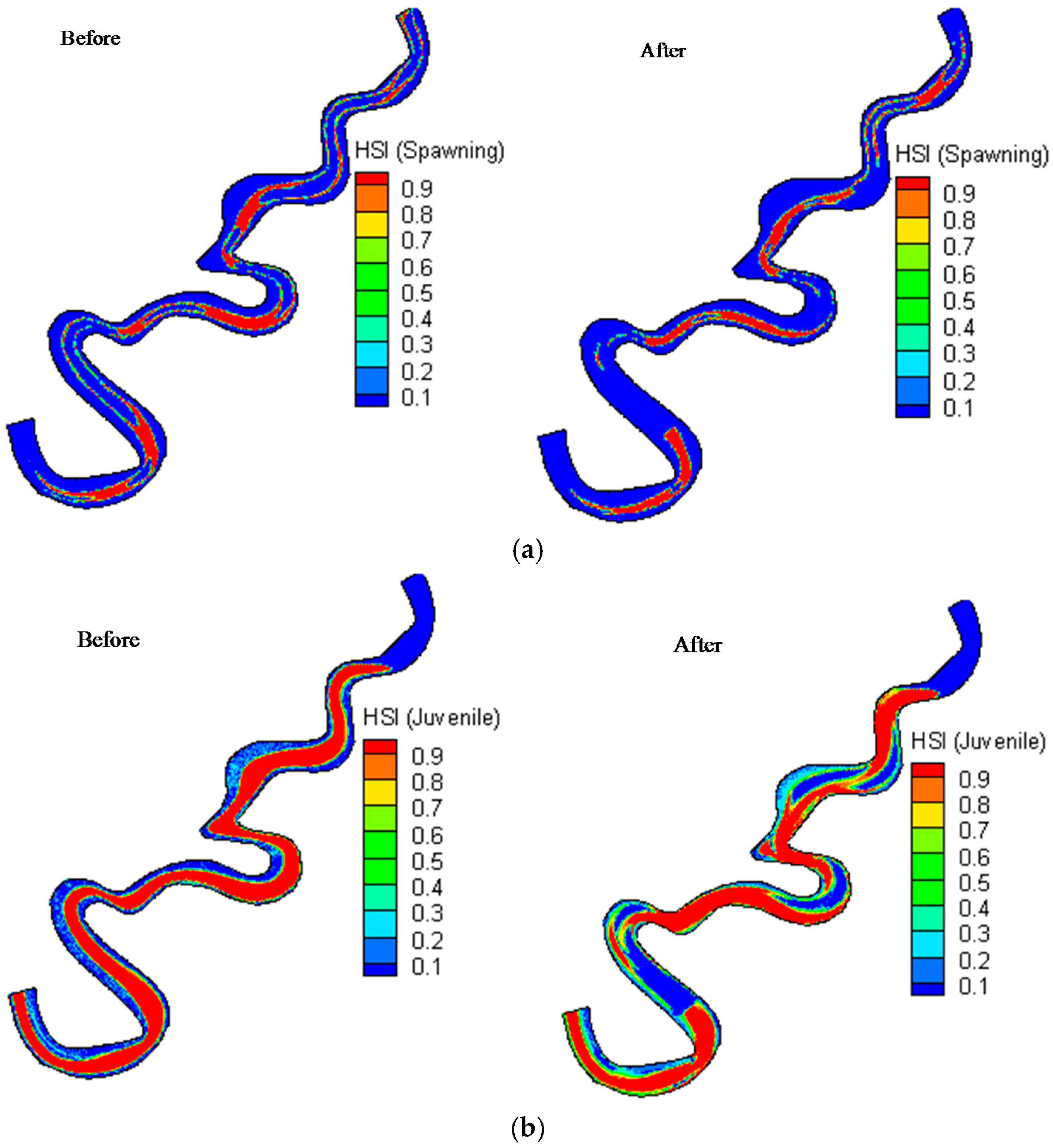
4.2.2. High Flow Effects on Spawning and Juvenile Rainbow Trout Habitat Suitability
4.3. Weighted Usable Area and Overall Usable Area Analyses
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| Ai | The area of the mesh i |
| Suspended load mass concentration at reference lever under equilibrium conditions | |
| CFD | Computational Fluid Dynamics |
| Suspended load concentration at reference lever | |
| Turbulence diffusivity scalar | |
| Non-dimensional skin friction number/shields number | |
| Critical shields value | |
| The eddy viscosity | |
| C | Chezy friction coefficient |
| d50 | Particle size parameter in 50 percent |
| fcor | The Coriolis parameter |
| g | Gravitational acceleration |
| Gr | Grashof number |
| h | Fluid column height |
| HFE | High Flow Effect |
| HSI | Habitat suitability index |
| M | The total number of grid mesh |
| OSI | Overall suitability index |
| P’ | The non-cohesive bed porosity |
| Qbs, Qbn | Bed-load flux |
| Re | Reynolds number |
| SIv, SId, SIs, SIt | Suitability index for velocity, water depth, substrates and temperature |
| t | Time |
| T | Temperature |
| U, V | Depth average velocity components in x and y directions respectively |
| WUA | Weighted usable areas |
| Z-Z0 | Water depth |
| η | Water surface elevation |
| τb | Bed shear stresses |
| τxx τxy τyx, τyy | Depth-average Reynolds (turbulent) stresses |
| ρs | Sediment density |
| ρw | Water density |
References
- Almeida, G.; Rodríguez, J. Integrating Sediment Dynamics into Physical Habitat Models. In Proceedings of the 18th World Imacs/ModSim Congress, Cairns, Australia, 13–17 July 2009; pp. 2258–2264.
- McDonald, R.; Nelson, J.; Paragamian, V.; Barton, G. Modeling the effect of flow and sediment transport on white sturgeon spawning habitat in the Kootenai River, Idaho. J. Hydraul. Eng. 2010, 136, 1077–1092. [Google Scholar] [CrossRef]
- Wang, Z.; Lee, J.H.; Xu, M. Eco-hydraulics and eco-sedimentation studies in china. J. Hydraul. Res. 2013, 51, 19–32. [Google Scholar] [CrossRef]
- Bunn, S.E.; Arthington, A.H. Basic principles and ecological consequences of altered flow regimes for aquatic biodiversity. Environ. Manag. 2002, 30, 492–507. [Google Scholar] [CrossRef]
- Ward, J.; Tockner, K.; Schiemer, F. Biodiversity of floodplain river ecosystems: Ecotones and connectivity. Regul. Rivers Res. Manag. 1999, 15, 125–139. [Google Scholar] [CrossRef]
- Ward, J.V.; Stanford, J. Ecological connectivity in alluvial river ecosystems and its disruption by flow regulation. Regul. Rivers Res. Manag. 1995, 11, 105–119. [Google Scholar] [CrossRef]
- Wang, Y.; Xia, Z. Assessing spawning ground hydraulic suitability for chinese sturgeon (acipenser sinensis) from horizontal mean vorticity in Yangtze River. Ecol. Model. 2009, 220, 1443–1448. [Google Scholar] [CrossRef]
- Yi, Y.; Wang, Z.; Yang, Z. Two-dimensional habitat modeling of Chinese sturgeon spawning sites. Ecol. Model. 2010, 221, 864–875. [Google Scholar] [CrossRef]
- Milhous, R.T.; Wegner, D.L.; Waddle, T. User’s Guide to the Physical Habitat Simulation System (Phabism); US Fish and Wildlife Service: Washington, DC, USA, 1984.
- Parasiewicz, P. Mesohabsim: A concept for application of instream flow models in river restoration planning. Fisheries 2001, 26, 6–13. [Google Scholar] [CrossRef]
- Parasiewicz, P. Upscaling: Integrating habitat model into river management. Can. Water Resour. J. 2003, 28, 283–299. [Google Scholar] [CrossRef]
- Parasiewicz, P. The mesohabsim model revisited. River Res. Appl. 2007, 23, 893–903. [Google Scholar] [CrossRef]
- Noack, M. Modelling Approach for Interstitial Sediment Dynamics and Reproduction of Gravel-spawning Fish. Ph.D. Thesis, Universität Stuttgart, Stuttgart, Germany, 2012. [Google Scholar]
- Lessard, J.L.; Hayes, D.B. Effects of elevated water temperature on fish and macroinvertebrate communities below small dams. River Res. Appl. 2003, 19, 721–732. [Google Scholar] [CrossRef]
- Armstrong, J.; Kemp, P.; Kennedy, G.; Ladle, M.; Milner, N. Habitat requirements of atlantic salmon and brown trout in rivers and streams. Fisheries Res. 2003, 62, 143–170. [Google Scholar] [CrossRef]
- Bovee, K.D. A Guide to Stream Habitat Analysis Using the Instream Flow Incremental Methodology. Ifip No. 12; US Fish and Wildlife Service: Fort Collins, CO, USA, 1982.
- Bovee, K.D. Development and Evaluation of Habitat Suitability Criteria for Use in the Instream Flow Incremental Methodology; USDI Fish and Wildlife Service: Washington, DC, USA, 1986.
- Gard, M. Comparison of spawning habitat predictions of phabsim and river2d models. Int. J. River Basin Manag. 2009, 7, 55–71. [Google Scholar] [CrossRef]
- Gard, M. Response to Williams (2010) on Gard (2009): Comparison of spawning habitat predictions of phabsim and river2d models. Int. J. River Basin Manag. 2010, 8, 121–125. [Google Scholar] [CrossRef]
- Steffler, P.; Blackburn, J. River 2D Two-Dimensional Depth Averaged Model of River Hydrodynamics and Fish Habitat Introduction to Depth Averaged Modeling and User’s Manual; University of Albert: Edmonton, AB, Canada, 2002. [Google Scholar]
- Voichick, N.; Wright, S.A. Water-Temperature Data for the Colorado River and Tributaries between Glen Canyon Dam and Spencer Canyon, Northern Arizona, 1988–2005; U.S. Geological Survey Data Series 251; U.S. Geological Survey: Reston, VA, USA, 2007.
- Vernieu, W.S. Effects of the 2008 High-flow Experiment on Water Quality in Lake Powell and Glen Canyon Dam Releases, Utah-Arizona; 2331-1258; US Geological Survey: Reston, VA, USA, 2010.
- Makinster, A.S.; Persons, W.R.; Avery, L.A. Status and Trends of the Rainbow Trout Population in the Lees Ferry Reach of the Colorado River Downstream from Glen Canyon Dam, Arizona, 1991–2009; 2328-0328; US Geological Survey: Reston, VA, USA, 2011.
- Makinster, A.S.; Persons, W.R.; Avery, L.A.; Bunch, A.J. Colorado River Fish Monitoring in Grand Canyon, Arizona; 2000 to 2009 Summary; 2331-1258; US Geological Survey: Reston, VA, USA, 2010.
- Yao, W.; Rutschmann, P. Three high flow experiment releases from glen canyon dam on rainbow trout and flannelmouth sucker habitat in colorado river. Ecol. Eng. 2015, 75, 278–290. [Google Scholar] [CrossRef]
- Yao, W.; Rutschmann, P.; Bamal, S. Modeling of river velocity, temperature, bed deformation and its effects on rainbow trout (Oncorhynchus mykiss) habitat in lees ferry, colorado river. Int. J. Environ. Res. 2014, 8, 887–896. [Google Scholar]
- Huang, P.; Bardina, J.; Coakley, T. Turbulence modeling validation, testing, and development. NASA Tech. Memo. 1997, 110446. [Google Scholar]
- Launder, B.E.; Spalding, D.B. Lectures in Mathematical Models of Turbulence; Academic Press: London, UK, 1972. [Google Scholar]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Tominaga, Y.; Stathopoulos, T. Turbulent Schmidt numbers for CFD analysis with various types of flowfield. Atmo. Environ. 2007, 41, 8091–8099. [Google Scholar] [CrossRef]
- Coleman, S.; Nikora, V. Exner equation: A continuum approximation of a discrete granular system. Water Resour. Res. 2009, 45. [Google Scholar] [CrossRef]
- Ackers, P.; White, W.R. Sediment transport: New approach and analysis. J. Hydraul. Div. 1973, 99, 2041–2060. [Google Scholar]
- Meyer-Peter, E.; Müller, R. Formulas for bed-load transport. In Proceedings of the 2nd Meeting of the International Association for Hydraulic Structures Research, Stockholm, Sweden, 7–9 June 1948; pp. 39–64.
- Van Rijn, L.C. Principles of Sediment Transport in Rivers, Estuaries and Coastal Seas; Aqua publications: Amsterdam, The Netherlands, 1993; Volume 1006. [Google Scholar]
- Wu, W.; Rodi, W.; Wenka, T. 3d numerical modeling of flow and sediment transport in open channels. J. Hydraul. Engin. 2000, 126, 4–15. [Google Scholar] [CrossRef]
- Jowett, I.G.; Davey, A.J. A comparison of composite habitat suitability indices and generalized additive models of invertebrate abundance and fish presence–habitat availability. Trans. Am. Fish. Soc. 2007, 136, 428–444. [Google Scholar] [CrossRef]
- Lambert, T.R.; Hanson, D.F. Development of habitat suitability criteria for trout in small streams. River Res. Appl. 1989, 3, 291–303. [Google Scholar] [CrossRef]
- Matthews, K.; Berg, N. Rainbow trout responses to water temperature and dissolved oxygen stress in two Southern California stream pools. J. Fish Biol. 1997, 50, 50–67. [Google Scholar] [CrossRef]
- Milhous, R.T.; Updike, M.A.; Schneider, D.M. Physical Habitat Simulation System Reference Manual: Version II; US Fish and Wildlife Service: Washington, DC, USA, 1989.
- Moir, H.; Gibbins, C.N.; Soulsby, C.; Youngson, A. Phabsim modelling of Atlantic salmon spawning habitat in an upland stream: Testing the influence of habitat suitability indices on model output. River Res. Appl. 2005, 21, 1021–1034. [Google Scholar] [CrossRef]
- Mouton, A.M.; Schneider, M.; Depestele, J.; Goethals, P.L.; De Pauw, N. Fish habitat modelling as a tool for river management. Ecol. Eng. 2007, 29, 305–315. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Peric, M.; Leonard, A. Computational Methods for Fluid Dynamics (Vol. 3); Springer: Berlin, Germany, 1996. [Google Scholar]
- Patankar, S. Numerical Heat Transfer and Fluid Flow; CRC press: New York, NY, USA, 1980. [Google Scholar]
- Yao, W.; Bamal, S.; Rutschmann, P. Simulating High-Flow Effects (HFE) on River Deformation and Rainbow Trout (Oncorhynchus mykiss) Habitat; CRC Press: New York, NY, USA, 2014; pp. 2471–2476. [Google Scholar]
- Yao, W.W.; Kumar, V.; Rutschmann, P. Simulating Dam Effects on River Deformation and Rainbow Trout (Oncorhynchus mykiss) Population Number; CRC press: New York, NY, USA, 2014; pp. 2477–2483. [Google Scholar]
- Graf, J.B. Measured and predicted velocity and longitudinal dispersion at steai) y and unsteady flow, colorado river, glen canyon dam to lake mead. JAWRA J. Am. Water Resour. Assoc. 1995, 31, 265–281. [Google Scholar] [CrossRef]
- Korman, J.; Kaplinski, M.; Melis, T.S. Effects of High-Flow Experiments from Glen Canyon Dam on Abundance, Growth, and Survival Rates of Early Life Stages of Rainbow Trout in the Lees Ferry Reach of the Colorado River; 2331-1258; US Geological Survey: Reston, VA, USA, 2010.
- Rosi-Marshall, E.J.; Kennedy, T.A.; Kincaid, D.W.; Cross, W.F.; Kelly, H.A.; Behn, K.A.; White, T.; Hall, R.O., Jr.; Baxter, C.V. Short-Term Effects of the 2008 High-Flow Experiment on Macroinvertebrates in Colorado River Below Glen Canyon Dam, Arizona; 2331-1258; US Geological Survey: Reston, VA, USA, 2010.
- Wright, S.A.; Anderson, C.R.; Voichick, N. A simplified water temperature model for the colorado river below glen canyon dam. River Res. Appl. 2009, 25, 675–686. [Google Scholar] [CrossRef]
- Yao, W. Application of the Ecohydraulic Model on Hydraulic and Water Resources Engineering. Ph.D. Thesis, Technische Universität München, München, Germany, 2016. [Google Scholar]

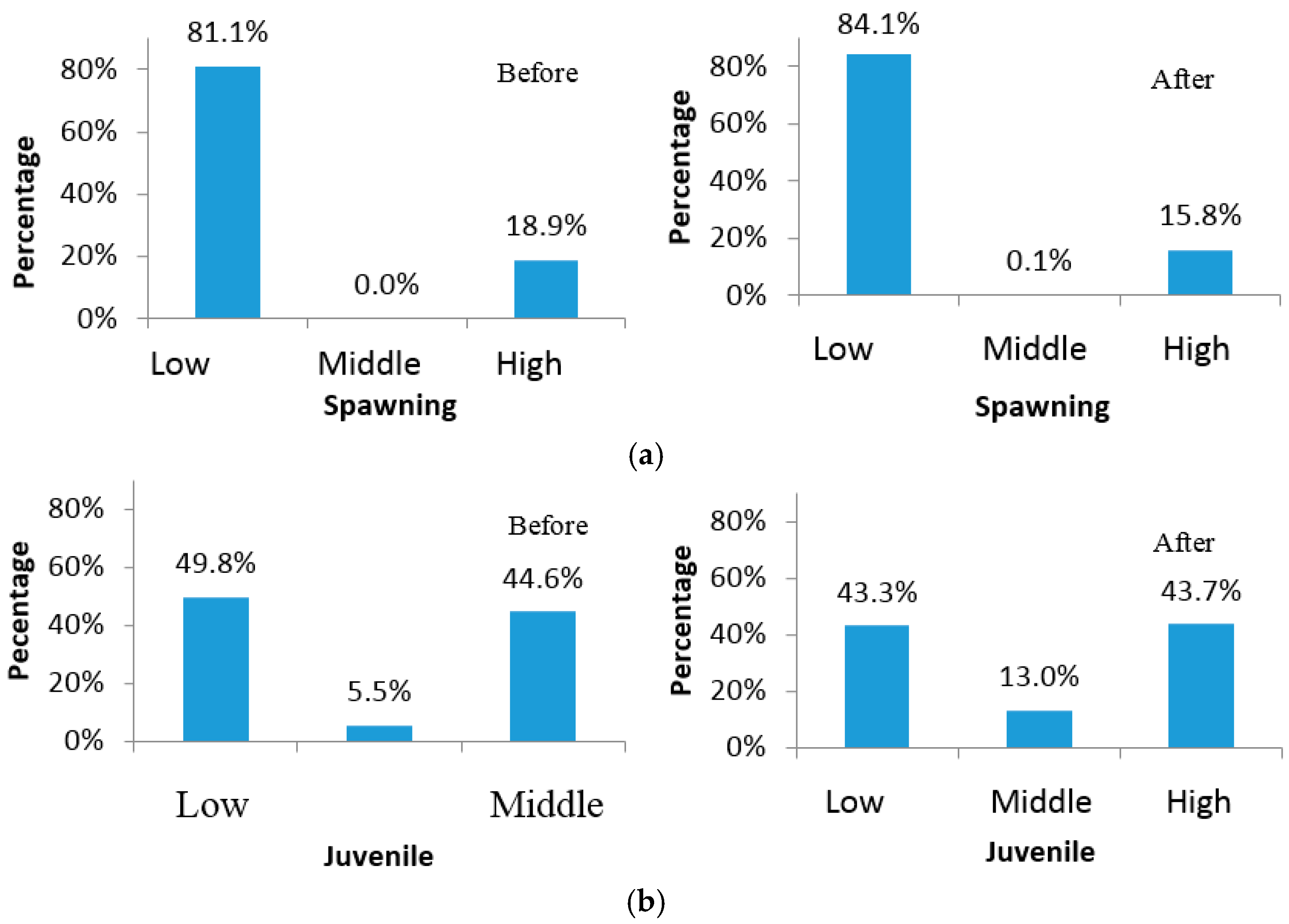
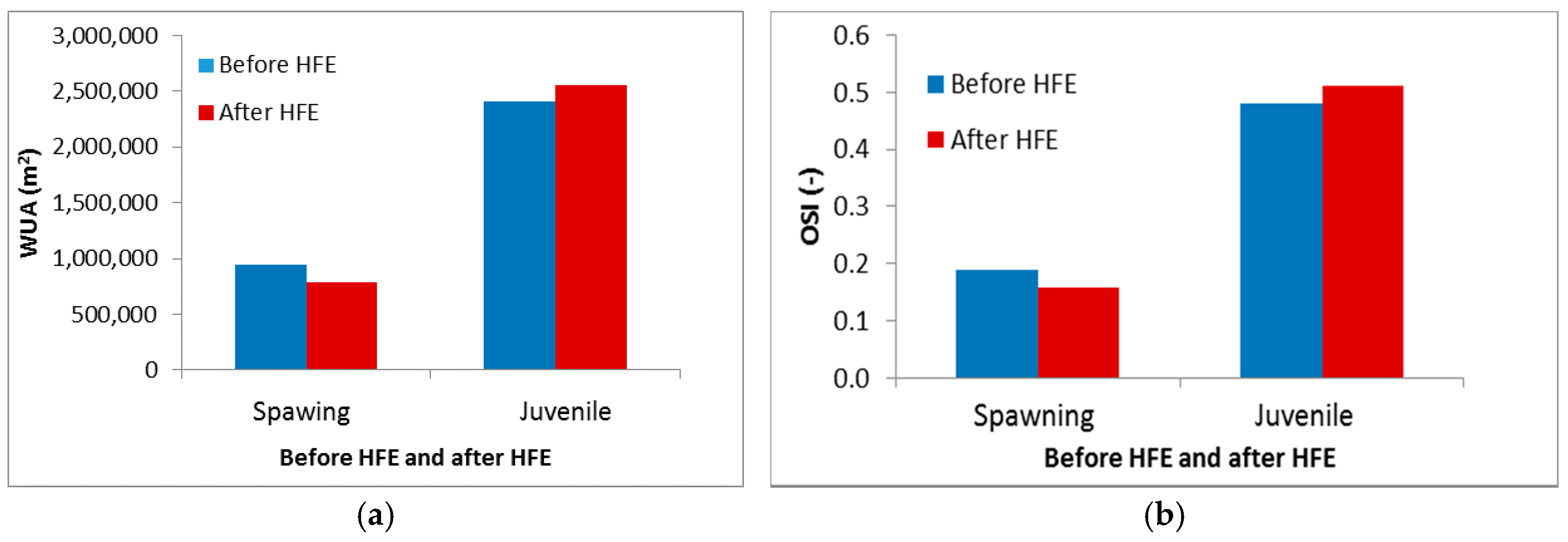
| HSI | High | 0.7–1.0 |
| Middle | 0.3–0.7 | |
| Low | 0–0.3 |
| Habitat Category | Spawning | Juvenile | ||
|---|---|---|---|---|
| WUA | Before | High | 9.46 × 105 | 2.23 × 106 |
| Middle | 1.03 × 103 | 2.75 × 105 | ||
| Low | 4.05 × 106 | 2.49 × 106 | ||
| After | High | 7.90 × 105 | 2.19 × 106 | |
| Middle | 3.10 × 103 | 6.51 × 105 | ||
| Low | 4.21 × 106 | 2.16 × 106 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, W.; Liu, H.; Chen, Y.; Zhang, W.; Zhong, Y.; Fan, H.; Li, L.; Bamal, S. Simulating Spawning and Juvenile Rainbow Trout (Oncorhynchus mykiss) Habitat in Colorado River Based on High-Flow Effects. Water 2017, 9, 150. https://doi.org/10.3390/w9020150
Yao W, Liu H, Chen Y, Zhang W, Zhong Y, Fan H, Li L, Bamal S. Simulating Spawning and Juvenile Rainbow Trout (Oncorhynchus mykiss) Habitat in Colorado River Based on High-Flow Effects. Water. 2017; 9(2):150. https://doi.org/10.3390/w9020150
Chicago/Turabian StyleYao, Weiwei, Huaxian Liu, Yuansheng Chen, Wenyi Zhang, Yu Zhong, Haiyan Fan, Linkai Li, and Sudeep Bamal. 2017. "Simulating Spawning and Juvenile Rainbow Trout (Oncorhynchus mykiss) Habitat in Colorado River Based on High-Flow Effects" Water 9, no. 2: 150. https://doi.org/10.3390/w9020150
APA StyleYao, W., Liu, H., Chen, Y., Zhang, W., Zhong, Y., Fan, H., Li, L., & Bamal, S. (2017). Simulating Spawning and Juvenile Rainbow Trout (Oncorhynchus mykiss) Habitat in Colorado River Based on High-Flow Effects. Water, 9(2), 150. https://doi.org/10.3390/w9020150






