Experimental Study of Sediment Incipient Velocity and Scouring in Submarine Cable Burial Areas
Abstract
1. Introduction
2. Materials and Methods
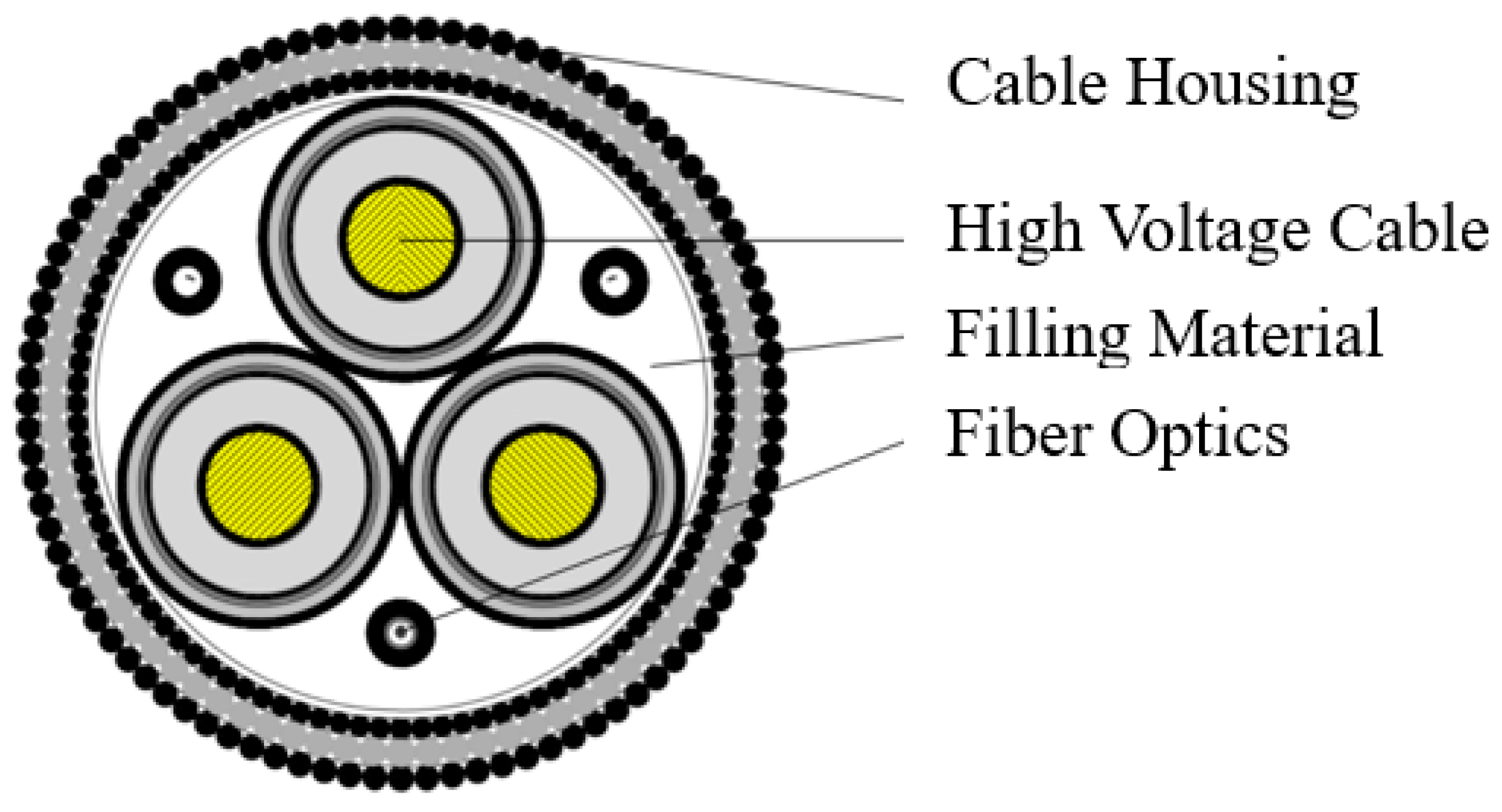
2.1. Simulated Submarine Cable
2.2. Experimental Equipment and Instruments
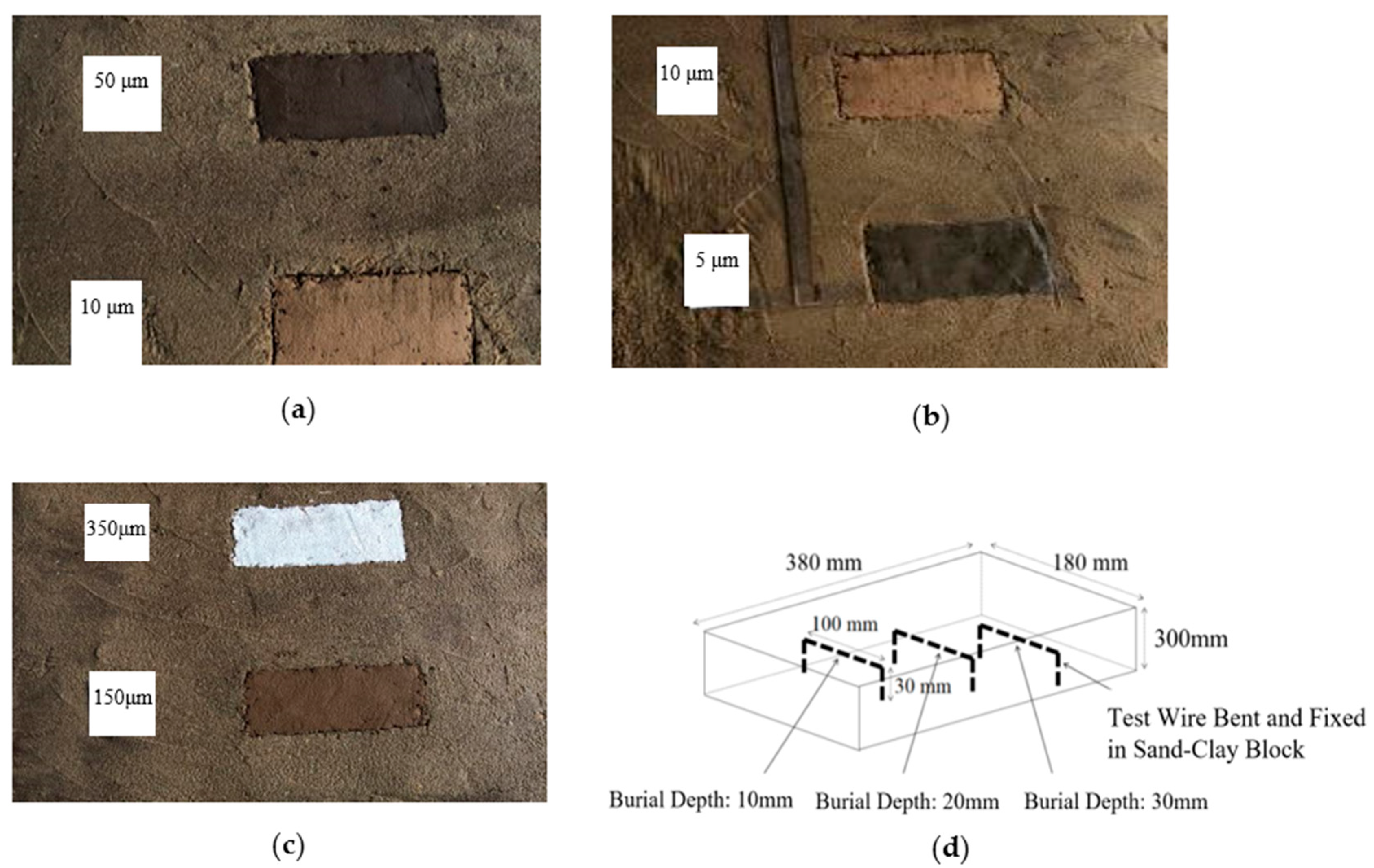
2.3. Model Design
2.4. Experimental Procedures
3. Experimental Results and Discussion
3.1. Sediment Incipient Velocity Formulas
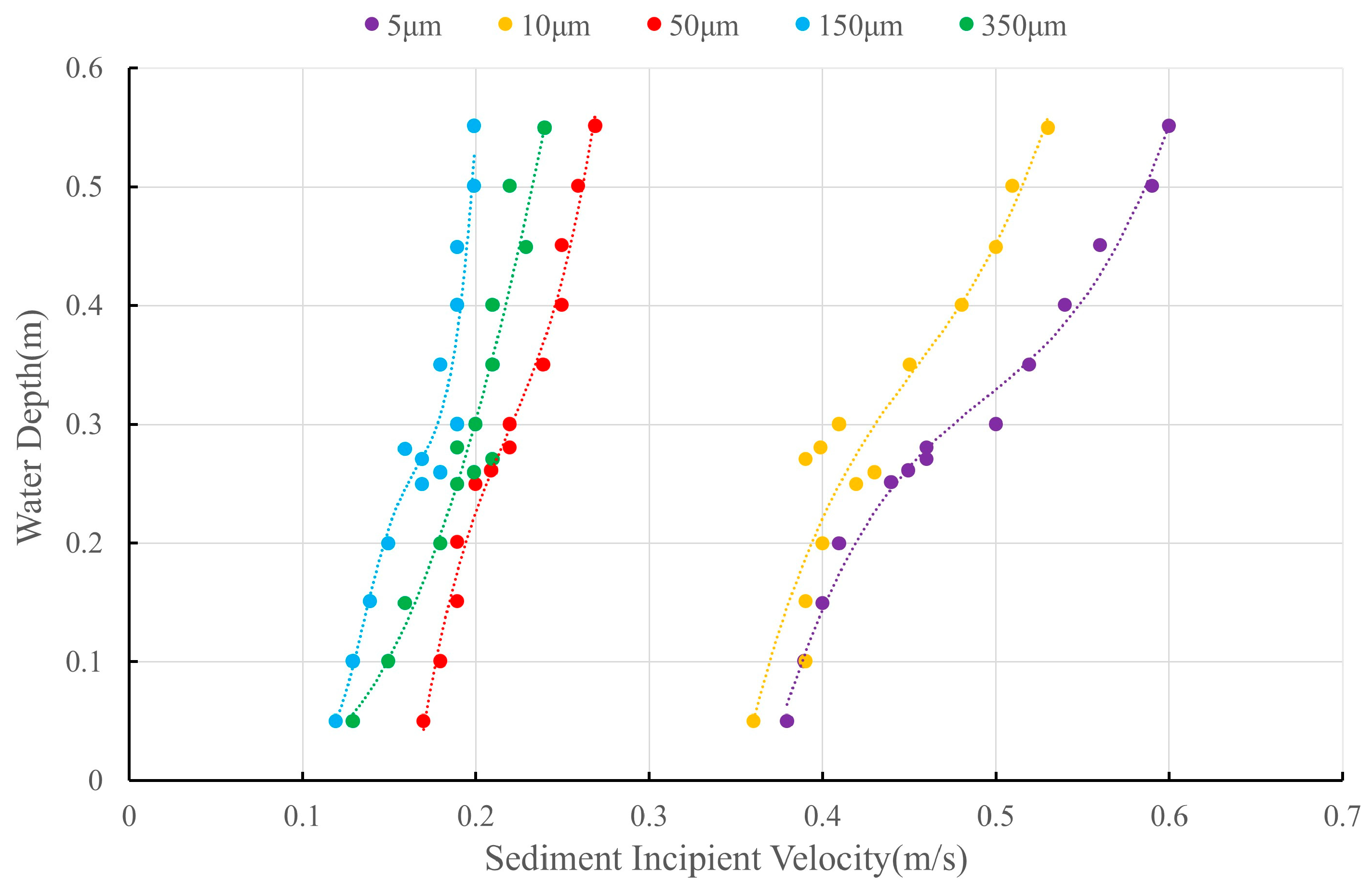
3.2. Experimental Results of Sediment Incipient Velocity
- 5 μm sediment: Most sensitive to depth changes, with a 0.19 m/s increase between 0.2 and 0.55 m.
- 10 μm sediment: Anomalous velocities at intermediate depths (0.26–0.28 m), suggesting experimental variability.
- 50 μm sediment: Marked velocity differences from the 10 μm group, indicating critical transitional behavior.
- 150 μm sediment: Minimal velocity variation (0.08 m/s range), lowest average velocity (0.12 m/s at 0.05 m).
- 350 μm sediment: Intermediate velocities between the 50 μm and 150 μm groups.
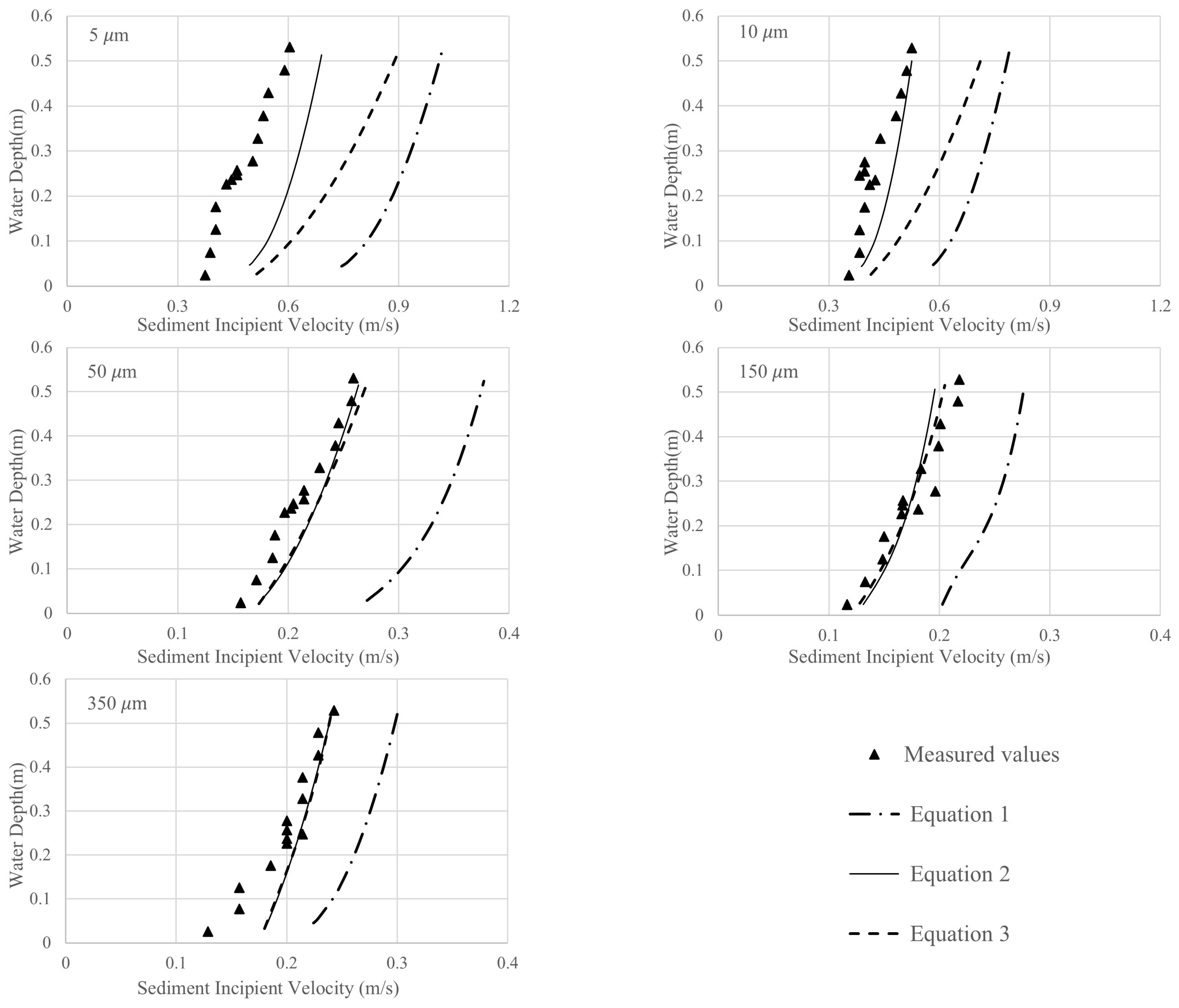
3.3. Modification of Sediment Incipient Velocity Formula
- For median sediment particle sizes ≥ 50 μm, the results are as follows:
- For median sediment particle sizes < 50 μm, the results are as follows:
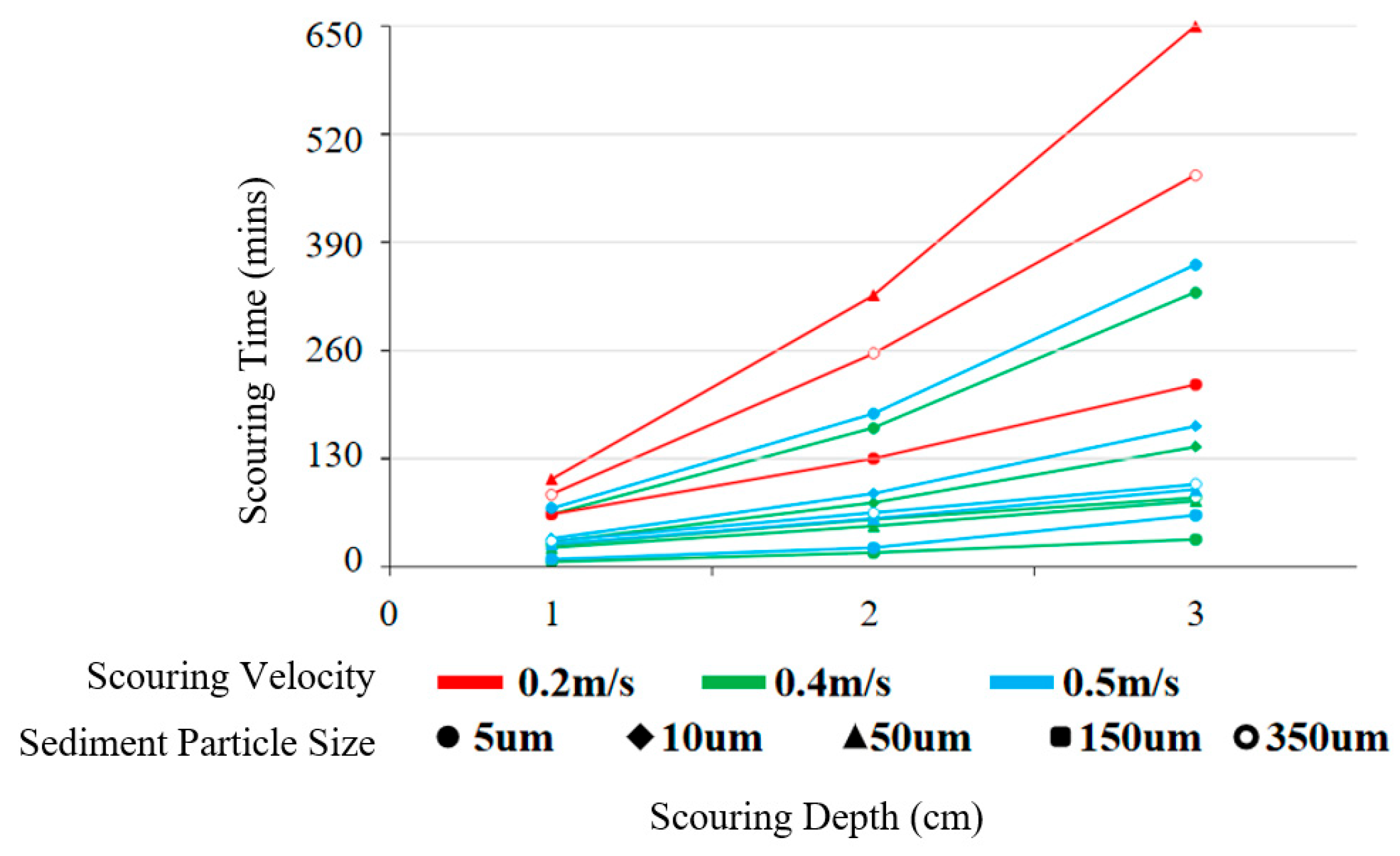
3.4. Scour Duration Test Results and Analysis
3.5. Scour Depth Test Results and Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, M.; Cheng, L.; Zang, Z. Experimental and Numerical Investigation of Local Scour around a Submerged Vertical Circular Cylinder in Steady Currents. Coast. Eng. 2010, 57, 709–721. [Google Scholar] [CrossRef]
- Zhang, M.; Huang, Y.; Bao, Y. The Mechanism of Shallow Submarine Landslides Triggered by Storm Surge. Nat. Hazards 2016, 81, 1373–1383. [Google Scholar] [CrossRef]
- Yang, Q.H.; Yang, Q.; Zhang, Y.Q. Numerical Investigation of the Flow-Field Characteristics around the Suspended Pipeline under Oblique Flows. Water Supply 2022, 22, 4361–4372. [Google Scholar] [CrossRef]
- Zhao, X.F.; Ba, Q.; Li, L.; Gong, P.; Ou, J.P. A Three-Index Estimator Based on Active Thermometry and a Novel Monitoring System of Scour under Submarine Pipelines. Sens. Actuators A Phys. 2012, 183, 115–122. [Google Scholar] [CrossRef]
- Lee, Y.S.; Kim, S.; Yu, Y. A Study on the Selection of Target Ship for the Protection of Submarine Power Cable. J. Korean Soc. Mar. Environ. Saf. 2018, 24, 662–669. [Google Scholar] [CrossRef]
- Wargo, R.; Davenport, T. Protecting Submarine Cables from Competing Uses. In Submarine Cables; Brill Nijhoff: Leiden, The Netherlands, 2014; pp. 255–279. [Google Scholar]
- Yoon, H.S.; Na, W.B. Anchor Drop Tests for a Submarine Power-Cable Protector. Mar. Technol. Soc. J. 2013, 47, 72–80. [Google Scholar] [CrossRef]
- Matsumoto, H.; Araki, E.; Kimura, T. Detection of Hydroacoustic Signals on a Fiber-Optic Submarine Cable. Sci. Rep. 2021, 11, 2797. [Google Scholar] [CrossRef]
- Dou, G.R. On the Starting Velocity of Sediment. J. Hydraul. Eng. 1960, 4, 44–60. (In Chinese) [Google Scholar]
- Zhang, R.J.; Xie, J.H.; Chen, W.B. River Dynamics; China Industry Press: Beijing, China, 1961. (In Chinese) [Google Scholar]
- Sun, Z.L.; Xie, J.H.; Duan, W.Z.; Xie, B.L. Research on the Starting Law of Non-Uniform Sand Grading. J. Water Resour. 1997, 10, 26–33. (In Chinese) [Google Scholar]
- Dodaro, G.; Tafarojnoruz, A.; Sciortino, G.; Adduce, C.; Calomino, F.; Gaudio, R. Modified Einstein Sediment Transport Method to Simulate the Local Scour Evolution Downstream of a Rigid Bed. J. Hydraul. Eng. 2016, 142, 04016041. [Google Scholar] [CrossRef]
- Tafarojnoruz, A.; Sharafati, A. New Formulations for Prediction of Velocity at Limit of Deposition in Storm Sewers Based on a Stochastic Technique. Water Sci. Technol. 2020, 81, 2634–2649. [Google Scholar] [CrossRef] [PubMed]
- Sharafati, A.; Tafarojnoruz, A.; Yaseen, Z.M. New Stochastic Modeling Strategy on the Prediction Enhancement of Pier Scour Depth in Cohesive Bed Materials. J. Hydroinform. 2020, 22, 457–472. [Google Scholar] [CrossRef]
- Guo, Z.; Zhou, W.; Zhu, C.; Yuan, F.; Rui, S. Numerical Simulations of Wave-Induced Soil Erosion in Silty Sand Seabeds. J. Mar. Sci. Eng. 2019, 7, 52. [Google Scholar] [CrossRef]
- Pike, S.M.; van Zyl, J.E.; Clayton, C.R.I. Scouring Damage to Buried Pipes Caused by Leakage Jets: Experimental Study. J. Pipeline Syst. Eng. Pract. 2018, 9, 04018020. [Google Scholar] [CrossRef]
- Fredsøe, J.; Hansen, E.A. Lift Forces on Pipelines in Steady Flow. J. Waterw. Port Coast. Ocean Eng. 1987, 113, 139–155. [Google Scholar] [CrossRef]
- Chiew, Y.M. Prediction of Maximum Scour Depth at Submarine Pipelines. J. Hydraul. Eng. 1991, 117, 452–466. [Google Scholar] [CrossRef]
- Hossein Kazeminezhad, M.; Yeganeh-Bakhtiary, A.; Etemad-Shahidi, A. Two-Phase Simulation of Wave-Induced Tunnel Scour beneath Marine Pipelines. J. Hydraul. Eng. 2012, 138, 517–529. [Google Scholar] [CrossRef]
- Rui, S.; Guo, Z.; Wang, L.; Wang, H.; Zhou, W. Inclined Loading Capacity of Caisson Anchor in South China Sea Carbonate Sand Considering the Seabed Soil Loss. Ocean Eng. 2022, 260, 111790. [Google Scholar] [CrossRef]
- Melville, B.; van Ballegooy, S.; Coleman, S.; Barkdoll, B. Scour Countermeasures for Wing-Wall Abutments. J. Hydraul. Eng. 2006, 132, 563–574. [Google Scholar] [CrossRef]
- Jung, C.K.; Park, H.S.; Yang, B.M. Establishment of EMTP Modeling Method Using Searching Coil Test for HVDC Submarine Cables. Trans. Korean Inst. Electr. Eng. 2010, 59, 1593–1599. [Google Scholar]
- Zhao, X.; Yan, X.; Zhang, X. Progress of Active Thermometry Method in Submarine Pipeline Scour Monitoring. In Proceedings of the ASME 2018 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, San Antonio, TX, USA, 10–12 September 2018; Volume 2, p. V002T05A014. [Google Scholar]
- Yang, S.; Tang, G.; Liu, Y.; Zhang, W.; Sun, J. CFD and machine learning approach based-predictive modeling of scouring below submarine pipeline under wave and current condition. J. Mar. Sci. Technol. 2024, 1–22. [Google Scholar]
- Welzel, M.; Raaijmakers, T.C.; van der Woude, J.H.; de Vet, P.L.M.; Hulscher, S.J.M.H. Scour variability across offshore wind farms (OWFs): Understanding drivers from field data and implications for prediction methods. Wind Energy Sci. Discuss. 2025, 1–35. [Google Scholar]
- Guo, Z.; Zhu, C.; Zhang, P.; Yuan, F.; Wang, X. Numerical Study of the Local Scouring Process and Influencing Factors of Semi-Exposed Submarine Cables. J. Mar. Sci. Eng. 2023, 11, 1349. [Google Scholar] [CrossRef]
- Paterson, D.M.; Hope, J.A.; Kenworthy, J.; Stark, J. Sticky stuff: Biological cohesion for scour and erosion prevention. Environ. Technol. 2022, 43, 4427–4438. [Google Scholar]
- Sun, Z.; Ding, K.; Li, Z. An Analytic Model of Typhoon Wind Field and Simulation of Storm Tides. Front. Mar. Sci. 2023, 10, 1253357. [Google Scholar] [CrossRef]



| Particle Size (μm) | 5 | 10 | 50 | 150 | 350 | |
|---|---|---|---|---|---|---|
| Scour Depth (cm) | ||||||
| 0.05 | 0.38 | 0.36 | 0.17 | 0.12 | 0.13 | |
| 0.1 | 0.39 | 0.39 | 0.18 | 0.13 | 0.15 | |
| 0.15 | 0.4 | 0.39 | 0.19 | 0.14 | 0.16 | |
| 0.2 | 0.41 | 0.4 | 0.19 | 0.15 | 0.18 | |
| 0.25 | 0.44 | 0.42 | 0.2 | 0.17 | 0.19 | |
| 0.26 | 0.45 | 0.43 | 0.21 | 0.18 | 0.2 | |
| 0.27 | 0.46 | 0.39 | 0.21 | 0.17 | 0.21 | |
| 0.28 | 0.46 | 0.4 | 0.22 | 0.16 | 0.19 | |
| 0.3 | 0.5 | 0.41 | 0.22 | 0.19 | 0.2 | |
| 0.35 | 0.52 | 0.45 | 0.24 | 0.18 | 0.21 | |
| 0.4 | 0.54 | 0.48 | 0.25 | 0.19 | 0.21 | |
| 0.45 | 0.56 | 0.5 | 0.25 | 0.19 | 0.23 | |
| 0.5 | 0.59 | 0.51 | 0.26 | 0.2 | 0.22 | |
| 0.55 | 0.60 | 0.53 | 0.27 | 0.2 | 0.24 | |
| Comparison of Incipient Velocity of 5 μm Sediment | Comparison of Incipient Velocity of 10 μm Sediment | |||||
|---|---|---|---|---|---|---|
| Water Depth (m) | Calculated Value (m/s) | Measured Value (m/s) | Deviation (%) | Calculated Value (m/s) | Measured Value (m/s) | Deviation (%) |
| 0.05 | 0.329 | 0.38 | 15.50 | 0.290 | 0.36 | 24.14 |
| 0.1 | 0.376 | 0.39 | 3.72 | 0.332 | 0.39 | 17.47 |
| 0.15 | 0.410 | 0.4 | −2.44 | 0.362 | 0.39 | 7.73 |
| 0.2 | 0.438 | 0.41 | −6.39 | 0.386 | 0.4 | 3.63 |
| 0.25 | 0.463 | 0.44 | −4.97 | 0.408 | 0.42 | 2.94 |
| 0.26 | 0.468 | 0.45 | −3.85 | 0.412 | 0.43 | 4.37 |
| 0.27 | 0.472 | 0.46 | −2.54 | 0.416 | 0.39 | −6.25 |
| 0.28 | 0.477 | 0.46 | −3.56 | 0.421 | 0.4 | −4.99 |
| 0.3 | 0.485 | 0.5 | 3.09 | 0.428 | 0.41 | −4.21 |
| 0.35 | 0.509 | 0.52 | 2.16 | 0.440 | 0.45 | 2.27 |
| 0.4 | 0.525 | 0.54 | 2.86 | 0.463 | 0.48 | 3.67 |
| 0.45 | 0.549 | 0.56 | 2.00 | 0.493 | 0.5 | 1.42 |
| 0.5 | 0.562 | 0.59 | 4.98 | 0.496 | 0.51 | 2.82 |
| 0.55 | 0.579 | 0.6 | 3.63 | 0.511 | 0.53 | 3.72 |
| Continuous scouring velocity 0.2 m/s | ||||||
| Particle size (μm) | 5 | 10 | 50 | 150 | 350 | |
| Scour depth (cm) | ||||||
| 1 | No scouring | 105 | 63 | 87 | ||
| 2 | 326 | 130 | 256 | |||
| 3 | 650 | 219 | 471 | |||
| Continuous scouring velocity 0.4 m/s | ||||||
| Particle size (μm) | 5 | 10 | 50 | 150 | 350 | |
| Scour depth (cm) | ||||||
| 1 | 70 | 34 | 27 | 9 | 31 | |
| 2 | 184 | 88 | 58 | 23 | 65 | |
| 3 | 363 | 169 | 93 | 62 | 99 | |
| Continuous scouring velocity 0.5 m/s | ||||||
| Particle size (μm) | 5 | 10 | 50 | 150 | 350 | |
| Scour depth (cm) | ||||||
| 1 | 63 | 30 | 23 | 6 | 26 | |
| 2 | 167 | 77 | 49 | 17 | 57 | |
| 3 | 330 | 144 | 79 | 33 | 98 | |
| 5 μm | 10 μm | ||||||||||
| 0.2 | 0.26 | 0.125 | No | 0.395 | 0.455 | 0.2 | 0.26 | 0.125 | No scouring | 0.448 | 0.476 |
| 0.27 | 0.123 | 0.391 | 0.444 | 0.27 | 0.123 | 0.443 | 0.465 | ||||
| 0.28 | 0.121 | 0.387 | 0.435 | 0.28 | 0.121 | 0.439 | 0.513 | ||||
| 0.4 | 0.26 | 0.251 | 0.000143 | 0.790 | 0.909 | 0.4 | 0.26 | 0.251 | 0.000294 | 0.896 | 0.952 |
| 0.27 | 0.246 | 0.000088 | 0.782 | 0.889 | 0.27 | 0.246 | 0.000185 | 0.887 | 0.930 | ||
| 0.28 | 0.241 | 0.000056 | 0.774 | 0.870 | 0.28 | 0.241 | 0.000123 | 0.878 | 1.026 | ||
| 0.5 | 0.26 | 0.313 | 0.000159 | 0.987 | 1.136 | 0.5 | 0.26 | 0.313 | 0.000333 | 1.120 | 1.190 |
| 0.27 | 0.307 | 0.000096 | 0.977 | 1.111 | 0.27 | 0.307 | 0.000213 | 1.109 | 1.163 | ||
| 0.28 | 0.302 | 0.000061 | 0.968 | 1.087 | 0.28 | 0.302 | 0.000149 | 1.098 | 1.282 | ||
| 50 μm | 150 μm | ||||||||||
| 0.2 | 0.26 | 0.125 | 0.000095 | 0.612 | 1.000 | 0.2 | 0.26 | 0.125 | 0.000159 | 0.791 | 1.176 |
| 0.27 | 0.123 | 0.000045 | 0.609 | 0.952 | 0.27 | 0.123 | 0.000149 | 0.787 | 1.111 | ||
| 0.28 | 0.121 | 0.000031 | 0.605 | 0.952 | 0.28 | 0.121 | 0.000112 | 0.782 | 1.176 | ||
| 0.4 | 0.26 | 0.251 | 0.000370 | 1.224 | 2.000 | 0.4 | 0.26 | 0.251 | 0.001111 | 1.582 | 2.353 |
| 0.27 | 0.246 | 0.000323 | 1.217 | 1.905 | 0.27 | 0.246 | 0.000714 | 1.573 | 2.222 | ||
| 0.28 | 0.241 | 0.000286 | 1.210 | 1.905 | 0.28 | 0.241 | 0.000256 | 1.565 | 2.353 | ||
| 0.5 | 0.26 | 0.313 | 0.000435 | 1.531 | 2.500 | 0.5 | 0.26 | 0.313 | 0.001667 | 1.978 | 2.941 |
| 0.27 | 0.307 | 0.000385 | 1.522 | 2.381 | 0.27 | 0.307 | 0.000909 | 1.967 | 2.778 | ||
| 0.28 | 0.302 | 0.000333 | 1.513 | 2.381 | 0.28 | 0.302 | 0.000625 | 1.956 | 2.941 | ||
| 350 μm | Note: | ||||||||||
| is the velocity of the pool (m/s) | |||||||||||
| 0.2 | 0.26 | 0.125 | 0.000115 | 0.745 | 1.053 | is water depth (cm) | |||||
| 0.27 | 0.123 | 0.000059 | 0.741 | 1.000 | is the Froude number | ||||||
| 0.28 | 0.121 | 0.000047 | 0.737 | 0.952 | |||||||
| 0.4 | 0.26 | 0.251 | 0.000323 | 1.489 | 2.105 | is scouring rate (m/s) | |||||
| 0.27 | 0.246 | 0.000294 | 1.481 | 2.000 | |||||||
| 0.28 | 0.241 | 0.000294 | 1.473 | 1.905 | is the relative velocity and the ratio of pool velocity to measured starting velocity | ||||||
| 0.5 | 0.26 | 0.313 | 0.000385 | 1.862 | 2.632 | ||||||
| 0.27 | 0.307 | 0.000323 | 1.852 | 2.500 | is the relative velocity and the ratio of pool velocity to calculated starting velocity | ||||||
| 0.28 | 0.302 | 0.000244 | 1.842 | 2.381 | |||||||
| Sediment Grain Size (μm) | Scouring Duration Mins (Including 2 h Scouring Balance Holding Time) | Measured Scour Depth (m) | Scouring Depth Calculation Value (m) |
|---|---|---|---|
| 5 | 290 | 0.27 | 0.243 |
| 10 | 482 | 0.31 | 0.275 |
| 50 | 1002 | 0.48 | 0.477 |
| 150 | The 30 cm thick sediment block was completely scoured, lasting 630 | 0.55 | 0.57 |
| 350 | 1157 | 0.54 | 0.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, F.; Yang, W.; Liu, F.; Zhu, L.; Sun, Z. Experimental Study of Sediment Incipient Velocity and Scouring in Submarine Cable Burial Areas. Water 2025, 17, 1310. https://doi.org/10.3390/w17091310
Chen F, Yang W, Liu F, Zhu L, Sun Z. Experimental Study of Sediment Incipient Velocity and Scouring in Submarine Cable Burial Areas. Water. 2025; 17(9):1310. https://doi.org/10.3390/w17091310
Chicago/Turabian StyleChen, Fanjun, Wankang Yang, Feng Liu, Lili Zhu, and Zhilin Sun. 2025. "Experimental Study of Sediment Incipient Velocity and Scouring in Submarine Cable Burial Areas" Water 17, no. 9: 1310. https://doi.org/10.3390/w17091310
APA StyleChen, F., Yang, W., Liu, F., Zhu, L., & Sun, Z. (2025). Experimental Study of Sediment Incipient Velocity and Scouring in Submarine Cable Burial Areas. Water, 17(9), 1310. https://doi.org/10.3390/w17091310







