Hydrogeological Assessment and Steady-State Groundwater Flow Modeling for Groundwater Management in the Golina River Sub-Basin, Northern Ethiopia, Using MODFLOW 6
Abstract
1. Introduction
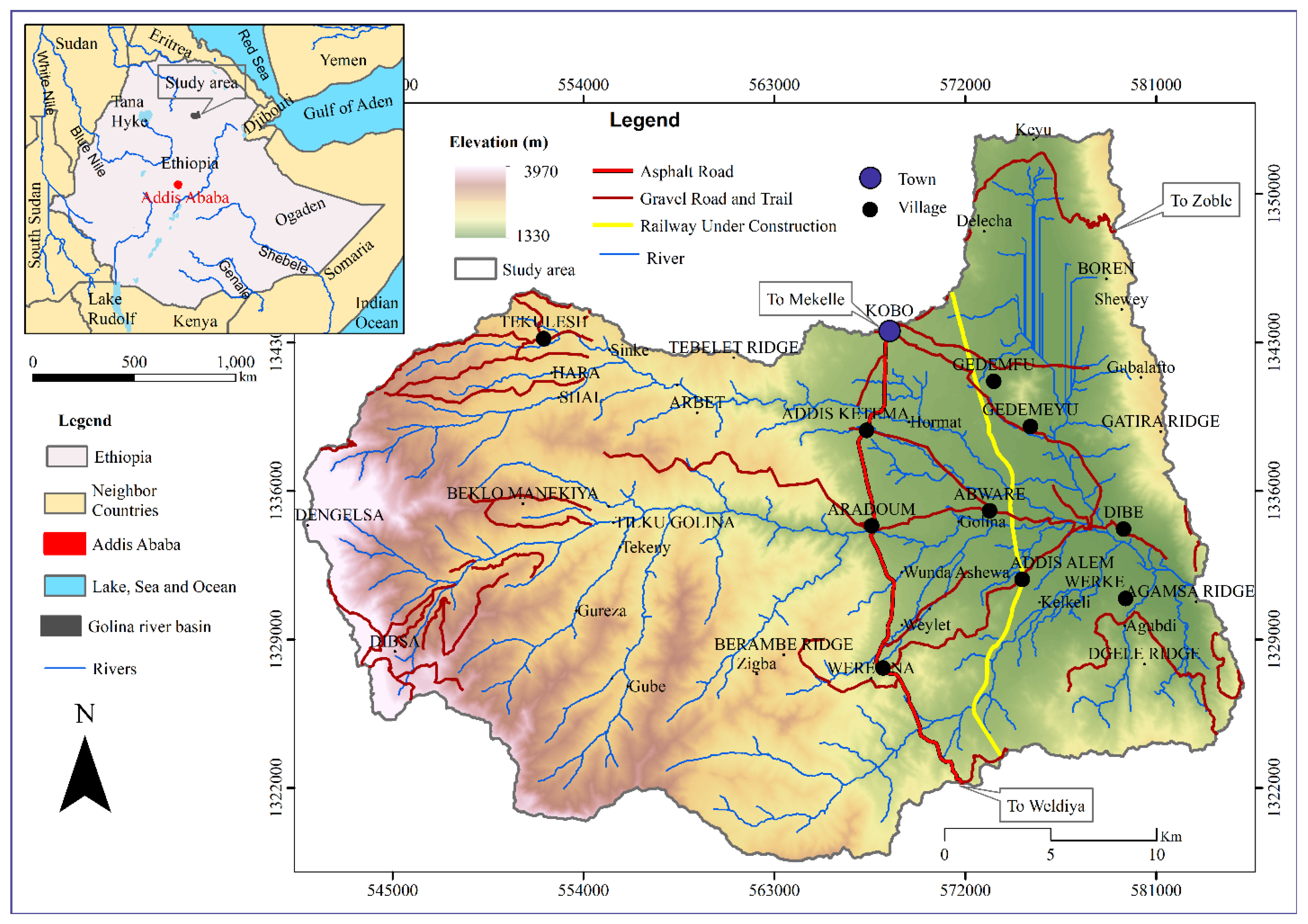
2. Study Area
3. Methods
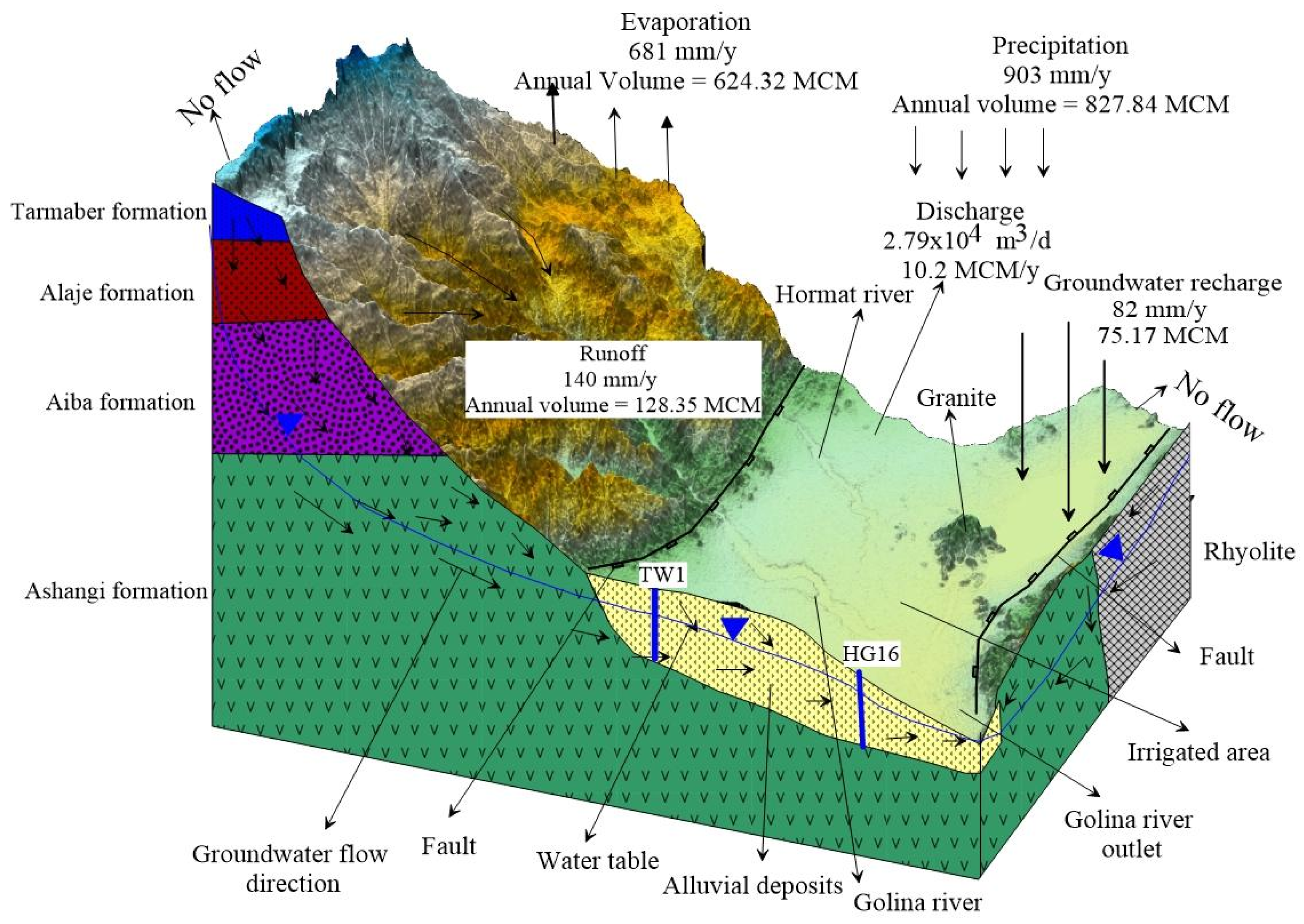
3.1. Conceptual Model
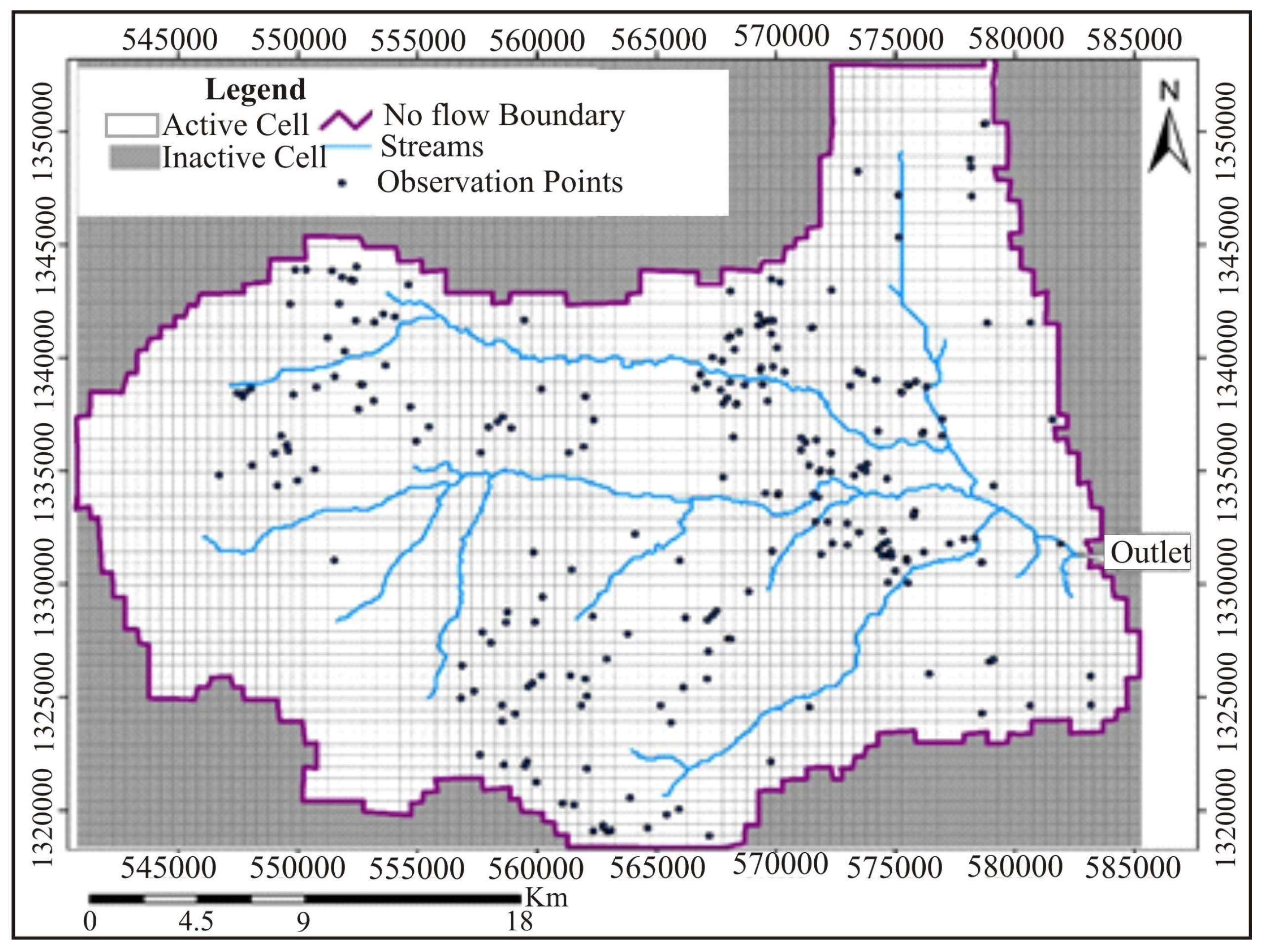
3.2. Study Area Modeling and Boundary Conditions
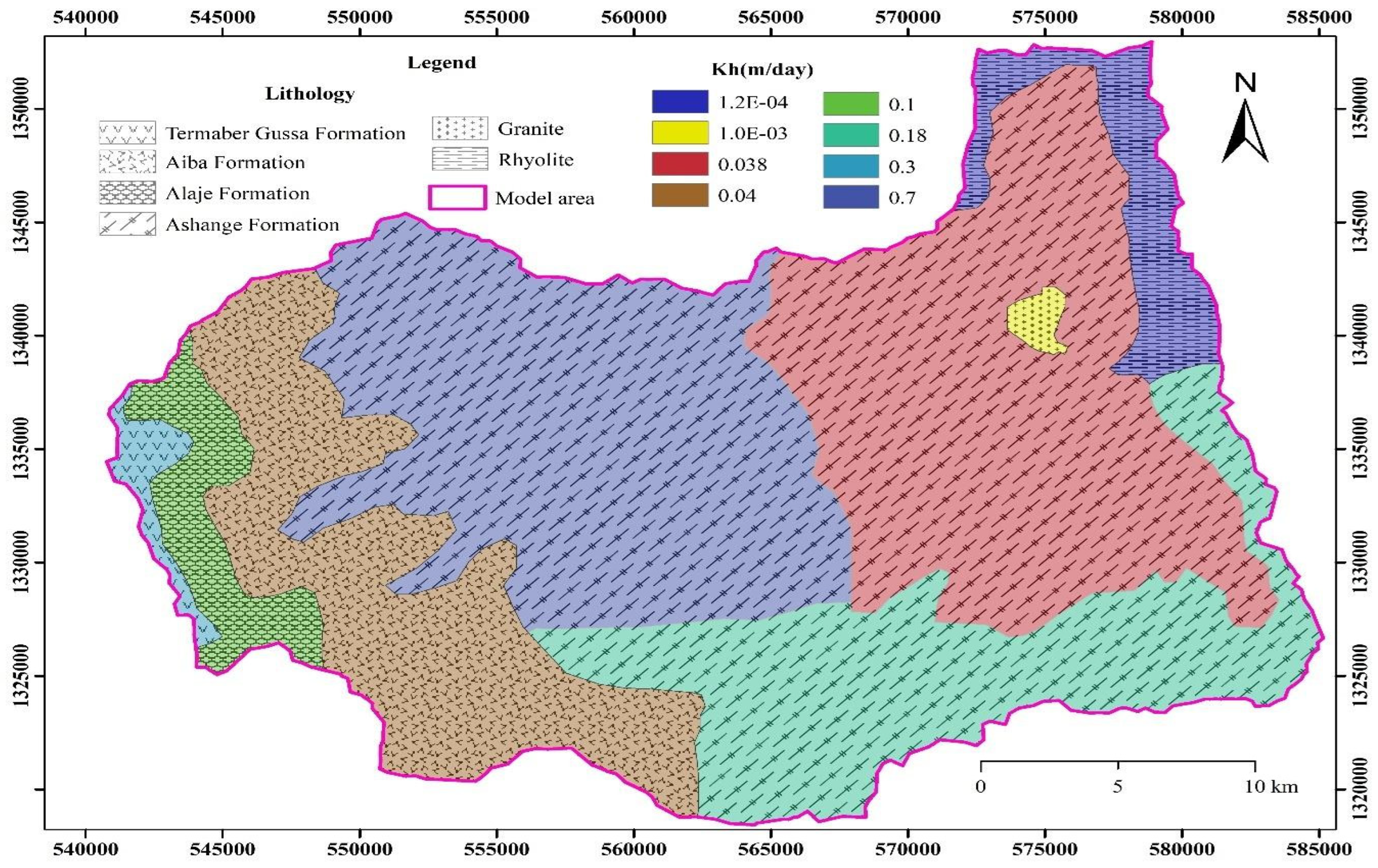
3.3. Model Input Parameters
3.3.1. Initial Hydraulic Head
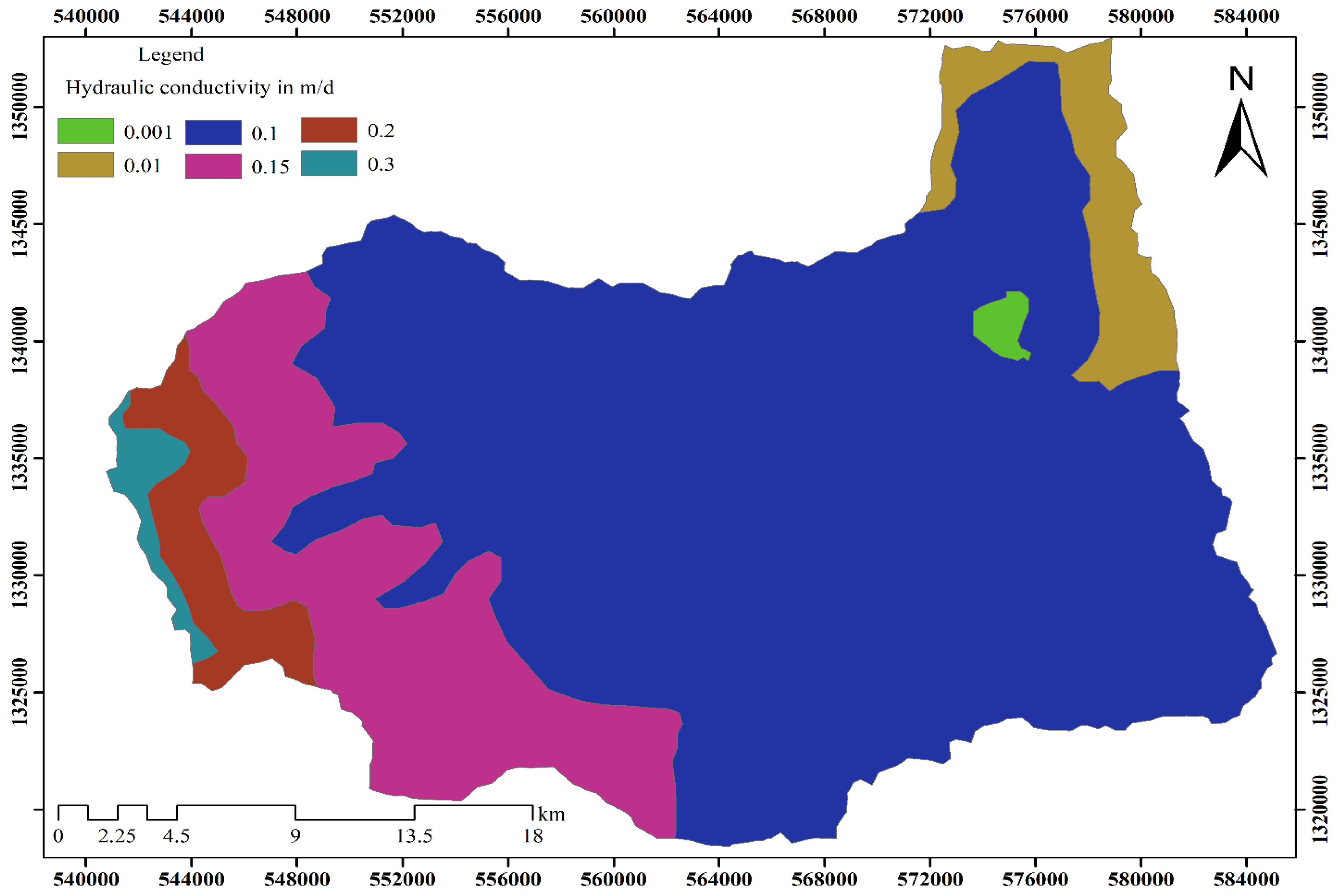
3.3.2. Aquifer Parameters
3.3.3. Recharge and Discharge
3.3.4. Drain
3.4. Groundwater Head Observations
4. Results and Discussion
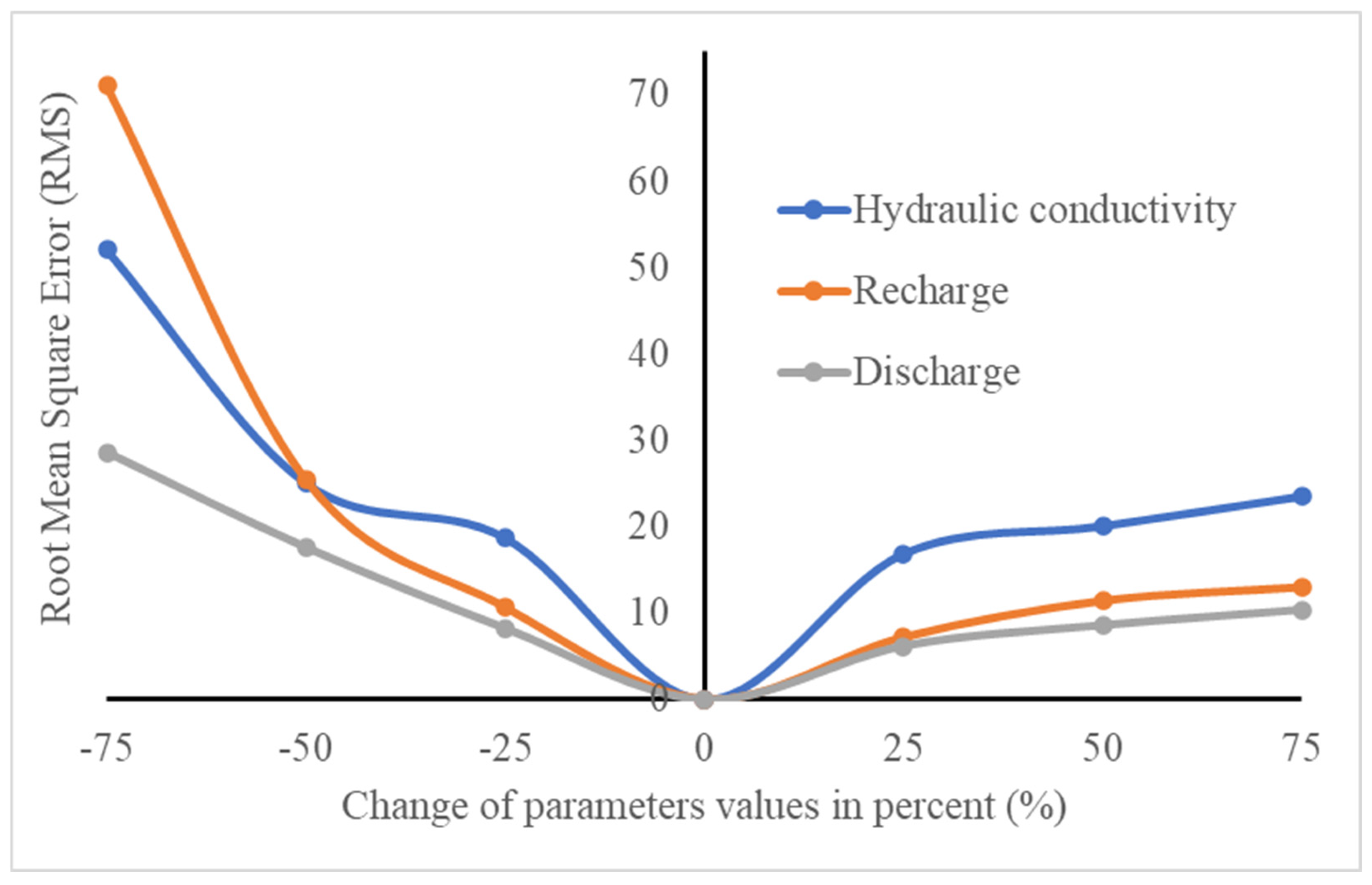
4.1. Model Sensitivity Analysis
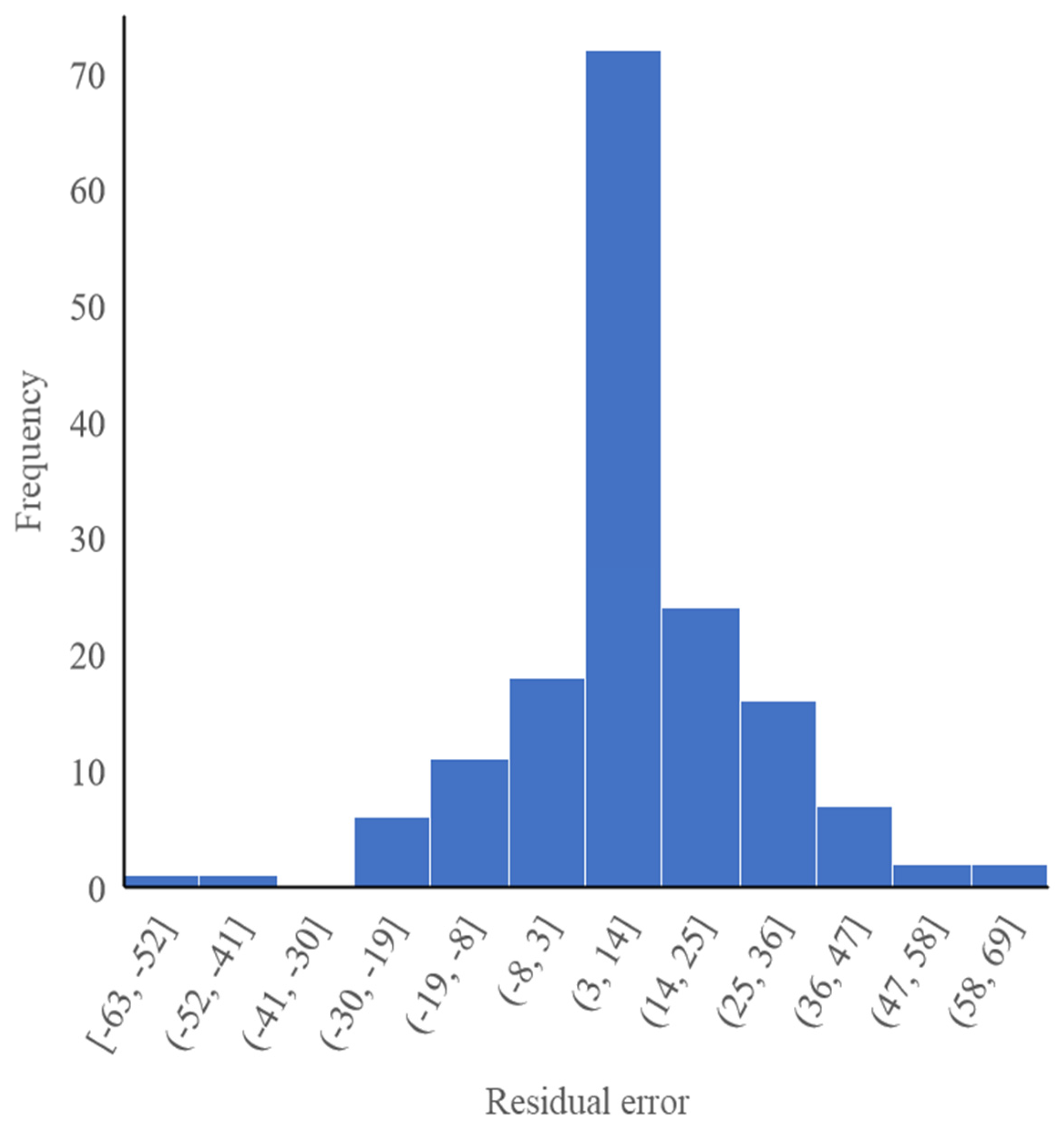
4.2. Model Calibration
4.3. Simulated Groundwater Head and Flow Direction
4.4. Water (Volumetric) Budget of the Model Area
4.5. Scenario Analysis
4.5.1. Effect of Increasing Pumping Rates
4.5.2. Effect of Decreasing Recharge
5. Conclusions and Recommendations
5.1. Conclusions
5.2. Recommendations
- ⮚
- It is recommended to carry out field studies to accurately assess the hydraulic conductivity of layer-2 in particular, because the current values are based on the scientific literature, and to improve those of layer-2.
- ⮚
- Establish a network of representative municipality and community stakeholders to monitor groundwater levels and estimate abstraction rates in representative wells in the sub-basin, particularly those used for extensive/intensive irrigation. This includes ensuring that wells are accessible for periodic groundwater-level measurements and that selected wells have reliable contactors that measure withdrawals.
- ⮚
- Similarly, identify river-level measuring stations at different locations along the Hormat, Golina, and Kelkelit rivers and at the mouth of the Golina River Sub-Basin and install fixed and portable instrumentation to accurately measure the flows of these rivers.
- ⮚
- Given the observed reduction in aquifer recharge and its impact on groundwater levels, based on the results obtained, it is urgent to develop recommendations and guidelines for the monitoring and sustainable management of wells, especially those used for agriculture, which are the ones that contribute most to the overexploitation of the aquifers.
- ⮚
- It is, therefore, recommended that decision-makers, water resource managers, and researchers, based on data already collected and those that will be collected periodically at the beginning and end of the dry session, contribute (1) to the modeling of groundwater flow under transient conditions (the researchers) and (2) to the optimization and strengthening of the capacity for sustainable management of the groundwater resources in the study area (the resource managers).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Freeze, R.A.; Cherry, J.A. Groundwater. In Groundwater; The Groundwather Foundation: Westerville, OH, USA, 1979. [Google Scholar]
- Singhal, B.B.S.; Gupta, R.P. Applied Hydrogeology of Fractured Rocks: Second Edition; Springer: Berlin/Heidelberg, Germany, 2010; ISBN 978-90-481-8798-0. [Google Scholar]
- Cushman, J.H.; Tartakovsky, D.M. The Handbook of Groundwater Engineering: Third Edition; Routhledge: Abingdon, UK, 2016; ISBN 978-1-4987-0305-5. [Google Scholar]
- Hu, B.X.; Wu, J.; He, C. On Stochastic Modeling of Groundwater Flow and Solute Transport in Multi-Scale Heterogeneous Formations. Comput. Appl. Math. 2004, 23, 121–152. [Google Scholar] [CrossRef][Green Version]
- Zektser, I.S.; Everett, L.G. Groundwater Resources of the World and Their Use; Unesco: Paris, France, 2004; ISBN 92-9220-007-0. [Google Scholar]
- Azeref, B.G.; Bushira, K.M. Numerical Groundwater Flow Modeling of the Kombolcha Catchment Northern Ethiopia. Model. Earth Syst. Environ. 2020, 6, 1233–1244. [Google Scholar] [CrossRef]
- Rossman, N.R.; Zlotnik, V.A. Review: Regional Groundwater Flow Modeling in Heavily Irrigated Basins of Selected States in the Western United States. Hydrogeol. J. 2013, 21, 1173. [Google Scholar] [CrossRef]
- Senent-Aparicio, J.; Peñafiel, L.; Alcalá, F.J.; Jimeno-Sáez, P.; Pérez-Sánchez, J. Climate Change Impacts on Renewable Groundwater Resources in the Andosol-Dominated Andean Highlands, Ecuador. Catena 2024, 236, 107766. [Google Scholar] [CrossRef]
- Varalakshmi, V.; Venkateswara Rao, B.; SuriNaidu, L.; Tejaswini, M. Groundwater Flow Modeling of a Hard Rock Aquifer: Case Study. J. Hydrol. Eng. 2014, 19, 877–886. [Google Scholar] [CrossRef]
- Yihdego, Y.; Danis, C.; Paffard, A. 3-D Numerical Groundwater Flow Simulation for Geological Discontinuities in the Unkheltseg Basin, Mongolia. Environ. Earth Sci. 2015, 73, 4119–4133. [Google Scholar] [CrossRef]
- Yihdego, Y.; Webb, J.A.; Leahy, P. Modelling of Lake Level under Climate Change Conditions: Lake Purrumbete in Southeastern Australia. Environ. Earth Sci. 2015, 73, 3855–3872. [Google Scholar] [CrossRef]
- Razack, M.; Furi, W.; Fanta, L.; Shiferaw, A. Water Resource Assessment of a Complex Volcanic System under Semi-Arid Climate Using Numerical Modeling: The Borena Basin in Southern Ethiopia. Water 2020, 12, 276. [Google Scholar] [CrossRef]
- Ahmed, S.; Jayakumar, R.; Salih, A. Groundwater Dynamics in Hard Rock Aquifers: Sustainable Management and Optimal Monitoring Network Design; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 978-1-4020-6539-2. [Google Scholar]
- Meyer, P.A.; Brouwers, M.; Martin, P.J. A Three-Dimensional Groundwater Flow Model of the Waterloo Moraine for Water Resource Management. Can. Water Resour. J. 2014, 39, 167–180. [Google Scholar] [CrossRef]
- Pétré, M.A.; Rivera, A.; Lefebvre, R. Numerical Modeling of a Regional Groundwater Flow System to Assess Groundwater Storage Loss, Capture and Sustainable Exploitation of the Transboundary Milk River Aquifer (Canada, USA). J. Hydrol. 2019, 575, 656–670. [Google Scholar] [CrossRef]
- Islam, M.B.; Firoz, A.B.M.; Foglia, L.; Marandi, A.; Khan, A.R.; Schüth, C.; Ribbe, L. A Regional Groundwater-Flow Model for Sustainable Groundwater-Resource Management in the South Asian Megacity of Dhaka, Bangladesh. Hydrogeol. J. 2017, 25, 617–637. [Google Scholar] [CrossRef]
- Michael, H.A.; Voss, C.I. Controls on Groundwater Flow in the Bengal Basin of India and Bangladesh: Regional Modeling Analysis. Hydrogeol. J. 2009, 17, 1561–1577. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, W. A Review of Regional Groundwater Flow Modeling. Geosci. Front. 2011, 2, 205–214. [Google Scholar] [CrossRef]
- Janos, D.; Molson, J.; Lefebvre, R. Regional Groundwater Flow Dynamics and Residence Times in Chaudière-Appalaches, Québec, Canada: Insights from Numerical Simulations. Can. Water Resour. J. 2018, 43, 214–239. [Google Scholar] [CrossRef]
- Desalegn, A. Groundwater Potential Evaluation and Flow Dynamics of Hormat, Golina River Catchment, Kobo Valley, North Ethiopia. Master’s Thesis, Addis Ababa University, Addis Ababa, Ethiopia, 2011. [Google Scholar]
- Tadesse, M.; Bekele, H.; Mitiku, B.; Teshome, M.; Besufekade, A.; Edris, M.; Burussa, G.; Yehualaeshet, E.; Alemu, S.; Tadesse, A. Geology, Geochemistry and Gravity Survey of the Maychew Area: Memoir 31; Ministry of Mines, Geological Survey of Ethiopia, Basic Geoscience Mapping Core Process: Addis Ababa, Ethiopia, 2011; p. 68.
- Tadesse, N.; Nedaw, D.; Woldearegay, K.; Gebreyohannes, T.; Steenbergen, F.V. Groundwater Management for Irrigation in the Raya and Kobo Valleys, Northern Ethiopia. Int. J. Earth Sci. Eng. 2015, 8, 1104–1114. [Google Scholar]
- Gebru, H.; Gebreyohannes, T.; Hagos, E. Identification of Groundwater Potential Zones Using Analytical Hierarchy Process (AHP) and GIS-Remote Sensing Integration, the Case of Golina River Sub-Basin, Northern Ethiopia. Int. J. Adv. Remote Sens. GIS 2020, 9, 3289–3311. [Google Scholar] [CrossRef]
- Gebru, H.; Gebreyohannes, T.; Hagos, E. WetSpass Model and Chloride Mass Balance Based Groundwater Recharge Estimation: The Case of Golina River Sub-Basin, Northern Ethiopia. Sustain. Water Resour. Manag. 2023, 9, 188. [Google Scholar] [CrossRef]
- Gebru, H.; Gebreyohannes, T.; Hagos, E. Ascertaining the Source of Major Dissolved Solute and Recharge Mechanism of Groundwater in the Golina River Sub-Basin, Northern Ethiopia. Daagu Int. J. Basic Appl. Res. 2023, 5, 120–143. [Google Scholar]
- Kresic, N.; Mikszewski, A. Hydrogeological Conceptual Site Models: Data Analysis and Visualization; Routhledge: Abingdon, UK, 2013; ISBN 978-1-4398-5228-6. [Google Scholar]
- Anderson, M.P.; Woessner, W.W.; Hunt, R.J. Applied Modeling Groundwater Simulation of Flow and Advective Transport; Elsevier: Amsterdam, The Netherlands, 2015; ISBN 978-0-12-058103-0. [Google Scholar]
- Winter, T.C. The Concept of Hydrologic Landscapes. J. Am. Water Resour. Assoc. 2001, 37, 335–349. [Google Scholar] [CrossRef]
- Darcy, H. Les Fontaines Publiques de La Ville de Dijon. In Recherche; Bnf Site Institutionnel: Paris, France, 1856. [Google Scholar]
- Lavoisier, A.L. Traité Élémentaire de Chimie (Elementary Treatise on Chemistry); Smithsonian Libraries: Paris, France, 1789. [Google Scholar]
- Langevin, C.D.; Hughes, J.D.; Banta, E.R.; Niswonger, R.G.; Panday, S.; Provost, A.M. Documentation for the MODFLOW 6 Groundwater Flow Model; U.S. Geological Survey: Reston, VA, USA, 2017. [CrossRef]
- Winston, R.B. ModelMuse Version 4: A Graphical User Interface for MODFLOW 6; U.S. Geological Survey: Reston, VA, USA, 2019; Volume 10. [CrossRef]
- Chahar, B.R. Groundwater Hydrology; McGraw Hill Education: New Delhi, India, 2015; ISBN 978-93-392-0463-1. [Google Scholar]
- Gebreyohannes, T.; De Smedt, F.; Walraevens, K.; Gebresilassie, S.; Hussien, A.; Hagos, M.; Amare, K.; Deckers, J.; Gebrehiwot, K. Regional groundwater flow modeling of the Geba basin, northern Ethiopia. Hydrogeol. J. 2017, 25, 639–655. [Google Scholar] [CrossRef]
- Balcha, S.K.; Hulluka, T.A.; Awass, A.A.; Bantider, A.; Ayele, G.T.; Walsh, C.L. Numerical Groundwater Flow Modeling under Future Climate Change in the Central Rift Valley Lakes Basin; Ethiopia. J. Hydrol. Reg. Stud. 2024, 52, 101733. [Google Scholar] [CrossRef]
- Bushra, A.H. Quantitative Status, Vulnerability and Pollution of Groundwater Resources in Different Environmental and Climatic Contexts in Sardinia and in Ethiopia. Ph.D. Thesis, University degli Studi di Cagliari, Cagliari, Italy, 2011. [Google Scholar]
- Manghi, F.; Williams, D.; Safely, J.; Hamdi, M.R. Groundwater Flow Modeling of the Arlington Basin to Evaluate Management Strategies for Expansion of the Arlington Desalter Water Production. Water Resour. Manag. 2012, 26, 21–41. [Google Scholar] [CrossRef]







| K (m/d) (Average) | Productivity | No. Boreholes | Lithological Features of the Distinguished 4 Zones |
|---|---|---|---|
| <1 (0.67) | Very low | 10 | 1-Clay-dominated; a mix of clay with sand, silt, and gravel. |
| 1 < K < 5 (3.25) | Low | 18 | 2-Fine-sand-dominated; a mix of sand and gravel with silt. |
| 5 < K < 20 (8.78) | Moderate | 27 | 3-Medium-sand-dominated; a mix of sand and gravel. |
| >20 (27.26) | High | 11 | 4-Coarse-sand-dominated; a mix of sand and gravel, boulders, and cobbles. |
| K, m/Day | Lithological Features of the Distinguished 4 Zones |
|---|---|
| 0.3 | 1-Termaber Gussa formation |
| 0.15 | 2-Aiba formation |
| 0.2 | 3-Alaje formation |
| 0.1 | 4-Ashangi formation |
| 0.001 | Rhyolites |
| 0.01 | Granites |
| Parameter | Min | Max | Mean | St. Dev |
|---|---|---|---|---|
| Borehole Depth (m) | 44.30 | 212.00 | 129.52 | 36.93 |
| Static Water Level (m) | 0.00 | 58.78 | 18.28 | 9.76 |
| Dynamic Water Level (m) | 12.47 | 80.90 | 34.92 | 13.67 |
| Drawdown (m) | 1.00 | 54.80 | 16.64 | 11.85 |
| Discharge (L/s) | 2.50 | 85.00 | 48.03 | 20.29 |
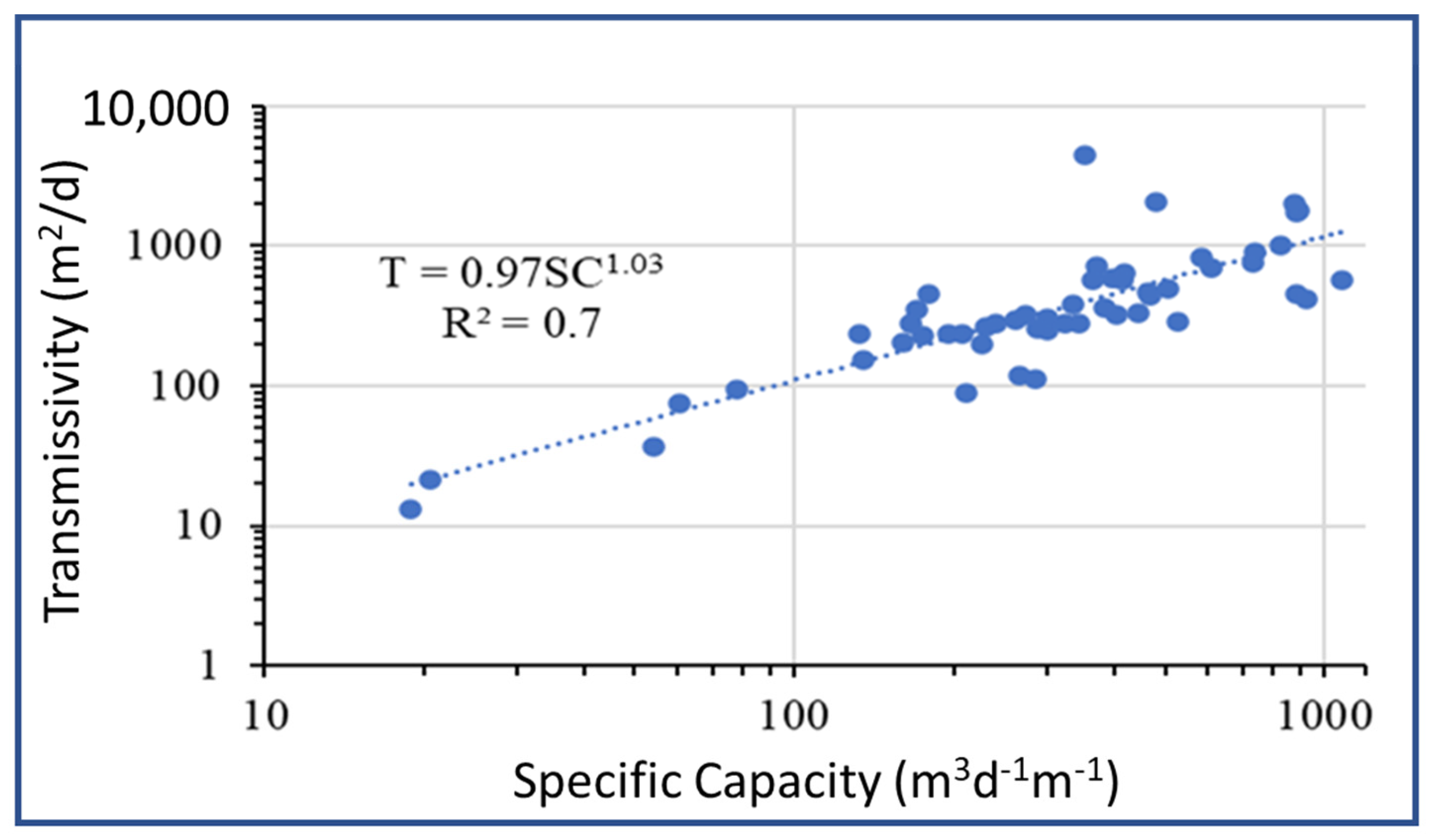
| Specific Capacity (m3/d−/m) | 15.77 | 1076.64 | 360.98 | 250.84 |
| Transmissivity (m2/d) | 7.90 | 4510.00 | 495.11 | 669.58 |
| Hydraulic Conductivity (m/d) | 0.081 | 125.00 | 10.31 | 17.45 |
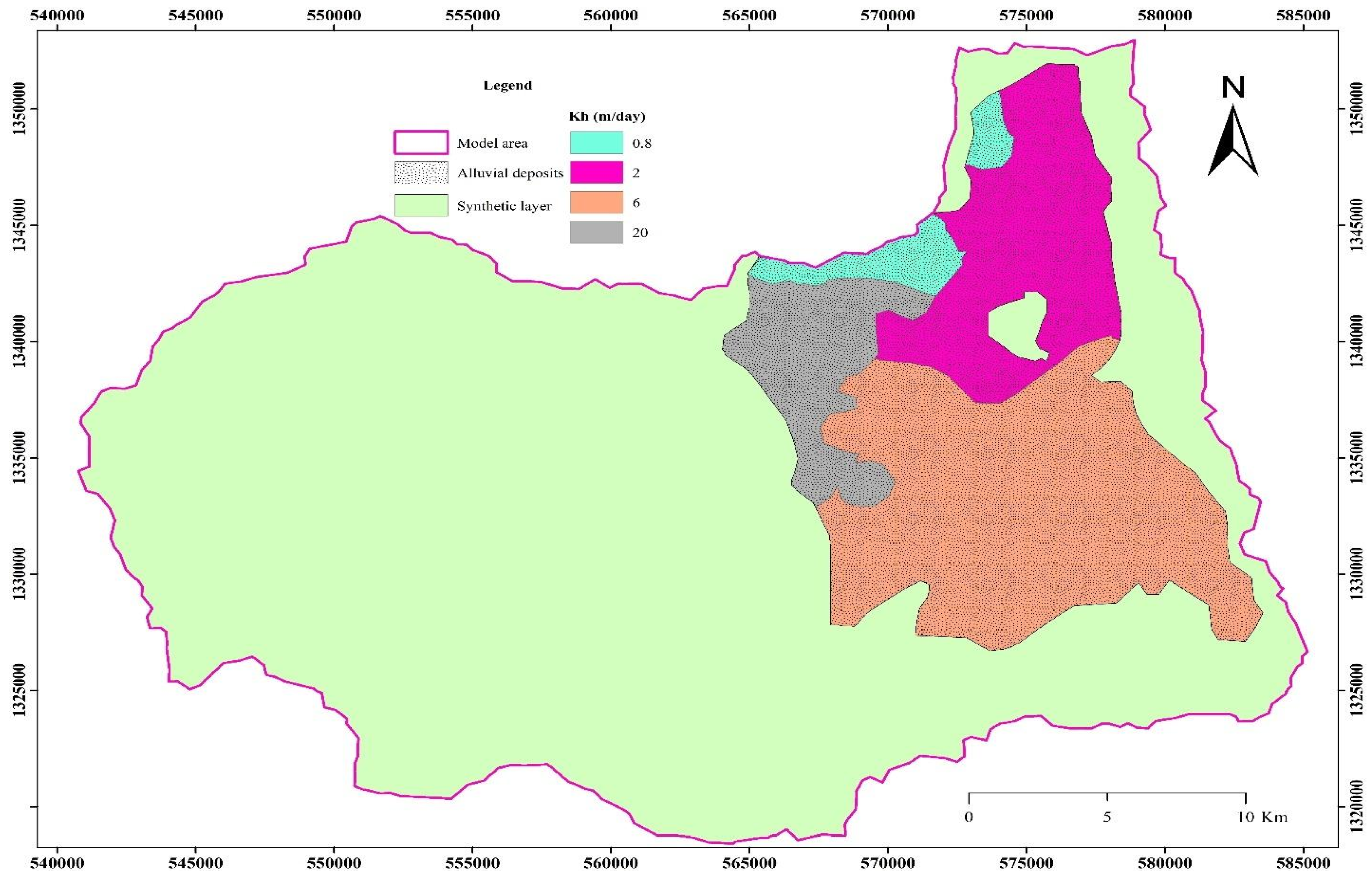
| Layer | Lithology | Zone | Calibrated Hydraulic Conductivity (Kh) in m/d | |
|---|---|---|---|---|
| 1 | Alluvial deposit | Zone-1 | 0.8 | |
| Zone-2 | 2 | |||
| Zone-3 | 6 | |||
| Zone-4 | 20 | |||
| 2 | Weathered and fractured magmatic rocks | Termaber formation | - | 0.3 |
| Alaje formation | - | 0.1 | ||
| Aiba formation | - | 0.04 | ||
| Ashangi formation | Zone-1 | 0.7 | ||
| Zone-2 | 3.8 × 10−2 | |||
| Zone-3 | 0.18 | |||
| Granite | - | 1.0 × 10−3 | ||
| Rhyolite | - | 1.2 × 10−4 |
| Flow Types | Inflow | Outflow | Difference |
|---|---|---|---|
| Well | 0.0000 | 27,945.2046 (Domestic and irrigation) | −27,945.2046 |
| Drain | 0.0000 | 179,830.7328 (drain discharge) | −179,830.7328 |
| Recharge | 207,775.8297 (precipitation) | 0.0000 | 207,775.8297 |
| Total | 207,775.8297 | 207,775.9373 | −0.1076 |
| Percentage Discrepancy | 0.00 |
| Flow Terms | 25% | 50% | 75% | |||
|---|---|---|---|---|---|---|
| Inflow | Outflow | Inflow | Outflow | Inflow | Outflow | |
| Well | 0.000 | 34,931.51 | 0.000 | 41,917.81 | 0.000 | 48,904.11 |
| Drain | 0.000 | 172,844.48 | 0.000 | 165,858.15 | 0.000 | 158,871.99 |
| Discharge rate increase | 6986.31 | 13,972.62 | 20,958.93 | |||
| Recharge | 207,775.83 | 0.000 | 207,775.83 | 0.000 | 207,775.83 | 0.000 |
| Total | 207,775.83 | 207,775.98 | 207,775.83 | 207,775.96 | 207,775.83 | 207,776.09 |
| Difference | −0.1534 | −0.1269 | −0.2599 | |||
| % Discrepancy | −0.00 | −0.00 | −0.00 | |||
| Change in head (m) | 4.64 | 10.18 | 17.38 | |||
| Flow Terms | 25% | 50% | 75% | |||
|---|---|---|---|---|---|---|
| Inflow | Outflow | Inflow | Outflow | Inflow | Outflow | |
| Well | 0.000 | 27,945.20 | 0.000 | 27,945.20 | 0.000 | 48,904.11 |
| Drain | 0.000 | 127,886.65 | 0.000 | 75,942.75 | 0.000 | 23,998.85 |
| Recharge | 155,831.87 | 0.000 | 103,887.91 | 0.000 | 51,943.96 | 0.000 |
| Total | 155,831.87 | 155,831.86 | 103,887.91 | 103,887.95 | 51,943.96 | 51,944.06 |
| Difference | −1.6 × 10−2 | −3.6 × 10−2 | −9.8 × 10−2 | |||
| % Discrepancy | 0.00 | −0.00 | −0.00 | |||
| Change in head (m) | 6.00 | 15.29 | 46.97 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gebru, H.; Gebreyohannes, T.; Hagos, E.; Perilli, N. Hydrogeological Assessment and Steady-State Groundwater Flow Modeling for Groundwater Management in the Golina River Sub-Basin, Northern Ethiopia, Using MODFLOW 6. Water 2025, 17, 949. https://doi.org/10.3390/w17070949
Gebru H, Gebreyohannes T, Hagos E, Perilli N. Hydrogeological Assessment and Steady-State Groundwater Flow Modeling for Groundwater Management in the Golina River Sub-Basin, Northern Ethiopia, Using MODFLOW 6. Water. 2025; 17(7):949. https://doi.org/10.3390/w17070949
Chicago/Turabian StyleGebru, Hindeya, Tesfamichael Gebreyohannes, Ermias Hagos, and Nicola Perilli. 2025. "Hydrogeological Assessment and Steady-State Groundwater Flow Modeling for Groundwater Management in the Golina River Sub-Basin, Northern Ethiopia, Using MODFLOW 6" Water 17, no. 7: 949. https://doi.org/10.3390/w17070949
APA StyleGebru, H., Gebreyohannes, T., Hagos, E., & Perilli, N. (2025). Hydrogeological Assessment and Steady-State Groundwater Flow Modeling for Groundwater Management in the Golina River Sub-Basin, Northern Ethiopia, Using MODFLOW 6. Water, 17(7), 949. https://doi.org/10.3390/w17070949






