Study on Shear Creep Characteristics of Carbonaceous Mud Shale at Different Moisture Contents Under Temperature Cycling
Abstract
:1. Introduction
2. Experimental Scheme
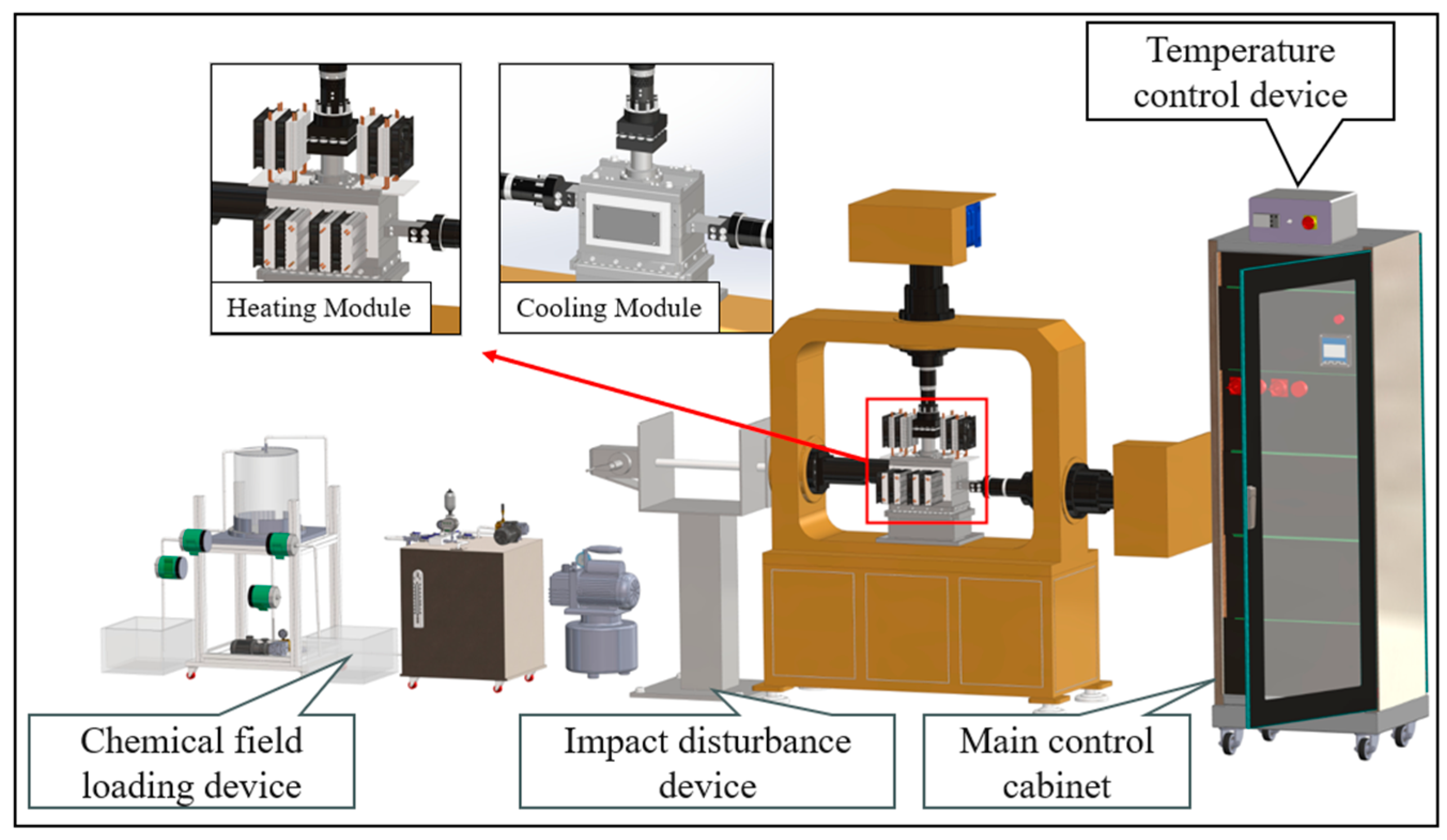
2.1. Experimental Instruments
2.2. Rock Sample Preparation
2.3. Conventional Straight Cut Test
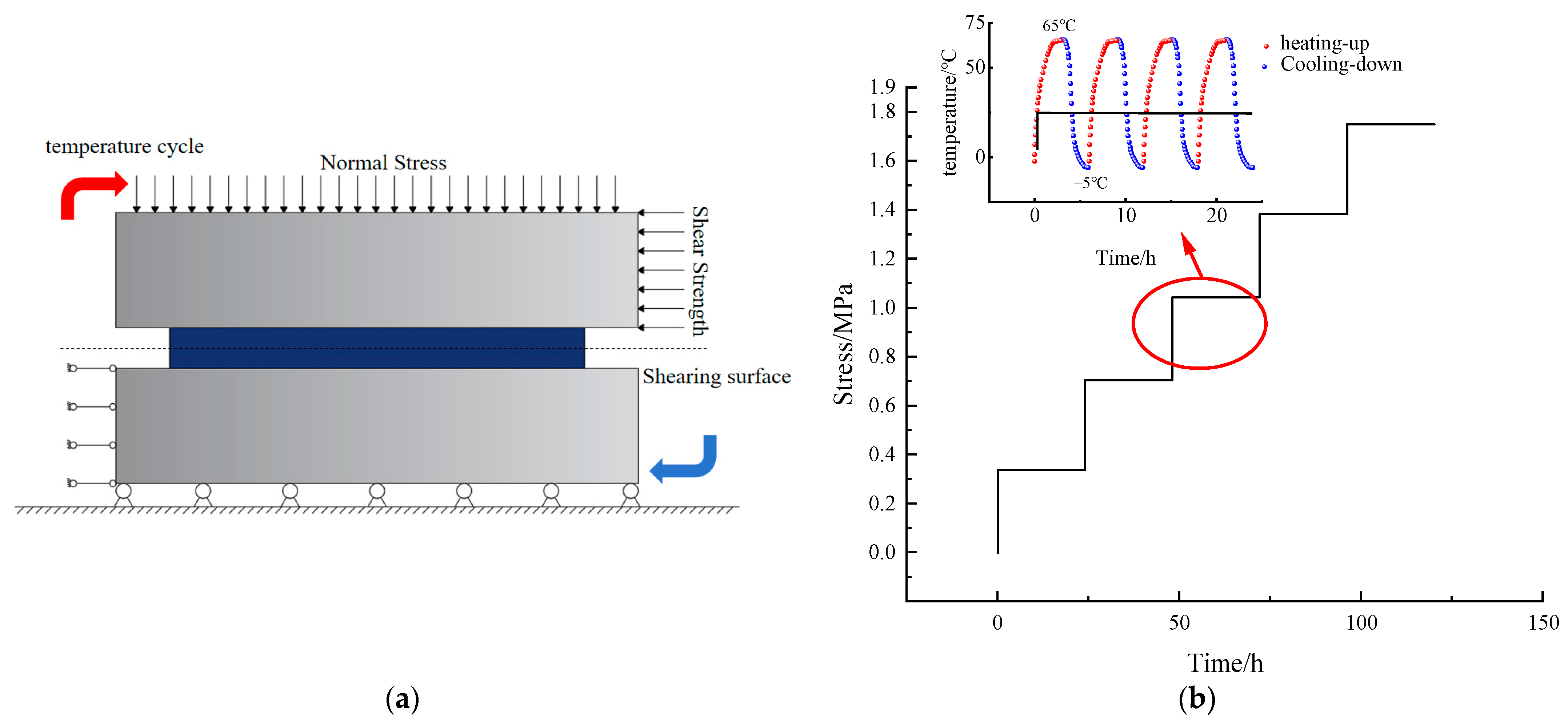
2.4. Shear Creep Test
3. Analysis of Experimental Results
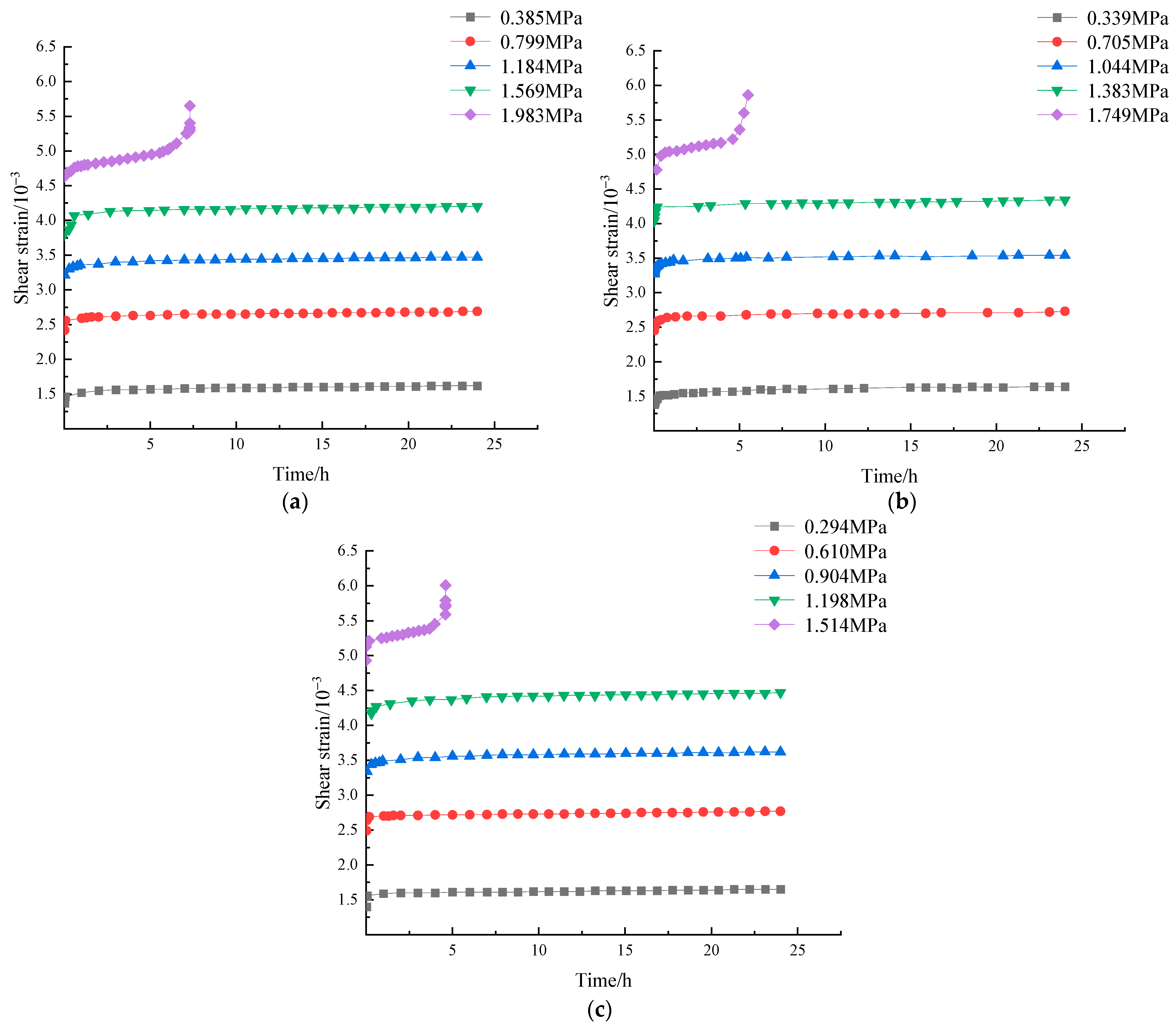
3.1. Analysis of Overall Creep Curve
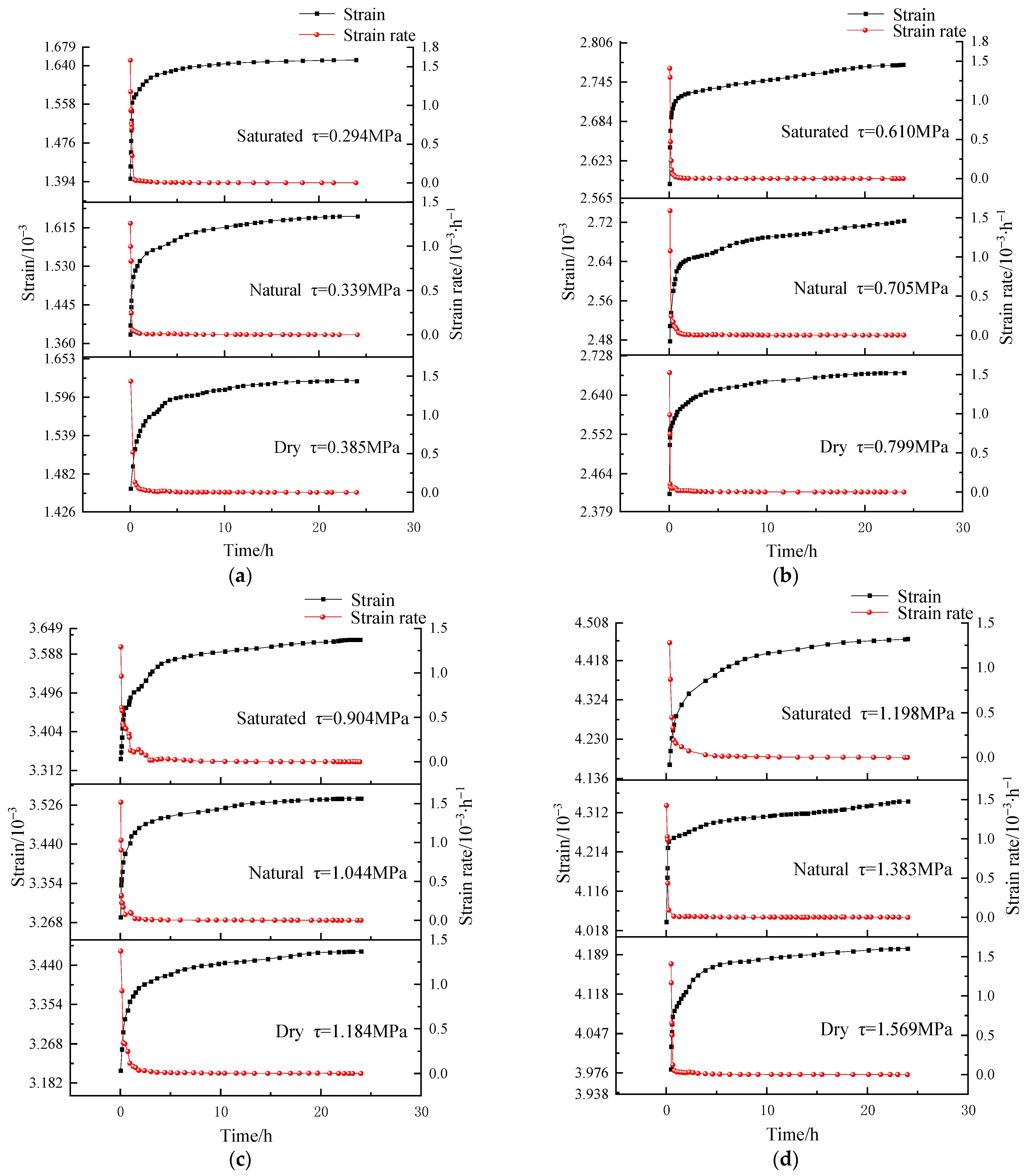
3.2. Analysis of Creep Rate
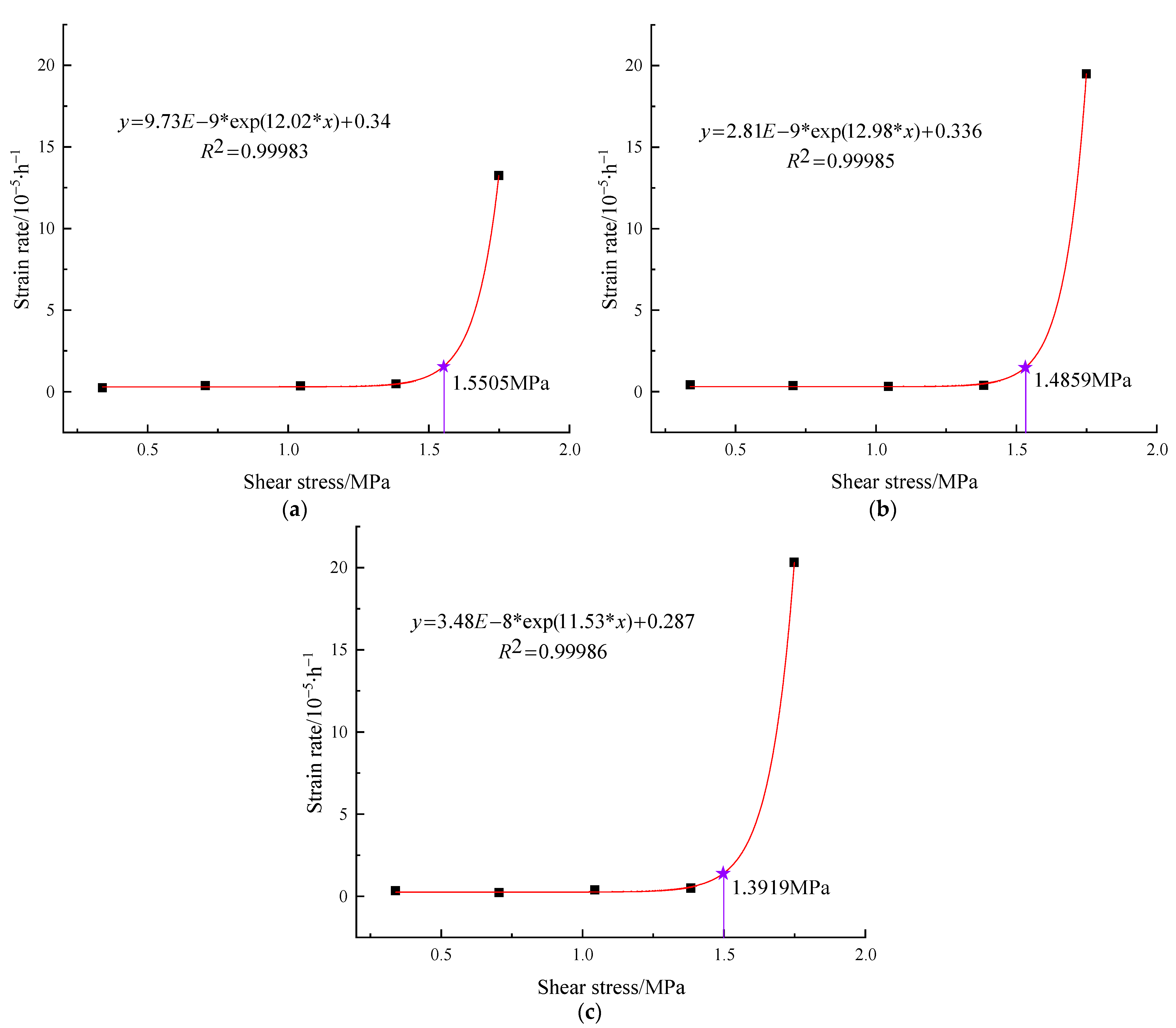
3.3. Analysis of Long-Term Strength
4. Creep Constitutive Model
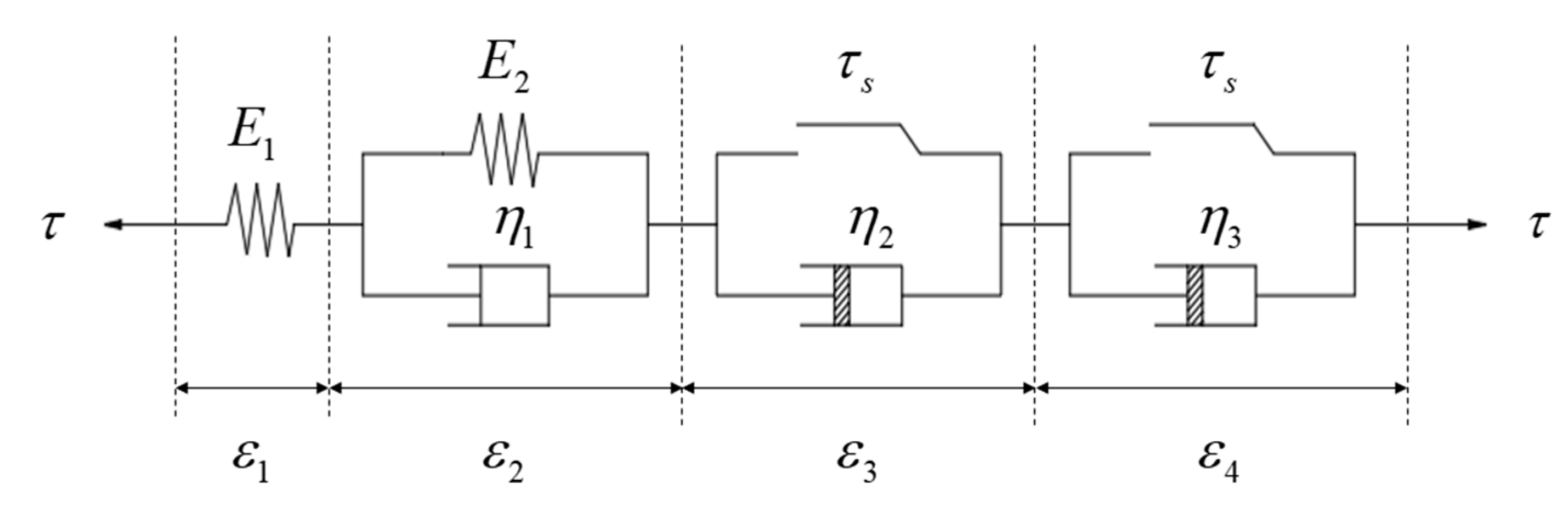
4.1. Constitutive Equation Considering Water Content Damage Under Temperature Cycling
4.2. Establishment of Creep Damage Constitutive Equation
5. Model Validation
6. Conclusions
- Under the influence of temperature cycling, the instantaneous strain generated after each load level applied during the creep process of carbonaceous mud shale significantly increased with rising water content, resulting in a marked reduction in overall creep time. This alteration has important implications for slope stability, particularly in the context of precipitation and seasonal climate changes, where the creep behavior of rock masses may accelerate the occurrence of slope failures.
- In the initial stages, when shear stress is low, the deceleration creep phase of carbonaceous mud shale lasts for a shorter duration. As shear stress progressively increases, the duration of this phase gradually lengthens. Under temperature cycling, the steady-state creep phase of carbonaceous mud shale with higher moisture content progressively shortens. Furthermore, with temperature cycling, the long-term strength of carbonaceous mud shale decreases as moisture content increases. Collectively, these changes contribute to a reduction in the long-term strength of slopes, thereby heightening the risk of landslides.
- Building upon the traditional rheological model, nonlinear Newtonian viscoelasticity is introduced, and corrections are made to the elastic modulus and viscosity coefficient, ultimately leading to the establishment of a shear creep constitutive model for carbonaceous shale that accounts for water content damage. After validation, the fitted curve of the constitutive model closely matches the experimental curve, effectively characterizing the entire shear creep process in carbonaceous shale. The R2 values exceed 0.95, indicating higher fitting accuracy compared to other traditional models, thus addressing the limitation of the original model in representing complex stress damage effects. The successful application of this model can enhance the accuracy of simulating the mechanical behavior of slope rock masses under various environmental conditions, providing theoretical support for the prediction and prevention of slope landslides.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tao, T.; Shi, W.; Xiong, S.; Liang, F.; Zhang, J. Experimental study on deformation and failure of a mining slope under the action of rainfall. Can. Geotech. J. 2023, 61, 1902–1919. [Google Scholar] [CrossRef]
- Li, G.; Hu, Z.; Wang, D.; Wang, L.; Wang, Y.; Zhao, L.; Jia, H.; Fang, K. Instability mechanisms of slope in open-pit coal mines: From physical and numerical modeling. Int. J. Min. Sci. Technol. 2024, 34, 1509–1528. [Google Scholar] [CrossRef]
- He, M.; Feng, J.; Sun, X. Stability evaluation and optimal excavated design of rock slope at Antaibao open pit coal mine, China. Int. J. Rock Mech. Min. Sci. 2008, 45, 289–302. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Li, X.; Tang, S.; Gong, B.; Jiang, S. Evolutionary characteristics of the fracture network in rock slopes under the combined influence of rainfall and excavation. Bull. Eng. Geol. Environ. 2025, 84, 47. [Google Scholar] [CrossRef]
- Yu, M.; Zuo, J.; Sun, Y.; Mi, C.; Li, Z. Investigation on fracture models and ground pressure distribution of thick hard rock strata including weak interlayer. Int. J. Min. Sci. Technol. 2022, 32, 137–153. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Wang, J.; Gong, B.; Li, X. Numerical Simulation of Overburden Deformation Mechanism and Surface Settlement Characteristics Induced by Underground Coal Mining: A Case Study. Geol. J. 2025, 60, 1–18. [Google Scholar] [CrossRef]
- Sun, Q.; Dai, S.; Hao, R.; Xiao, Y. Study on mechanical properties and acoustic emission characteristics of composite rock mass with different thicknesses of weak interlayer. Sci. Rep. 2024, 14, 27835. [Google Scholar] [CrossRef] [PubMed]
- He, L.; Shang, Y.; Lei, X.; Li, G. Destabilization Mechanism and Prevention Technology of Open-Pit Mine Slope with Soft Interlayer Under Rainfall Infiltration Conditions: A Case Study. Min. Met. Explor. 2024, 41, 859–873. [Google Scholar] [CrossRef]
- Li, J.; Hu, B.; Sheng, J.; Zhang, Z. Failure mechanism and treatment of mine Landslide with gently-inclined weak interlayer: A case study of Laoyingzui landslide in Emei, Sichuan, China. Geomech. Geophys. Geo-Energ. Env. 2024, 10, 61. [Google Scholar] [CrossRef]
- Li, J.; Hu, B.; Sheng, J.; Huang, L. A Fractional-Order Creep-Damage Model for Carbonaceous Shale Describing Coupled Damage Caused by Rainfall and Blasting. Fractal Fract. 2024, 8, 459. [Google Scholar] [CrossRef]
- Ma, B.; Zhao, Z.; Li, P.; Liu, Q.; Yang, C.; Li, J.; Luo, W. Shear Creep Properties of Weak Interlayer in Slope Based on Stationary Parameter Fractional Derivative Burgers Model. Geomech. Geophys. Geo-Energ. Env. 2019, 42, 337–348. [Google Scholar] [CrossRef]
- Gao, Y.; Jiang, S.; Xie, J.; Wei, W.; Jiang, Q. Experimental study on the shear creep characteristics of joints under wetting-drying cycles. Int. J. Rock. Mech. Min. Sci. 2023, 169, 105458. [Google Scholar] [CrossRef]
- Gutiérrez-Ch, J.; Senent, S.; Graterol, E.; Zeng, P.; Jimenez, R. Rock shear creep modelling: DEM—Rate process theory approach. Int. J. Rock. Mech. Min. Sci. 2023, 161, 105295. [Google Scholar] [CrossRef]
- Liu, J.; Chang, D.; Yu, Q. Influence of freeze-thaw cycles on mechanical properties of a silty sand. Eng. Geol. 2016, 210, 23–32. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Hou, D.; Jiang, S.; Gong, B.; Li, X. Simulating the Failure Mechanism of High-Slope Angles Under Rainfall-Mining Coupling Using MatDEM. Water 2025, 17, 414. [Google Scholar] [CrossRef]
- Dai, Z.; Huang, K.; Chi, Z.; Chen, S. Model test study on the deformation and stability of rainfall-induced expansive soil slope with weak interlayer. Bull. Eng. Geol. Environ. 2024, 83, 76. [Google Scholar] [CrossRef]
- Kodama, T.; Goto, T.; Fujii, Y.; Hagan, P. The effects of water content, temperature and loading rate on strength and failure process of frozen rocks. Int. J. Rock Mech. Min. Sci. 2013, 62, 1–13. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, C.; Liu, G.; Wu, P.; Cao, P.; Zhang, H. The Influence About Coupled Initial Water Content and Local Expansion on Mechanical Properties and Deformation of the Tunnel in Mudstone. Indian Geotech. J. 2024, 55, 62–78. [Google Scholar] [CrossRef]
- Chen, J.; Jiang, H.; Liu, Y.; Wu, Y.; Zhang, X.; Pan, W. Study on the Influence of Water Content on the Shear Behavior of the Soil–Structure Interface Under a Temperature Field. Buildings 2025, 15, 1. [Google Scholar] [CrossRef]
- Jiang, Z.; Wang, H. Study on Shear Creep Characteristics and Creep Model of Soil-Rock Mixture Considering the Influence of Water Content. Front. Phys. 2022, 10, 819709. [Google Scholar] [CrossRef]
- Zhou, Z.; Cai, X.; Cao, W.; Li, X.; Xiong, C. Influence of Water Content on Mechanical Properties of Rock in Both Saturation and Drying Processes. Rock Mech. Rock Eng. 2016, 49, 3009–3025. [Google Scholar] [CrossRef]
- Bao, M.; Chen, Z.; Zhang, L.; Nian, G.; Zhu, T.; Hao, J. Experimental study on the sliding instability mechanism of slopes with weak layers under creeping action. Measurement 2023, 212, 112690. [Google Scholar] [CrossRef]
- Shi, Z.; Zhao, S.; Qiao, Y.; He, M. Stability analysis of an expansive soil-embankment slope with a soft interlayer. IOP Conf. Ser. Earth Environ. Sci. 2020, 570, 032022. [Google Scholar] [CrossRef]
- Gu, D.; Liu, H.; Gao, X.; Huang, D.; Zhang, W. Influence of cyclic wetting–drying on the shear strength of limestone with a soft interlayer. Rock Mech. Rock Eng. 2021, 54, 4369–4378. [Google Scholar] [CrossRef]
- Gao, Y.; Wei, W.; Hua, D.; Jiang, Q. Study on creep characteristics of mudstone with different initial water contents in soaking conditions and its engineering applications. Bull. Eng. Geol. Environ. 2022, 81, 380. [Google Scholar] [CrossRef]
- Sass, O. Rock moisture measurements: Techniques, results, and implications for weathering. Earth Surf. Process Landf. 2010, 30, 359–374. [Google Scholar] [CrossRef]
- Vásárhelyi, B.; Ván, P. Influence of water content on the strength of rock. Eng. Geol. 2006, 84, 70–74. [Google Scholar] [CrossRef]
- DZ/T 0276.2-2015, Test Code for Physical and Mechanical Properties of Rocks Part 2: Test for Water Content in Rocks [S]. China, 2015.
- Gan, J.K.M.; Fredlund, D.G.; Rahardjo, H. Determination of the shear strength parameters of an unsaturated soil using the direct shear test. Can. Geotech. J. 1988, 25, 500–510. [Google Scholar] [CrossRef]
- Zhang, F.; Jiang, A.; Yang, X. Shear creep experiments and modeling of granite under dry-wet cycling. Bull. Eng. Geol. Environ. 2021, 80, 5897–5908. [Google Scholar] [CrossRef]
- Wang, Y.; Li, J. Investigation on Shear Creep Mechanical Properties of Soft Rock. Adv. Mater. Res. 2011, 1278, 261–263. [Google Scholar] [CrossRef]
- Wang, Y.; Deng, H.; Lu, T.; Zhao, Z. Research on Shear Creep Property of Soft Rock in Dam Foundation. Adv. Mater. Res. 2011, 1269, 243–249. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, H.; Zhang, Y.; Lyu, C.; Liu, Y.; Wang, W. Deterioration Mechanism and Statistical Damage Constitutive Model of Red-Bed Soft Rock with Different Moisture Content. Pure Appl. Geophys. 2024, 2024, 1–20. [Google Scholar] [CrossRef]
- Miao, C.; Jiang, M.; Wang, L.; Yang, J.; Sun, X. Experimental Study on Long-Term Strength and Creep Characteristics of Sandstone Under Different Water Content and Confining Pressure. Rock Mech. Rock Eng. 2024, 57, 9035–9054. [Google Scholar] [CrossRef]
- Arutyunyan, A.R.; Saitova, R.R. Exact and approximate solutions of the system of interrelated equations of the theory of creep and long-term strength. J. Phys. Conf. Ser. 2022, 2231, 012011. [Google Scholar] [CrossRef]
- Yang, S.; Cheng, L. Non-stationary and nonlinear visco-elastic shear creep model for shale. Int. J. Rock Mech. Min. Sci. 2011, 48, 1011–1020. [Google Scholar] [CrossRef]
- Bao, M.; Chen, Z.; Nian, G.; Zhang, L.; Zhu, T. Study on creep properties of weak layer rock of slope under damage coupling. Bull. Eng. Geol. Environ. 2024, 83, 154. [Google Scholar] [CrossRef]
- Wang, J.; Zheng, J.; Deng, J.; Yu, Y.; Zhu, J.; Huang, X. Effect of moisture content, temperature, and microstructure on the thermal conductivity of loess and its prediction model. Bull. Eng. Geol. Environ. 2024, 83, 206. [Google Scholar] [CrossRef]
- Weng, X.; Hou, L.; Li, X.; Li, Z.; Zhou, R. Three-Dimensional Numerical Realization And Application Of Collapsible Loess Constitutive Model Considering Initial Water Content. Geotech. Geol. Eng. 2024, 43, 15. [Google Scholar] [CrossRef]
- Wang, W.; Yao, Q.; Tang, C.; Li, H.; Liu, H.; Shan, C. Mechanical Properties Damage, Fracture Evolution, and Constitutive Model of Siltstone under the Effect of Moisture Content. Geofluids 2022, 2022, 8599808. [Google Scholar] [CrossRef]
- Xu, M.; Jin, D.; Song, E.; Shen, D. A rheological model to simulate the shear creep behavior of rockfills considering the influence of stress states. Acta Geotech. 2018, 13, 1313–1327. [Google Scholar] [CrossRef]
- Deng, P.; Liu, Q.; Lu, H. FDEM numerical study on the mechanical characteristics and failure behavior of heterogeneous rock based on the Weibull distribution of mechanical parameters. Comput. Geotech. 2023, 154, 105138. [Google Scholar] [CrossRef]
- Zhu, Z.; Tian, H.; Wang, R.; Jiang, G.; Dou, B.; Mei, G. Statistical thermal damage constitutive model of rocks based on Weibull distribution. Arab. J. Geosci. 2021, 14, 495. [Google Scholar] [CrossRef]
- Wang, D.; Chen, X.; Qi, J.; Peng, L. Assessment on strength reduction schemes for geotechnical stability analysis involving the Drucker-Prager criterion. J. Cent. South Univ. 2021, 28, 3238–3245. [Google Scholar] [CrossRef]
- Wang, B.; Wang, F.; Wang, Q. Damage constitutive models of concrete under the coupling action of freeze–thaw cycles and load based on Lemaitre assumption. Constr. Build. Mater. 2018, 173, 332–341. [Google Scholar] [CrossRef]
- Yan, S.; Hu, B.; Wei, E.; Ma, L.; Lei, T. Analysis of shear creep characteristics of carbonaceous mud shale in Huangshan limestone mining area under osmotic pressure. China Min. Mag. 2023, 32, 245–252. (In Chinese) [Google Scholar] [CrossRef]
- Yin, W.; Wang, X.; Zheng, S.; Zhang, K.; Zhang, F. Triaxial creep test and damage model study of layered red sandstone under freeze-thaw cycles. Case Stud. Constr. Mater. 2024, 21, e03785. [Google Scholar] [CrossRef]
- Wang, W.; Yao, Q.; Tang, C.; Li, X.; Chong, Z.; Xu, Q. Experimental study on the shear characteristics and weakening mechanism of water-bearing rock joints. Bull. Eng. Geol. Environ. 2021, 80, 7653–7668. [Google Scholar] [CrossRef]





| Rock Sample | Dry | Natural | Saturated |
|---|---|---|---|
| Sample number | S-01 | S-02 | S-03 |
| moisture content/% | 0 | 1.5 | 3.2 |
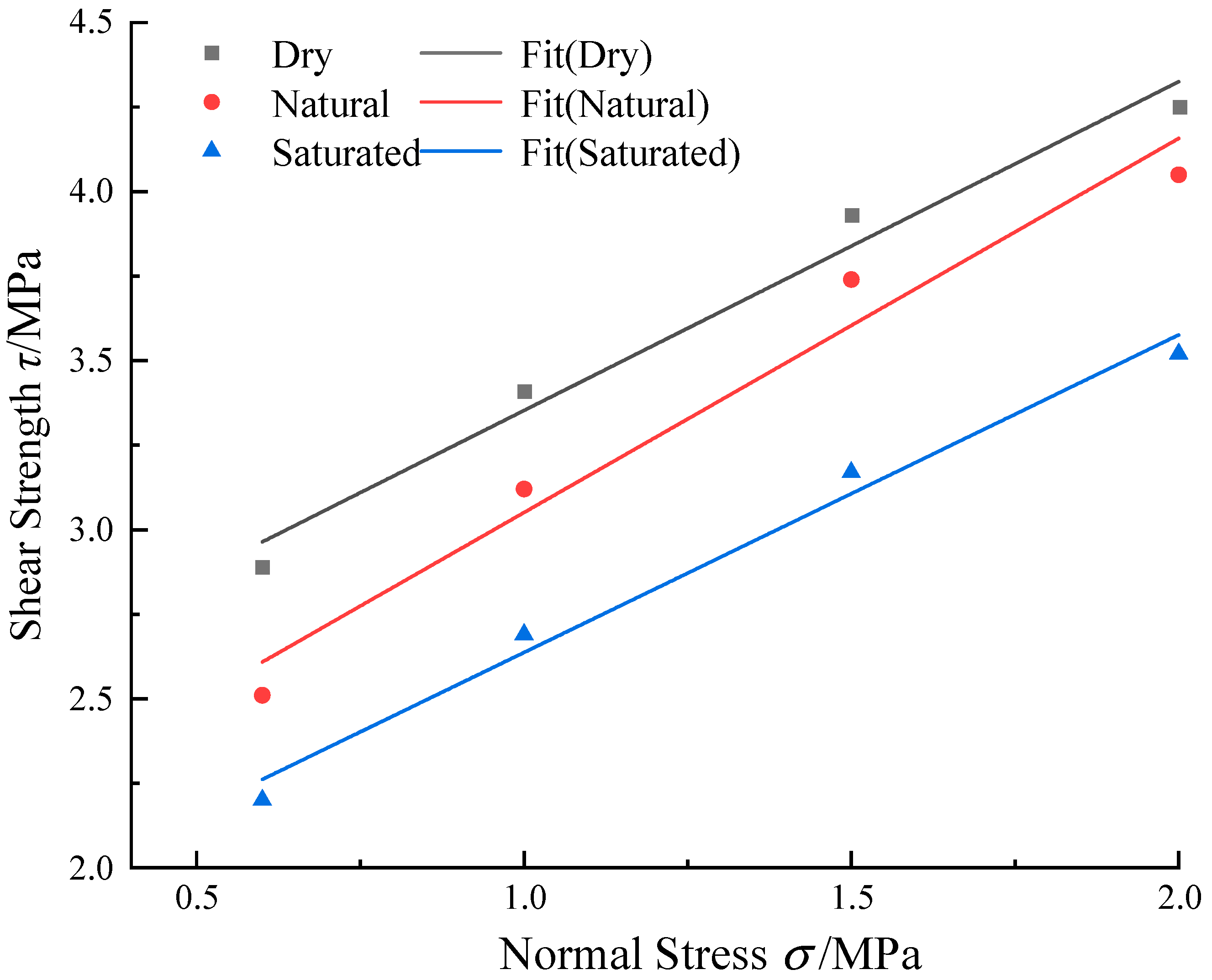
| Moisture Content | Normal Stress σ/MPa | Fitted Curve | Shear Strength τ/MPa | Cohesion C/MPa | /° |
|---|---|---|---|---|---|
| Dry | 0.6 | 2.96 | 2.25 | ||
| 1.0 | 3.35 | ||||
| 1.5 | 3.83 | ||||
| 2.0 | 4.32 | ||||
| Natural | 0.6 | 2.61 | 1.59 | 44.02 | |
| 1.0 | 3.05 | ||||
| 1.5 | 3.60 | ||||
| 2.0 | 4.16 | ||||
| Saturated | 0.6 | 2.26 | |||
| 1.0 | 2.63 | ||||
| 1.5 | 3.10 | ||||
| 2.0 | 3.57 |
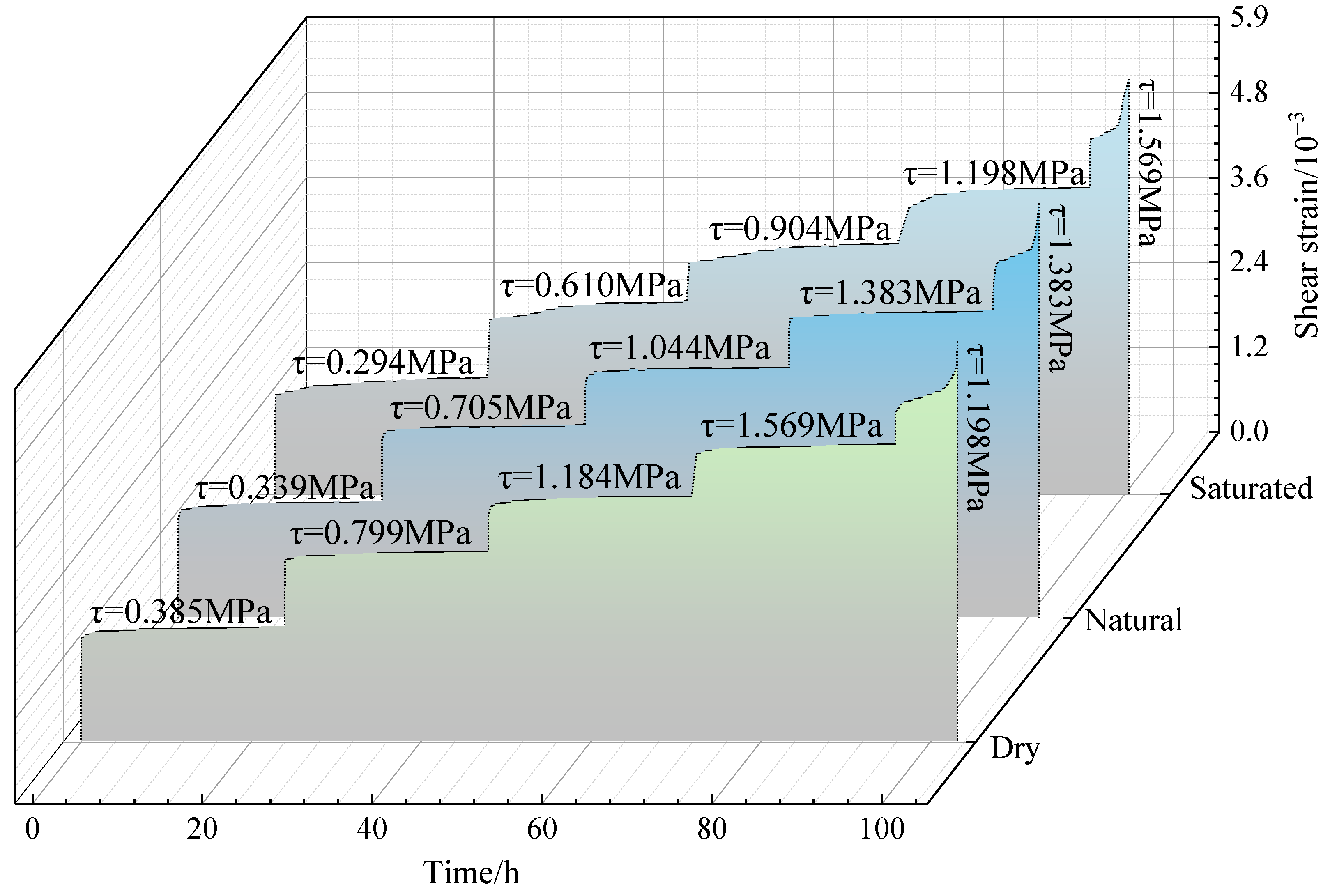
| Rock Sample | Number of Cycles | Normal Stress σ/MPa | Shear Strength τ/MPa | Moisture Content | Level 1 Shear Stress/MPa | Level 2 Shear Stress/MPa | Level 3 Shear Stress/MPa | Level 4 Shear Stress/MPa | Level 5 Shear Stress/MPa |
|---|---|---|---|---|---|---|---|---|---|
| S-01 | 16 | 0.6 | 3.35 | 0 | 0.385 | 0.799 | 1.184 | 1.569 | 1.983 |
| S-02 | 3.83 | 1.5 | 0.339 | 0.705 | 1.044 | 1.383 | 1.749 | ||
| S-03 | 4.32 | 3.2 | 0.294 | 0.610 | 0.904 | 1.198 | 1.514 |
| Moisture Content | Shear Stress | Instantaneous Elastic Modulus /GPa | Viscoelastic Modulus /GPa | Viscosity Coefficient /MPa∙h | Viscosity Coefficient /MPa∙h | Viscosity Coefficient /MPa∙h | Coefficient of Thermal Expansion /℃−1 | ||
|---|---|---|---|---|---|---|---|---|---|
| Dry | 0.385 | 0.834 | 8.582 | 646.96 | 17.57 | 1107.60 | 0.39 | 5.56 × 10−6 | 0.981 |
| 0.799 | 1.039 | 10.022 | 599.03 | 0.45 | 5.18 × 10−6 | 0.975 | |||
| 1.184 | 0.768 | 17.494 | 437.34 | 0.44 | 6.96 × 10−6 | 0.979 | |||
| 1.569 | 1.068 | 46.630 | 578.56 | 0.61 | 3.55 × 10−6 | 0.953 | |||
| 1.983 | 0.689 | 39.198 | 391.80 | 0.50 | 5.57 × 10−6 | 0.969 | |||
| Natural | 0.339 | 0.572 | 6.880 | 587.52 | 15.26 | 998.31 | 0.57 | 5.74 × 10−6 | 0.985 |
| 0.705 | 0.663 | 12.525 | 415.79 | 0.89 | 5.89 × 10−6 | 0.957 | |||
| 1.044 | 0.483 | 15.291 | 392.17 | 0.62 | 6.22 × 10−6 | 0.971 | |||
| 1.383 | 0.802 | 26.835 | 326.11 | 0.74 | 6.85 × 10−6 | 0.962 | |||
| 1.749 | 0.594 | 32.749 | 500.98 | 1.03 | 7.42 × 10−6 | 0.980 | |||
| Saturated | 0.294 | 0.593 | 5.551 | 547.93 | 12.39 | 726.53 | 0.81 | 4.61 × 10−6 | 0.992 |
| 0.610 | 0.780 | 17.546 | 681.93 | 0.66 | 5.71 × 10−6 | 0.931 | |||
| 0.904 | 0.318 | 14.432 | 316.87 | 0.85 | 6.17 × 10−6 | 0.958 | |||
| 1.198 | 0.454 | 20.844 | 259.46 | 1.27 | 6.95 × 10−6 | 0.981 | |||
| 1.514 | 0.635 | 27.722 | 289.79 | 1.42 | 7.43 × 10−6 | 0.983 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, F.; Hu, B.; Zhong, Z.; Zhang, H.; Li, H. Study on Shear Creep Characteristics of Carbonaceous Mud Shale at Different Moisture Contents Under Temperature Cycling. Water 2025, 17, 567. https://doi.org/10.3390/w17040567
Xue F, Hu B, Zhong Z, Zhang H, Li H. Study on Shear Creep Characteristics of Carbonaceous Mud Shale at Different Moisture Contents Under Temperature Cycling. Water. 2025; 17(4):567. https://doi.org/10.3390/w17040567
Chicago/Turabian StyleXue, Fei, Bin Hu, Zhuoxi Zhong, Hongjun Zhang, and Haiying Li. 2025. "Study on Shear Creep Characteristics of Carbonaceous Mud Shale at Different Moisture Contents Under Temperature Cycling" Water 17, no. 4: 567. https://doi.org/10.3390/w17040567
APA StyleXue, F., Hu, B., Zhong, Z., Zhang, H., & Li, H. (2025). Study on Shear Creep Characteristics of Carbonaceous Mud Shale at Different Moisture Contents Under Temperature Cycling. Water, 17(4), 567. https://doi.org/10.3390/w17040567






