Hydrodynamic Optimization of Non-Pressurized Tunnel Intersection of Pumped Storage Power Station

Abstract

The geometry of non-pressurized tunnel intersections governs the hydraulic behavior of the confluence flows, which are critical to the safe operation of pumped storage power stations. To address the issue of water surface levels exceeding the permissible height of the vertical walls at the intersection of the sediment discharge and emptying tunnels close to the lower reservoir of a pumped storage power station, a hydraulic model with a scale of 1:45 was constructed to optimize the intersection design. The optimization process included replacing the straight connection with an arc connection, incorporating an energy dissipation basin into the emptying tunnel, reducing the intersection angle, and increasing the arc radius. During the optimization, the hydraulic behavior of the confluence flow was thoroughly analyzed. This study determined that an arc connection with a 21° intersection angle represented the optimal design. Using the RNG k-ε turbulence model and the volume-of-fluid (VOF) method, a three-dimensional (3D) numerical model was developed to further evaluate the flow patterns, velocity fields, and bottom pressure distributions under both the optimized-design and model-verification conditions. The numerical simulation results, validated against experimental data, exhibited close agreement. The findings demonstrate that the optimized design ensures compliance with specifications, as the maximum water depth no longer exceeds the height of the straight walls. This study offers valuable insights for optimizing tunnel intersections of high-elevation-difference non-pressurized tunnels in pumped storage power stations.

1. Introduction

Efforts to reduce greenhouse gas emissions and promote sustainable development have accelerated the planning and construction of pumped storage power stations in China, supported by favorable policies [1]. Known for their significant lifecycle carbon reduction benefits, pumped storage power stations play a crucial role in modern sustainable energy systems. However, the sediment discharge tunnels and emptying tunnels of these stations operate under challenging conditions, characterized by high water heads, high flow velocities, and complex hydraulic dynamics. Numerous studies have examined the hydraulic challenges associated with flood discharge tunnels [2,3,4,5,6], with particular attention to the cross-arrangement of tunnels.

Spiral flow is often observed in confluence flows at tunnel intersections due to intense water mixing. The interaction between tributary and main flows leads to abrupt changes in streamlines, vortex formation, and significant hydraulic disturbances downstream. Previous research has primarily focused on areas such as flow structures [7,8,9,10,11,12,13,14,15,16,17], hydrodynamic characteristics [18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34], and the effects of confluence angles and flow ratios on hydraulic performance in rivers and open channels [35,36,37,38,39,40,41]. However, studies specifically addressing confluence flow dynamics in non-pressurized tunnels, particularly under high-head and high-velocity conditions, remain limited.

Zhang [42] analyzed the hydraulic behavior of confluence flows at intersections of non-pressurized tunnels in small-slope tailing ponds, focusing on confluence ratios and intersection angles. Yang [43] investigated how openings at the top of branch tunnels influence hydraulic behavior, including flow velocity, pressure, and water depth. Du et al. [44] studied flow patterns, backwater height, and pressure distribution in confluence areas of main and branch tunnels in tailing-pond flood discharge systems under adverse conditions.

While these studies provide valuable insights, they primarily address scenarios involving low flow rates in tailing ponds. Qiu et al. [45] explored the impact of energy dissipation basins in branch tunnels on hydraulic behavior in confluence areas. Liu et al. [46] proposed installing arc-shaped baffles on the tunnel roof downstream of confluence sections to mitigate the impact of high-velocity flows on tunnel structures. Li et al. [47] evaluated the effects of baffles with varying heights on downstream hydraulic behavior through model testing.

In general, most research has focused on low-flow systems and seldom considers the challenges posed by high-head, high-velocity conditions in pumped storage systems. This study examines the hydraulic behavior of confluence flows in sediment discharge and emptying tunnels of the lower reservoir in a pumped storage power station. A scaled hydraulic model (1:45) was developed to optimize the intersection design, followed by numerical simulations to analyze hydraulic behavior under both design and extreme flow conditions. The findings provide practical guidance for optimizing intersection shapes and ensuring the safe operation of sediment discharge and emptying tunnels in high-head, high-flow pumped storage systems.

2. Project Overview

The sediment discharge tunnel and emptying tunnel of a pumped storage power station in Tianshui, Gansu Province, China, are arranged to merge at the straight section, as shown in Figure 1. There is a tunnel to discharge the flood and sediment from the reservoir, named the sediment discharge tunnel, as shown in Figure 2. The tunnel has a total length of 1780 m, with an inlet floor elevation of 1782 m. There is another tunnel to discharge the flood, maintenance, and emergency emptying of the reservoir, named the emptying tunnel, as shown in Figure 3. The emptying tunnel measures 750.6 m in length, with an inlet floor elevation of 1730 m. The elevation at the intersection of the two tunnels is 1659.66 m, while the outlet elevation is 1649.26 m.

Bottom-flow energy dissipation is implemented downstream of the outlet to manage hydraulic forces effectively. Both tunnels feature a circular-arch and straight-wall cross-sectional design, with dimensions of 6 m × 8 m (width × height) after the confluence.

3. Methodology

This study begins with a standard physical model to evaluate whether the original design meets the required hydraulic specifications. If the design does not meet the specifications, optimization of the shape is initiated. Subsequently, a numerical model is developed to investigate the hydraulic behavior of the optimized design. The accuracy of the numerical model is validated through comparisons with results from the physical model tests. Finally, the experimental data are analyzed. The overall research framework is illustrated in Figure 4.

3.1. Experimental Methods

3.1.1. Physical Experiment

The hydraulic model test was conducted at the Water Conservancy Hall of the Yellow River Conservancy Technical Institute, China. The model was designed based on the gravity similarity criterion, using a full-scale normal model with a length scale of 1:45. The key parameters and their corresponding scaling factors are listed in

Table 1. Scaling parameters.

Scale Name	Calculation Formula	Adopted Value
Geometric scale	λL	45
Flow rate scale	${\mathrm{\lambda }}_{\mathrm{L}}^{5/2}$	13,584.11
Velocity scale	${\mathrm{\lambda }}_{\mathrm{L}}^{1/2}$	6.71
Roughness scale	${\mathrm{\lambda }}_{\mathrm{L}}^{1/6}$	1.89

.

The sediment discharge and emptying tunnels were constructed from transparent plexiglass to allow clear observation of hydraulic elements and flow patterns. To enhance visual access, the tops of the tunnels were left open [48]. The hydraulic model setup is shown in Figure 5.

The flow rate was measured using an electromagnetic flowmeter with an accuracy of ±0.5%. Water surface profiles were recorded using a movable measuring needle with an accuracy of ±0.5 mm, while flow velocities were measured with a propeller current meter with an accuracy of ±2%.

3.1.2. Numerical Experiment

In this study, the OpenFOAM-v2306 software package was used for three-dimensional (3D) numerical simulations [34,49], employing the RNG k-ε turbulence model. The fundamental governing equations are provided in Equations (1)–(4):

Continuity equation:

$${\displaystyle \frac{\partial \rho }{\partial t}}+{\displaystyle \frac{\partial \rho u_i}{\partial x_i}}=0$$

(1)

Momentum equation:

$${\displaystyle \frac{\partial \rho u_i}{\partial \mathrm{t}}}+{\displaystyle \frac{\partial }{\partial x_j}}\rho u_i{u}_j=-{\displaystyle \frac{\partial p}{\partial x_i}}+{\displaystyle \frac{\partial }{\partial x_j}}\left[\left(\mu +{\mu }_t\right)\left({\displaystyle \frac{\partial u_i}{\partial x_j}}+{\displaystyle \frac{\partial u_j}{\partial x_i}}\right)\right]$$

(2)

Turbulent kinetic energy k equation:

$${\displaystyle \frac{\partial \left(\rho k\right)}{\partial t}}+{\displaystyle \frac{\partial \left(\rho u_{i}k\right)}{\partial x_i}}=-{\displaystyle \frac{\partial }{\partial x_i}}\left[\left(\mu +{\displaystyle \frac{{\mu }_i}{{\sigma }_k}}\right){\displaystyle \frac{\partial \mathrm{k}}{\partial x_i}}\right]+G_k-\rho \epsilon$$

(3)

Turbulence dissipation rate ε equation:

$${\displaystyle \frac{\partial \left(\rho \epsilon \right)}{\partial t}}+{\displaystyle \frac{\partial \left(\rho u_i\epsilon \right)}{\partial x_j}}=-{\displaystyle \frac{\partial }{\partial x_i}}\left[\left(\mu +{\displaystyle \frac{{\mu }_t}{{\sigma }_{\epsilon }}}\right){\displaystyle \frac{\partial \epsilon }{\partial x_i}}\right]+C_{1\epsilon }\rho {\displaystyle \frac{\mathrm{\epsilon }}{\mathrm{k}}}{G}_k-{\mathrm{C}}_{2\mathrm{\epsilon }}\rho {\displaystyle \frac{{\mathrm{\epsilon }}^2}{\mathrm{k}}}$$

(4)

where ${\mu }_t=C_{\mu }{\displaystyle \frac{{\mathrm{k}}^2}{\mathrm{\epsilon }}}$, $C_{1\mathrm{\epsilon }}=1.42-{\displaystyle \frac{\mathrm{\eta }(1-\eta /{\eta }_0)}{1+\beta {\mathrm{\eta }}^3}}$, $\eta =Sk/\mathrm{\epsilon }$, $S=\sqrt{2\overline{S_{ij}{S}_{ij}}}$, $G_k=2\rho C_{\mu }{\displaystyle \frac{{\mathrm{k}}^2}{\mathrm{\epsilon }}}\left(S_{ij}{S}_{ij}\right)$, $S_{ij}=0.5\left(\partial u_i/\partial x_j+\partial u_j/\partial x_i\right)$, ρ is density (kg/m³), t is time (s), u_i is the flow velocity component (m/s), x_i is the coordinate component (m), p is the pressure (Pa), μ is the viscosity coefficient (Pa·s), μ_t is the turbulent viscosity coefficient (Pa·s), σ_k and σ_ε are the Prandtl numbers of k and ε turbulence, respectively, and G_k is the turbulent kinetic energy induced by the flow velocity gradient. The values of the model parameters are as follows: σ_k = 0.7179; σ_ε = 0.7179; C_2ε = 1.92; C_μ = 0.09; η₀ = 4.38; and β = 0.009.

The numerical model utilized a coordinate system with its origin located at the junction of the right inlet wall and the bottom plate of the sediment discharge tunnel. In this system, the positive x-axis was aligned with the flow direction of the sediment discharge tunnel, the y-axis extended perpendicular to the flow direction toward the left-hand side wall, and the z-axis pointed upward, perpendicular to the bottom plate.

The pressure inlet boundaries were defined at the inlets of the sediment discharge and emptying tunnels, while the non-pressurized tunnel section was designated as a pressure outlet. The upper surface of the model region was treated as an air inlet, maintained at an absolute pressure of 1 standard atmosphere (atm). The overall configuration of the numerical model is shown in Figure 6a.

To resolve the flow dynamics, an unstructured mesh grid was employed, with boundary layer refinement applied near wall surfaces to improve accuracy in regions of high gradients. Additionally, a structured grid with three layers was configured, incorporating a growth ratio of 0.69.

To balance computational efficiency and accuracy, a grid independence analysis was performed by comparing computational times and results for minimum grid cell sizes of 0.01 m, 0.03 m, 0.05 m, and 0.10 m. Based on the maximum flow velocity corresponding to the smallest grid size [22], a minimum grid size of 0.03 m was selected as the optimal compromise, ensuring sufficient precision while minimizing computational cost.

To further enhance resolution in critical areas, additional refinement to 0.01 m was applied within the intersection zones, where complex flow dynamics occur. The final grid consisted of 6,754,230 nodes and 1,261,773 elements, as shown in Figure 6b.

The computational domain was discretized using the finite volume method [34], and the PISO algorithm was applied for pressure-velocity coupling. The second-order upwind scheme solved the momentum equations, while the first-order upwind scheme was used for turbulence kinetic energy and dissipation rates. Temporal discretization followed the first-order implicit scheme, with a convergence residual threshold set to 0.001. To accurately track the air–water interface and compute the water surface profile, the volume of fluid (VOF) method was applied [49] for precise simulation of free surface dynamics.

3.2. Optimization Plans

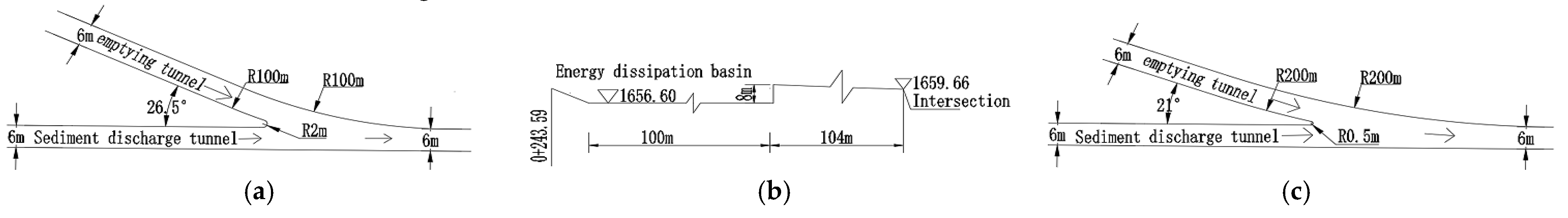
Based on the station’s operational scheduling requirements, the design and verification conditions were established, as shown in

Table 2. Test conditions.

Working Condition	Return Period of Flood (Years)	Flow Rate (m3·s−1)		Reservoir Water Level (m)
		Sediment Discharge Tunnel	Emptying Tunnel	Sediment Discharge Tunnel	Emptying Tunnel
Design	200	141	85.7	1790.58	1772.38
Verification	1000	214.4	139.1	1791.6	1772.7

. The original intersection layout featured a 26.5° intersection angle with a linear connection, as illustrated in Figure 1. A significant issue was identified in the intersection area, where the flow surged against the right straight wall, causing the maximum water depth to exceed the wall height of 6 m. This violated the “Hydraulic Tunnel Design Code” (NB/T 10391-2020) [50], which stipulates that the water surface should not rise above the height of the straight wall in sections designed with a circular-arch straight wall. This issue posed a potential risk to the safe operation of non-pressurized tunnels.

To resolve this problem, three optimization schemes for the intersection shape were proposed as follows:

• Modifying the straight-line connection to an arc-shaped connection.
• Adding an energy dissipation basin to the emptying tunnel.
• Reducing the intersection angle and increasing the arc radius.

The layouts and specific dimensions of the three optimized shapes are shown in Figure 7a–c.

4. Results and Analysis

4.1. Physical Test Results of the Confluence Zone

4.1.1. Flow Patterns

The flow patterns for shape (a) under both design and verification conditions are shown in Figure 8. The circular connection gradually increased the intersection width, reducing the impact of the emptying tunnel flow on the main flow within the sediment discharge tunnel. While this design improved flow conditions compared to the original linear connection, the water flow still reached the top under verification conditions. To further mitigate the flow velocity before entering the sediment discharge tunnel, shape (b) introduced an energy dissipation basin in the emptying tunnel (see Figure 7b).

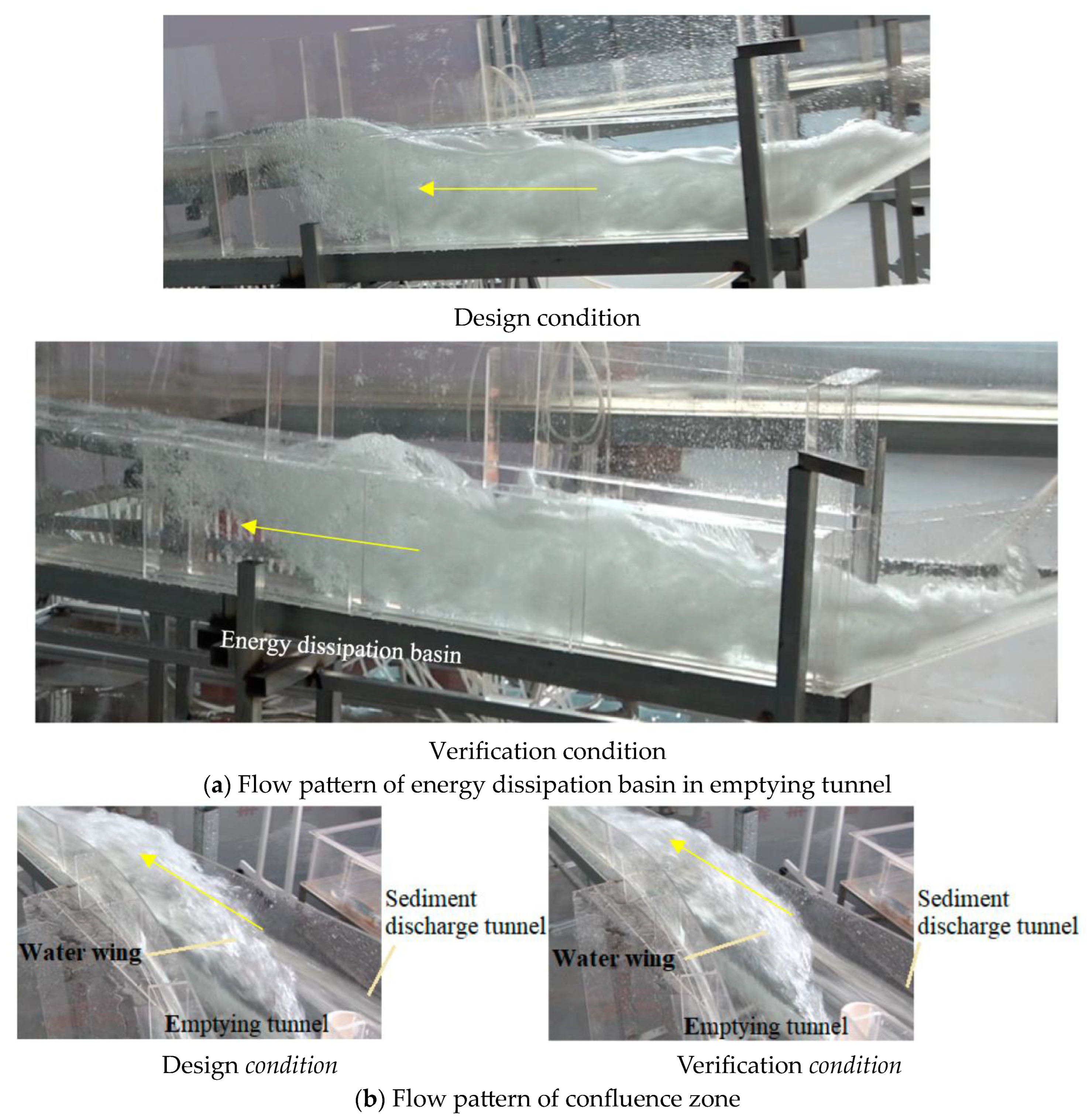
The flow patterns within the energy dissipation basin of the emptying tunnel for shape (b) under both design and verification conditions are presented in Figure 9a, while the flow patterns in the confluence area are shown in Figure 9b. As seen in Figure 9a, the significant water level drop in the emptying tunnel and the high flow velocity entering the energy dissipation basin create rapid and energetic flow. Near the end of the basin, the flow slows significantly, forming a hydraulic jump. This jump generates highly turbulent and mixed flow, accompanied by vigorous surface fluctuations. Under verification conditions, the maximum water depth within the energy dissipation basin exceeds the wall height by approximately 1 m.

Figure 9b illustrates that under both design and verification conditions, the flow in the confluence area remains highly turbulent and mixed. The water surface exhibits pronounced left-right oscillations, with the flow predominantly biased toward the right side.

The flow patterns for shape (c) under both design and verification conditions are shown in Figure 10. With a reduced intersection angle and an increased circular radius, the impact of the water flow in the emptying tunnel on the main flow in the sediment discharge tunnel is significantly reduced. The elevated flows shift leftward before rebounding and impacting the right wall.

Under all three shapes, the water surface of the confluence flow rises due to the impact. The Froude number (Fr) at the intersection area exceeds 1 (

Table 3. Entrance condition of intersection area.

Working Conditions	Shape	Intersection Angle (Degrees)	Water Depth/m		Flow Velocity/(m·s−1)		Momentum Ratio	Fr		Confluence Ratio
			Emptying Tunnel	Sediment Discharge Tunnel	Emptying Tunnel	Sediment Discharge Tunnel		Emptying Tunnel	Sediment Discharge Tunnel
Design	(a)	26.5	1.26	1.72	12.37	14.69	0.51	3.52	3.58	0.61
	(b)	26.5	1.65	1.70	8.05	14.63	0.33	2.00	3.58	0.61
	(c)	21.0	1.17	1.71	13.26	14.71	0.55	3.92	3.59	0.61
Verification	(a)	26.5	1.73	2.38	14.23	16.05	0.58	3.46	3.32	0.65
	(b)	26.5	2.18	2.38	10.14	16.14	0.41	2.19	3.34	0.65
	(c)	21.0	1.61	2.39	15.16	16.11	0.61	3.82	3.33	0.65

Remarks: momentum ratio = momentum of the emptying tunnel/momentum of the sediment discharge tunnel. Confluence ratio = flow of the emptying tunnel/flow of the sediment discharge tunnel.

), indicating supercritical flow. For shapes (a) and (b), the elevated confluence flow is skewed toward the right sediment discharge tunnel, compressing the main flow and causing high-velocity flow to collide with the right straight wall. In contrast, the flow pattern for shape (c) differs significantly: the elevated confluence flow initially deflects toward the left wall before rebounding to impact the right wall. The reduced intersection angle in shape (c) helps mitigate the impact of the emptying tunnel flow on the main flow in the sediment discharge tunnel. Thus, the intersection angle plays a critical role in influencing the flow pattern in the confluence area.

4.1.2. Water Depth and Velocity

For all three shapes, the maximum water depth was observed at the right wall. Shapes (a) and (b) exhibited maximum water depths of 6.75 m and 6.89 m, respectively, both exceeding the permissible wall height of 6 m specified by the Code for Hydraulic Tunnel Design [50]. Shape (b) further increased the water depth compared to shape (a), despite reducing the flow velocity in the confluence area under both design and verification conditions. However, the Froude number (Fr) remained greater than 1, indicating supercritical flow (Table 3). As a result, the maximum water depth along the right wall in the confluence region for shape (b) was higher than that for shape (a).

In the energy dissipation basin, the water flow is highly turbulent, and significant surface fluctuations cause the water surface to exceed the permissible wall height of 6 m. The addition of an energy dissipation basin to the high-drop, high-velocity emptying tunnel in this study does not resolve the issue of water overtopping the right vertical wall in the intersection area. Whether an energy dissipation basin can improve flow conditions in the confluence area for low-drop, low-velocity tunnels requires further analysis, depending on specific engineering contexts.

Shape (c) effectively addressed the issue, with a maximum water depth of 5.13 m under verification conditions, meeting the specification requirements. Compared to shapes (a) and (b), shape (c) exhibited a reduced maximum water depth at the right wall, although the width of the maximum water depth distribution zone along the flow direction increased.

Unlike the flow patterns observed in open-channel confluence zones [23], the confluence region of the high-drop convergence tunnels in this study displays distinct characteristics for all three shapes under non-pressurized flow. These include a high-velocity zone on the right side, a relatively low-velocity zone on the left side, a flow recovery zone downstream of the confluence, and water wings. Notably, no backflow was observed. For shape (c), the velocity distribution in the confluence and downstream areas near the left and right vertical walls under both design and verification conditions is shown in Figure 11.

As shown in Figure 11, the flow velocity near the right vertical wall in the confluence area is higher than near the left wall under both conditions. This is due to the inflow from the emptying outlet, which exerts a blocking and impinging effect on the main flow in the sediment discharge tunnel, causing water flow contraction and increased velocity on the right side of the confluence. Downstream, at a section 150 m from the confluence, the velocity distribution near the left and right walls becomes more uniform.

4.2. Numerical Simulation Results for Different Intersection Shapes

The optimized intersection shape (c) effectively resolved the issue of the maximum water depth exceeding the 6 m wall height observed in the original design. To gain deeper insights into the hydraulic behavior of the confluence area, a 3D numerical model, reflecting the prototype dimensions, was developed. The boundary conditions for both the design and verification conditions are outlined in

Table 4. Boundary conditions.

Location	Boundary Condition Type	Design Condition	Verification Condition
Inlet of sediment discharge tunnel	Water level (m)	1662.406	1663.486
	flow velocity (m/s)	16.62	17.92
Inlet of emptying tunnel	Water level (m)	1662.96	1663.32
	flow velocity (m/s)	12.23	16.71
Outlet of sediment discharge tunnel	Flow rate (m3/s)	226.7	353.5

.

4.2.1. Water Surface Profile

The water surface profile is a crucial indicator for evaluating the effectiveness of the optimized intersection shape and the rationality of the resulting flow pattern. Figure 12a presents the simulated water surface profiles along the left and right walls in the confluence area under the verification condition, while Figure 12b compares the simulated and experimental values of the water surface profile along the right wall.

A comparison between Figure 10 and Figure 12a reveals that the numerical simulation accurately captures the fluctuations in water levels along both walls, showing consistent trends. This alignment highlights the simulation’s effectiveness in reflecting the complex flow dynamics in the confluence area.

Figure 12b illustrates that the simulated water surface profile along the right wall is higher than the physical model result. The intense mixing and complex flow near the intersection pier result in rapid flow changes, which may cause deviations in the experimental measurements. In the mixing zone, approximately 60 m downstream of the intersection pier, the average relative error between the simulated and experimental water depths is 14.95%. However, further downstream in the confluence area, the agreement between the simulation and experimental results improves significantly, with an average relative error of 4.66%. Overall, the numerical simulation aligns well with the physical model test results.

Both the numerical and physical model results confirm that, under design and verification conditions for the optimized shape (c), the maximum water depth remains below the 6 m wall height, meeting the requirements of the Code for Hydraulic Tunnel Design [50]. This validates the effectiveness of the optimized intersection shape, which features an intersection angle of 21° and circular connections.

4.2.2. Cross-Sectional Flow Velocity

Table 5. Comparison of maximum section velocities.

x/m	Design Condition			Verification Condition
	Model Test Value (m s−1)	Simulated Value (m s−1)	Error (%)	Model Test Value (m s−1)	Simulated Value (m s−1)	Error (%)
60	14.892	13.818	7.21	17.056	17.682	3.67
127	14.209	13.401	5.69	17.661	16.202	8.26
166	14.750	13.837	6.19	16.720	16.872	0.91
205	13.720	14.191	3.44	16.759	16.791	0.19
293	14.814	14.988	1.17	16.129	16.304	1.09

compares the maximum flow velocities between the simulated and experimental values under both design and verification conditions. The analysis shows that the maximum relative error in flow velocity, 8.26%, occurs at the intersection of the sediment discharge and emptying tunnels (x = 60 m and x = 127 m). This area experiences intense mixing and rapid flow changes, which contribute to larger discrepancies between the simulated and experimental results. In contrast, the relative error decreases significantly in the downstream recovery zone, where flow conditions stabilize. The average relative errors for the design and verification conditions are 4.74% and 2.82%, respectively, indicating good overall agreement between the simulated and experimental flow velocity data.

Figure 13 shows the velocity contours and streamlines near the bottom surface under both design and verification conditions. After entering the confluence, the water flow from the emptying tunnel compresses and interacts with the main flow in the sediment discharge tunnel, causing the streamlines to slightly curve while maintaining an overall near-parallel pattern. As the water from the emptying tunnel joins the main flow, it impacts the sediment discharge tunnel, causing a contraction of the water flow on the right side of the confluence area and a slight increase in flow velocity. Meanwhile, the flow velocity on the left side of the confluence area decreases slightly. Overall, the near-bottom velocity distribution remains relatively uniform. The Froude number (Fr) in the simulated computational area ranges from 2.219 to 4.569 under design conditions and from 2.409 to 4.194 under verification conditions. The flows in the confluence area under both conditions are supercritical, with no backflow observed.

4.2.3. Bottom Pressure

Figure 14 illustrates the pressure distribution on the bottom plate of the confluence area under both design and verification conditions. The highest pressure is observed near the intersection pier, reaching 66 kPa under design conditions and 71.6 kPa under verification conditions. Small negative pressure zones appear on both sides of the intersection pier, highlighting the importance of strict surface flatness control during construction and enhanced monitoring during operation. Beyond the intersection pier, the pressure distribution on the bottom plate in the confluence and downstream regions becomes relatively uniform, with an average pressure of 17 kPa under design conditions and 25 kPa under verification conditions.

5. Discussion

The experimental results indicate that the variation in flow velocity uniformity at the confluence is closely related to the confluence ratio and the intersection angle. An increase in the confluence ratio (tributary flow rate/mainstream flow rate) significantly enhances the non-uniformity of flow velocity. A similar positive trend is observed with changes in the intersection angle. In numerical model experiments, when the intersection angle was reduced to 21°, the results showed that the water surface level in the confluence area was below the straight wall, leading to its acceptance as the optimized scheme. The feasibility of this approach was further confirmed by physical model tests.

Additionally, the analysis of the experimental results also revealed that high-drop non-pressurized tunnel intersections exhibit significant three-dimensional flow structures. High-velocity confluence flows demonstrate distinct jet-deflection characteristics. After the tributary flows imports into the mainstream, the flow’s longitudinal velocity and pressure distributions exhibit complex patterns of mutual squeezing, upward impact, and helical deflection. This finding provides a better explanation for the challenges in designing the geometry of high-drop non-pressurized tunnel intersections.

The broader implications of this study extend beyond pumped storage power stations. The methodologies and insights developed here are applicable to similar hydraulic systems, such as urban drainage networks and flood discharge structures, where efficient confluence flow management is essential. By systematically analyzing flow patterns, velocity distributions, and structural pressures, this study lays a strong foundation for improving the design and operation of high-performance hydraulic systems under challenging conditions. The results also provide valuable insights into high-drop, non-pressurized tunnel intersections and their optimized design, offering practical solutions for future engineering applications. These findings emphasize the need for a multidisciplinary approach, combining advanced numerical modeling, experimental research, and field validation to address the inherent complexities of hydraulic engineering and achieve more resilient infrastructure solutions.

6. Conclusions

The hydraulic model test found that the water surface elevations in the originally planned intersection area of the sediment discharge and emptying tunnels exceeded the height of the vertical walls. After conducting a series of tests, the optimal intersection shape was identified, which featured an arc connection with a 21° intersection angle. A 3D numerical model of the confluence zone for this optimized shape was then developed to analyze flow patterns, velocity distribution, and bottom pressure under both the originally designed and the optimized conditions. The following conclusions were drawn:

Reducing the intersection angle and increasing the circular connection radius effectively decreases the impact between the two flows in the intersection area, thereby reducing turbulence and mixing intensity. The confluence flow observed in this study was characterized by intense mixing and significant water surface oscillations. Due to the high elevation drop and large flow rates, supercritical flow dominated the confluence area, with no backflows detected.

The optimized intersection shape (c) successfully addressed the issue of the water depth exceeding the 6 m wall height. Under both design and verification conditions, the maximum water depth along the right straight wall remained below 6 m, meeting the requirements outlined in the Code for Hydraulic Tunnel Design. This confirms the validity of the optimized intersection design, which features a 21° intersection angle and arc connections.

In all three optimized shapes, the confluence area of the high-elevation-drop non-pressurized tunnels exhibited a distinct flow structure as follows: a high-velocity zone on the right, a low-velocity zone on the left, a flow recovery zone downstream of the confluence, and the formation of water wings. No backflows were observed in any of the designs.

Despite these findings, research on high-speed flow structures in the intersection area remains limited. The lack of extensive field prototype observations further complicates the understanding of these complex flow dynamics, involving turbulence, vortex formation, and pressure fluctuations, all of which can significantly impact the tunnel’s structural integrity and operational efficiency. Additionally, the high velocities can lead to increased erosion and wear on tunnel surfaces, highlighting the need for more detailed studies to ensure the long-term stability and safety of these critical infrastructures. Future research should focus on further investigating 3D flow structures, the key factors influencing hydraulic characteristics, and their quantifiable relationships to enhance understanding and improve design practices.